Numerical Investigation of Signal Launch Imperfections for Edge Mount RF Connectors
Abstract
:1. Introduction
2. PCB Test Boards for Signal Launching Investigations
3. 3D Numerical Models of Test PCBs
4. Results and Discussion
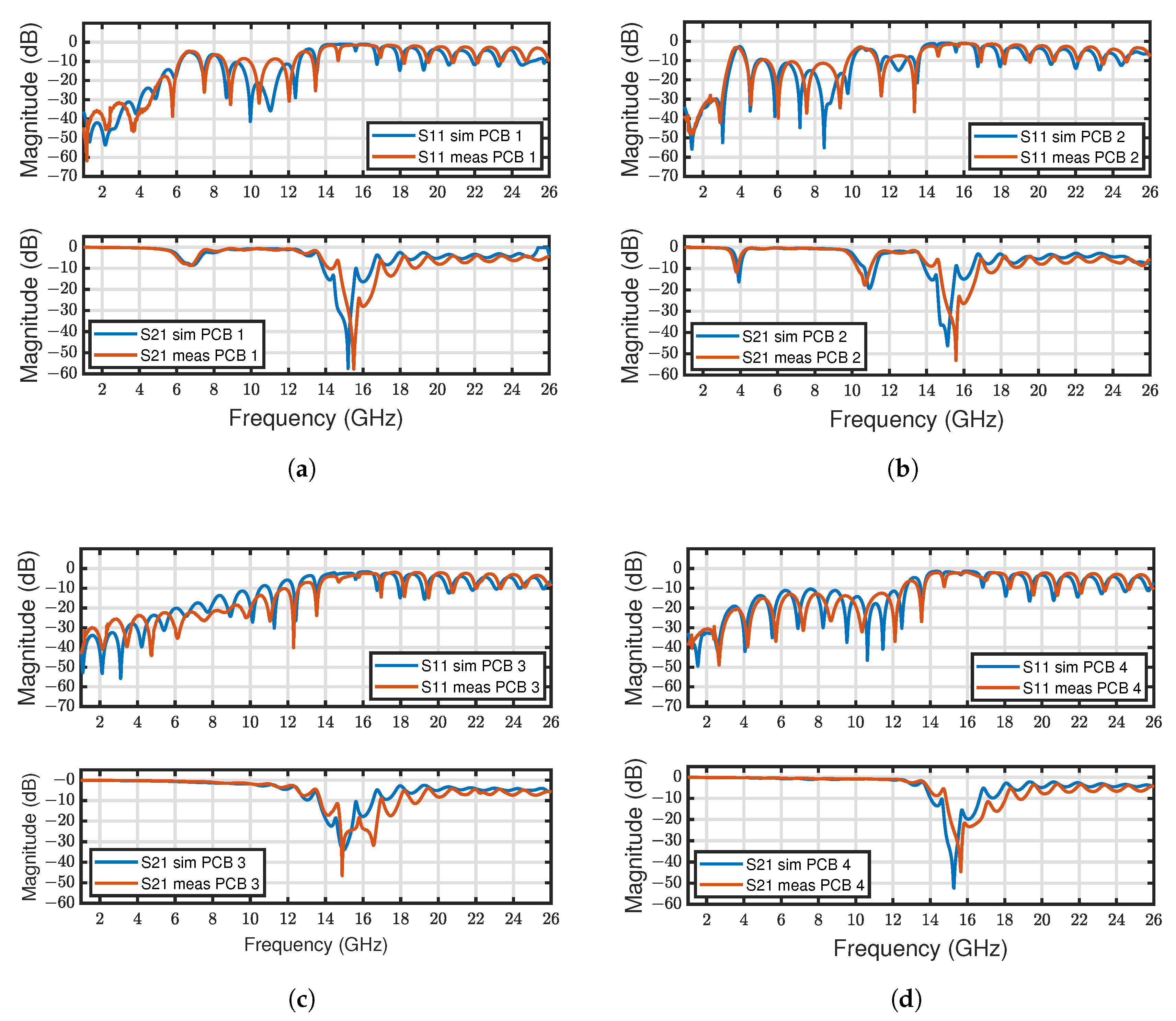
4.1. VNA Measurement
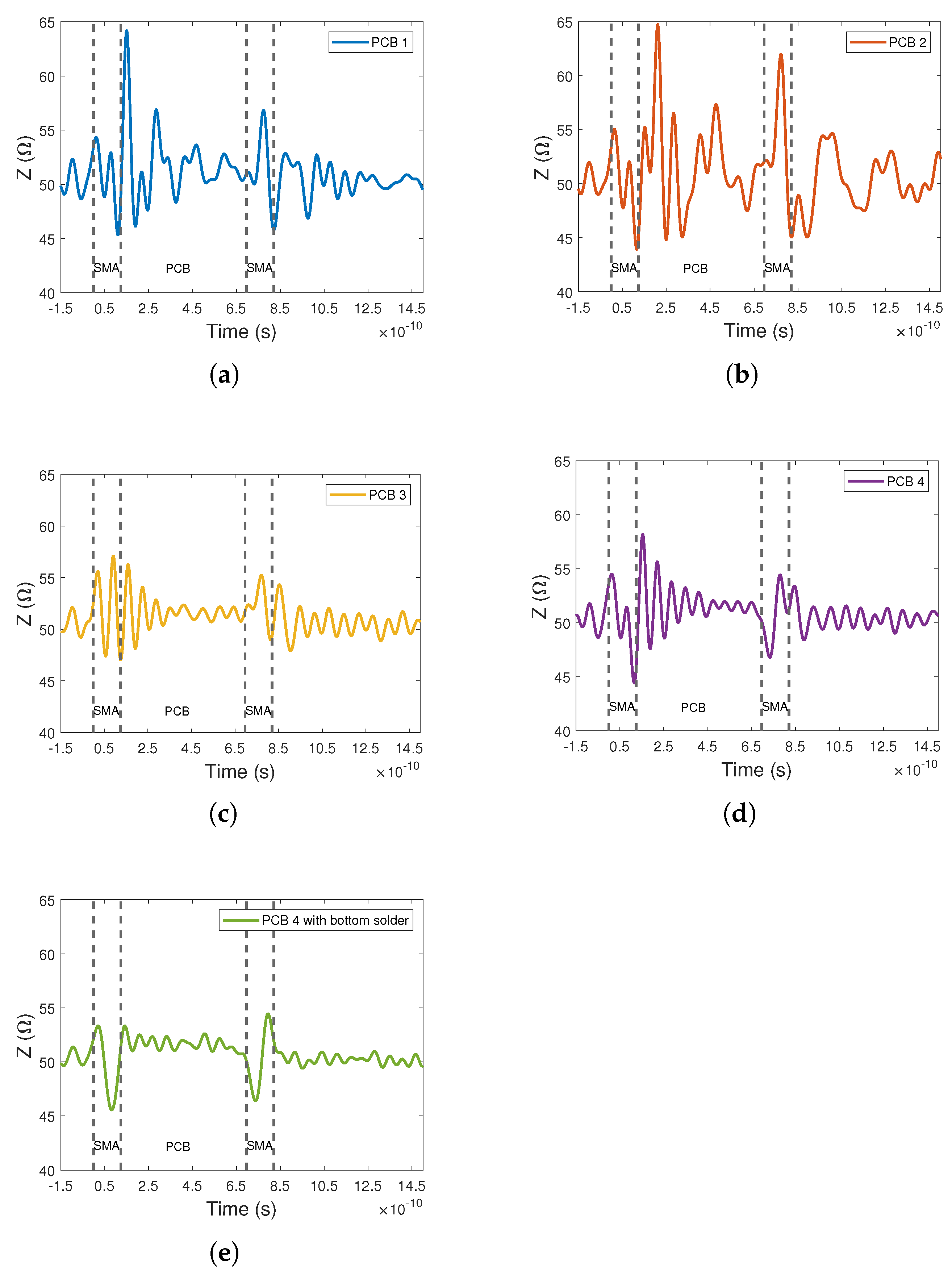
4.2. TDR Measurement
4.3. Measurement Summary
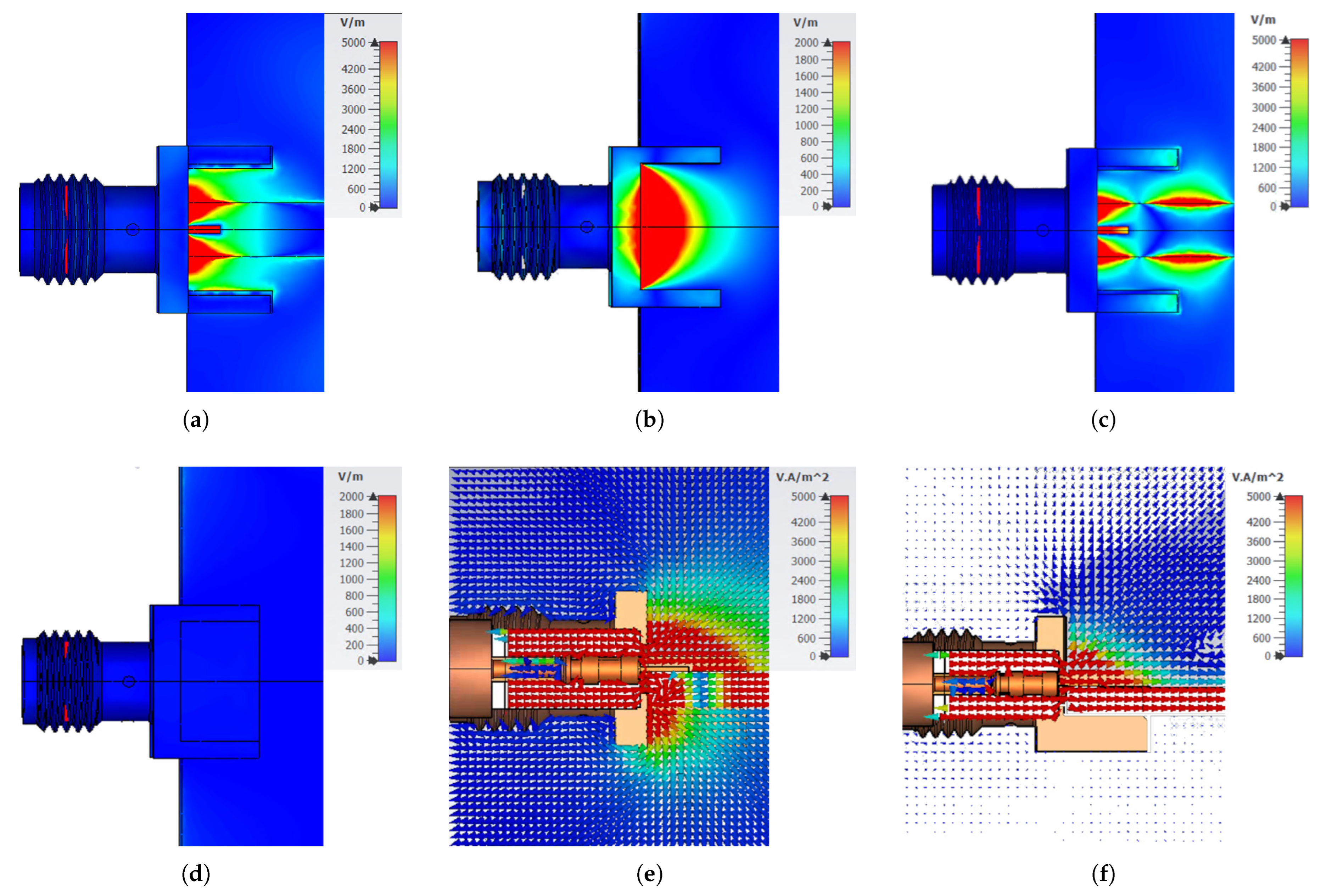
4.4. Simulation and EM Field Analysis
4.5. EM Field Analysis Summary
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Wadell, B.C. Transmission Line Design Handbook, 1st ed.; Artech House, Inc.: Norwood, MA, USA, 1991; pp. 479–481. [Google Scholar]
- Shoaib, N. Vector Network Analyzer (VNA) Measurements and Uncertainty Assessment, 1st ed.; Springer: Cham, Switzerland, 2017; pp. 1–55. [Google Scholar]
- WR-SMA Round Post for PCB 1.6 mm. Available online: https://www.we-online.com/katalog/de/ROUND_POST_FOR_PCB_1_6_M_M (accessed on 19 January 2022).
- Stavoli, R.; Correia, D.; Soubh, E. Demystifying Edge Launch Connectors. Signal Integr. J. 2019. Available online: https://www.signalintegrityjournal.com/articles/1094-demystifying-edge-launch-connectors (accessed on 19 January 2022).
- Almuqati, N.; Sigmarsson, H. 3D Microstrip Line Taper on Ultra-low Dielectric Constant Substrate. In Proceedings of the 2019 IEEE 20th Wireless and Microwave Technology Conference (WAMICON), Cocoa Beach, FL, USA, 8–9 April 2019. [Google Scholar]
- Gupta, S.; Sebak, A.R.; Devabhaktuni, K.D. Optimum Launch-Taper Matching Technique for mm-Wave Applications. In Proceedings of the 2017 IEEE MTT-S International Microwave and RF Conference (IMaRC), Ahmedabad, India, 11–13 December 2017. [Google Scholar]
- Drak, O.T.; Liubina, M.L. Coaxial to Microstrip Transition Matching Method. In Proceedings of the 2021 IEEE Conference of Russian Young Researchers in Electrical and Electronic Engineering (ElConRus), St. Petersburg, Russia, 26–29 January 2021. [Google Scholar]
- Liang, H.; Laskar, J.; Barnes, H.; Estreich, D. Design and optimization for coaxial-to-microstrip transition on multilayer substrates. In Proceedings of the 2001 IEEE MTT-S International Microwave Sympsoium Digest (Cat. No.01CH37157), Phoenix, AZ, USA, 20–24 May 2001. [Google Scholar]
- Optimizing Test Boards for 50 GHz End Launch Connectors. Available online: https://mpd.southwestmicrowave.com/wp-content/uploads/2018/07/Optimizing-Test-Boards-for-50-GHz-End-Launch-Connectors.pdf (accessed on 19 January 2022).
- The Design and Test of Broadband Launches up to 50 GHz on Thin and Thick Substrates. Available online: https://www.hasco-inc.com/content/Technical_Articles/Design_and_Test_Broadband_Launches.pdf (accessed on 19 January 2022).
- Coonrod, J. Signal Launch Methods for RF/Microwave PCBs. Available online: https://www.signalintegrityjournal.com/articles/152-signal-launch-methods-for-rfmicrowave-pcbs (accessed on 19 January 2022).
- Ellison, J.; Smith, S.B.; Agili, S. Using a 2x-thru standard to achieve accurate de-embedding of measurements. Microw. Opt. Technol. Lett. 2020, 62, 675–682. [Google Scholar] [CrossRef]
- Stepins, D.; Asmanis, G.; Asmanis, A. Measuring Capacitor Parameters Using Vector Network Analyzers. Electronics 2014, 18, 29–38. [Google Scholar] [CrossRef] [Green Version]
- Coaxial PCB Connector PCB-Transmission Line Design Guide. Available online: https://www.we-online.com/catalog/media/o563287v410%20ANE012a%20EN.pdf (accessed on 11 March 2022).
- CST Studio Suite—Electromagnetic Field Simulation Software. Available online: https://www.3ds.com/products-services/simulia/products/cst-studio-suite/?utm_source=cst.com&utm_medium=301&utm_campaign=cst (accessed on 3 June 2022).
- RO4000 Laminates RO4003C and RO4350B—Data Sheet. Available online: https://rogerscorp.com/-/media/project/rogerscorp/documents/advanced-electronics-solutions/english/data-sheets/ro4000-laminates-ro4003c-and-ro4350b—data-sheet.pdf (accessed on 24 January 2022).
- Lischka, G. Design and Realization of Microstrip—Transitions up to 90 GHz. Master’s Thesis, Technical University of Vienna, Vienna, Austria, 2005. [Google Scholar]
- Paul, C.R. Introduction to Electromagnetic Compatibility (Wiley Series in Microwave and Optical Engineering, 2nd ed.; Wiley-Interscience: Hoboken, NJ, USA, 2006; pp. 199–204. [Google Scholar]
- Li, Y.; Zhou, J.; Shen, J.; Li, Q.; Qi, Y.; Chen, W.A. Ultra-low permittivity HSM/PTFE composites for high-frequency microwave circuit application. J. Mater. Sci. Mater. Electron. 2022, 33, 10096–10103. [Google Scholar] [CrossRef]
- Paul, C.R.; Nasar, S.A. Introduction to Electromagnetic Fields, 2nd ed.; Wiley-Interscience: Hoboken, NJ, USA, 1987; pp. 251–254. [Google Scholar]

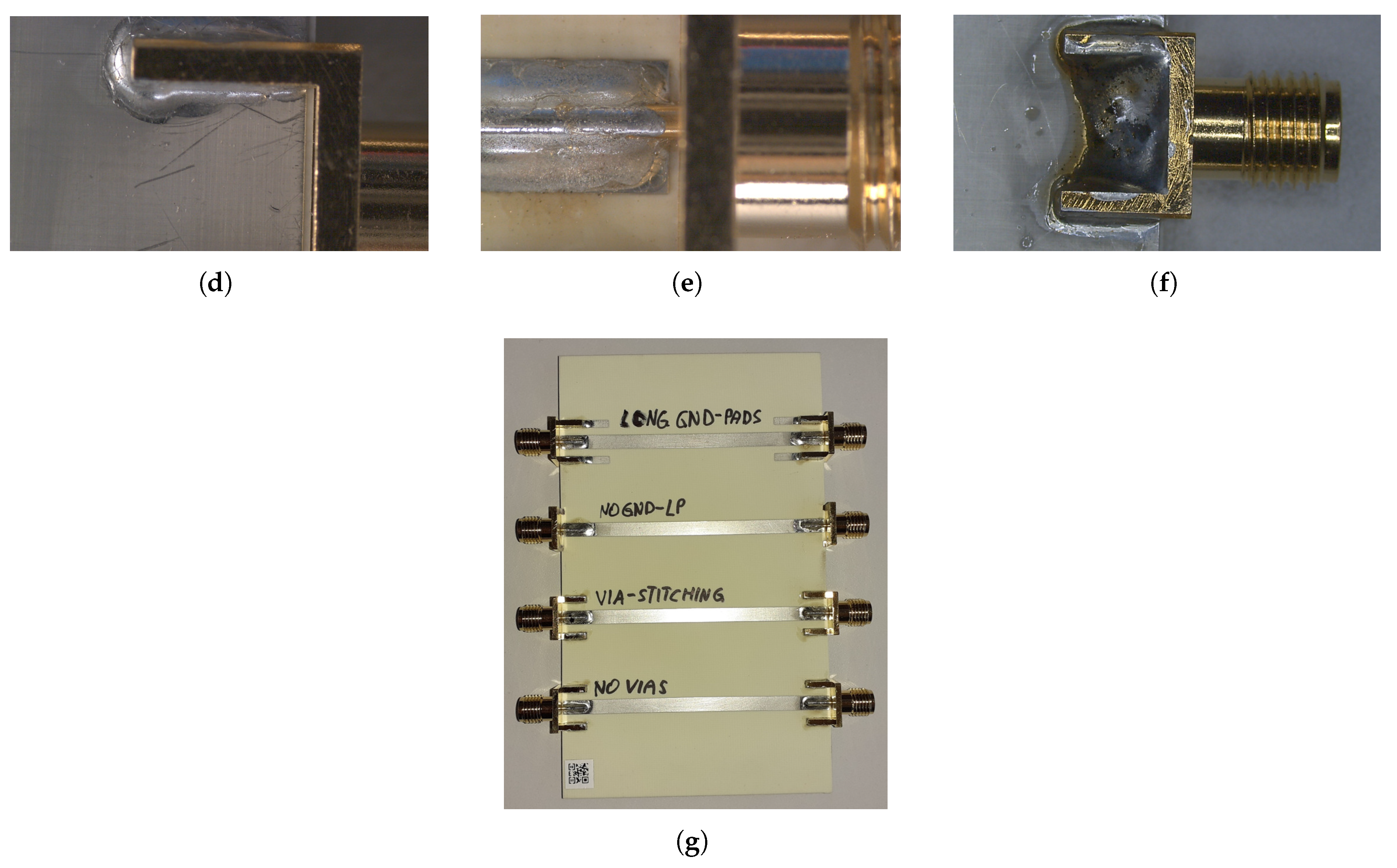

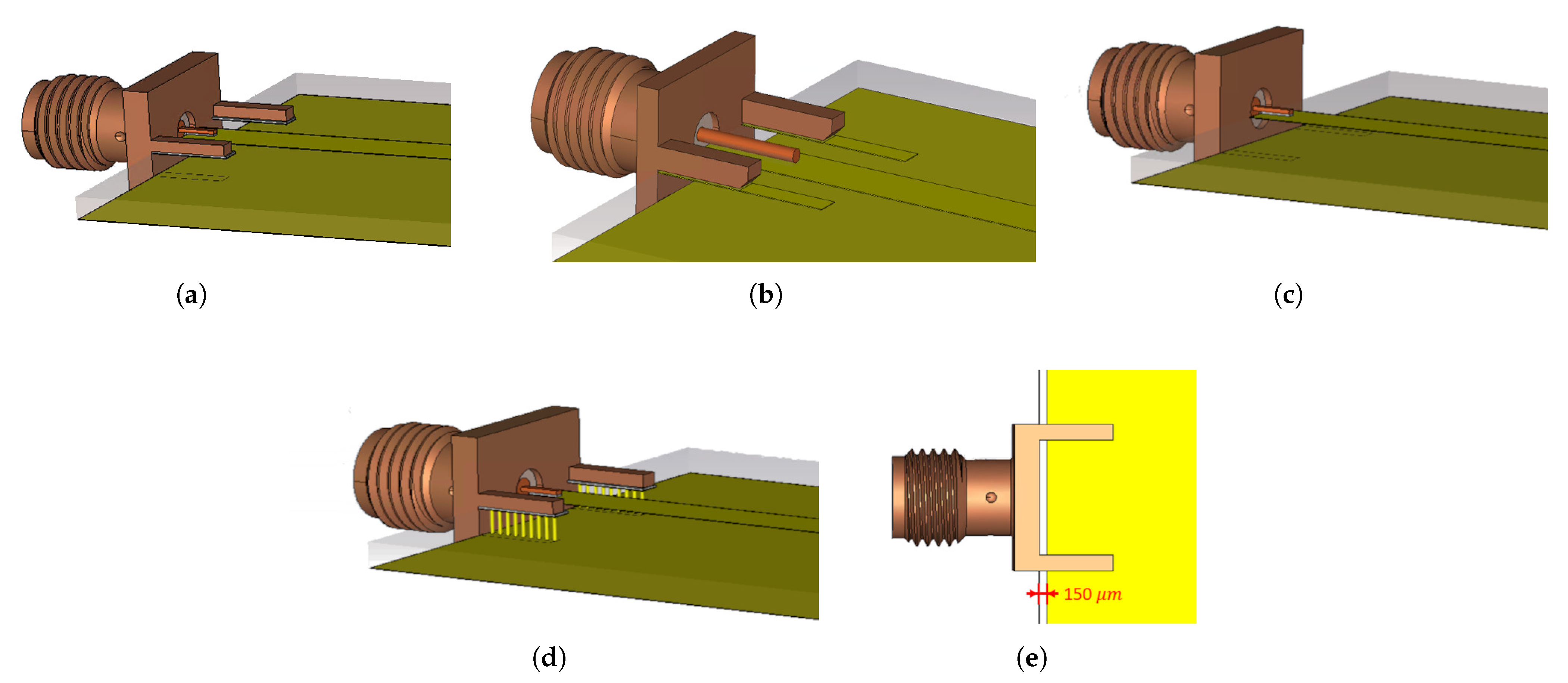


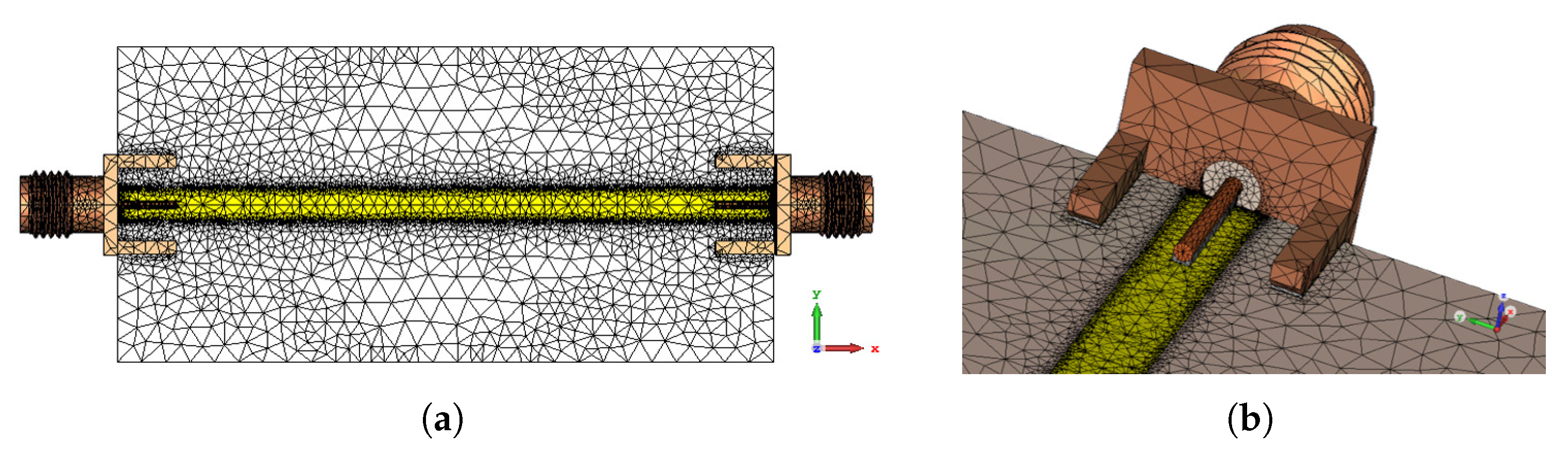


| Substrate | RO4350B ( ) [16] |
|---|---|
| L × H | 60 × 1.524 mm |
| Copper thickness | 44 m |
| Trace width | 3.1 mm |
| Signal launch (A × B) | |
| PCB 1 | 5.5 × 1.65 mm |
| PCB 2 | 11 × 1.65 mm |
| PCB 3 | 0 × 0 mm |
| PCB 4 | 5.5 × 1.65 mm |
| Body material | Brass with |
| Center contact material | Beryllium-Copper with |
| Isolation material | PTFE with = 1.55 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Riener, C.; Bauernfeind, T.; Kvasnicka, S.; Roppert, K.; Hackl, H.; Kaltenbacher, M. Numerical Investigation of Signal Launch Imperfections for Edge Mount RF Connectors. Electronics 2022, 11, 1990. https://doi.org/10.3390/electronics11131990
Riener C, Bauernfeind T, Kvasnicka S, Roppert K, Hackl H, Kaltenbacher M. Numerical Investigation of Signal Launch Imperfections for Edge Mount RF Connectors. Electronics. 2022; 11(13):1990. https://doi.org/10.3390/electronics11131990
Chicago/Turabian StyleRiener, Christian, Thomas Bauernfeind, Samuel Kvasnicka, Klaus Roppert, Herbert Hackl, and Manfred Kaltenbacher. 2022. "Numerical Investigation of Signal Launch Imperfections for Edge Mount RF Connectors" Electronics 11, no. 13: 1990. https://doi.org/10.3390/electronics11131990
APA StyleRiener, C., Bauernfeind, T., Kvasnicka, S., Roppert, K., Hackl, H., & Kaltenbacher, M. (2022). Numerical Investigation of Signal Launch Imperfections for Edge Mount RF Connectors. Electronics, 11(13), 1990. https://doi.org/10.3390/electronics11131990






