Investigation of the Optimum Diameter of the Ring Reflector for an Axial Virtual Cathode Oscillator
Abstract
:1. Introduction
2. System Description
2.1. Pulsed-Power Ssystem (10-Stage PFN-Marx Generator)
2.2. A High-Power Microwave Device (Axial Vircator)
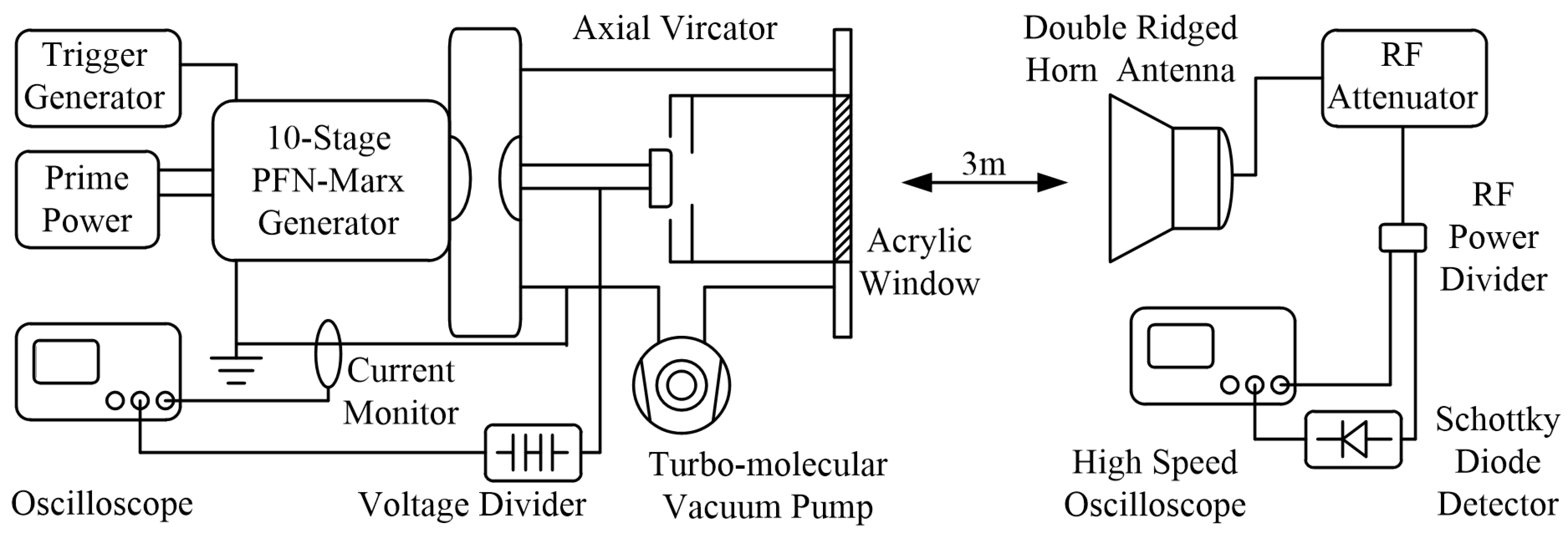
2.3. Measurement System
3. PIC Simulation and the Experimental Results
3.1. PIC Simulation
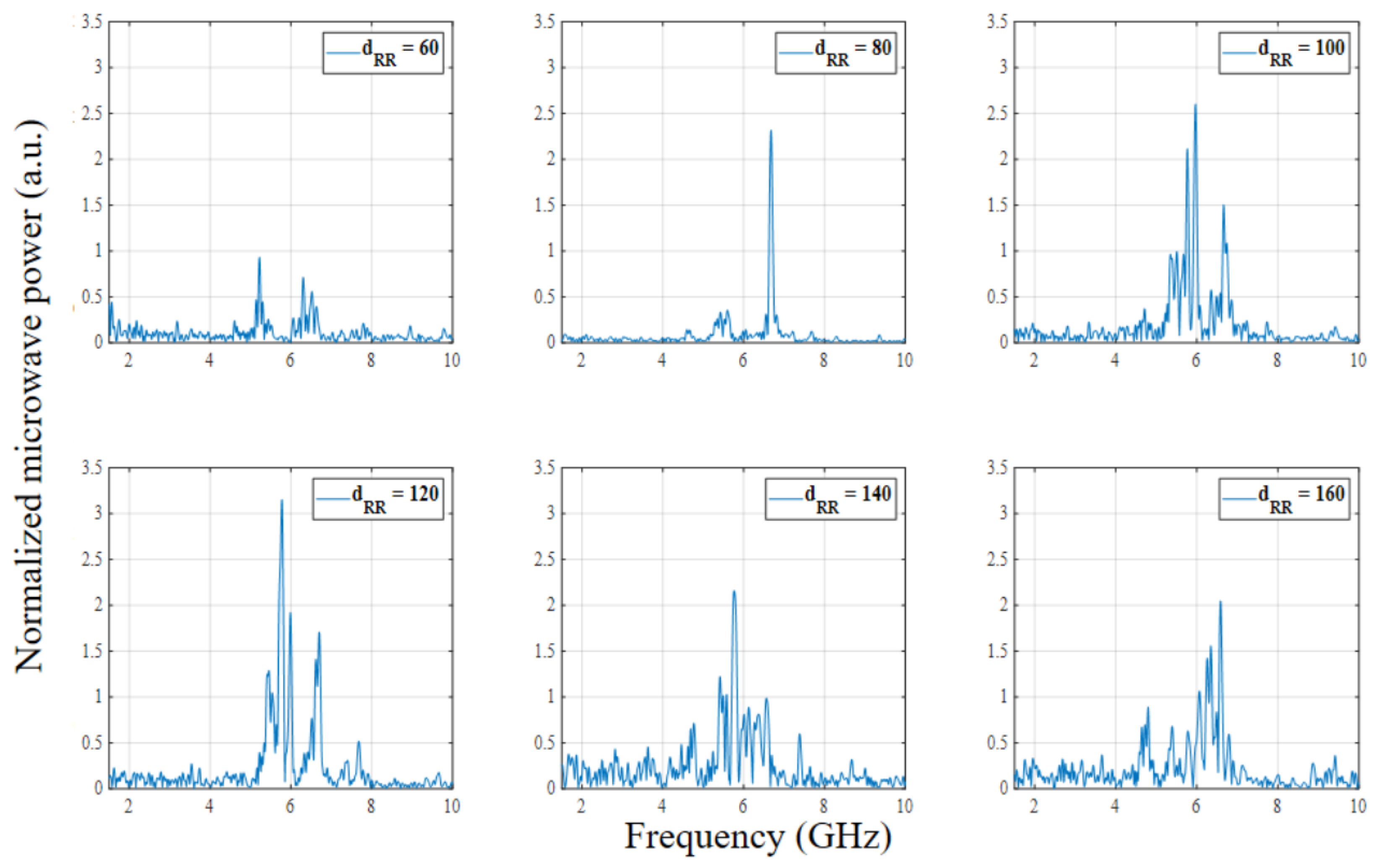
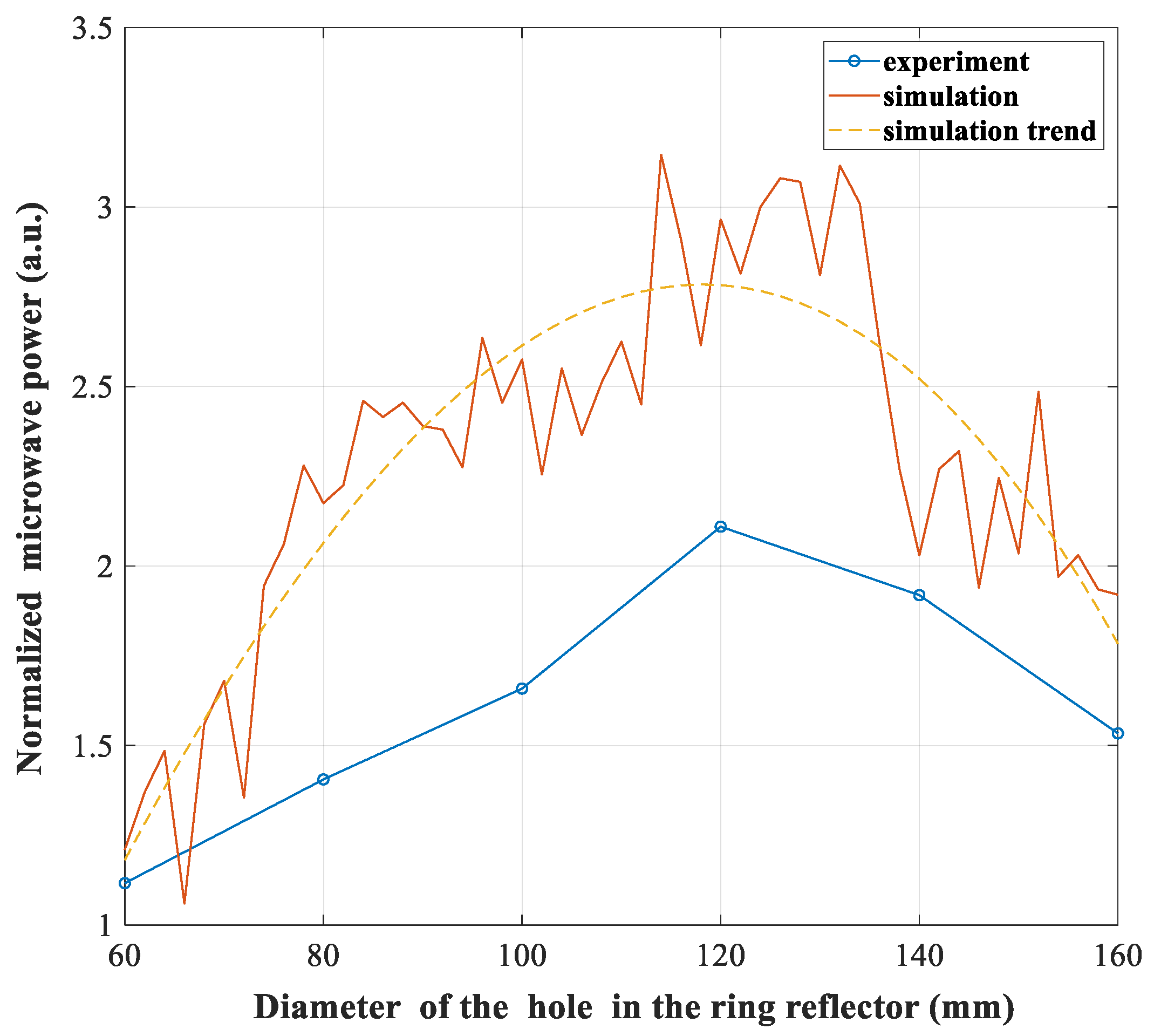
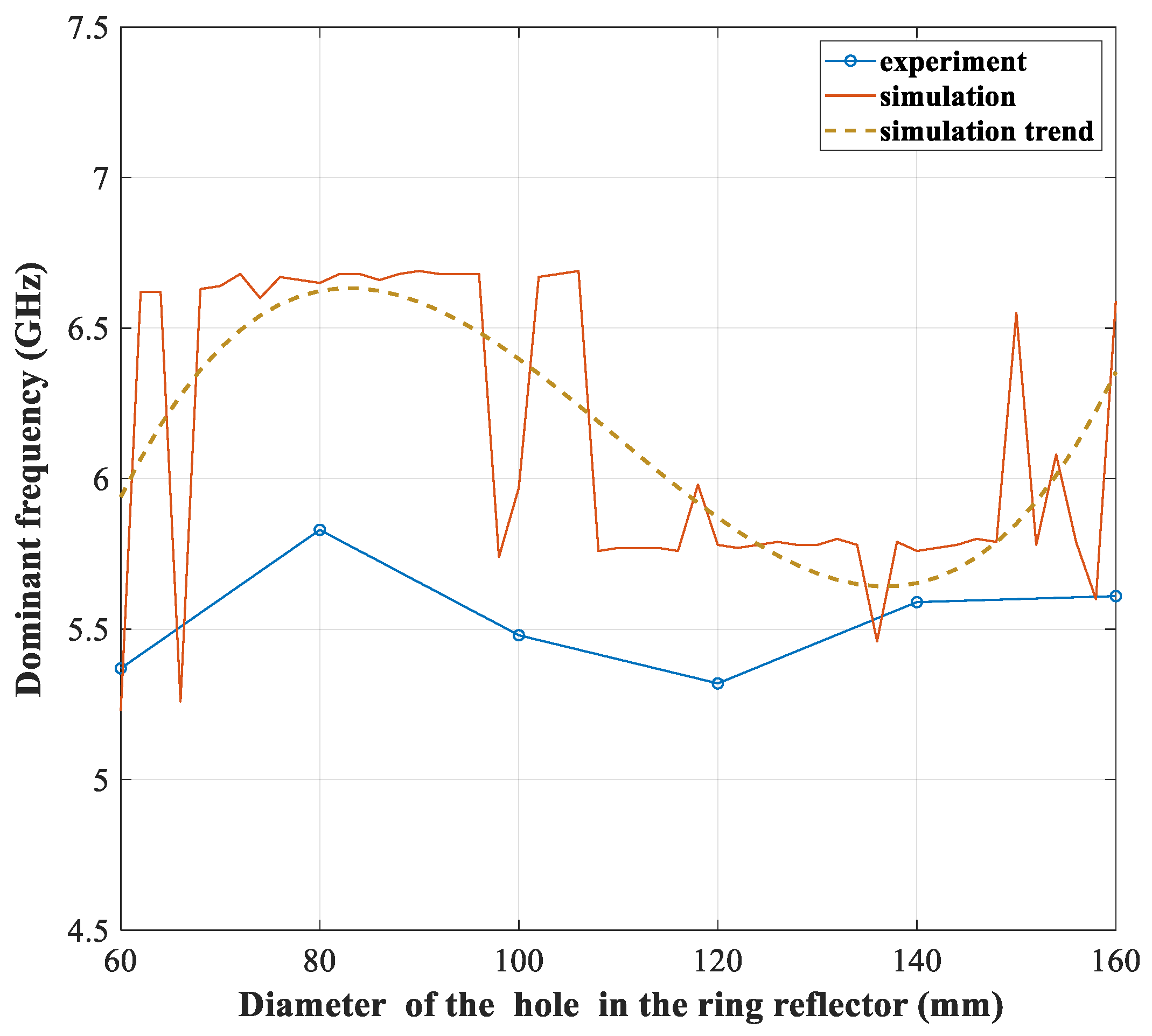
3.2. Experimental Results
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Feng, J.; Li, X.; Hu, J.; Cai, J. General Vacuum Electronics. J. Electromagn. Eng. Sci. 2020, 20, 1–8. [Google Scholar] [CrossRef]
- Barker, R.J.; Schamiloglu, E. High-Power Microwave Sources and Technologies; Wiley-IEEE Press: Hoboken, NJ, USA, 2001. [Google Scholar]
- Benford, J.; Swegle, J.A.; Schamiloglu, E. High Power Microwaves, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- Liu, Z.; Huang, H.; Jin, X.; Lei, L. Design of an X-Band Gigawatt Multibeam Relativistic Klystron Amplifier. IEEE Trans. Plasma Sci. 2014, 42, 3419–3422. [Google Scholar] [CrossRef]
- Jang, G.H.; Han, S.M.; Won, J.H.; Kim, J.H. HPM Technology Trend. JKIEES 2013, 24, 37–42. [Google Scholar]
- Schamiloglu, E. High Power Microwave Sources and Applications. IEEE MTT-S. 2004, 2, 1001–1004. [Google Scholar]
- Sedek, E.; Slomski, R. Overview of Microwave Direct Energy Weapons. In Proceedings of the 2015 Signal Processing Symposium (SPSympo), Debe, Poland, 10–12 June 2015; pp. 1–3. [Google Scholar]
- Giri, D.V.; Tesche, F.M. Classification of Intentional Electromagnetic Environments (IEME). IEEE Trans. Electromagn. Compat. 2004, 46, 322–328. [Google Scholar] [CrossRef]
- Farmer, W.A.; Cohen, B.I.; Eng, C.D. On the Validity of Certain Approximations Used in the Modeling of Nuclear EMP. IEEE Trans. Nucl. Sci. 2016, 63, 1259–1267. [Google Scholar] [CrossRef]
- Hao, R.; Zhang, X.; Gao, H.; Wu, H.; Cheng, J. A novel high-altitude electromagnetic pulse (HEMP) protection circuit for RF applications. Microelectron. Eng. 2019, 84, 1–8. [Google Scholar] [CrossRef]
- Kim, S.H.; Lee, C.J.; Kim, W.I.; Ko, K.C. Experimental Investigation into the Optimum Position of a Ring Reflector for an Axial Virtual Cathode Oscillator. Electronics 2021, 10, 1878. [Google Scholar] [CrossRef]
- Chen, Y.; Mankowski, J.; Walter, J.; Kristiansen, M. Cathode and Anode Optimization in a Virtual Cathode Oscillator. IEEE Trans. Dielectr. Electr. Insul. 2007, 14, 1037–1044. [Google Scholar] [CrossRef]
- Li, L.; Men, T.; Liu, L. Dynamics of virtual cathode oscillation analyzed by impedance changes in high-power diodes. Int. J. Appl. Phys. 2007, 102, 123309. [Google Scholar] [CrossRef]
- Walter, J.W.; Lynn, C.F.; Dickens, J.C.; Kristiansen, M. Operation of a Sealed-Tube-Vircator High-Power-Microwave Source. IEEE Trans. Plasma Sci. 2012, 40, 1618–1621. [Google Scholar] [CrossRef]
- Mumtaz, S.; Lim, J.S.; Ghimire, B. Enhancing the power of high power microwaves by using zone plate and investigations for the position of virtual cathode inside the drift tube. J. Plasma Phys. 2018, 25, 103113. [Google Scholar] [CrossRef]
- Parson, J.M.; Lynn, C.F.; Scott, M.C.; Calico, S.E.; Dickens, J.C.; Neuber, A.A.; Mankowski, J.J. A Frequency Stable Vacuum-Sealed Tube High-Power Microwave Vircator Operated at 500 Hz. IEEE Electron Device Lett. 2015, 36, 508–510. [Google Scholar] [CrossRef]
- Andersson, J.; Jansson, M.; Aberg, D. Frequency Dependence of the Anod-Cathode Gap Spacing in a Coaxial Vircator System. IEEE Trans. Plasma Sci. 2013, 41, 2758–2762. [Google Scholar] [CrossRef]
- Jiang, W. Time-Frequency Analysis of Virtual-Cathode Oscillator. IEEE Trans. Plasma Sci. 2010, 38, 1325–1328. [Google Scholar] [CrossRef]
- Cassany, B.; Desanlis, T.; Galtie, A.; Gardelle, J.; Modin, P.; Voisin, L. High current fast Marx generator and vircator experiments. In Proceedings of the Conference Record of the Twenty-Sixth International Power Modulator Symposium, 2004 and 2004 High-Voltage Workshop, San Francisco, CA, USA, 23–26 May 2004; pp. 441–444. [Google Scholar]
- Rainwater, K.; Darunett, D.; Lynn, C.; Dickens, J.; Neuber, A.; Mankowski, J. A 160 J, 100 Hz rep rate, compact Marx generator for driving and HPM source. In Proceedings of the 2016 IEEE International Power Modulator and High Voltage Conference (IPMHVC), San Francisco, CA, USA, 6–9 July 2016; pp. 228–230. [Google Scholar]
- Barnett, D.H.; Neuber, A.A.; Dickens, J.C.; John, J.; Mankowski, J. CST Particle-In-Cell Modeling of A Tunable Reflex-Triode Vircator. In Proceedings of the 2018 IEEE International Power Modulator and High Voltage Conference (IPMHVC), Jackson, WY, USA, 3–7 June 2018; pp. 133–135. [Google Scholar]
- Elfsberg, M.; Hurtig, T.; Larsson, A.; Moller, C.; Nyholm, E. Experimental Studies of Anode and Cathode Materials in a Repetitive Driven Axial Vircator. IEEE Trans. Plasma Sci. 2008, 36, 688–693. [Google Scholar] [CrossRef]
- Jeon, W.; Sung, K.Y.; Lim, J.E.; Song, K.B.; Seo, Y.; Choi, E.H. A Diode Design Study of the Virtual Cathode Oscillator with a Ring-Type Reflector. IEEE Trans. Plasma Sci. 2005, 33, 2011–2016. [Google Scholar] [CrossRef]
- Champeaux, S.; Gouard, P.; Cousin, R.; Larour, J. Improved Design of a Multistage Axial Vircator with Reflectors for Enhanced Performances. IEEE Trans. Plasma Sci. 2016, 44, 31–38. [Google Scholar] [CrossRef]
- Jeon, W.; Lim, J.E.; Moon, M.W.; Jung, K.B.; Park, W.B.; Shin, H.M.; Seo, Y.; Choi, E.H. Output characteristics of the high-power microwave generated from a coaxial vircator with a bar reflector in a drift region. IEEE Trans. Plasma Sci. 2006, 34, 937–944. [Google Scholar] [CrossRef]
- Champeaux, S.; Gouard, P.; Cousin, R.; Larour, J. Numerical evaluation of the role of reflectors to maximize the power efficiency of an axial Vircator. In Proceedings of the 2013 IEEE 14th International Vacuum Electronics Conference (IVEC), Paris, France, 21–23 May 2013; pp. 1–2. [Google Scholar]
- Moller, C.; Hurtig, T.; Larsson, A.; Nyholm, E. Numerical simulations of the influence of a reflector in a coaxial vircator. In Proceedings of the 2019 IEEE Pulsed Power Conference, Washington, DC, USA, 28 June–2 July 2009; pp. 529–532. [Google Scholar]
- Zhang, H.; Yang, J.; Lin, J.; Yang, X. A compact bipolar pulse-forming network-Marx generator based on pulse transformers. AIP 2013, 84, 114705. [Google Scholar] [CrossRef]
- Barnentt, D.H.; Rainwater, K.; Dickens, J.C.; Neuber, A.A.; Mankowski, J.J. A Reflex Triode System with Multicavity Adjustment. IEEE Trans. Plasma Sci. 2019, 47, 1472–1476. [Google Scholar] [CrossRef]
- Sullivan, D.J. High Power Microwave Generation from a Virtual Cathode Osillator (Vircator). IEEE Tans Nuclear Sci. 1983, 30, 3426–3428. [Google Scholar] [CrossRef]
- Adhikary, B.; Shyam, A.; Shukla, R.; Sharma, S.K. Fast Marx Generator for Directly Driving a Virtual Cathode Oscillator. J Korean Phys Soc. 2011, 59, 3476–3480. [Google Scholar] [CrossRef]
- Lassalle, F.; Morell, A.; Loyen, A.; Chanconie, T.; Roques, B.; Toury, M.; Vezinet, R. Development and Test of a 400-kV PFN Marx with Compactness and Rise Time Optimization. IEEE Trans. Plasma Sci. 2018, 46, 3313–3319. [Google Scholar] [CrossRef]





| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Capacitance | 4.17 nF | Inductance | 37.5 nH |
| PFN stage | 6 | Marx stage | 10 |
| Charging voltage | −30 kV | Erected voltage | −300 kV |
| Pulse width | 150 ns | Characteristic impedance | 30 Ω |
| Quantity | Value | Quantity | Value |
|---|---|---|---|
| Diameter | 200 mm | Electron emitting electric field | 100 kV/m |
| Length | 300 mm | Transparency of the mesh anode | 70% |
| Cathode surface | 200 mm | Pulse width | 25 ns |
| Anode surface | 26 mm | Flat top voltage | 150 kV |
| dRR | Pmin (MW) | Pmax (MW) | Pavg (MW) | Frequency (GHz) |
|---|---|---|---|---|
| Basic axial | 8.7 | 12.3 | 11.22 | 5.54 |
| 60 mm | 6.09 | 14.93 | 12.53 | 5.37 |
| 80 mm | 12.21 | 17.37 | 15.77 | 5.83 |
| 100 mm | 12.93 | 22.68 | 18.61 | 5.48 |
| 120 mm | 15.7 | 27.03 | 23.67 | 5.32 |
| 140 mm | 19.36 | 24.43 | 21.53 | 5.59 |
| 160 mm | 15.44 | 21.3 | 17.21 | 5.61 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, W.-I.; Kim, S.-H.; Ko, K.-C. Investigation of the Optimum Diameter of the Ring Reflector for an Axial Virtual Cathode Oscillator. Electronics 2022, 11, 2002. https://doi.org/10.3390/electronics11132002
Kim W-I, Kim S-H, Ko K-C. Investigation of the Optimum Diameter of the Ring Reflector for an Axial Virtual Cathode Oscillator. Electronics. 2022; 11(13):2002. https://doi.org/10.3390/electronics11132002
Chicago/Turabian StyleKim, Wan-Il, Se-Hoon Kim, and Kwang-Cheol Ko. 2022. "Investigation of the Optimum Diameter of the Ring Reflector for an Axial Virtual Cathode Oscillator" Electronics 11, no. 13: 2002. https://doi.org/10.3390/electronics11132002
APA StyleKim, W. -I., Kim, S. -H., & Ko, K. -C. (2022). Investigation of the Optimum Diameter of the Ring Reflector for an Axial Virtual Cathode Oscillator. Electronics, 11(13), 2002. https://doi.org/10.3390/electronics11132002







