Design and Analysis of Magnetic Shielding Mechanism for Wireless Power Transfer System Based on Composite Materials
Abstract
:1. Introduction
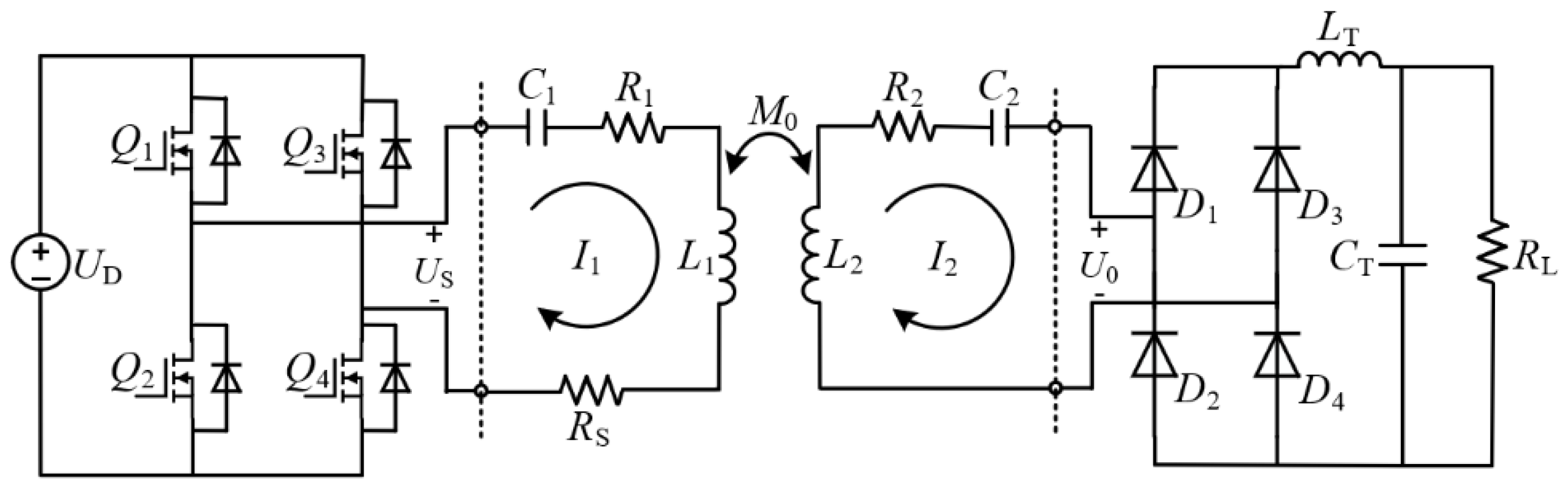
2. Theoretical Analysis of WPT System
3. Design and Research of Magnetic Shielding Mechanism
3.1. Defects in Ferrite
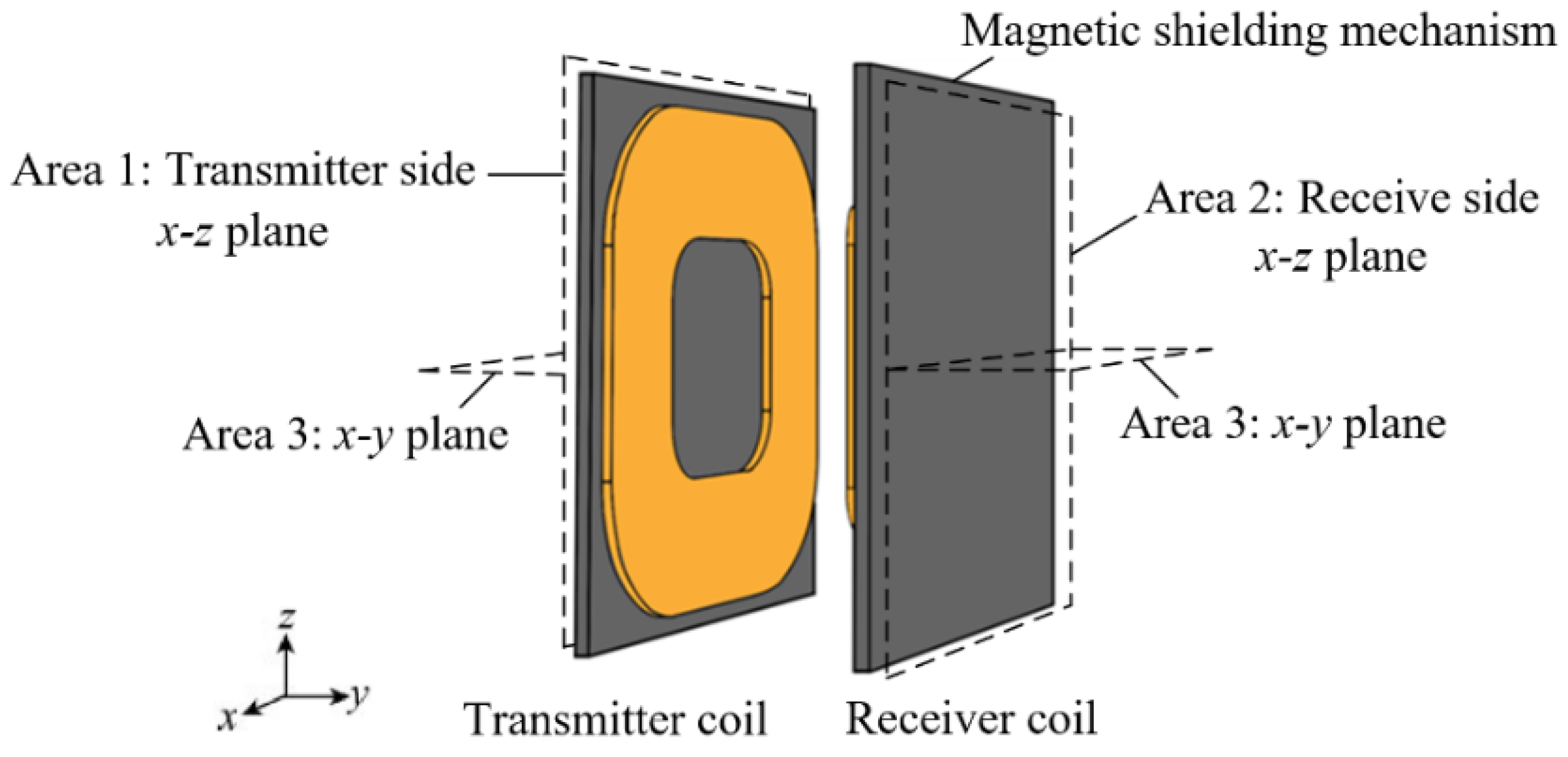
3.2. Research on New Magnetic Shielding Mechanism
4. Simulation Analysis
4.1. Coupling Mechanism Model
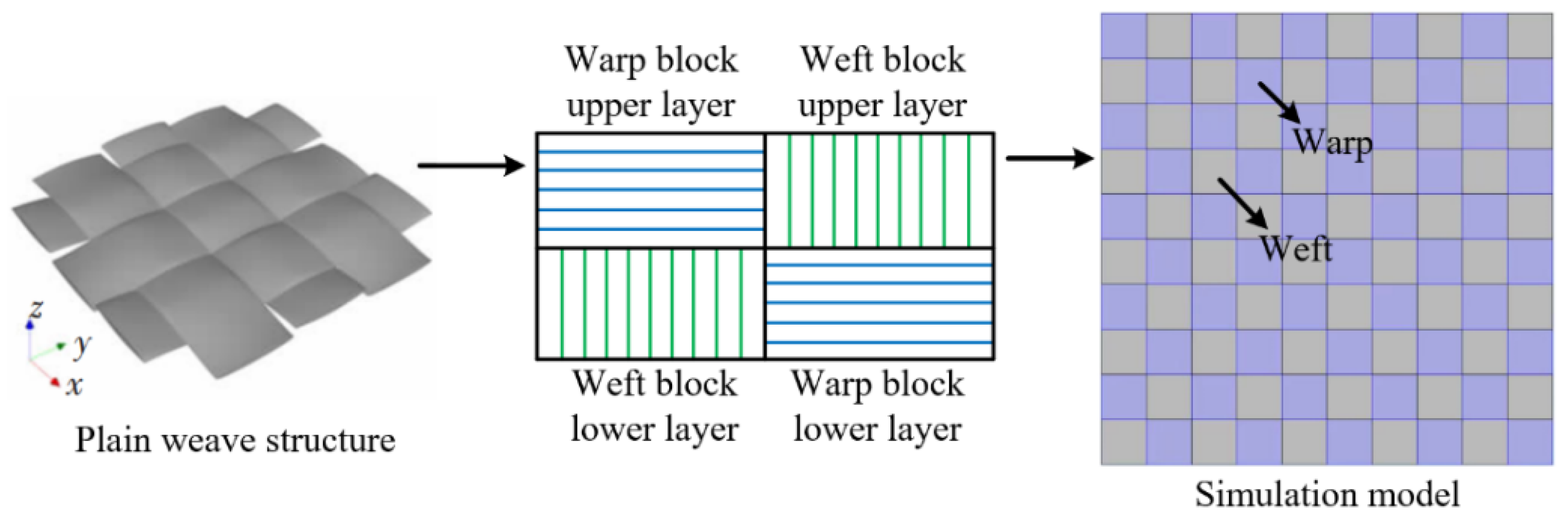
4.2. Quartz Fiber Model
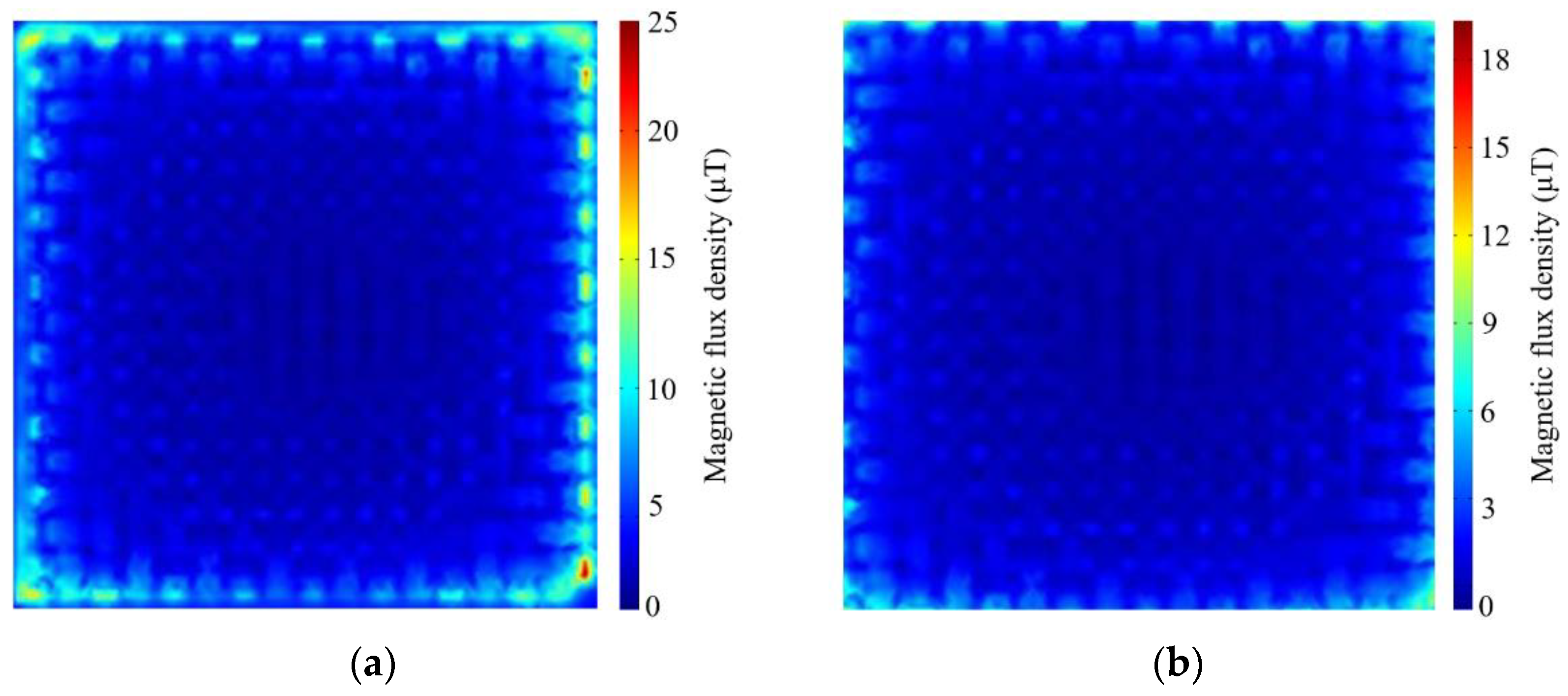
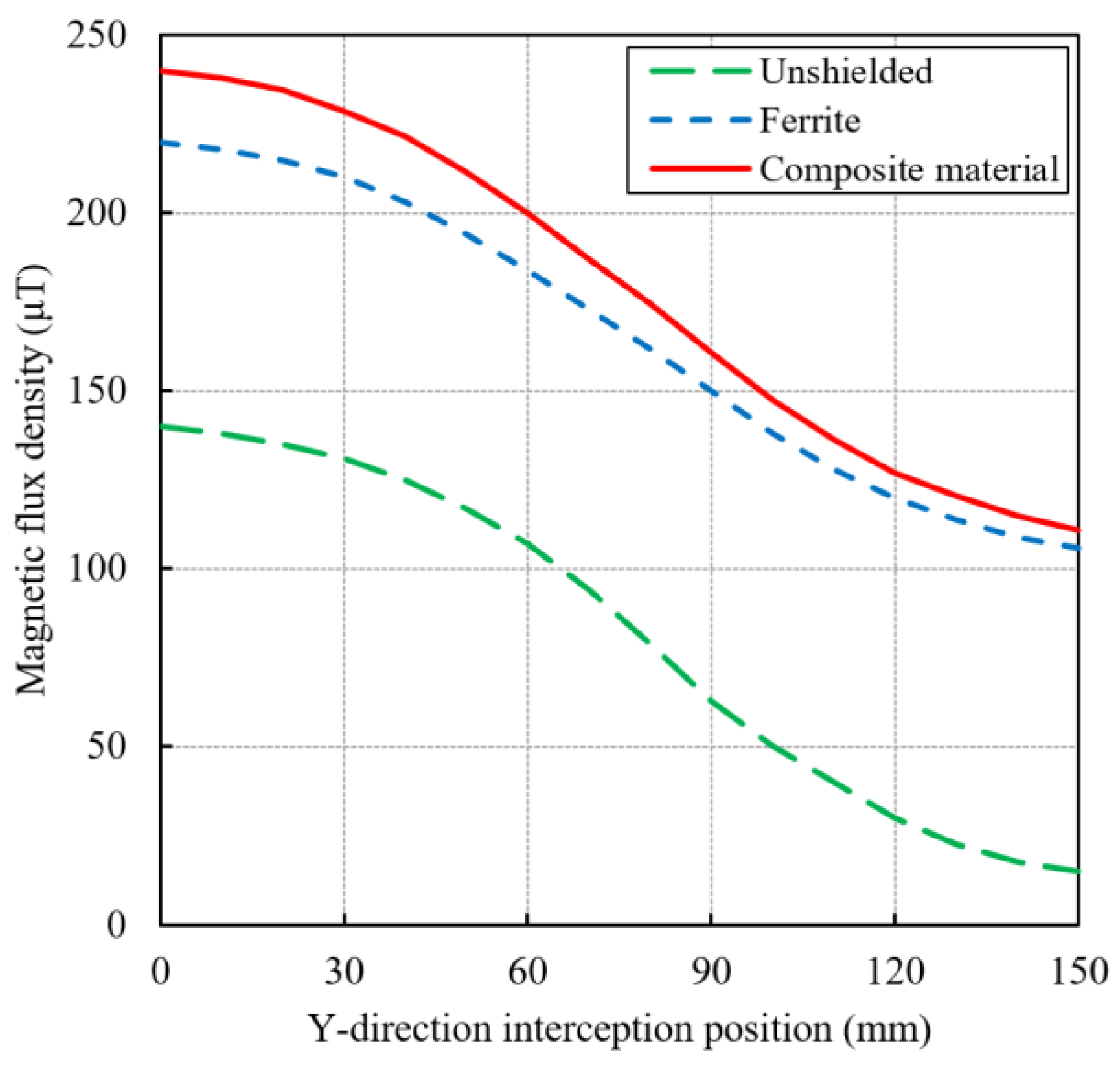
4.3. Magnetic Shielding Research
5. Experimental Verification
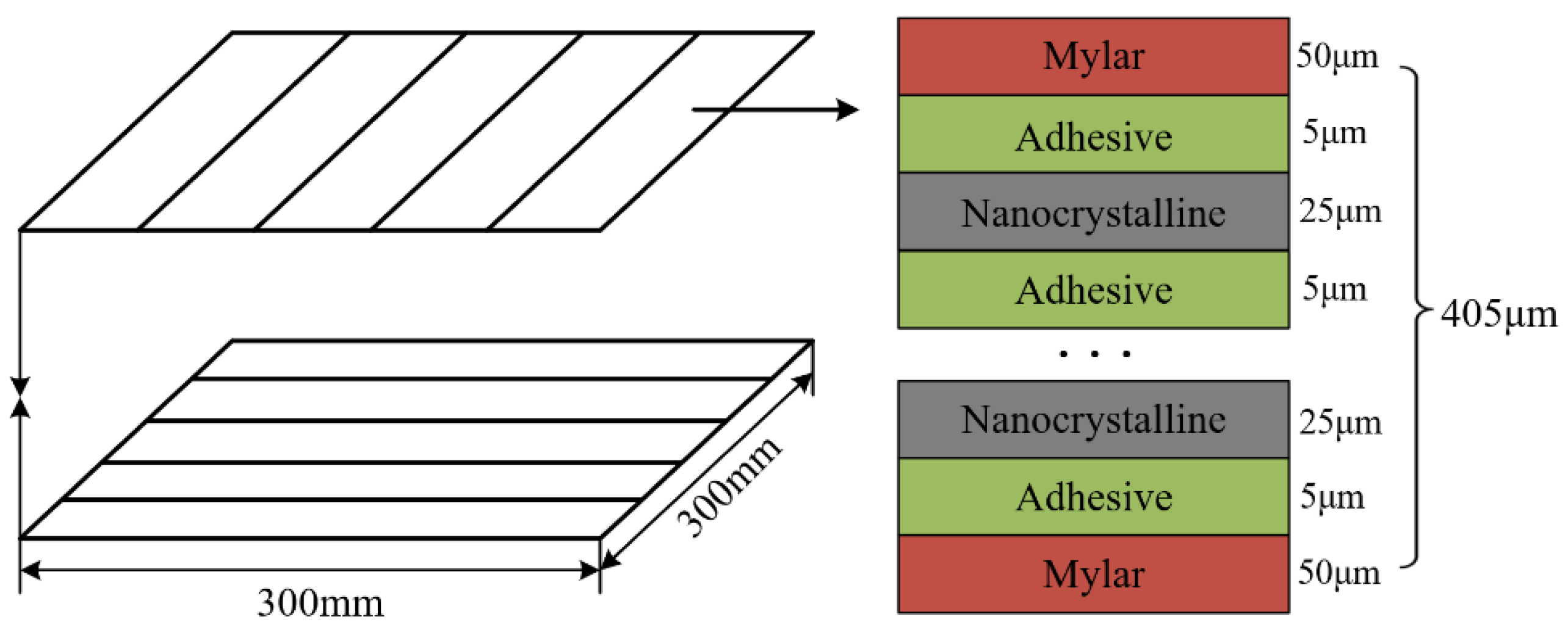
5.1. Composite Material Preparation
- (1)
- Quartz fiber was provided by Henan Shenjiu Tianhang New Material Co., Ltd. (Zhengzhou, China) under model number SJ108, with a plain-weave structure and a thickness of 0.1 mm;
- (2)
- Nanocrystals were provided by Hitachi Metals, Tokyo, Japan, in the form of iron-based nanocrystals (FeCuNbSiB) with a permeability of 157,000 and a saturation flux density of 1.24 T;
- (3)
- Epoxy resin was provided by Tianjin Jingdong Chemical Composites Co., Ltd. (Tianjin, China) under model number 86#; the curing agent was methyl tetrahydrophthalic anhydride, which was provided by Wenzhou Qingming Chemical Co., Ltd. (Wenzhou, China).
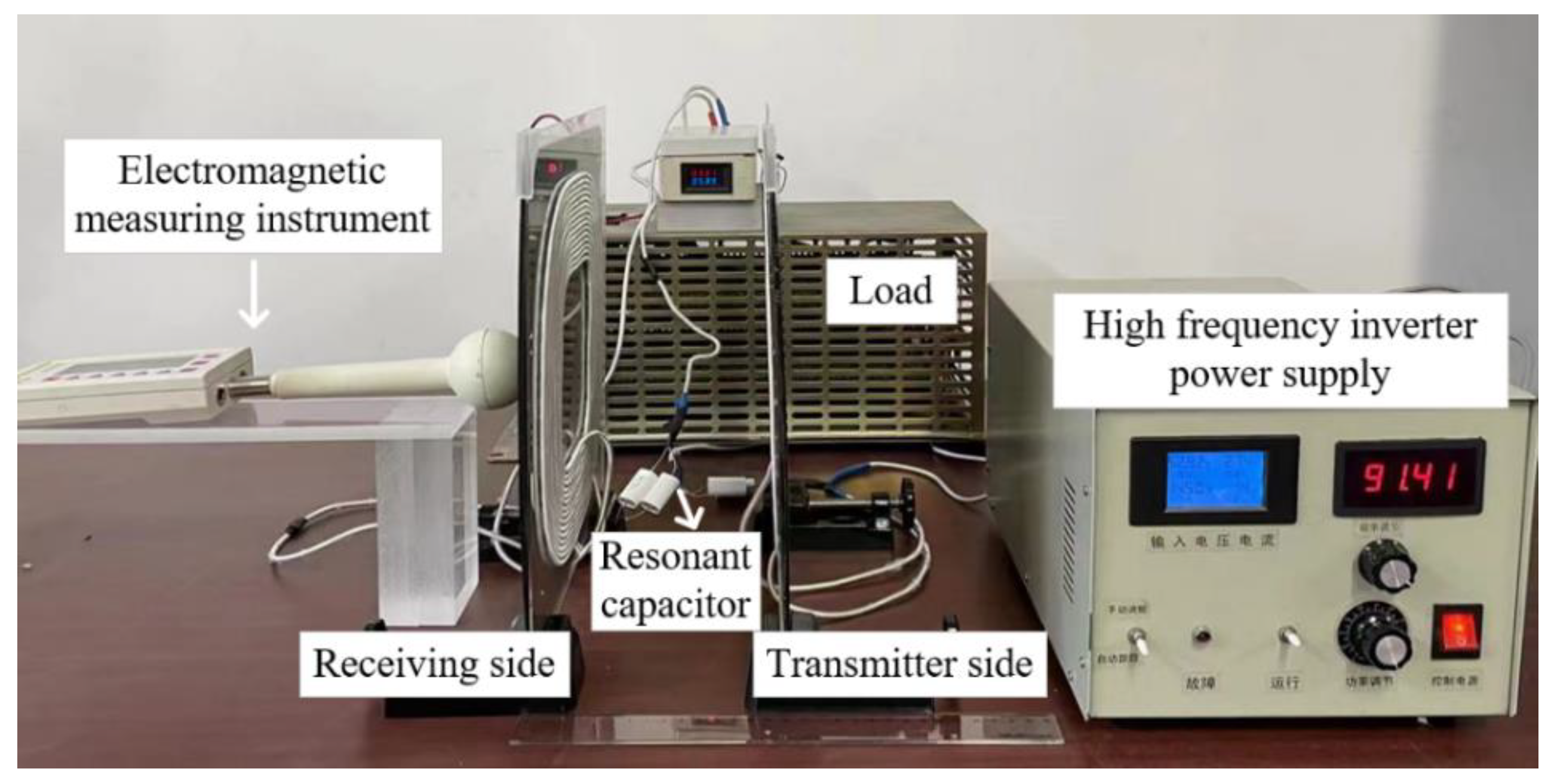
5.2. Experimental Platform Construction
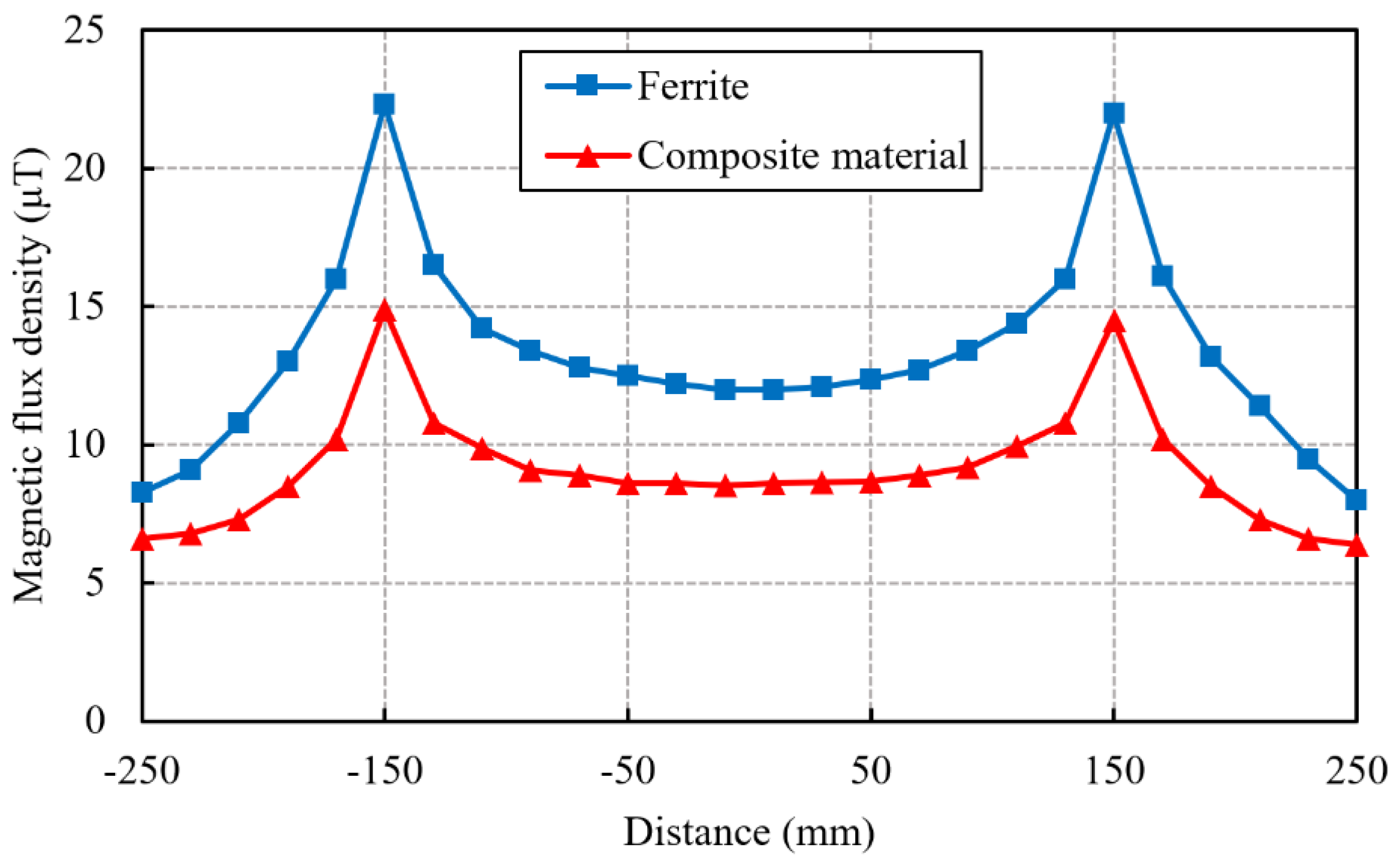
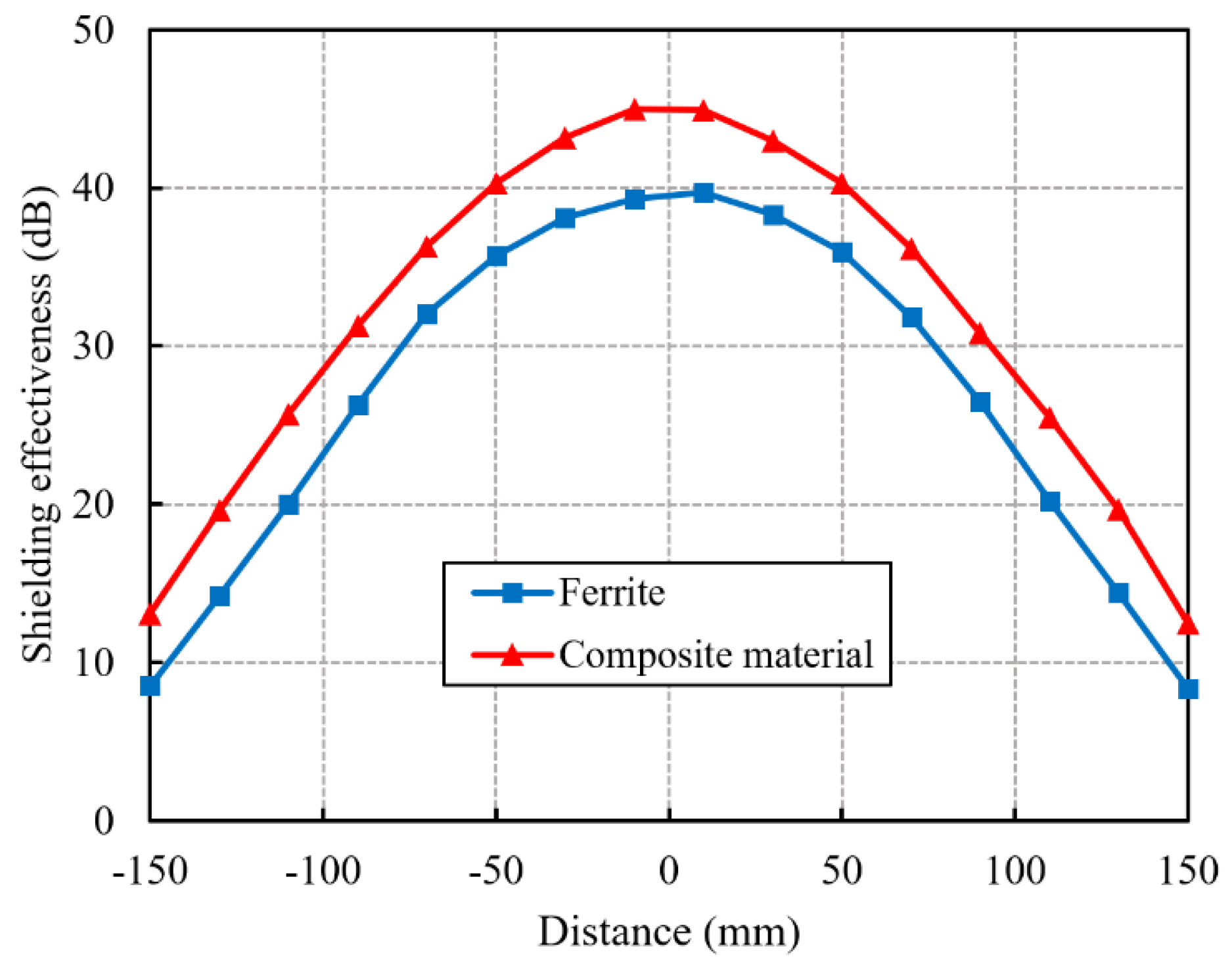
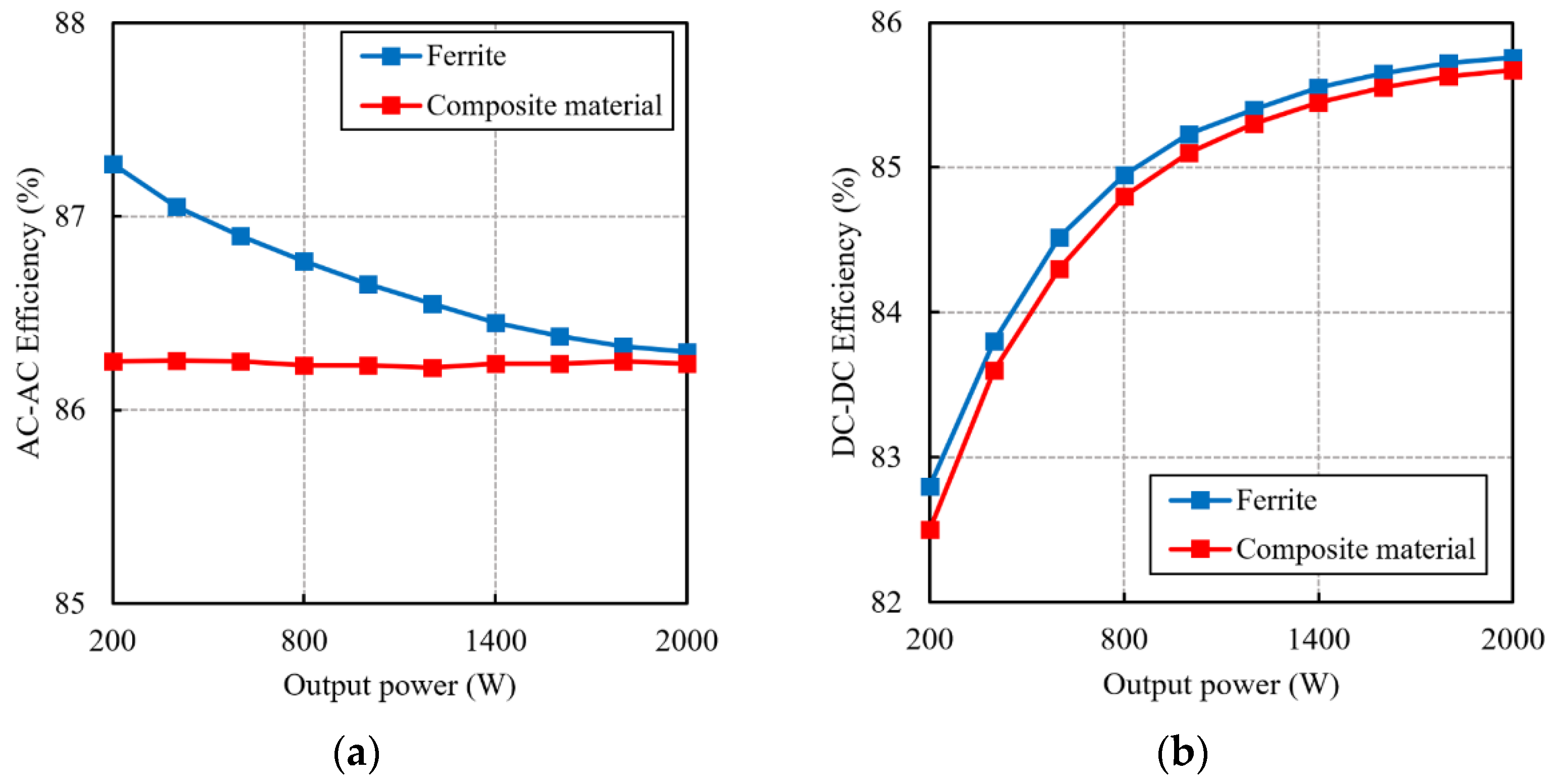
5.3. Experimental Results and Analysis
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Liu, Y.; Wu, T.; Fu, M. Interleaved Capacitive Coupler for Wireless Power Transfer. IEEE Trans. Power Electron. 2021, 36, 13526–13535. [Google Scholar] [CrossRef]
- Zhang, Z.; Pang, H.; Georgiadis, A.; Cecati, C. Wireless Power Transfer—An Overview. IEEE Trans. Ind. Electron. 2019, 66, 1044–1058. [Google Scholar] [CrossRef]
- Sun, J.; Cho, J.; Huang, A.; Kim, H.; Fan, J. Accurate Rectifier Characterization and Improved Modeling of Constant Power Load Wireless Power Transfer Systems. IEEE Trans. Power Electron. 2020, 35, 7840–7852. [Google Scholar] [CrossRef]
- Jiang, Y.; Wang, L.; Wang, Y.; Liu, J.; Li, X.; Ning, G. Analysis, Design, and Implementation of Accurate ZVS Angle Control for EV Battery Charging in Wireless High-Power Transfer. IEEE Trans. Ind. Electron. 2019, 66, 4075–4085. [Google Scholar] [CrossRef]
- Zhang, H.; Gao, S.; Ngo, T.; Wu, W.; Guo, Y. Wireless Power Transfer Antenna Alignment Using Intermodulation for Two-Tone Powered Implantable Medical Devices. IEEE Trans. Microwave Theory Tech. 2019, 67, 1708–1716. [Google Scholar] [CrossRef]
- Van, S.; Ngo, H.; Cotton, S. Wireless Powered Wearables Using Distributed Massive MIMO. IEEE Trans. Commun. 2020, 68, 2156–2172. [Google Scholar] [CrossRef] [Green Version]
- Zhou, J.; Zhang, B.; Xiao, W.; Qiu, D.; Chen, Y. Nonlinear Parity-Time-Symmetric Model for Constant Efficiency Wireless Power Transfer: Application to a Drone-in-Flight Wireless Charging Platform. IEEE Trans. Ind. Electron. 2019, 66, 4097–4107. [Google Scholar] [CrossRef]
- Seo, D.; Lee, J. Frequency-Tuning Method Using the Reflection Coefficient in a Wireless Power Transfer System. IEEE Microwave Wireless Compon. Lett. 2017, 27, 959–961. [Google Scholar] [CrossRef]
- Sun, Y.; Liao, Z.; Ye, Z.; Tang, C.; Wang, P. Determining the Maximum Power Transfer Points for MC-WPT Systems with Arbitrary Number of Coils. IEEE Trans. Power Electron. 2018, 33, 9734–9743. [Google Scholar] [CrossRef]
- Kim, J.; Kim, J.; Kong, S.; Kim, H.; Suh, I.; Suh, N.; Cho, D.; Ahn, S. Coil Design and Shielding Methods for a Magnetic Resonant Wireless Power Transfer System. Proc. IEEE 2013, 101, 1332–1342. [Google Scholar] [CrossRef]
- Shah, I.; Yoo, H. Assessing Human Exposure with Medical Implants to Electromagnetic Fields from a Wireless Power Transmission System in an Electric Vehicle. IEEE Trans. Electromagn. Compat. 2020, 62, 338–345. [Google Scholar] [CrossRef]
- Park, H.; Kwon, J.; Kwak, S.; Ahn, S. Magnetic Shielding Analysis of a Ferrite Plate with a Periodic Metal Strip. IEEE Trans. Magn. 2015, 51, 1–8. [Google Scholar] [CrossRef]
- Feng, J.; Li, Q.; Lee, F. A Square-Shaped Omnidirectional Wireless Charing Bowl with a Double Layer Electromagnetic Shield for Portable Device Applications. In Proceedings of the 2019 IEEE Energy Conversion Congress and Exposition (ECCE), Baltimore, MD, USA, 29 September–3 October 2019; pp. 4961–4966. [Google Scholar]
- Bima, M.; Bhattacharya, I.; Neste, C. Experimental Evaluation of Layered DD Coil Structure in a Wireless Power Transfer System. IEEE Trans. Electromagn. Compat. 2020, 62, 1477–1484. [Google Scholar] [CrossRef]
- Peng, W.; Chen, Z. Enhanced Planar Wireless Power Transfer Systems with Ferrite Material. In Proceedings of the 2018 IEEE Wireless Power Transfer Conference (WPTC), Montreal, QC, Canada, 3–7 June 2018; pp. 1–4. [Google Scholar]
- Strauch, L.; Pavlin, M.; Bregar, V. Optimization, Design, and Modeling of Ferrite Core Geometry for Inductive Wireless Power Transfer. Int. J. Appl. Electromagn. Mech. 2015, 49, 145–155. [Google Scholar] [CrossRef] [Green Version]
- Castelli-Dezza, F.; Mauri, M.; Dolara, A.; Leva, S.; Longo, M. Power Pad Design and Optimization for Contactless Electric Vehicle Battery Charging System. In Proceedings of the 2017 IEEE International Conference on Environment and Electrical Engineering and 2017 IEEE Industrial and Commercial Power Systems Europe (EEEIC/I&CPS Europe), Milan, Italy, 6–9 June 2017; pp. 1–6. [Google Scholar]
- Mohammad, M.; Choi, S.; Islam, Z.; Kwak, S.; Baek, J. Core Design and Optimization for Better Misalignment Tolerance and Higher Range of Wireless Charging of PHEV. IEEE Trans. Transp. Electrif. 2017, 3, 445–453. [Google Scholar] [CrossRef]
- Corti, F.; Grasso, F.; Paolucci, L.; Pugi, L.; Luchetti, L. Circular Coil for EV Wireless Charging Design and Optimization Considering Ferrite Saturation. In Proceedings of the 2019 IEEE 5th International forum on Research and Technology for Society and Industry (RTSI), Florence, Italy, 9–12 September 2019; pp. 279–284. [Google Scholar]
- Choi, B.; Kim, Y. New Structure Design of Ferrite Cores for Wireless Electric Vehicle Charging by Machine Learning. IEEE Trans. Ind. Electron. 2021, 68, 12162–12172. [Google Scholar] [CrossRef]
- Seo, S.; Jo, H.; Bien, F. Free Arrangement Wireless Power Transfer System with a Ferrite Transmission Medium and Geometry-Based Performance Improvement. IEEE Trans. Power Electron. 2020, 35, 4518–4532. [Google Scholar] [CrossRef]
- Pearce, M.; Covic, G.; Boys, J. Reduced Ferrite Double D Pad for Roadway IPT Applications. IEEE Trans. Power Electron. 2021, 36, 5055–5068. [Google Scholar] [CrossRef]
- Delgado, A.; Schoenberger, D.; Oliver, J.; Alou, P.; Cobos, J. Design Guidelines of Inductive Coils Using a Polymer Bonded Magnetic Composite for Inductive Power Transfer Systems in Electric Vehicles. IEEE Trans. Power Electron. 2020, 35, 7884–7893. [Google Scholar] [CrossRef]
- Xiong, M.; Wei, X.; Huang, Y.; Luo, Z.; Dai, H. Research on Novel Flexible High-Saturation Nanocrystalline Cores for Wireless Charging Systems of Electric Vehicles. IEEE Trans. Ind. Electron. 2021, 68, 8310–8320. [Google Scholar] [CrossRef]
- Gaona, D.; Jiang, C.; Long, T. Highly Efficient 11.1-kW Wireless Power Transfer Utilizing Nanocrystalline Ribbon Cores. IEEE Trans. Power Electron. 2021, 36, 9955–9969. [Google Scholar] [CrossRef]
- Wang, Y.; Calderon-Lopez, G.; Forsyth, A. High-Frequency Gap Losses in Nanocrystalline Cores. IEEE Trans. Power Electron. 2017, 32, 4683–4690. [Google Scholar] [CrossRef]
- Li, Y.; Zhao, J.; Yang, Q.; Liu, L.; Ma, J.; Zhang, X. A Novel Coil with High Misalignment Tolerance for Wireless Power Transfer. IEEE Trans. Magn. 2019, 55, 1–4. [Google Scholar] [CrossRef]
- Holloway, C.; Sarto, M.; Johansson, M. Analyzing Carbon-Fiber Composite Materials with Equivalent-Layer Models. IEEE Trans. Electromagn. Compat. 2005, 47, 833–844. [Google Scholar] [CrossRef]
- Campi, T.; Cruciani, S.; Santis, V.; Maradei, F.; Feliziani, M. Numerical Calculation of the Near Field Shielding for Carbon Fiber Reinforced Polymer (CFRP) Panels at Wireless Power Transfer Automotive Frequencies. In Proceedings of the 2018 IEEE Symposium on Electromagnetic Compatibility, Signal Integrity and Power Integrity (EMC, SI & PI), Long Beach, CA, USA, 30 July–3 August 2018; pp. 444–447. [Google Scholar]




| Parameter | Symbol | Value |
|---|---|---|
| Coil inner diameter | Rci | 130 mm |
| Coil outer diameter | Rco | 260 mm |
| Wire radius | r | 2.5 mm |
| Transmission distance | D | 150 mm |
| Coil turns | N | 15 |
| Magnetic shield thickness | T | 2 mm |
| Shield Type | Unshielded | Ferrite | Composite Material |
|---|---|---|---|
| Self-inductance (μH) | 62.31 | 106.23 | 109.35 |
| Mutual inductance (μH) | 7.54 | 19.51 | 20.78 |
| Coupling coefficient (k) | 0.121 | 0.183 | 0.19 |
| Shield Type | Unshielded | Ferrite | Composite Material |
|---|---|---|---|
| Self-inductance (μH) | 60.92 | 105.26 | 108.34 |
| Mutual inductance (μH) | 7.25 | 18.95 | 20.36 |
| Coupling coefficient (k) | 0.119 | 0.18 | 0.188 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, X.; Han, R.; Li, F.; Pan, X.; Chu, Z. Design and Analysis of Magnetic Shielding Mechanism for Wireless Power Transfer System Based on Composite Materials. Electronics 2022, 11, 2187. https://doi.org/10.3390/electronics11142187
Zhang X, Han R, Li F, Pan X, Chu Z. Design and Analysis of Magnetic Shielding Mechanism for Wireless Power Transfer System Based on Composite Materials. Electronics. 2022; 11(14):2187. https://doi.org/10.3390/electronics11142187
Chicago/Turabian StyleZhang, Xin, Rongmei Han, Fangzhou Li, Xuetong Pan, and Zhiqi Chu. 2022. "Design and Analysis of Magnetic Shielding Mechanism for Wireless Power Transfer System Based on Composite Materials" Electronics 11, no. 14: 2187. https://doi.org/10.3390/electronics11142187
APA StyleZhang, X., Han, R., Li, F., Pan, X., & Chu, Z. (2022). Design and Analysis of Magnetic Shielding Mechanism for Wireless Power Transfer System Based on Composite Materials. Electronics, 11(14), 2187. https://doi.org/10.3390/electronics11142187






