Physical Features of High-Density Barium–Tungstate–Phosphate (BTP) Glasses: Elastic Moduli, and Gamma Transmission Factors
Abstract
1. Introduction
2. Materials and Methods
2.1. High-Density Barium–Tungstate–Phosphate (BTP) Glasses
2.2. Elastic Moduli of Barium–Tungstate–Phosphate (BTP) Glasses
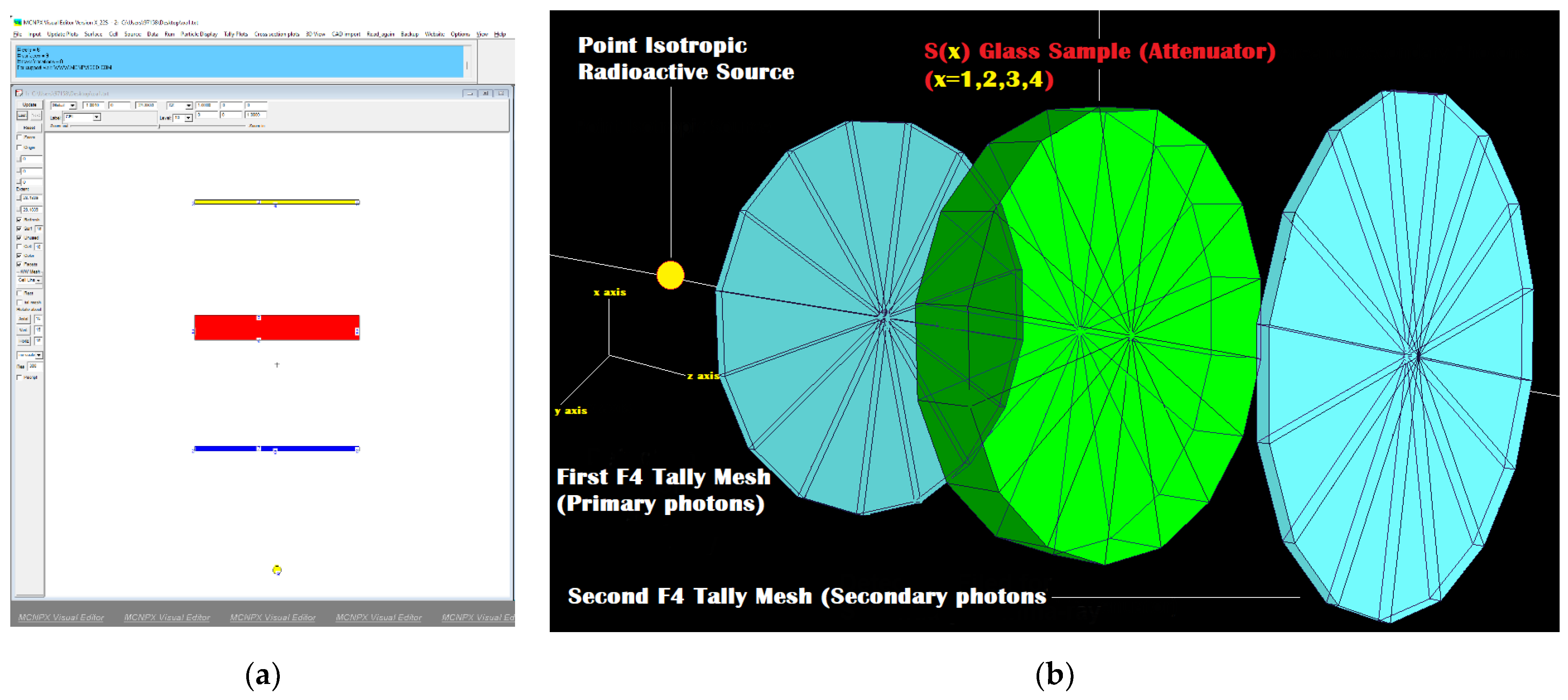
2.3. Radiation Shielding Parameters and Transmission Factors (TFs) of Barium–Tungstate–Phosphate (BTP) Glasses
3. Results and Discussions
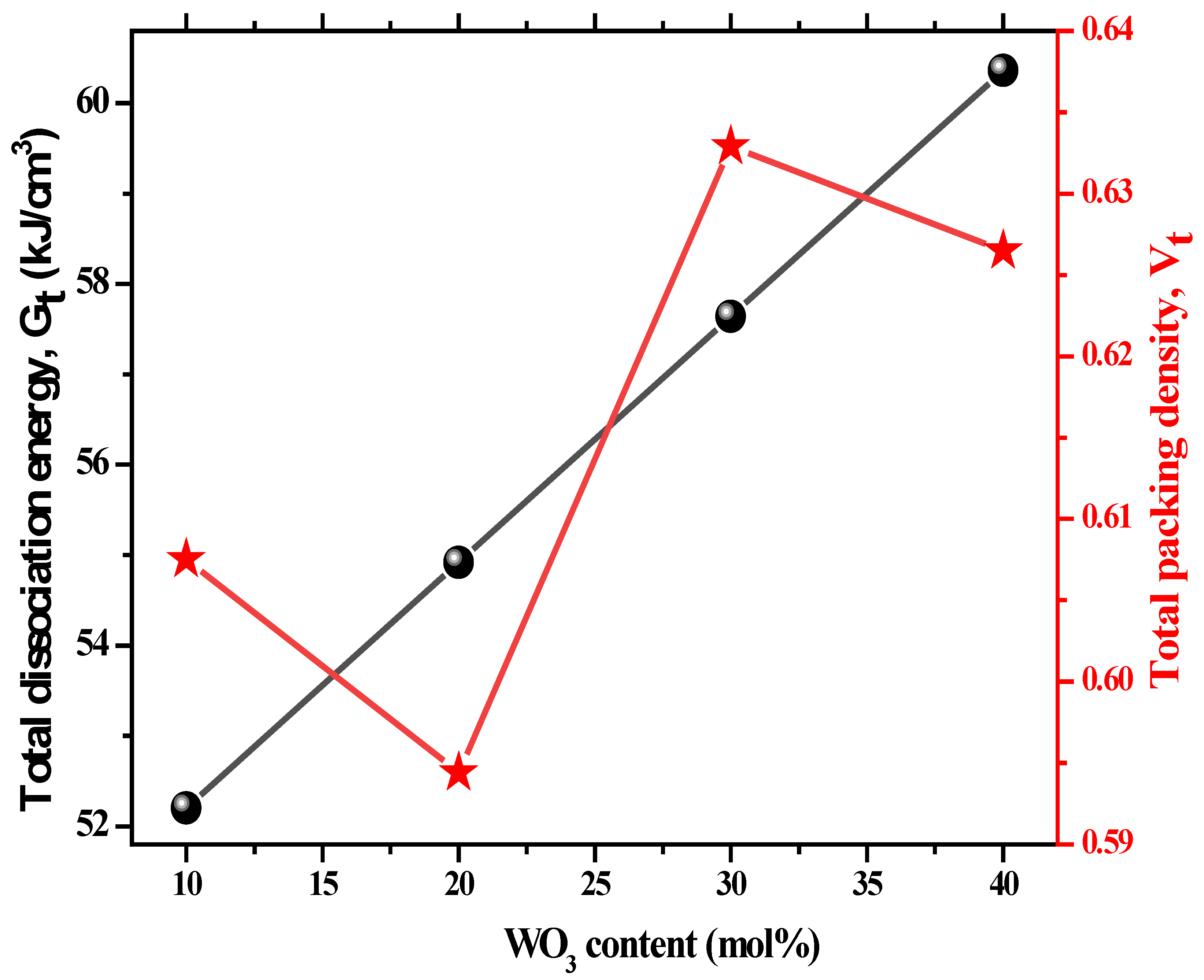
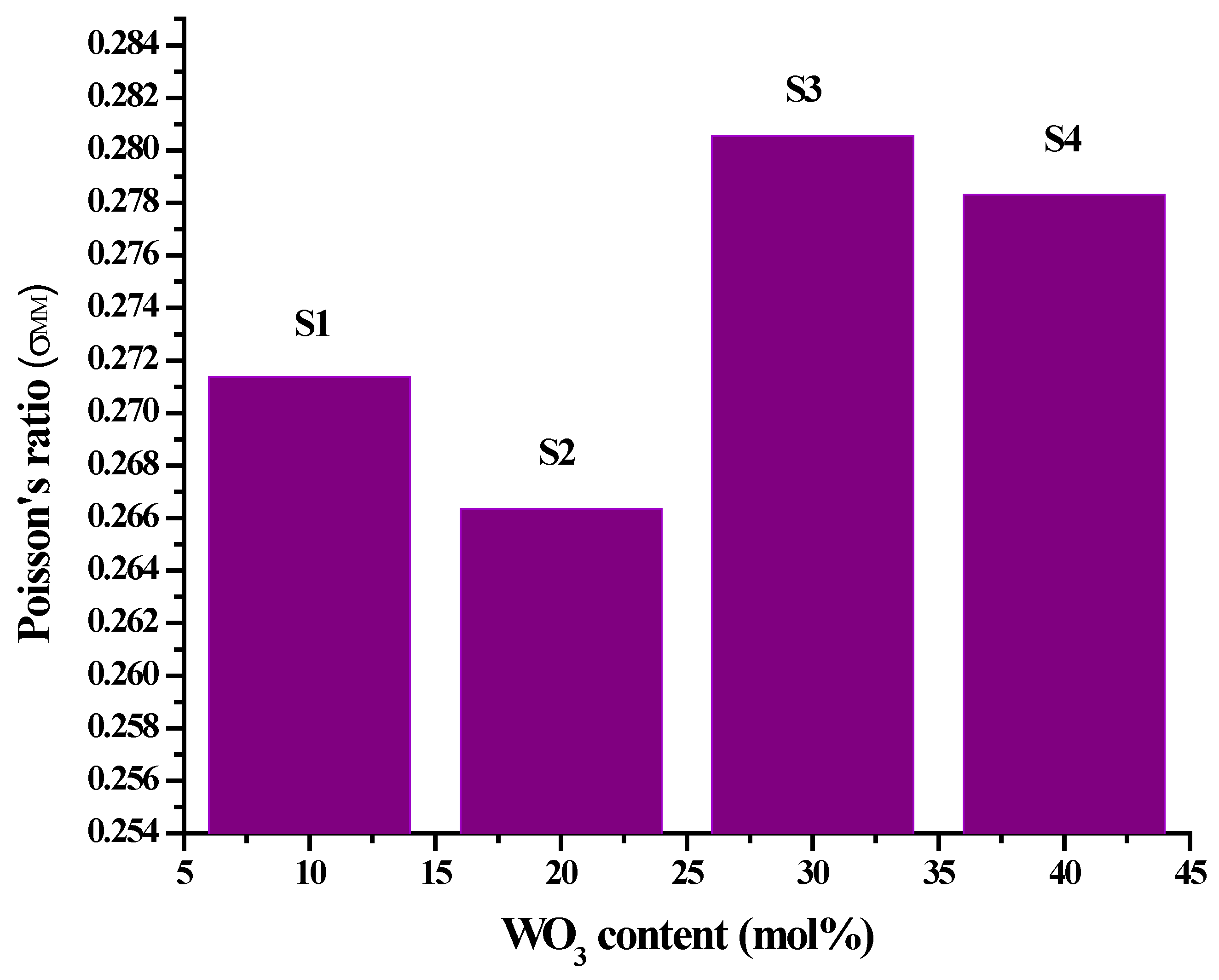
3.1. Elastic Moduli of Barium–Tungstate–Phosphate Glasses
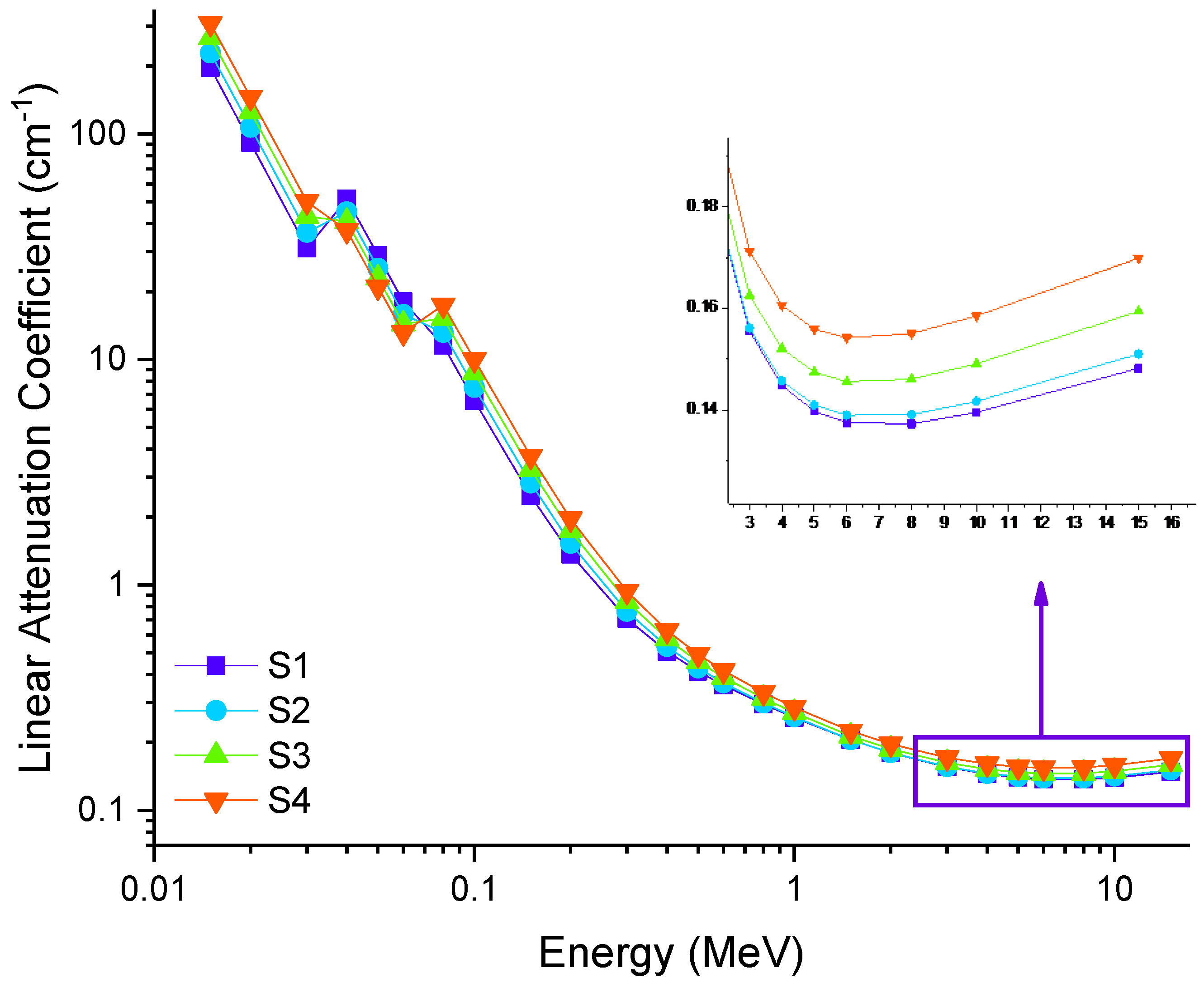
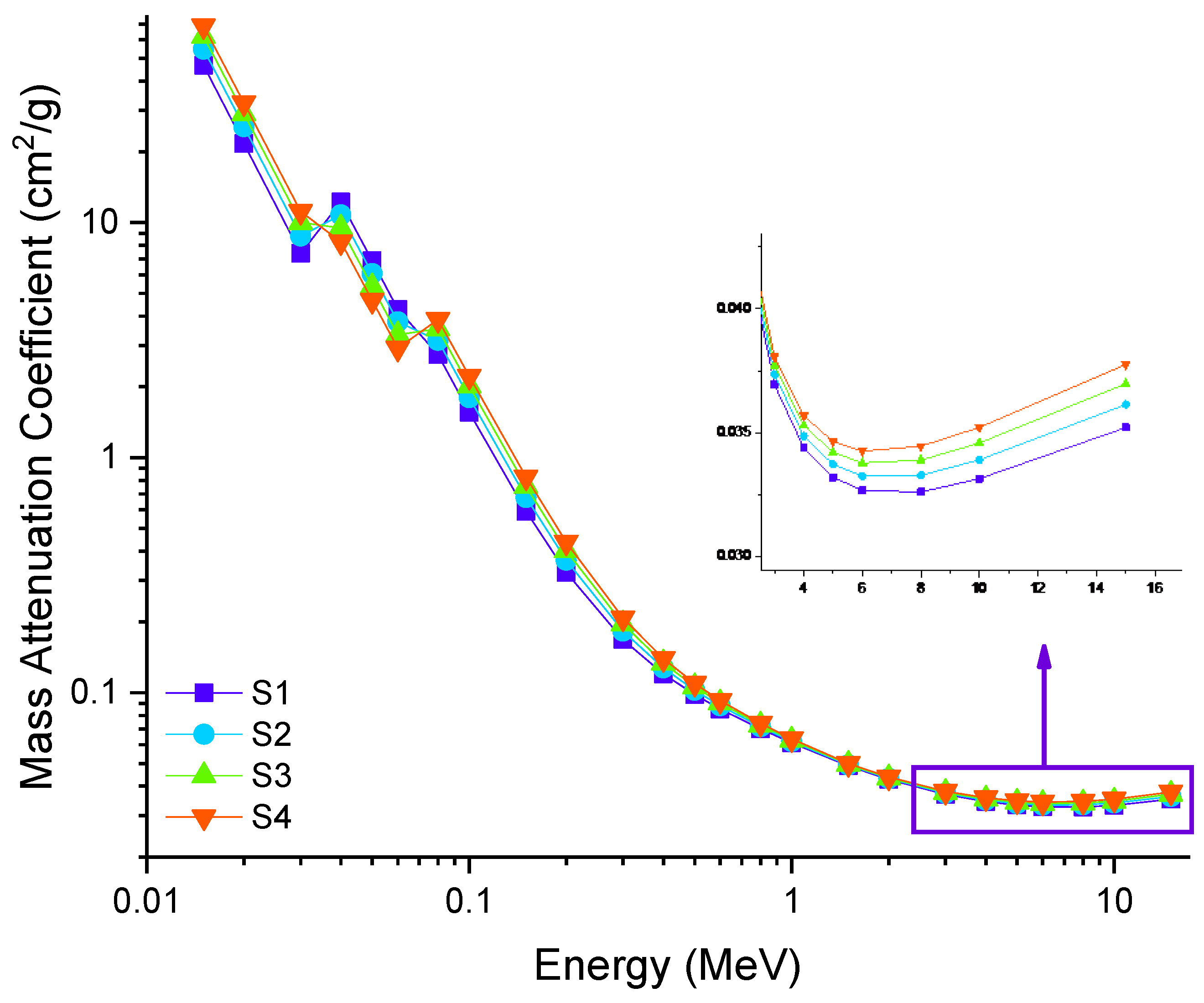
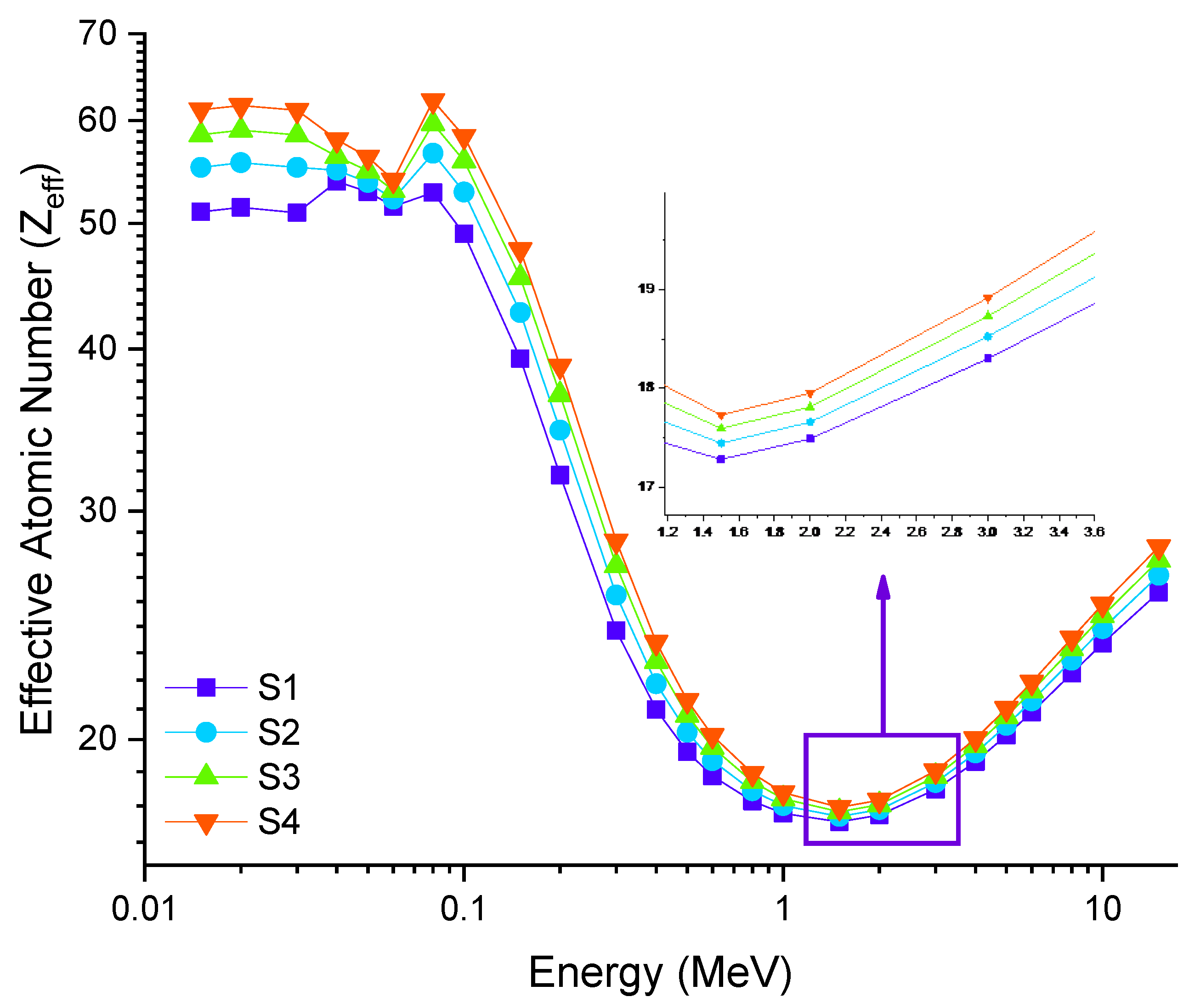
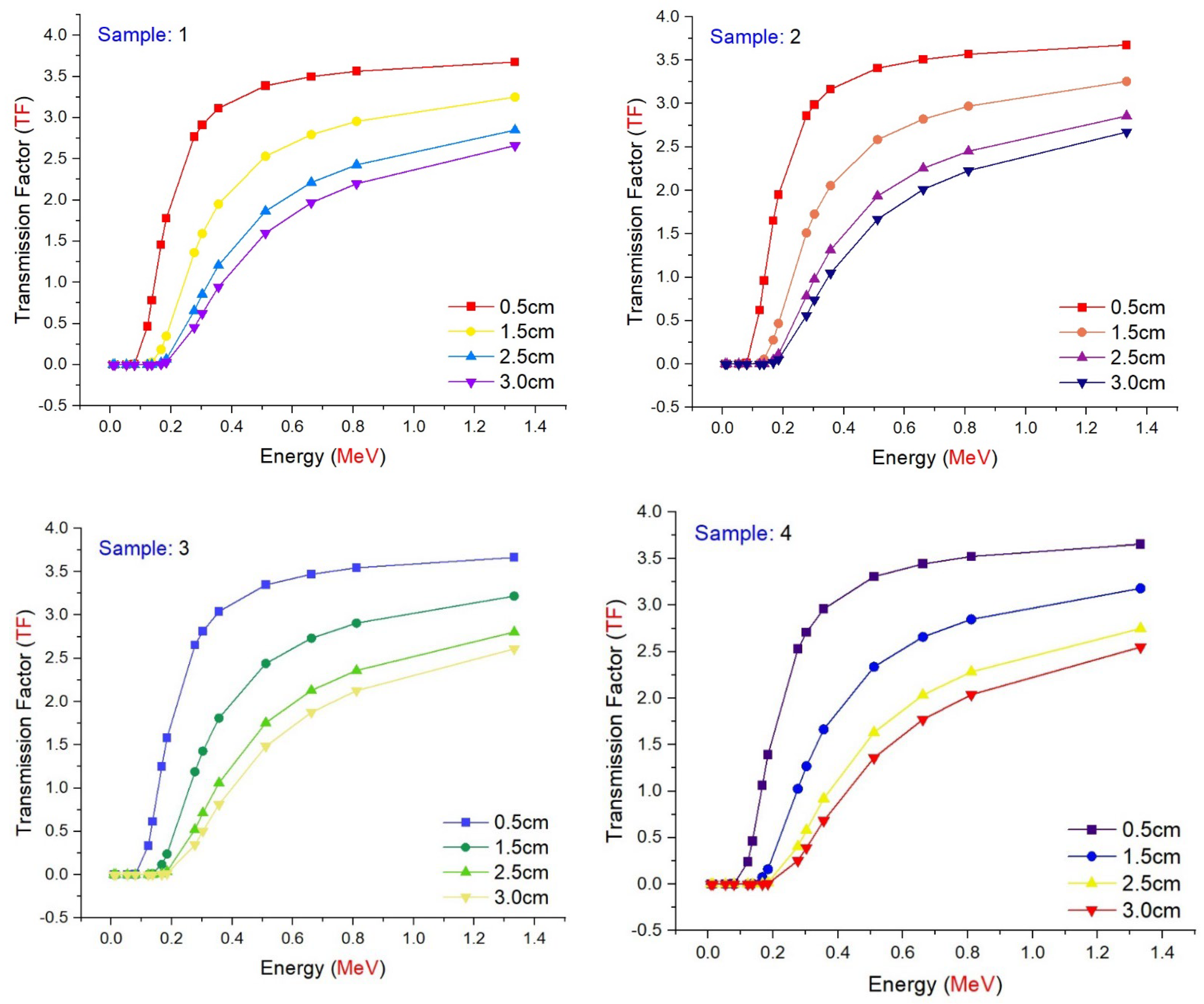
3.2. Radiation Shielding Features of Barium–Tungstate–Phosphate (BTP) Glasses
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ahn, S.; Kim, W.Y.; Lim, K.S.; Ryoo, S.M.; Sohn, C.H.; Seo, D.W.; Kwak, M.K.; Yoon, J.C. Advanced radiology utilization in a tertiary care emergency department from 2001 to 2010. PLoS ONE 2014, 9, e112650. [Google Scholar] [CrossRef] [PubMed]
- Elshami, W.; Abuzaid, M.; Pekkarinen, A.; Kortesniemi, M. Estimation of occupational radiation exposure for medical workers in radiology and cardiology in the united arab emirates: Nine hospitals experience. Radiat. Prot. Dosim. 2020, 189, 466–474. [Google Scholar] [CrossRef] [PubMed]
- Abuzaid, M.M.; Elshami, W.; Steelman, C. Measurements of radiation exposure of radiography students during their clinical training using thermoluminescent dosimetry. Radiat. Prot. Dosim. 2017, 179, 244–247. [Google Scholar] [CrossRef] [PubMed]
- Gurjar, O.P.; Jha, V.K.; Sharma, S.D. Radiation dose to radiotherapy technologists due to induced activity in high energy medical electron linear accelerators. Radiat. Prot. Environ. 2014, 37, 25. [Google Scholar] [CrossRef]
- Elshami, W.; Abuzaid, M.; Piersson, A.D.; Mira, O.; AbdelHamid, M.; Zheng, X.; Kawooya, M.G. Occupational dose and radiation protection practice in UAE: A retrospective cross-sectional cohort study (2002–2016). Radiat. Prot. Dosim. 2019, 187, 426–437. [Google Scholar] [CrossRef]
- Abuzaid, M.M.; Elshami, W.; Shawki, M.; Salama, D. Assessment of compliance to radiation safety and protection at the radiology department. Int. J. Radiat. Res. 2019, 17, 439–446. [Google Scholar]
- Abuzaid, M.M.; Elshami, W.; Hasan, H. Knowledge and adherence to radiation protection among healthcare workers at operation theater. Asian J. Sci. Res. 2018, 12, 54–59. [Google Scholar] [CrossRef][Green Version]
- NG, S.E.; SA, F. Assessment of awareness and practice of ionizing radiation protection procedures among exposed health care workers. Egypt. J. Occup. Med. 2020, 44, 529–544. [Google Scholar] [CrossRef]
- Geletu, G.M.; Abiko, F.; Sahlu, S. Implementation of a radiation protection system at four hospitals in Ethiopia. arXiv 2019, arXiv:1905.07271. [Google Scholar]
- Zuguchi, M.; Chida, K.; Taura, M.; Inaba, Y.; Ebata, A.; Yamada, S. Usefulness of non-lead aprons in radiation protection for physicians performing interventional procedures. Radiat. Prot. Dosim. 2008, 131, 531–534. [Google Scholar] [CrossRef]
- Scuderi, G.J.; Brusovanik, G.V.; Campbell, D.R.; Henry, R.P.; Kwon, B.; Vaccaro, A.R. Evaluation of non-lead-based protective radiological material in spinal surgery. Spine J. 2006, 6, 577–582. [Google Scholar] [CrossRef] [PubMed]
- Elshami, W.; Tekin, H.O.; Al-Buriahi, M.S.; Hegazy, H.H.; Abuzaid, M.M.; Issa, S.A.; Zaid, M.H.M.; Sidek, H.A.A.; Matori, K.; Zakaly, H.M. Developed selenium dioxide-based ceramics for advanced shielding applications: Au2O3 impact on nuclear radiation attenuation. Results Phys. 2021, 24, 104099. [Google Scholar] [CrossRef]
- Deliormanlı, A.M.; Ensoylu, M.; Issa, S.A.; Elshami, W.; Al-Baradi, A.M.; Al-Buriahi, M.S.; Tekin, H.O. WS2/bioactive glass composites: Fabrication, structural, mechanical and radiation attenuation properties. Ceram. Int. 2021, 47, 29739–29747. [Google Scholar] [CrossRef]
- ALMisned, G.; Elshami, W.; Issa, S.A.; Susoy, G.; Zakaly, H.M.; Algethami, M.; Rammah, Y.S.; Ene, A.; Al-Ghamdi, S.A.; Ibraheem, A.A.; et al. Enhancement of gamma-ray shielding properties in cobalt-doped heavy metal borate glasses: The role of lanthanum oxide reinforcement. Materials 2021, 14, 7703. [Google Scholar] [CrossRef] [PubMed]
- Saudi, H.A.; Tekin, H.O.; Zakaly, H.M.H.; Issa, S.A.M.; Susoy, G.; Zhukovsky, M. The impact of samarium (III) oxide on structural, optical and radiation shielding properties of thallium-borate glasses: Experimental and numerical investigation. Opt. Mater. 2021, 114, 110948. [Google Scholar] [CrossRef]
- Tekin, H.O.; Issa, S.A.M.; Ahmed, E.M.; Rammah, Y.S. Lithium-fluoro borotellurite glasses: Nonlinear optical, mechanical, characteristics and gamma radiation protection characteristics. Radiat. Phys. Chem. 2022, 190, 109819. [Google Scholar] [CrossRef]
- Kilic, G.; Ilik, E.; Issa, S.A.M.; Issa, B.; Al-Buriahi, M.S.; Issever, U.G.; Zakaly, H.M.H.; Tekin, H.O. Ytterbium (III) oxide reinforced novel TeO2-B2O3-V2O5 glass system: Synthesis and optical, structural, physical and thermal properties. Ceram. Int. 2021, 47, 18517–18531. [Google Scholar] [CrossRef]
- Saudi, H.; Zakaly, H.M.; Issa, S.A.; Tekin, H.; Hessien, M.; Rammah, Y.; Henaish, A. Fabrication, FTIR, physical characteristics and photon shielding efficacy of CeO2 /sand reinforced borate glasses: Experimental and simulation studies. Radiat. Phys. Chem. 2021, 191, 109837. [Google Scholar] [CrossRef]
- Alalawi, A.; Al-Buriahi, M.S.; Rammah, Y.S. Radiation shielding properties of PNCKM bioactive glasses at nuclear medicine energies. Ceram. Int. 2020, 46, 15027–15033. [Google Scholar] [CrossRef]
- Abouhaswa, A.S.; El-Agawany, F.I.; Ahmed, E.M.; Rammah, Y.S. Optical, magnetic characteristics, and nuclear radiation shielding capacity of newly synthesized barium boro-vanadate glasses: B2O3-BaF2-Na2O-V2O5. Radiat. Phys. Chem. 2021, 192, 109922. [Google Scholar] [CrossRef]
- Issa, S.A.M.; Rashad, M.; Zakaly, H.M.H.; Tekin, H.O.; Abouhaswa, A.S. Nb2O5-Li2O-Bi2O3-B2O3 novel glassy system: Evaluation of optical, mechanical, and gamma shielding parameters. J. Mater. Sci. Mater. Electron. 2020, 31, 22039–22056. [Google Scholar] [CrossRef]
- Rammah, Y.; Olarinoye, I.; El-Agawany, F.; Ahmed, E.M.; Salem, W.M. Influence of Sm2O3 content on photon and fast neutron interaction parameters of zinc-tellurite glasses. Radiat. Phys. Chem. 2022, 192, 109914. [Google Scholar] [CrossRef]
- Rammah, Y.S.; El-Agawany, F.I.; Abdel Wahab, E.A.; Hessien, M.M.; Shaaban, K.S. Significant impact of V2O5 content on lead phosphor-arsenate glasses for mechanical and radiation shielding applications. Radiat. Phys. Chem. 2022, 193, 109956. [Google Scholar] [CrossRef]
- Tekin, H.O.; Issa, S.A.M.; Kilic, G.; Zakaly, H.M.H.; Tarhan, N.; Sidek, H.A.A.; Matori, K.A.; Zaid, M.H.M. A systematical characterization of TeO2-V2O5 glass system using boron (Iii) oxide and neodymium (iii) oxide substitution: Resistance behaviors against ionizing radiation. Appl. Sci. 2021, 11, 3035. [Google Scholar] [CrossRef]
- Rammah, Y.S.; Olarinoye, I.O.; El-Agawany, F.I.; El-Adawy, A.; Yousef, E. The impact of PbF2 on the ionizing radiation shielding competence and mechanical properties of TeO2-PbF2 glasses and glass-ceramics. Ceram. Int. 2020, 47, 2547–2556. [Google Scholar] [CrossRef]
- Rammah, Y.S.; Zakaly, H.M.H.; Issa, S.A.M.; Tekin, H.O.; Hessien, M.M.; Saudi, H.A.; Henaish, A.M.A. Fabrication, physical, structural, and optical investigation of cadmium lead-borate glasses doped with Nd3+ ions: An experimental study. J. Mater. Sci. Mater. Electron. 2021, 33, 1877–1887. [Google Scholar] [CrossRef]
- Hegazy, H.H.; Al-Buriahi, M.S.; Alresheedi, F.; El-Agawany, F.I.; Sriwunkum, C.; Neffati, R.; Rammah, Y.S. Nuclear shielding properties of B2O3-Bi2O3-SrO glasses modified with Nd2O3: Theoretical and simulation studies. Ceram. Int. 2020, 47, 2772–2780. [Google Scholar] [CrossRef]
- Tekin, H.O.; ALMisned, G.; Susoy, G.; Ali, F.T.; Baykal, D.S.; Ene, A.; Issa, S.A.M.; Rammah, Y.S.; Zakaly, H.M.H. Transmission Factor (TF) Behavior of Bi2O3-TeO2-Na2O-TiO2-ZnO Glass System: A Monte Carlo Simulation Study. Sustainability 2022, 14, 2893. [Google Scholar] [CrossRef]
- Zhang, B.; He, F.; Cao, X.; Wei, M.; Zheng, C.; Xie, J. The effect of TiO2 and B2O3 on sintering behavior and crystallization behavior of SrO-BaO-B2O3-SiO2 glass-ceramics. Ceram. Int. 2022, 48, 7013–7023. [Google Scholar] [CrossRef]
- Zhang, B.; He, F.; Cao, X.; Ren, H.; Yan, D.; Xie, J. Effect of SiO2/BaO ratio on sintering behavior, crystallization behavior, and properties of SrO-BaO-B2O3-SiO2 glass-ceramics. Ceram. Int. 2021, 47, 19043–19051. [Google Scholar] [CrossRef]
- Agar, O.; Khattari, Z.Y.; Sayyed, M.I.; Tekin, H.O.; Al-Omari, S.; Maghrabi, M.; Zaid, M.H.M.; Kityk, I.V. Evaluation of the shielding parameters of alkaline earth based phosphate glasses using MCNPX code. Results Phys. 2018, 12, 101–106. [Google Scholar] [CrossRef]
- Rammah, Y.S.; Kilic, G.; El-Mallawany, R.; Issever, U.G.; El-Agawany, F.I. Investigation of optical, physical, and gamma-ray shielding features of novel vanadyl boro-phosphate glasses. J. Non-Cryst. Solids 2020, 533, 119905. [Google Scholar] [CrossRef]
- Zakaly, H.M.H.; Saudi, H.A.; Issa, S.A.M.; Rashad, M.; Elazaka, A.I.; Tekin, H.O.; Saddeek, Y.B. Alteration of optical, structural, mechanical durability and nuclear radiation attenuation properties of barium borosilicate glasses through BaO reinforcement: Experimental and numerical analyses. Ceram. Int. 2021, 47, 5587–5596. [Google Scholar] [CrossRef]
- Doweidar, H.; El-Damrawi, G.; Agammy, E.F. El Structural correlations in BaO-PbO-B2O3 glasses as inferred from FTIR spectra. Vib. Spectrosc. 2014, 73, 90–96. [Google Scholar] [CrossRef]
- Sene, F.F.; Martinelli, J.R.; Gomes, L. Synthesis and characterization of niobium phosphate glasses containing barium and potassium. Non-Cryst. Solids 2004, 348, 30–37. [Google Scholar] [CrossRef]
- Koudelka, L.; Šubčík, J.; Mošner, P.; Gregora, I.; Montagne, L.; Delevoye, L.; Gregora, I. Glass-forming ability and structure of glasses in ZnO-WO3-P2O5 system. Phys. Chem. Glasses Eur. J. Glass Sci. Technol. B 2012, 53, 79–85. [Google Scholar]
- Koudelka, L.; Rösslerová, I.; Mošner, P.; Černošek, Z.; Lissová, M.; Liška, M.; Montagne, L.; Delevoye, L. Structure and properties of lead tungstate-phosphate glasses. Phys. Chem. Glasses Eur. J. Glass Sci. Technol. B 2012, 53, 86–92. [Google Scholar]
- Kalenda, P.; Koudelka, L.; Mošner, P.; Montagne, L.; Revel, B. Glass-forming ability and the structure of glasses in the BaO-WO3-P2O5 system. J. Non-Cryst. Solids 2020, 541, 120145. [Google Scholar] [CrossRef]
- Makishima, A.; Mackenzie, J.D. Direct Calculation of Young’s Modulus of Glass. J. Non-Cryst. Solids 1973, 12, 35–45. [Google Scholar] [CrossRef]
- Inaba, S.; Fujino, S.; Morinaga, K. Young’s Modulus and Compositional Parameters of Oxide Glasses. J. Am. Ceram. Soc. 1999, 82, 3501–3507. [Google Scholar] [CrossRef]
- Olarinoye, I.O.; El-Agawany, F.I.; El-Adawy, A.; Yousef, E.; Rammah, Y.S. Mechanical features, alpha particles, photon, proton, and neutron interaction parameters of TeO2-V2O3-MoO3 semiconductor glasses. Ceram. Int. 2020, 46, 23134–23144. [Google Scholar] [CrossRef]
- Rammah, Y.S.; Olarinoye, I.O.; El-Agawany, F.I.; El-Adawy, A.; Gamal, A.; Yousef, E. Elastic moduli, photon, neutron, and proton shielding parameters of tellurite bismo-vanadate (TeO2-V2O5-Bi2O3) semiconductor glasses. Ceram. Int. 2020, 46, 25440–25452. [Google Scholar] [CrossRef]
- Elkhoshkhany, N.; Syala, E.; Yousef, E. Concentration dependence of the elastic moduli, thermal properties, and non-isothermal kinetic parameters of Yb3+ doped multicomponent tellurite glass system. Results Phys. 2020, 16, 102876. [Google Scholar] [CrossRef]
- Almateri, M.; Agar, O.; Altunsoy, E.E.; Kilicoglu, O.; Sayyed, M.I.; Tekin, H.O. Photon and neutron shielding characteristics of samarium doped lead alumino borate glasses containing barium, lithium and zinc oxides determined at medical diagnostic energies. Results Phys. 2019, 12, 2123–2128. [Google Scholar] [CrossRef]
- IAEA. RSICC Computer Code Collection, MCNPX User’s Manual Version 2.4.0. Monte Carlo N-Particle Transport Code System for Multiple and High Energy Applications; IAEA: Vienna, Austria, 2004; Available online: https://inis.iaea.org/search/searchsinglerecord.aspx?recordsFor=SingleRecord&RN=39098954 (accessed on 1 October 2022).
- Şakar, E.; Özpolat, Ö.F.; Alım, B.; Sayyed, M.I.; Kurudirek, M. Phy-X/PSD: Development of a user friendly online software for calculation of parameters relevant to radiation shielding and dosimetry. Radiat. Phys. Chem. 2019, 166, 108496. [Google Scholar] [CrossRef]
- Akkurt, I.; Tekin, H.O. Radiological Parameters for Bismuth Oxide Glasses Using Phy-X/PSD Software. Emerg. Mater. Res. 2020, 9, 1020–1027. [Google Scholar] [CrossRef]
- Ozge Kilicoglu, H.O. Tekin. Bioactive glasses and direct effect of increased K2O additive for nuclear shielding performance: A comparative investigation. Ceram. Int. 2019, 46, 1323–1333. [Google Scholar] [CrossRef]
- Lakshminarayana, G.; Kumar, A.; Tekin, H.; Issa, S.A.; Al-Buriahi, M.; Dong, M.; Lee, D.-E.; Yoon, J.; Park, T. Illustration of distinct nuclear radiation transmission factors combined with physical and elastic characteristics of barium boro-bismuthate glasses. Results Phys. 2021, 31, 105067. [Google Scholar] [CrossRef]
- El-Taher, A.; Zakaly, H.M.H.; Pyshkina, M.; Allam, E.A.; El-Sharkawy, R.M.; Mahmoud, M.E.; Abdel-Rahman, M.A.E. A comparative Study Between Fluka and Microshield Modeling Calculations to study the Radiation-Shielding of Nanoparticles and Plastic Waste composites. Z. Fur Anorg. Und Allg. Chem. 2021, 647, 1083–1090. [Google Scholar] [CrossRef]



| Samples Code | Elemental Compositions (wt%) | Composition (mol%) | Density, ρ (g/cm3) ± 0.01 [38] | Molar Volume, Vm (cm3/mol) ± 0.01 [38] | |||||
|---|---|---|---|---|---|---|---|---|---|
| O | P | Ba | W | BaO | P2O5 | WO3 | |||
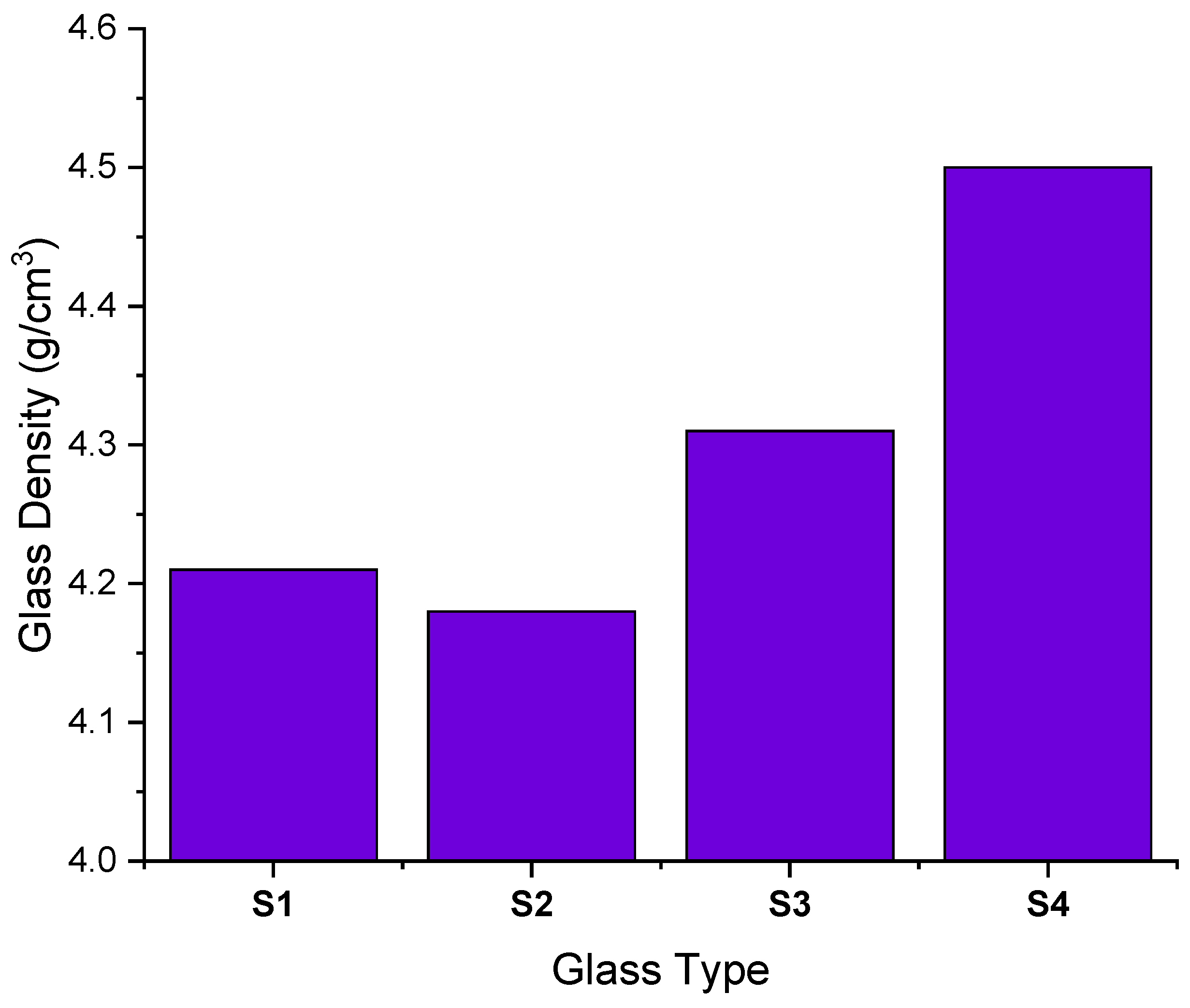
| S1 | 0.286015 | 0.158207 | 0.438403 | 0.117376 | 50 | 40 | 10 | 4.21 | 37.2 |
| S2 | 0.291817 | 0.150655 | 0.333981 | 0.223546 | 40 | 40 | 20 | 4.18 | 39.4 |
| S3 | 0.297091 | 0.143792 | 0.239075 | 0.320043 | 30 | 40 | 30 | 4.31 | 38.3 |
| S4 | 0.301905 | 0.137526 | 0.152438 | 0.40813 | 20 | 40 | 40 | 4.5 | 40 |
| Oxide | Vi (cm3/mol) [37,38] | Gi (kJ/cm3) [37,38] |
|---|---|---|
| BaO | 13.1 | 40.6 |
| WO3 | 21.3 | 67.8 |
| P2O5 | 34.8 | 62.8 |
| Parameters and Elastic Moduli | S1 | S2 | S3 | S4 |
|---|---|---|---|---|
| ± 0.001 | 0.607 | 0.594 | 0.633 | 0.627 |
| ± 0.001 (kJ/cm3) | 52.2 | 54.92 | 57.64 | 60.36 |
| ± 0.01 (GPa) | 63.426 | 65.291 | 72.961 | 75.631 |
| ± 0.001 (GPa) | 46.239 | 46.572 | 55.412 | 56.859 |
| ± 0.001 (Gpa) | 24.944 | 25.779 | 28.488 | 29.582 |
| ± 0.001 (Gpa) | 79.414 | 80.858 | 93.301 | 96.204 |
| ± 0.001 (GPa) | 0.271 | 0.266 | 0.281 | 0.278 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zakaly, H.M.H.; Tekin, H.O.; Rammah, Y.S.; Issa, S.A.M.; Alomari, A.H.; Ali, F.T.; Baykal, D.S.; Elshami, W.; Abulyazied, D.E.; ALMisned, G.; et al. Physical Features of High-Density Barium–Tungstate–Phosphate (BTP) Glasses: Elastic Moduli, and Gamma Transmission Factors. Electronics 2022, 11, 4095. https://doi.org/10.3390/electronics11244095
Zakaly HMH, Tekin HO, Rammah YS, Issa SAM, Alomari AH, Ali FT, Baykal DS, Elshami W, Abulyazied DE, ALMisned G, et al. Physical Features of High-Density Barium–Tungstate–Phosphate (BTP) Glasses: Elastic Moduli, and Gamma Transmission Factors. Electronics. 2022; 11(24):4095. https://doi.org/10.3390/electronics11244095
Chicago/Turabian StyleZakaly, Hesham M. H., Huseyin O. Tekin, Yasser S. Rammah, Shams A. M. Issa, Ali Hamed Alomari, Fatema T. Ali, Duygu Sen Baykal, Wiam Elshami, D. E. Abulyazied, Ghada ALMisned, and et al. 2022. "Physical Features of High-Density Barium–Tungstate–Phosphate (BTP) Glasses: Elastic Moduli, and Gamma Transmission Factors" Electronics 11, no. 24: 4095. https://doi.org/10.3390/electronics11244095
APA StyleZakaly, H. M. H., Tekin, H. O., Rammah, Y. S., Issa, S. A. M., Alomari, A. H., Ali, F. T., Baykal, D. S., Elshami, W., Abulyazied, D. E., ALMisned, G., Mostafa, A. M. A., & Ene, A. (2022). Physical Features of High-Density Barium–Tungstate–Phosphate (BTP) Glasses: Elastic Moduli, and Gamma Transmission Factors. Electronics, 11(24), 4095. https://doi.org/10.3390/electronics11244095













