8-Plate Multi-Resonant Coupling Using a Class-E2 Power Converter for Misalignments in Capacitive Wireless Power Transfer
Abstract
:1. Introduction
2. Materials and Methods
2.1. Implementation of Multi-Resonance to Minimize the Impact of Misalignment
2.1.1. Frequency Splitting in WPT Systems
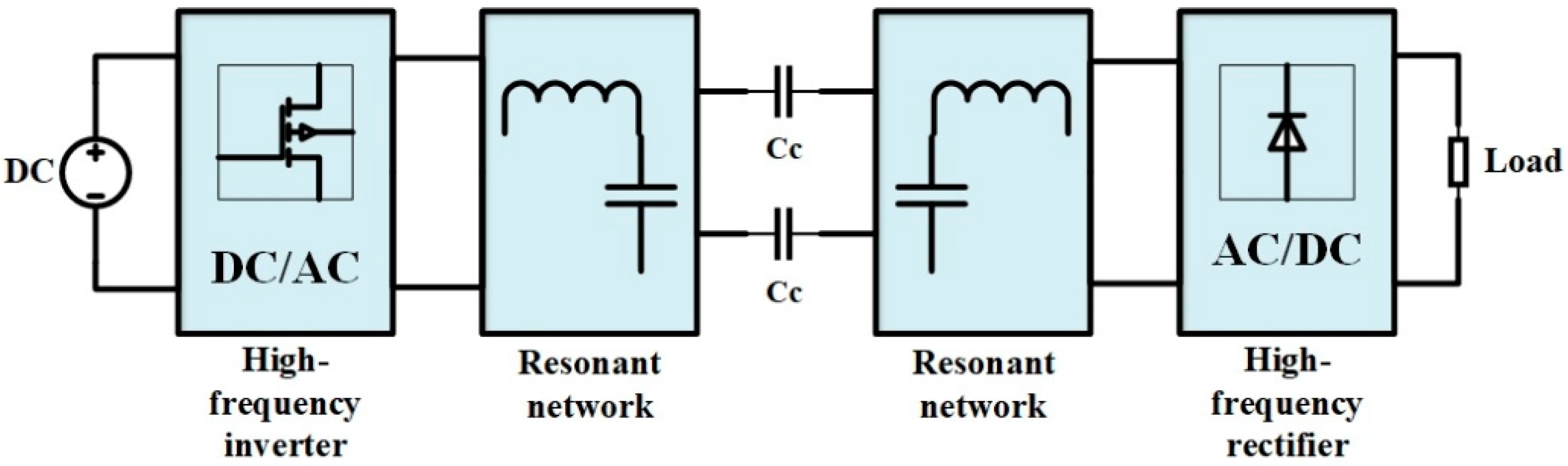
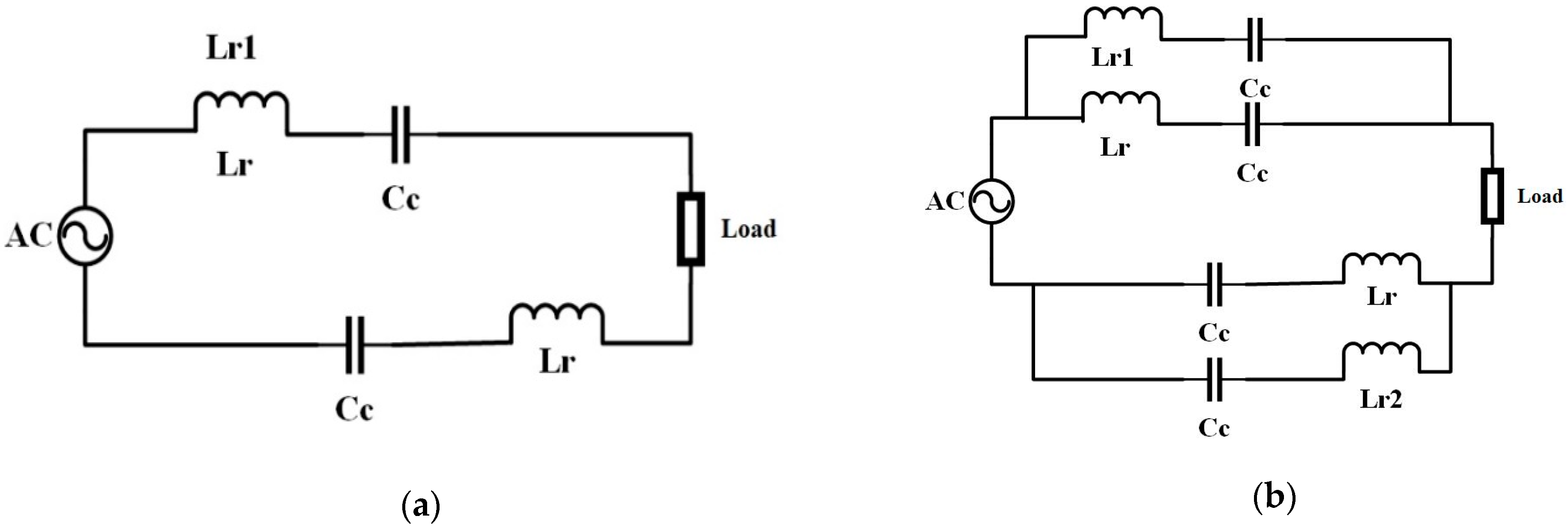
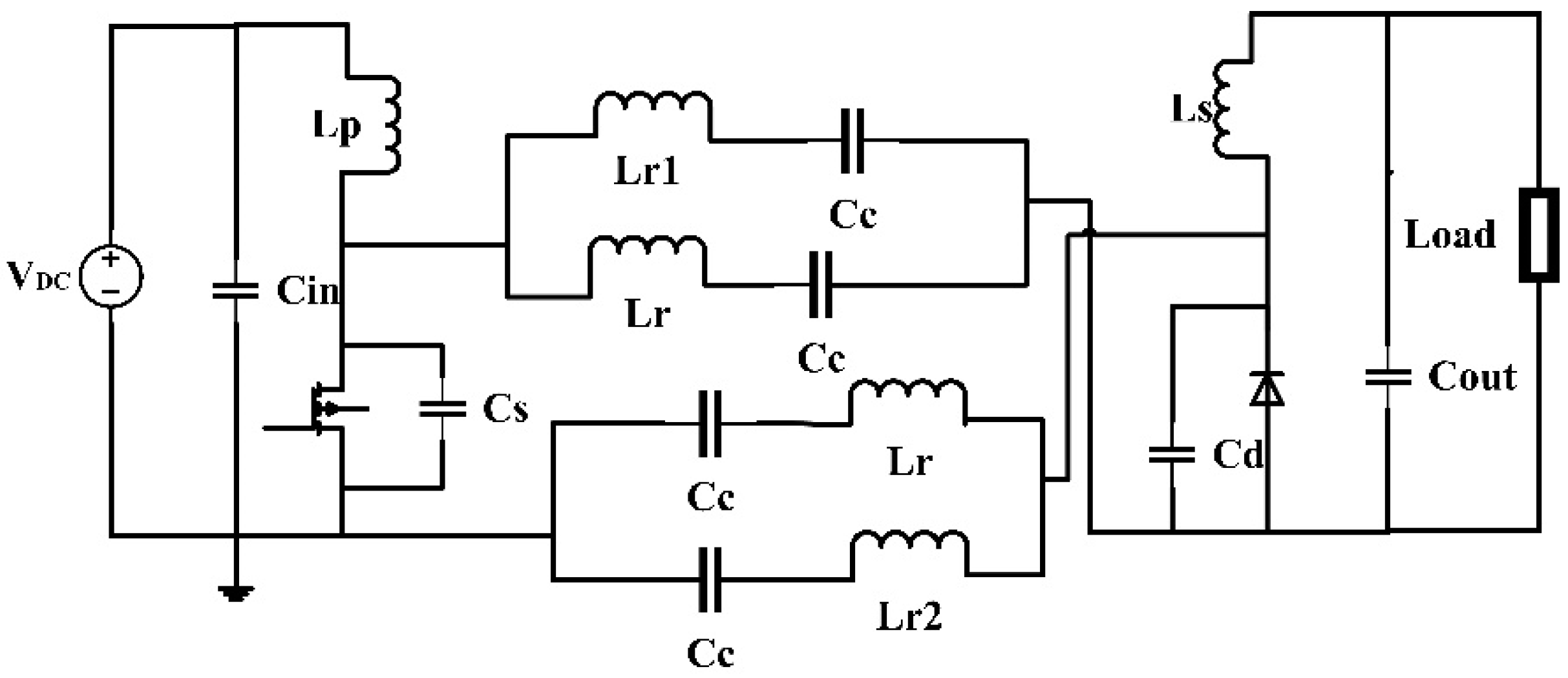
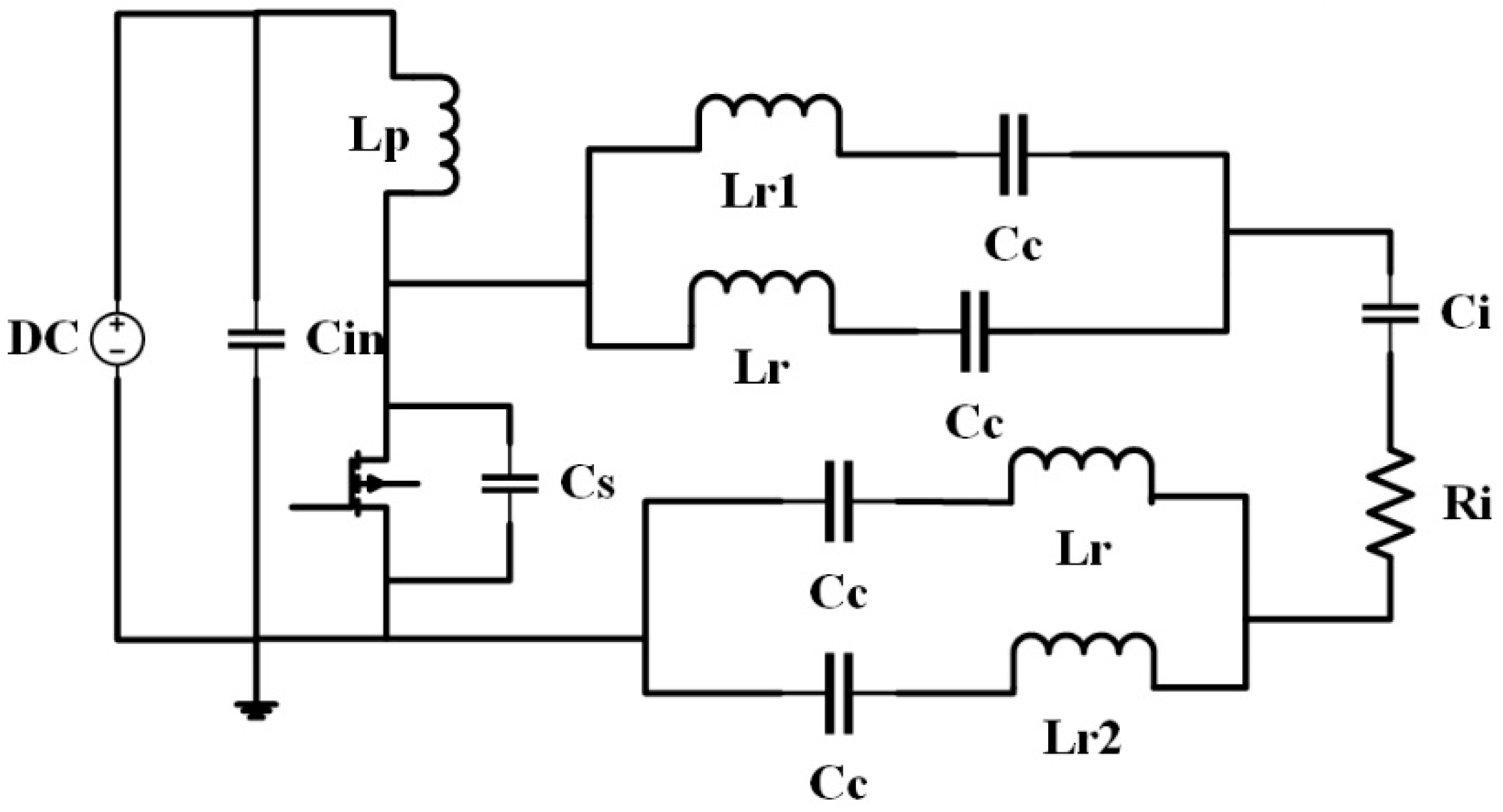
2.1.2. Class-E2 with a 8-Plate Multi-Resonant Coupling Structure
2.2. Impedance Analysis of the 8-Plate Multi-Resonant Coupling Structure
3. Results and Discussions
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- da Silva, G.G.; Petry, C.A. Capacitive Wireless Power Transfer System Applied to Low-Power Mobile Device Charging. Int. J. Electr. Energy 2015, 3, 230–234. [Google Scholar] [CrossRef] [Green Version]
- Regensburger, B.; Afridi, K.K. Challenges and Solutions to Passive Rectification in Multi-MHz Frequency Capacitive Wireless Power Transfer Systems for Electric Vehicle Charging. In Proceedings of the 2020 IEEE Energy Conversion Congress and Exposition, Detroit, MI, USA, 11–15 October 2020; pp. 5482–5486. [Google Scholar] [CrossRef]
- Yang, L.; Zhang, Y.; Li, X.; Jian, J.; Wang, Z.; Huang, J.; Ma, L.; Tong, X. Analysis and Design of Four-Plate Capacitive Wireless Power Transfer System for Undersea Applications. CES Trans. Electr. Mach. Syst. 2021, 5, 202–211. [Google Scholar] [CrossRef]
- Tamura, M.; Murai, K.; Matsumoto, M. Design of Disposable Film-Type Capacitive Wireless Charging for Implantable Medical Devices. In Proceedings of the 2021 IEEE MTT-S International Microwave Symposium (IMS), Atlanta, GA, USA, 7–25 June 2021; pp. 58–61. [Google Scholar] [CrossRef]
- Park, C.; Park, J.; Shin, Y.; Kim, J.; Huh, S.; Park, S.; Ahn, S. Separated Circular Capacitive Coupler for Reducing Cross-Coupling Capacitance in Drone Wireless Power Transfer System. IEEE Trans. Microw. Theory Tech. 2020, 68, 3978–3985. [Google Scholar] [CrossRef]
- Allamehzadeh, H. Wireless Power Transfer (WPT) Fundamentals with Resonant Frequency-Dependent Parameters, Energy Transfer Efficiency, and Green Technology Applications. In Proceedings of the 2021 IEEE 48th Photovoltaic Specialists Conference (PVSC), Fort Lauderdale, FL, USA, 20–25 June 2021; pp. 0036–0040. [Google Scholar] [CrossRef]
- Minnaert, B.; Costanzo, A.; Monti, G.; Mongiardo, M. Capacitive Wireless Power Transfer with Multiple Transmitters: Efficiency Optimization. Energies 2020, 13, 3482. [Google Scholar] [CrossRef]
- Lu, F.; Zhang, H.; Mi, C. A Review on the Recent Development of Capacitive Wireless Power Transfer Technology. Energies 2017, 10, 1752. [Google Scholar] [CrossRef] [Green Version]
- Regensburger, B.; Kumar, A.; Sinha, S.; Doubleday, K.; Pervaiz, S.; Popovic, Z.; Afridi, K. High-Performance Large Air-Gap Capacitive Wireless Power Transfer System for Electric Vehicle Charging. In Proceedings of the 2017 IEEE Transportation Electrification Conference and Expo (ITEC), Chicago, IL, USA, 22–24 June 2017; pp. 638–643. [Google Scholar]
- Vincent, D.; Huynh, P.S.; Patnaik, L.; Williamson, S.S. Prospects of Capacitive Wireless Power Transfer (C-WPT) for Unmanned Aerial Vehicles. In Proceedings of the 2018 IEEE PELS Workshop on Emerging Technologies: Wireless Power Transfer (WoW), Montreal, QC, Canada, 3–7 June 2018; pp. 1–5. [Google Scholar]
- Zhang, H.; Lu, F.; Hofmann, H.; Liu, W.; Mi, C.C. A Four-Plate Compact Capacitive Coupler Design and LCL-Compensated Topology for Capacitive Power Transfer in Electric Vehicle Charging Application. IEEE Trans. Power Electron. 2016, 31, 8541–8551. [Google Scholar]
- Bezawada, Y.; Fu, R.; Zhang, Y. Impacts of Coupling Plates on Single-Switch Capacitive-Coupled WPT Systems. In Proceedings of the 2019 IEEE PELS Workshop on Emerging Technologies: Wireless Power Transfer (WoW), London, UK, 18–21 June 2019; pp. 330–334. [Google Scholar]
- Zhang, H.; Lu, F.; Hofmann, H.; Liu, W.; Mi, C.C. Six-Plate Capacitive Coupler to Reduce Electric Field Emission in Large Air-Gap Capacitive Power Transfer. IEEE Trans. Power Electron. 2017, 33, 665–675. [Google Scholar] [CrossRef]
- Ahmad, S.; Hattori, R.; Muharam, A. Generalized Circuit Model of Shielded Capacitive Power Transfer. Energies 2021, 14, 2826. [Google Scholar] [CrossRef]
- Luo, B.; Long, T.; Guo, L.; Dai, R.; Mai, R.; He, Z. Analysis and Design of Inductive and Capacitive Hybrid Wireless Power Transfer System for Railway Application. IEEE Trans. Ind. Appl. 2020, 56, 3034–3042. [Google Scholar] [CrossRef]
- Vincent, D.; Huynh, P.S.; Azeez, N.A.; Patnaik, L.; Williamson, S.S. Evolution of Hybrid Inductive and Capacitive AC Links for Wireless EV Charging—A Comparative Overview. IEEE Trans. Transp. Electrif. 2019, 5, 1060–1077. [Google Scholar] [CrossRef]
- Vincent, D.; Sang, P.H.; Williamson, S.S. Feasibility Study of Hybrid Inductive and Capacitive Wireless Power Transfer for Future Transportation. In Proceedings of the 2017 IEEE Transportation Electrification Conference and Expo (ITEC), Chicago, IL, USA, 22–24 June 2017; pp. 229–233. [Google Scholar] [CrossRef]
- Sinha, S.; Kumar, A.; Regensburger, B.; Afridi, K.K. Active Variable Reactance Rectifier—A New Approach to Compensating for Coupling Variations in Wireless Power Transfer Systems. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 8, 2022–2040. [Google Scholar] [CrossRef]
- Cipriani, E.; Colantonia, P.; Giannini, F.; Giofre, R. The Switchws Mode Power Amplifiers. Advanced Microwave and Millimeter Wave Technologies; Mukherjee, M., Ed.; IntechOpen: London, UK, 2010; pp. 359–388. [Google Scholar]
- Roshani, S.; Roshani, S. Design of a High Efficiency Class-F Power Amplifier with Large Signal and Small Signal Measurements. Measurement 2020, 149, 106991. [Google Scholar] [CrossRef]
- Bezawada, Y.; Zhang, Y. A Case Study: Influence of Circuit Impedance on the Performance of Class-E2 Resonant Power Converter for Capacitive Wireless Power Transfer. Electronics 2021, 10, 1461. [Google Scholar] [CrossRef]
- Lecluyse, C.; Minnaert, B.; Kleemann, M. A Review of the Current State of Technology of Capacitive Wireless Power Transfer. Energies 2021, 14, 5862. [Google Scholar] [CrossRef]
- Li, H.; Wang, K.; Fang, J.; Tang, Y. Pulse Density Modulated ZVS Full-Bridge Converters for Wireless Power Transfer Systems. IEEE Trans. Power Electron. 2018, 34, 369–377. [Google Scholar] [CrossRef]
- Kumar, A.; Sinha, S.; Sepahvand, A.; Afridi, K.K. Improved Design Optimization for High-Efficiency Matching Networks. IEEE Trans. Power Electron. 2017, 33, 37–50. [Google Scholar] [CrossRef]
- Niu, W.; Gu, W.; Chu, J.; Shen, A. Frequency Splitting Patterns in Wireless Power Relay Transfer. IET Circuits Devices Syst. 2014, 8, 561–567. [Google Scholar] [CrossRef]
- Suarez, C.; Kalmes, M.; Suffeleers, J.; Martinez, W. Frequency Splitting in an LCLC Capacitive Wireless Power Transfer System for Electric Vehicle Charging. In Proceedings of the IECON 2020 The 46th Annual Conference of the IEEE Industrial Electronics Society, Singapore, 18–21 October 2020; pp. 3622–3627. [Google Scholar] [CrossRef]
- Huang, R.; Zhang, B.; Qiu, D.; Zhang, Y. Frequency Splitting Phenomena of Magnetic Resonant Coupling Wireless Power Transfer. IEEE Trans. Magn. 2014, 50, 1–4. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhao, Z. Frequency Splitting Analysis of Two-Coil Resonant Wireless Power Transfer. IEEE Antennas Wirel. Propag. Lett. 2014, 13, 400–402. [Google Scholar] [CrossRef]
- Jennings, R.; Bezawada, Y.; Zhang, Y.; Fu, R. Sensitivity of Conversion Efficiency with Variable Load Assignment in Class-E Resonant Inverter and Its Derivatives. In Proceedings of the 2016 IEEE International Telecommunications Energy Conference (INTELEC), Austin, TX, USA, 23–27 October 2016; pp. 1–7. [Google Scholar] [CrossRef]
- Chokkalingam, B.; Padmanaban, S.; Leonowicz, Z.M. Class E Power Amplifier Design and Optimization for the Capacitive Coupled Wireless Power Transfer System in Biomedical Implants. Energies 2017, 10, 1409. [Google Scholar] [CrossRef]
- Lu, K.; Nguang, S.K.; Ji, S.; Wei, L. Design of Auto Frequency Tuning Capacitive Power Transfer System Based on Class-E 2 dc/dc Converter. IET Power Electron. 2017, 10, 1588–1595. [Google Scholar] [CrossRef]
- Kazimierczuk, M.K.; Czarkowski, D. Resonant Power Converters; John and Wiley and Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
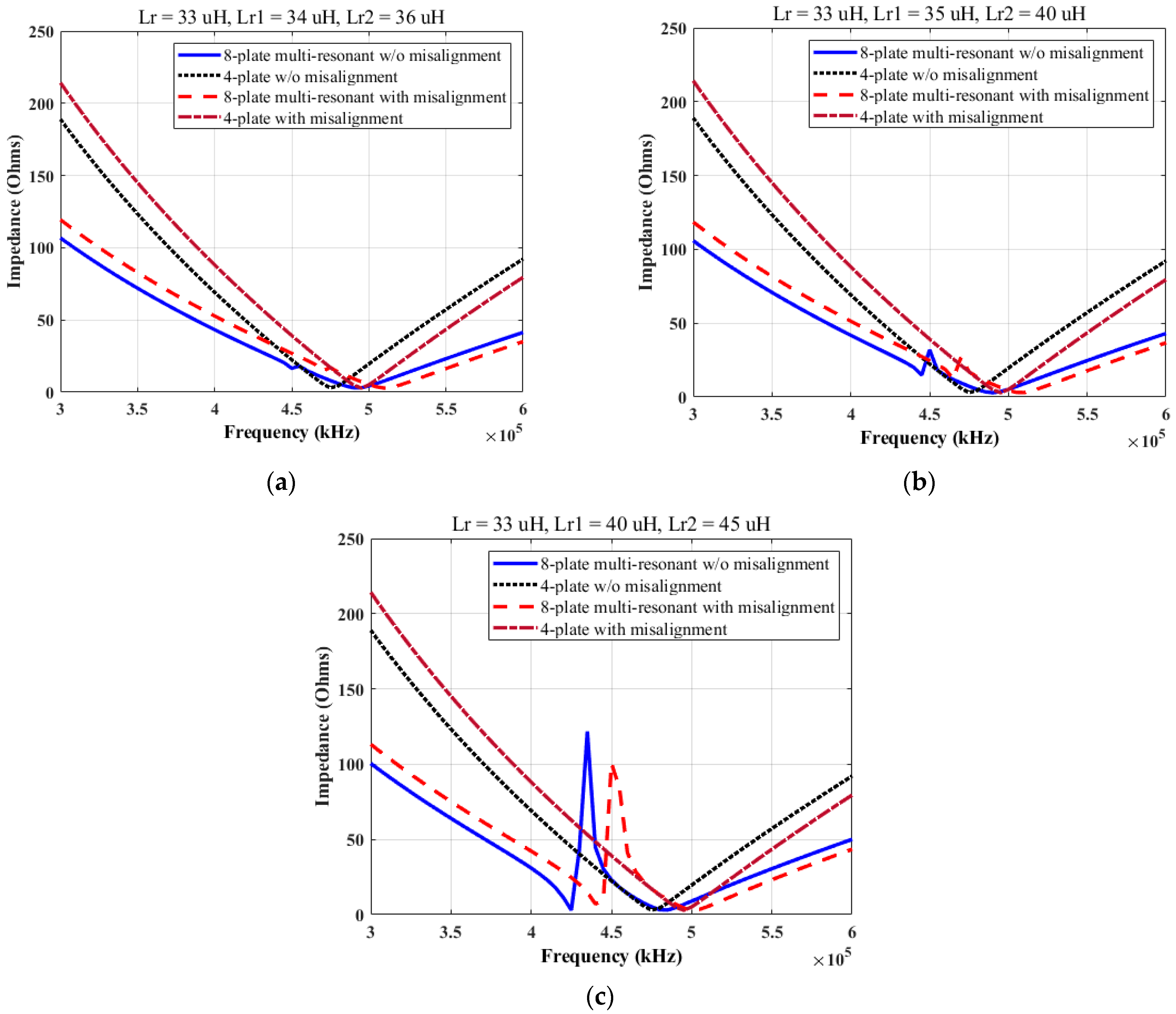





| Case | Lr | Lr1 | Lr2 |
|---|---|---|---|
| a | 33 μH | 34 μH | 36 μH |
| b | 33 μH | 35 μH | 40 μH |
| c | 33 μH | 40 μH | 45 μH |
| Components | Parameters | Values for 4-Plate | Values for 8-Plate |
|---|---|---|---|
| DC link/input capacitor | Cin | 100 nF | 100 nF |
| Choke/primary inductor | Lp | 33 μH | 33 μH |
| Shunt capacitor at MOSFET | Cs | 1 nF | 1 nF |
| Coupling capacitor | Cc | 3.75 nF | 3.75 nF |
| Resonant inductors | Lr | 33 μH | 33 μH |
| Lr1 | 37 μH | ||
| Lr2 | 40 μH | ||
| Shunt capacitor at diode | Cd | 1 nF | 1 nF |
| Secondary inductor | Ls | 33 μH | 33 μH |
| Output capacitor | Cout | 1 μF | 1 μF |
| Load resistor | RL | 2.4 Ω | 2.4 Ω |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bezawada, Y.; Dhali, S.K. 8-Plate Multi-Resonant Coupling Using a Class-E2 Power Converter for Misalignments in Capacitive Wireless Power Transfer. Electronics 2022, 11, 635. https://doi.org/10.3390/electronics11040635
Bezawada Y, Dhali SK. 8-Plate Multi-Resonant Coupling Using a Class-E2 Power Converter for Misalignments in Capacitive Wireless Power Transfer. Electronics. 2022; 11(4):635. https://doi.org/10.3390/electronics11040635
Chicago/Turabian StyleBezawada, Yashwanth, and Shirshak K. Dhali. 2022. "8-Plate Multi-Resonant Coupling Using a Class-E2 Power Converter for Misalignments in Capacitive Wireless Power Transfer" Electronics 11, no. 4: 635. https://doi.org/10.3390/electronics11040635
APA StyleBezawada, Y., & Dhali, S. K. (2022). 8-Plate Multi-Resonant Coupling Using a Class-E2 Power Converter for Misalignments in Capacitive Wireless Power Transfer. Electronics, 11(4), 635. https://doi.org/10.3390/electronics11040635






