On the Influence of Fractional-Order Resonant Capacitors on Zero-Voltage-Switching Quasi-Resonant Converters

Abstract

This paper focuses on the influence of the fractional-order (FO) resonant capacitor on the zero-voltage-switching quasi-resonant converter (ZVS QRC). The FO impedance model of the capacitor is introduced to the circuit model of the ZVS QRC; hence, a piecewise smooth FO model is developed for the converter. Numerical solutions of the converter are obtained by using both the fractional Adams–Bashforth–Moulton (F-ABM) method and Oustaloup’s rational approximation method. In addition, the analytical solution of the converter is obtained by the Grünwald–Letnikov (GL) definition, which reveals the influence of the FO resonant capacitor on the zero-crossing point (ZCP) and resonant state of the converter. An experimental platform was built to verify the results of the theoretical analysis and numerical calculation.

1. Introduction

Soft-switching techniques are widely used in power converters to reduce switching losses and lift system efficiency [1,2]. A variety of soft-switching schemes based on high-frequency resonant impedance networks have been proposed one after the other, such as the zero-voltage-switching (ZVS) and zero-current-switching (ZCS) techniques, to name but a few [3,4,5]. It is generally accepted that the parameter configuration of passive components is of great importance for the design of different types of high-frequency resonant networks that can be used for soft switching [6,7,8].

The resonant converter can achieve ZVS with the assistance of capacitors [9,10]. For this kind of converters that contains the LC resonant tank, capacitors, as a vital part of the LC resonant tank, usually play a pivotal role in the process of realizing resonance. However, there is a common phenomenon of parameter drift in capacitors. For example, in the literature [11,12], one can find that the capacitance and equivalent series resistance (ESR) of the capacitor considerably changes with the temperature, aging, frequency, and component tolerance. Specifically, electrolyte evaporation, oxide layer deterioration for electrolytic capacitors, and the excessive self-healing for metalized polypropylene film (MPPF) capacitors are also the reasons for the parameter drift of the capacitor [13,14]. In most existing works, the parameter drift characteristics of capacitors have been widely studied, and different kinds of integer-order (IO) impedance models have been proposed. The characteristic analysis of resonant converters relies on traditional integer-order lumped-parameter models, and their time domain solutions depend on solving the initial-value problem of IO differential equations. However, with the ever-deepening research, the topology of the models becomes increasingly complex, as those surveyed in the literature [15,16,17]. In addition, IO impedance models usually cannot effectively reflect the correlation between the microscopic characteristics of the electronic components, the parameter drift of the component parameters, and the performance of the converters [18,19]. As a result, the analysis of the errors will be introduced to the design of the resonant converters, thereby affecting the working performance of circuit systems [20,21,22,23].

In recent years, researchers have discovered the presence of fractional-order phenomena in fields such as mechanics, physical engineering, and materials science [24]. Existing studies indicate that fractional-order models can more accurately reflect the physical phenomena of actual systems [25]. Especially in the field of circuits, the fractional-order characteristics of electronic components have been revealed. For example, the literature [26] uncovers the FO characteristic of MOSFETs’ interelectrode capacitance. The literature [27] reveals the FO characteristics of the capacitors by exploring the electrochemical principles of aluminum electrolytic capacitors. The literature [28] investigates a new implementation of a passive FO capacitor based on a single-component FO inductor, which truly reflects the infinite-dimensional nature of the fractional-order impedance characteristic. The memristor is also a nonlinear device with memory characteristics [29]. On the basis of the aforementioned studies, scholars have conducted modeling and analysis research on fractional-order converters [30,31,32].

On the other hand, due to the existence of switching devices, the FO models for DC–DC converters have been established, such as FO Buck, Boost, Buck–Boost, and Flyback converters, which exhibit strong nonlinear characteristics [33,34,35]. These models not only improve modeling accuracy, but also accurately describe the true dynamic behavior of the converters, providing a more precise theoretical basis for optimizing converter control. However, most of the research focuses primarily on the modeling and analysis of fractional-order converters with the lower orders and lacks characteristic analysis from the time domain perspective.

For the ZVS QRC, a kind of circuit system that relies on resonant operating points for circuit design, the influence of the fractional characteristics of the capacitance on circuit performance is awaiting study. Accordingly, the contents and contributions of this paper include the following points:

1.

In order to reveal the influence of factional-order (FO) resonant capacitors on the ZVS QRC, this work develops an FO piecewise-smooth model for the converter, which contains the FO impedance model of the resonant capacitor.

2.

The time domain resonant characteristics of the converter are analyzed by using the proposed FO piecewise-smooth model. Time domain analytical solutions of the converter are calculated by using the Grünwald–Letnikov (GL) definition of fractional calculus. In addition, numerical solutions are also obtained for comparison and validation, where the fractional Adams–Bashforth–Moulton (F-ABM) method and Oustaloup’s rational approximation method are used.

3.

Both circuit-level simulation and an experimental platform are built to verify the theoretical analysis and numerical calculations. Compared with the IO-based approach, the accuracy of the FO-model-based analysis is improved, and some of the resonant performances of the converter, such as the zero-voltage crossing time and voltage ripples, can be revealed in a more accurate way.

The remainder of the paper is organized as follows: Section 2 introduces the relationship between the FO characteristics of the resonant capacitor and the switching modes of the converter through an FO piecewise-smooth model. Section 3 solves the initial-value problem of fractional differential equations, and the the quantitative relationship among resonant conditions, the zero-crossing voltage, and the FO of the resonant capacitor are obtained. In Section 4, both the simulation and experimental results are given for the validation of the theoretical analysis and numerical calculations via the F-ABM method and GL definition. Section 5 gives the conclusion.

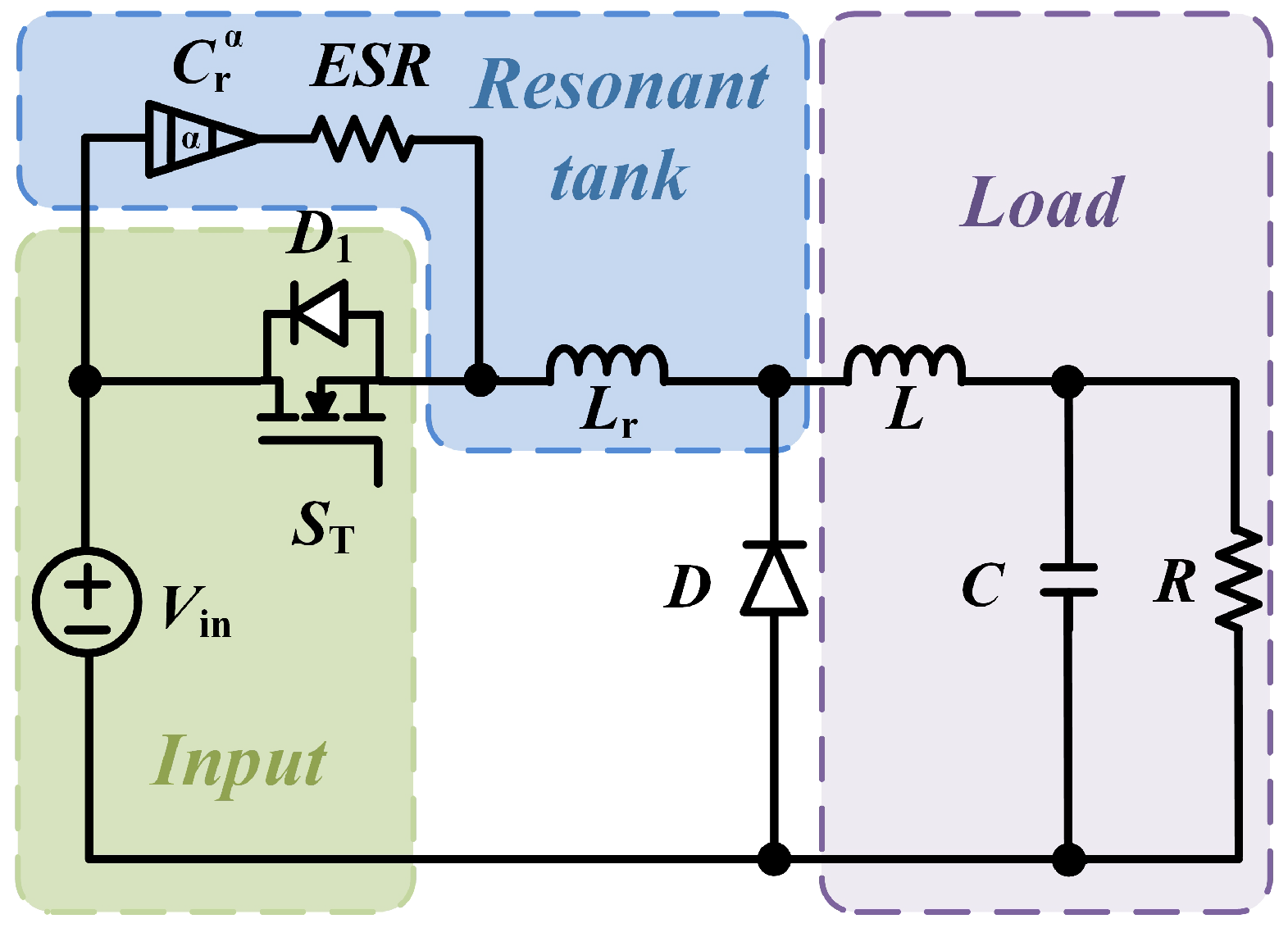
2. Circuit Analysis Framework Based on Fractional-Order Piecewise-Smooth Model

Figure 1 shows a typical ZVS QRC, in which a resonant capacitor $C_r$ is in parallel with the power switch $S_T$ and the power diode $D_1$. Therefore, the charging or discharging state of the resonant capacitor changes with the on or off state of the switch $S_T$, thus affecting the resonant state of the $L_r{C}_r$ resonant unit and the switching mode of the converter. Note that the resonant capacitor $C_r$ is modeled by a simplified fractional-order impedance model, which comprises an ideal fractional-order capacitor $C_r^{\alpha }$ and a series resistance $R_{\Omega }$ [27]. Then, the switching mode and working states of the converter can be deduced according to the KVL law and the KCL law.

2.1. Switching Mode Analysis

The schematic in Figure 1 can provide the adjustment of the resonant frequency by ensuring zero-voltage-switching conditions, i.e., the voltage $u_{S_T}\left(t\right)$ or $u_{Cr}\left(t\right)$ must be zero whenever the switch $S_T$ is closed, as per the waveforms in Figure 2. In one steady-state switching period, the converter experiences six switching modes, the equivalent circuits of which are depicted in Figure 3.

1.

Mode 1 ($t_0-t_1$): In this time period, $S_T$ is turned off, and the resonant capacitor $C_r$ is charged by $L_r$ and L. The voltage $u_{Cr\left(t\right)}$ increases until the voltage $V_D$ become zero and the voltage $u_{Cr\left(t\right)}$ reaches the input voltage $V_{in}$; thus, the converter in this period can be governed by:

$$\left\{\begin{array}{c}{i}_{Lr}\left(t\right)=I\hfill \\ C_1\frac{d^{\alpha }{u}_{c1}}{d{t}^{\alpha }}=i_{Lr}\left(t\right)\hfill \\ i_{Lr}\left(t_0\right)=I,u_{Cr}\left(t_0\right)=0\hfill \end{array}\right.$$

(1)

where $C_1$ is the nominal capacitance of the resonant capacitor $C_r$, $\alpha$ is the fractional order, I is the current of inductor L.

2.

Mode 2 ($t_1-t_2$): In this time period, the resonant capacitor $C_r$ is charged by $L_r$, diode D is in conduction state, and $C_r$, $L_r$, and $V_{in}$ form a resonant unit. While the current $i_{Lr}\left(t\right)$ continues to decrease, the voltage $u_{Cr}\left(t\right)$ continues to rise. At time $t_2$, $u_{Cr}\left(t\right)$ reaches its peak value. Due to the voltage $u_{Cr}\left(t\right)$ rising from the initial value to the peak value of the resonance progress, the duration of this mode is a quarter cycle and is determined by:

$$\Delta t_2=t_2-t_1=\frac{1}{4}·\frac{2\pi }{{\omega }_{rs}}=\frac{\pi }{2}{\left(\frac{sin\frac{\alpha \pi }{2}}{L_r{C}_1}\right)}^{-\frac{1}{1+\alpha }}$$

(2)

in which $L_r$ is the inductance of the resonant inductor $L_r$ and ${\omega }_{rs}$ is the resonant angle frequency.

3.

Mode 3 ($t_2-t_3$): In this time period, the resonant inductor $L_r$ and the resonant capacitor $C_r$ charge and discharge each other. The current $i_{Lr}\left(t\right)$ changes direction. The decrease of the voltage $u_{Cr}\left(t\right)$ leads the voltage $u_{Lr}$ to increase to zero. At time $t_3$, $u_{Cr}\left(t\right)$ equals $V_{in}$ and $i_{Lr}\left(t\right)$ reaches its reverse resonant peak. The duration of this mode is analyzed similarly to mode 2, and it is given by:

$$\Delta t_3=t_3-t_2=\frac{\pi }{2}{\left(\frac{sin\frac{\alpha \pi }{2}}{L_r{C}_1}\right)}^{-\frac{1}{1+\alpha }}$$

(3)

4.

Mode 4 ($t_3-t_4$): The resonant capacitor $C_r$ is reversely charged by the resonant inductor $L_r$, and the voltage $u_{Cr}\left(t\right)$ continues to decrease. When $u_{Cr}\left(t\right)$ decreases to zero, this mode ends.

The converter in the resonant process of $t_1$ to $t_4$ can be governed by:

$$\left\{\begin{array}{c}{L}_r\frac{d{i}_{Lr}}{dt}+u_{C_1}\left(t\right)+u_{R_{\Omega }}\left(t\right)=V_{in}\hfill \\ C_1\frac{d^{\alpha }{u}_{c1}}{d{t}^{\alpha }}=i_{Lr}\left(t\right)\hfill \\ i_{Lr}\left(t_1\right)=I,u_{Cr}\left(t_1\right)=V_{in}\hfill \end{array}\right.$$

(4)

where $V_{in}$ is the input voltage and $u_{R_{\Omega }}$ is the voltage of $R_{\Omega }$.

5.

Mode 5 ($t_4-t_6$): In this time period, the diode $D_1$ conducts and the voltage $u_{Cr}\left(t\right)$ is clamped to zero. The voltage of resonant inductor $u_{Lr}$ is constant and equal to $V_{in}$, and the current $i_{Lr}\left(t\right)$ linearly decays to zero at time $t_5$; the converter in this mode can be described by:

$$\left\{\begin{array}{c}{L}_r\frac{d{i}_{Lr}}{dt}=V_{in}\hfill \\ u_{Cr}=0\hfill \end{array}\right.$$

(5)

6.

Mode 6 ($t_6-t_0$): The switch $S_T$ is turned on. At time $t_6$, resonant inductor current $i_{Lr}\left(t\right)$ equals I, and diode D is turned off.

$$\left\{\begin{array}{c}{i}_{Lr}=I_L\hfill \\ u_{Cr}=0\hfill \end{array}\right.$$

(6)

The variations of the times $\Delta t_2$ and $\Delta t_3$ versus the fractional-order $\alpha$ of the resonant capacitor have a similar trend, which is give in Figure 4:

Based on the above, one can find that the ZVS QRC with a fractional-order resonant capacitor can be governed by piecewise-smooth fractional-order models. In order to reveal it’s resonant characteristics, it is essential to calculate the fractional differential Equation (4), so that the initial-value problem of the fractional-order differential equation arises.

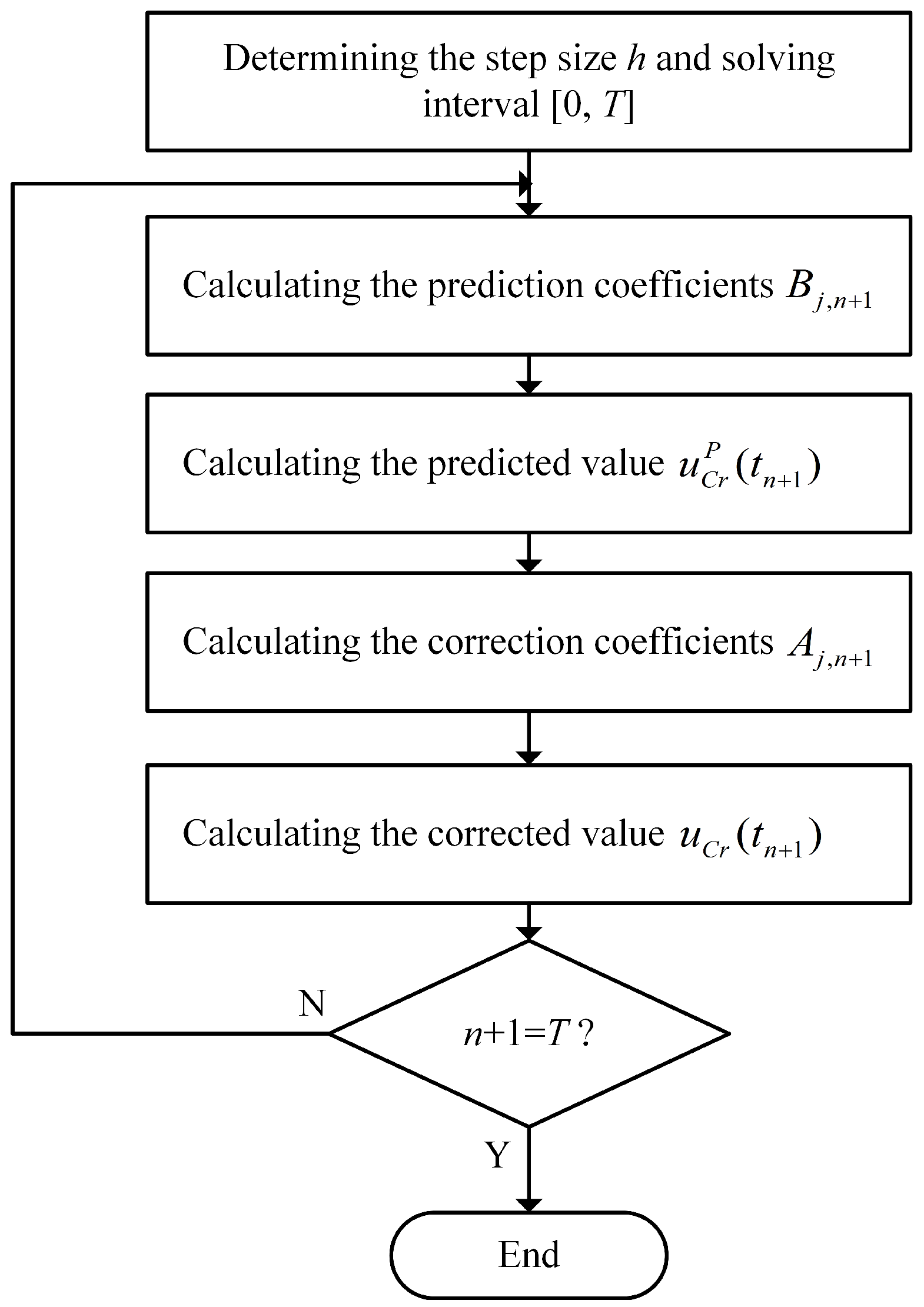
2.2. Computation Approaches

2.2.1. Solutions Obtained by F-ABM Method

For any nonlinear dynamical system with initial values, the fractional-order differential equation describing its dynamics is:

$$\left\{\begin{array}{c}\frac{d^{\alpha }x}{d{t}^{\alpha }}=f(t,x\left(t\right)),0\le t<T\hfill \\ x^{\left(k\right)}\left(0\right)=x_0^{\left(k\right)},k=0,1,\cdots ,n-1\hfill \end{array}\right.$$

(7)

where $\alpha \in (n-1,n)$, $n\in IN$.

The integral equation describing this nonlinear dynamical system is:

$$x\left(t\right)=\sum _{k=0}^{n-1}{x}_0^{\left(k\right)}\frac{t^k}{k!}+\frac{1}{\Gamma \left(\alpha \right)}{\int }_0^t{(t-\tau )}^{\alpha -1}f(\tau ,x\left(\tau \right))d\tau$$

(8)

Based on the above definition of F-ABM, let $h=\frac{T}{N},t_n=nh,n=0,1,\cdots ,N\in Z^{+}$. The fractional differential equations in Equations (1) and (4) can be written in the following discrete form [36]:

$$\begin{array}{c}{u}_{Cr}\left(t_{n+1}\right)=u_{Cr}\left(t_0\right)+\frac{h^{\alpha }}{\Gamma (2+\alpha )}f\left[t_{n+1},u_{Cr}^p\left(t_{n+1}\right)\right]+\frac{h^{\alpha }}{\Gamma (2+\alpha )}{\displaystyle \sum _{j=0}^n}{A}_{j,n+1}f\left[t_j,u_{Cr}^p\left(t_j\right)\right]\hfill \end{array}$$

(9)

where n is any integer, and the correction coefficient $A_{j,n+1}$ is

$$A_{j,n+1}=\left\{\begin{array}{c}{n}^{\alpha +1}-(n-\alpha ){(n+1)}^{\alpha },j=0\hfill \\ {(n-j+2)}^{\alpha +1}+{(n-j)}^{\alpha +1}-2{(n-j+1)}^{\alpha +1},1\le j\le n\hfill \\ 1,j=n+1\hfill \end{array}\right.$$

(10)

The predicted value $u_{Cr}^p\left(t_{n+1}\right)$ in Equation (9) is

$$u_{Cr}^p\left(t_{n+1}\right)=u_{Cr}\left(t_0\right)+\frac{1}{\Gamma \left(\alpha \right)}\sum _{j=0}^n{B}_{j,n+1}f[t_j,u_{Cr}\left(t_j\right)]$$

(11)

in which the prediction coefficient $B_{j,n+1}$ is

$$B_{j,n+1}=\frac{h^{\alpha }}{\alpha }\left[{(n+1-j)}^{\alpha }-{(n-j)}^{\alpha }\right]$$

(12)

The flow chart of the F-ABM method is shown in Figure 5:

2.2.2. Solutions Obtained by Oustaloup’s Rational Approximation Method

The ideal fractional-order derivation and differential can be approximated by a pre-designed rational fraction [37]:

$$Z\left(s\right)=K{s}^{\alpha }\approx K\underset{x\to \infty }{lim}\frac{{\displaystyle \prod _{i=0}^{N-1}}(s+z_i)}{{\displaystyle \prod _{i=0}^N}(s+p_i)}=Z_1\left(s\right)$$

(13)

where K is the pre-designed gain parameter and the number N of pole-zero doublets is determined by the pre-determined amplitude–frequency characteristic error and the pre-designed frequency range:

$$N=Integer(\frac{log(\frac{{\omega }_{max}}{p_0})}{log(ab)})+1$$

(14)

in which $a={10}^{[y/10(1-\alpha \left)\right]}$, $b={10}^{[y/10\alpha ]}$, $p_0=p_T{10}^{[y/20\alpha ]}$, $p_T=3\alpha$, ${\omega }_{max}=100$ MHz, and y is the pre-determined error.

The expression for the zeros and poles can be obtained through inductive recursion relationships:

$$\left\{\begin{array}{c}{p}_i={\left(ab\right)}^i{p}_0\hfill \\ z_i={\left(ab\right)}^{i}a{p}_0\hfill \end{array}\right.$$

(15)

The rational fraction in Equation (13) is partially expanded and transformed into the following transfer function:

$$Z_1\left(s\right)=\sum _{i=0}^N\frac{K_i}{s+{\sigma }_i}$$

(16)

The parameter $K_i$ and ${\sigma }_i$ can be obtained by the transform-obtained zero-pole representation in a rational fraction form using the $zp2tf$ and $residue$ functions. The meaning of the first function $zp2tf$ is transforming the parameters of the zero-pole filter into the form of the transfer function, and the meaning of the second function $residue$ is the partial fraction expansion. The values of $R_i$ and $C_i$ in the impedance approximation circuit are calculated by using the following formula:

$$\left\{\begin{array}{c}{R}_i=\frac{K_i}{{\sigma }_i}\hfill \\ C_i=\frac{1}{K_i}\hfill \end{array}\right.$$

(17)

A simplified fractional-order capacitor model is introduced in the ZVS QRC. $C_{eq}=\frac{{\omega }^{\alpha -1}C}{sin\frac{\alpha \pi }{2}}$, and $ESR=R_{\Omega }+\frac{cos\frac{\alpha \pi }{2}}{{\omega }^{\alpha }C}$. The capacitance value, ESR, and impedance modulus of the fractional-order resonant capacitor are measured by a high-precision LCR meter in Figure 6, and the fractional-order of the capacitor is predicted by the method in the previous work [27], which can be verified by the impedance–frequency curve and resistance–frequency curve shown in Figure 7. By data fitting, we found that the fractional-order $\alpha$ of this resonant capacitor is around 0.987.

The above results indicate that the CBB film capacitor has fractional-order characteristics. However, there are no such components in existing simulation software. Therefore, we used Oustaloup’s approximation method to build such a fractional-order capacitor in the simulation for comparison, the structure of which is shown in Figure 8. An ideal fractional-order capacitor can be obtained by connecting the resistor and the capacitor in parallel as a unit in series, which is verified by the amplitude frequency characteristic and the phase frequency characteristic in Figure 9. Obviously, the frequency range for this approximation is from ${10}^2$ Hz to ${10}^5$ Hz and the pre-determined error y is less than 3 dB. The resistors $R_1$ and $R_2$ in

Table 1. The fractional-order capacitor parameters.

Parameters	FO Capacitor
Capacitance C	0.5 μF
Order $\alpha$	0.987
Resistor $R_1$	$2.34\times {10}^{-8}\Omega$
Resistor $R_2$	$2\times {10}^8\Omega$
Capacitor $C_1$	0.667 μF
Capacitor $C_2$	0.396 μF

are the components involved in the capacitor and resistor parallel units in the method.

3. Resonant State Analysis

3.1. Analytical Solutions Obtained by GL Definition

The GL definition method provides a theoretical solution to the resonance equation system, and it serves as a reference in comparison with the F-ABM and Oustaloup numerical methods mentioned above. The GL definition of the FO $\alpha$ derivative of function $f\left(t\right)$ is obtained [38]:

$$\begin{array}{c}{}_{t_0}{}^{\mathit{GL}}D_t^{\alpha }f\left(t\right)=\underset{h\to 0}{lim}\frac{1}{h^{\alpha }}{\displaystyle \sum _{j=0}^{\left[(t-t_0/h)\right]}}{(-1)}^j\left(\begin{array}{c}\alpha \hfill \\ j\hfill \end{array}\right)f(t-jh)\approx \frac{1}{h^{\alpha }}{\displaystyle \sum _{j=0}^{\left[(t-t_0/h)\right]}}{\omega }_j^{\left(\alpha \right)}f(t-jh)\hfill \end{array}$$

(18)

where ${}_{t_0}{}^{\mathit{GL}}D_t^{\alpha }f\left(t\right)$ is the fractional-order calculus operator, t is an independent variable, h is the step size, the superscript GL represents to the Grünwald–Letnikov definition, and ${\omega }_j^{\left(\alpha \right)}={(-1)}^j\left(\begin{array}{c}\alpha \hfill \\ j\hfill \end{array}\right)$ is the binomial coefficient, which can be obtained by the recurrence formula:

$${\omega }_0^{\left(\alpha \right)}=1,{\omega }_j^{\left(\alpha \right)}=(1-\frac{\alpha +1}{j}){\omega }_{j-1}^{\left(\alpha \right)},j=1,2\cdots$$

(19)

By the GL definition, the time domain expressions of Equation (4) are transformed into:

$$L_r{C_1}_{t_1}^{GL}{D}_{u_{c1}}^{\alpha +1}{u}_{c1}\left(t\right)+R_{\Omega }{C_1}_{t_1}^{GL}{D}_{u_{c1}}^{\alpha }{u}_{c1}\left(t\right){+}_{t_1}^{GL}{D}_{u_{c1}}^0{u}_{c1}\left(t\right)=V_{in}$$

(20)

in which the fractional-order $\alpha$ equals 0.987, $L_r$ is the inductance of the resonant inductor, $C_1$ is the capacitance of the resonant capacitor, and $R_{\Omega }$ is the resistance of the resonant capacitor shown in Figure 1. $t_1$ is the starting point of the resonant progress shown in Figure 2.

By solving Equations (18) and (20), the voltage $u_{c1}\left(t\right)$ and current $i_{Lr}\left(t\right)$ of the resonance process ($t_1-t_4$) are obtained:

$$\left\{\begin{array}{c}{u}_{c1}\left(t\right)=V_{in}+R_{\Omega }{C}_1\underset{h\to 0}{lim}\frac{1}{h^{\alpha }}{\displaystyle \sum _{j=0}^{\left[(t-t_0/h)\right]}}{\omega }_j^{\left(\alpha \right)}{u}_{c1}(t-jh)+L_r{C}_1\underset{h\to 0}{lim}\frac{1}{h^{\alpha +1}}{\displaystyle \sum _{j=0}^{\left[(t-t_0/h)\right]}}{\omega }_j^{(\alpha +1)}{u}_{c1}(t-jh)\hfill \\ i_{Lr}\left(t\right)=C_1\underset{h\to 0}{lim}\frac{1}{h^{\alpha }}{\displaystyle \sum _{j=0}^{\left[(t-t_0/h)\right]}}{\omega }_j^{\left(\alpha \right)}{u}_{c1}(t-jh)\hfill \end{array}\right.$$

(21)

Let Equation (21) equal zero; one can obtain the analytical expressions of the ZCP. In addition, one can obtain the analytical expressions of the peak and valley values of the state variables. It can be seen that the order of the resonant capacitance is explicitly included in these equations. Therefore, the above analytical expressions can be directly used to analyze the influence of fractional order on the resonant state of the ZVS QRC.

The results of the numerical solution for the ZVS QRC based on the F-ABM method, Oustaloup’s method, and the GL definition method are obtained as shown in Figure 10. It can be observed that the waveforms obtained by the three approaches coincide with each other. Therefore, the results of the numerical calculation and simulation by these three methods can be used as a reference for the theoretical analysis.

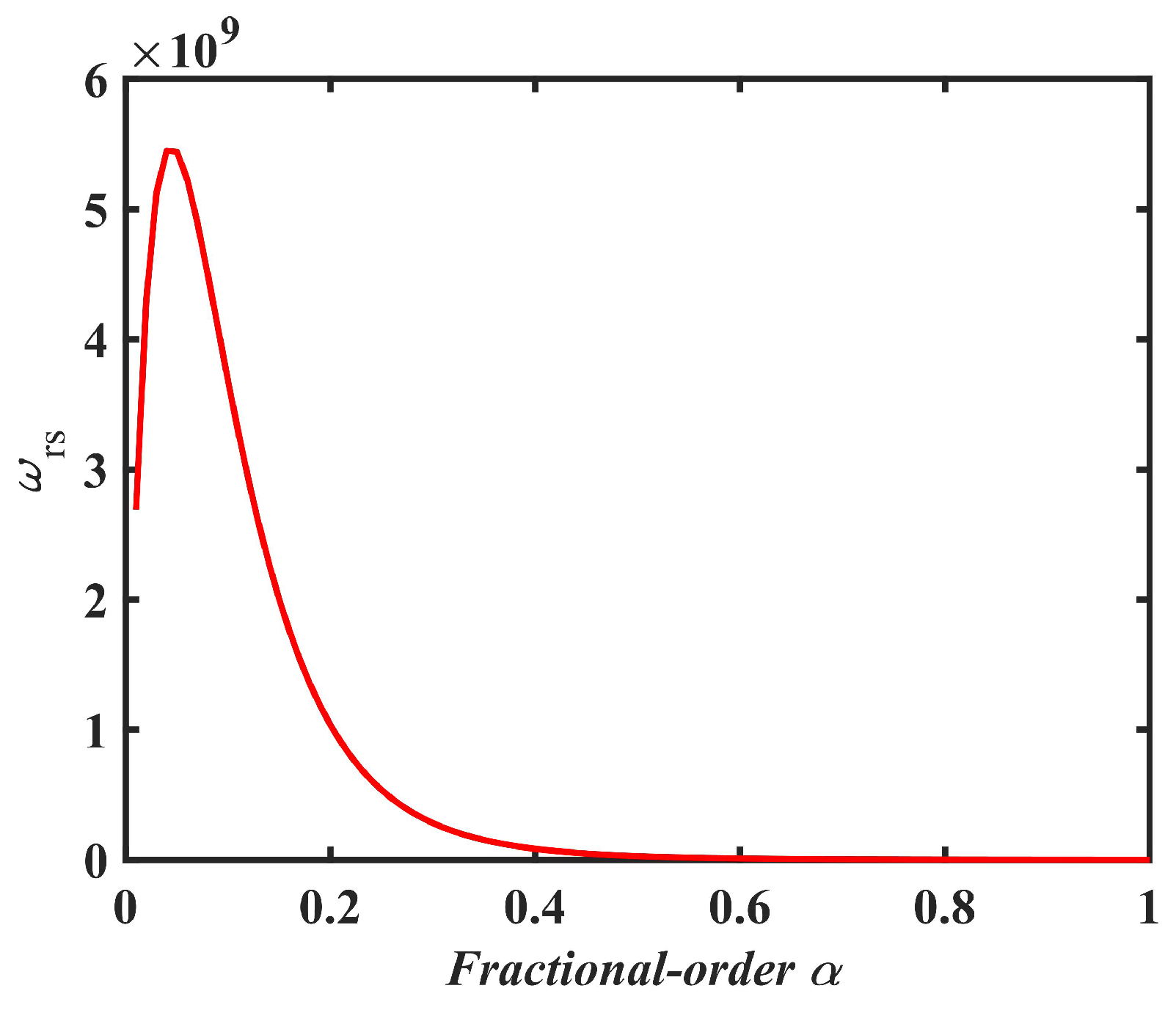
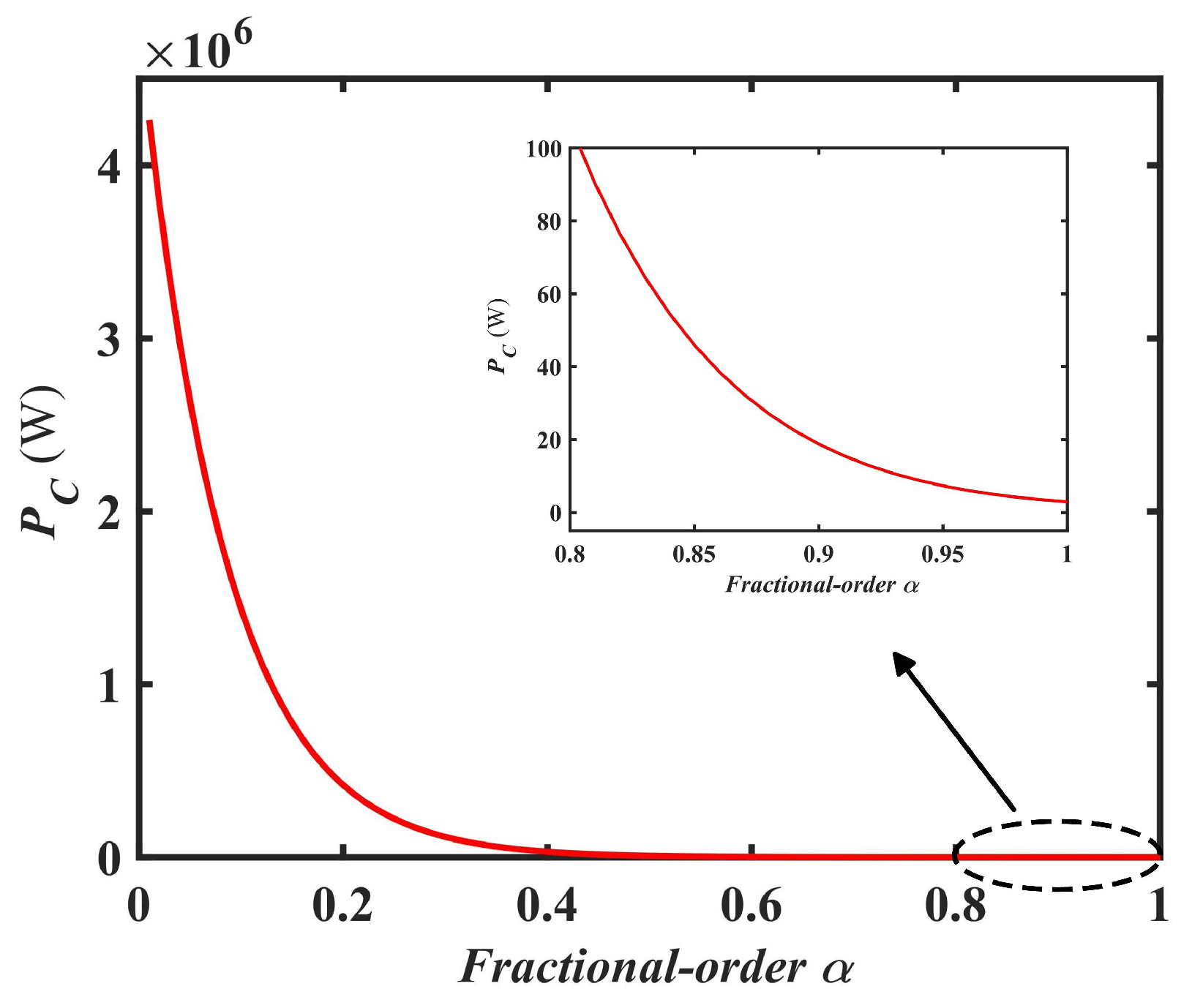
3.2. Influence of the Fractional-Order Resonant Capacitor

According to the above theoretical analysis, the state variables of the ZVS QRC with different orders of the resonant capacitor can be obtained, as shown in Figure 11.

To more specifically show the data changes in Figure 11, a summary of the ZCP and the peak resonant voltage change with the order $\alpha$ is given in

Table 2. Peak value and ZCP at different orders $\alpha$.

Order $\mathit{\alpha }$	Peak Value	ZCP
1	15.72 V	12.78 μs
0.975	16.83 V	9.67 μs
0.95	19.02 V	7.78 μs
0.925	22.48 V	5.34 μs
0.9	25.53 V	4.22 μs

. It can be seen that, with the decrease of the order $\alpha$, the peak resonant voltage is obviously increased, and the ZCP shows a shortening trend.

The resonant progress of ZVS QRC is shown in Figure 3b,c, and its resonant unit is a fractional-order RLC series circuit as given in Figure 12. The impedance Z of this fractional-order RLC series circuit is given by:

$$Z=R_{\Omega }+j\omega L_r+\frac{1}{{\left(j\omega \right)}^{\alpha }{C}_1}$$

(22)

By Euler’s formulation, the impedance Z of the fractional-order RLC series circuit can be rewritten as:

$$Z=R_{\Omega }+\frac{cos\frac{\alpha \pi }{2}}{{\omega }^{\alpha }{C}_1}+j(\omega L_r-\frac{sin\frac{\alpha \pi }{2}}{{\omega }^{\alpha }{C}_1})$$

(23)

Let the imaginary part in Equation (23) equal zero; the resonant angle frequency ${\omega }_{rs}$ is described by:

$${\omega }_{rs}=\sqrt[1+\alpha ]{\frac{sin\frac{\alpha \pi }{2}}{L_r{C}_1}}$$

(24)

In can be seen in Equation (24) that the resonant angle frequency of the converter is highly dependent on the values of its resonant elements. Tolerances in these components can lead to variations in the actual resonant frequency from its designed value. This shift can reduce the efficiency of the energy transfer within the circuit. The influence on resonant angle frequency ${\omega }_{rs}$ of different FO αs is shown in Figure 13. It can be seen that, with the increase of $\alpha$, the resonant angle frequency ${\omega }_{rs}$ increases first and then decreases; when $\alpha$ equals 0.05, it reaches the peak value.

The quality factor (Q-factor) of a resonant circuit, which indicates its bandwidth and energy loss characteristics, can be affected by the tolerances of the resonant elements. A higher tolerance can lead to a lower Q-factor, implying greater energy dissipation and a broader resonance peak, potentially affecting the circuit’s selectivity and performance. In this manuscript, the Q-factor of a ZVS-QRC with an order-$\alpha$ resonant capacitor is:

$$Q=\frac{\left|\mathrm{Im}\left\{Z_{RLC}\left({\omega }_{rs}\right)\right\}\right|}{\mathrm{Re}\left\{Z_{RLC}\left({\omega }_{rs}\right)\right\}}=\frac{{\omega }_{rs}^{\alpha +1}{L}_r{C}_1-sin\frac{\alpha \pi }{2}}{{\omega }_{rs}^{\alpha +1}{R}_{\Omega }{C}_1+cos\frac{\alpha \pi }{2}}$$

(25)

The power losses caused by the resonant capacitor are usually determined by its ESR [39], that is:

$$P_{loss}=ESR·i_{cap,RMS}^2$$

(26)

where $i_{cap,RMS}^2$ is the RMS value of the current through the resonant capacitor and ESR is the equivalent series resistance of the resonant capacitor. According to the FO equivalent impedance model of the resonant capacitor, the value of the ESR is:

$$ESR=R_{\Omega }+\frac{cos\frac{\alpha \pi }{2}}{{\omega }^{\alpha }{C}_1}$$

(27)

It can be seen that the ESR of the FO capacitor model not only includes the IO capacitor model $R_{\Omega }$, but is also related to the order $\alpha$. By changing the order $\alpha$, one can obtain the variation trend of the power losses of resonant capacitor $P_C$ with the order $\alpha$, as depicted in Figure 14. This validates the fractional-order characteristics present in actual capacitors and reveals the limitations of IO capacitor models.

4. Validation and Discussion

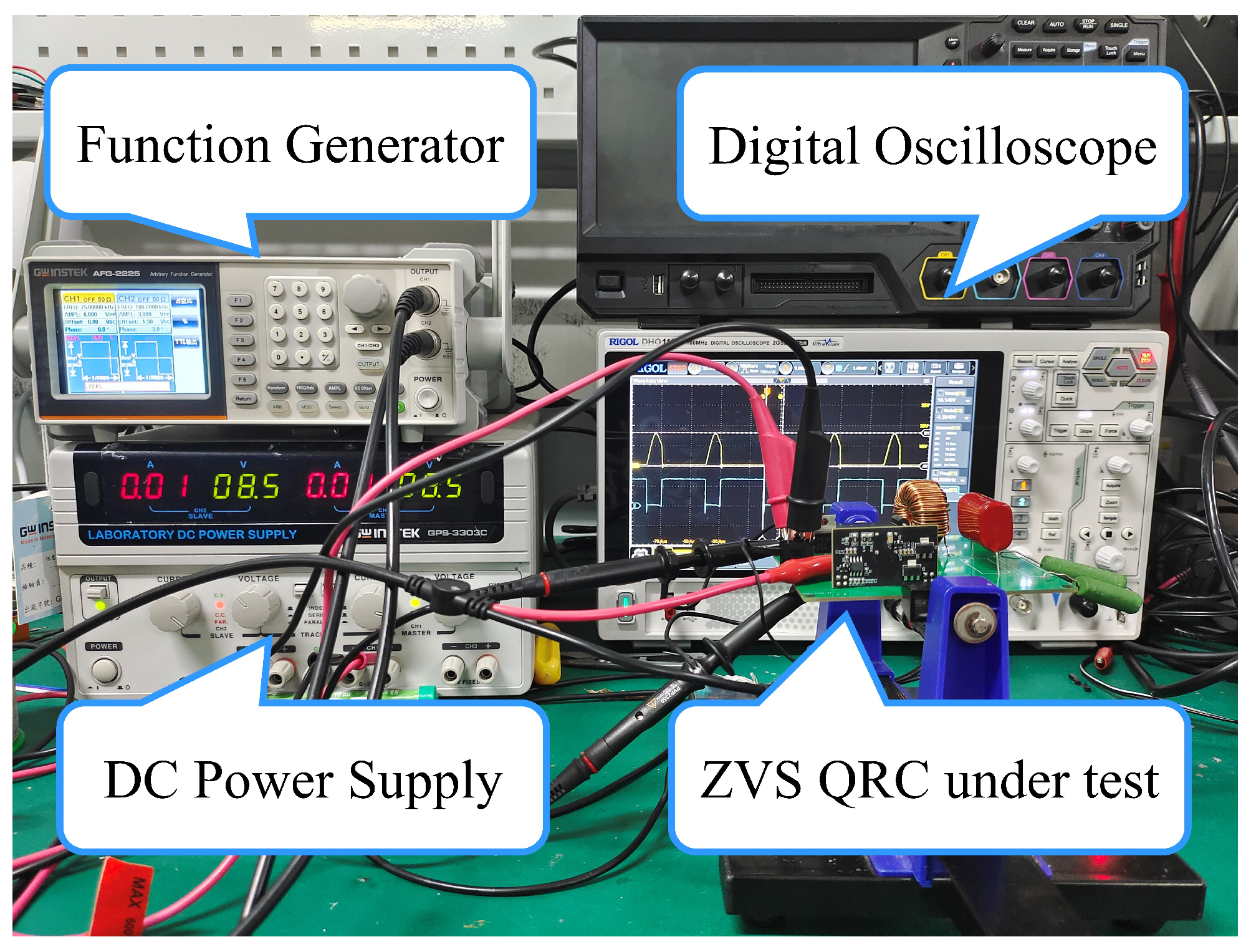
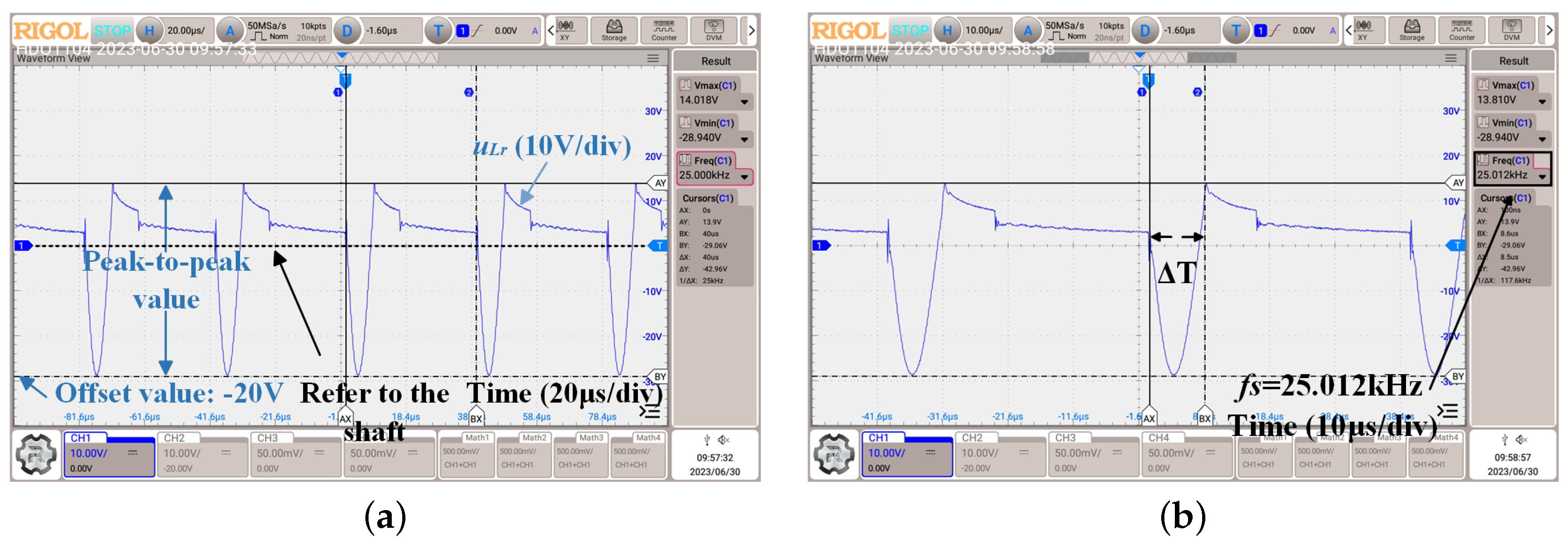
4.1. Experiment Configuration

Experiments were carried out to validate the theoretical analysis and calculation results. The experiment configurations are depicted in

Table 3. Parameter configuration in the experiments.

Device	Parameter	Device	Parameter
Input voltage $V_{in}$	8.5 V	Power MOSFET	K0203
Duty cycle D	0.79	Resonant capacitor (KYET) $C_r$	0.5 μF
Resonant inductor (Sunltech) $L_r$	9.4 μH	Capacitor (KYET) C	1 μF
Inductor L	599 μH	Frequency $f_s$	25 kHz
Load (RESI) R	3 $\Omega$	Power diode D	IN5822
Peak-to-peak value of signal voltage $V_{pp}$	8 V	Offset value of signal voltage	0

, and a glimpse of the test scene is depicted in Figure 15, while the design process of the parameters was as follows:

Step 1: According to the simple parameter expression obtained in the ZVS QRC model with an ideal resonant capacitor, the approximate range of $L_r$, $C_r$, L, C, R, D, $S_T$, and $V_{in}$ can be determined.

Step 2: The C, R, Z, and $\theta$ of the capacitor are collected by the LCR meter in Figure 6 from low frequency to high frequency, then the parameters $\alpha$ of the simplified FO capacitor are identified by the differential evolution method.

Step 3: Construct an FO capacitor with order $\alpha$ by Oustaloup’s rational approximation method as shown in Figure 8.

Step 4: Verify the accuracy and effectiveness of the design methodology presented in the PSIM simulation and experiments.

4.2. Practical Implications

This work actually constructs a bridge between the microscopic characteristics of capacitors, the variation law of capacitor parameters, and the working characteristics of resonant converters by introducing the method of fractional calculus. The findings of this work have the following possible practical implications:

1.

Power conversion efficiency: By using FO-based modeling and analysis approaches, more accurate analyzing results can be obtained, thus benefiting the parameter design and component selection of circuit systems that contain components with fractional-order characteristics. From the research in this article, it can be seen that the working state of ZVS QRCs is closely related to the FO characteristics of the resonant capacitor. Accordingly, the authors intend to analyze the power conversion efficiency of ZVS QRCs and its relationship with the FO characteristics of resonant capacitors in future works.

2.

Controller design and optimization: The FO characteristics of resonant capacitors of course have effects on the frequency domain characteristics of circuit systems. Therefore, when designing controllers for such converters in the future, it is also necessary to study the relationship between the FO characteristics of resonant capacitors and the frequency domain characteristics of ZVS QRCs. The FO piecewise-smooth model established in this work can be further transformed into the s domain by the Laplace transform for research in the aforementioned fields.

Therefore, it is necessary to promote the application of FO-related methods in a vast variety of practical applications, such as electric vehicle applications, where various electrochemical components are used and FO characteristics are commonly present. But, the main challenges are as follows:

1.

The universality of FO parameter characteristics: Actual electronic devices’ parameters are often influenced by environmental conditions, manufacturing variances, and operational circumstances. This work only tested a batch of CBB film capacitors under constant temperature and working conditions. It remains to be studied whether CBB capacitors manufactured by other manufacturers still have the same FO parameter characteristics under more complex working conditions.

2.

Computational demands: One can find that both the analyzed solutions and numerical solutions of an FO converter are in complicated iterative forms. Therefore, by using FO models in design and analysis, the computational cost will be increased to some extent.

To overcome these challenges, we plan to conduct more extensive testing scenarios in future works, testing CBB capacitors from different manufacturers, verifying whether they have a unified parameter variation pattern, and establishing a universal model.

4.3. Performance Analysis and Comparison

The experiment waveforms are give in Figure 16 and Figure 17. One can find that the experiment waveforms of the ZVS QRC are similar to the simulation results with fractional-order resonant capacitors.

It can be seen from Figure 16 and Figure 17 that the theoretical calculation and simulation results shown in Figure 10 coincide with the experimental waveforms. In Figure 17, the blue solid lines are the voltage $u_{Cr}$ of the resonant capacitor $C_r$ and the red solid lines show the MOSFET driving signal $v_{gs}$. After conducting a thorough analysis and evaluation based on several key performance indicators (KPIs) of the resonant converter, such as the zero-crossing point (ZCP) and peak voltage (PV), we have determined that Oustaloup’s method offers better performance and optimization for the specific application and conditions outlined in our study. In order to explore the influence of the FO resonant capacitors,

Table 4. Comparisons of PV between FO, IO values, and experiments.

	PV (V)	Relative Errors
IO model in PSIM	15.70	5.99%
Oustaloup’s ($\alpha =0.987$)	17.01	1.86%
F-ABM ($\alpha =0.987$)	16.45	1.50%
GL definition ($\alpha =0.987$)	15.71	5.93%
Oustaloup’s ($\alpha =1$)	15.72	5.87%
F-ABM ($\alpha =1$)	15.87	4.97%
GL definition ($\alpha =1$)	15.88	4.91%
Experiments	16.70	∖

and

Table 5. Comparisons of ZCP between FO, IO values, and experiments.

	Time (μs)	$\Delta {\mathit{T}}_1$	$\Delta {\mathit{T}}_2$	$\Delta {\mathit{T}}_3$	$\Delta {\mathit{T}}_4$	ZCP	Relative Errors
Method
IO model		2.58	3.40	3.40	3.30	12.67	16.77%
Oustaloup’s ($\alpha =0.987$)		1.92	3.02	3.20	2.86	10.98	1.20%
F-ABM ($\alpha =0.987$)		2.12	3.23	3.44	2.97	11.76	8.39%
GL definition ($\alpha =0.987$)		2.16	3.19	3.22	2.99	11.56	7.00%
Oustaloup’s ($\alpha =1$)		2.52	3.40	3.50	3.35	12.77	17.70%
F-ABM ($\alpha =1$)		2.17	3.25	3.46	2.99	11.87	9.40%
GL definition ($\alpha =1$)		2.16	3.19	3.23	3.00	11.58	7.19%
Experiments		2.04	3.10	3.13	2.58	10.85	∖

list the peak resonant voltage and ZCP obtained by different methods for comparison and verification for an FO $\alpha$ equal to 0.987 and $\alpha$ equal to 1. One can find that Oustaloup’s method represents the PV and ZCP of the resonant capacitor error generally within 2% and the F-ABM method represents the PV of the resonant capacitor error generally within 2%, while the FO model in calculating the ZCP error is within 9%, which is less than the error of the ideal IO capacitor model including parasitic parameters. It is proven that the FO has a better fitting effect than the ideal IO capacitor model.

5. Conclusions

The FO characteristics of capacitors have been widely confirmed in existing works. In order to reveal the influence of factional-order resonant capacitors on ZVS QRCs, this work develops an FO piecewise-smooth model for the converter, which contains the FO impedance model of the resonant capacitor. To analyze the resonant characteristics of the converter, numerical solutions based on the F-ABM method and Oustaloup’s rational approximation method were calculated, and analytical solutions were also deduced based on the GL definition. With the decrease of the order $\alpha$, the peak resonant voltage obviously increased, and the ZCP showed a shortening trend. The experimental data showed that the F-ABM method is more accurate in describing the PV of a resonant capacitor, Oustaloup’s method has more advantages in calculating the ZCP and PV, and the FO capacitor model can reflect the real working conditions of the capacitor more exactly, which further confirms the fractional-order characteristics of the capacitor. Compared with the IO-based approach, the accuracy of the time domain analysis can be improved, and some microscopic phenomena of resonant converters can be better explained by using the modeling and calculation methods based on the fractional calculus. However, the fractional calculus-related theory and computing methods are still developing, and the universality and ease of use of methods still need to be widely verified.