A 10 µH Inductance Standard in PCB Technology with Enhanced Protection against Magnetic Fields
Abstract
:1. Introduction
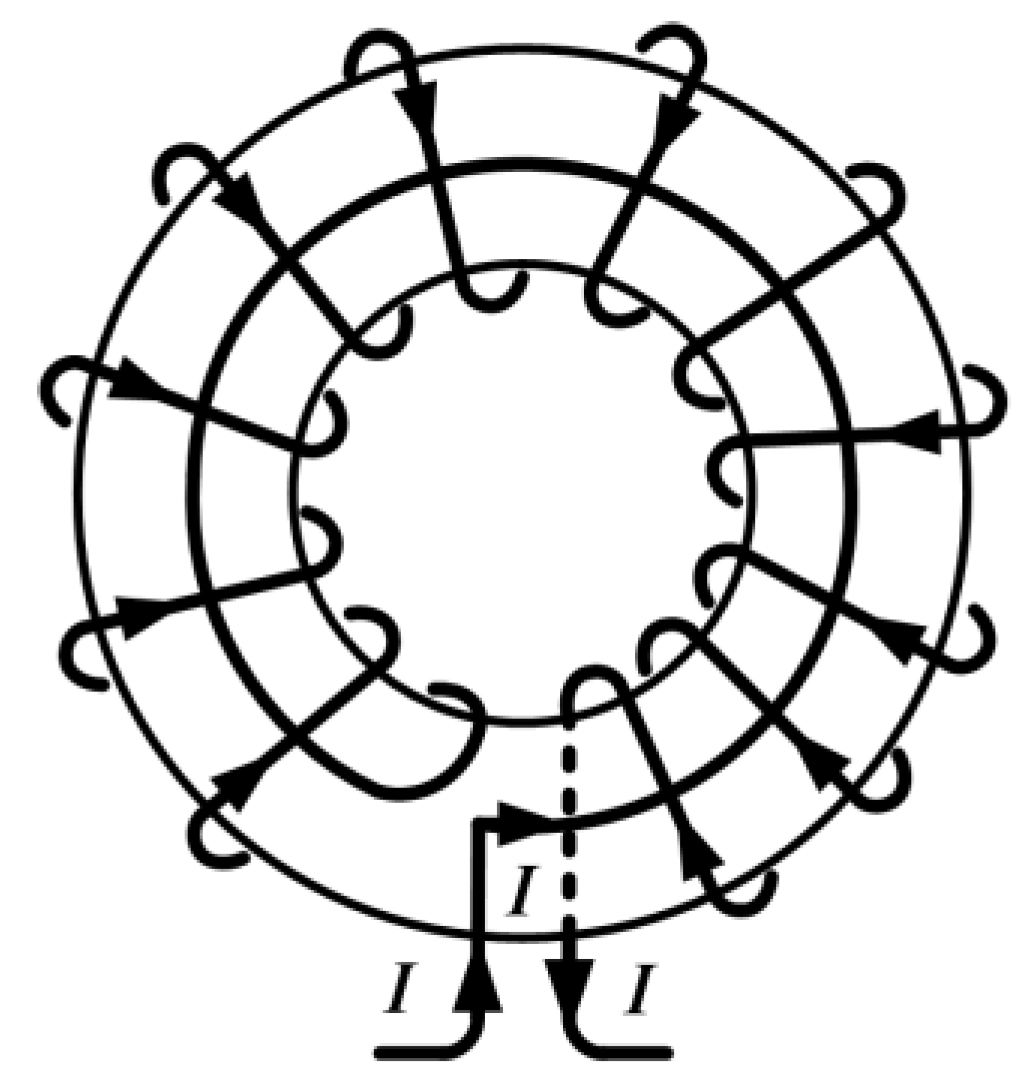
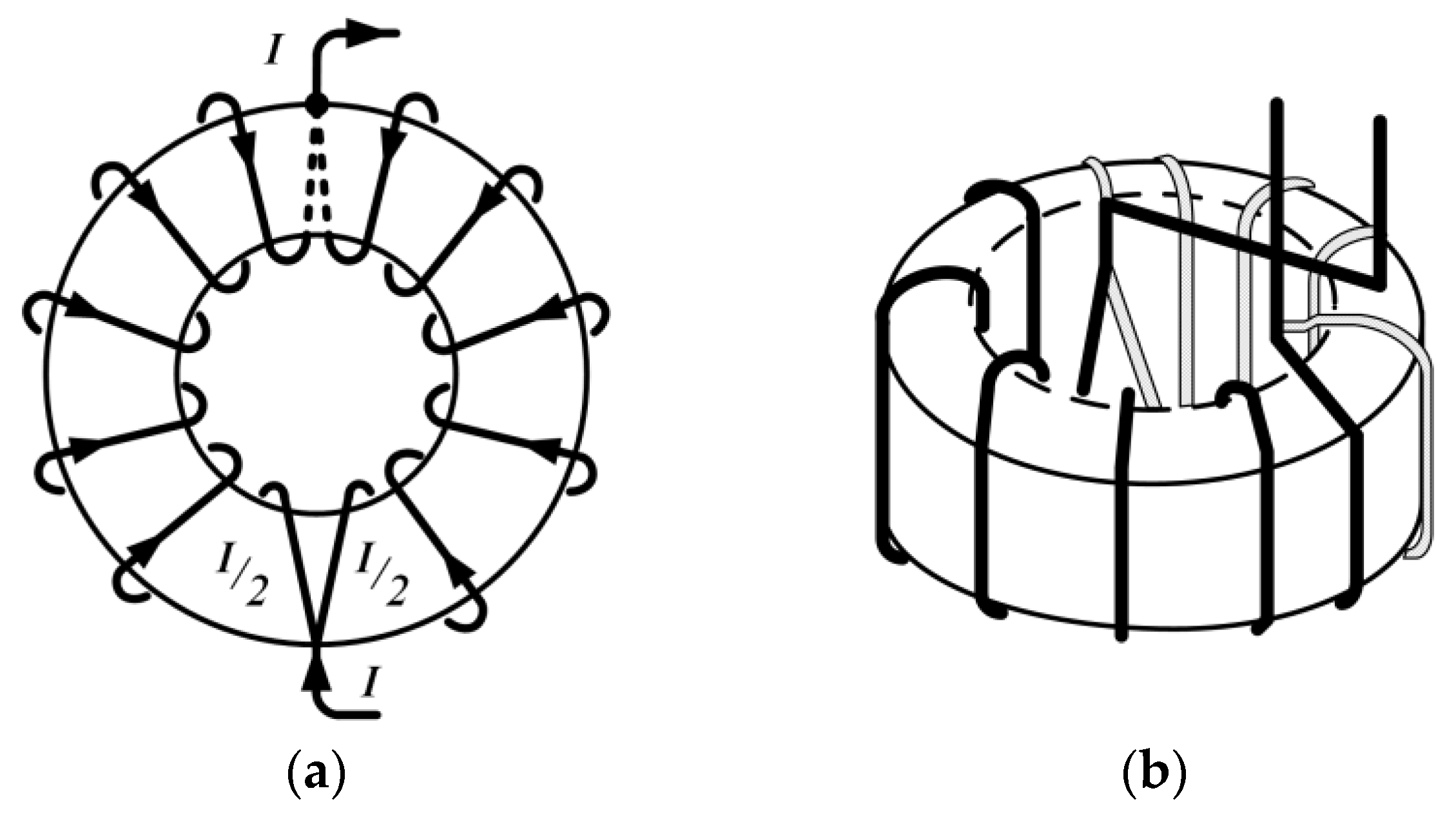
2. Proposed Compensation of External Magnetic Fields
3. Design of the System
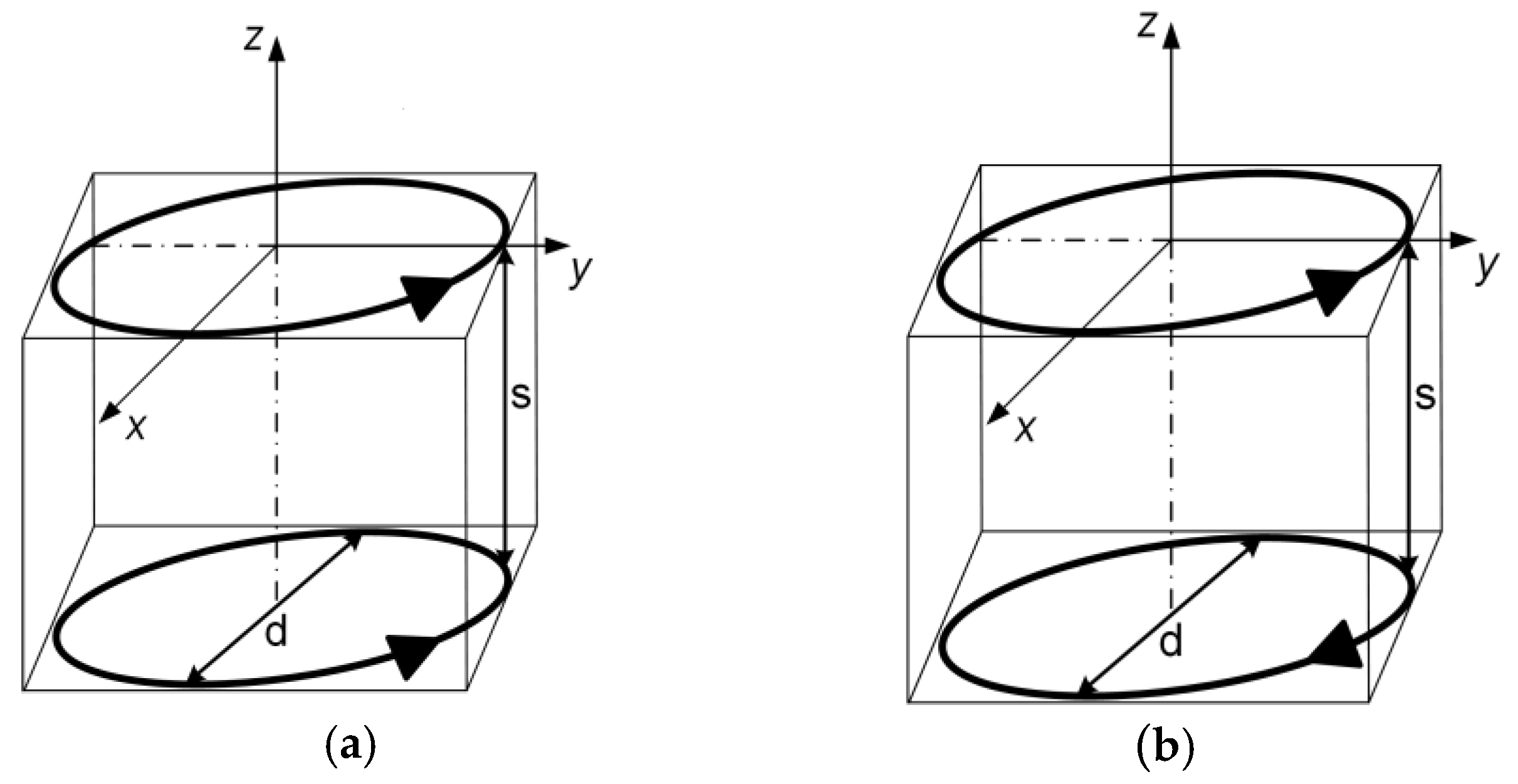
3.1. Inductance of a Spiral Rectangular Coil
3.2. FEM Calculations
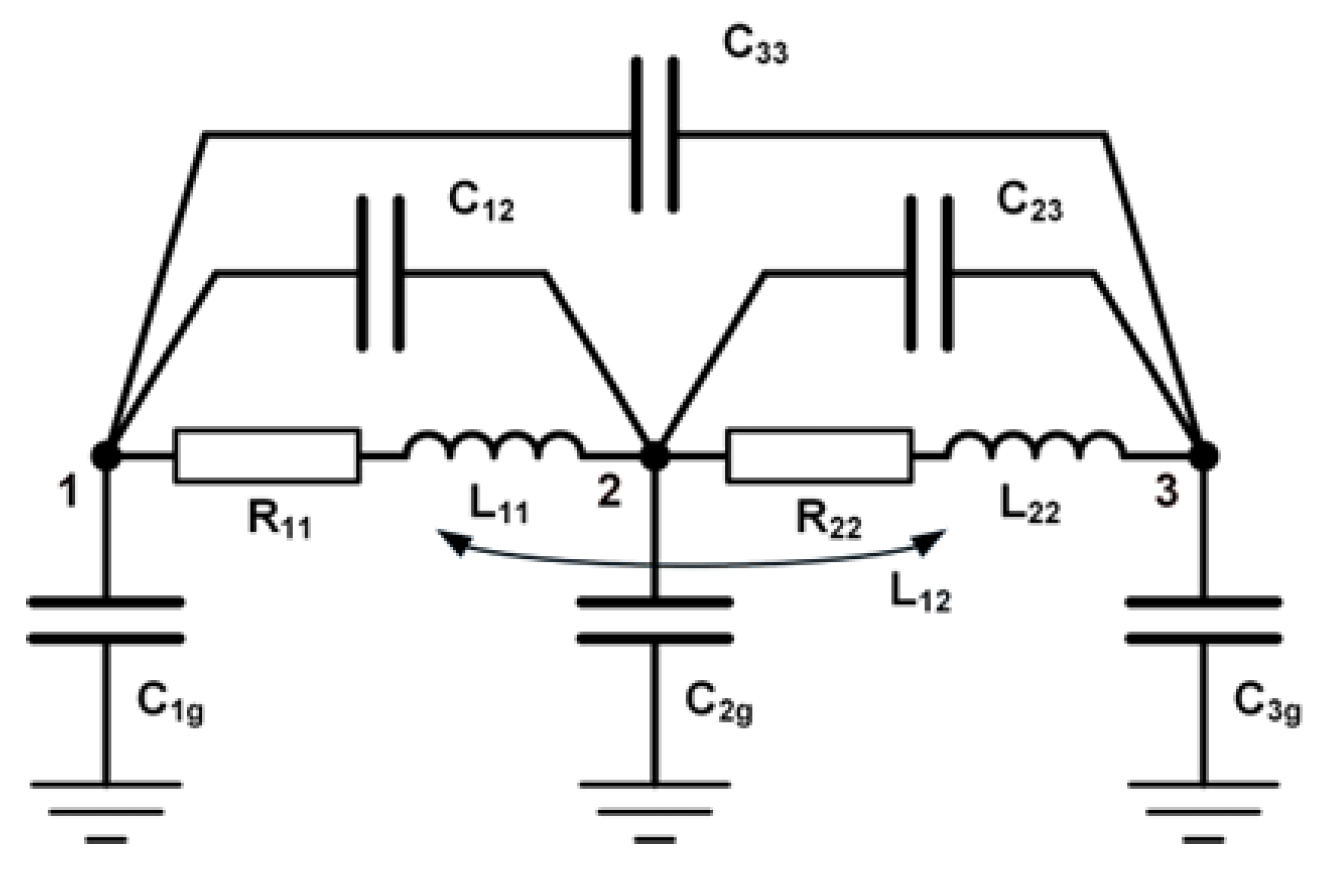
3.3. Enclosure and Electrostatic Shielding
4. Temperature Coefficients—Materials and Methods
4.1. Measurement Setup
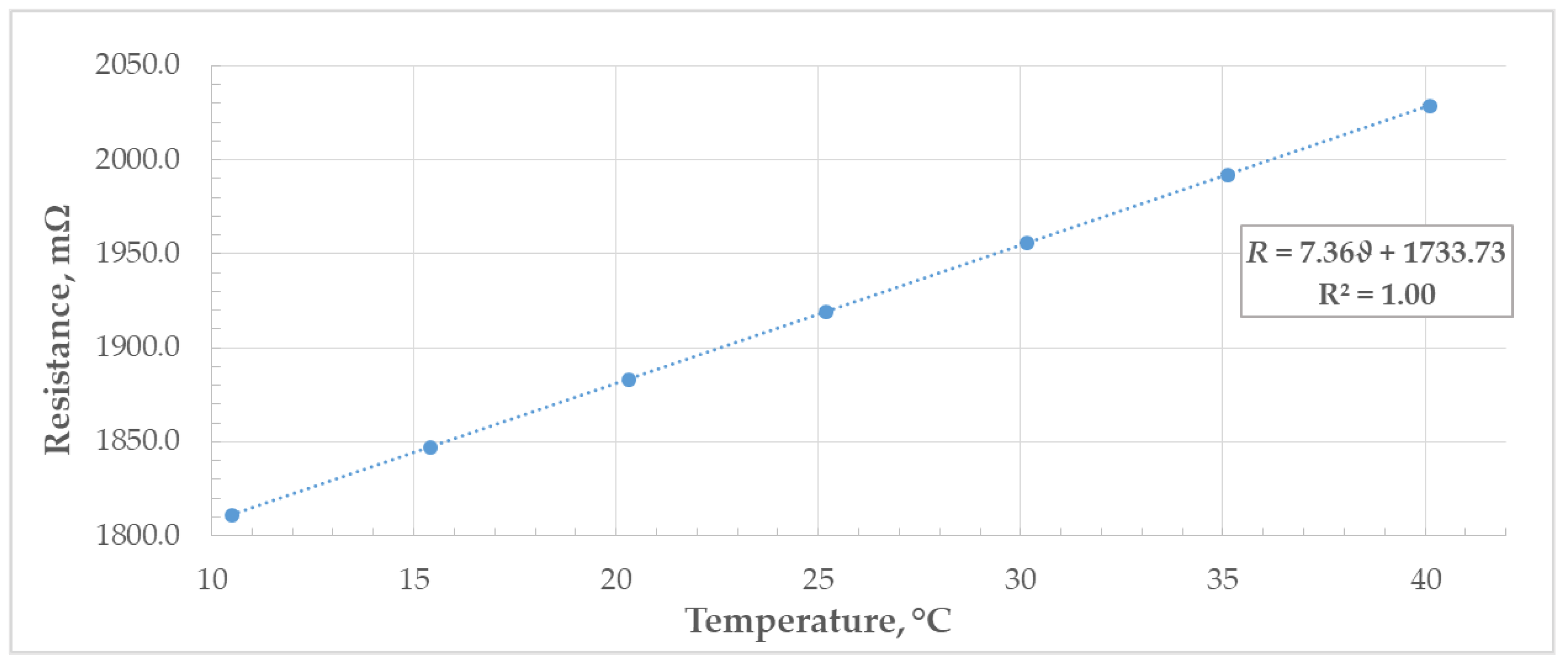
4.2. Determination of the Temperature Coefficient of Resistance (TCR)
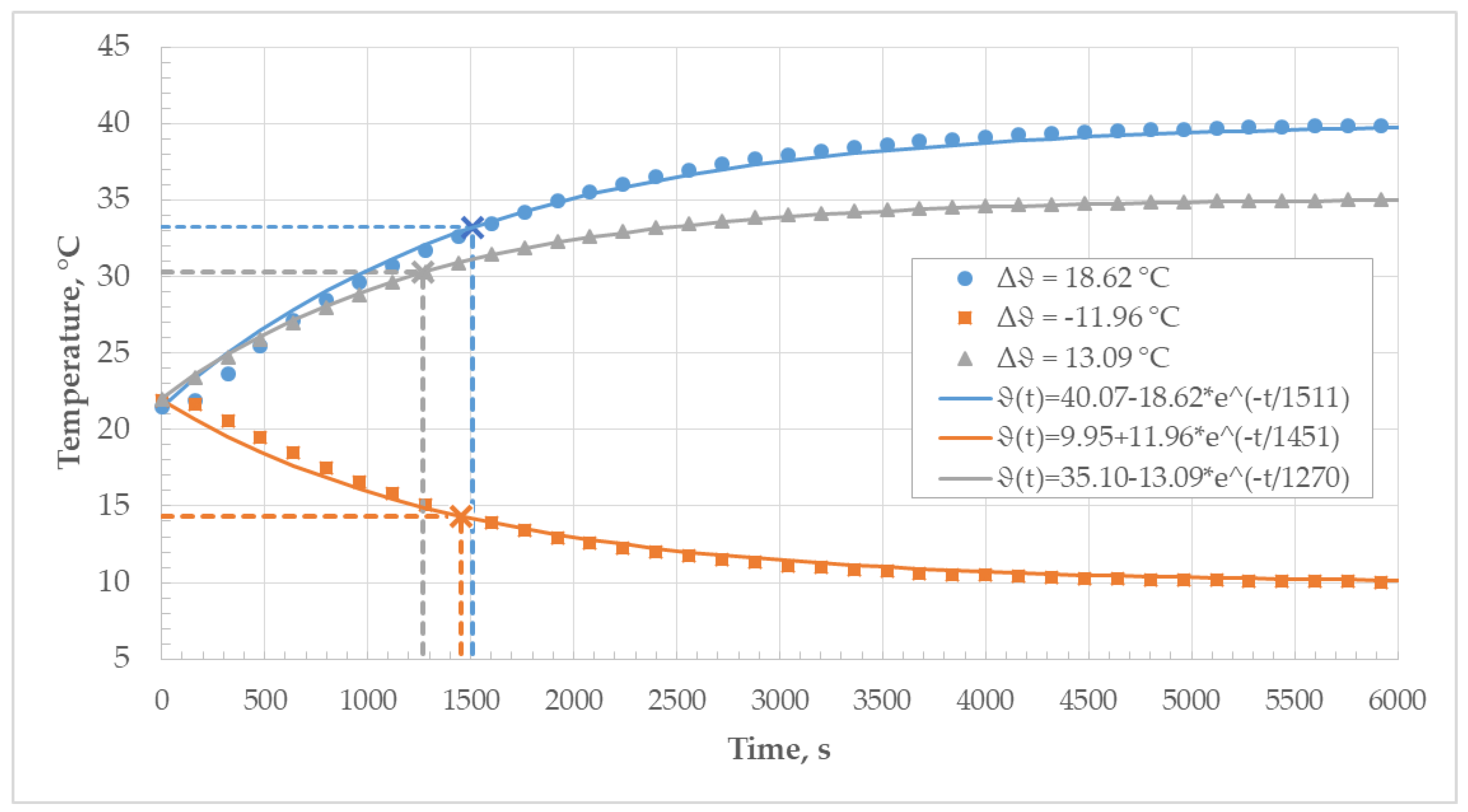
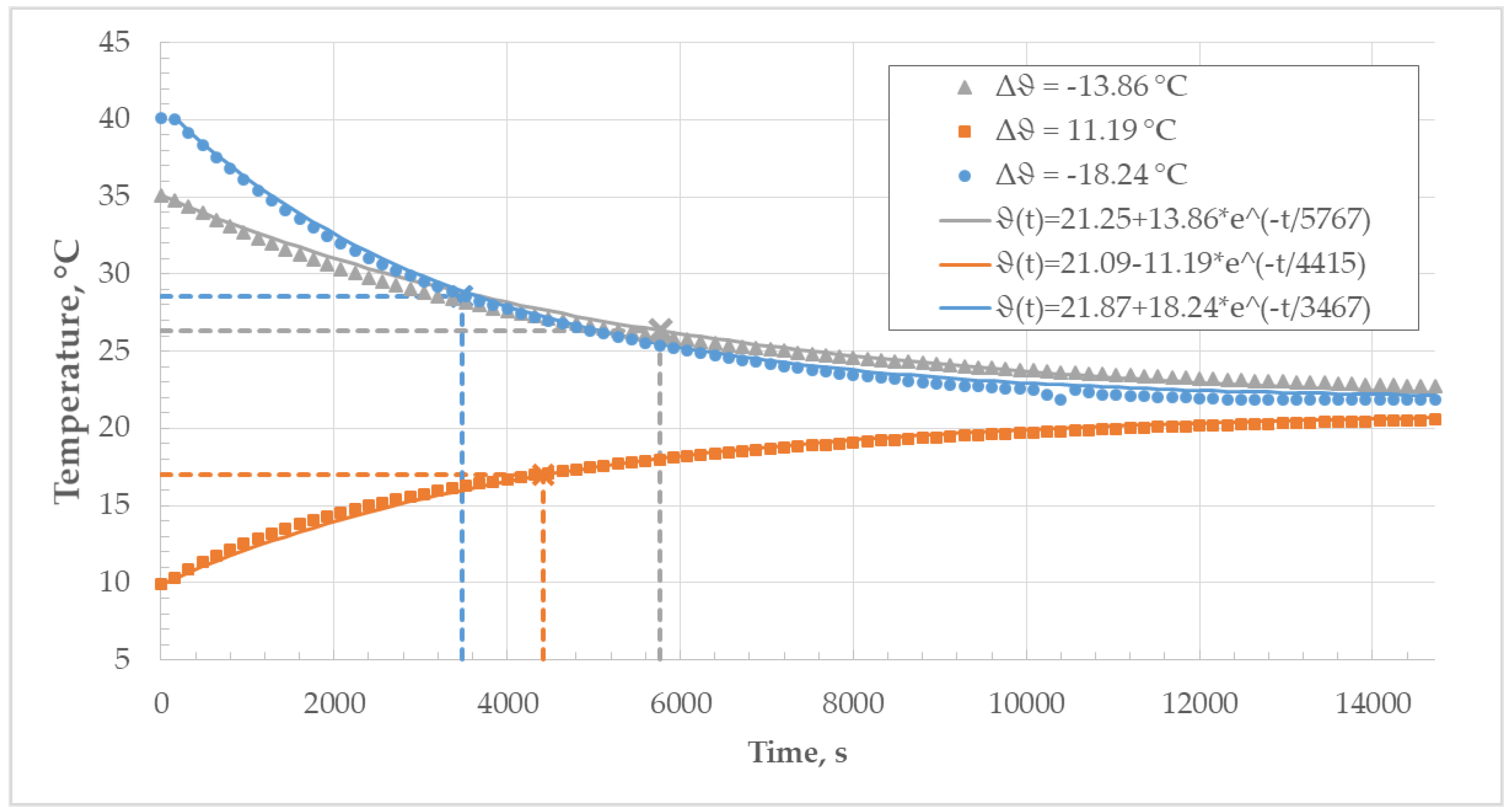
4.3. Determination of Thermal Time Constant
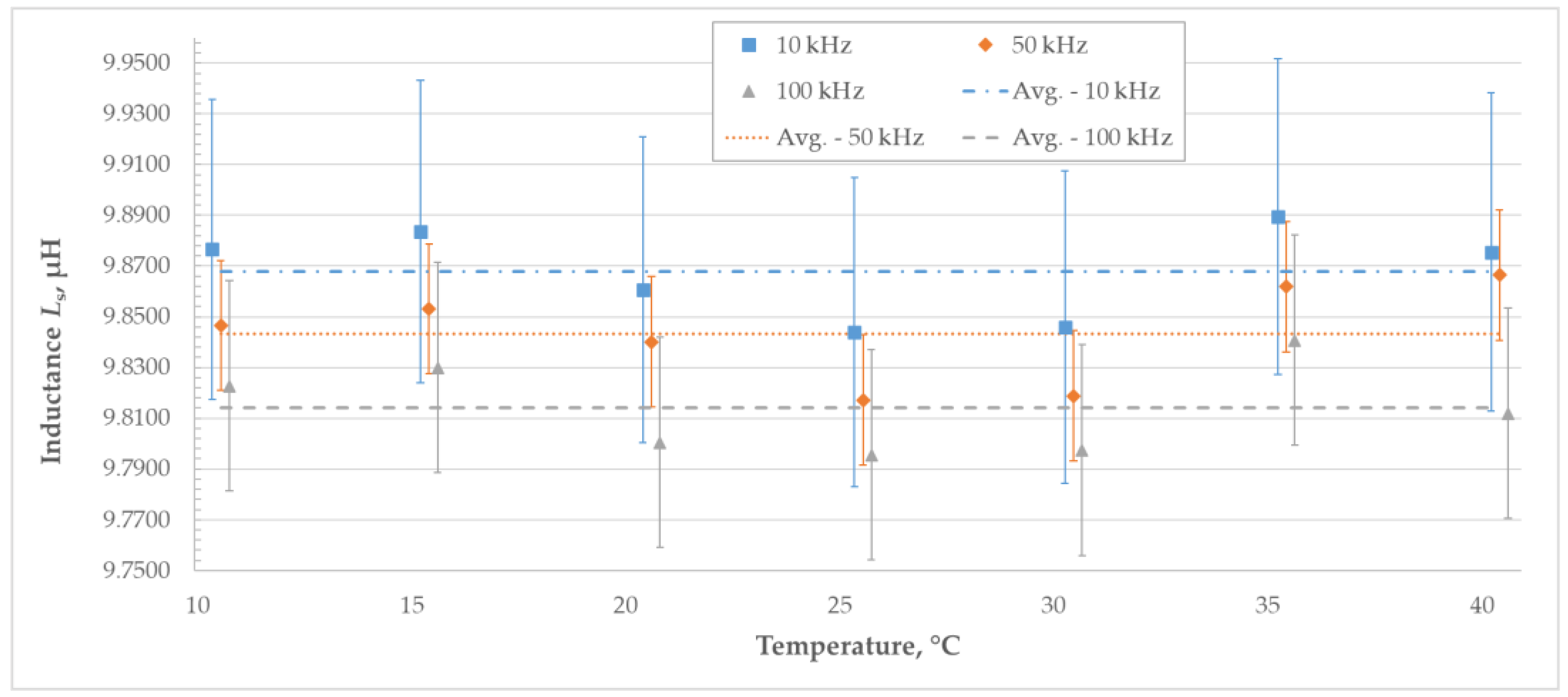
4.4. Measurement of Inductance
5. Results
5.1. Temperature Coefficient of Resistance
5.2. Determination of Thermal Time Constant τ
5.3. Measurement of Series Inductance and Series AC Resistance
6. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Northrop, R.B. Introduction to Instrumentation and Measurements; Taylor & Francis: Oxfordshire, UK, 2005. [Google Scholar]
- Xin, W.; Wenjun, L.; Cheng, D.; Xiaobing, H.; Fang, S. The national inductance standard in NIM. In Proceedings of the Conference on Precision Electromagnetic Measurements. Conference Digest, CPEM 2000 (Cat. No.00CH37031), Sydney, Australia, 14–19 May 2000; pp. 139–140. [Google Scholar] [CrossRef]
- Horsky, J.; Horska, J. Simulated inductance standard. In Proceedings of the Conference Digest Conference on Precision Electromagnetic Measurements, Ottwa, ON, Canada, 16–21 June 2002; pp. 188–189. [Google Scholar] [CrossRef]
- Funck, T.; Müller, A.; Bothe, H. Synthetic Inductance Standards Made Up of Capacitances and Gyrators. IEEE Trans. Instrum. Meas. 2021, 70, 2002504. [Google Scholar] [CrossRef]
- Overney, F.; Jeanneret, B. Realization of an inductance scale traceable to the quantum Hall effect using an automated synchronous sampling system. Metrologia 2010, 47, 690. [Google Scholar] [CrossRef]
- Dyer, S.A. (Ed.) Survey on Instrumentation and Measurement; Wiley-Interscience: Hoboken, NJ, USA, 2001. [Google Scholar]
- Hersh, J.F. New standard inductors—more terminals less inductance. Gen. Radio Exp. 1960, 34. Available online: https://www.ietlabs.com/pdf/GR_Experimenters/1960/GenRad_Experimenter_Oct_1960.pdf (accessed on 3 July 2024).
- Sarker, M.T.; Tan, A.H.; Yap, T.T.V. Input Spectrum Design for Identification of a Thermostat System. IEEE Access 2023, 11, 2920–2927. [Google Scholar] [CrossRef]
- Augustyn, J.; Kampik, M. Improved Sine-Fitting Algorithms for Measurements of Complex Ratio of AC Voltages by Asynchronous Sequential Sampling. IEEE Trans. Instrum. Meas. 2019, 68, 1659–1665. [Google Scholar] [CrossRef]
- Gulmez, Y.; Gulmez, C.; Turhan, E.; Ozkan, T. Temperature control system for inductance standard. In Proceedings of the Conference on Precision Electromagnetic Measurements, Conference Digest, CPEM 2000 (Cat. No.00CH37031), Sydney, Australia, 14–19 May 2000; pp. 143–144. [Google Scholar] [CrossRef]
- Wang, Z.; Ying, W.; Zeng, Y.; Wang, S.; Jiang, C.; Long, T.; Zhao, H. Equally Split PCB Inductor (ESPI) Design for High Energy Density and Low Near-Field Radiation. IEEE Trans. Power Electron. 2024, 39, 4963–4968. [Google Scholar] [CrossRef]
- Ding, Y.; Wang, X.; Allen, M.G. A PCB-Integrated Inductor With an Additively Electrodeposited Laminated NiFe Core for MHz DC–DC Power Conversion. IEEE Trans. Power Electron. 2023, 38, 15157–15161. [Google Scholar] [CrossRef]
- Son, G.; Li, Q. PCB Winding Coupled Inductor Design and Common-Mode EMI Noise Reduction for SiC-Based Soft-Switching Three-Phase AC–DC Converter. IEEE Trans. Power Electron. 2022, 37, 14514–14526. [Google Scholar] [CrossRef]
- Anurag, A.; Barbosa, P. PCB Based Inductor Structure for MV Applications. IEEE Trans. Power Electron. 2024, 39, 1980–1984. [Google Scholar] [CrossRef]
- Zhu, F.; Li, Q. A Novel PCB-Embedded Coupled Inductor Structure for a 20-MHz Integrated Voltage Regulator. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 10, 7452–7463. [Google Scholar] [CrossRef]
- Yu, Z.; Yang, X.; Wei, G.; Zhou, Y.; Xiao, Y.; Qin, M.; Wang, L. A Novel Pyramid Winding for PCB Planar Inductors With Fewer Copper Layers and Lower AC Copper Loss. IEEE Trans. Power Electron. 2022, 37, 11461–11468. [Google Scholar] [CrossRef]
- Huang, Z.; Son, G.; Li, Q.; Lee, F.C. Balance Techniques and PCB Winding Magnetics for Common-Mode EMI Noise Reduction in Three-Phase AC–DC Converters. IEEE Trans. Power Electron. 2022, 37, 3130–3142. [Google Scholar] [CrossRef]
- Bang, J.; Park, Y.; Jung, K.; Choi, J. A Compact Low-Cost Wideband Shielded-Loop Probe with Enhanced Performance for Magnetic Near-Field Measurements. IEEE Trans. Electromagn. Compat. 2020, 62, 1921–1928. [Google Scholar] [CrossRef]
- Martinez, P.A.; Navarro, E.A.; Victoria, J.; Suarez, A.; Torres, J.; Alcarria, A.; Perez, J.; Amaro, A.; Menendez, A.; Soret, J. Design and study of a wide-band printed circuit board near-field probe. Electronics 2021, 10, 2201. [Google Scholar] [CrossRef]
- Chou, Y.T.; Lu, H.C. Magnetic near-field probes with high-pass and notch filters for electric field suppression. IEEE Trans. Microw. Theory Tech. 2013, 61, 2460–2470. [Google Scholar] [CrossRef]
- Filipašić, M.; Dadić, M. A Two-Turn Shielded-Loop Magnetic Near-Field PCB Probe for Frequencies up to 3 GHz. Sensors 2023, 23, 7308. [Google Scholar] [CrossRef] [PubMed]
- Shao, W.; Li, J.; Huang, Q.; Shao, E.; Liu, J.; He, X.; Zhou, C. A two-turn loop active magnetic field probe design for high sensitivity near-field measurement. IET Sci. Meas. Technol. 2022, 16, 40–46. [Google Scholar] [CrossRef]
- Rydler, K.-E.; Tarasso, V.; Bergsten, T. Scaling of inductance to pH-level. In Proceedings of the Conference on Precision Electromagnetic Measurements, Daejeon, Republic of Korea, 13–18 June 2010; pp. 384–385. [Google Scholar]
- Kibble, B.P.; Rayner, G.H. Coaxial AC Bridges; Adam Hilger Ltd.: Bristol, UK, 1984. [Google Scholar]
- Becker, R. Electromagnetic Fields and Interactions; Dover: New York, NY, USA, 1982. [Google Scholar]
- Bosanac, T. Teoretska Elektrotehnika 1; Tehnička knjiga: Zagreb, Croatia, 1970. [Google Scholar]
- Mohan, S.S.; del Mar Hershenson, M.; Boyd, S.P.; Lee, T.H. Simple Accurate Expressions for Planar Inductances. IEEE J. Solid-State Circuits 1999, 34, 1419–1424. [Google Scholar] [CrossRef]
- Robichaud, A.; Boudreault, M.; Deslandes, D. Theoretical analysis of resonant wireless power transmission links composed of electrically small loops. Prog. Electromagn. Res. 2013, 143, 485–501. [Google Scholar] [CrossRef]
- Grover, F.W. Inductance Calculations; Courier Corporation: North Chelmsford, MA, USA, 2013. [Google Scholar]
- Mills, M.K. Self inductance formulas for multi-turn rectangular loops used with vehicle detectors. In Proceedings of the 33rd IEEE Vehicular Technology Conference, Toronto, ON, Canada, 25–27 May 1983; pp. 65–73. [Google Scholar]
- Tavakkoli, H.; Abbaspour-Sani, E.; Khalilzadegan, A.; Abazari, A.-M.; Rezaadeh, G. Mutual inductance calculation between two coaxial planar spiral coils with an arbitrary number of sides. Microelectron. J. 2019, 85, 98. [Google Scholar] [CrossRef]
- Hussain, I.; Woo, D.K. Inductance Calculation of Single-Layer Planar Spiral Coil. Electronics 2022, 11, 750. [Google Scholar] [CrossRef]
- Smajic, J.; Steinmetz, T.; Rüegg, M.; Tanasic, Z.; Obrist, R.; Tepper, J.; Carlen, M. Simulation and Measurement of Lightning-Impulse Voltage Distributions Over Transformer Windings. IEEE Trans. Magn. 2014, 50, 7013604. [Google Scholar] [CrossRef]
- Špikić, D.; Švraka, M.; Vasić, D. Effectiveness of Electrostatic Shielding in High-Frequency Electromagnetic Induction Soil Sensing. Sensors 2022, 22, 3000. [Google Scholar] [CrossRef] [PubMed]
- Chen, H.; Lin, W.; Shao, S.; Wu, X.; Zhang, J. Application of Tunnel Magnetoresistance for PCB Tracks Current Sensing in High-Frequency Power. IEEE Trans. Instrum. Meas. 2023, 72, 9003011. [Google Scholar] [CrossRef]
- E5061B, ENA Vector Network Analyzer Data Sheet. Available online: https://www.keysight.com/us/en/assets/7018-02242/data-sheets/5990-4392.pdf (accessed on 3 July 2024).
- Kuhn, W.B.; Boutz, A.P. Measuring and Reporting High Quality Factors of Inductors Using Vector Network Analyzers. IEEE Trans. Microw. Theory Tech. 2010, 58, 1046–1055. [Google Scholar] [CrossRef]
- Wojnowski, M.; Sommer, G.; Weigel, R. Device Characterization Techniques Based on Causal Relationships. IEEE Trans. Microw. Theory Tech. 2012, 60, 2203–2219. [Google Scholar] [CrossRef]
- Zvizdic, D.; Veliki, T.; Grgec Bermanec, L. Realization of the Temperature Scale in the Range from 234.3 K (Hg Triple Point) to 1084.62 °C (Cu Freezing Point) in Croatia. Int. J. Thermophys. 2008, 29, 984–990. [Google Scholar] [CrossRef]



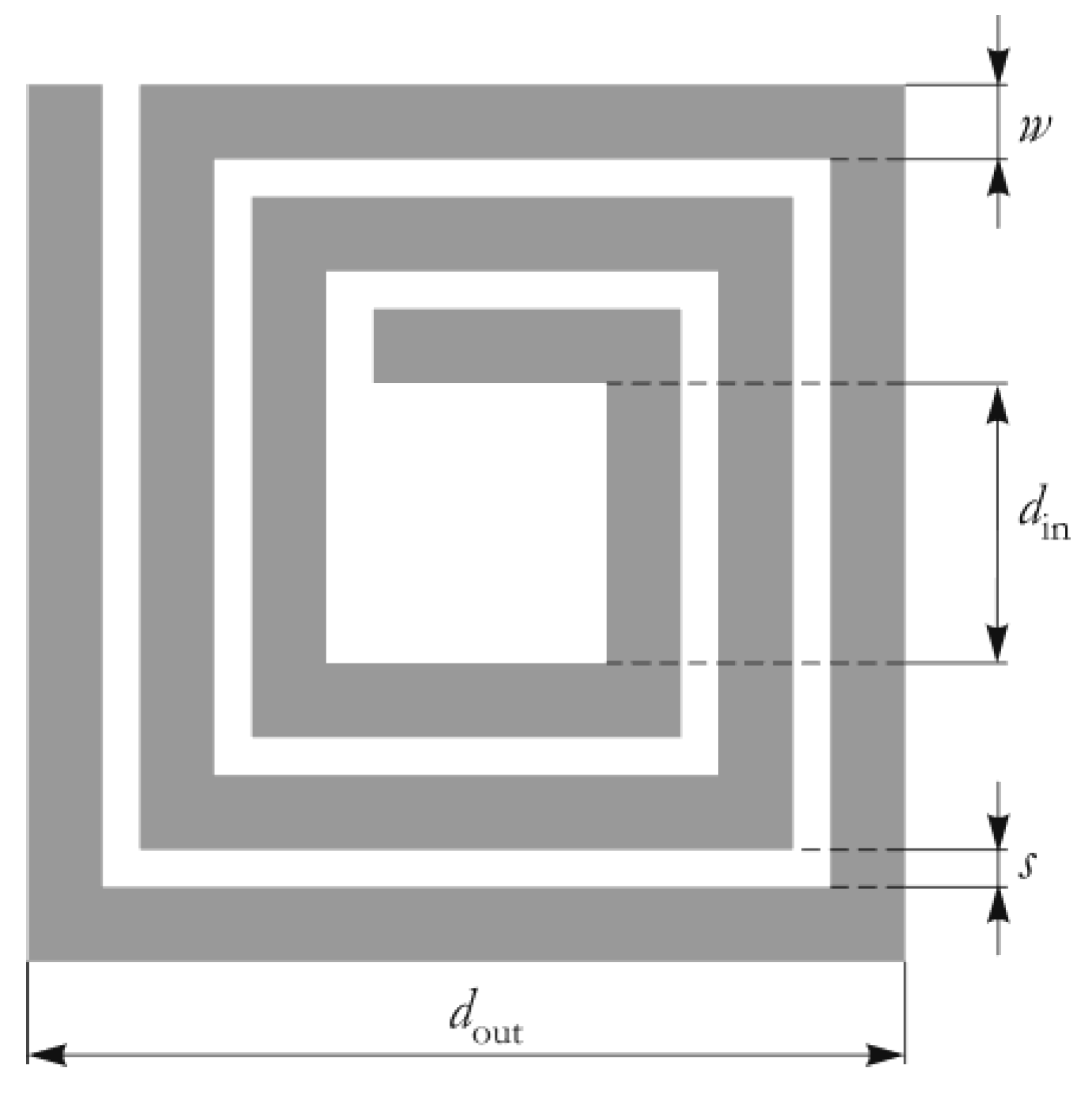


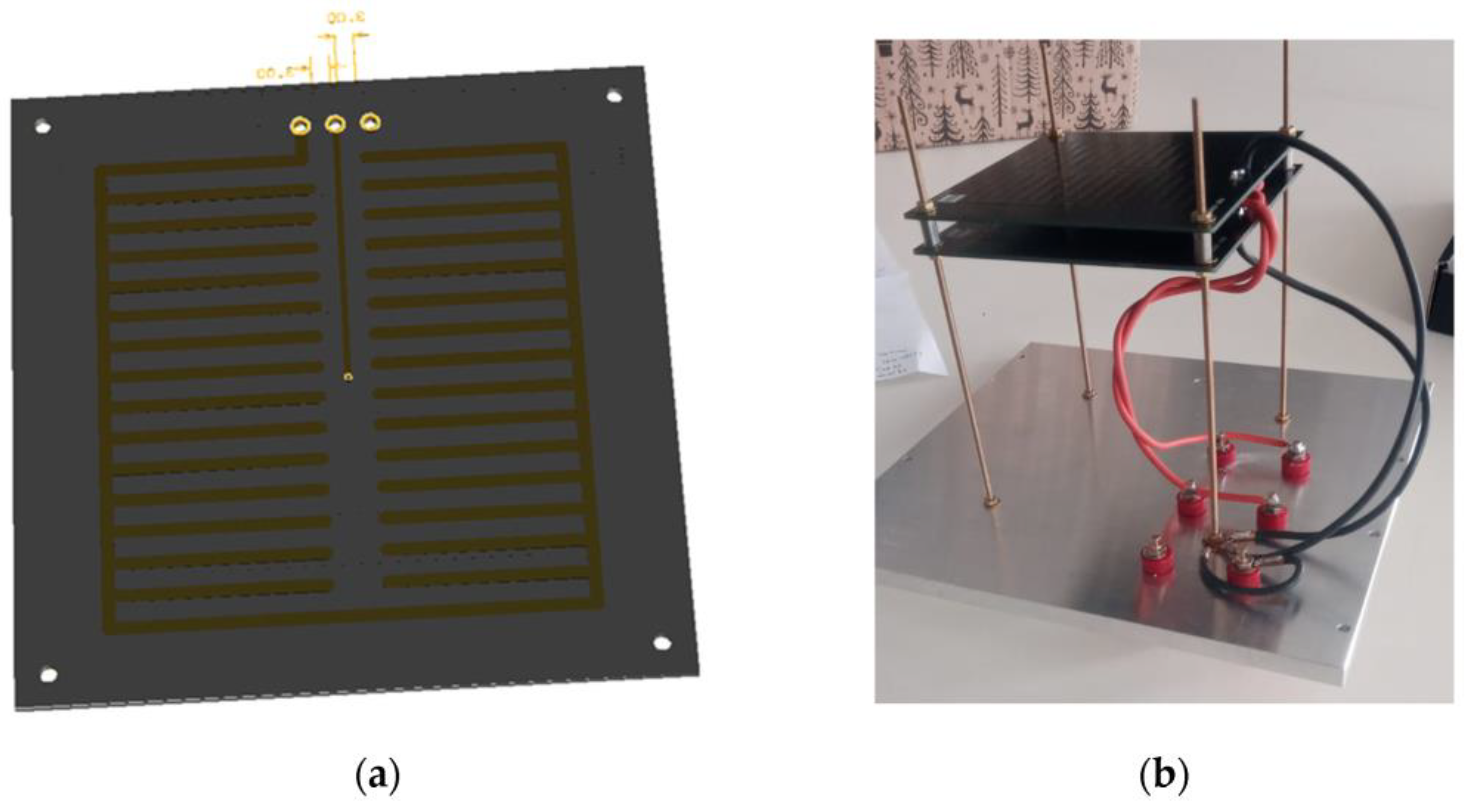
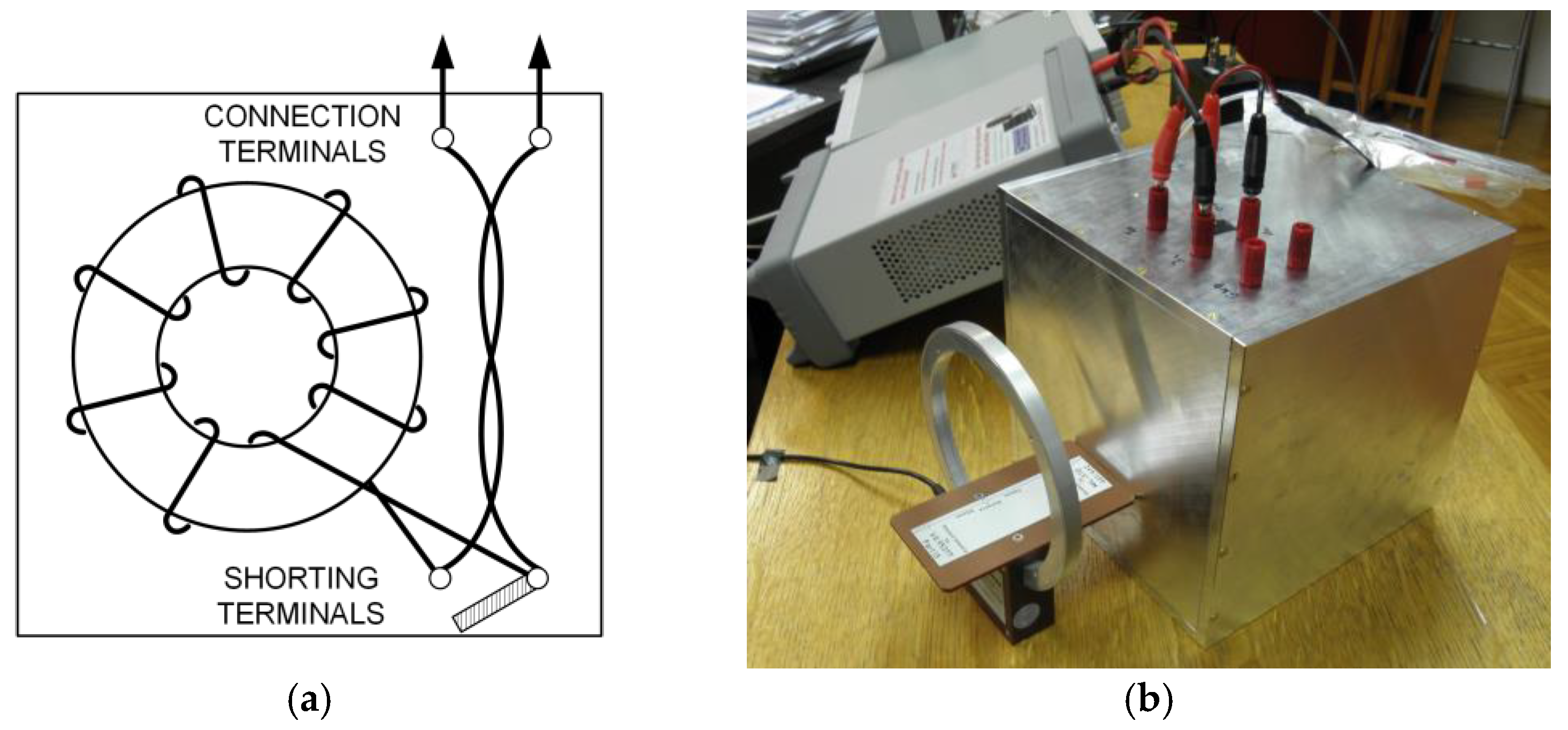

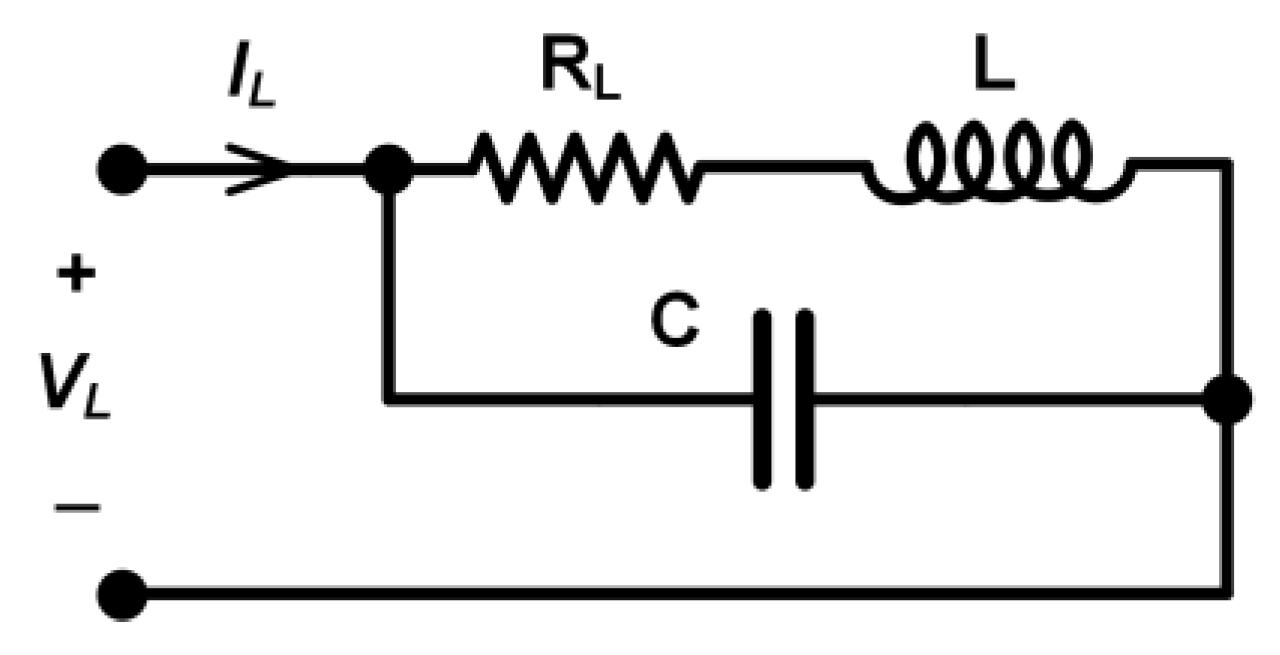
| Number of Turns (N) | Space between the Last Two Turns (D), mm | Inductance (L), µH |
|---|---|---|
| 18 | 1 | 8.91 |
| 19 | 1 | 10.25 |
| 19 | 1.5 | 10.21 |
| 19 | 2 | 10.17 |
| Distance between Coils (d), mm | Equivalent Inductance (Leq), µH |
|---|---|
| 20 | 15.7 |
| 15 | 13.86 |
| 8.5 | 9.8 |
| 7.5 | 8.81 |
| Temperature, °C | Resistance, mΩ | Measurement Uncertainty (k = 2), mΩ |
|---|---|---|
| 10.49 | 1810.9 | 0.7 |
| 15.41 | 1847.2 | 0.5 |
| 20.32 | 1883.3 | 0.4 |
| 25.20 | 1919.3 | 0.4 |
| 30.15 | 1955.7 | 0.5 |
| 35.11 | 1992.1 | 0.5 |
| 40.11 | 2028.9 | 0.5 |
| Initial Temperature (), °C | Final Temperature (), °C | Thermal Time Constant (τ), s | |
|---|---|---|---|
| Method 1 | Method 2 | ||
| 21.45 | 40.07 | 1553 | 1511 |
| 21.91 | 9.95 | 1467 | 1451 |
| 22.01 | 35.10 | 1279 | 1270 |
| Initial Temperature (), °C | Final Temperature (), °C | Thermal Time Constant (τ), s | |
|---|---|---|---|
| Method 1 | Method 2 | ||
| 35.11 | 21.25 | 5293 | 5767 |
| 9.90 | 21.09 | 4143 | 4415 |
| 40.11 | 21.87 | 3507 | 3467 |
| Temperature, °C | Inductance (Ls), µH | |||||
|---|---|---|---|---|---|---|
| at 10 kHz | U (k = 2) | at 50 kHz | U (k = 2) | at 100 kHz | U (k = 2) | |
| 10.11 | 9.88 | 0.06 | 9.85 | 0.03 | 9.82 | 0.04 |
| 14.98 | 9.88 | 0.06 | 9.85 | 0.03 | 9.83 | 0.04 |
| 20.17 | 9.86 | 0.06 | 9.84 | 0.03 | 9.80 | 0.04 |
| 25.11 | 9.84 | 0.06 | 9.82 | 0.03 | 9.80 | 0.04 |
| 30.03 | 9.85 | 0.06 | 9.82 | 0.03 | 9.80 | 0.04 |
| 35.00 | 9.89 | 0.06 | 9.86 | 0.03 | 9.84 | 0.04 |
| 39.98 | 9.88 | 0.06 | 9.87 | 0.03 | 9.81 | 0.04 |
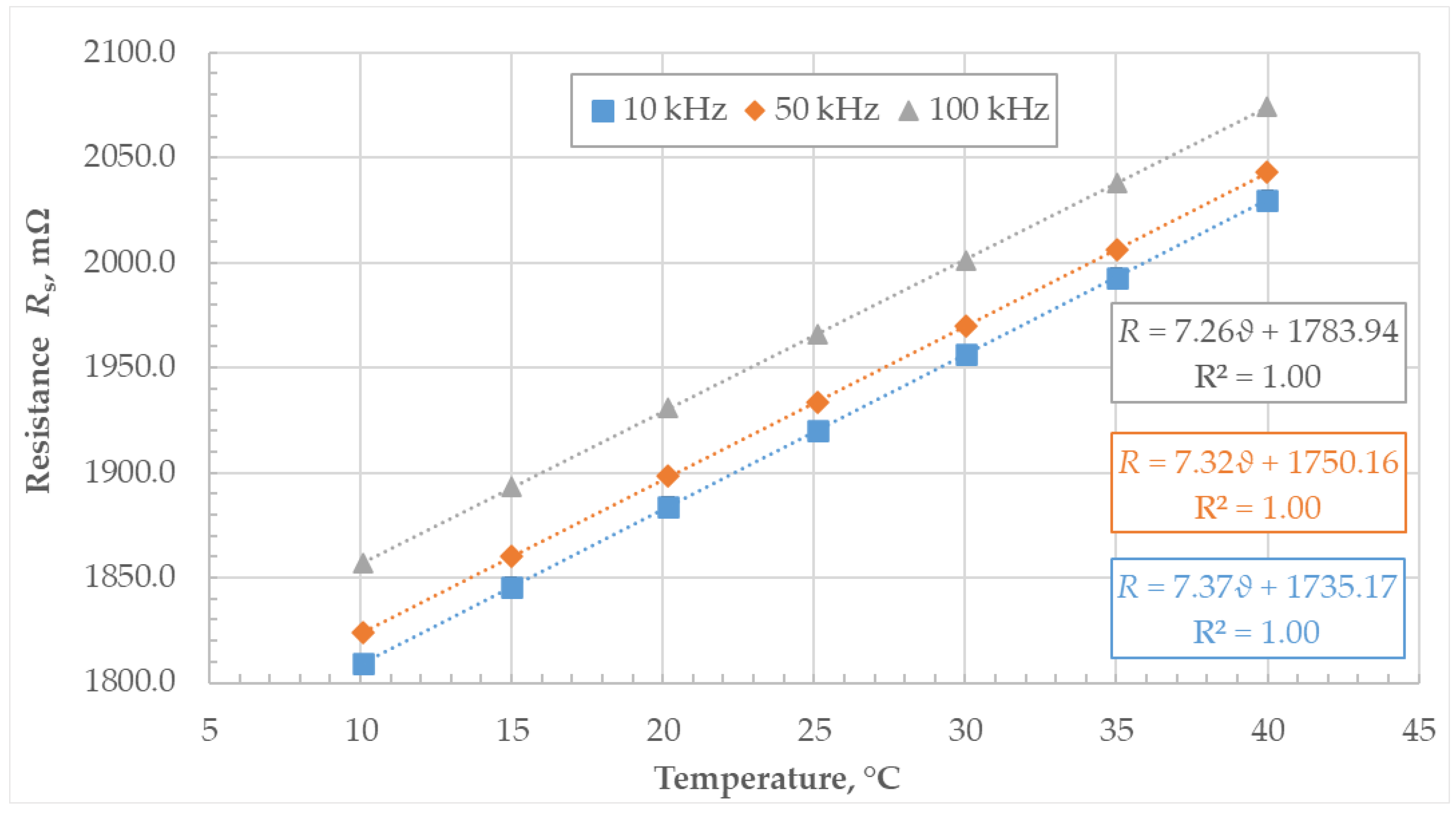
| Temperature, °C | Resistance (Rs), mΩ | |||||
|---|---|---|---|---|---|---|
| at 10 kHz | U (k = 2) | at 50 kHz | U (k = 2) | at 100 kHz | U (k = 2) | |
| 10.11 | 1809.3 | 3.4 | 1823.8 | 5.3 | 1856.9 | 8.7 |
| 14.98 | 1845.8 | 3.5 | 1860.2 | 5.3 | 1893.1 | 8.7 |
| 20.17 | 1884.1 | 3.5 | 1898.3 | 5.3 | 1930.9 | 8.7 |
| 25.11 | 1920.1 | 3.5 | 1933.8 | 5.3 | 1965.7 | 8.7 |
| 30.03 | 1956.2 | 3.6 | 1969.7 | 5.4 | 2001.4 | 8.7 |
| 35.00 | 1992.9 | 3.6 | 2006.3 | 5.4 | 2037.8 | 8.8 |
| 39.98 | 2029.9 | 3.7 | 2043.2 | 5.4 | 2074.5 | 8.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martinović, Ž.; Dadić, M.; Matas, I.; Grgec Bermanec, L. A 10 µH Inductance Standard in PCB Technology with Enhanced Protection against Magnetic Fields. Electronics 2024, 13, 3009. https://doi.org/10.3390/electronics13153009
Martinović Ž, Dadić M, Matas I, Grgec Bermanec L. A 10 µH Inductance Standard in PCB Technology with Enhanced Protection against Magnetic Fields. Electronics. 2024; 13(15):3009. https://doi.org/10.3390/electronics13153009
Chicago/Turabian StyleMartinović, Žarko, Martin Dadić, Ivan Matas, and Lovorka Grgec Bermanec. 2024. "A 10 µH Inductance Standard in PCB Technology with Enhanced Protection against Magnetic Fields" Electronics 13, no. 15: 3009. https://doi.org/10.3390/electronics13153009
APA StyleMartinović, Ž., Dadić, M., Matas, I., & Grgec Bermanec, L. (2024). A 10 µH Inductance Standard in PCB Technology with Enhanced Protection against Magnetic Fields. Electronics, 13(15), 3009. https://doi.org/10.3390/electronics13153009






