Self-Switching Wireless Power Transfer System Design with Constant Current/Constant Voltage Output Features Based on LCC-LCL/S Topology
Abstract
:1. Introduction
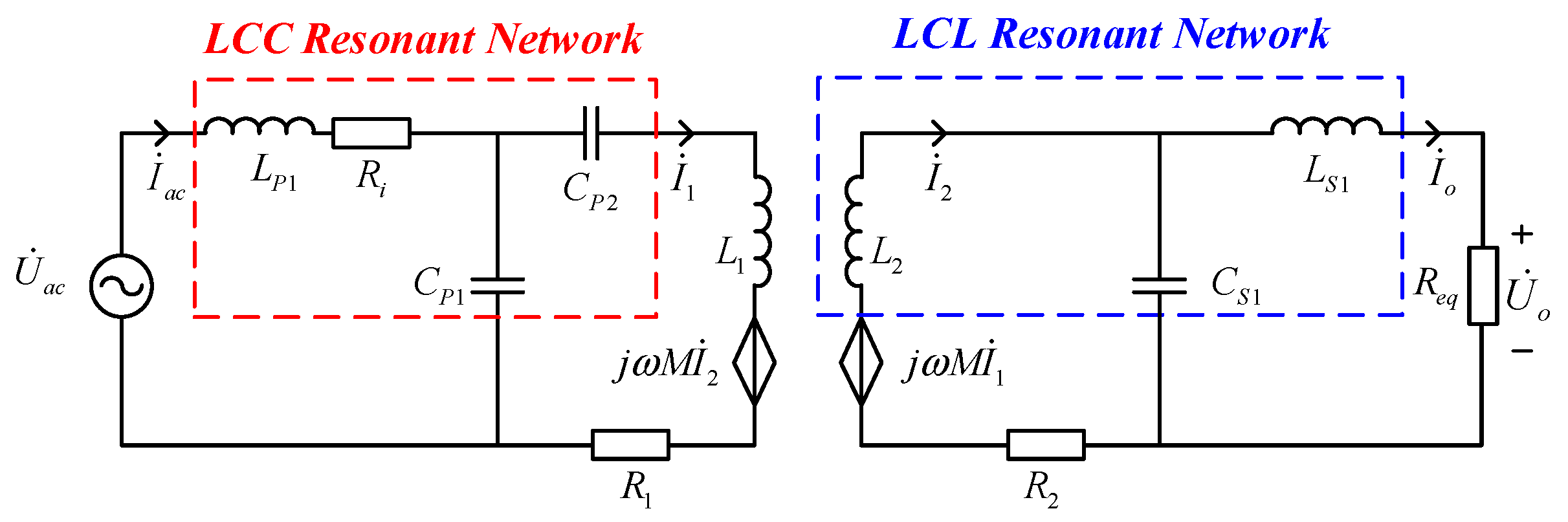
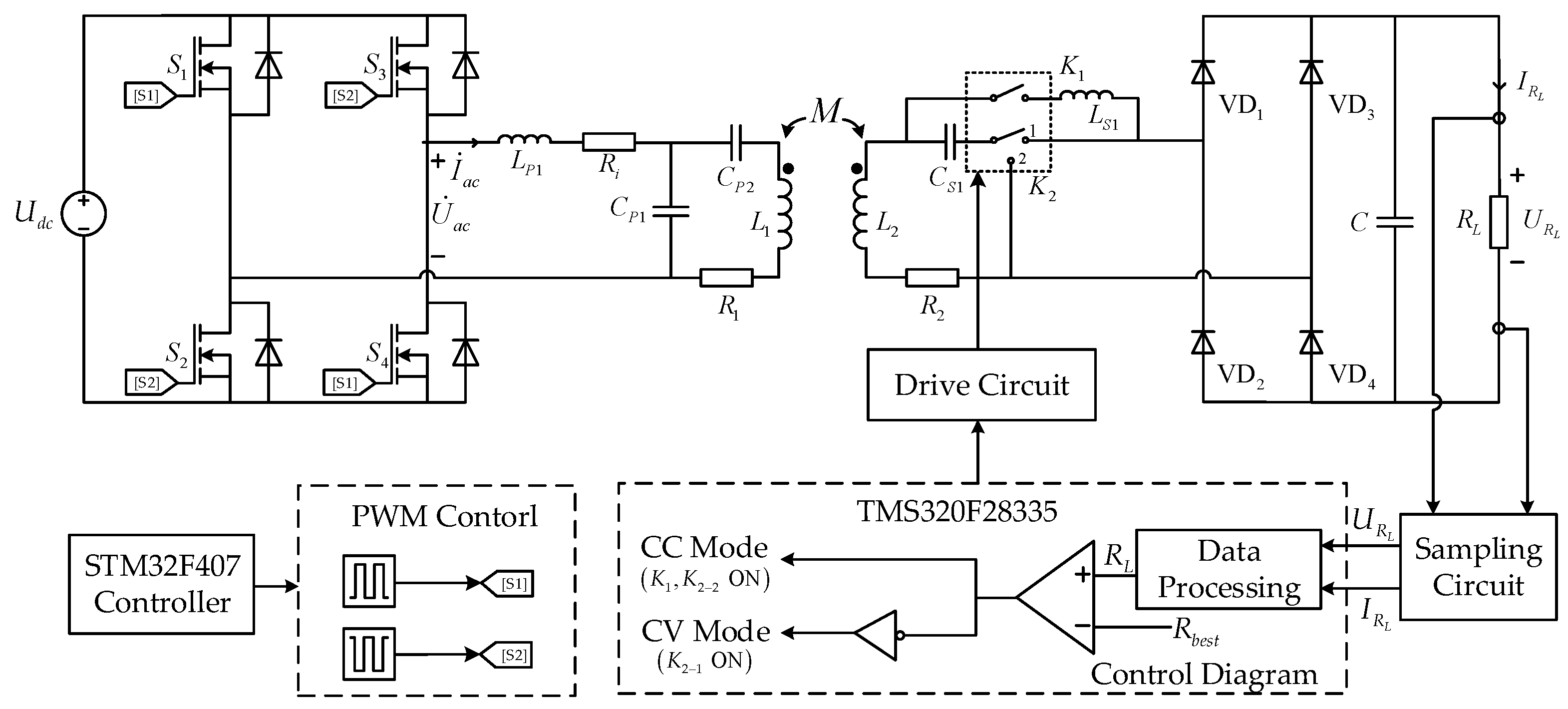
2. System Topology Analysis
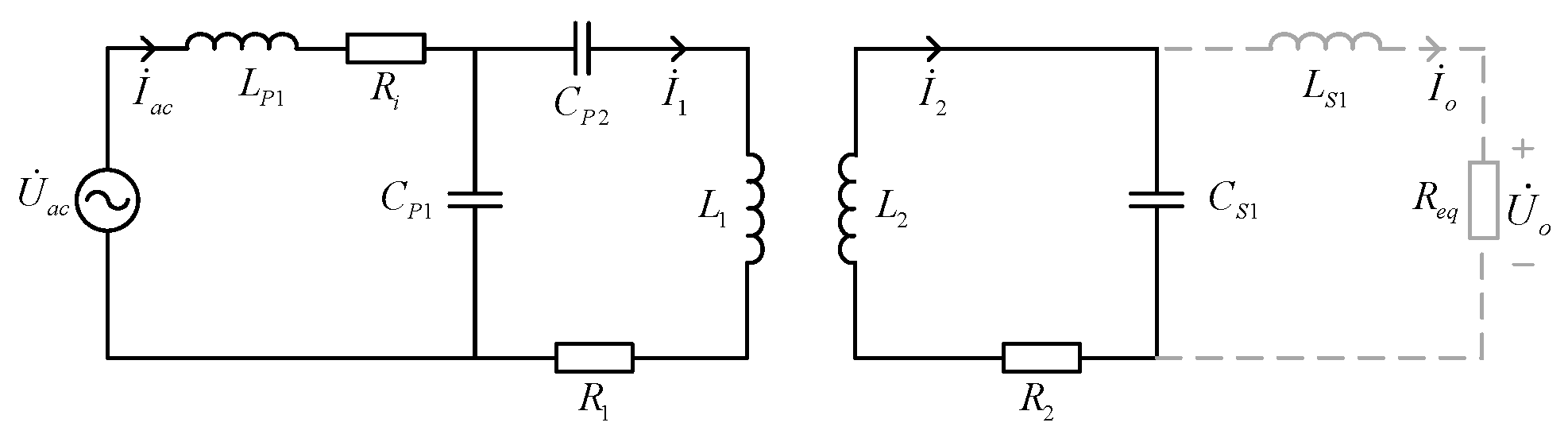
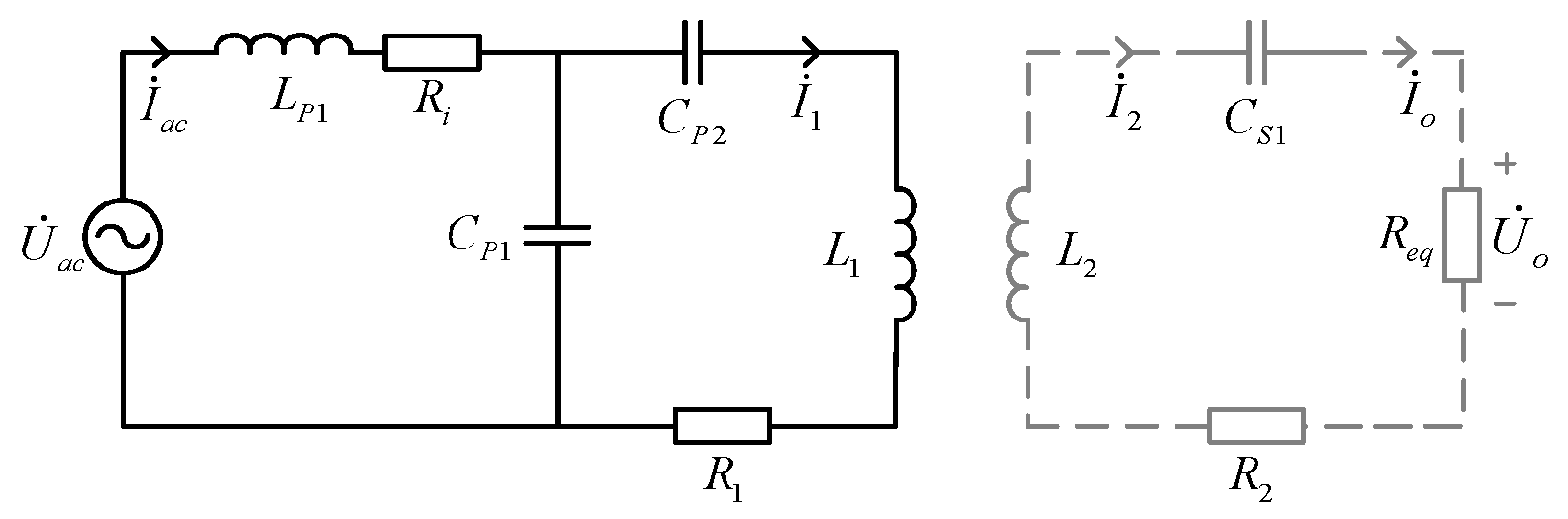
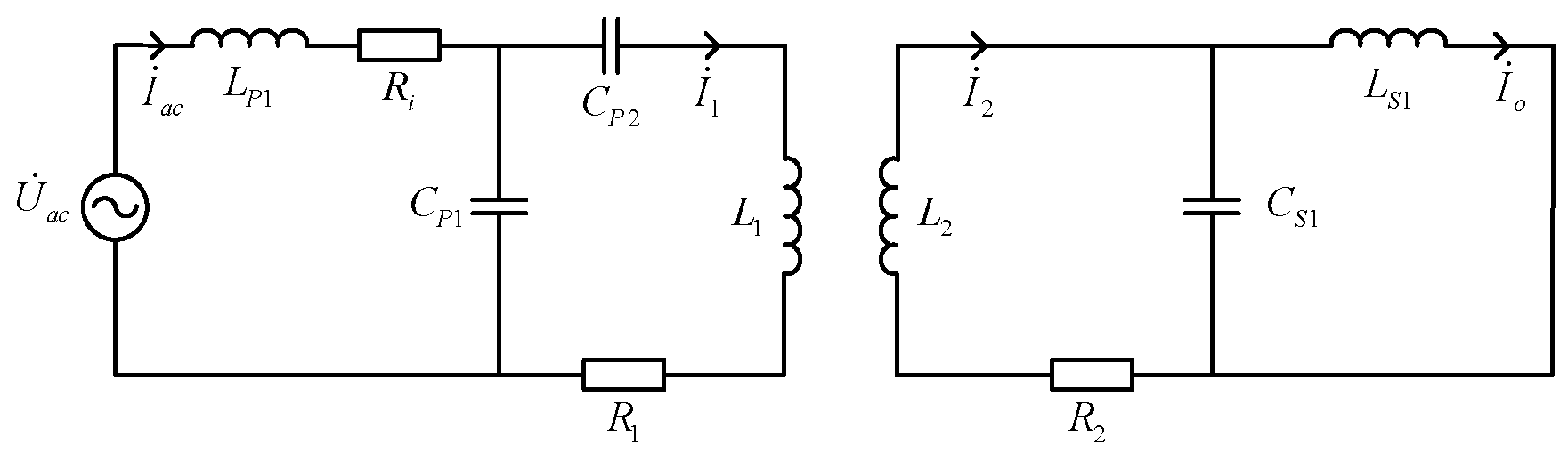
2.1. LCC-LCL Topology Analysis
2.2. LCC-S Topology Analysis
3. System Parameters Design
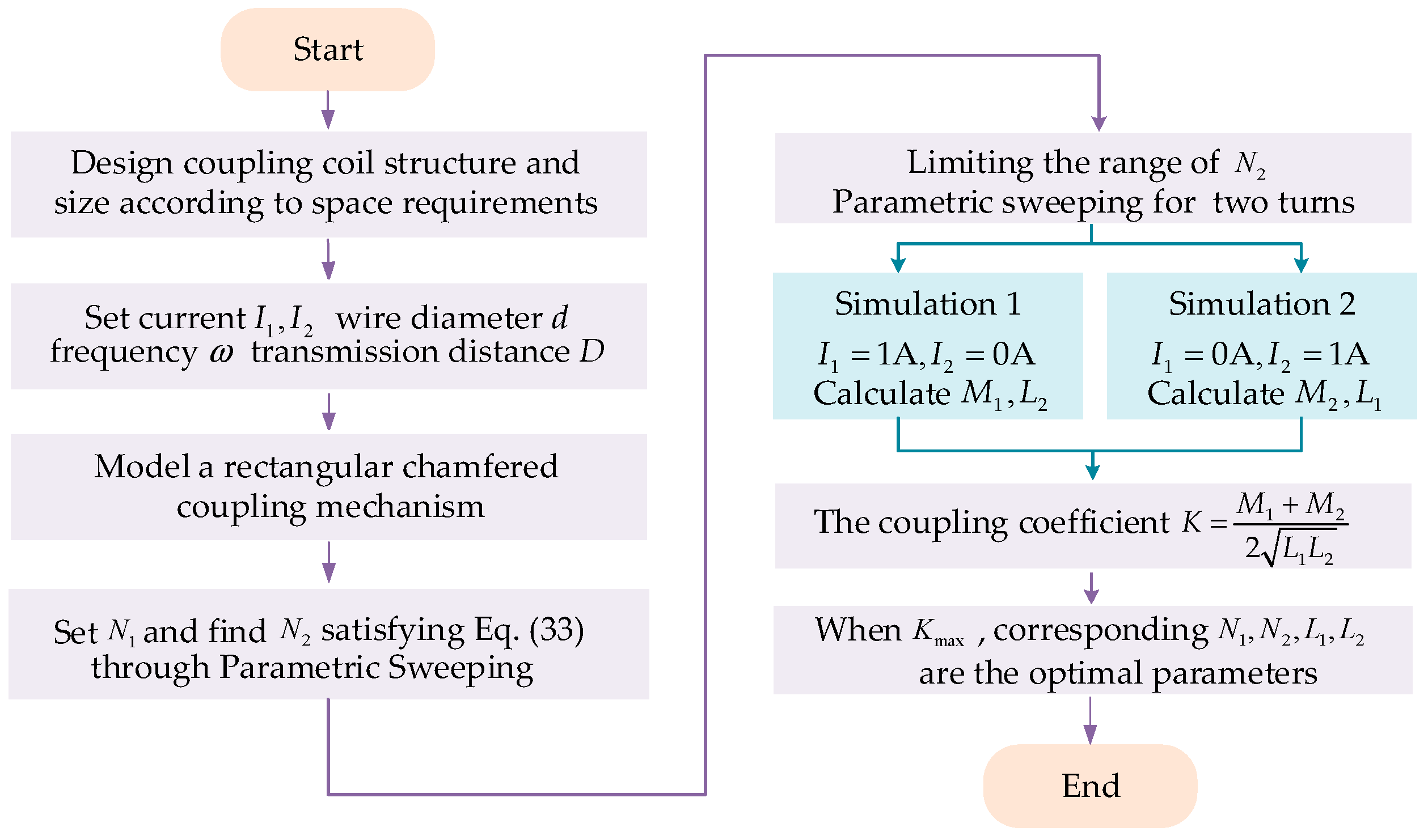
3.1. Design of Coupling Mechanism Parameter
3.2. Design of Compensation Components Parameter
4. Mode Switching Point Design
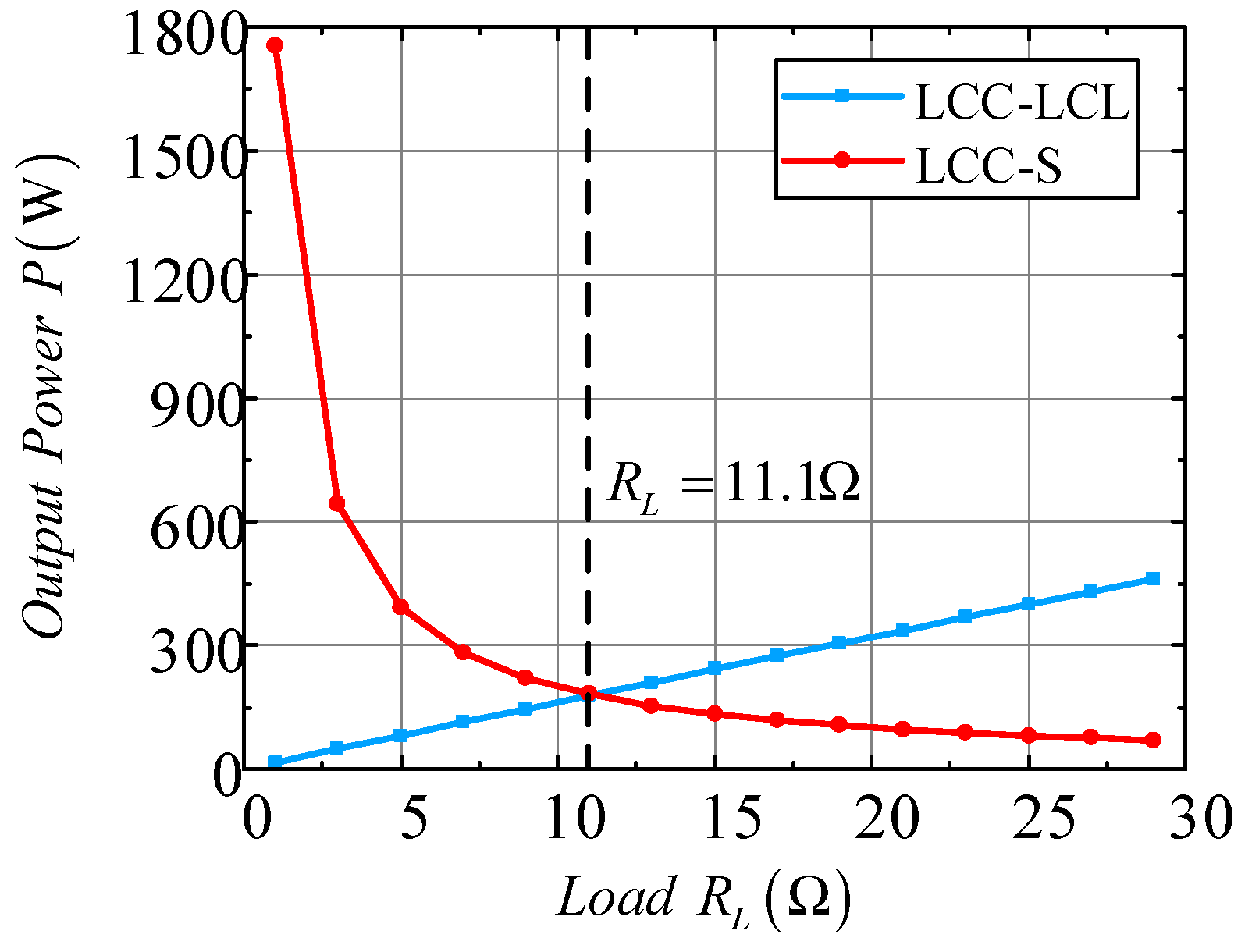
4.1. Analysis of the Load Switching Points
4.2. Optimality Verification of the Switching Point
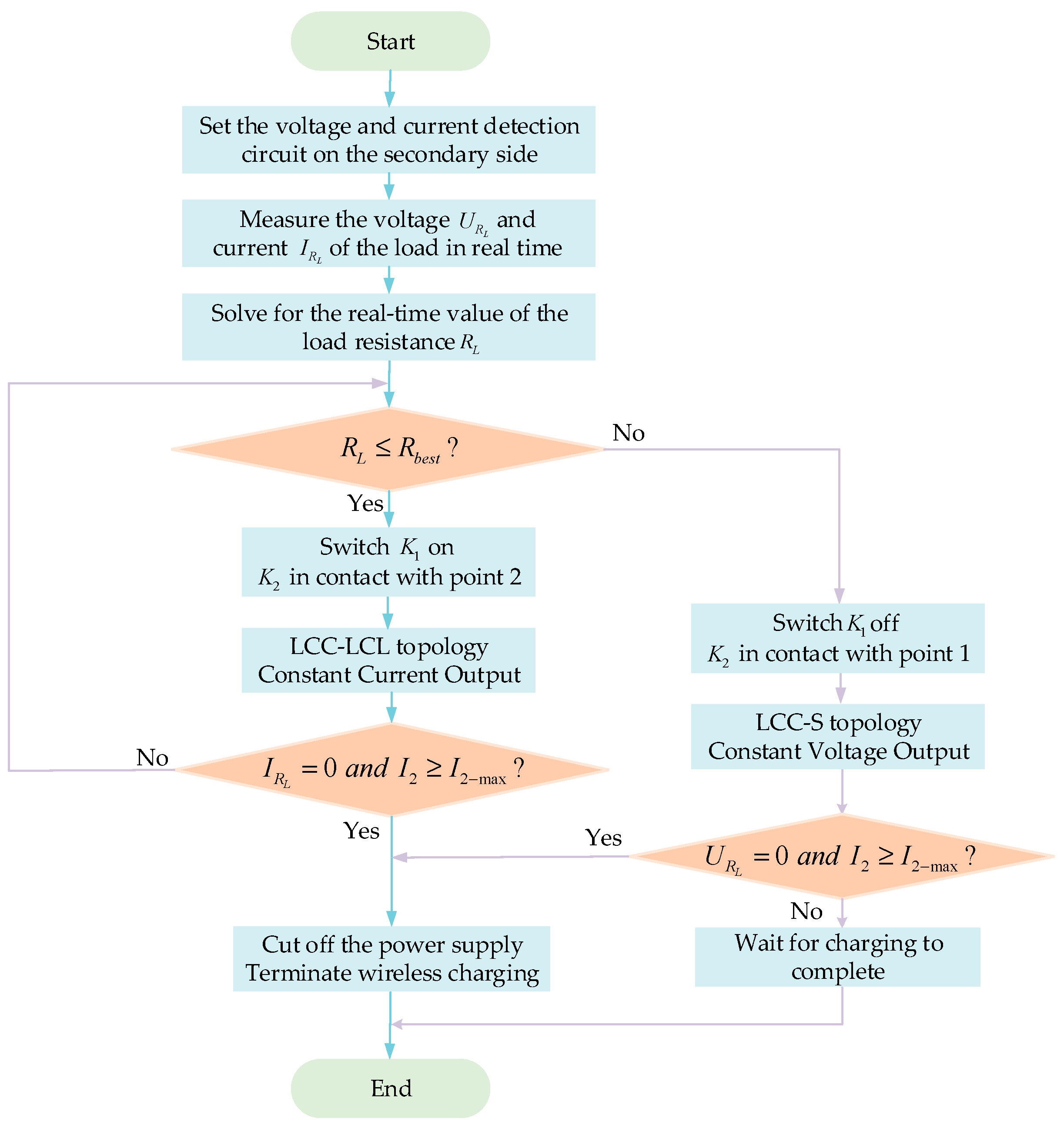
4.3. Design of Switching Control Strategy
4.3.1. Analysis of System No-Load Condition
4.3.2. Analysis of Load Short-Circuit Condition
5. System Simulation and Experiment Verification
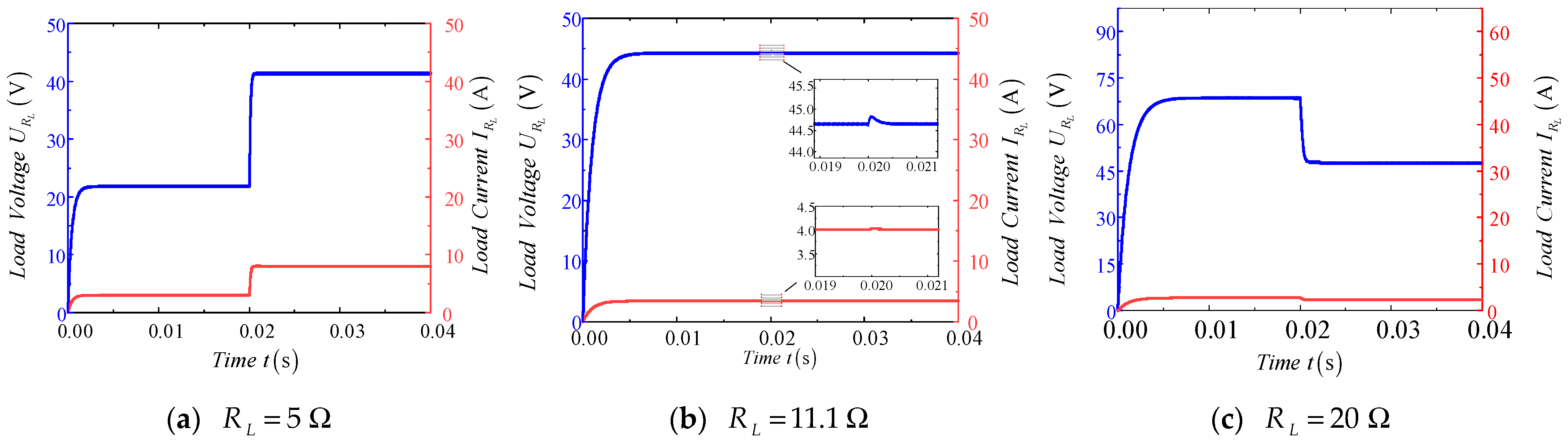
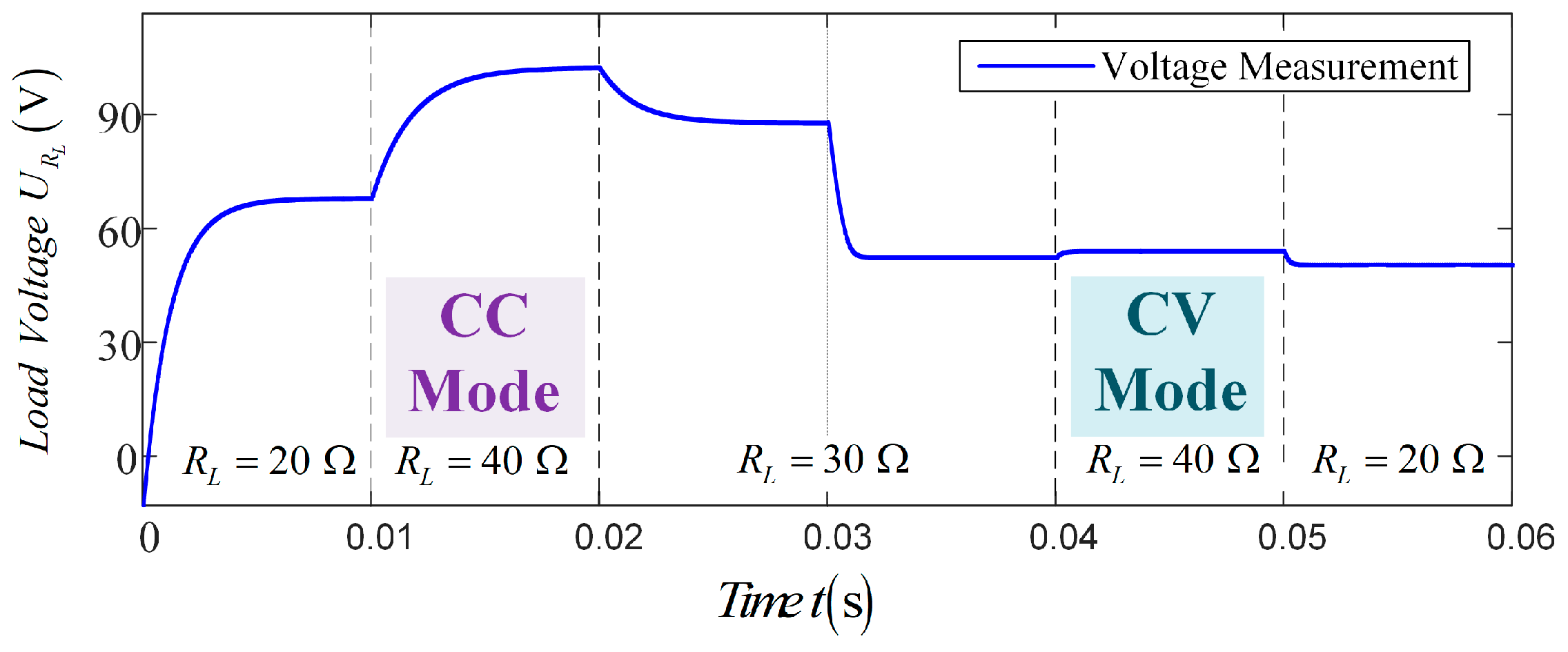
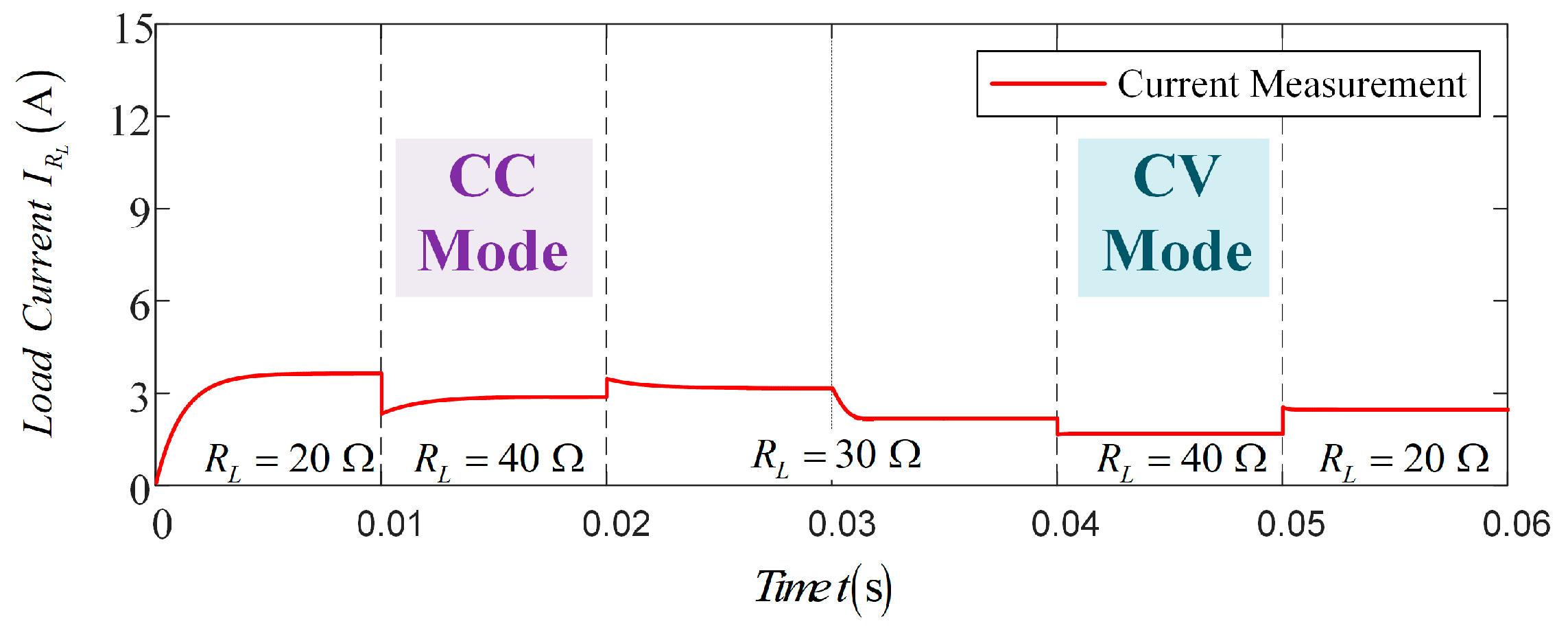
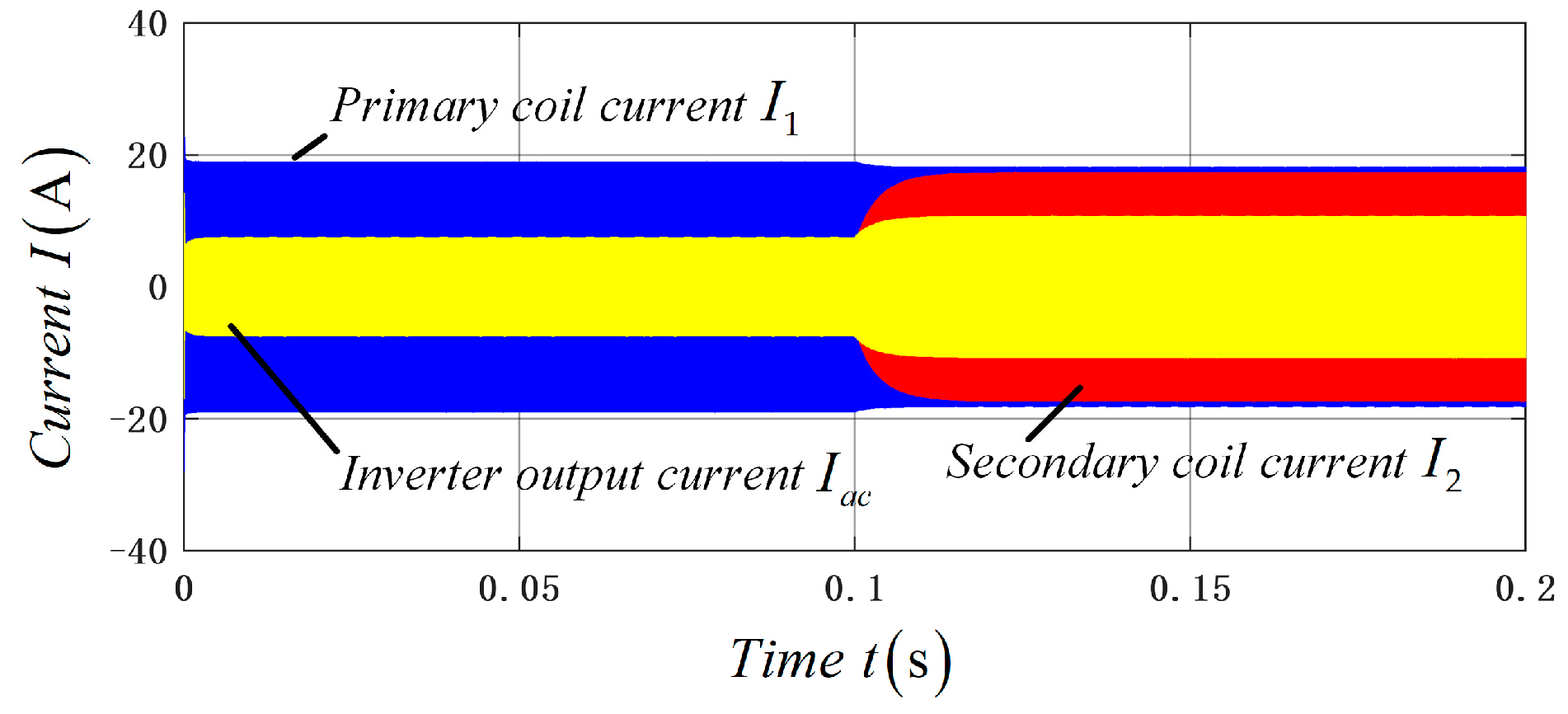
5.1. Results Analysis of Simulation
5.2. Results Analysis of the Experiment
- Compared with Refs. [35,36], a novel hybrid topology is designed to realize the constant output and switch charging state of the system, using fewer compensation components and two switches, and the proposed system parameters design methodology is used to achieve the efficient output of the system under ZPA conditions.
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| LCC-LCL/S | The reconfigurable topology is composed of LCC-LCL and LCC-S. |
| WPT | Wireless power transfer |
| CC | Constant current |
| CV | Constant voltage |
| ZPA | Zero phase angle |
References
- Vinod, M.; Kishan, D.; Kannan, R.; Iqbal, A.; Mohammed Sulthan, S. Primary side control strategies for battery charging regulation in wireless power transfer systems for EV applications. IET Power Electron. 2024, 1–12. [Google Scholar] [CrossRef]
- Liu, S.G.; Ye, Z.X.; Lu, W.B. Electric vehicle dynamic wireless charging technology based on multi-parallel primary coils. IEEE Int. Conf. Electron. Commun. Eng (ICECE) 2018, 12, 120–124. [Google Scholar]
- Liu, X.; Gao, F.; Niu, H.; Sun, G.; Wang, T.; Wang, H. A Series–Parallel Transformer-Based WPT System for 400-V and 800-V Electric Vehicles with Z1 or Z2 Class. IEEE Trans. Power Electron. 2024, 39, 1749–1761. [Google Scholar] [CrossRef]
- Yang, B.; Zhang, Y.; Zhu, C.; Sahoo, S.; Chen, Y.; Mai, R.; He, Z.; Blaabjerg, F. A Design Method to Minimize Detuning for Double-Sided LCC-Compensated IPT System Improving Efficiency Versus Air Gap Variation. IEEE Trans. Power Electron. 2024, 39, 1723–1737. [Google Scholar] [CrossRef]
- Gnanavendan, S.; Selvaraj, S.K.; Dev, S.J.; Mahato, K.K.; Swathish, R.S.; Sundaramali, G.; Accouche, O.; Azab, M. Challenges, Solutions and Future Trends in EV-Technology: A Review. IEEE Access 2024, 12, 17242–17260. [Google Scholar] [CrossRef]
- Pang, S.; Xu, J.Y.; Li, H.Y.; Ma, Q.; Li, X. Dual-Frequency Modulation to Achieve Power Independent Regulation for Dual-Load Underwater Wireless Power Connector. IEEE J. Emerg. Sel. Top. Power Electron. 2023, 11, 2377–2389. [Google Scholar] [CrossRef]
- Mostafa, A.; Wang, Y.; Tangirala, S.; Zhang, H.; Lu, F. A 5 kW Hull-Compatible Inductive Charging System with 360° Folded Spatial Unipolar Coupler for Autonomous Underwater Vehicles (AUVs). IEEE Trans. Ind. Appl. 2023, 59, 7001–7012. [Google Scholar] [CrossRef]
- Shah, I.A.; Zada, M.; Shah, S.A.A.; Basir, A.; Yoo, H. Flexible Metasurface-Coupled Efficient Wireless Power Transfer System for Implantable Devices. IEEE Trans. Microw. Theory Tech. 2024, 72, 2534–2547. [Google Scholar] [CrossRef]
- Xue, Z.; Chau, K.T.; Liu, W.; Hua, Z. Magnetic-Free Wireless Self-Direct Drive Motor System for Biomedical Applications with High-Robustness. IEEE Trans. Power Electron. 2024, 39, 2882–2891. [Google Scholar]
- Pahlavan, S.; Ashtiani, S.J. Rotation-Tolerant Wireless Power Transmission Scheme with Smart Positioning for Cognitive Research on Moving Animals. IEEE Trans. Biomed. Circuits Syst. 2024, 18, 123–130. [Google Scholar] [CrossRef]
- Yang, Y.; Cao, G.; Li, H. A Positioning and Direction-Guided Approach of the WPT System Based on the Triple-U Coil. IEEE Sens. J. 2023, 23, 26473–26485. [Google Scholar] [CrossRef]
- Khan, S.R.; Desmulliez, M.P.Y. Investigation of Capsule Localization using 3-D Printed Spiral WPT Coil. In Proceedings of the 2023 17th European Conference on Antennas and Propagation (EuCAP), Florence, Italy, 26–31 March 2023; pp. 1–5. [Google Scholar]
- Zhou, J.L.; Zhang, B.; Xiao, W.X.; Qiu, D.Y.; Chen, Y.F. Nonlinear parity-time-symmetric model for constant efficiency wireless power transfer: Application to a drone-in-flight wireless charging platform. IEEE Trans. Ind. Electron. 2019, 66, 4097–4107. [Google Scholar] [CrossRef]
- Hannan, M.A.; Hoque, M.D.M.; Hussain, A.; Yusof, Y.; Ker, A.P.J. State-of-the-art and energy management system of lithium-ion batteries in electric vehicle applications: Issues and recommendations. IEEE Access 2018, 6, 19362–19378. [Google Scholar] [CrossRef]
- Wang, D.Y.; Fu, C.W.; Zhao, Q.L.; Hu, T. A PSO-based optimization design of W-type non contact transformer for stable power transfer in DWPT system. IEEE Trans. Ind. Appl. 2022, 58, 1211–1221. [Google Scholar] [CrossRef]
- Sun, Z.; Chi, Y.; Wang, Y.; Guan, Y.; Xu, D. Analysis and Design of a High Frequency Wireless Battery Charging System with Smooth Mode Transition Characteristics. IEEE Trans. Power Electron. 2023, 38, 13273–13285. [Google Scholar] [CrossRef]
- Mai, R.K.; Chen, Y.; Li, Y.; Zhang, Y.Y.; Cao, G.Z.; He, Z.Y. Inductive Power Transfer for Massive Electric Bicycles Charging Based on Hybrid Topology Switching with a Single Inverter. IEEE Trans. Power Electron. 2017, 32, 5897–5906. [Google Scholar] [CrossRef]
- Chen, Y.; Zhang, H.; Park, S.-J.; Kim, D.-H. A Comparative Study of S-S and LCCL-S Compensation Topologies in Inductive Power Transfer Systems for Electric Vehicles. Energies 2019, 12, 1913. [Google Scholar] [CrossRef]
- Yeo, T.-D.; Kwon, D.; Khang, S.-T.; Yu, J.-W. Design of Maximum Efficiency Tracking Control Scheme for Closed-Loop Wireless Power Charging System Employing Series Resonant Tank. IEEE Trans. Power Electron. 2017, 32, 471–478. [Google Scholar] [CrossRef]
- Wu, L.H.; Zhang, B.; Jiang, Y.W. Position-Independent Constant Current or Constant Voltage Wireless Electric Vehicles Charging System without Dual-Side Communication and DC–DC Converter. IEEE Trans. Ind. Electron. 2022, 69, 7930–7939. [Google Scholar] [CrossRef]
- Mo, Y.H.; Wang, Y.; Xiao, J.; Chen, S.N.; Wu, N.; Wu, X.R. Analysis of MC-WPT Analysis in LCC-S Resonant Network Based on Frequency Switching Control. In Proceedings of the 2023 IEEE 5th International Conference on Civil Aviation Safety and Information Technology (ICCASIT), Dali, China, 11–13 October 2023; pp. 235–239. [Google Scholar]
- Yang, L.; Geng, Z.; Jiang, S.; Wang, C. Analysis and Design of an S/PS−Compensated WPT System with Constant Current and Constant Voltage Charging. Electronics 2022, 11, 1488. [Google Scholar] [CrossRef]
- Voottipruex, K.; Mujjalinvimut, E.; Sangswang, A.; Naetiladdanon, S. A Load-Independent Operation of WPT under Frequency Bifurcation for Battery Charging Applications. In Proceedings of the IECON 2020 the 46th Annual Conference of the IEEE Industrial Electronics Society, Singapore, 18–21 October 2020; pp. 2573–2578. [Google Scholar]
- Yang, L.; Ren, L.; Shi, Y.Y.; Wang, M.; Geng, Z. Analysis and Design of an S/S/P-Compensated Three-Coil Structure WPT System with Constant Current and Constant Voltage Output. IEEE J. Emerg. Sel. Top. Power Electron. 2023, 11, 2487–2500. [Google Scholar] [CrossRef]
- Kim, M.; Joo, D.-M.; Lee, B.K. Design and control of inductive power transfer system for electric vehicles considering wide variation of output voltage and coupling coefficient. IEEE Trans. Power Electron. 2019, 34, 1197–1208. [Google Scholar] [CrossRef]
- Cheng, B.; He, L. High-Order Network Based General Modeling Method for Improved Transfer Performance of the WPT System. IEEE Trans. Power Electron. 2021, 36, 12375–12388. [Google Scholar] [CrossRef]
- Li, K.; Gong, Z.; Liu, Q.; Zheng, S.Y.; Liu, J.Y.; Wang, S.Z. A MCR-WPT system based on LCL-S/P hybrid compensation network with CC/CV and maximum power optimization suiting for battery charging. IET Power Electron. 2024, 17, 351–363. [Google Scholar] [CrossRef]
- Qu, X.; Han, H.; Wong, S.; Tse, C.K.; Chen, W. Hybrid IPT topologies with constant current or constant voltage output for battery charging applications. IEEE Trans. Power Electron. 2015, 30, 6329–6337. [Google Scholar] [CrossRef]
- Chen, Y.; Yang, B.; Kou, Z.H.; He, Z.Y.; Cao, G.Z.; Mai, R.K. Hybrid and Reconfigurable IPT Systems with High-Misalignment Tolerance for Constant-Current and Constant-Voltage Battery Charging. IEEE Trans. Power Electron. 2018, 33, 8259–8269. [Google Scholar] [CrossRef]
- Li, Y.; Hu, J.; Liu, M.; Chen, Y.; Chan, K.W.; He, Z.; Mai, R. Reconfigurable Intermediate Resonant Circuit Based WPT System with Load-Independent Constant Output Current and Voltage for Charging Battery. IEEE Trans. Power Electron. 2019, 34, 1988–1992. [Google Scholar] [CrossRef]
- Shen, Z.W.; Zhou, H.Y.; Tang, H.M.; Zhuang, Y.H.; Mao, X.K.; Zhang, Y.M. A Misalignment-Insensitive Wireless Charging System with Constant-Voltage and Constant-Current Charging Capabilities. In Proceedings of the 2023 10th International Conference on Power and Energy Systems Engineering (CPESE), Nagoya, Japan, 8–10 September 2023; 43–48. [Google Scholar]
- Cheng, B.; He, L.; Liu, H. Optimization Method for High-Order Wireless Power Transfer System Considering Gain Constraint and Components Stress. IEEE Trans. Transp. Electrif. 2023. [Google Scholar] [CrossRef]
- Gyimothy, S.; Kaya, S.; Obara, D.; Shimada, M.; Masuda, M.; Bilicz, S.; Pavo, J.; Varga, G. Loss Computation Method for Litz Cables with Emphasis on Bundle-Level Skin Effect. IEEE Trans. Magn. 2019, 55, 1–4. [Google Scholar] [CrossRef]
- Zhang, Y.; Wei, G.; Wang, C.; Yin, Y.; Feng, J.; Na, T.; Song, K.; Zhu, C. A Hybrid Compensation Topology with Constant Current and Constant Voltage Outputs for Wireless Charging System. IEEE Trans. Transp. Electrif. 2023, 9, 2070–2080. [Google Scholar] [CrossRef]
- Hwang, S.-H.; Chen, Y.; Zhang, H.; Lee, K.-Y.; Kim, D.-H. Reconfigurable Hybrid Resonant Topology for Constant Current/Voltage Wireless Power Transfer of Electric Vehicles. Electronics 2020, 9, 1323. [Google Scholar] [CrossRef]
- Guo, X.; Qi, Y.-S.; Liu, L.-Q.; Jia, S.-Y.; Mi, L.-J. Resonant wireless charging scheme. Energy Rep. 2023, 9 (Suppl. S3), 612–621. [Google Scholar] [CrossRef]
- Li, Y.; Hu, J.F.; Chen, F.B.; Liu, S.P.; Yan, Z.T.; He, Z.Y. A New-Variable-Coil-Structure-Based IPT System with Load-Independent Constant Output Current or Voltage for Charging Electric Bicycles. IEEE Trans. Power Electron. 2018, 33, 8226–8230. [Google Scholar] [CrossRef]





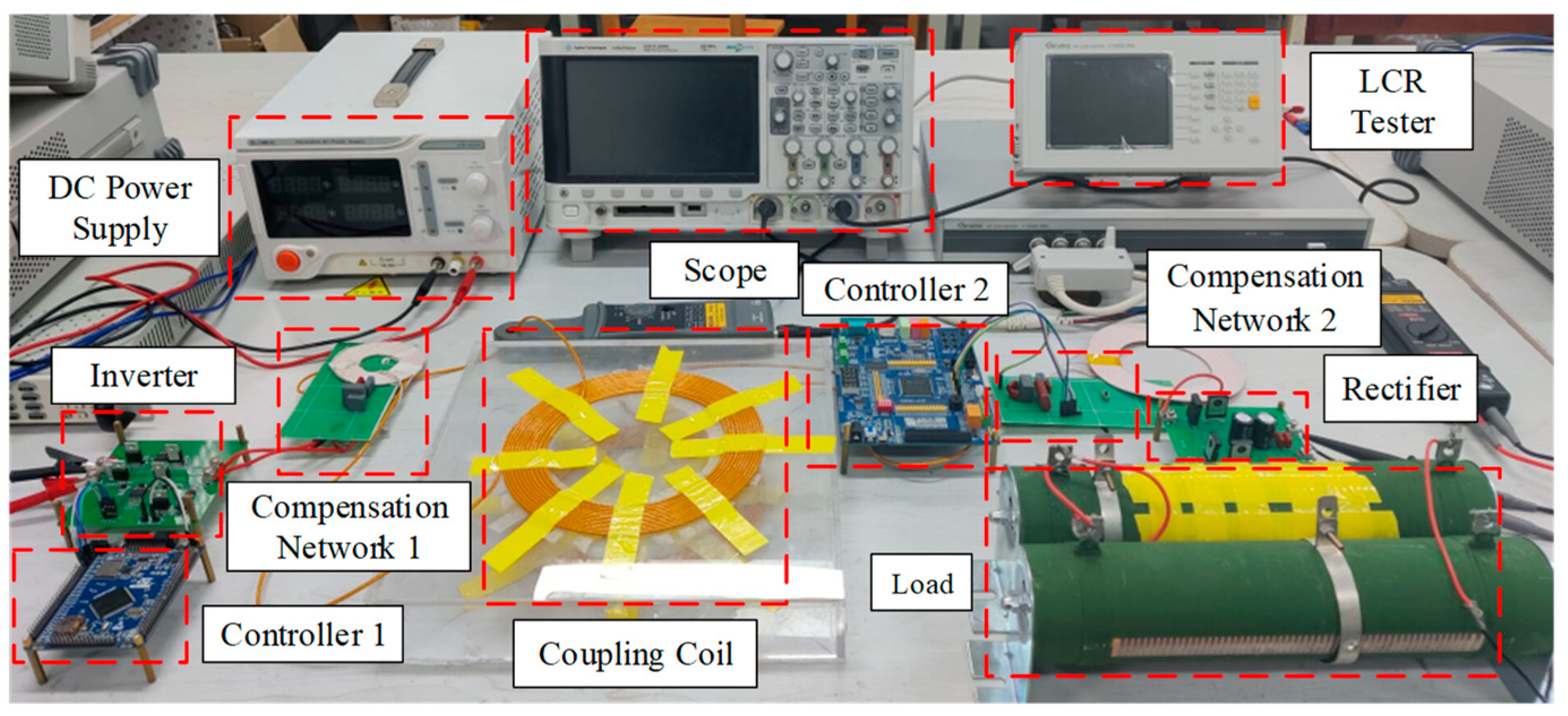
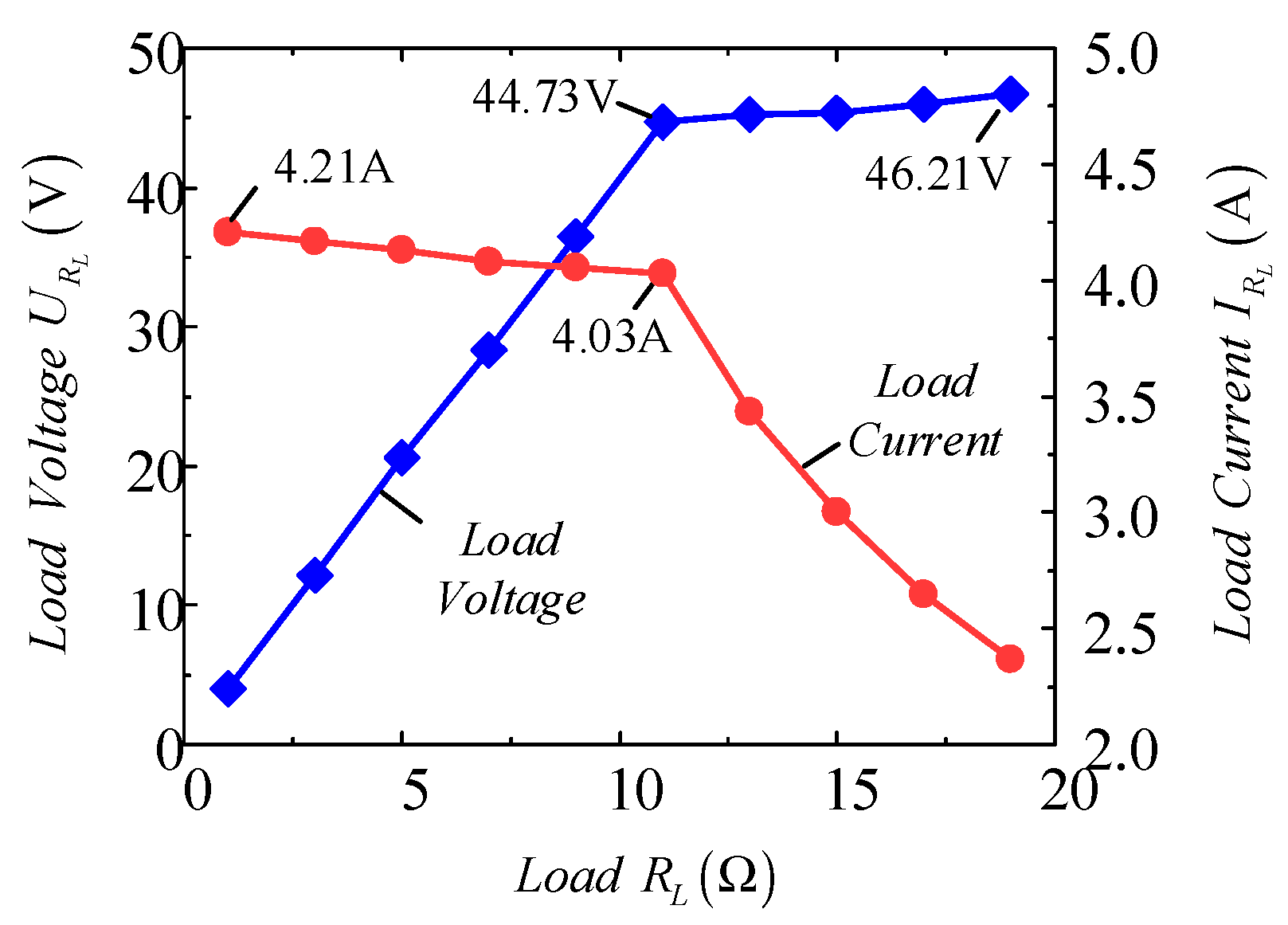



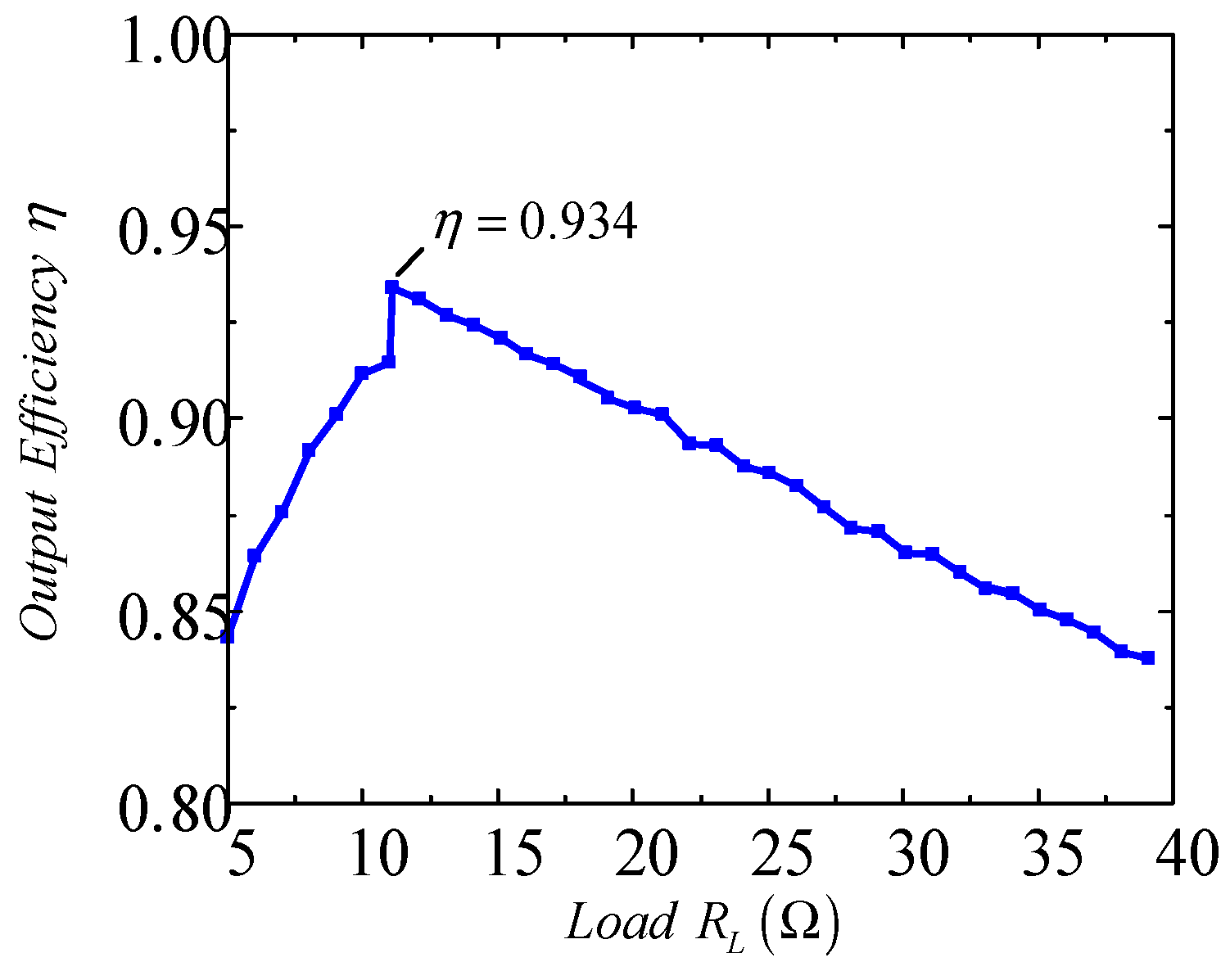
| Symbol | Variable | Value |
|---|---|---|
| DC input | ||
| Operating frequency | ||
| Coil-1 self-inductance | ||
| Coil-2 self-inductance | ||
| Primary side inductance | ||
| Primary side parallel capacitance | ||
| Primary side series capacitance | ||
| Secondary side capacitance | ||
| Secondary side inductance | ||
| Filter capacitance | ||
| Mutual inductance |
| Ref. [29] | Ref. [34] | Ref. [35] | Ref. [36] | Ref. [37] | Proposed | |
|---|---|---|---|---|---|---|
| Topology | Four-coil | Three-coil | S/LCC-S | LCL-LCL/S | Four-coil | LCC-LCL/S |
| Coils | 4 | 3 | 2 | 2 | 4 | 2 |
| Switches | 2 | 1 | 2 | 2 | 2 | 2 |
| Location of switches | Secondary side | Primary side | Primary side | Secondary side | Primary side | Secondary side |
| Primary side compensation components | 4 | 2 | 4 | 2 | 3 | 2 |
| Secondary side compensation components | 8 | 1 | 1 | 2 | 1 | 2 |
| Efficiency | 93.9% | 94.4% | 92.58% | 84% | 91.014% | 93.4% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, T.; Huang, W.; Rao, T.; Chang, Y.; Yan, H. Self-Switching Wireless Power Transfer System Design with Constant Current/Constant Voltage Output Features Based on LCC-LCL/S Topology. Electronics 2024, 13, 1729. https://doi.org/10.3390/electronics13091729
Song T, Huang W, Rao T, Chang Y, Yan H. Self-Switching Wireless Power Transfer System Design with Constant Current/Constant Voltage Output Features Based on LCC-LCL/S Topology. Electronics. 2024; 13(9):1729. https://doi.org/10.3390/electronics13091729
Chicago/Turabian StyleSong, Tingting, Wencong Huang, Tianbiao Rao, Yufang Chang, and Huaicheng Yan. 2024. "Self-Switching Wireless Power Transfer System Design with Constant Current/Constant Voltage Output Features Based on LCC-LCL/S Topology" Electronics 13, no. 9: 1729. https://doi.org/10.3390/electronics13091729
APA StyleSong, T., Huang, W., Rao, T., Chang, Y., & Yan, H. (2024). Self-Switching Wireless Power Transfer System Design with Constant Current/Constant Voltage Output Features Based on LCC-LCL/S Topology. Electronics, 13(9), 1729. https://doi.org/10.3390/electronics13091729








