Neoteric Fuzzy Control Stratagem and Design of Chopper fed Multilevel Inverter for Enhanced Voltage Output Involving Plug-In Electric Vehicle (PEV) Applications

Abstract

The utilization of plug-in electric vehicles (PEV) has started to garner more attention worldwide considering the environmental and economic benefits. This has led to the invention of new technologies and motifs associated with batteries, bidirectional converters and inverters for Electric Vehicle applications. In this paper, a novel design and control of chopper circuit is proposed and configured with the series and parallel connection of the power electronic based switches for two-way operation of the converter. The bidirectional action of the proposed converter makes it suitable for plug-in electric vehicle applications as the grid is becoming smarter. The DC–DC converter is further interfaced with the designed multilevel inverter (MLI). The reduced switches associated with the novel design of MLI have overcome the cons associated with the conventional inverters in terms of enhanced performance in the proposed design. Further, novel control strategies have been proposed for the DC–DC converter based on Proportional Integral (PI) and Fuzzy based control logic. For the first time, the performance of the entire system is evaluated based on the comparison of proposed PI, fuzzy, and hybrid controllers. New rules have been formulated for the Fuzzy based controllers that are associated with the Converter design. This has further facilitated the interface of bidirectional DC–DC converter with the proposed MLI for an enhanced output voltage. The results indicate that the proposed hybrid controller provides better performance in terms of voltage gain, ripple, efficiency and overall aspects of power quality that forms the crux for PEV applications. The novelty of the design and control of the overall topology has been manifested based on simulation using MATLAB/SIMULINK.

1. Introduction

Plug-in electric vehicles (PEV)-based technology has gained a lot of interest considering the environmental concerns to reduce pollutant emissions and its contribution towards green energy. The recent development in the concepts of smart grid and microgrid, smart meters, and smart homes anticipates PEVs to emerge as a smart solution for transportation [1,2,3,4,5].

It could be seen from Figure 1 that the Smart Grid comprises of several domains. One such domain of IEEE 2030 Interoperability Standard is the effective interaction of renewable energy resources and PEVs with the power system network [6]. The electric vehicle systems are mainly battery-based and plug-in topologies. Usually, a battery is incorporated for absorbing the regenerative breaking and to achieve good transient performance. In order to interface the PEV’s with the grid, converters and inverters are required. The performance of EV’s are enhanced in terms of power quality and efficiency when they are fed with a controlled high gain converter topologies. The high gain (step-up) and isolation between source and load segments in DC–DC converters can be easily achieved by using high frequency isolated topologies. But the drawbacks associated with these kinds of topologies are numerous. To overcome the limitations, non-isolated converters are implemented [7]. These types of converters utilize mutually coupled inductors instead of heavy and highly rated transformers which lead to losses. The coupled inductor configurations have less input current ripple and possess better internal characteristics. Apart from this, many research works are being carried out involving DC–DC converters, such as bidirectional circuits, dual active bridge, and voltage-doubler cell that provide better output performance [8,9,10]. The controlled converters are more beneficial in terms of gain, ripple and fast operations. Papers in literature have implemented converters based on conventional controllers like PID [11,12]. Multilevel inverter (MLI) topologies have gained more attention due to its advantages such as high efficiency, reduced harmonics (enhanced power quality), and better electromagnetic interference (EMI). MLIs are classified into many types based on the connection and devices used in the configuration [13].

In this paper, there are several contributions in the form of research. Several new controllers based on PI and Fuzzy controllers are proposed for the DC–DC converter. Further, a hybrid controller has also been proposed for the DC–DC converter. The DC–DC converter is further fed to a newly developed MLI with a single voltage source and reduced power electronic switches. The enhanced performance in terms of power quality and output voltage from the proposed design and controllers forms the quintessence of PEV applications before being interfaced with the grid. So far, none of the research work in literature have discussed about developing new controllers for PEV applications towards enhanced performance of the converter output. The enhanced performance in the output voltage plays a vital role in the synchronization of PEVs with the smart grid. For the first time, the performance comparison of the entire converter system with the novel proposed PI, fuzzy and hybrid controllers are manifested in this paper. New rules have been devised for the Fuzzy based controllers. Research outcome of this paper in the form of simulation using MATLAB/SIMULINK clearly depicts that the proposed novel controllers for novel converter design provides better performance in terms of voltage gain, ripple, efficiency and overall aspects of power quality.

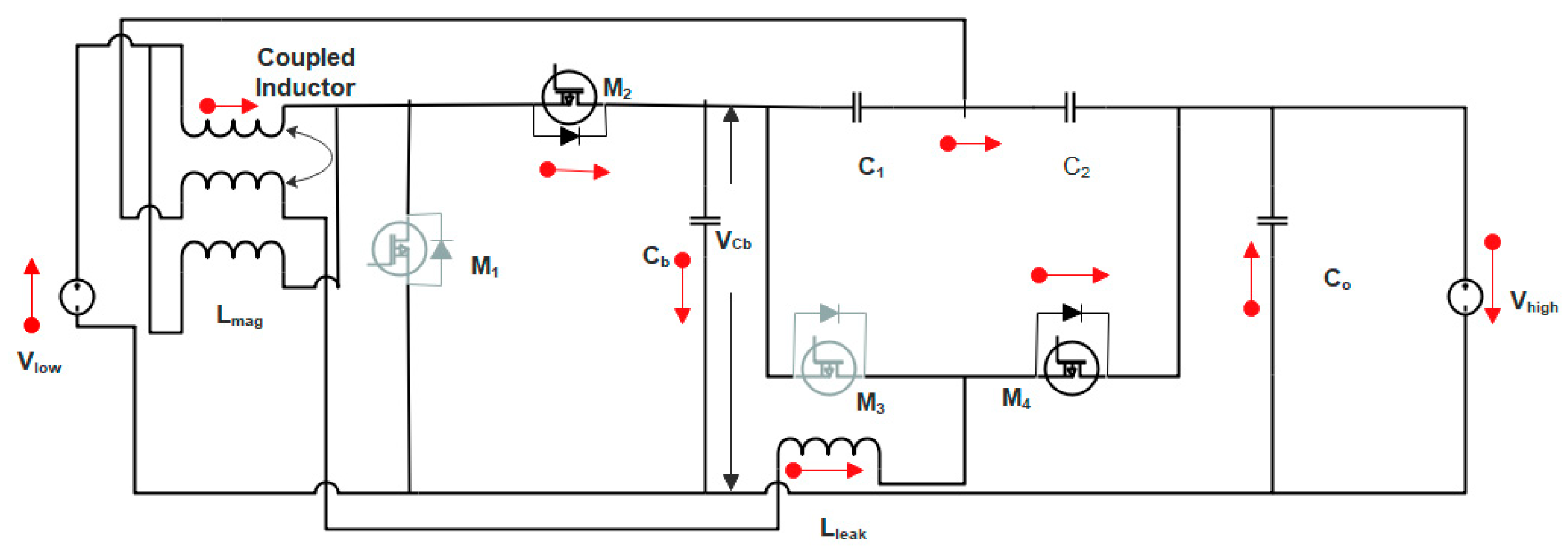
2. Chopper Configuration

The circuit topology of the proposed converter comprises of a DC source input voltage V_s, the power MOSFET switches M₁-M₄, capacitors C_b, C₁, and C₂, and the coupled inductor with primary and secondary winding, N_p and N_s respectively. The model of the coupled inductor bidirectional DC–DC converter also has a magnetizing inductor L_mag and a leakage inductor L_leak. The coupled inductor configuration provides high voltage gain and the reduced current ripple. The high conversion gain (boost mode) can be achieved by voltage doubler cell mechanism [14] based on the capacitor C_b. The proposed chopper circuit is shown in Figure 2.

The voltage conversion is improved by inserting the secondary winding of coupled inductor into the voltage doubler cell. The primary winding along with the magnetizing inductor i.e, L_mag acts as a filter for the converter. The switches M₁, M₂, M₃, and M₄ are connected in series and form a switch bridge between the high voltage side V_h and battery voltage V_b. The two bridges are divided by a common capacitor C_b. The segment of V_h and C_b is the conventional buck-boost bidirectional converter and the latter is a dual active half bridge (DAHB) bidirectional converter. Due to the minimization of circulating current and soft switching mechanism of switches, highest efficiency can be perceived. The voltage matching can be made easily with this proposed configuration. Since the output voltage of V_cb is fed to the input terminal of DAHB bidirectional converter (BDC), the voltage control on the two windings of coupled inductor is achieved. It can be mathematically denoted as follows:

$$\frac{Vcb}{\left(Vh-Vcb\right)}=\frac{N1}{N2}=\frac{1}{n}$$

(1)

The significance of the converter circuit used in this work is listed below:

• Since, the voltage on the two segments of the converter are matched with that of the turns ratio of coupled inductor, the current circulating across the switches are reduced and soft switching is also accomplished.
• High voltage conversion is achieved with the transformer less configuration.
• High power density is attained due to the winding of inductors on the same core.

2.1. Operating Modes

The proposed bidirectional DC–DC converter has two operating modes, i.e., step-down mode and step-up mode based on whether the PEV is charging or discharging respectively. The switching states are as follows.

2.1.1. Mode I (t₀ < t < t₁)

Due to the negative currents i_b and i_Lleak the switch M₂ and M₄ is turned ON. The switch M₂ gets turned off at t₀ and the parasitic diode of M₁ is ON due to the negative current of i_b. The equivalent circuit for mode I is shown in Figure 3.

2.1.2. Mode II (t₁ < t < t₂)

During mode 1 [t₀, t₁] and mode 2 [t₁, t₂], the magnetizing and leakage currents increases linearly due to the positive voltage on the corresponding inductors. The equivalent circuit for mode II is shown in Figure 4.

2.1.3. Mode III (t₂ < t < t₃)

In this mode, M₄ is turned off at t₂, the parasitic diode of M₃ is ON due to the positive value of leakage current. The equivalent circuit for mode III is shown in Figure 5.

2.1.4. Mode IV (t₃ < t < t₄)

During mode 3 [t₂, t₃] and mode 4 [t₃, t₄], the battery is discharged and supplies power to the load. So, the magnetizing inductor is charged by V_b, and i_Lmag keeps increasing linearly. In this stage the battery power is supplied to the high voltage side, and C_b is charged while C_o is discharged. The equivalent circuit for mode IV is shown in Figure 6.

2.1.5. Mode V (t₄ < t < t₅)

In this mode M₁ is turned off at t₄, and the parasitic diode of M₂ is ON due to the positive values of magnetizing and leakage currents. The equivalent circuit for mode VII is shown in Figure 7.

2.1.6. Mode VI (t₅ < t < t₆)

During mode 5 [t₄, t₅] and mode 6 [t₅, t₆], both magnetizing and leakage currents decreases linearly. Both the battery and the energy stored in L_mag are discharged and supply power to the capacitor C₁. The equivalent circuit for mode VI is shown in Figure 8.

2.1.7. Mode VII (t₆ < t < t₇)

At t₆, M₃ is turned off and the parasitic diode of M₄ conducts because of the negative value of leakage current. The equivalent circuit for mode VII is shown in Figure 9.

2.1.8. Mode VIII (t₇ < t < t₈)

In mode 7 [t₆, t₇] and mode 8 [t₇, t₈], the battery is discharged and supply power to the high-voltage side, and C₂ is discharged while C_o is charged. The equivalent circuit for mode VIII is shown in Figure 10.

2.2. Voltage Equations

2.2.1. Voltage Conversion Ratio

The Voltage conversion ratio is given by the following equation,

$$\mathrm{G}=\frac{\mathrm{Vh}}{\mathrm{Vb}}=\frac{\left(\mathrm{n}+1\right)}{\left(1-\mathrm{D}\right)}$$

(2)

where,

G—Voltage gain

V_h—High voltage

V_b—Battery voltage

n—Turns ratio

D—Duty ratio

2.2.2. Voltage across Capacitance

The voltage across various capacitances is,

$${\mathrm{Vc}}_{\mathrm{b}}=\frac{Vb}{\left(1-D\right)}$$

(3)

$${\mathrm{Vc}}_1=\frac{\left(1-D\right)}{\left(Vh-Vcb\right)}=nVb$$

(4)

$${\mathrm{Vc}}_2=D\left(Vh-Vcb\right)=\frac{D}{\left(1-D\right)}nVb$$

(5)

where,

V_cb—Voltage across capacitor C_b

V_c1—Voltage across capacitor C₁

V_c2—Voltage across capacitor C₂

D—Duty ratio

n—Turns ratio

3. Multilevel Inverter

The proposed nine level inverter consists of two circuits, one is a developed SC circuit (DSCC) at the frontend of the inverter and another circuit is a conventional H-bridge circuit. The H-bridge circuit connected at the backend of the inverter is used for producing the negative voltage levels of the output [15,16,17]. The circuit configuration of multilevel inverter is shown in Figure 11.

The hybrid multilevel inverter can be operated based on the following switching sequence and it can produce nine level output. The switching sequences are given in

Table 1. Switching combinations for multilevel inverter (MLI).

Vo	S1	S2	S3	S4	S5	S6	S7	S8	S9
2Vdc	High	Low	High	High	High	High	High	Low	Low
3Vdc/2	High	Low	Low	High	High	High	Low	Low	High
Vdc	High	Low	Low	High	High	Low	Low	High	Low
Vdc/2	High	Low	Low	High	Low	Low	Low	High	High
0	Low	High	Low	High	Low	Low	Low	High	High
–Vdc/2	Low	High	High	Low	Low	Low	Low	High	High
–Vdc	Low	High	High	Low	High	Low	Low	High	Low
–3Vdc/2	Low	High	High	Low	High	High	Low	Low	High
2Vdc	Low	High	High	Low	High	High	High	Low	Low

.

• To acquire the voltage level of +2Vdc the switches S1, S4 of the H-bridge circuit and the switches S5, S6, and S7 of the DSCC circuit is held High.
• To acquire the voltage level of +3Vdc/2 the switches S1, S4 of the H-bridge circuit and the switches S5, S6, and S9 of the DSCC circuit is held High.
• The voltage level of +Vdc is achieved by holding the switches S1 and S4 of the H-bridge circuit and the switches S5 and S8 of the DSCC circuit at High position.
• To obtain the next level of multilevel output i.e., +Vdc/2, the switches S1 and S4 of the H-bridge circuit and the switches S8 and S9 of the DSCC circuit is held High.
• The zero level voltage is obtained by holding the switches S2 and S4 of the H-bridge circuit and the switches S8 and S9 of the DSCC circuit at High position.
• To achieve the voltage level of –Vdc/2, the switches S2 and S3 of the H-bridge circuit and the switches S8 and S9 of the DSCC circuit is held High.
• The negative cycle of V_dc (i.e., –Vdc) is achieved by holding the switches S2 and S3 of the H-bridge circuit and the switches S5 and S8 of the DSCC circuit is held High.
• To obtain the voltage level of –3Vdc/2, the switches S2 and S3 of the H-bridge circuit and the switches S5, S6, and S9 of the DSCC circuit is held High.
• To acquire the voltage level of –2Vdc the switches S2 and S3 of the H-bridge circuit and the switches S5, S6, and S7 of the DSCC circuit is held High.

4. Controller Circuit

4.1. PI Controller

The PI controller is one of the familiar techniques used for controlling a system. It is mainly used to eliminate the steady state error. This type of controller produces an output signal containing two terms, one proportional to the operating signal and the other proportional to its integral [18]. The block diagram of the proposed system with PI controller for both voltage and current control is shown in Figure 12.

The closed loop system shown in above Figure 12, regulates the voltage and current by comparing the actual value with the desired value. In this system, the high voltage (V_h) is compared with a reference voltage and an error is generated. This error signal is given to the controller block where the control process is carried out and a steady state value is generated. Similarly, the control of current block is carried out. Some of the drawbacks of this system are high rise time and settling time, less speed of the response. To overcome this, fuzzy logic control is introduced because of its simplicity, ease of design and ease of implementation. The above mentioned drawbacks are comparatively less in a fuzzy based system. The designed values for PI controller is given in

Table 2. Design parameters for PI controller.

Parameter	Kp	Ki
Range	1.5	1

.

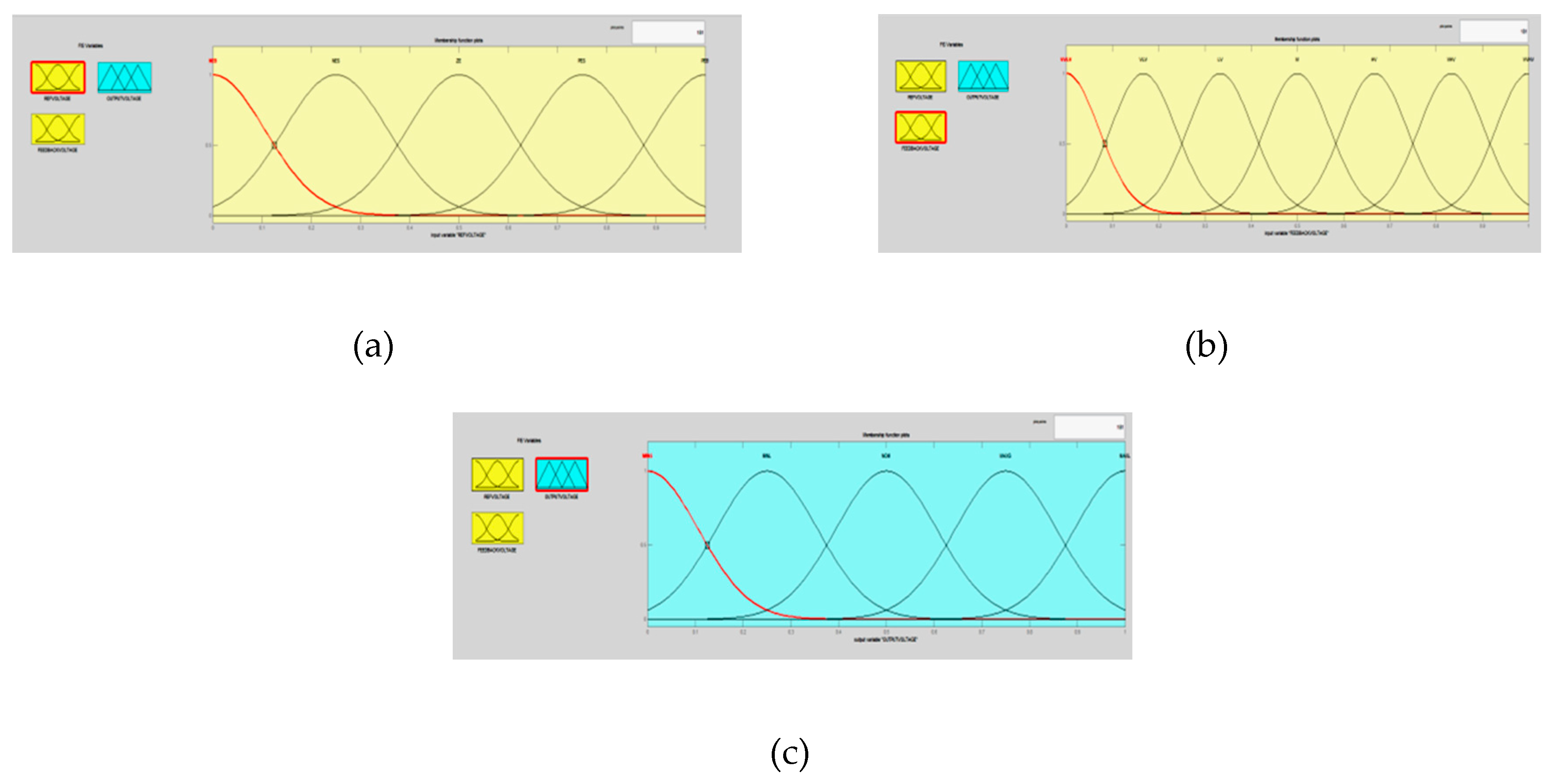
4.2. Proposed Fuzzy Logic Based Controller

Fuzzy logic control (FLC) is a technique to epitomize human-like thinking into a control system. It works based on the concept of human deductive thinking to infer conclusions from the past experience and is designed to emulate the former. The typical control system is usually modeled with a physical reality whereas the fuzzy controller incorporates equivocal human logic into programs [19,20,21,22]. This fuzzy logic is applicable for systems which is difficult to represent by mathematical models. The basic block diagram of fuzzy logic controller is shown in Figure 13.

4.2.1. Rule 1

In the first rule, two input variables such as ‘error (e)’ and ‘change in error (ce)’ are taken along with an output variable ‘Output1’. Triangular membership function is used for all the variables in the given fuzzy set. For input 1 and input 2 (i.e.) error and change in error, the linguistic variables Negative (N), Zero (Z), and Positive (P) are assumed. For output, the variables are taken as Low (L), Medium (M) and High (H). The proposed rule is given in

Table 3. Rules for fuzzy—Rule 1.

	e	N	Z	P
ce
N		L	L	M
Z		L	M	H
P		M	H	H

.

The input and output membership functions for the above-mentioned rule is given below in Figure 14 a–c respectively.

The overall rule viewer is shown in Figure 15.

The surface view of rule 1 is shown in Figure 16.

Figure 16, shows the 3D view of the proposed fuzzy rule. In this plot, the error and change in error are taken along the x and y-axis whereas the output is taken along the z-axis.

4.2.2. Rule 2

In this rule, two input variables such as ‘error (e)’ and ‘change in error (ce)’ are taken along with an output variable ‘Output1’. In this case, combinations of two different membership functions are used i.e., Trapezoidal MF for Input 1 and Gauss MF for Input 2. The output membership function is given by Triangular MF. For input 1 and input 2 (i.e.) error and change in error, the linguistic variables Negative Big (NB), Negative Small (NS), Zero (Z), Positive Small (PS), and Positive Big (PB) are assumed. For output, the variables are taken as Very Large (VL), Large (L), Medium (M), High (H), and Very High (VH). The proposed rule is given in

Table 4. Rules for fuzzy—Rule 2.

	e	NB	NS	Z	PS	PB
ce
NB		VL	VL	L	M	M
NS		VL	VL	L	M	M
Z		L	L	M	M	VH
PS		M	M	H	VH	VH
PB		M	M	H	VH	VH

.

The input and output membership functions for the above-mentioned rule is given below in Figure 17.

The overall rule viewer is shown in Figure 18.

The surface view of rule 2 is shown in Figure 19.

Figure 19, shows the 3D view of the proposed fuzzy rule. In this plot, the error and change in error are taken along the x and y-axis whereas the output is taken along the z-axis.

4.2.3. Rule 3

In this rule, two input variables such as ‘error (e) (Reference Voltage) ’ and ‘change in error (ce) (Feedback Voltage)’ are taken along with an output variable ‘Output1’. Here Gauss MF is used for all the three cases. For input 1 and input 2 (i.e.) error and change in error, the linguistic variables Negative Error Big (NE-B), Negative Error Small (NE-S), Zero Error (ZE), Positive Error Small (PE-S) and Positive Error Big (PE-B) are assumed. For output, the variables are taken as Very Very Low Voltage (VVLV), Very Low Voltage (VLV), Low Voltage (LV), Medium (M), High Voltage (HV), Very High Voltage (VHV) and Very Very High Voltage (VVHV). The proposed rule is given in

Table 5. Rules for fuzzy—Rule 3.

	R.V	NE-B	NE-S	ZE	PE-S	PE-B
F.V
VVLV		MINL	MINL	MINL	MINS	MINS
VLV		MINL	MINL	MINS	MINS	NOM
LV		MINL	MINL	MINS	NOM	MINS
M		MINS	MINS	NOM	MAXS	MAXS
HV		MINS	NOM	MAXS	MAXS	MAXL
VHV		NOM	MAXS	MAXS	MAXL	MAXL
VVHV		MAXS	MAXS	MAXL	MAXL	MAXL

.

The input and output membership functions for the above-mentioned rule is given below in Figure 20.

The overall rule viewer is shown in Figure 21.

The surface view of rule 3 is given in Figure 22.

Figure 22, shows the 3D view of the proposed fuzzy rule. In this plot, the error (reference voltage) and change in error (feedback voltage) are taken along the x-axis and y-axis whereas the output voltage is taken along the z-axis.

4.2.4. Rule 4

In this rule, two input variables such as ‘error (e) (Reference Current)’ and ‘change in error (ce) (Feedback Current)’ are taken along with an output variable ‘Output1’. In this case, combinations of two different membership functions are used i.e., Trapezoidal MF for Input 1 and Gauss MF for Input 2. The output membership function is given by g-bell MF. For input 1 and input 2 (i.e) error and change in error, the linguistic variables such as −2.5, −1.5, 0, 1.5, 2.5 are assumed. For output, the variables are taken as −1.01, −0.31, −0.01, 0.29, 0.99. The proposed rule is given in

Table 6. Rules for fuzzy—Rule 4.

	RC	−2.5	−1.5	0	1.5	2.5
FC
−2.5		−1.01	−1.01	−1.01	−0.31	−0.01
−1.5		−1.01	−1.01	−0.31	−0.01	0.29
0		−1.01	−0.31	−0.01	0.29	0.99
1.5		−0.31	−0.01	0.29	0.99	0.99
2.5		−0.01	0.29	0.99	0.99	0.99

.

The input and output membership functions for the above mentioned rule is given below in Figure 23.

The overall rule viewer plot is shown in Figure 24.

The surface plot for rule 4 is given in Figure 25.

Figure 25 shows the 3D view of the proposed fuzzy rule. In this plot, the error (reference current) and change in error (feedback current) are taken along the x and y-axis whereas the output is taken along the z-axis.

The schematic of proposed fuzzy logic controller is shown in Figure 26.

Individual controllers for voltage and current are designed using fuzzy technique. The reference value is compared with the feedback value and an error is generated. This error is fed to the fuzzy inference engine along with the change in error value for fuzzification process. Finally, the fuzzified value is generated and is converted to crisp set by defuzzification process before feeding it to the converter system.

4.3. Novel Hybrid Controller

The hybrid controller is the combination of PI and Fuzzy Logic Controller. In this paper, the four different configurations of hybrid controllers are proposed:

4.3.1. Proposed Hybrid Controller-1

In this configuration, the voltage control of the converter is done with a hybrid controller made up of Fuzzy and PI controller. The block diagram of hybrid controller-1 is shown in Figure 27.

The reference voltage is compared with a feedback voltage and an error signal is generated. The generated error signal and change in error signal is fed to the fuzzy controller. Both the signals are fuzzified based on the proposed rule base and the defuzzified output is given to the PI controller. The signal generated by the PI controller is given to the MOSFET switches.

The current control is done using a PI controller and the diagram is shown in Figure 28.

4.3.2. Proposed Hybrid Controller-2

In hybrid controller-2, Individual Fuzzy controllers are used for error and change in error signals. The fuzzifiedoutput is converted into a crisp value by taking a product of the output signal and the error. The same process is carried out for the change in error signal and both the signals are compared and fed to a PI controller for further accuracy. The block diagram for voltage control is shown in Figure 29.

The current is controlled with the help of a PI controller. The control process is depicted in below Figure 30.

4.3.3. Proposed Hybrid Controller-3

The third configuration of hybrid controller implements fuzzy logic for both the voltage and current control. The block diagram of both the voltage and current control is shown in Figure 31, respectively.

Figure 32 shows the control block for the current using fuzzy logic technique.

4.3.4. Proposed Hybrid Controller-4

This configuration is similar to that of type-2 hybrid controller. In voltage control, the fuzzified output of both error and change in error signals are converted into a crisp value by taking a product of the output signal and the input error signals. These signals are compared and fed to a PI controller for further accuracy. Here, the current is also controlled by fuzzy based system. The block diagram for each control technique is shown in Figure 33 and Figure 34 respectively.

5. Simulation Result

The proposed chopper and inverter circuit is tested with different proposed controllers based on PI and Fuzzy techniques under a simulation environment using MATLAB/SIMULINK. The simulation parameters are shown in

Table 7. Simulation parameters.

Specifications
Input Voltage (Vb)	40 V
Output Voltage (Vh)	300 V
Duty ratio (D)	0.4–0.6
Turns Ratio (n)	3
Leakage Inductance (Lleak)	25 µH
Magnetising Inductance (Lmag)	20 µH
Ca1,Ca2	10 µF
Ch	470 µF
Cb	2.5 µF

.

Using the above values, the simulation of converter circuit is done in MATLAB/SIMULINK and it is shown in Figure 35.

Similarly, the multilevel inverter is also designed in MATLAB environment and is shown in Figure 36.

5.1. Bidirectional Converter and Multilevel Inverter with PI Controller

The output voltage and ripple waveforms for DC–DC converter with PI control are shown in Figure 37 and Figure 38 respectively.

From Figure 37, it is clear that the output voltage is maintained constant at a value of 279.8 V and has nominal output voltage ripple.

Figure 38, clearly shows that the ripple of output voltage is within the permissible limit. It is measured to be 4 V

The nine level output voltage waveform for multilevel inverter interfaced with the PI controlled bidirectional dc/dc converter is shown in Figure 39.

Figure 39 shows a nine level stepped output with a voltage of 551.2 V. It could be clearly witnessed that the inverter has a stable output thereby manifesting the efficacy of the proposed controller.

5.2. Bidirectional Converter and Multilevel Inverter with Fuzzy Controller

The converter provides a better voltage profile when is operated with the proposed fuzzy logic controller. The corresponding result is shown in Figure 40.

The fuzzy based BDC gives an output voltage of 283.4 V and it is also stable for a given time period. It also has reduced ripple voltage around 3.5 V which is lesser than the nominal value. The ripple waveform is shown in Figure 41.

From Figure 42, it is clear that fuzzy based controller gives better voltage profile compared to conventional controllers.

5.3. Bidirectional Converter and Multilevel Inverter with Hybrid Controller-1

This novel controller comprises of both PI and Fuzzy together to form a hybrid configuration. The reference voltage and the actual voltage is compared, and an error signal is generated. The generated error signal along with the change in error signal is given to the fuzzy controller in order to reduce the ripple and enhance the output voltage. The enhanced output from fuzzy block set is further controlled using a PI controller to achieve a better output voltage.

The voltage waveform for Hybrid controller-1 is shown in Figure 43. It gives and enhanced output voltage at a range of 297.7 V with reduced ripple content.

Figure 44 shows a ripple waveform of hybrid controller topology with a value of 3.3 V.

Figure 45 indicates that the output voltage of MLI is high when compared to the above investigated control circuits. It is in the range of 587.17 V.

5.4. Bidirectional Converter and Multilevel Inverter with Hybrid Controller-2

The output voltage waveform for bidirectional DC–DC converter with Hybrid controller-4 shown in Figure 46 provides an output voltage of 300.3 V.

Figure 47 clearly indicates that the voltage ripple at the output side of the converter is maintained low at a range of 2.9 V.

The Figure 48 shows an output voltage graph at a range of 574.5 V. The proposed hybrid controller-2 gives better results when compared to conventional PI and basic Fuzzy topologies.

5.5. Bidirectional Converter and Multilevel Inverter with Hybrid Controller-3

This type of controller gives better performance in terms of high voltage and ripple reduction when compared to the above-mentioned configurations. The voltage waveform is shown in Figure 49.

Figure 49 clearly depicts that this type gives a higher voltage of 303.4 V with very low ripple content. The ripple is calculated to be 2.5 V, which is very low when compared to the other proposed controller outputs. It is shown in Figure 50.

Figure 51 clearly shows that, the hybrid controller 3 gives a very good gain in the voltage level of the output signal. It is measured to be 592.8 V and it is maintained stable.

5.6. Bidirectional Converter and Multilevel Inverter with Hybrid Controller-4

Figure 52 shows the output voltage waveform for bidirectional DC–DC converter with hybrid controller-4 with an output voltage of 301.9 V.

Figure 53 shows that the output voltage ripple is at nominal range and it is measured to be 2.8 V.

Figure 54 shows an output voltage waveform for multilevel inverter interfaced with hybrid controller-4-based BDC. In this type, a maximum voltage of 578.3 V is achieved.

6. Discussion

The performance of converter is analyzed with different controller circuits and the results are compared. From the results, it is understood that the proposed Hybrid controller circuits operates in a better way when connected to a converter circuit. This controlled converter is also tested with multilevel inverter and the output voltages are measured and tabulated. A comparison is made among all the controllers and the results are tabulated in

Table 8. Comparison of proposed controller circuits for BDC interfaced with MLI.

PARAMETERS	PI Based BDC with MLI	Fuzzy Based BDC with MLI	Hybrid Controller for BDC interfaced with MLI
			Type I	Type II	Type III	Type IV
Output Voltage (BDC) (in Volts)	279.9	282.6	297.7	300.3	303.4	301.9
Ripple Voltage(BDC) (in Volts)	4	3.5	3.3	2.9	2.5	2.8
Output voltage for MLI (in Volts)	551.2	556.4	589.17	574.5	592.8	578.4

.

From the above table, it is clear that the Hybrid controller (Type III) produces a voltage of 303.4 V which is higher when compared to the conventional controllers. The voltage ripple is also reduced to very low level. The output of the multilevel inverter has also gained a huge variation and it is measured to be 592.8 V.

The output performance characteristics of converter based on PI and fuzzy in terms of time domain are given in

Table 9. Performance parameters.

Parameter	PI Based Converter	Fuzzy Based Converter
Rise Time	0.01 s	0.001 s
Settling Time	0.6 s	0.3 s
Peak Time	0.06 s	0.04 s

.

7. Conclusions

In this work, a novel MLI fed with a controlled bidirectional chopper circuit is designed. The converter employed in this system gives an enhanced output with the help of a voltage doubler mechanism. The converter output is further controlled, and a signal is generated with reduced ripple. For the first time, the performance of the entire system is evaluated based on the comparison of proposed PI, fuzzy, and hybrid controllers based on new rules devised for the fuzzy controllers. It could be well witnessed from the results that the proposed hybrid controller provides better performance in terms of voltage gain, ripple, efficiency, and overall aspects of power quality that forms the crux for PEV applications.

8. Future Scope

The research work presented in this paper will not only serve as a reference for several researchers working in the smart grid domain but will serve as a benchmark for employing hybrid based smart/intelligent controllers for ancillary services provided by smart inverters in the form of future work.