The Single-Active-Electron Approximation with Angular-Momentum-Dependent Potentials: Application to the Helium Atom
Abstract
:1. Introduction
2. Potentials for Helium in the ℓ-SAE Approximation
3. TISE-Based Atomic Properties
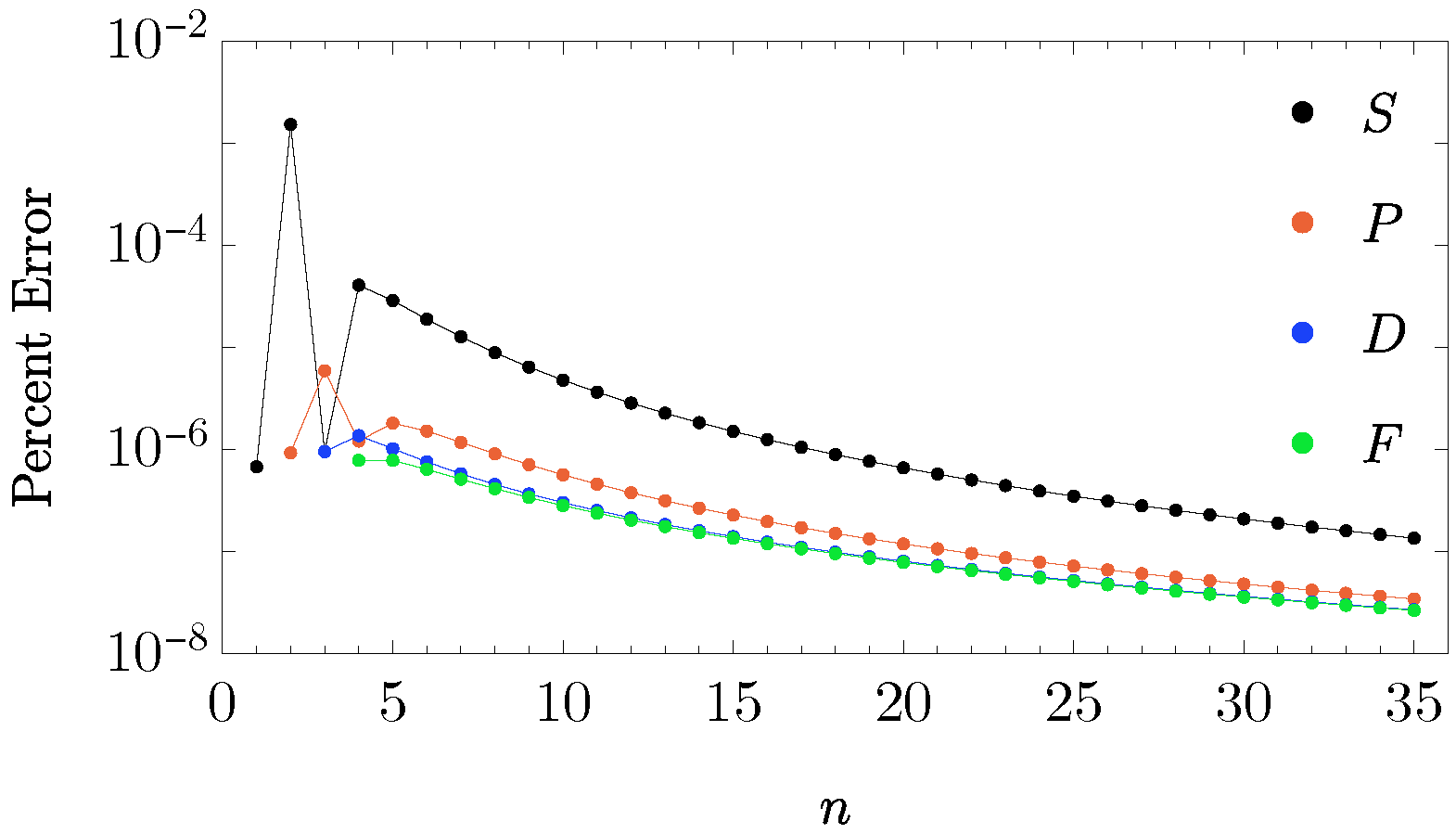
3.1. Oscillator Strengths
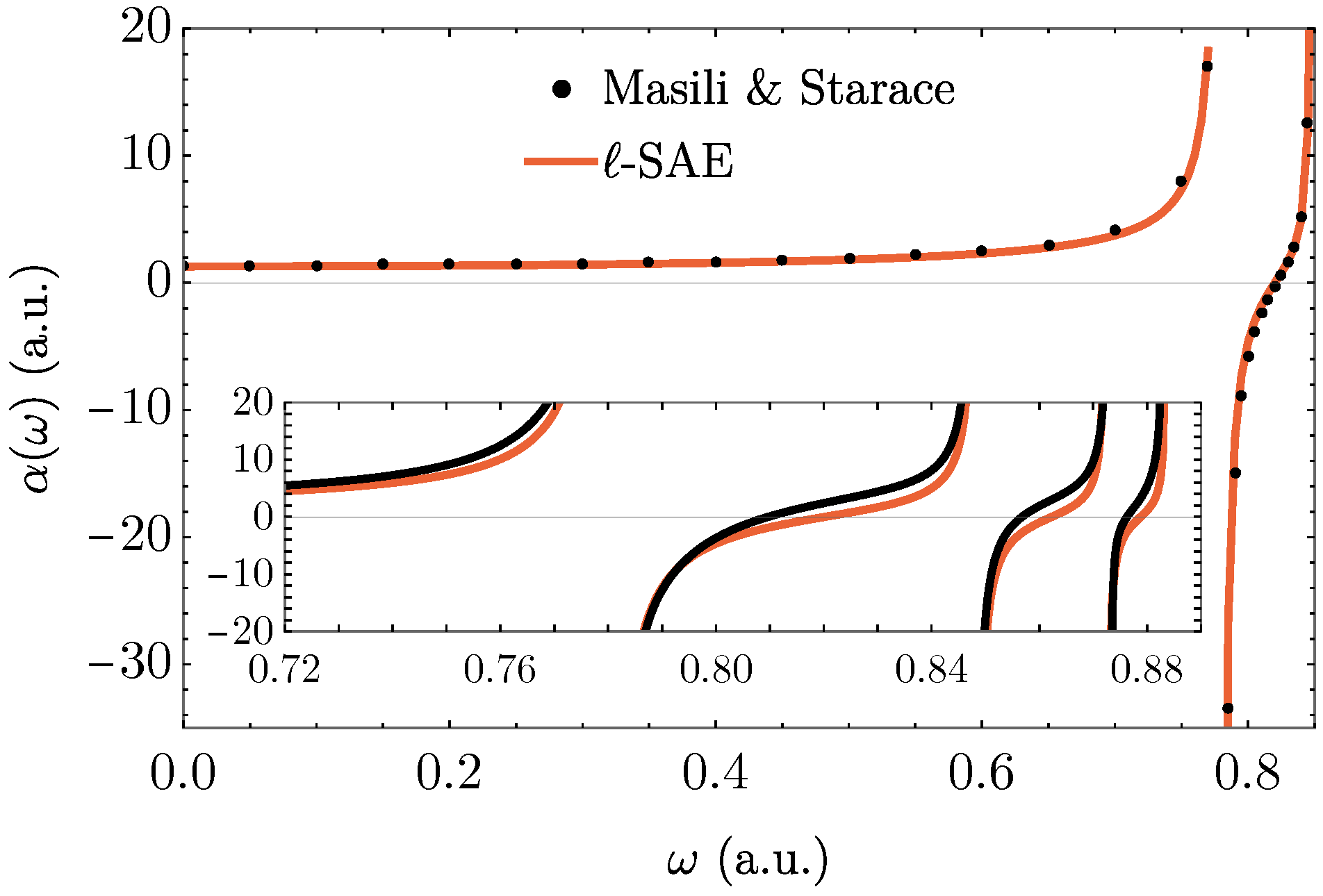
3.2. Static and Dynamic Polarizabilities
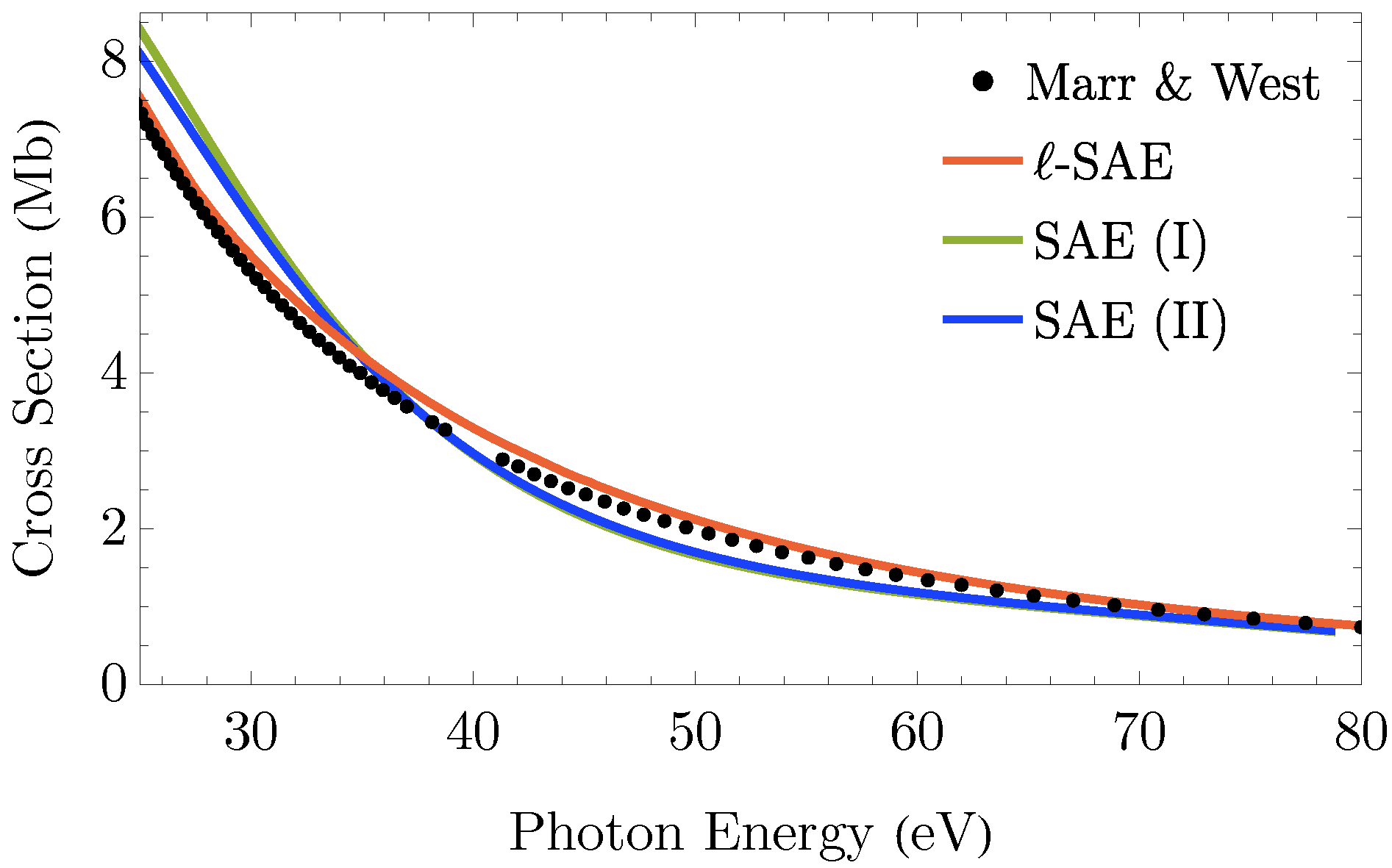
3.3. Photoionization Cross Section
4. Solution of the TDSE in the ℓ-SAE Approximation
4.1. Generalities
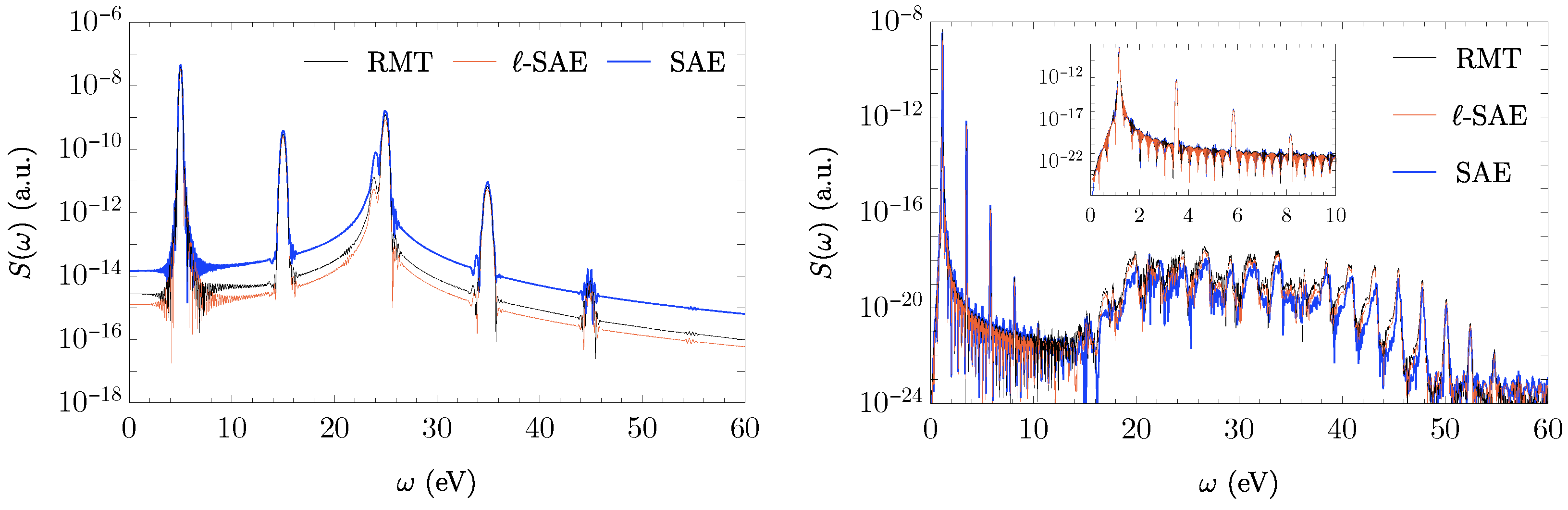
4.2. High-Order Harmonic Generation (HHG)
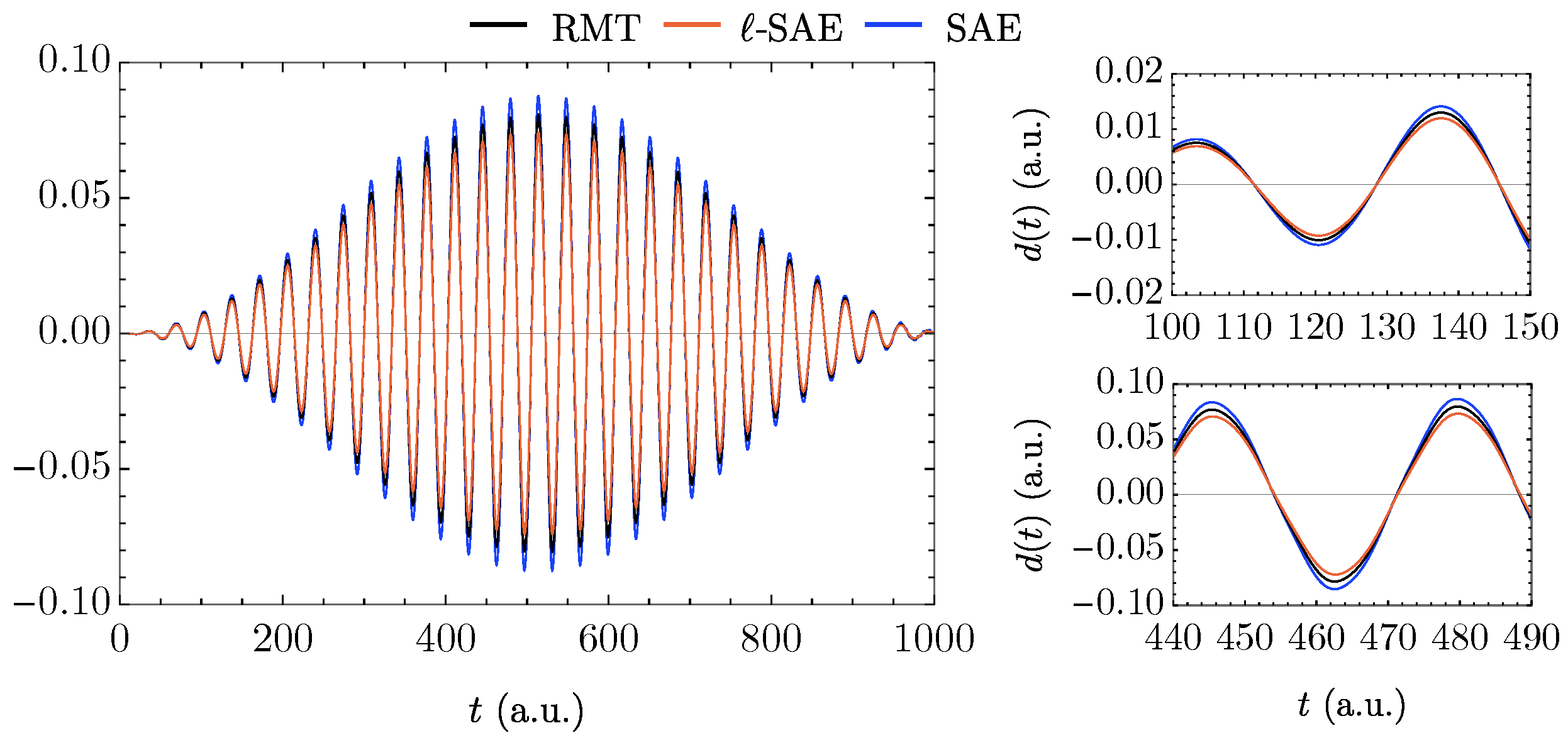
4.2.1. Induced Dipole Moment
4.2.2. Spectral Density
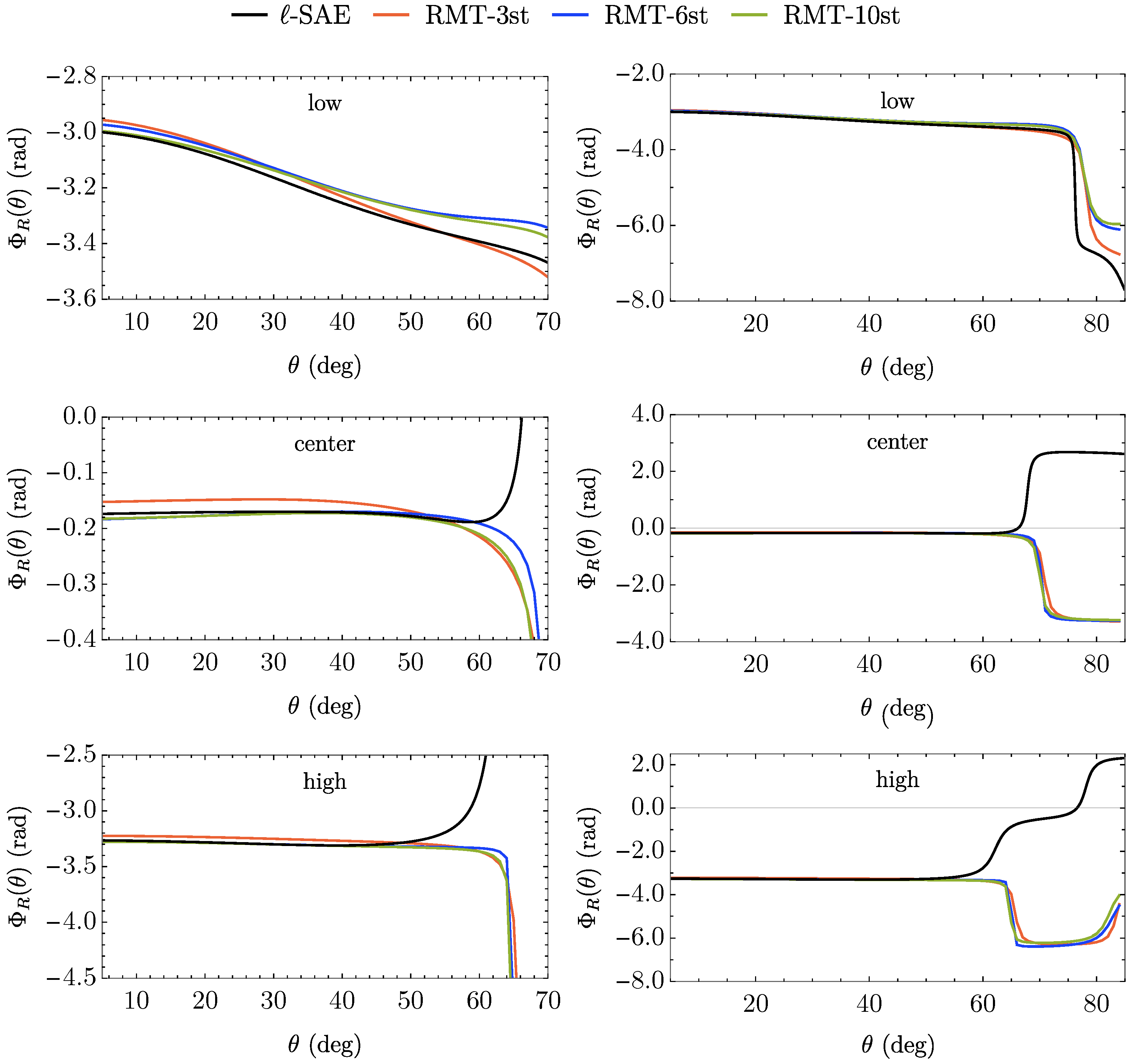
4.3. 3SB-RABBITT
5. Conclusions
6. A Few Words on Barry Irwin Schneider by J.C.d.V.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| SAE | Single Active Electron |
| RABBITT | Reconstruction of Attosecond Beating By Interference of Two-photon Transitions |
| TISE | Time-Independent Schrödinger Equation |
| TDSE | Time-Dependent Schrödinger Equation |
| HHG | High-order Harmonic Generation |
| RMT | R-Matrix with Time dependence |
| SB | Side-Band |
| IR | Infra-Red |
| XUV | Extreme Ultra-Violet |
| 1 | While SAE considerations for molecules are possible, we focus on atomic targets in this work. |
| 2 | As shown in [14], local potentials with ℓ-dependence can be derived from an ℓ-independent non-local potential. |
| 3 | As usual, , and |
| 4 | For example, releasing for . |
| 5 | A factor of 2 is needed in (6) if either or . |
| 6 | A detailed derivation of these equations is provided on pp. 241–244 in [23]. |
References
- Smyth, E.S.; Parker, J.S.; Taylor, K. Numerical Integration of the Time-Dependent Schrödinger Equation for Laser-Driven Helium. Comput. Phys. Commun. 1998, 114, 1–14. [Google Scholar] [CrossRef]
- Brown, A.C.; Armstrong, G.S.; Benda, J.; Clarke, D.D.; Wragg, J.; Hamilton, K.R.; Mašín, Z.; Gorfinkiel, J.D.; van der Hart, H.W. RMT: R-Matrix with Time-Dependence. Solving the Semi-Relativistic, Time-Dependent Schrödinger Equation for General, Multielectron Atoms and Molecules in Intense, Ultrashort, Arbitrarily Polarized Laser Pulses. Comp. Phys. Commun. 2020, 250, 107062. [Google Scholar] [CrossRef]
- Koch, O.; Kreuzer, W.; Scrinzi, A. Approximation of the Time-Dependent Electronic Schrödinger Equation by MCTDHF. Appl. Math. Comput. 2006, 173, 960–976. [Google Scholar] [CrossRef]
- Marques, M.A.; Castro, A.; Bertsch, G.F.; Rubio, A. Octopus: A First-Principles Tool for Excited Electron–Ion Dynamics. Comput. Phys. Commun. 2003, 151, 60–78. [Google Scholar] [CrossRef]
- Bauer, D.; Koval, P. Qprop: A Schrödinger-Solver for Intense Laser–Atom Interaction. Comput. Phys. Commun. 2006, 174, 396–421. [Google Scholar] [CrossRef]
- Fu, Y.; Zeng, J.; Yuan, J. PCTDSE: A Parallel Cartesian-Grid-Based TDSE Solver for Modeling Laser–Atom Interactions. Comput. Phys. Commun. 2017, 210, 181–192. [Google Scholar] [CrossRef]
- Reiff, R.; Joyce, T.; Jaroń-Becker, A.; Becker, A. Single-Active Electron Calculations of High-Order Harmonic Generation from Valence Shells in Atoms for Quantitative Comparison with TDDFT Calculations. J. Phys. Commun. 2020, 4, 065011. [Google Scholar] [CrossRef]
- Cowan, R.D. The Theory of Atomic Structure and Spectra; Los Alamos Series in Basic and Applied Sciences; University of California Press, Ltd.: Berkeley, CA, USA, 1981. [Google Scholar] [CrossRef]
- Kulander, K.; Rescigno, T. Effective Potentials for Time-Dependent Calculations of Multiphoton Processes in Atoms. Comput. Phys. Commun 1991, 63, 523–528. [Google Scholar] [CrossRef]
- Ivanov, I.A.; Kheifets, A.S. Harmonic Generation for Atoms in Fields of Varying Ellipticity: Single-Active-Electron model with Hartree-Fock Potential. Phys. Rev. A 2009, 79, 053827. [Google Scholar] [CrossRef]
- Muller, H.G. Numerical Simulation of High-Order Above-Threshold-Ionization Enhancement in Argon. Phys. Rev. A 1999, 60, 1341–1350. [Google Scholar] [CrossRef]
- Bartschat, K. (Ed.) Computational Atomic Physics: Electron and Positron Collisions with Atoms and Lons; Springer: Berlin/Heidelberg, Germany, 1996. [Google Scholar] [CrossRef]
- Parker, J.S.; Smyth, E.S.; Taylor, K.T. Intense-Field Multiphoton Ionization of Helium. J. Phys. B 1998, 31, L571. [Google Scholar] [CrossRef]
- Lassaut, M.; Vinh Mau, N. l-Dependent Local Potentials Equivalent to a Non-Local Potential. Nucl. Phys. A 1990, 518, 441–448. [Google Scholar] [CrossRef]
- Norcross, D.W. Application of Scattering Theory to the Calculation of Alkali Negative-Ion Bound States. Phys. Rev. Lett. 1974, 32, 192–195. [Google Scholar] [CrossRef]
- Greene, C.H.; Aymar, M. Spin-Orbit Effects in the Heavy Alkaline-Earth Atoms. Phys. Rev. A 1991, 44, 1773–1790. [Google Scholar] [CrossRef]
- Laughlin, C.; Victor, G.A. Model-Potential Methods. In Advances in Atomic and Molecular Physics; Bates, D., Bederson, B., Eds.; Academic Press: Cambridge, MA, USA, 1989; Volume 25, pp. 163–194. [Google Scholar] [CrossRef]
- Marinescu, M.; Sadeghpour, H.R.; Dalgarno, A. Dispersion Coefficients for Alkali-Metal Dimers. Phys. Rev. A 1994, 49, 982–988. [Google Scholar] [CrossRef]
- Moores, D.L.; Norcross, D.W. The Scattering of Electrons by Sodium Atoms. J. Phys. B 1972, 5, 1482. [Google Scholar] [CrossRef]
- Thumm, U.; Norcross, D.W. Evidence for Very Narrow Shape Resonances in Low-Energy Electron-Cs Scattering. Phys. Rev. Lett. 1991, 67, 3495–3498. [Google Scholar] [CrossRef]
- Bartschat, K. Low-Energy Electron Scattering from Caesium Atoms-Comparison of a Semirelativistic Breit-Pauli and a Full Relativistic Dirac Treatment. J. Phys. B 1993, 26, 3595. [Google Scholar] [CrossRef]
- Bray, I. Convergent Close-Coupling Method for the Calculation of Electron Scattering on Hydrogenlike Targets. Phys. Rev. A 1994, 49, 1066–1082. [Google Scholar] [CrossRef]
- Joachain, C.J.; Kylstra, N.J.; Potvliege, R.M. Atoms in Intense Laser Fields; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar] [CrossRef]
- Li, P.C.; Sheu, Y.L.; Laughlin, C.; Chu, S.I. Dynamical Origin of Near- and Below-Threshold Harmonic Generation of Cs in an Intense Mid-Infrared Laser Field. Nat. Commun. 2015, 6, 7178. [Google Scholar] [CrossRef]
- Nikolopoulos, L.A.A. Elements of Photoionization Quantum Dynamics Methods; Morgan & Claypool Publishers: San Rafael, CA, USA, 2019; pp. 2053–2571. [Google Scholar] [CrossRef]
- Perry, M.D.; Szoke, A.; Kulander, K.C. Resonantly Enhanced Above-Threshold Ionization of Helium. Phys. Rev. Lett. 1989, 63, 1058–1061. [Google Scholar] [CrossRef] [PubMed]
- Tong, X.M.; Lin, C.D. Empirical Formula for Static Field Ionization Rates of Atoms and Molecules by Lasers in the Barrier-Suppression Regime. J. Phys. B 2005, 38, 2593. [Google Scholar] [CrossRef]
- Green, A.E.S.; Sellin, D.L.; Zachor, A.S. Analytic Independent-Particle Model for Atoms. Phys. Rev. 1969, 184, 1–9. [Google Scholar] [CrossRef]
- Sarsa, A.; Gálvez, F.; Buendía, E. Parameterized Optimized Effective Potential for the Ground State of the Atoms He through Xe. At. Data Nucl. Data Tables 2004, 88, 163–202. [Google Scholar] [CrossRef]
- Kulander, K.C. Time-Dependent Hartree-Fock Theory of Multiphoton Ionization: Helium. Phys. Rev. A 1987, 36, 2726–2738. [Google Scholar] [CrossRef]
- Kramida, A.; Ralchenko, Y.; Reader, J.; NIST ASD Team. NIST Atomic Spectra Database (Ver. 5.12); National Institute of Standards and Technology: Gaithersburg, MD, USA, 2024. Available online: https://physics.nist.gov/asd (accessed on 25 August 2024). [CrossRef]
- Baye, D. The Lagrange-Mesh Method. Phys. Rep. 2015, 565, 1–107. [Google Scholar] [CrossRef]
- del Valle, J.C.; Nader, D.J. Toward the Theory of the Yukawa Potential. J. Math. Phys. 2018, 59, 102103. [Google Scholar] [CrossRef]
- James, F.; Roos, M. Minuit—A System for Function Minimization and Analysis of the Parameter Errors and Correlations. Comput. Phys. Commun. 1975, 10, 343–367. [Google Scholar] [CrossRef]
- Froese-Fischer, C.; Brage, T.; Jönsson, P. Computational Atomic Structure: An MCHF Approach; Routledge: New York, NY, USA, 1997. [Google Scholar] [CrossRef]
- Birk, P.; Stooß, V.; Hartmann, M.; Borisova, G.D.; Blättermann, A.; Heldt, T.; Bartschat, K.; Ott, C.; Pfeifer, T. Attosecond transient absorption of a continuum threshold. J. Phys. B 2020, 53, 124002. [Google Scholar] [CrossRef]
- Bethe, H.A.; Salpeter, E.E. Quantum Mechanics of One-and Two-Electron Atoms; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013. [Google Scholar] [CrossRef]
- Drake, G.W.F. (Ed.) Springer Handbook of Atomic, Molecular, and Optical Physics, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2023. [Google Scholar] [CrossRef]
- Masili, M.; Starace, A.F. Static and Dynamic Dipole Polarizability of the Helium Atom Using Wave Functions Involving Logarithmic Terms. Phys. Rev. A 2003, 68, 012508. [Google Scholar] [CrossRef]
- Starace, A. Photoionization of Atoms. In Springer Handbook of Atomic, Molecular, and Optical Physics; Drake, G.W.F., Ed.; Springer: New York, NY, USA, 2006; pp. 379–390. [Google Scholar] [CrossRef]
- Marr, G.; West, J. Absolute Photoionization Cross-Section Tables for Helium, Neon, Argon, and Krypton in the VUV Spectral Regions. At. Data Nucl. Data Tables 1976, 18, 497–508. [Google Scholar] [CrossRef]
- Douguet, N.; Grum-Grzhimailo, A.N.; Gryzlova, E.V.; Staroselskaya, E.I.; Venzke, J.; Bartschat, K. Photoelectron Angular Distributions in Bichromatic Atomic Ionization Induced by Circularly Polarized VUV Femtosecond Pulses. Phys. Rev. A 2016, 93, 033402. [Google Scholar] [CrossRef]
- Douguet, N.; Bartschat, K. Photoelectron Momentum Distributions in the Strong-Field Ionization of Atomic Hydrogen by Few-Cycle Elliptically Polarized Optical Pulses. Phys. Rev. A 2022, 106, 053112. [Google Scholar] [CrossRef]
- Bondy, A.T.; Saha, S.; del Valle, J.C.; Harth, A.; Douguet, N.; Hamilton, K.R.; Bartschat, K. High-Order Harmonic Generation in Helium: A Comparison Study. Phys. Rev. A 2024, 109, 043113. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifschits, E.M. The Classical Theory of Fields; Course of Theoretical Physics; Pergamon Press: Oxford, UK, 1975; Volume 2. [Google Scholar] [CrossRef]
- Bharti, D.; Srinivas, H.; Shobeiry, F.; Bondy, A.T.; Saha, S.; Hamilton, K.R.; Moshammer, R.; Pfeifer, T.; Bartschat, K.; Harth, A. Multi-sideband Interference Structures by High-Order Photon-Induced Continuum-Continuum Transitions in Helium. Phys. Rev. A 2024, 109, 023110. [Google Scholar] [CrossRef]
- Bondy, A.T.; del Valle, J.C.; Saha, S.; Hamilton, K.R.; Bharti, D.; Harth, A.; Bartschat, K. R-Matrix with Time-Dependence Calculations for Three-Sideband RABBITT in Helium. Eur. Phys. J. D 2024, 78, 106. [Google Scholar] [CrossRef]
- Bharti, D.; del Valle, J.C.; Bondy, A.; Bartschat, K.; Moshammer, R.; Pfeifer, T.; Harth, A. Control of Attosecond Photoemission Time Delays by Quantum-State Interference. 2025; in preparation. [Google Scholar]
- Abu-Samha, M.; Madsen, L.B. Theory of Strong-Field Ionization of Aligned CO2. Phys. Rev. A 2009, 80, 023401. [Google Scholar] [CrossRef]


| ℓ | |||
|---|---|---|---|
| 0 | 0.49574 | 1.19128 | |
| 1 | 0.29052 | 1.19128 | |
| 0.10516 | 1.19128 |
| State | Energy (a.u.) | |
|---|---|---|
| NIST | ℓ-SAE | |
| −2.9036 | −2.9036 | |
| −2.1460 | −2.1427 | |
| −2.1238 | −2.1238 | |
| −2.0613 | −2.0613 | |
| −2.0556 | −2.0557 | |
| −2.0551 | −2.0551 | |
| −2.0336 | −2.0337 | |
| −2.0313 | −2.0313 | |
| −2.0313 | −2.0313 | |
| −2.0311 | −2.0311 | |
| 0.241254 | 0.328273 | |||
| 0.276165 | 0.376440 | |||
| 0.066093 | 0.156859 | 0.625162 | ||
| 0.073435 | 0.151342 | 0.626193 | ||
| 0.027180 | 0.047910 | 0.141081 | 0.880303 | |
| 0.029863 | 0.049155 | 0.143889 | 0.858021 | |
| 0.714401 | ||||
| 0.710164 | ||||
| 0.121109 | 0.649095 | |||
| 0.120270 | 0.648104 | |||
| 1.015314 | 0.002622 | |||
| 1.015083 | 0.002492 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
del Valle, J.C.; Bartschat, K. The Single-Active-Electron Approximation with Angular-Momentum-Dependent Potentials: Application to the Helium Atom. Atoms 2025, 13, 43. https://doi.org/10.3390/atoms13050043
del Valle JC, Bartschat K. The Single-Active-Electron Approximation with Angular-Momentum-Dependent Potentials: Application to the Helium Atom. Atoms. 2025; 13(5):43. https://doi.org/10.3390/atoms13050043
Chicago/Turabian Styledel Valle, Juan Carlos, and Klaus Bartschat. 2025. "The Single-Active-Electron Approximation with Angular-Momentum-Dependent Potentials: Application to the Helium Atom" Atoms 13, no. 5: 43. https://doi.org/10.3390/atoms13050043
APA Styledel Valle, J. C., & Bartschat, K. (2025). The Single-Active-Electron Approximation with Angular-Momentum-Dependent Potentials: Application to the Helium Atom. Atoms, 13(5), 43. https://doi.org/10.3390/atoms13050043







