Low-Energy Electron Elastic Total Cross Sections for Ho, Er, Tm, Yb, Lu, and Hf Atoms
Abstract
:1. Introduction
2. Method of Calculation
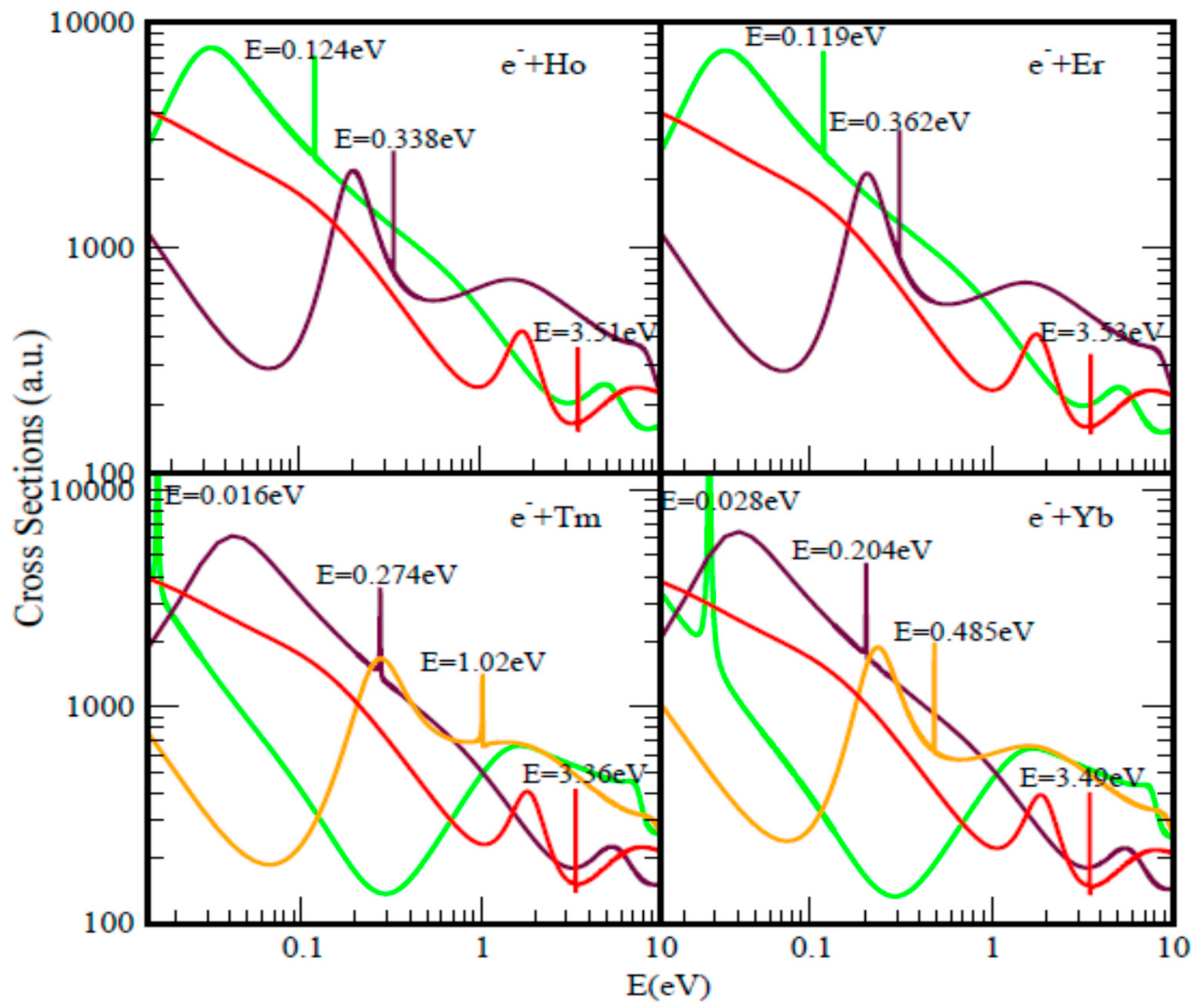
3. Results
4. Summary and Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Kasdan, K.; Lineberger, W.C. Alkali-metal negative ions. II. Laser photoelectron spectrometry. Phys. Rev. A 1974, 10, 1658. [Google Scholar] [CrossRef]
- Msezane, A.Z.; Felfli, Z.; Sokolovski, D. Novel mechanism for nanoscale catalysis. J. Phys. B 2010, 43, 201001. [Google Scholar] [CrossRef]
- Msezane, A.Z.; Felfli, Z.; Sokolovski, D. Cold fusion mechanism in nanoscale catalysis. Eur. News 2010, 41, 11. [Google Scholar]
- Cheng, S.-B.; Castleman, A.W. Direct experimental observation of weakly-bound character of the attached electron in europium anion. Sci. Rep. 2015, 5, 12414. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Felfli, Z.; Msezane, A.Z. Conundrum in Measured Electron Affinities of Complex Heavy Atoms. JAMCNP 2018, 5, 73–80. [Google Scholar] [CrossRef] [Green Version]
- Msezane, A.Z. Negative Ion Binding Energies in Complex Heavy Systems. JAMCNP 2018, 5, 195–204. [Google Scholar] [CrossRef] [Green Version]
- Hotop, H.; Lineberger, W.C. Dye-laser photodetachment studies of Au−, Pt−, PtN−, and Ag−. J. Chem. Phys. 2003, 58, 2379. [Google Scholar] [CrossRef]
- Andersen, T.; Haugen, H.K.; Hotop, H. Binding Energies in Atomic Negative Ions: III. J. Phys. Chem. Ref. Data 1999, 28, 1511. [Google Scholar] [CrossRef]
- Zheng, W.; Li, X.; Eustis, S.; Grubisic, A.; Thomas, O.; De Clercq, H.; Bowen, K. Anion photoelectron spectroscopy of Au−(H2O) 1, 2, Au2-(D2O) 1–4, and AuOH−. Chem. Phys. Lett. 2007, 444, 232–236. [Google Scholar] [CrossRef]
- Gibson, D.; Davies, B.J.; Larson, D.J. The electron affinity of platinum. J. Chem. Phys. 1993, 98, 5104. [Google Scholar] [CrossRef]
- Bilodeau, R.C.; Scheer, M.; Haugen, H.K.; Brooks, R.L. Near-threshold laser spectroscopy of iridium and platinum negative ions: Electron affinities and the threshold law. Phys. Rev. A 1999, 61, 012505. [Google Scholar] [CrossRef]
- Leimbach, D.; Sundberg, J.; Guo, Y.; Ahmed, R.; Ballof, J.; Bengtsson, L.; Pamies, F.B.; Borschevsky, A.; Chrysalidis, K.; Eliav, E.; et al. The electron affinity of astatine. arXiv 2002, arXiv:2002.11418. [Google Scholar]
- Huang, D.-L.; Dau, P.D.; Liu, H.T.; Wang, L.-S. High-resolution photoelectron imaging of cold C60- anions and accurate determination of the electron affinity of C60 . J. Chem. Phys. 2014, 140, 224315. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Brink, C.; Andersen, L.H.; Hvelplund, P.; Mathur, D.; Voldstad, J.D. Laser photodetachment of C60− and C70− ions cooled in a storage ring. Chem. Phys. Lett. 1995, 233, 52–56. [Google Scholar] [CrossRef]
- Msezane, A.Z.; Felfli, Z. New insights in low-energy electron-fullerene interactions. Chem. Phys. 2018, 503, 50–55. [Google Scholar] [CrossRef]
- Felfli, Z.; Msezane, A.Z. Simple method for determining fullerene negative ion formation. Eur. Phys. J. D 2018, 72, 78. [Google Scholar] [CrossRef]
- Andersen, H.H.; Andersen, T.; Pedersen, U.V. Search for stable or metastable negative Yb ions. J. Phys. B 1998, 31, 2239. [Google Scholar] [CrossRef]
- Davis, V.T.; Thompson, J.S. Measurement of the electron affinity of thulium. Phys. Rev. A 2001, 65, 010501. [Google Scholar] [CrossRef] [Green Version]
- Felfli, Z.; Msezane, A.Z.; Sokolovski, D. Resonances in low-energy electron elastic cross sections for lanthanide atoms. Phys. Rev. A 2009, 79, 012714. [Google Scholar] [CrossRef] [Green Version]
- O’Malley, S.M.; Beck, D.R. Valence calculations of lanthanide anion binding energies: 6p attachments to 4fn6s2 thresholds. Phys. Rev. A 2008, 78, 012510. [Google Scholar] [CrossRef]
- Davis, V.T.; Thompson, J.S. An experimental investigation of the atomic europium anion. Phys. B 2004, 37, 1961. [Google Scholar] [CrossRef]
- Msezane, A.Z.; Felfli, Z. Atomic Negative Ions Creation: Application in Nanocatalysis. In Advances in Nanotechnology; Bartul, Z., Trenor, J., Eds.; Nova Science Publishers Inc.: New York, NY, USA, 2017; Volume 17, pp. 1–41. ISBN 978-1-53611-004-3. [Google Scholar]
- Felfli, Z.; Msezane, A.Z.; Sokolovski, D. Strong resonances in low-energy electron elastic total and differential cross sections for Hf and Lu atoms. Phys. Rev. A 2008, 78, 030703. [Google Scholar] [CrossRef]
- Davis, V.T.; Thompson, J.S. Measurement of the electron affinity of lutetium. J. Phys. B 2001, 34, L433. [Google Scholar] [CrossRef]
- Vosko, S.H.; Chevary, J.A. Prediction of a further irregularity in the electron filling of subshell: Lu-(Xe)4f145d16s26p1 and its relation to the group IIIB anions. J. Phys. B 1993, 26, 873. [Google Scholar] [CrossRef]
- Eliav, E.; Kaldor, U.; Ishikawa, Y. Transition energies of ytterbium, lutetium, and lawrencium by the relativistic coupled-cluster method. Phys. Rev. A 1995, 52, 291. [Google Scholar] [CrossRef]
- Felfli, Z.; Msezane, A.Z. Negative Ion Formation in Low-Energy Electron Collisions with the Actinide Atoms Th, Pa, U, Np and Pu. Appl. Phys. Res. 2019, 11, 52. [Google Scholar] [CrossRef] [Green Version]
- Luo, Z.; Chen, X.; Li, J.; Ning, C. Precision measurement of the electron affinity of niobium. Phys. Rev. A 2016, 93, 020501. [Google Scholar] [CrossRef]
- Cole, L.A.; Perdew, J.P. Calculated electron affinities of the elements. Phys. Rev. A 1982, 25, 1265. [Google Scholar] [CrossRef]
- Calaminici, P.; Mejia-Olvera, R. Structures, Frequencies, and Energy Properties of Small Neutral, Cationic, and Anionic Niobium Clusters. J. Phys. Chem. C 2011, 115, 11891–11897. [Google Scholar] [CrossRef]
- Feigerle, C.S.; Corderman, R.R.; Bobashev, S.V.; Lineberger, W.C. Binding energies and structure of transition metal negative ions. J. Chem. Phys. 1981, 74, 1580. [Google Scholar] [CrossRef]
- Pan, L.; Beck, D.R. Calculations of Hf-electron affinity and photodetachment partial cross sections. J. Phys. B 2010, 43, 025002. [Google Scholar] [CrossRef]
- Felfli, Z.; Msezane, A.Z.; Sokolovski, D. Low-energy electron elastic collision cross sections for ground and excited Tm, Lu and Hf atoms. NIMB 2011, 269, 1046–1052. [Google Scholar] [CrossRef] [Green Version]
- Hiscox, A.; Brown, B.M.; Marletta, M. On the low energy behavior of Regge poles. J. Math. Phys. 2010, 51, 102104. [Google Scholar] [CrossRef]
- Frautschi, S.C. Regge Poles and S-matrix Theory; Benjamin: New York, NY, USA, 1963; Chapter X. [Google Scholar] [CrossRef]
- D’Alfaro, V.; Regge, T.E. Potential Scattering; North-Holland Pub. Co.: Amsterdam, The Netherlands, 1965. [Google Scholar]
- Thylwe, K.W. On relativistic shifts of negative-ion resonances. Eur. Phys. J. D 2012, 66, 7. [Google Scholar] [CrossRef]
- Mulholland, H.P. An asymptotic expansion for Σ(2n+1)exp (Àσ(n+1/2)2). Proc. Camb. Phil. Soc. 1928, 24, 280–289. [Google Scholar] [CrossRef]
- Macek, J.H.; Krstic, P.S.; Ovchinnikov, S.Y. Regge Oscillations in Integral Cross Sections for Proton Impact on Atomic Hydrogen. Phys. Rev. Lett. 2004, 93, 183203. [Google Scholar] [CrossRef]
- Sokolovski, D.; Felfli, Z.; Ovchinnikov, S.Y.; Macek, J.H.; Msezane, A.Z. Regge oscillations in electron-atom elastic cross sections. Phys. Rev. A 2007, 76, 012705. [Google Scholar] [CrossRef]
- Dolmatov, V.K.; Amusia, M.Y.; Chernysheva, L.V. Electron elastic scattering off A@C60: The role of atomic polarization under confinement. Phys. Rev. A 2017, 95, 012709. [Google Scholar] [CrossRef] [Green Version]
- Felfli, Z.; Belov, S.; Avdonina, N.B.; Marletta, M.; Msezane, A.Z.; Naboko, S.N. Regge Poles Trajectories for Nonsingular Potentials: The Thomas-Fermi Potentials. In Proceedings of the Third International Workshop on Contemporary Problems in Mathematical Physics; Govaerts, J., Hounkonnou, M.N., Msezane, A.Z., Eds.; World Scientific: Singapore, 2004; pp. 217–232. ISBN 981-256-030-0. [Google Scholar]
- Belov, S.; Thylwe, K.-E.; Marletta, M.; Msezane, A.Z.; Naboko, S.N. On Regge pole trajectories for a rational function approximation of Thomas–Fermi potentials. J. Phys. A 2010, 43, 365301. [Google Scholar] [CrossRef]
- Burke, P.G.; Tate, C. A PROGRAM FOR CALCULATING REGGE TRAJECTORIES IN POTENTIAL SCATTERING. Comp. Phys. Commun. 1969, 1, 97. [Google Scholar] [CrossRef]
- Connor, J.N.L. New theoretical methods for molecular collisions: The complex angular-momentum approach. J. Chem. Soc. Faraday Trans. 1990, 86, 1627. [Google Scholar] [CrossRef]
- Regge, T. Bound states, shadow states and Mandelstam representation. Nuovo Cim. 1960, 18, 947–956. [Google Scholar] [CrossRef]
- Fabrikant, I.I.; Lebedev, V.S. Quenching of Rydberg states by atoms with small electron affinities. J. Phys. B 2000, 33, 1521. [Google Scholar] [CrossRef]
- Reicherts, M.; Roth, T.; Gopalan, A.; Ruf, M.W.; Hotop, H.; Desfrancois, C.; Fabrikant, I.I. Controlled formation of weakly bound atomic negative ions by electron transfer from state-selected Rydberg atoms. Eur. Phys. Lett. 1997, 40, 129. [Google Scholar] [CrossRef] [Green Version]
- Bahrim, C.; Thumm, U. Low-lying 3Po and 3Se states of Rb−, Cs−, and Fr−. Phys. Rev. A 2000, 61, 022722. [Google Scholar] [CrossRef]
- Johnson, W.R.; Guet, C. Elastic scattering of electrons from Xe, Cs+, and Ba2+. Phys. Rev. A 1994, 49, 1041. [Google Scholar] [CrossRef]
- Felfli, Z.; Msezane, A.Z. Resonances in low-energy electron elastic scattering from Fullerenes. J. Phys. Conf. Series 2017, 875, 052014. [Google Scholar] [CrossRef] [Green Version]
- Burrow, P.D.; Michejda, J.A.; Comer, J. Low-energy electron scattering from Mg, Zn, Cd and Hg: Shape resonances and electron affinities. J. Phys. B 1976, 9, 3225. [Google Scholar] [CrossRef]
- Davis, V.T.; Thompson, J.; Covington, A. Laser photodetachment electron spectroscopy studies of heavy atomic anions. Nucl. Instrum. Meth. Phys. Res. B 2005, 241, 118–124. [Google Scholar] [CrossRef] [Green Version]
- Tang, R.; Chen, X.; Fu, X.; Wang, H.; Ning, C. Electron affinity of the hafnium atom. Phys. Rev. A 2018, 98, 020501. [Google Scholar] [CrossRef]
- Nadeau, M.J.; Litherland, A.E.; Garwan, M.A.; Zhao, X.L. Electric dissociation of negative ions—II. Nucl. Instrum. Meth. Phys. Res. B 1994, 92, 265–269. [Google Scholar] [CrossRef]
- Chevary, J.A.; Vosko, S.H. More theoretical evidence for binding of a 6p electron in the lanthanide anions: Tm-[Xe]4f136s26p1. J. Phys. B 1994, 27, 657. [Google Scholar] [CrossRef]
- Dzuba, V.A.; Gribakin, G.F. Correlation-potential method for negative ions and electron scattering. Phys. Rev. A 1994, 49, 2483. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Vosko, S.H.; Chevary, J.A.; Mayer, I.L. Predictions of stable Yb-in the Po1/2 state: The importance of spin-orbit coupling. J. Phys. B 1991, 24, L225. [Google Scholar] [CrossRef]
- Tang, R.; Si, R.; Fei, Z.; Fu, X.; Lu, Y.; Brage, T.; Liu, H.; Chen, C.; Ning, C. Candidate for Laser Cooling of a Negative Ion: High-Resolution Photoelectron Imaging of Th−. Phys. Rev. Lett. 2019, 123, 203002. [Google Scholar] [CrossRef]
- Si, R.; Froese Fischer, C. Electron affinities of At and its homologous elements Cl, Br, I. Phys. Rev. A 2018, 98, 052504. [Google Scholar] [CrossRef] [Green Version]
- Li, J.; Zhao, Z.; Andersson, M.; Zhang, X.; Chen, C. Theoretical Study for the Electron Affinities of Negative Ions with the MCDHF Method. J. Phys. B 2012, 45, 165004. [Google Scholar] [CrossRef]
- Zollweg, R.J. Electron Affinities of the Heavy Elements. J. Chem. Phys. 1969, 50, 4251. [Google Scholar] [CrossRef]


| Atom. | BEs | EAs | BEs | BEs | BEs | EAs | SR-1 | SR-2 | SR-3 | R-T Min |
|---|---|---|---|---|---|---|---|---|---|---|
| GRS | EXPT | MS-1 | EX-1 | EX-2 | Theory | GRS | ||||
| Ho | 3.51 | N/A | 0.338 | 0.124 | - | 0.338 [19] | 1.73 | 0.205 | 0.0331 | 3.43 |
| Er | 3.53 | N/A | 0.362 | 0.119 | - | 0.362 [19] | 1.8 | 0.191 | 0.0343 | 3.49 |
| Tm | 3.36 | 1.029 [18] | 1.02 | 0.274 | 0.016 | 0.032 [55] | 1.81 | 0.273 | 0.042 | 3.38 |
| 0.027–0.136 [56] | ||||||||||
| 1.02 [5] | ||||||||||
| Yb | 3.49 | <0.003 [17] | 0.485 | 0.204 | 0.028 | 0.036 [57] | 1.76 | 0.231 | 0.039 | 3.51 |
| 0.054 [58] | ||||||||||
| Lu | 4.09 | 0.346 [24,53] | 1.92 | 0.292 | 0.029 | 0.257 [26] | 2.01 | 0.801 | 0.046 | 4.07 |
| 0.190 [25] | ||||||||||
| Hf | 1.68 | 0.178 [54] | 0.525 | 0.113 | 0.017 | 0.114 [32] | 0.821 | 0.252 | 0.021 | 1.67 |
| 0.113 [33] |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Felfli, Z.; Msezane, A.Z. Low-Energy Electron Elastic Total Cross Sections for Ho, Er, Tm, Yb, Lu, and Hf Atoms. Atoms 2020, 8, 17. https://doi.org/10.3390/atoms8020017
Felfli Z, Msezane AZ. Low-Energy Electron Elastic Total Cross Sections for Ho, Er, Tm, Yb, Lu, and Hf Atoms. Atoms. 2020; 8(2):17. https://doi.org/10.3390/atoms8020017
Chicago/Turabian StyleFelfli, Zineb, and Alfred Z. Msezane. 2020. "Low-Energy Electron Elastic Total Cross Sections for Ho, Er, Tm, Yb, Lu, and Hf Atoms" Atoms 8, no. 2: 17. https://doi.org/10.3390/atoms8020017
APA StyleFelfli, Z., & Msezane, A. Z. (2020). Low-Energy Electron Elastic Total Cross Sections for Ho, Er, Tm, Yb, Lu, and Hf Atoms. Atoms, 8(2), 17. https://doi.org/10.3390/atoms8020017





