Casimir-Polder Interaction of an Atom with a Cavity Wall Made of Phase-Change Material out of Thermal Equilibrium
Abstract
1. Introduction
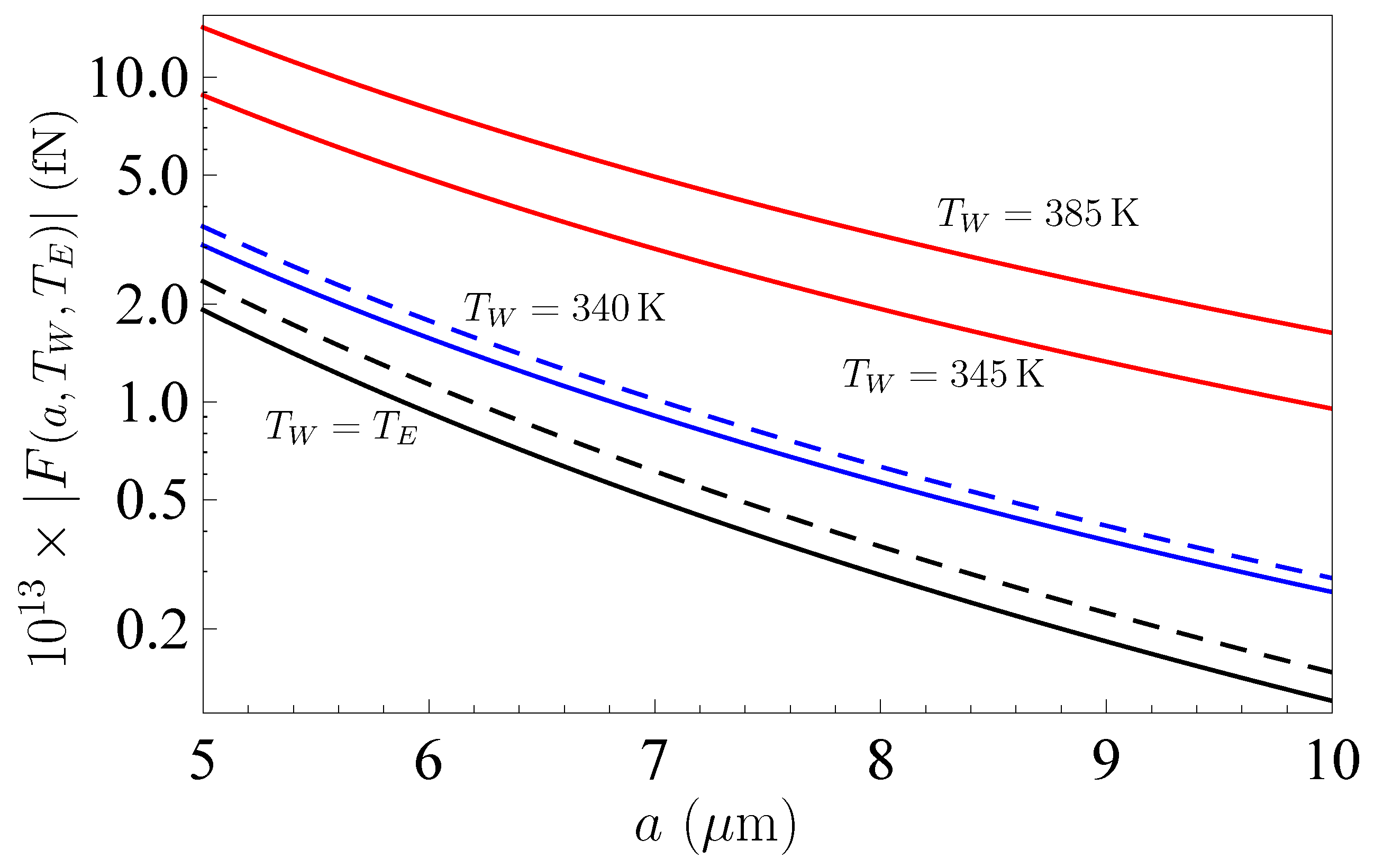
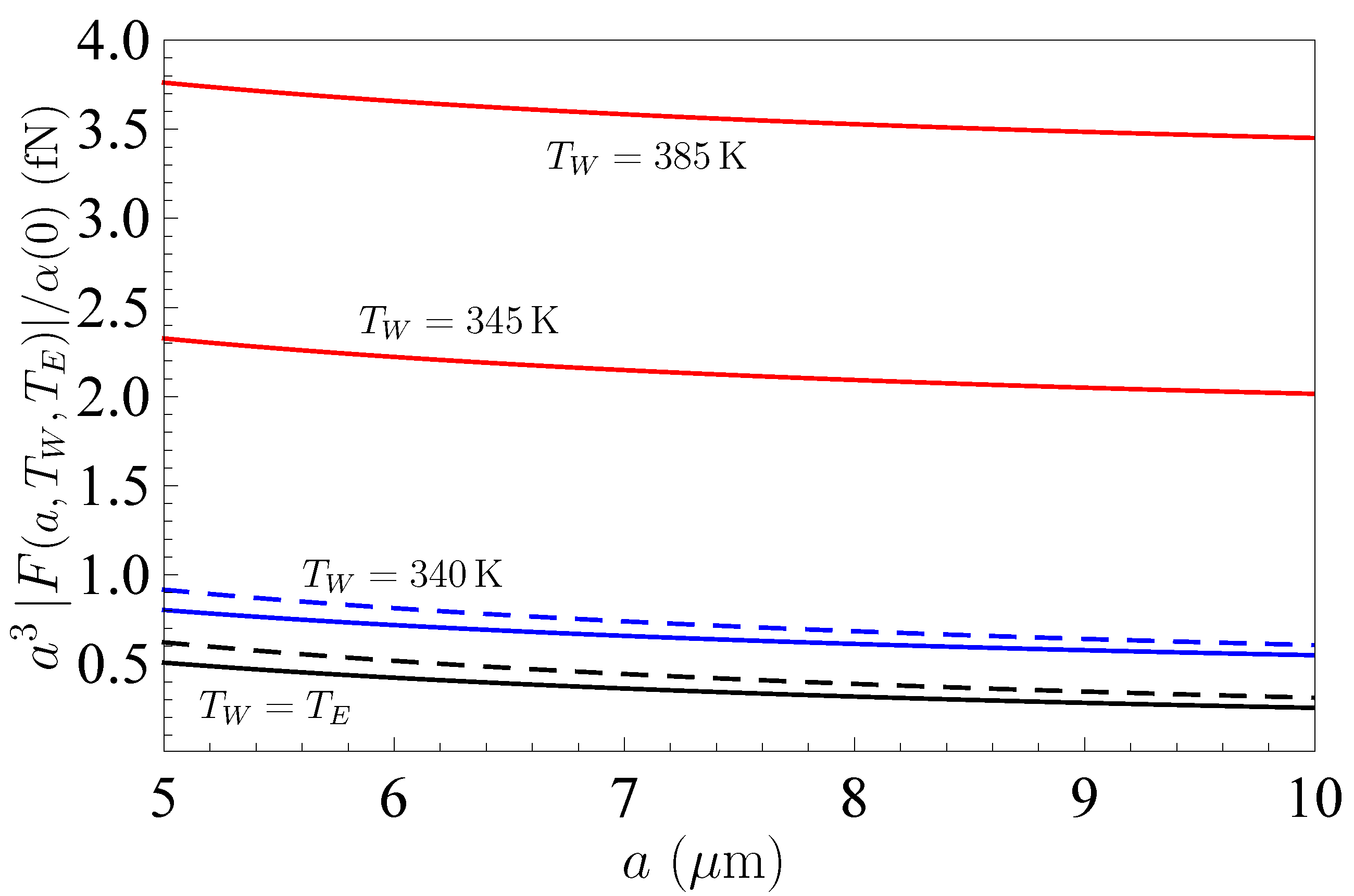
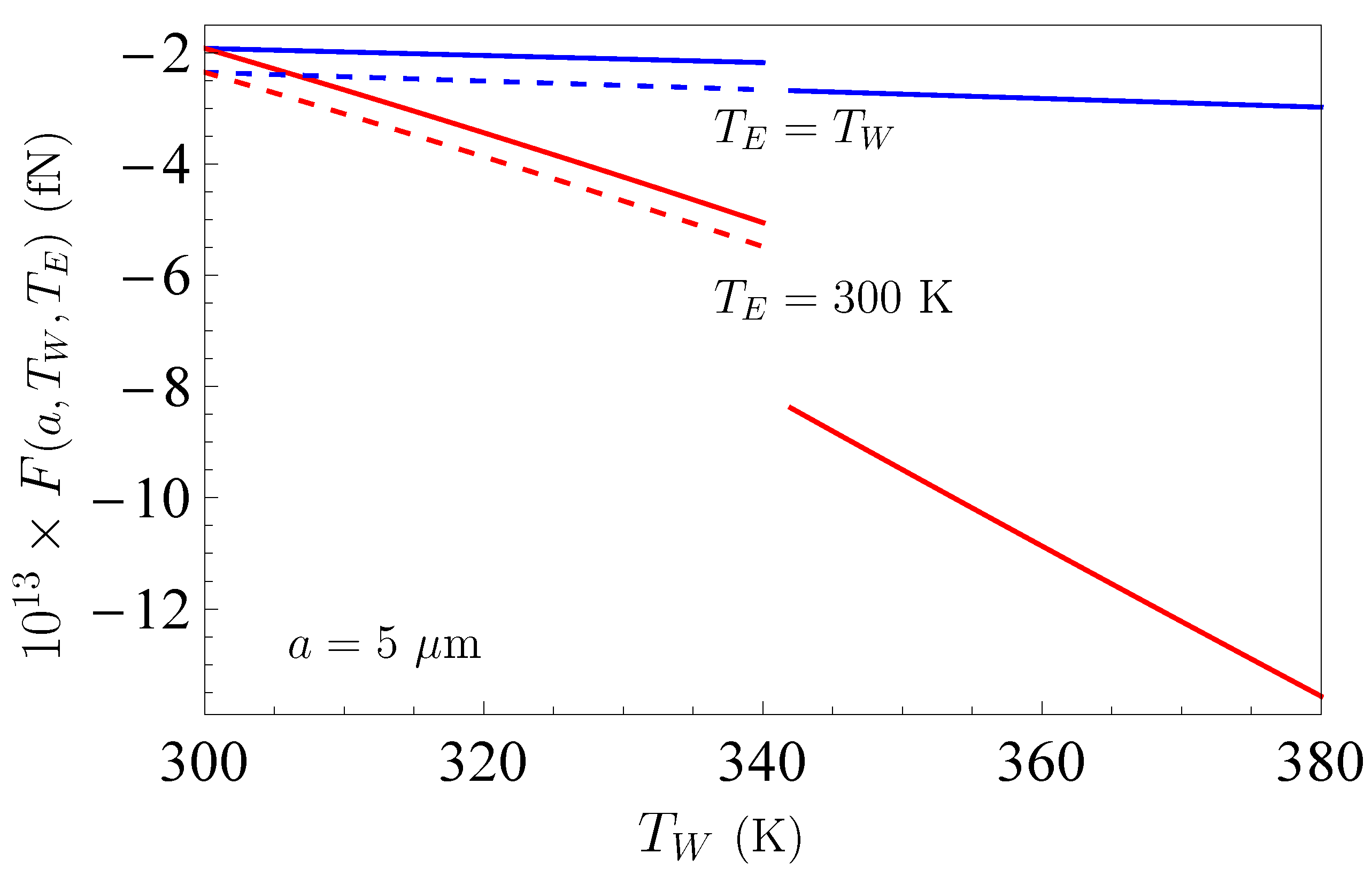
2. Nonequilibrium Casimir-Polder Force in the Micrometer Separation Range
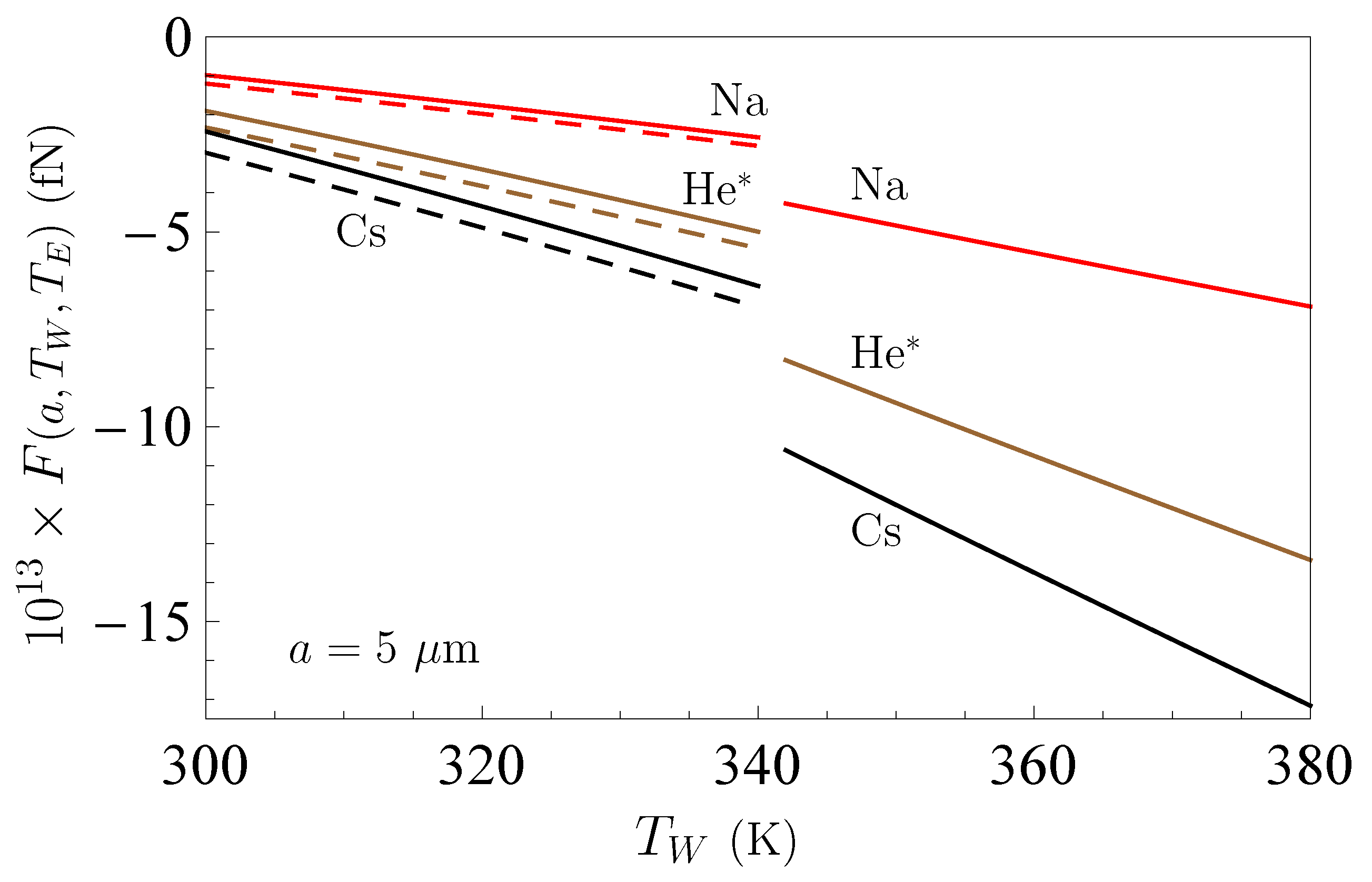
3. The Casimir-Polder Force between Different Atoms and VO Film on a Sapphire Wall
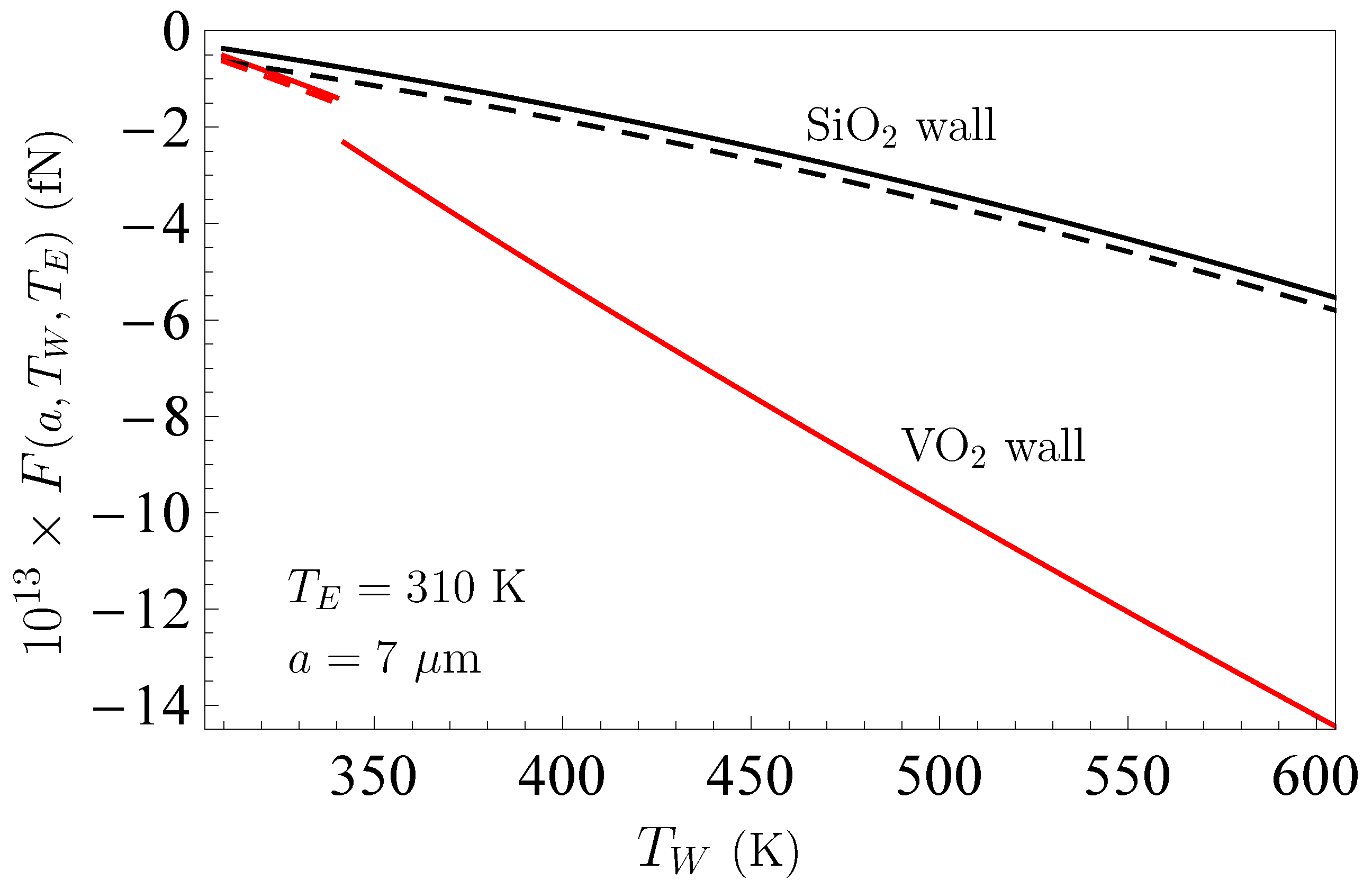
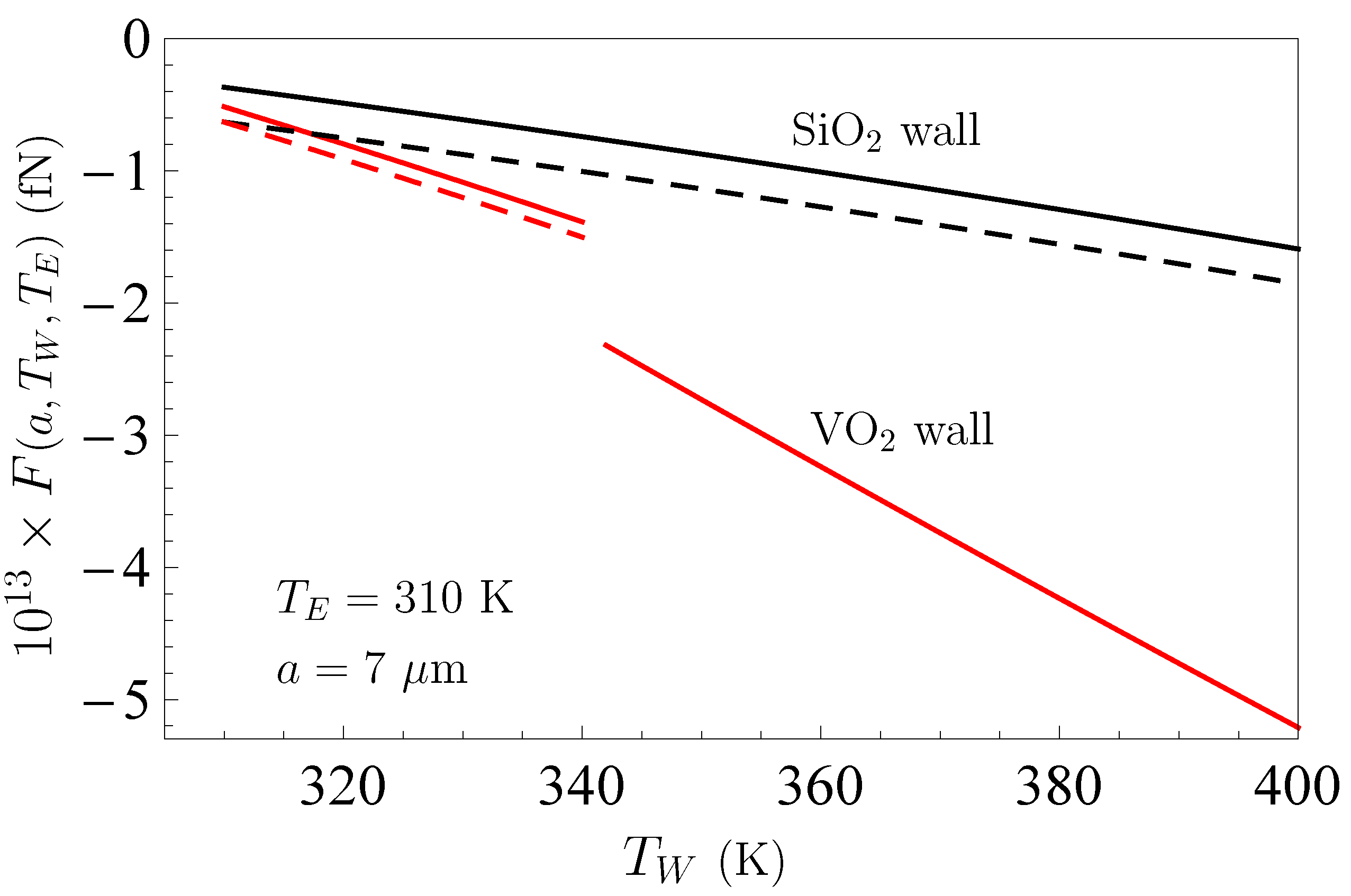
4. Comparison between the Nonequilibrium Casimir-Polder Forces for the Phase-Change and Dielectric Materials
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Parsegian, V.A. Van der Waals Forces: A Handbook for Biologists, Chemists, Engineers, and Physicists; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- London, F. Zur Theorie und Systematik der Molecularkräfte. Z. Phys. 1930, 63, 245–279. [Google Scholar] [CrossRef]
- Casimir, H.B.G.; Polder, D. The influence of retardation on the London-van der Waals forces. Phys. Rev. 1948, 73, 360–372. [Google Scholar] [CrossRef]
- Dzyaloshinskii, I.E.; Lifshitz, E.M.; Pitaevskii, L.P. The general theory of van der Waals forces. Usp. Fiz. Nauk 1961, 73, 381–422, (Adv. Phys. 1961, 10, 165–209). [Google Scholar] [CrossRef]
- Lifshitz, E.M. The theory of molecular attractive forces between solids. Zh. Eksp. Teor. Fiz. 1955, 29, 94–110, Reprint in Sov. Phys. JETP 1956, 2, 73–83. [Google Scholar]
- Lifshitz, E.M.; Pitaevskii, L.P. Statistical Physics, Part II; Pergamon: Oxford, UK, 1980. [Google Scholar]
- Mahanty, J.; Ninham, B.W. Dispersion Forces; Academic: London, UK, 1976. [Google Scholar]
- Nayak, V.U.; Edwards, D.O.; Masuhara, N. Scattering of 4He Atoms Grazing the Liquid- 4He Surface. Phys. Rev. Lett. 1983, 50, 990–993. [Google Scholar] [CrossRef]
- Berkhout, J.J.; Luiten, O.J.; Setija, I.D.; Hijmans, T.W.; Mizusaki, T.; Walraven, J.T.M. Quantum reflection: Focusing of hydrogen atoms with a concave mirror. Phys. Rev. Lett. 1989, 63, 1689–1693. [Google Scholar] [CrossRef]
- Doyle, J.M.; Sandberg, J.C.; Yu, I.A.; Cesar, C.L.; Kleppner, D.; Greytak, T.J. Hydrogen in the submillikelvin regime: Sticking probability on superfluid 4He. Phys. Rev. Lett. 1991, 67, 603–607. [Google Scholar] [CrossRef]
- Yu, I.A.; Doyle, M.J.; Sandberg, J.C.; Cesar, C.L.; Kleppner, D.; Greytak, T.J. Evidence for universal quantum reflection of hydrogen from liquid 4He. Phys. Rev. Lett. 1993, 71, 1589–1593. [Google Scholar] [CrossRef]
- Shimizu, F. Specular Reflection of Very Slow Metastable Neon Atoms from a Solid Surface. Phys. Rev. Lett. 2001, 86, 987–991. [Google Scholar] [CrossRef]
- Friedrich, H.; Jacoby, G.; Meister, C.J. Quantum reflection by Casimir-van der Waals potential tails. Phys. Rev. A 2002, 65, 032902. [Google Scholar] [CrossRef]
- Druzhinina, V.; DeKieviet, M. Experimental Observation of Quantum Reflection far from Threshold. Phys. Rev. Lett. 2003, 91, 193202. [Google Scholar] [CrossRef] [PubMed]
- Oberst, H.; Tashiro, Y.; Shimizu, K.; Shimizu, F. Quantum reflection of He* on silicon. Phys. Rev. A 2005, 71, 052901. [Google Scholar] [CrossRef]
- Judd, T.E.; Scott, R.G.; Martin, A.M.; Kaczmarek, B.; Fromhold, T.M. Quantum reflection of ultracold atoms from thin films, graphene and semiconductor heterostructures. New J. Phys. 1983, 13, 083020. [Google Scholar] [CrossRef]
- Rojas-Lorenzo, G.; Rubayo-Soneira, J.; Miret-Artés, S.; Pollak, E. Influence of realistic atom wall potentials in quantum reflection traps. Phys. Rev. A 2007, 75, 022902. [Google Scholar]
- Harber, D.M.; McGuirk, J.M.; Obrecht, J.M.; Cornell, E.A. Thermally Induced Losses in Ultra-Cold Atoms Magnetically Trapped Near Room-Temperature Surfaces. J. Low Temp. Phys. 2003, 133, 229–238. [Google Scholar] [CrossRef]
- Leanhardt, A.E.; Shin, Y.; Chikkatur, A.P.; Kielpinski, D.; Ketterle, W.; Pritchard, D.E. Bose-Einstein Condensates Near a Microfabricated Surface. Phys. Rev. Lett. 2003, 90, 100404. [Google Scholar] [CrossRef] [PubMed]
- Harber, D.M.; Obrecht, J.M.; McGuirk, J.M.; Cornell, E.A. Measurement of the Casimir-Polder force through center-of-mass oscillations of a Bose-Einstein condensate. Phys. Rev. A 2005, 72, 033610. [Google Scholar] [CrossRef]
- Lin, Y.-j.; Teper, I.; Chin, C.; Vuletić, V. Impact of the Casimir-Polder Potential and Johnson Noise on Bose-Einstein Condensate Stability Near Surfaces. Phys. Rev. Lett. 2004, 92, 050404. [Google Scholar] [CrossRef]
- Pethick, C.; Smith, H. Bose-Einstein Condensation in Dilute Gases; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar]
- Babb, J.F.; Klimchitskaya, G.L.; Mostepanenko, V.M. Casimir-Polder interaction between an atom and a cavity wall under the influence of real conditions. Phys. Rev. A 2004, 70, 042901. [Google Scholar] [CrossRef]
- Antezza, M.; Pitaevskii, L.P.; Stringari, S. Effect of the Casimir-Polder force on the collective oscillations of a trapped Bose-Einstein condensate. Phys. Rev. A 2004, 70, 053619. [Google Scholar] [CrossRef]
- Caride, A.O.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Zanette, S.I. Dependences of the van der Waals atom-wall interaction on atomic and material properties. Phys. Rev. A 2005, 71, 042901. [Google Scholar] [CrossRef]
- Babb, J.F. Long-range atom-surface interactions for cold atoms. J. Phys. Conf. Ser. 2005, 19, 001. [Google Scholar] [CrossRef]
- Mostepanenko, V.M.; Babb, J.F.; Caride, A.O.; Klimchitskaya, G.L.; Janette, S.I. Dependence of the Casimir-Polder interaction between atom and a cavity wall on atomic and material properties. J. Phys. A Math. Gen. 2006, 39, 6583–6588. [Google Scholar] [CrossRef]
- Blagov, E.V.; Klimchitskaya, G.L.; Mostepanenko, V.M. van der Waals interaction between a microparticle and a single-walled carbon nanotube. Phys. Rev. B 2007, 75, 235413. [Google Scholar] [CrossRef]
- Bezerra, V.B.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Romero, C. Lifshitz theory of atom-wall interaction with applications to quantum reflection. Phys. Rev. A 2008, 78, 042901. [Google Scholar] [CrossRef]
- Safari, H.; Welsch, D.-G.; Buhmann, S.Y.; Scheel, S. van der Waals potentials of paramagnetic atoms. Phys. Rev. A 2008, 78, 062901. [Google Scholar] [CrossRef]
- Bimonte, G.; Klimchitskaya, G.L.; Mostepanenko, V.M. Impact of magnetic properties on atom-wall interactions. Phys. Rev. A 2009, 79, 042906. [Google Scholar] [CrossRef]
- Haakh, H.; Intravaia, F.; Henkel, C.; Spagnolo, S.; Passante, R.; Power, B.; Sols, F. Temperature dependence of the magnetic Casimir-Polder interaction. Phys. Rev. A 2009, 80, 062905. [Google Scholar] [CrossRef]
- Ellingsen, S.Å.; Buhmann, S.Y.; Scheel, S. Temperature-Independent Casimir-Polder Forces Despite Large Thermal Photon Numbers. Phys. Rev. Lett. 2010, 104, 223003. [Google Scholar] [CrossRef]
- Chaichian, M.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Tureanu, A. Thermal Casimir-Polder interaction of different atoms with graphene. Phys. Rev. A 2012, 86, 012515. [Google Scholar] [CrossRef]
- Passante, R.; Rizzuto, L.; Spagnolo, S.; Tanaka, S.; Petrosky, T.Y. Harmonic oscillator model for the atom-surface Casimir-Polder interaction energy. Phys. Rev. A 2012, 85, 062109. [Google Scholar] [CrossRef]
- Ribeiro, S.; Scheel, S. Shielding vacuum fluctuations with graphene. Phys. Rev. A 2013, 88, 042519, Erratum in 2014, 89, 039904(E). [Google Scholar] [CrossRef]
- Arora, B.; Kaur, H.; Sahoo, B.K. C3 coefficients for the alkali atoms interacting with a graphene and carbon nanotube. J. Phys. B 2014, 47, 155002. [Google Scholar] [CrossRef]
- Kaur, K.; Kaur, J.; Arora, B.; Sahoo, B.K. Emending thermal dispersion interaction of Li, Na, K and Rb alkali-metal atoms with graphene in the Dirac model. Phys. Rev. B 2014, 90, 245405. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mostepanenko, V.M. Classical Casimir-Polder force between polarizable microparticles and thin films including graphene. Phys. Rev. A 2014, 89, 012516. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mostepanenko, V.M. Impact of graphene coating on the atom-plate interaction. Phys. Rev. A 2014, 89, 062508. [Google Scholar] [CrossRef]
- Knusnutdinov, N.; Kashapov, R.; Woods, L.M. Casimir-Polder effect for a stack of conductive planes. Phys. Rev. A 2016, 94, 012513. [Google Scholar] [CrossRef]
- Fuchs, S.; Crosse, J.A.; Buhmann, S.Y. Casimir-Polder shift and decay rate in the presence of nonreciprocal media. Phys. Rev. A 2017, 95, 023805. [Google Scholar] [CrossRef]
- Fuchs, S.; Bennett, R.; Krems, R.V.; Buhmann, S.Y. Nonadditivity of Optical and Casimir-Polder Potentials. Phys. Rev. Lett. 2018, 121, 083603. [Google Scholar] [CrossRef]
- Bordag, M.; Klimchitskaya, G.L.; Mostepanenko, V.M. Nonperturbative theory of atom-surface interaction: Corrections at short separations. J. Phys. Condens. Matter 2018, 30, 055003. [Google Scholar] [CrossRef]
- Henkel, C.; Klimchitskaya, G.L.; Mostepanenko, V.M. Influence of the chemical potential on the Casimir-Polder interaction between an atom and gapped graphene or a graphene-coated substrate. Phys. Rev. A 2018, 97, 032504. [Google Scholar] [CrossRef]
- Henkel, C.; Joulain, K.; Mulet, J.P.; Greffet, J.J. Radiation forces on small particles in thermal near fields. J. Opt. A Pure Appl. Opt. 2002, 4, S109–S114. [Google Scholar] [CrossRef]
- Antezza, M.; Pitaevskii, L.P.; Stringari, S. New Asymptotic Behavior of the Surface-Atom Force out of Thermal Equilibrium. Phys. Rev. Lett. 2005, 95, 113202. [Google Scholar] [CrossRef]
- Antezza, M.; Pitaevskii, L.P.; Stringari, S.; Svetovoy, V.B. Casimir-Lifshitz force out of thermal equilibrium. Phys. Rev. A 2008, 77, 022901. [Google Scholar] [CrossRef]
- Obrecht, J.M.; Wild, R.J.; Antezza, M.; Pitaevskii, L.P.; Stringari, S.; Cornell, E.A. Measurement of the temperature dependence of the Casimir-Polder force. Phys. Rev. Lett. 2007, 98, 063201. [Google Scholar] [CrossRef] [PubMed]
- Bezerra, V.B.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Romero, C. Advance and prospects in constraining the Yukawa-type corrections to Newtonian gravity from the Casimir effect. Phys. Rev. D 2010, 81, 055003. [Google Scholar] [CrossRef]
- Bezerra, V.B.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Romero, C. Constraints on the parameters of an axion from measurements of the thermal Casimir-Polder force. Phys. Rev. D 2014, 89, 035010. [Google Scholar] [CrossRef]
- Castillo-Garza, R.; Chang, C.-C.; Jimenez, D.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Experimental approaches to the difference in the Casimir force due to modifications in the optical properties of the boundary surface. Phys. Rev. A 2007, 75, 062114. [Google Scholar] [CrossRef]
- Torricelli, G.; van Zwol, P.J.; Shpak, O.; Binns, C.; Palasantzas, G.; Kooi, B.J.; Svetovoy, V.B.; Wuttig, M. Switching Casimir forces with phase-change materials. Phys. Rev. A 2010, 82, 010101(R). [Google Scholar] [CrossRef]
- Bordag, M.; Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. Advances in the Casimir Effect; Oxford University Press: Oxford, UK, 2015. [Google Scholar]
- Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. The Casimir force between real materials: Experiment and theory. Rev. Mod. Phys. 2009, 81, 1827–1885. [Google Scholar] [CrossRef]
- Shklovskii, B.I.; Efros, A.L. Electronic Properties of Doped Semiconductors; Springer: Berlin, Germany, 1984. [Google Scholar]
- Mott, N.F. Metal–Insulator Transitions; Taylor and Francis: London, UK, 1990. [Google Scholar]
- Chen, F.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Demonstration of optically modulated dispersion forces. Opt. Express 2007, 15, 4823–4829. [Google Scholar] [CrossRef] [PubMed]
- Chen, F.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Control of the Casimir force by the modification of dielectric properties with light. Phys. Rev. B 2007, 76, 035338. [Google Scholar] [CrossRef]
- Chang, C.C.; Banishev, A.A.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Reduction of the Casimir Force from Indium Tin Oxide Film by UV Treatment. Phys. Rev. Lett. 2011, 107, 090403. [Google Scholar] [CrossRef]
- Banishev, A.A.; Chang, C.C.; Castillo-Garza, R.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Modifying the Casimir force between indium tin oxide film and Au sphere. Phys. Rev. B 2012, 85, 045436. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mostepanenko, V.M. Conductivity of dielectric and thermal atom-wall interaction. J. Phys. A Math. Theor. 2008, 41, 312002. [Google Scholar] [CrossRef][Green Version]
- Geyer, B.; Klimchitskaya, G.L.; Mostepanenko, V.M. Thermal quantum field theory and the Casimir interaction between dielectrics. Phys. Rev. D 2005, 72, 085009. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. Casimir-Polder interaction between an atom and a dielectric plate: Thermodynamics and experiment. J. Phys. A Math. Theor. 2008, 41, 432001. [Google Scholar] [CrossRef][Green Version]
- Klimchitskaya, G.L.; Korikov, C.C. Casimir entropy for magnetodielectrics. J. Phys. Condens. Matter 2015, 27, 214007. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mostepanenko, V.M. Casimir free energy of dielectric films: Classical limit, low-temperature behavior and control. J. Phys. Condens. Matter 2017, 29, 275701. [Google Scholar] [CrossRef]
- Korikov, C.C.; Mostepanenko, V.M. Nernst heat theorem for the Casimir-Polder interaction between a magnetizable atom and ferromagnetic dielectric plate. Mod. Phys. Lett. A 2020, 35, 2040010. [Google Scholar] [CrossRef]
- Bimonte, G.; López, D.; Decca, R.S. Isoelectronic determination of the thermal Casimir force. Phys. Rev. B 2016, 93, 184434. [Google Scholar] [CrossRef]
- Woods, L.M.; Dalvit, D.A.R.; Tkatchenko, A.; Rodriguez-Lopez, P.; Rodriguez, A.W.; Podgornik, R. Materials perspective on Casimir and van der Waals interactions. Rev. Mod. Phys. 2016, 88, 045003. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mostepanenko, V.M. An alternative response to the off-shell quantum fluctuations: A step forward in resolution of the Casimir puzzle. Eur. Phys. J. C 2020, 80, 900. [Google Scholar] [CrossRef]
- Antezza, M. Surface-atom force out of thermal equilibrium and its effect on ultra-cold atoms. J. Phys. A Math. Gen. 2006, 39, 6117–6126. [Google Scholar] [CrossRef]
- Intravaia, F.; Henkel, C.; Antezza, M. Fluctuation-Induced Forces Between Atoms and Surfaces: The Casimir-Polder Interaction. In Casimir Physics; Dalvit, D., Milonni, P., Roberts, D., da Rosa, F., Eds.; Springer: Heidelberg, Germany, 2011; pp. 345–391. [Google Scholar]
- Yan, Z.-C.; Babb, J.F. Long-range interactions of metastable helium atoms. Phys. Rev. A 1998, 58, 1247–1252. [Google Scholar] [CrossRef]
- Brühl, R.; Fouquet, P.; Grisenti, R.E.; Toennies, J.P.; Hegerfeldt, G.C.; Köhler, T.; Stoll, M.; Walter, C. The van der Waals potential between metastable atoms and solid surfaces: Novel diffraction experiments vs. theory. Europhys. Lett. 2002, 59, 357–363. [Google Scholar] [CrossRef]
- Derevianko, A.; Johnson, W.R.; Safronova, M.S.; Babb, J.F. High-Precision Calculations of Dispersion Coefficients, Static Dipole Polarizabilities, and atom-wall interaction Constants for Alkali-Metal Atoms. Phys. Rev. Lett. 1999, 82, 3589–3593. [Google Scholar] [CrossRef]
- Safronova, M.S.; Williams, C.J.; Clark, C.W. Relativistic many-body calculations of electric-dipole matrix elements, lifetimes, and polarizabilities in rubidium. Phys. Rev. A 2004, 69, 022509. [Google Scholar] [CrossRef]
- Derevianko, A.; Porsev, S.G. Determination of lifetimes of 6PJ levels and ground-state polarizability of Cs from the van der Waals coefficient C6. Phys. Rev. A 2002, 65, 053403. [Google Scholar] [CrossRef]
- Zylbersztejn, A.; Mott, N.F. Metal-insulator transition in vanadium dioxide. Phys. Rev. B 1975, 11, 4383–4395. [Google Scholar] [CrossRef]
- Verleur, H.W.; Barker, A.S., Jr.; Berglund, C.N. Optical Properties of VO2 between 0.25 and 5 eV. Phys. Rev. 1968, 172, 788–798. [Google Scholar] [CrossRef]
- Verleur, H.W. Determination of optical constants from reflectance or transmission measurements of bulk crystals or thin films. J. Opt. Soc. Am. 1968, 58, 1356–1364. [Google Scholar] [CrossRef]
- Ashcroft, N.W.; Mermin, N.D. Solid State Physics; Saunders Colledge: Philadelphia, PA, USA, 1976. [Google Scholar]
- Ingold, G.-L.; Klimchitskaya, G.L.; Mostepanenko, V.M. Nonequilibrium effects in the Casimir force between two similar metallic plates kept at different temperatures. Phys. Rev. A 2020, 101, 032506. [Google Scholar] [CrossRef]
- Chen, F.; Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. New Features of the Thermal Casimir Force at Small Separations. Phys. Rev. Lett. 2003, 90, 160404. [Google Scholar] [CrossRef]
- Hough, D.B.; White, L.H. The calculation of Hamaker constant from Lifshitz theory with application to wetting phenomena. Adv. Colloid Interface Sci. 1980, 14, 3–41. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Klimchitskaya, G.L.; Mostepanenko, V.M. Casimir-Polder Interaction of an Atom with a Cavity Wall Made of Phase-Change Material out of Thermal Equilibrium. Atoms 2021, 9, 4. https://doi.org/10.3390/atoms9010004
Klimchitskaya GL, Mostepanenko VM. Casimir-Polder Interaction of an Atom with a Cavity Wall Made of Phase-Change Material out of Thermal Equilibrium. Atoms. 2021; 9(1):4. https://doi.org/10.3390/atoms9010004
Chicago/Turabian StyleKlimchitskaya, Galina L., and Vladimir M. Mostepanenko. 2021. "Casimir-Polder Interaction of an Atom with a Cavity Wall Made of Phase-Change Material out of Thermal Equilibrium" Atoms 9, no. 1: 4. https://doi.org/10.3390/atoms9010004
APA StyleKlimchitskaya, G. L., & Mostepanenko, V. M. (2021). Casimir-Polder Interaction of an Atom with a Cavity Wall Made of Phase-Change Material out of Thermal Equilibrium. Atoms, 9(1), 4. https://doi.org/10.3390/atoms9010004





