Studies of the Effect of Seasonal Cycle on the Equatorial Quasi-Biennial Oscillation with a Chemistry-Climate Model
Abstract
1. Introduction
2. Model Simulations
3. Results
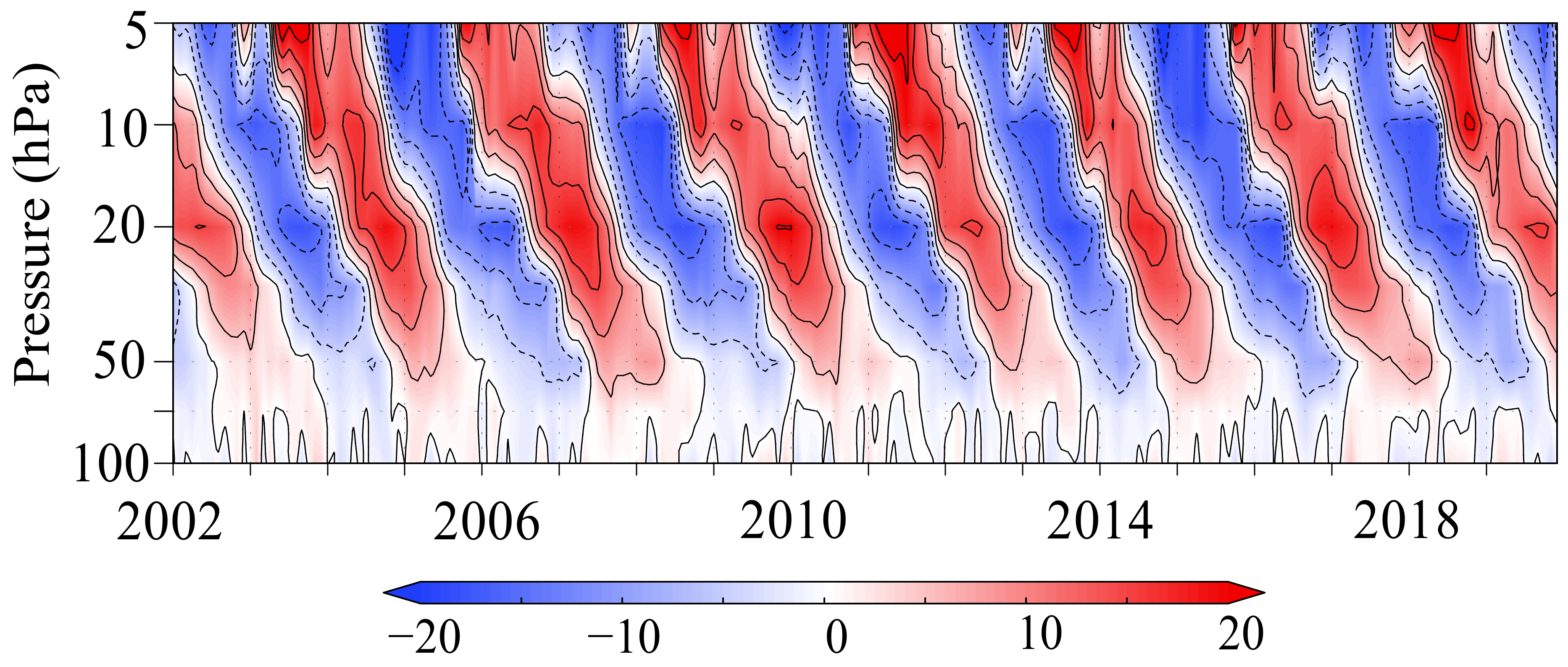
3.1. Control Run
3.2. Perpetual Runs


4. Discussion

5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Baldwin, M.P.; Gray, L.J.; Dunkerton, T.J.; Hamilton, K.; Haynes, P.H.; Randel, W.J.; Holton, J.R.; Alexander, M.J.; Hirota, I.; Horinouchi, T.; et al. The quasi-biennial oscillation. Rev. Geophys. 2001, 39, 179–229. [Google Scholar] [CrossRef]
- Lindzen, R.S.; Holton, J.R. A theory of quasi-biennial oscillation. J. Atmos. Sci. 1968, 25, 1095–1107. [Google Scholar] [CrossRef]
- Holton, J.R.; Lindzen, R.S. An updated theory for the quasi-biennial cycle of the tropical stratosphere. J. Atmos. Sci. 1972, 29, 1076–1080. [Google Scholar] [CrossRef]
- Plumb, R.A.; Bell, R.C. A model of the quasi-biennial oscillation on an equatorial beta-plane. Q. J. R. Meteorol. Soc. 1982, 108, 335–352. [Google Scholar] [CrossRef]
- Haynes, P.; Hitchcock, P.; Hitchman, M.; Yoden, S.; Hendon, H.; Kiladis, G.; Kodera, K.; Simpson, I. The influence of the stratosphere on the tropical troposphere. J. Meteorol. Soc. Jpn. 2021, 99, 803–845. [Google Scholar] [CrossRef]
- Hitchman, M.H.; Yoden, S.; Haynes, P.H.; Kumar, V.; Tegtmeier, S. An observational history of the direct influence of the stratospheric quasi-biennial oscillation on the tropical and subtropical upper troposphere and lower stratosphere. J. Meteorol. Soc. Jpn. 2021, 99, 239–267. [Google Scholar] [CrossRef]
- Camp, C.D.; Tung, K.K. The influence of the solar cycle and QBO on the late-winter stratospheric polar vortex. J. Atmos. Sci. 2007, 64, 1267–1283. [Google Scholar] [CrossRef]
- Petrick, C.; Matthes, K.; Dobslaw, H.; Thomas, M. Impact of the solar cycle and the QBO on the atmosphere and the ocean. J. Geophys. Res. 2012, 117, D17111. [Google Scholar] [CrossRef]
- Dunkerton, T.J.; Delisi, D.P. Climatology of the equatorial lower stratosphere. J. Atmos. Sci. 1985, 42, 376–396. [Google Scholar] [CrossRef]
- Dunkerton, T.J. Annual variation of deseasonalized mean flow acceleration in the equatorial lower stratosphere. J. Meteorol. Soc. Jpn. 1990, 68, 499–508. [Google Scholar] [CrossRef]
- Pawson, S.; Labitzke, K.; Lenschow, R.; Naujokat, B.; Rajewski, B.; Wiesner, M.; Wohlfart, R.-C. Climatology of the Northern Hemisphere Stratosphere Derived from Berlin Analyses, Pt. 1, Monthly Means. In Meteorologische Abhandlungen, Neue Folge. Serie A; Verlag von Dietrich Reimer: Berlin, Germany, 1993; 299p. [Google Scholar]
- Wallace, J.M.; Panetta, R.L.; Estberg, J. Representation of the equatorial stratospheric quasi-biennial oscillation in EOF phase space. J. Atmos. Sci. 1993, 50, 1751–1762. [Google Scholar] [CrossRef]
- Taguchi, M. Observed connection of the stratospheric quasi-biennial oscillation with El Niño–Southern Oscillation in radiosonde data. J. Geophys. Res. 2010, 115, D18120. [Google Scholar] [CrossRef]
- Yuan, W.; Geller, M.A.; Love, P.T. ENSO influence on QBO modulations of the tropical tropopause. Quart. J. Roy. Meteor. Soc. 2014, 140, 1670–1676. [Google Scholar] [CrossRef]
- Dunkerton, T.J. Modification of stratospheric circulation by trace constituent changes? J. Geophys. Res. 1983, 88, 10831–10836. [Google Scholar] [CrossRef]
- Naujokat, B. An update of the observed quasi-biennial oscillation of the stratospheric winds over the tropics. J. Atmos. Sci. 1986, 43, 1873–1877. [Google Scholar] [CrossRef]
- Salby, M.; Callaghan, P. Connection between the solar cycle and the QBO: The missing link. J. Clim. 2000, 13, 328–338. [Google Scholar] [CrossRef]
- Pascoe, C.L.; Gray, L.J.; Crooks, S.A.; Juckes, M.N.; Baldwin, M.P. The quasi-biennial oscillation: Analysis using ERA-40 data. J. Geophys. Res. 2005, 110, D08105. [Google Scholar] [CrossRef]
- Shibata, K.; Naoe, H. Decadal amplitude modulations of the stratospheric quasi-biennial oscillation. J. Meteorol. Soc. Jpn. 2021, 100, 29–44. [Google Scholar] [CrossRef]
- Onogi, K.; Tsutsui, J.; Koide, H.; Sakamoto, M.; Kobayashi, S.; Hatsushika, H.; Matsumoto, T.; Yamazaki, N.; Kamahori, H.; Takahashi, K.; et al. The JRA-25 Reanalysis. J. Meteorol. Soc. Jpn. 2007, 85, 369–432. [Google Scholar] [CrossRef]
- Taguchi, M.; Shibata, K. Diagnosis of annual synchronization of the quasi-biennial oscillation: Results from JRA-25/JCDAS reanalysis and MRI chemistry-climate model data. J. Meteorol. Soc. Jpn. 2013, 91, 243–256. [Google Scholar] [CrossRef]
- Kinnersley, J.S.; Pawson, S. The descent rates of the shear zones of the equatorial QBO. J. Atmos. Sci. 1996, 53, 1937–1949. [Google Scholar] [CrossRef]
- Hampson, J.E.; Haynes, P.H. Phase alignment of the tropical stratospheric QBO in the annual cycle. J. Atmos. Sci. 2004, 21, 2627–2637. [Google Scholar] [CrossRef]
- Rajendran, K.; Moroz, I.M.; Read, P.L.; Osprey, S.M. Synchronisation of the equatorial QBO by the annual cycle in tropical upwelling in a warming climate. Q. J. R. Meteorol. Soc. 2016, 142, 1111–1120. [Google Scholar] [CrossRef]
- Monier, E.; Weare, B.C. Climatology and trends in the forcing of the stratospheric zonal-mean flow. Atmos. Chem. Phys. 2011, 11, 12751–12771. [Google Scholar] [CrossRef]
- Williamson, G.S.; Williamson, D.L. Circulation statistics from seasonal and perpetual January and July simulations with the NCAR Community Climate Model (CCM1): R15. 1987. NCAR Technical Note, NCAR/TN-302+STR. University Corporation for Atmospheric Research. Available online: https://opensky.ucar.edu/islandora/object/technotes:413 (accessed on 5 June 2022).
- Yoden, S.; Yamaga, T.; Pawson, S.; Langematz, U. A composite analysis of the stratospheric sudden warmings simulated in a perpetual January integration of the Berlin TSM GCM. J. Meteorol. Soc. Jpn. 1999, 77, 431–445. [Google Scholar] [CrossRef]
- Shibata, K. Simulations of ozone feedback effects on the equatorial quasi-biennial oscillation with a chemistry–climate model. Climate 2021, 9, 123. [Google Scholar] [CrossRef]
- Shibata, K.; Deushi, M. Simulation of the stratospheric circulation and ozone during the recent past (1980–2004) with the MRI chemistry-climate model. In CGER’s Supercomputer Monograph Report; National Institute for Environmental Studies: Tsukuba, Japan, 2008; Volume 13, 154p. [Google Scholar]
- Hines, C.O. Doppler-spread parameterization of gravity-wave momentum deposition in the middle atmosphere. Part 2: Broad and quasi monochromatic spectra, and implementation. J. Atmos. Sol.-Terr. Phys. 1997, 59, 387–400. [Google Scholar] [CrossRef]
- Rayner, N.A.; Parker, D.E.; Horton, E.B.; Folland, C.K.; Alexander, L.V.; Rowell, D.P.; Kent, E.C.; Kaplan, A. Global analyses of sea surface temperature, sea ice, and night marine air temperature since the late nineteenth century. J. Geophys. Res. 2003, 108, 4407. [Google Scholar] [CrossRef]
- Morgenstern, O.; Giorgetta, M.; Shibata, K. Chemistry climate models and scenarios. In SPARC CCMVal Report on the Evaluation of Chemistry-Climate Models; Eyring, V., Shepherd, T.G., Waugh, D.W., Eds.; SPARC Report No. 5, WCRP-132, WMO/TD-No. 1526; SPARC: Starnberg, Germany, 2010; pp. 17–70. Available online: http://www.atmosp.physics.utoronto.ca/SPARC (accessed on 5 June 2022).
- Kawatani, Y.; Hamilton, K.; Miyazaki, K.; Fujiwara, M.; Anstey, J.A. Representation of the tropical stratospheric zonal wind in global atmospheric reanalyses. Atmos. Chem. Phys. 2016, 16, 6681–6699. [Google Scholar] [CrossRef]
- Garcia, R.R.; Dunkerton, T.J.; Lieberman, R.S.; Vincent, R.A. Climatology of the semiannual oscillation of the tropical middle atmosphere. J. Geophys. Res. 1997, 102, 26019–26032. [Google Scholar] [CrossRef]
- Smith, A.K.; Garcia, R.R.; Moss, A.C.; Mitchell, N.J. The semiannual oscillation of the tropical zonal wind in the middle atmosphere derived from satellite geopotential height retrievals. J. Atmos. Sci. 2017, 74, 2413–2425. [Google Scholar] [CrossRef]
- Kawatani, Y.; Hirooka, T.; Hamilton, K.; Smith, A.K.; Fujiwara, M. Representation of the equatorial stratopause semiannual oscillation in global atmospheric reanalyses. Atmos. Chem. Phys. 2020, 20, 9115–9133. [Google Scholar] [CrossRef]
- Charlton, A.J.; Polvani, L.M. A new look at stratospheric sudden warmings, Part I: Climatology and modeling benchmarks. J. Clim. 2007, 20, 449–469. [Google Scholar] [CrossRef]
- Hamilton, K.; Mahlman, J.D. General circulation model simulation of the semiannual oscillation in the tropical middle atmosphere. J. Atmos. Sci. 1988, 45, 3212–3235. [Google Scholar] [CrossRef]
- Ray, E.A.; Alexander, M.J.; Holton, J.R. An analysis of the structure and forcing of the equatorial semiannual oscillation in zonal wind. J. Geophys. Res. 1998, 103, 1759–1774. [Google Scholar] [CrossRef]
- Shibata, K.; Deushi, M. Partitioning between resolved wave forcing and unresolved gravity wave forcing to the quasi-biennial oscillation as revealed with a coupled chemistry-climate model. Geophys. Res. Lett. 2005, 32, L12820. [Google Scholar] [CrossRef]
- Duchon, C.E. Lanczos filtering in one and two dimensions. J. Appl. Meteor. 1979, 18, 1016–1022. [Google Scholar] [CrossRef]
- Ling, X.-D.; London, J. The quasi-biennial oscillation of ozone in the tropical middle stratosphere: A one-dimensional model. J. Atmos. Sci. 1986, 43, 3122–3137. [Google Scholar]
- Naoe, H.; Deushi, M.; Yoshida, K.; Shibata, K. Future changes in the ozone quasi-biennial oscillation with increasing GHGs and ozone recovery in CCMI simulations. J. Clim. 2017, 30, 6977–6997. [Google Scholar] [CrossRef]
- Lubis, S.W.; Matthes, K.; Omrani, N.; Harnik, N.; Wahl, S. Influence of the QBO and sea surface temperature variability on downward wave coupling in the Northern Hemisphere. J. Atmos. Sci. 2016, 73, 1943–1965. [Google Scholar] [CrossRef]
- Silverman, V.; Harnik, N.; Matthes, K.; Lubis, S.W.; Wahl, S. Radiative effects of ozone waves on the Northern Hemisphere polar vortex and its modulation by the QBO. Atmos. Chem. Phys. 2018, 18, 6637–6659. [Google Scholar] [CrossRef]
- Holton, J.R.; Tan, H.-C. The quasi-biennial oscillation in the Northern Hemisphere lower stratosphere. J. Meteorol. Soc. Jpn. 1982, 60, 140–148. [Google Scholar] [CrossRef]
- Naoe, H.; Shibata, K. Equatorial quasi-biennial oscillation influence on northern winter extratropical circulation. J. Geophys. Res. 2010, 115, D19102. [Google Scholar] [CrossRef]
- Inoue, M.; Takahashi, M.; Naoe, H. Relationship between the stratospheric quasi-biennial oscillation and tropospheric circulation in northern autumn. J. Geophys. Res. 2011, 116, D24115. [Google Scholar] [CrossRef]
- Richter, J.H.; Matthes, K.; Calvo, N.; Gray, L.J. Influence of the quasi-biennial oscillation and El Niño–Southern Oscillation on the frequency of sudden stratospheric warmings. J. Geophys. Res. 2011, 116, D20111. [Google Scholar] [CrossRef][Green Version]
- Hansen, F.; Matthes, K.; Petrick, C.; Wang, W. The influence of natural and anthropogenic factors on major stratospheric sudden warmings. J. Geophys. Res. 2014, 119, 8117–8136. [Google Scholar] [CrossRef][Green Version]








Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shibata, K. Studies of the Effect of Seasonal Cycle on the Equatorial Quasi-Biennial Oscillation with a Chemistry-Climate Model. Climate 2022, 10, 99. https://doi.org/10.3390/cli10070099
Shibata K. Studies of the Effect of Seasonal Cycle on the Equatorial Quasi-Biennial Oscillation with a Chemistry-Climate Model. Climate. 2022; 10(7):99. https://doi.org/10.3390/cli10070099
Chicago/Turabian StyleShibata, Kiyotaka. 2022. "Studies of the Effect of Seasonal Cycle on the Equatorial Quasi-Biennial Oscillation with a Chemistry-Climate Model" Climate 10, no. 7: 99. https://doi.org/10.3390/cli10070099
APA StyleShibata, K. (2022). Studies of the Effect of Seasonal Cycle on the Equatorial Quasi-Biennial Oscillation with a Chemistry-Climate Model. Climate, 10(7), 99. https://doi.org/10.3390/cli10070099




