Characteristics of a Heat Exchanger in a Liquid Rocket Engine Using Conjugate Heat Transfer Coupling with Open-Source Tools

Abstract

Since a heat exchanger used in a gas generator of an open-cycle liquid rocket engine was operated in a high-temperature environment, the coupled analysis for heat transfer characteristics and structural integrity should be performed simultaneously. For these reasons, a numerical analysis of the heat exchanger in a liquid rocket engine was performed to elucidate the effects of heat transfer and structural deformation simultaneously using conjugate heat transfer (CHT) analysis and open-source tools. For the baseline heat exchanger, which had an inner helically coiled tube with nine turns ($N_c=9$), the heat transfer characteristics were investigated and findings showed that the heat transfer performance was reduced from the sixth turn. Further analysis was performed to examine the effect of the number of turns in terms of heat flux and the corresponding pressure drop and the weight of the structure. The results indicated that the heat exchanger with $N_c=3$ had a significantly reduced outlet temperature due to an excessively shortened flow residence time. The heat exchanger with $N_c=6$ showed an outlet temperature similar to that of the baseline; it also presented advantages in terms of the pressure drop and structure weight. In addition, the thermal deformation and stress caused by temperature changes were numerically investigated to consider the structural integrity of the heat exchanger with $N_c=\mathrm{3,6},9$. Further numerical analyses were performed at various flow rates. As the flow rate of helium increased, the amount of heat received from the high-temperature exhaust gas from the gas generator increased but the outlet temperature of helium decreased gradually. Finally, the temperature difference between the outer and inner walls increased due to the high heat flux in the region around the inlet, resulting in an increase in thermal stress. Based on these results, the optimal shape and flow rate of the system were identified. Furthermore, the heat transfer performance was found to correlate with the flow characteristics of the coiled tube.

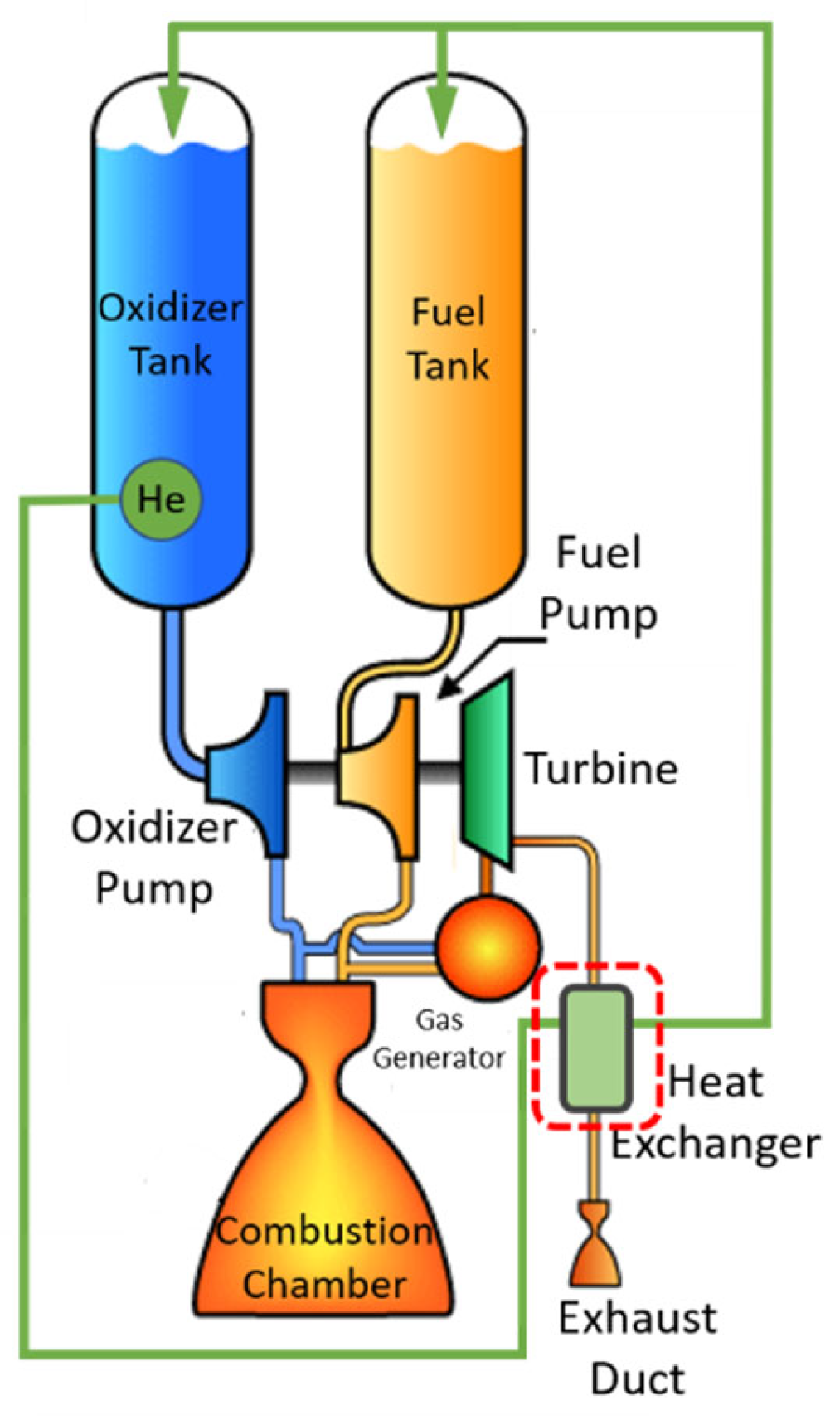
1. Introduction

Liquid propulsion systems are mainly divided into pressurized and pump-fed ones. For the high performance of space launch vehicles, pump-fed liquid rocket engines have been widely used like Saturn V and Falcon 9 in the USA and Ariane 5 in Europe [1,2,3]. The liquid rocket engine of the Korean space launch vehicle Nuri is currently being developed in Korea, also using an engine system with a turbopump [4]. This engine system generates the thrust of the launch vehicle by boosting the propellant, which is stored at low pressure, to high pressure through the turbopump system and by supplying the high-pressure propellant to the main combustion chamber. The complicated engine structure makes it difficult to design and assemble the engine system but it significantly reduces the launch vehicle’s weight by lowering the storage pressure of the propellant tank. However, an additional combustor, such as a gas generator or a preburner, is required to operate the turbines in the turbopump, which is necessary for the operation of the main engine [5].

Pump-fed liquid propulsion systems are classified as open or closed depending on the path of the fluid that drives a preburner for turbopump operation. In an open system as shown in Figure 1, a gas generator is operated under fuel-rich conditions to achieve the thermally stable operation of the turbopump and dump the gas out to the ambient environment.

A heat exchanger is adopted for the pressurant to operate the launch vehicle efficiently via energy exchange with exhaust gases from the gas generator; these exhaust gases contribute slightly to thrust performance. This heat exchanger expands the pressurant from a storage tank installed inside a cryogenic oxidizer tank using high-temperature exhaust gas. The expanded pressurant is supplied back to the propellant tank as an efficient pressurant to supply propellants to the turbopump and drive pneumatic valves, among others [6]. A lot of research has been conducted on various pressurized systems. Matsumoto et al. [7] proposed a new self-pressurized system for propellants to reduce the total system weight by using a steady mathematical model. Karimi et al. [8,9] investigated a pressurized system with a special capsule stored by high-pressure gas in the upper-stage engine and proved that the heat exchanger installed in front of the tank can improve the performance of the pressurized system. Li et al. [10] considered a pressurized feed system of the dual-thrust hybrid rocket motor and studied the influences of some structural and initial state parameters on the performance of the motor.

The present heat exchanger is used to reuse the high-temperature exhaust combustion gas at this time. Helium, as a pressurant in the present study, is heated using the heat exchanger for fuel and oxidizer pressurization which enables the storage of helium for low-temperature pressurization, thereby reducing the weight of the storage tank. In addition, this approach prevents the efficiency reduction caused by freezing parts of the liquid rocket engine or lowering the temperatures of the fuel and oxidant [5,7].

For the stable operation of heat exchangers in liquid rocket engines, thermal expansion and the consequent structural deformation should be considered thoroughly to prevent possible problems, such as physical contact between the exchangers and exhaust duct due to excessive thermal expansion and so on. Therefore, the present study aims to investigate these aspects to establish a design procedure that maintains thermal and structural integrity.

Previous numerical studies have been conducted through separate conventional thermo-fluid and structural analyses for different purposes [11,12,13,14]. However, the interaction between the thermo-fluid and the structure affects each other’s behavior in actual operation. Therefore, numerical techniques that can simultaneously perform thermo-fluid and structural analyses are needed to simulate actual scenarios. Furthermore, a more realistic or practical approach is necessary to overcome ideal and simple conditions such as constant wall heat flux or an isothermal wall boundary. Careful consideration is needed under complex flow fields with different flow streams and other solid domains such as gas turbine blades [15].

In present heat exchangers, heat transfer occurs between helium (for pressurizing the oxidizer), high-temperature combustion gas from the gas generator, and the heat exchanger structure. If each domain is analyzed separately, then the results are likely to be under- or overestimated compared with those under actual operating conditions because coupled boundary conditions are improperly exchanged. Therefore, numerical analysis should be performed simultaneously considering the heat transfer in each domain. In this study, we perform a realistic numerical analysis of a heat exchanger with conjugate heat transfer (CHT); we consider thermo-fluid and structural analyses simultaneously using open-source codes [16]. Various commercial codes, such as Ansys Fluent and Abaqus, are widely used to perform CFD and CAE but they are costly. Recently, open-source numerical tools have been used to perform fluid and structural analyses to overcome the limits of commercial codes. These open-source codes can be used for free and their effectiveness and reliability are continuously validated by many users. OpenFOAM 5.0 is a widely used open-source code and contemporary tool for fluid analysis [17] and it is continuously evolving through active information sharing and exchange by multiple users. For structural analysis, CalculiX 2.15, consisting of a solver (CCX) and a pre/postprocessor (CGX), is an open-source finite element analysis software that uses an input format similar to that of Abaqus [18]. The preCICE is a coupling library for multiphysics analysis, such as fluid–structure interaction (FSI), conjugate heat transfer (CHT), and so on [19]. Data such as heat transfer characteristics and structural deformation are exchanged at the interfaces of the fluid and structural domains through preCICE. This data exchange involves transferring the initial data of node 1 in one domain A to node 2 in domain B located close to node 1. In this method, the fluid and structural domains are coupled by exchanging their data. The preCICE’s coupling function allows the interaction of separate analysis solvers, such as OpenFOAM and CalculiX. In this respect, we numerically analyze a heat exchanger in an open-cycle liquid rocket engine using CHT analysis and these open-source codes through preCICE. In this manner, the performance of the heat exchanger and its structural integrity in the presence of heat are evaluated and verified. Furthermore, we want to provide a useful heat transfer correlation for the thermal design of heat exchangers with coiled shapes in the relatively high-temperature combustion gas environment.

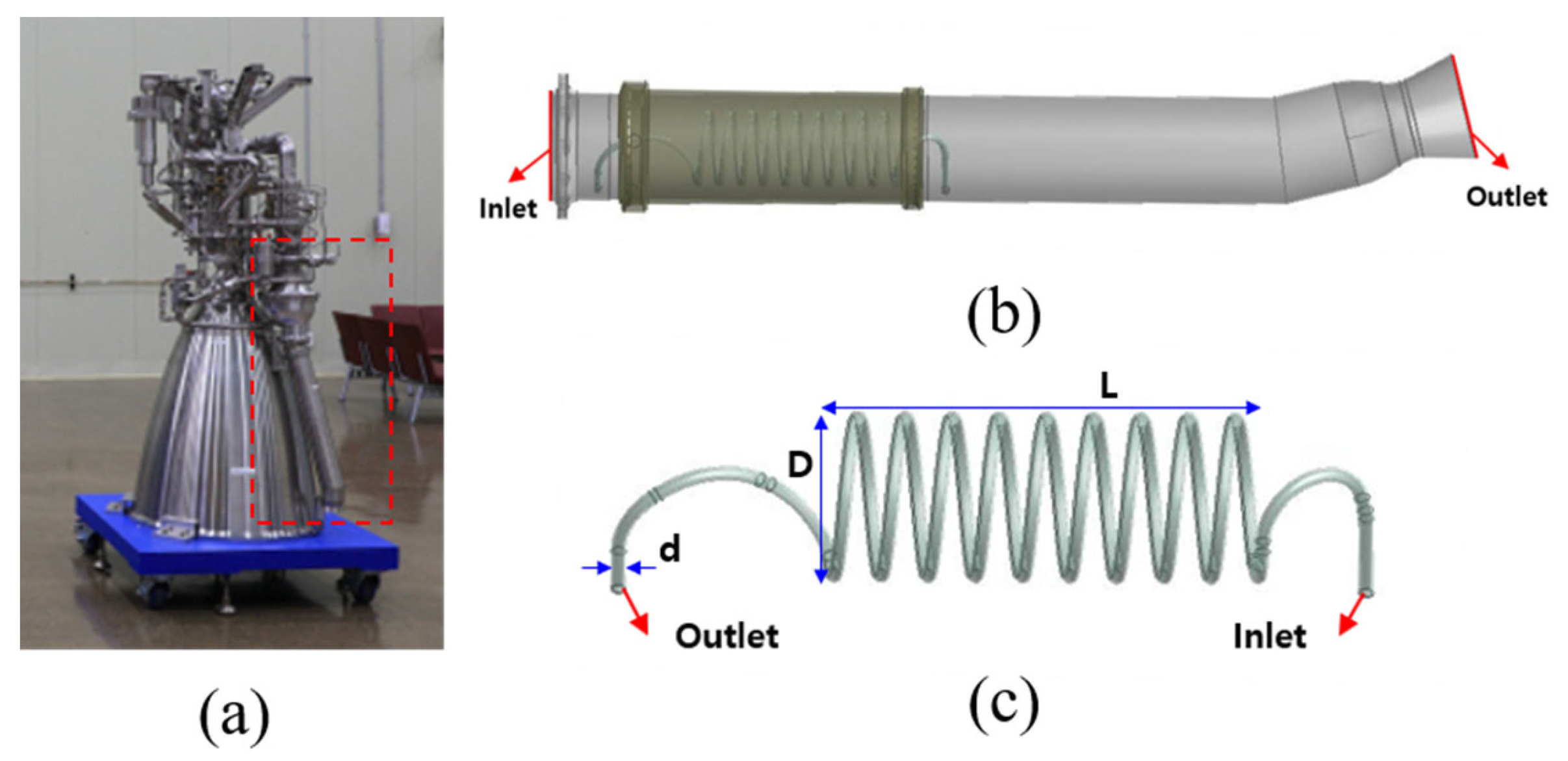
2. Numerical Setup

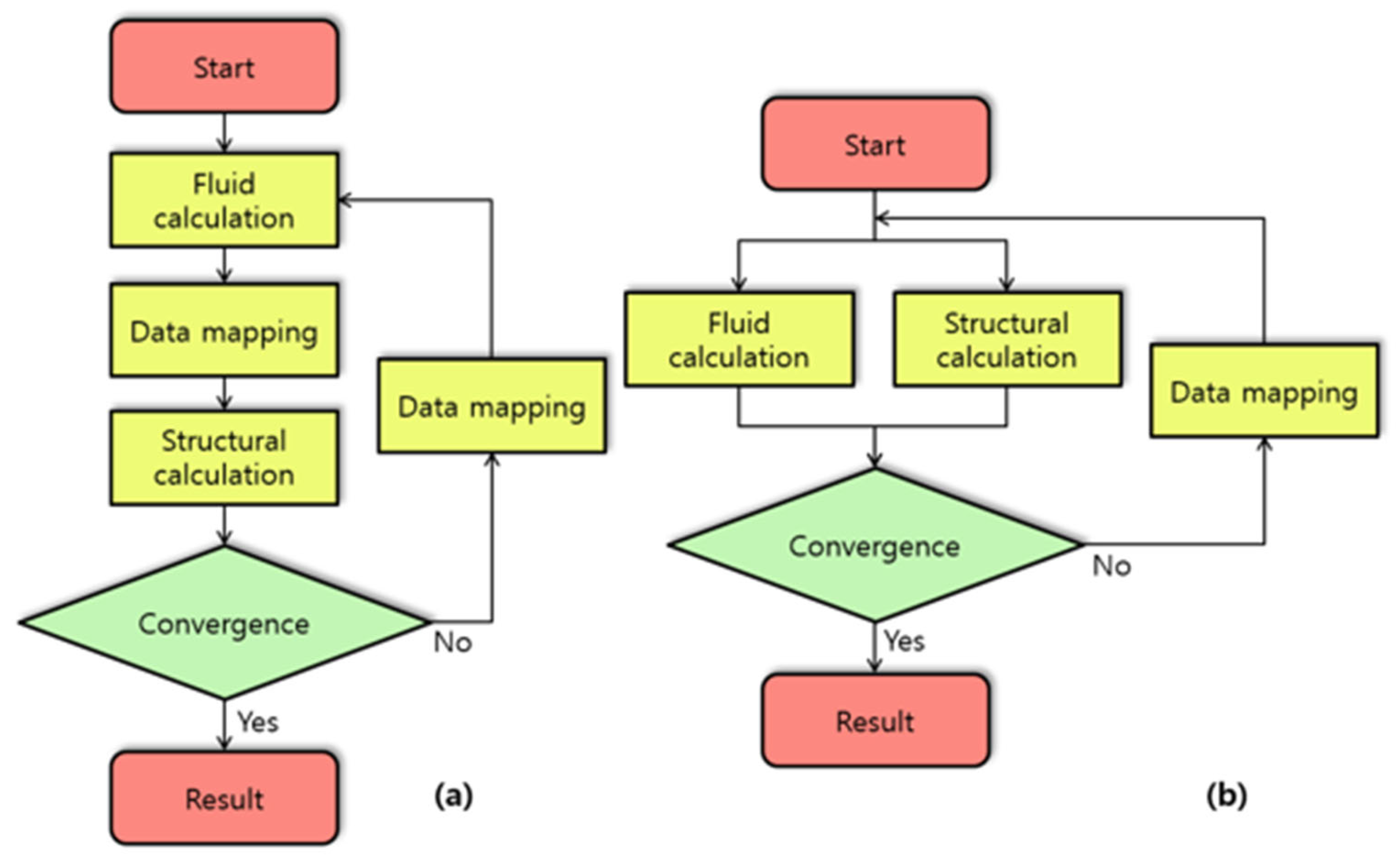
2.1. Methodology

Various studies have been conducted to implement the FSI and CHT analyses through multiphysics couplings. By comparing the experimental fluid analysis findings obtained by previous studies using ANSYS Fluent and OpenFOAM 2.3.1, Bhusare et al. verified that the difference between the results of the open-source and commercial codes was within 3% [20]. Yoon et al. compared the calculation results and time consumption of the commercial structural analysis code Abaqus and the open-source code CalculiX. Findings confirmed that the open-source and commercial codes qualitatively and quantitatively showed similar performance and results [21].

In this study, OpenFOAM 5.0 and CalculiX 2.15, open-source codes for fluid and structural analyses, respectively, were used to perform CHT analysis between the wall and working fluid of a heat exchanger. The preCICE tool was used to couple the interfaces of both solvers in every calculation step [22].

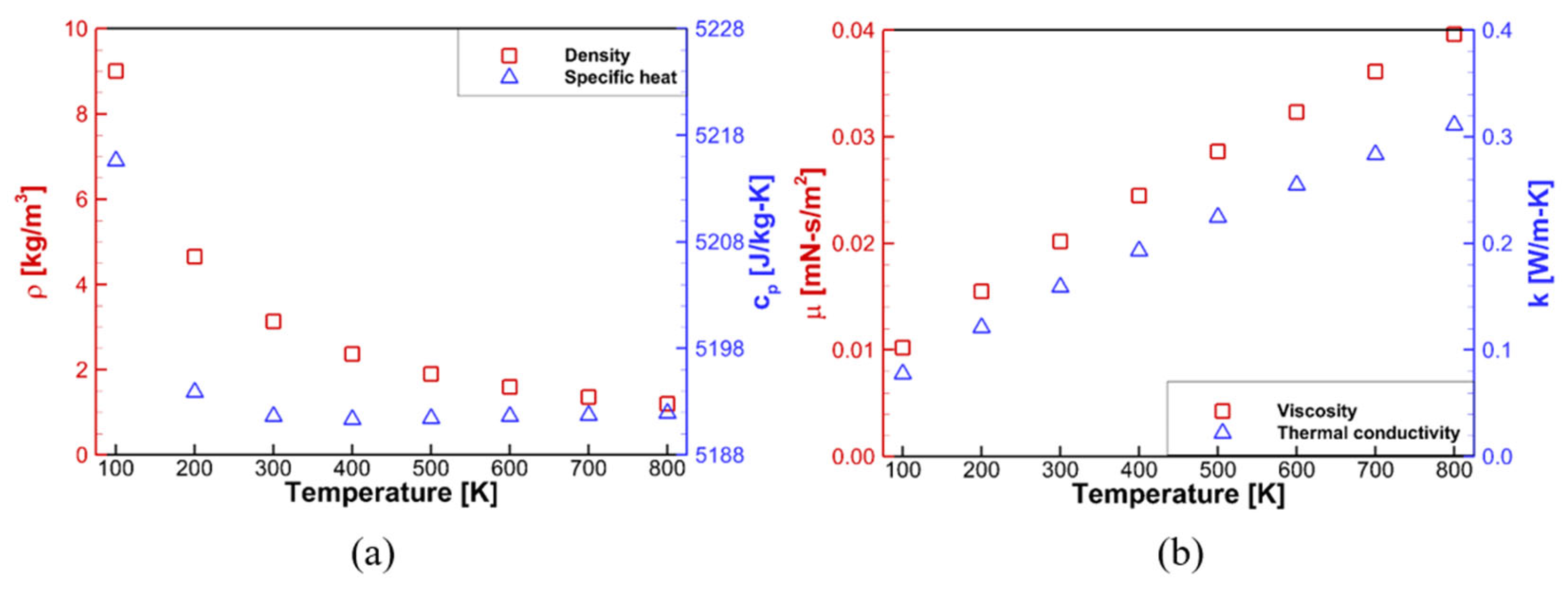
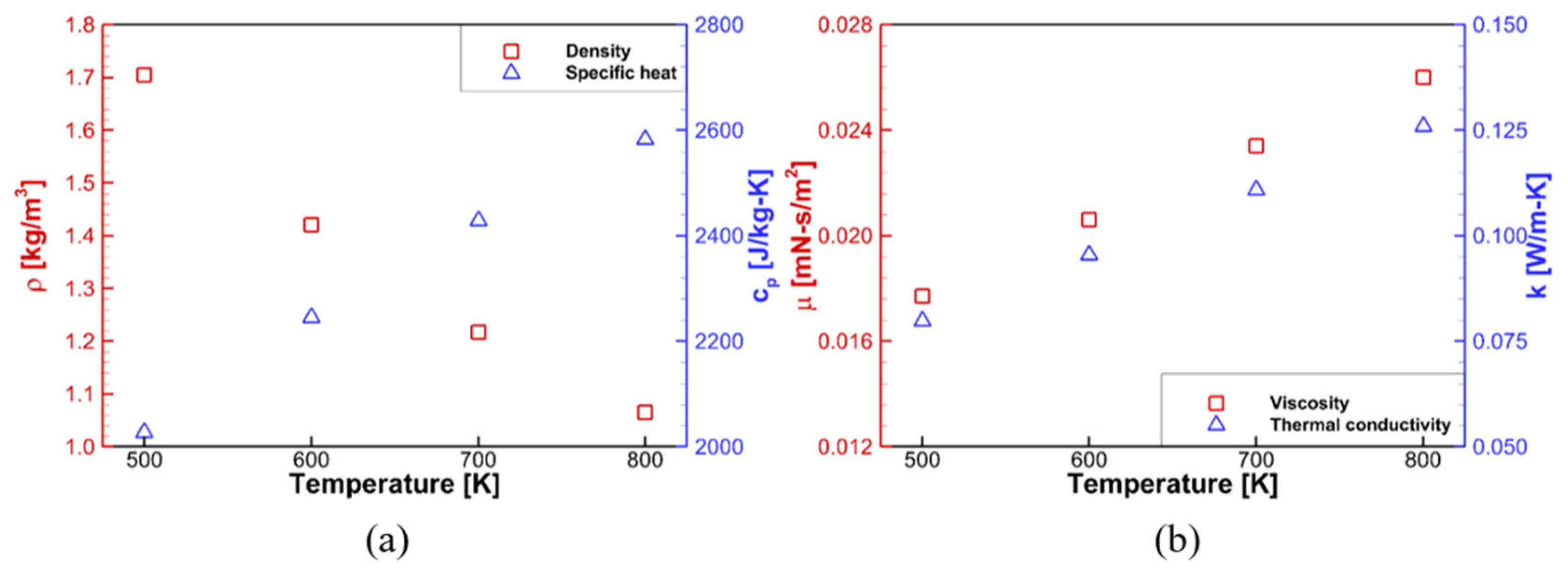
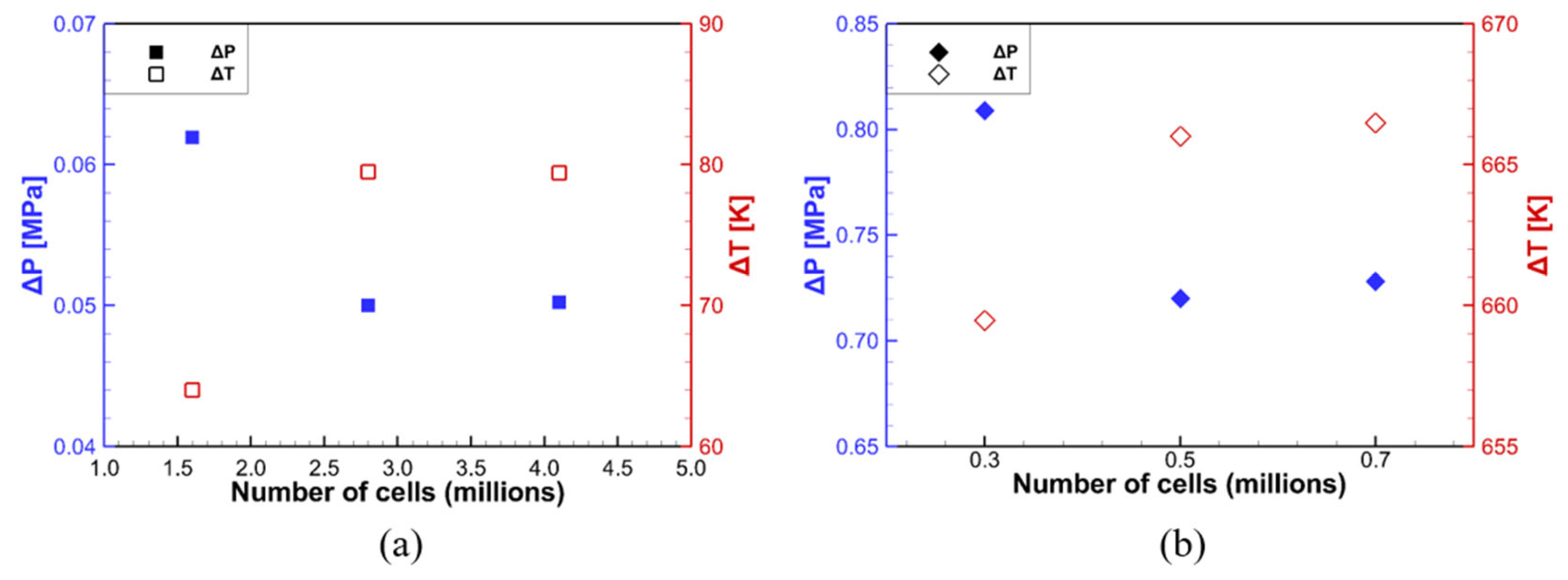
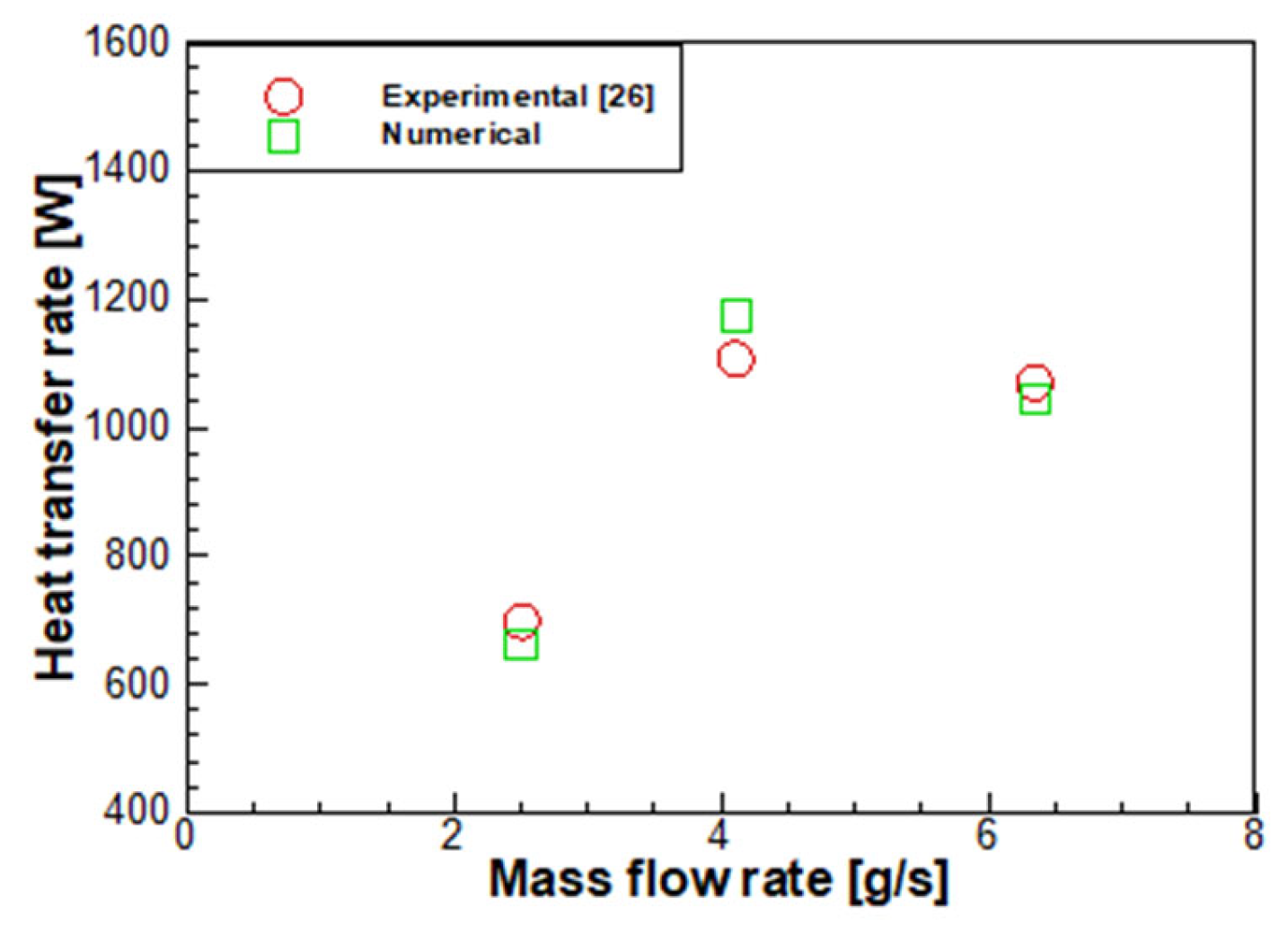
2.2. Numerical Analysis

The geometric configuration of the studied heat exchanger is shown in Figure 2. Fuel-rich and high-temperature exhaust gas from a gas generator (GG gas) flowed along an external flow path to the heat exchanger, which can be seen in Figure 1 and Figure 2. The fluid inside the helically coiled tube was helium, which is usually used as pressurant, i.e., pressurizing gas for propellants in actual liquid rocket engine systems. In this study, low-temperature helium flowed through an inner helically coiled tube with nine turns ($N_c=9$) and then heated for its pressurization function. This helically coiled tube could enhance the efficiency of heat transfer of the internal helium flow by increasing the flow residence time and contact heat transfer area.

Table 1. Design conditions of the baseline heat exchanger.

	GG Gas	Helium
Fluid solver	OpenFOAM
Structural solver	CalculiX
Inlet Temperature [K]	773	92
Outlet Pressure [MPa]	0.15	2.8
Mass flow rate [kg/s]	1.03	0.003

summarizes the computational and boundary conditions of the analyzed heat exchanger. Under these design conditions, the inlet temperature of GG gas was 773 K, the mass flow rate was 1.03 kg/s, and the outlet pressure was ${1.5\times 10}^5$ Pa. As the temperature of GG gas would decrease through the heat transfer with helium, a non-reacting flow condition was assumed in the GG gas region. In the case of helium, the inlet temperature was 92 K, the mass flow rate was 0.003 kg/s, and the outlet pressure was ${2.8\times 10}^6$ Pa. In addition, a standard k-epsilon model was used to calculate the turbulent effects. STS 316L was used as the material for the heat exchanger wall and outer exhaust duct. As the temperature variation in two fluids in their flow paths cannot be negligible, the effect of temperature dependence was included in the evaluation of the thermodynamic and transport properties of GG gas and pressurizing helium. The properties of GG gas were assessed via fuel-rich combustion analysis [23]. Fitted data for temperatures based on these properties at various temperatures, as shown in Figure 3 and Figure 4, were used to evaluate the fluid properties.

In the CHT analysis of the heat exchanger using the open-source codes, each fluid and structure were separated into four domains (helium, GG gas, helically coiled tube, and outer casing of heat exchanger). The temperature data and heat transfer coefficient at each interface should be exchanged between the fluid and structural domains for proper coupling. Such coupling was implemented using preCICE [19]. Figure 5 shows a flowchart of the coupling calculation. In the case of serial coupling, each solver solved the iterations alternately. The first participant used data from the previous iteration of the second participant to compute its solution for the current iteration. Then, the first participant sent the data to the second one, which then computed its solution for the current iteration. In the case of parallel coupling, both solvers solved simultaneously using each other’s data from the previous iteration.

For the time-marching, explicit and implicit methods can be used. In the case of an explicit scheme, both participants are only executed once per time window. In the implicit scheme, the participants are executed multiple times until convergence. Therefore, the implicit scheme is more stable than the explicit one but acceleration techniques need to be applied to reduce the calculation time [19,24]. In the present calculation, the parallel-explicit scheme was applied.

Various mapping methods can be applied for an efficient mapping process under the different grid distributions. The nearest-neighbor method, which transfers data without interpolation through the first-order scheme, was applied in this work. Consistent mapping, a method used for intensive quantities (such as temperature and heat flux), was applied to the mapping constraint; in this approach, the value at a coarse node is the same as the value at the corresponding fine node [25].

First, a check for grid dependency was performed for the outer fluid (GG gas) domain to validate the reliability of the present methodology. Numerical analyses with about 1.4 million, 2.8 million, and 4.1 million grids for this domain were conducted, as shown in Figure 6a. For the grid number of 2.8 million, the thermofluidic characteristics of the GG gas domain showed satisfactory convergence agreement with each other and the difference between the numerical and experimental results was less than 5%. Therefore, 2.8 million was used as the grid number for the GG gas domain in the following investigation.

For the inner fluid (helium) domain, simulations with 0.3 million, 0.5 million, and 0.7 million meshes were tested, as shown in Figure 6b. All test results of pressure drop and temperature increase showed that the difference was less than 5% and 0.5 million grids were adequate for the modeling of the inner fluid considering the convergence and calculation time. Consequently, a system with about 3.3 million grids was adopted in the numerical simulations of all fluid domains.

Before the characteristics of the heat exchanger were studied, these numerical results were compared with previous experimental results [26] to validate the present CHT analysis approach, which features fluid–structure coupling using preCICE. These previous experiments were performed from the viewpoint of the heat transfer of helium flowing through a coiled flow path and the CHT simulations in the present paper were performed under the same conditions. Figure 7 shows a comparison of the numerical results and the abovementioned experimental findings in terms of the total heat transfer rate between the inlet and outlet of the heat exchanger. As shown in Figure 7, the error between the experimental and numerical results was less than 5%; thus, satisfactory agreement was obtained along with successful validation.

3. Results

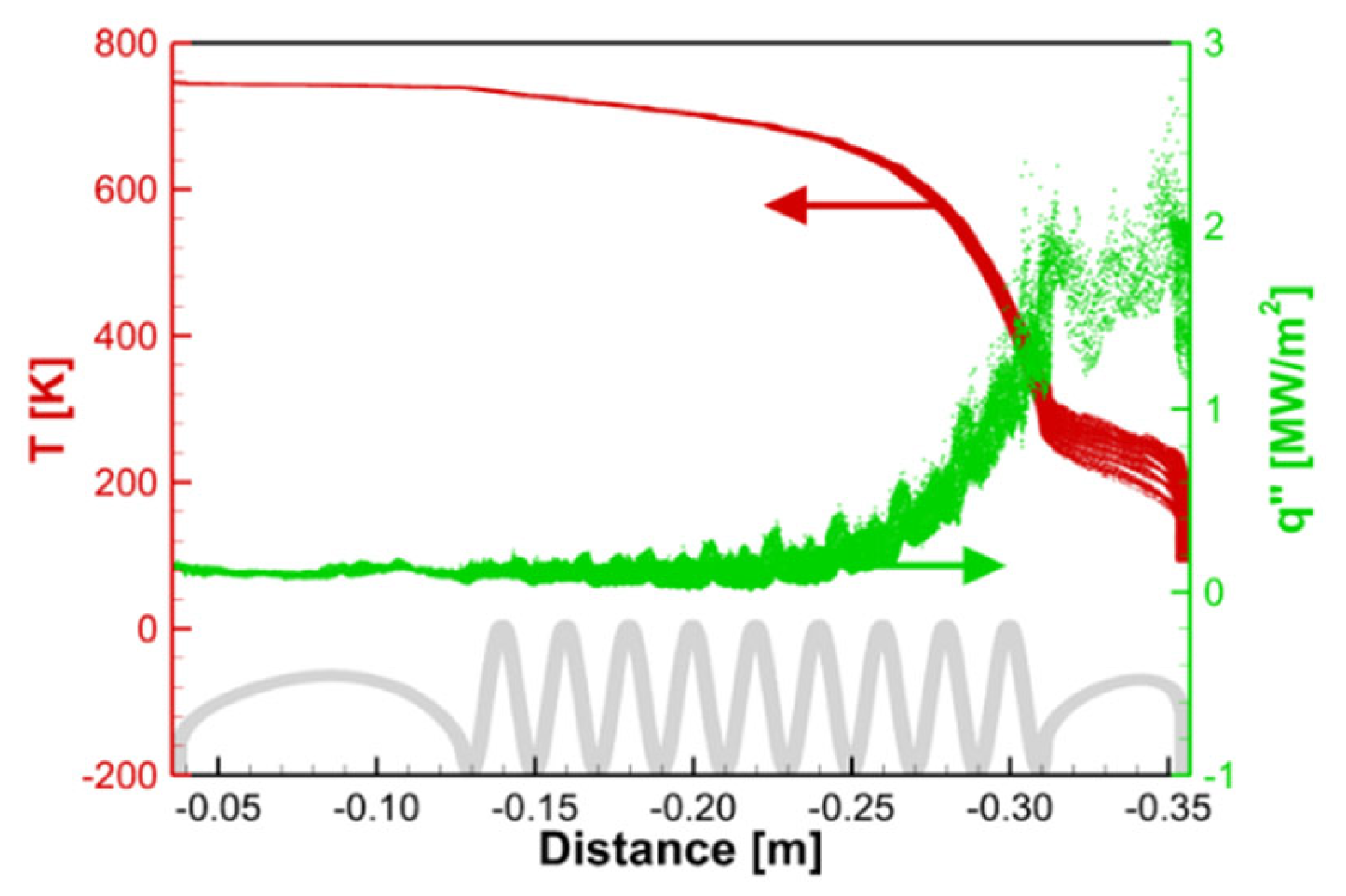
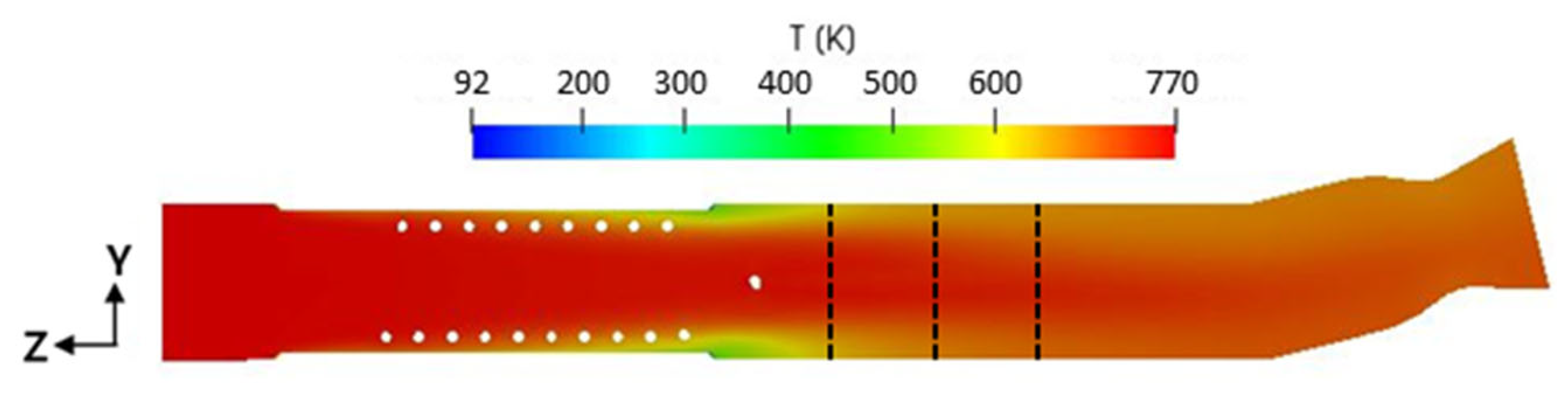
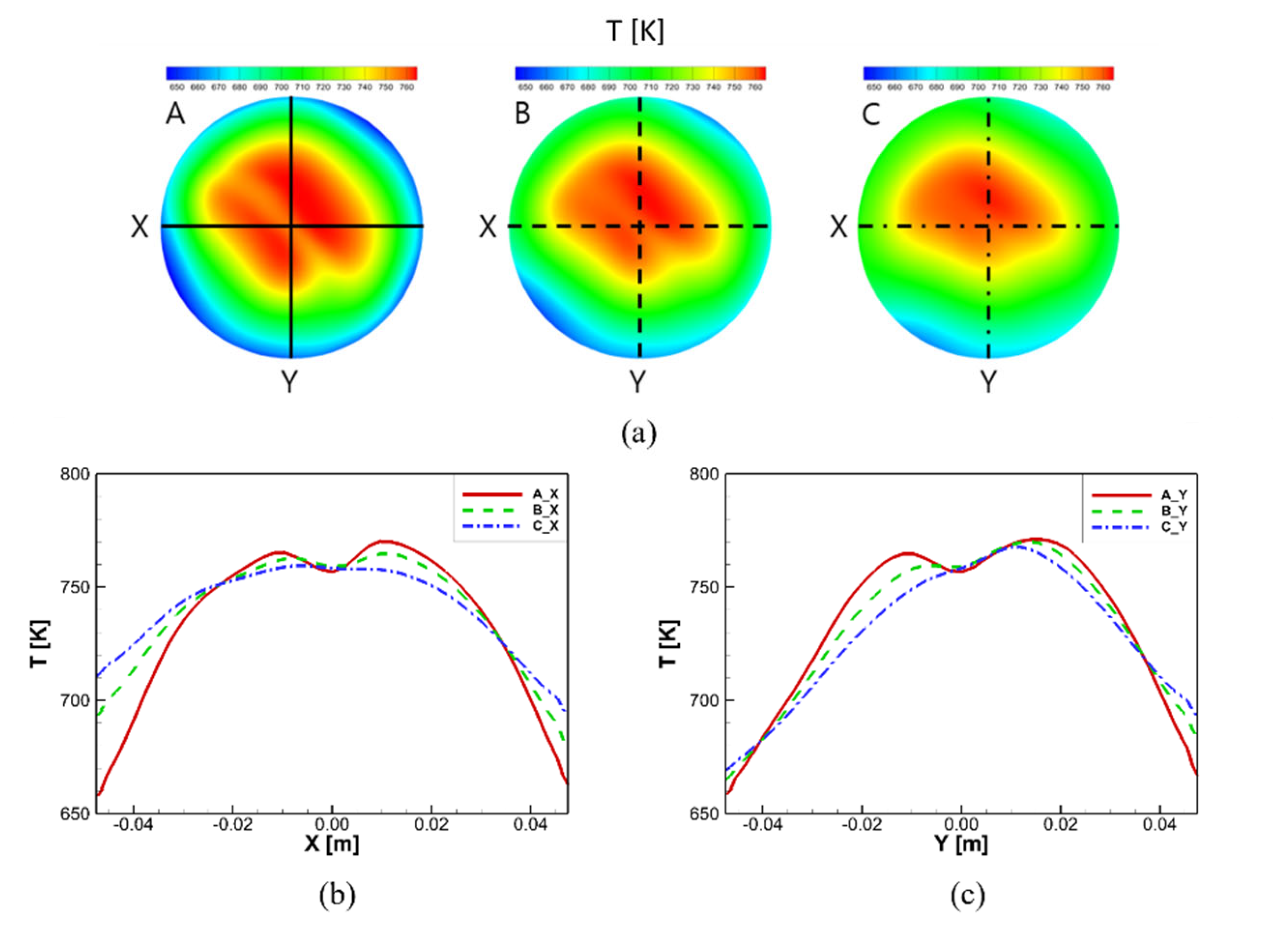
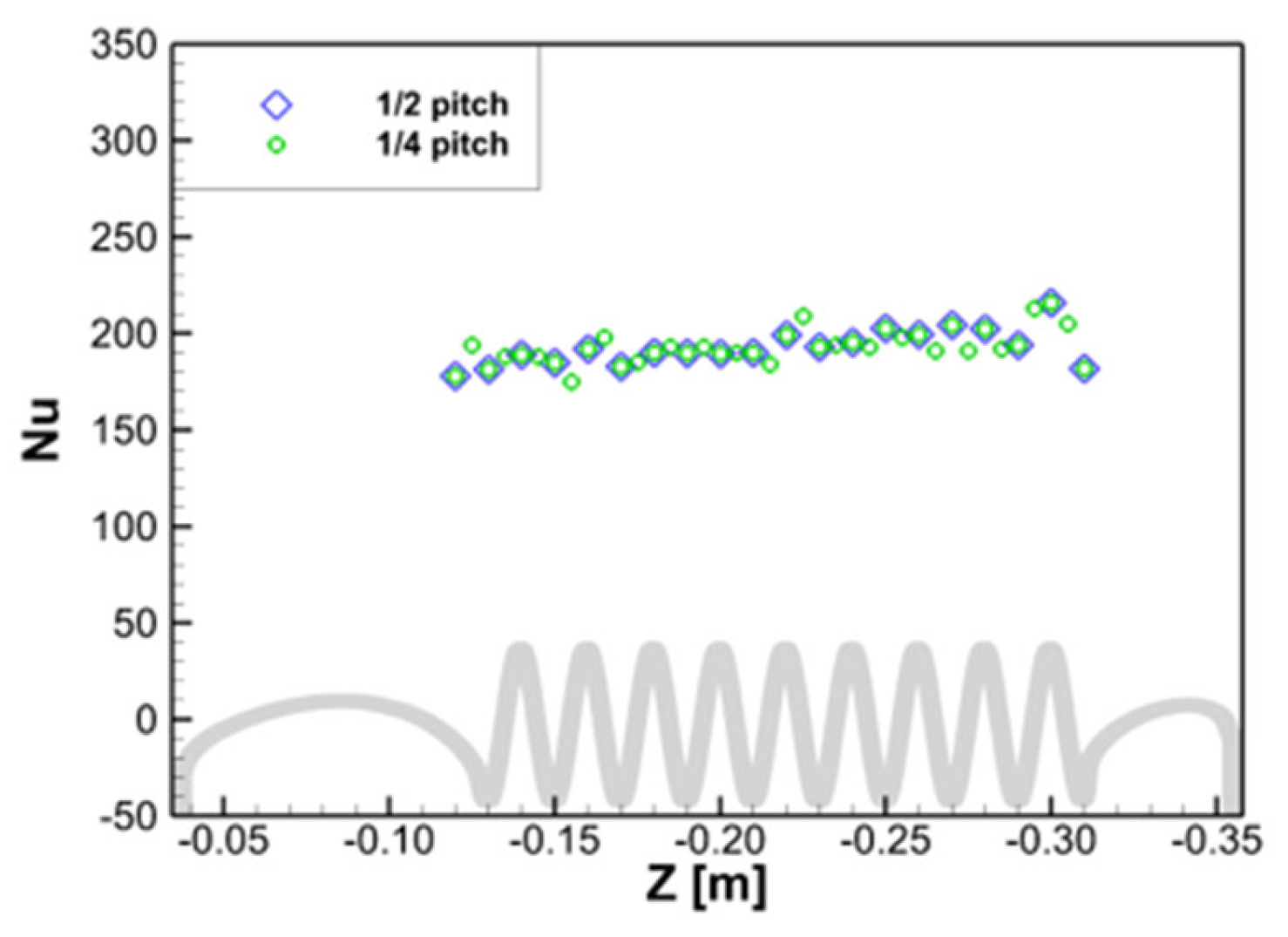
3.1. Behaviors of Helium in Helically-Coiled Tube with Nine Turns

The thermofluidic behaviors of helium in the helically-coiled tube with nine turns ($N_c=9$) in the baseline heat exchanger were numerically characterized. Figure 8 shows the distributions of temperature and pressure in the helically-coiled tube. As shown in Figure 8a, the temperature of helium increases through heat transfer from the high-temperature GG gas. As a result, helium is heated near the exit of this heat exchanger to about 758 K, which is close to the temperature of GG gas. For the pressure distribution of helium, a pressure drop of 0.7 MPa is required to reach the target mass flow rate in the case of $N_c=9$, as shown in Figure 8b.

Table 2. Pressure drop and temperature difference in a heat exchanger with $N_c=9$.

Helium P [MPa]	0.72
GG gas P [MPa]	0.05
Helium T [K]	666.0
GG gas T [K]	79.5

summarizes the required pressure drop and the temperature difference in each fluid due to heat exchange. Through heat transfer, the temperature of helium increases to about 758 K and the temperature of GG gas decreases to 693.5 K. The temperature difference in helium is higher than that of GG gas because the mass flow rate of GG gas is about 300 times larger than that of helium although the specific heat of helium is higher than that of GG gas.

The temperature and heat flux along the axial direction of the helically-coiled tube are shown in Figure 9 to elucidate the thermal characteristics. Near the inlet port, the local temperature is distributed with large variations and it becomes almost uniform as the flow moves toward the outlet. This behavior near the inlet is attributed to the prevalence of heat transfer along the coiled tube from near the hot wall region to the center of the tube. On the contrary, a low heat flux is typical near the outlet due to the decreased temperature difference between the hot GG gas and helium caused by the continuous heat transfer.

Figure 10 shows the temperature distribution of GG gas in the sliced center surface (y–z plane) of the exhaust duct. The temperature of GG gas decreases as the gas passes through the region of heat transfer due to the low-temperature helium. The high-temperature region is distributed in the central region of the outer casing of the heat exchanger where relatively less heat transfer occurs. In addition, temperature becomes increasingly uniform due to heat convection as the GG gas flows to the outlet. We verify the effects of convection by investigating the temperature distributions in regions A, B, and C, which are in the rear part of the heat exchanger. The x and y axis in Figure 11a show the distances from the center of the cross-sectional surface to A, B, and C. As shown in Figure 8, the temperature difference between the wall and center is relatively large in region A compared with the other regions. Moreover, a high-temperature region is seen near the center; it is not substantially affected by the low-temperature helium passing through the circumference of the GG gas flow path. As the flow proceeds from A to B and C, the temperature difference between the wall and center decreases due to heat convection. These temperature distributions caused by the convective heat transfer of GG gas and helium were used as the structural boundary conditions in predicting the thermal deformation and thermal stress, as discussed in Section 3.3.

Figure 12 shows the heat flux distribution in the helium and GG gas domains. The heat fluxes in the GG gas domain are lower than those in the helium domain because the former domain has a wide heat transfer area due to the thickness of the helically-coiled tube. As shown in Figure 12, the two domains have similar tendencies of heat flux depending on axial location. In addition, they have similar estimated heat transfer rates (less than 1% error). Therefore, the CHT coupling for these domains of the heat exchanger is qualitatively valid.

In Figure 9, the temperature change and heat flux of helium decreases as the flow progresses to the outlet. The normalized heat flux at the cross section of each location in the helically-coiled tube was investigated and is shown in Figure 13 to consider the heat transfer characteristics in detail. The normalized heat flux is the ratio of the maximum average heat flux of the first cross section (${q^{”}}_{max}$) and the average and the standard deviation of heat flux of all cross sections. The heat flux after the sixth turn in the helically-coiled tube maintains a low value ($0.05\times {q^{”}}_{max}$) and becomes nearly constant. Therefore, efficient heat transfer cannot be established after the sixth turn. In this respect, the shape of the baseline helically-coiled tube ($N_c=9$) is not assumed to be an optimized shape in the viewpoint of the number of turns. The averaged heat flux at the cross section of each location was further investigated from the viewpoint of geometric configuration (number of turns [$N_c$]) by considering the location from which the heat flux starts to decrease, as shown in Figure 13, to determine the optimal number of turns.

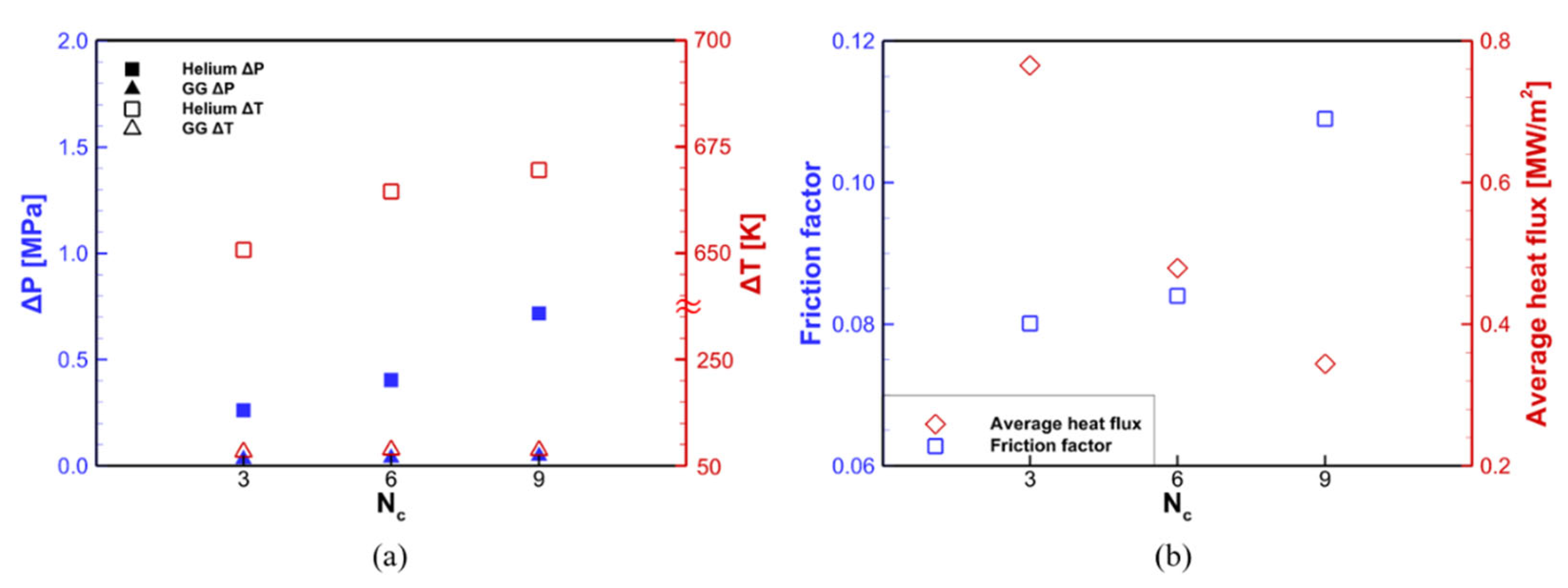
3.2. Effect of Number of Turns ($N_c$)

CHT analyses were performed on the heat exchanger with $N_c=3$ and $N_c=6$ to consider the effect of geometry on the heat transfer characteristics. Figure 14 shows the required pressure drop and temperature difference in each fluid due to heat transfer for each number of turns. As $N_c$ increases, the required pressure drop increases due to the lengthened flow residence time. In the case of GG gas, regardless of the $N_c$ value, the overall flow shape does not significantly change because the diameter of the GG gas domain is much larger than that of the helically-coiled tube. Therefore, the variation in pressure drop is much smaller than that in the case of helium. The temperature difference in GG gas with $N_c$ is smaller than that of helium because GG gas has a higher mass flow rate than helium. As for the helium outlet temperature, the heat exchanger with $N_c=3$ shows a large difference of 20 K from the baseline design ($N_c=9$) whereas the difference in the heat exchanger with $N_c=6$ is 5 K. If the helically-coiled tube is designed based only on heat transfer efficiency, then the outlet temperature of helium for $N_c=3$ is considerably lower than that of the baseline design ($N_c=9$) and sufficient heat transfer characteristics cannot be achieved. In this respect, the heat exchanger with $N_c=6$ can perform similarly to the baseline design, even if the flow residence time decreases according to the decreased number of turns.

In addition, the performance of the heat exchanger can be evaluated in terms of heat flux and friction factor. The friction factor has been often used to elucidate the performance of various heat exchangers in terms of useful dimensionless numbers such as the De or Re and the Nu number in previous studies [27]. Therefore, the characteristics of the friction factor should be included to quantify the heat transfer characteristics by correlating the trend of the friction factor according to the $N_c$. In this respect, the variations in the friction factor and average heat flux for various $N_c$ are shown in Figure 14b. The friction factor in the present study is defined as $\mathrm{f}=\left(\frac{d}{L}\right)\left(\frac{∆p}{\rho V^2/2}\right)$ where d is the inner diameter and L is the length of the coiled tube. As the $N_c$ decreases (in other words, the length of the heat exchanger decreases), the effect of friction becomes relatively less along the tube. On the contrary, since the fluid–structure interface area increases with the $N_c$, the average heat flux relatively decreases due to the increased area and the high-temperature difference between the hot burnt gas and He.

The variation in heat fluxes was investigated to elucidate the optimal condition of $N_c$. Figure 15 shows the normalized heat flux at the cross-section of each location of the heat exchanger with $N_c=3$ and $N_c=6$. In the case of $N_c=3$, the heat flux varies continuously along the axial direction. Thus, more considerable heat transfer may occur at the exit of the coiled tube and some expendable heat is not transferred to the helium. Consequently, the heat transfer is suboptimal due to the decreases in the area and corresponding residence time of heat transfer. Therefore, the outlet temperature is significantly lower than that for $N_c=9$ and a high heat flux ($\approx 0.2\times {q^{”}}_{max}$) near the exit of the coiled tube is maintained. In the case of $N_c=6$, the outlet temperature is almost identical to that for $N_c=9$ but a relatively lower heat flux ($\approx 0.07\times {q^{”}}_{max}$) is observed at the end of the helically-coiled tube. Hence, optimal heat transfer is obtained from the helically-coiled tube with six turns. The results of all three cases indicate that the heat exchanger with $N_c=3$ is insufficient for the heat transfer from the hot GG gas to helium. On the contrary, the heat exchanger with $N_c=6$ shows similar heat transfer characteristics to that with $N_c=9$ but the required pressure drop and corresponding structural mass are lower than those of the heat exchanger with $N_c=9$, such as $m_6=0.72 m_9$. In this respect, the number of turns of 6 or more is the optimal one of the helically-coiled tubes of the studied heat exchanger.

Due to the high temperature difference in the physical structures of heat exchangers, thermal stress and the resulting deformation can occur, thus compromising the structural integrity of heat exchangers. Therefore, thermal stress and deformation were numerically predicted to verify the structural integrity of the studied heat exchanger in the presence of temperature changes. As a preventive measure, the exit of the outer casing of the heat exchanger was initially designed to have a bent configuration. Hence, thermal expansion through heat transfer was checked.

Fixed physical boundary conditions were applied to the inlet and outlet of the helically-coiled tube. In addition, fixed boundary conditions were applied to the inlet of the outer casing of the heat exchanger to consider the axial thermal deformation of the casing, as shown in Figure 2. CHT and structural coupling were established through by interchanging the thermal and structural boundary conditions, as described in Section 2.

The structural analysis results for each number of turns ($N_c$) of the helically-coiled tube are shown in Figure 16 and Figure 17. Figure 16 shows the thermal stress distributions due to the temperature difference in the helically-coiled tube. Regions of high thermal stress are concentrated at the inlet of the helium tube due to the large temperature difference at the inlet of the helically-coiled tube as shown in Figure 16c specifically. As a result of fact that the distance between the inlet port and the helically-coiled tube is much shorter than that between the outlet port and the tube, relatively small deformation due to thermal expansion is predicted near the inlet port; such deformation appears as high-stress regions caused by the fixed boundary condition. In addition, the 0.2% offset yield stress (elastic limit) of STS 316L at room temperature is generally about 226 MPa but it increases as the temperature decreases [28,29]. STS 316L has a yield strength of 200 MPa above 450 K. The safety factor for this yield strength, which is shown in

Table 3. Safety factors for thermal stress for various $N_c$.

${\mathbf{N}}_{\mathbf{c}}$	$\mathbf{Safety} \mathbf{Factor} \left[\frac{\mathit{Y}\mathit{i}\mathit{e}\mathit{l}\mathit{d} \mathit{s}\mathit{t}\mathit{r}\mathit{e}\mathit{s}\mathit{s}}{\mathit{M}\mathit{a}\mathit{x}\mathit{i}\mathit{m}\mathit{u}\mathit{m} \mathit{s}\mathit{t}\mathit{r}\mathit{e}\mathit{s}\mathit{s}}\right]$
3	1.88
6	1.61
9	1.08

, decreases with the number of turns. For a small number of turns of the helically-coiled tube, the space where the structure can be deformed is wide in a limited area within the fixed parts of the tube (inlet and outlet), so the predicted stress is relatively small.

Since the safety factor exceeds 1 for all numbers of turns, no structural integrity issues will occur. However, for $N_c=9$, the safety factor for thermal stress is very close to 1, with a relatively small margin. Considering the heat transfer efficiency in Section 3.2 and this result on structural integrity, the helically-coiled tube with six turns is effective from the viewpoints of both heat transfer and structural integrity.

Figure 17 shows the thermal deformation due to the temperature difference in helically-coiled tubes. In this figure, the original configuration is shown in grayscale and the amount of deformation is enhanced by 20 times. The maximum deformation is about 1 mm in all helically-coiled tubes. This deformation could not cause the unwanted problems, such as physical contact with the wall surface of the heat exchanger resulting from the expansion of helically-coiled tube due to thermal stress.

Figure 18 shows the thermal deformation of the outer casing of the heat exchanger due to temperature differences. The body part of the outer casing forms a straight line parallel to the nozzle but the end part is designed to be curved parallel to the axial direction from the nozzle so that additional thrust can be obtained during flight, as shown in Figure 2. This bent part generally has a higher thermal stress value than the other parts. According to the present analysis, thermal deformation of up to 3.8 mm will occur. If this deformation value is considered as acceptable in the design of the outer casing of the heat exchanger, then the thrust through the GG gas discharged after heat transfer can be efficiently used by designing a bent shape of the outlet straight. In addition, since GG gas has a smaller change in pressure drop and smaller temperature difference in the overall flow shape due to the relatively larger area and higher mass flow rate than helium, there is no significant relationship between the geometric shape of the helically-coiled tube and the deformation of the outer casing of the heat exchanger.

3.3. Effect of Mass Flow Rate ($\dot{m}$)

The heat exchange performance of the studied heat exchanger can be greatly affected by not only the shape of the tube but also the flow rate. Therefore, additional numerical analyses were performed to analyze the effect of the helium flow rate on heat transfer characteristics. Five cases were set, from 2 g/s to 6 g/s with steps of 1 g/s, to analyze the heat transfer trend before and after the design flow rate; the boundary conditions were the same as those in the baseline case except the flow rate. As seen in Figure 19 and Figure 20, the amount of heat received from the GG gas increases overall with the flow rate of helium. In the helium region, as the flow rate increases, the temperature difference decreases from 670 K to 654 K and the pressure drop increases from 0.34 MPa to 2.73 MPa. A similar result is observed in the GG gas region but it does not have a significant variation because the outer fluid region has a larger area than the heat exchanger. As shown in Figure 20b, the friction factor did not show significant variation with the flow rate of helium. Compared with the results in Figure 14b, it can be thought that the friction factor is dominantly dependent on the physical configuration in the present study such as the number of turns in the coiled tube rather than the flow rate. On the contrary, the variation in the averaged heat flux is much dependent on the flow rate and physical configuration. These characteristics should be scrutinized to provide some guidelines for the design of heat exchangers with the coiled configurations. Further consideration on the heat transfer characteristics will be addressed in Section 3.4.

Figure 21 shows that the thermal stress of the helically-coiled tube increases with the flow rate. As shown in Figure 21 and Figure 22, the heat flux increases with the flow rate, resulting in an increase in the temperature difference between the outer and inner walls of the heat exchanger. Therefore, an increase in the temperature difference leads to higher thermal stress which exceeds the yield stress of the material at flow rates of 4 g/s or more. These results shown in

Table 4. Safety factors for thermal stress at various $\dot{m}$.

$\dot{\mathit{m}}$ [g/s]	$\mathbf{Safety} \mathbf{Factor} \left[\frac{\mathit{Y}\mathit{i}\mathit{e}\mathit{l}\mathit{d} \mathit{s}\mathit{t}\mathit{r}\mathit{e}\mathit{s}\mathit{s}}{\mathit{M}\mathit{a}\mathit{x}\mathit{i}\mathit{m}\mathit{u}\mathit{m} \mathit{s}\mathit{t}\mathit{r}\mathit{e}\mathit{s}\mathit{s}}\right]$
2	1.09
3	1.08
4	0.88
5	0.83
6	0.79

indicate that the safety factor is less than 1 and should be carefully considered from the viewpoint of structural design.

3.4. Analysis of Heat Transfer Characteristics

Since helium flows rapidly inside the heat exchanger, the convective heat transfer between the two fluids occupies a large proportion. The parameters of heat transfer should be investigated according to factors such as geometric configurations and flow conditions. Dimensionless analysis was performed on the studied heat exchanger, similarly to a previous study [27,30], to understand these heat transfer characteristics. Their change according to flow rate variation was analyzed as the relationship between the Dean number (De), which represents fluid behavior in coiled tubes, and the Nusselt number (Nu), which represents the heat transfer characteristics. De and Nu are defined as

$$\mathrm{D}\mathrm{e}=\mathrm{R}\mathrm{e}\sqrt{\raisebox{1ex}{$d$}\!\left/ \!\raisebox{-1ex}{$D$}\right.}, Nu=\frac{hd}{k}$$

(1)

where d is the inner diameter of the coiled tube, D is the coil diameter, Re is the Reynolds number, h is the heat transfer coefficient, and k is the thermal conductivity of helium, respectively.

Since the local helium temperature in the tube varies considerably, the local value of any part cannot represent the overall heat transfer characteristics. Therefore, the area from the beginning to the end of the tube was divided into equally spaced sections to calculate the local value of De and Nu for each section. Then, we analyzed these characteristics using the mean of these local values. The criteria for dividing the sections were set to 1/2 pitch (19 sections) and 1/4 pitch (37 sections). These two methods do not significantly differ, as shown in Figure 23, so a section was selected at every 1/2 pitch of the coiled tube in all cases.

The correlation between the mean De and Nu according to the number of turns and helium flow rate is shown in Figure 24. The resident time decreases with the number of turns, resulting in a lower average temperature of helium. Since the viscosity of helium decreases with temperature, De tends to increase as the number of turns decreases, even at the same flow rate. A similar trend is observed when the flow rate of helium is doubled; this trend may be related to the $N_c$ of the helically-coiled tube.

As the flow rate increases, De increases gradually. Nu tends to be proportional to the increase in De because the amount of heat transfer by convection increases with the flow rate. A trend line is drawn from this correlation and the equation of this line is fitted as

$$Nu=0.211\times {De}^{0.8} (2\le \dot{m}\le 6 \left[\raisebox{1ex}{$\mathrm{g}$}\!\left/ \!\raisebox{-1ex}{$\mathrm{s}$}\right.\right])$$

(2)

Consequently, the heat transfer characteristics can be related to the flow in the coiled tube by an exponent of 0.8. This tendency shows the feasible behavior of the heat exchanger with a coiled tube and can be used as one of the thermal correlations to design the similar heat exchanger configuration in open-cycle liquid rocket engines.

4. Conclusions

In this study, a heat exchanger in an open-cycle liquid rocket engine was analyzed through CHT analysis using open-source codes. The heat exchanger was divided into four domains to predict its heat transfer characteristics and the thermal deformation and stress caused by temperature changes. The following conclusions were obtained:

(1)

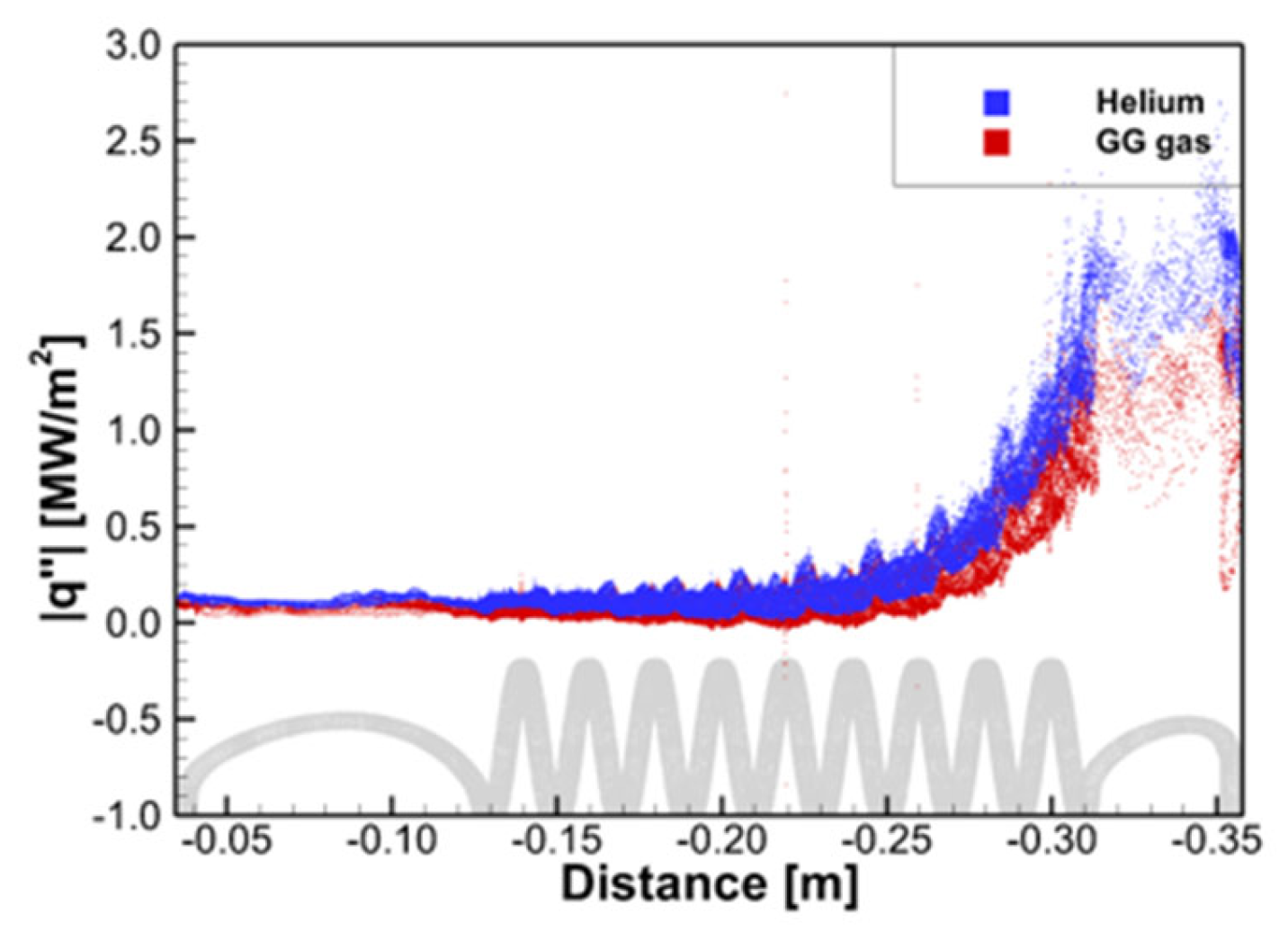
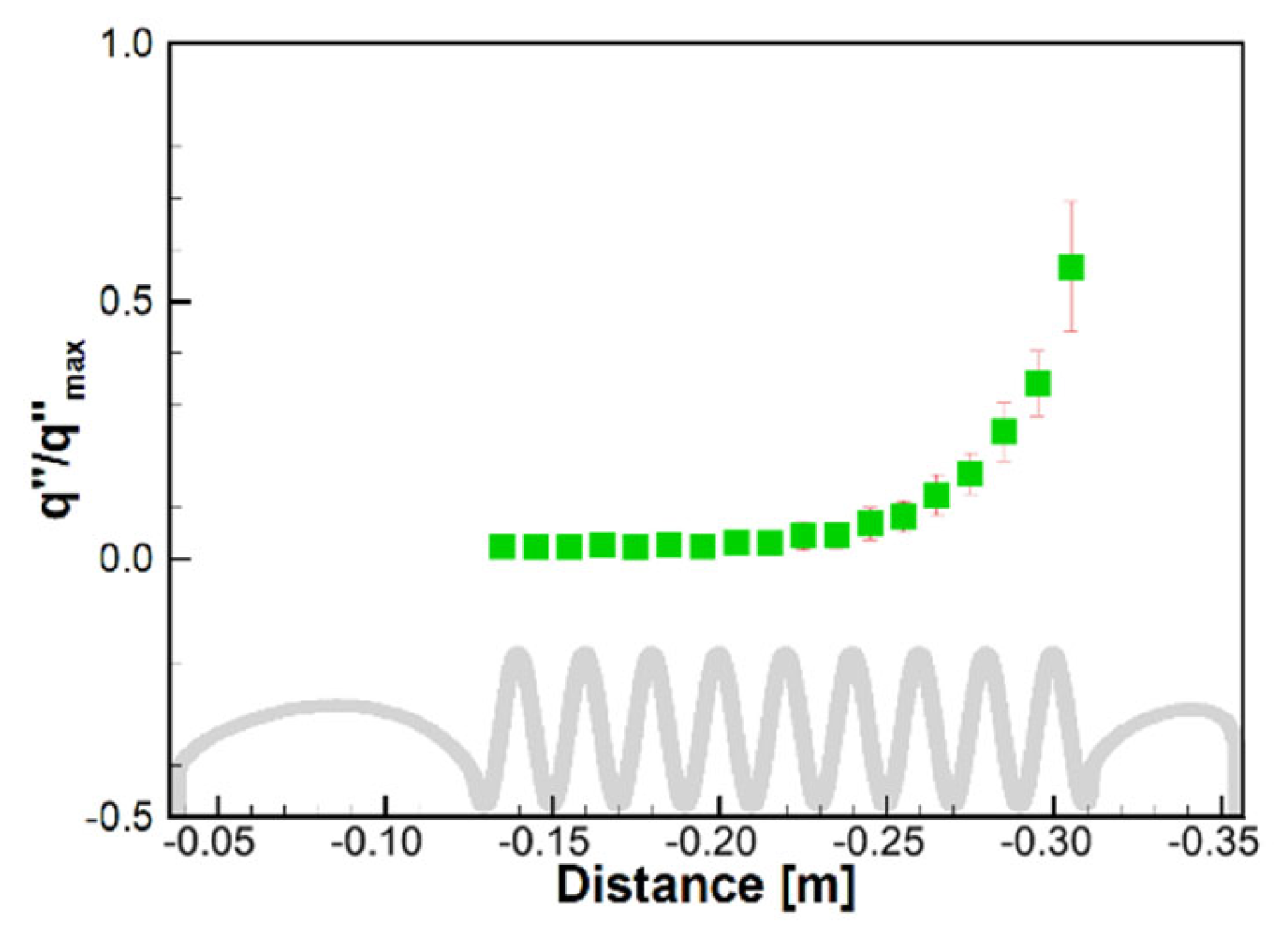
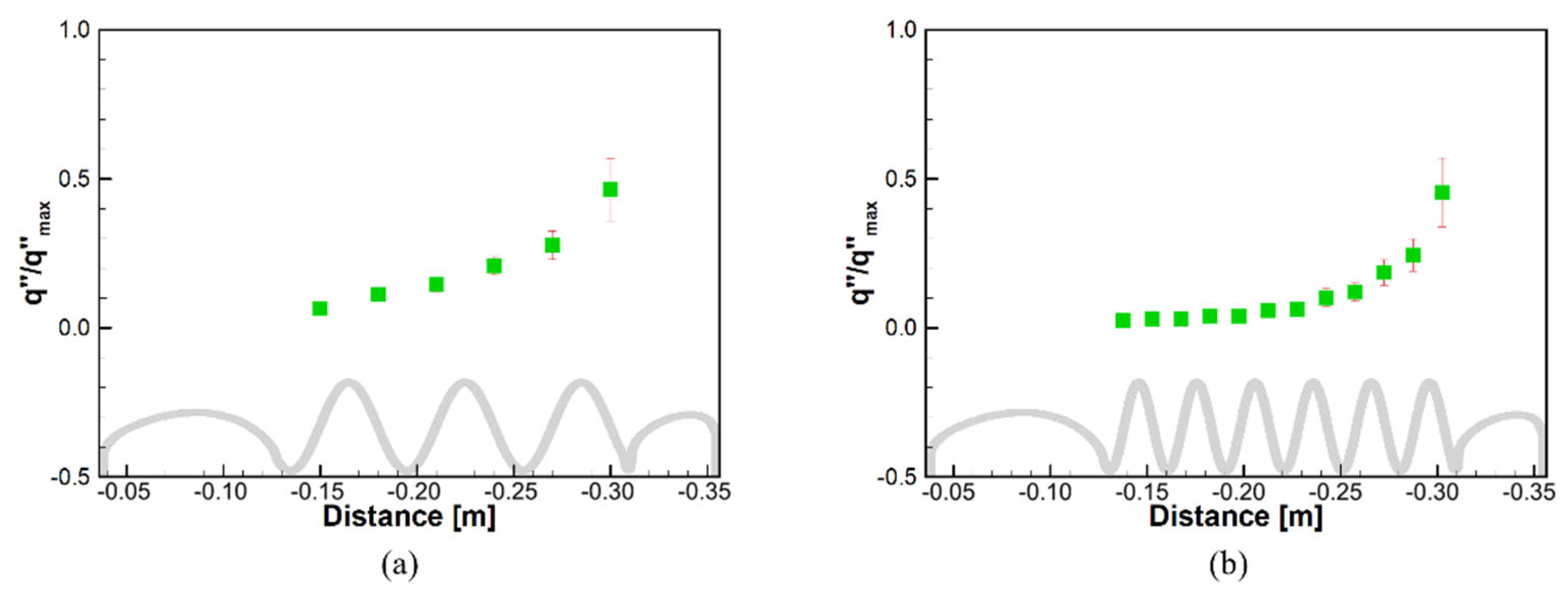
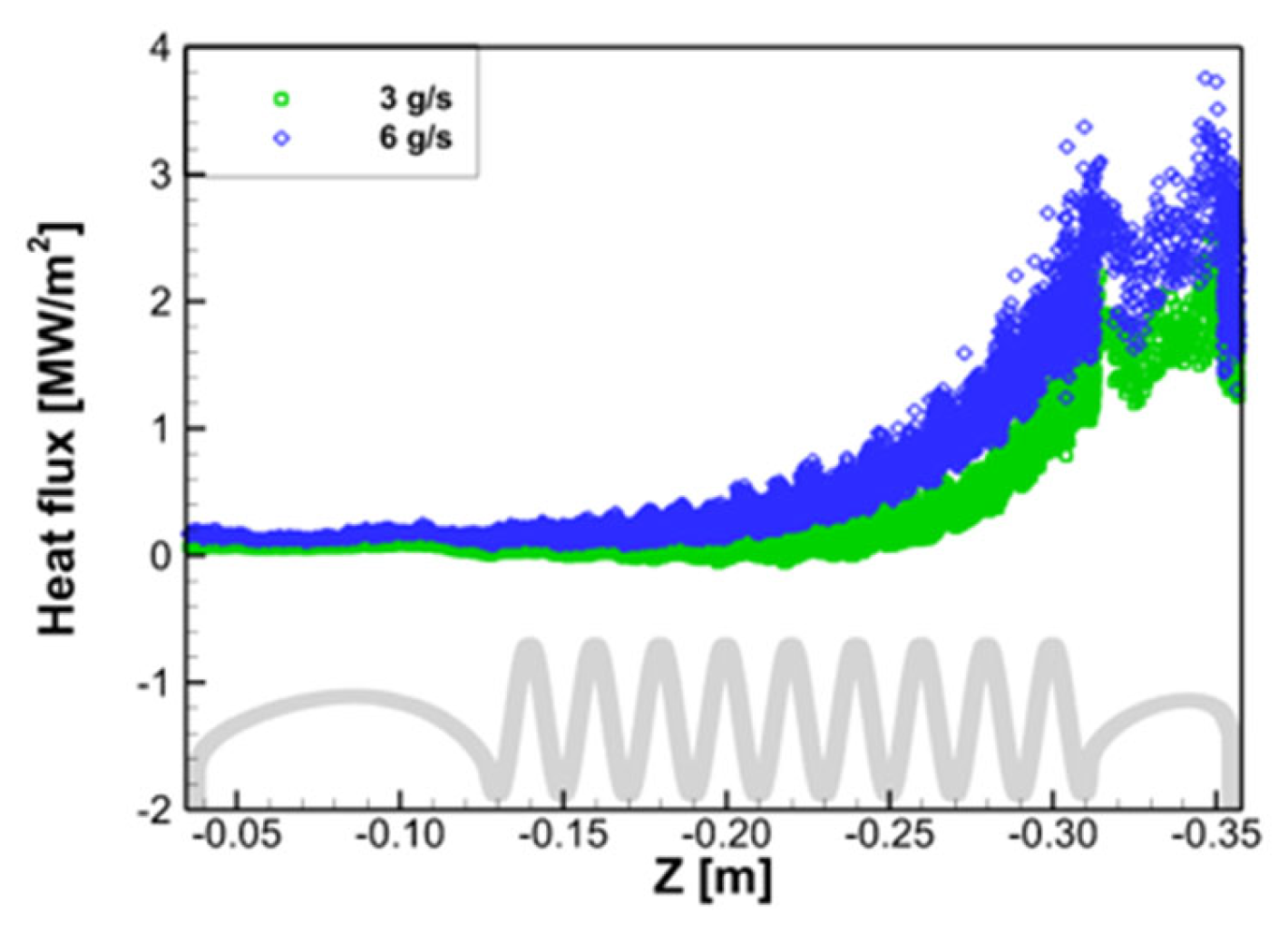
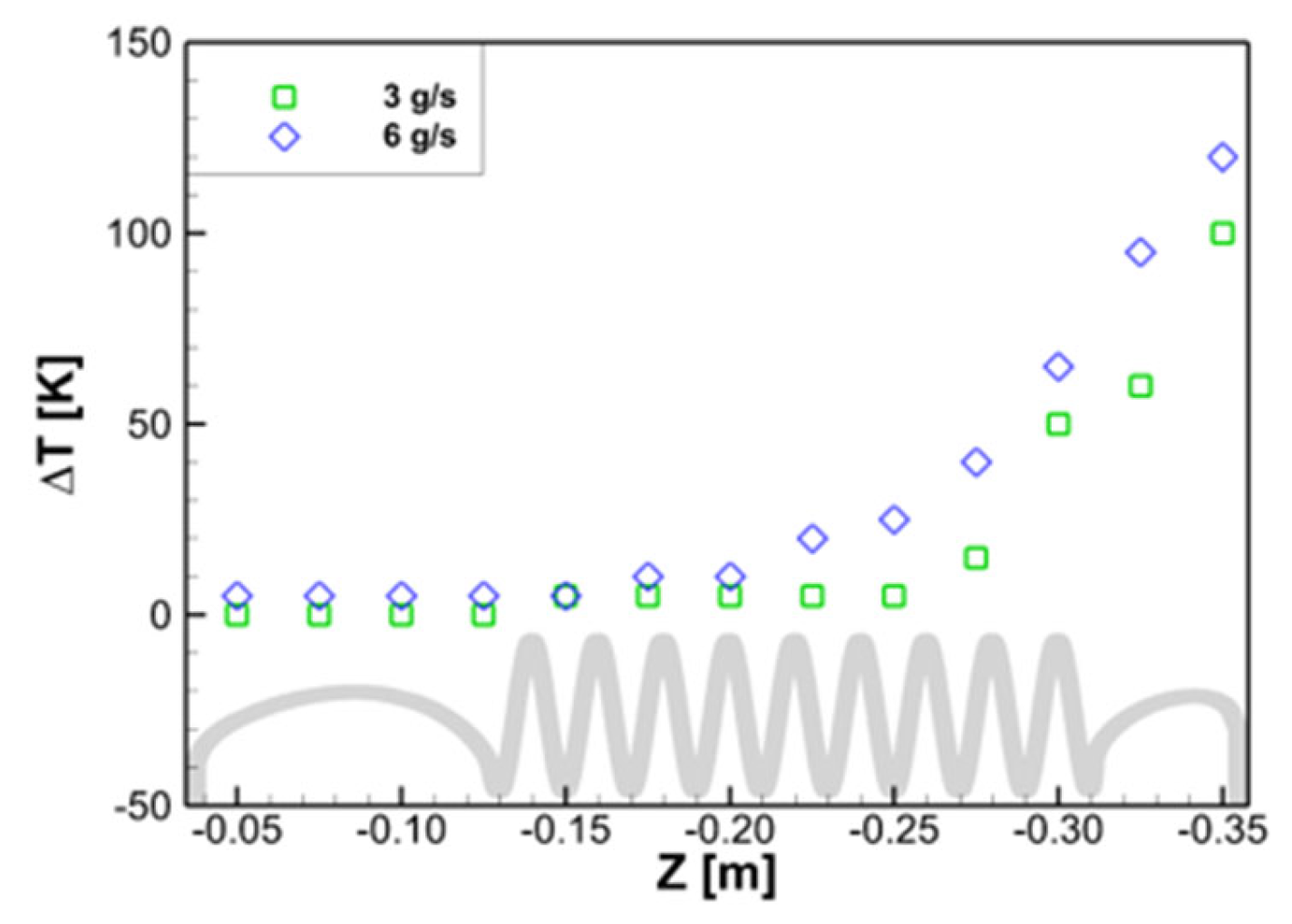
For the baseline heat exchanger ($N_c$ = 9), the characteristics of heat transfer were investigated. As the temperature difference between GG gas and helium decreased along the coiled tube, the heat flux decreased dramatically, resulting in a ${q^{”}}_{max}$ value of about 5% after the sixth turn of the coiled tube. Therefore, the baseline heat exchanger is overdesigned from the viewpoint of heat transfer optimality. Therefore, further numerical analysis was performed to predict the effect of $N_c$ on the performance of the heat exchanger;

(2)

In the case of $N_c$ = 3, the outlet temperature was 20 K lower than that in the case of $N_c$ = 9 due to the excessively-reduced residence time and heat transfer area. Therefore, the heat exchanger with $N_c$ = 3 had a small number of pitches and thus poor heat transfer performance. For $N_c$ = 6, the outlet temperature was 5 K lower than that for $N_c$ = 9, the pressure drop of helium was 0.3 MPa, and the weight of the helically-coiled tube structure was reduced by 72% compared with that for $N_c$ = 9. Consequently, the optimal number of turns of the coiled tube was six or more;

(3)

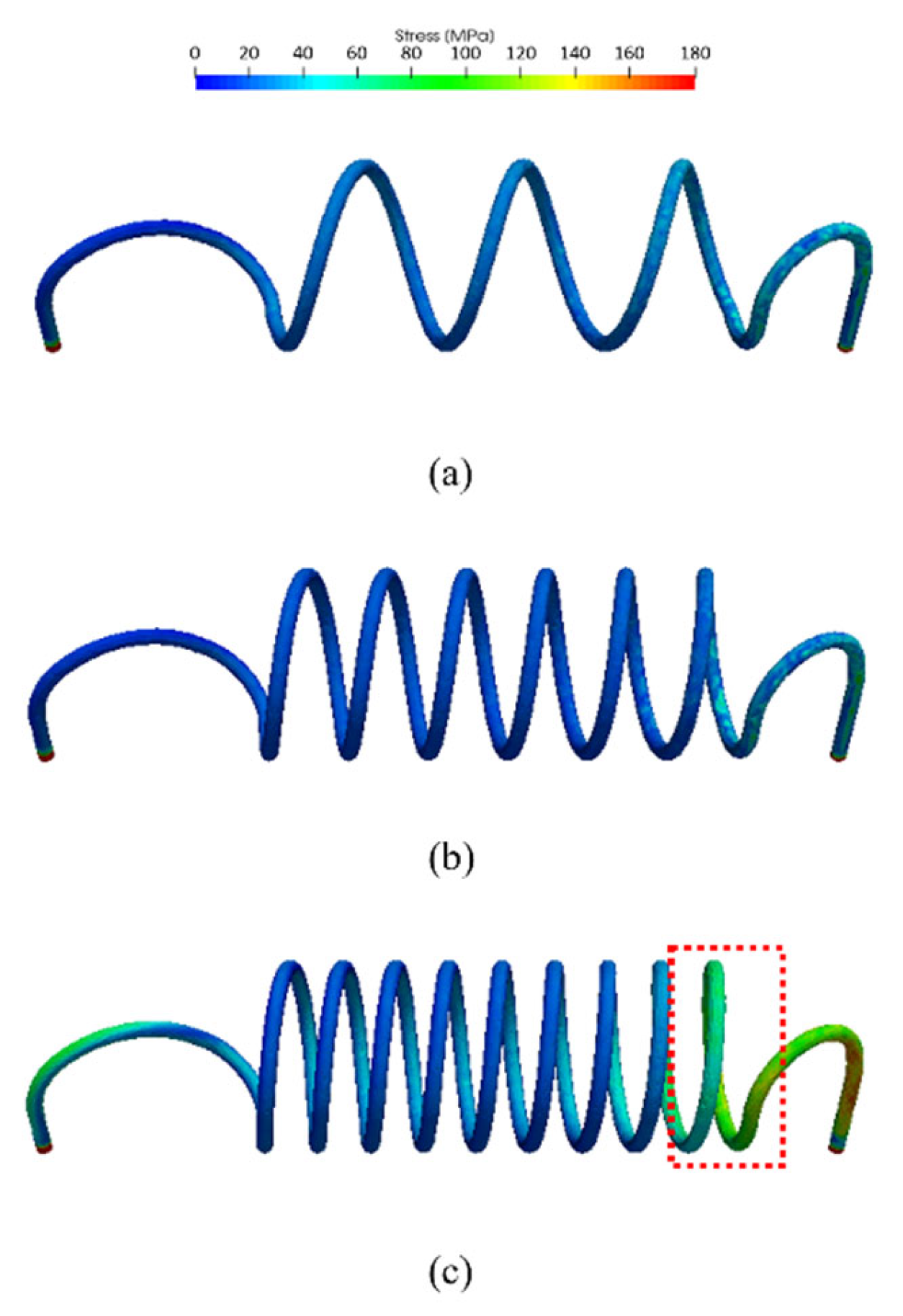
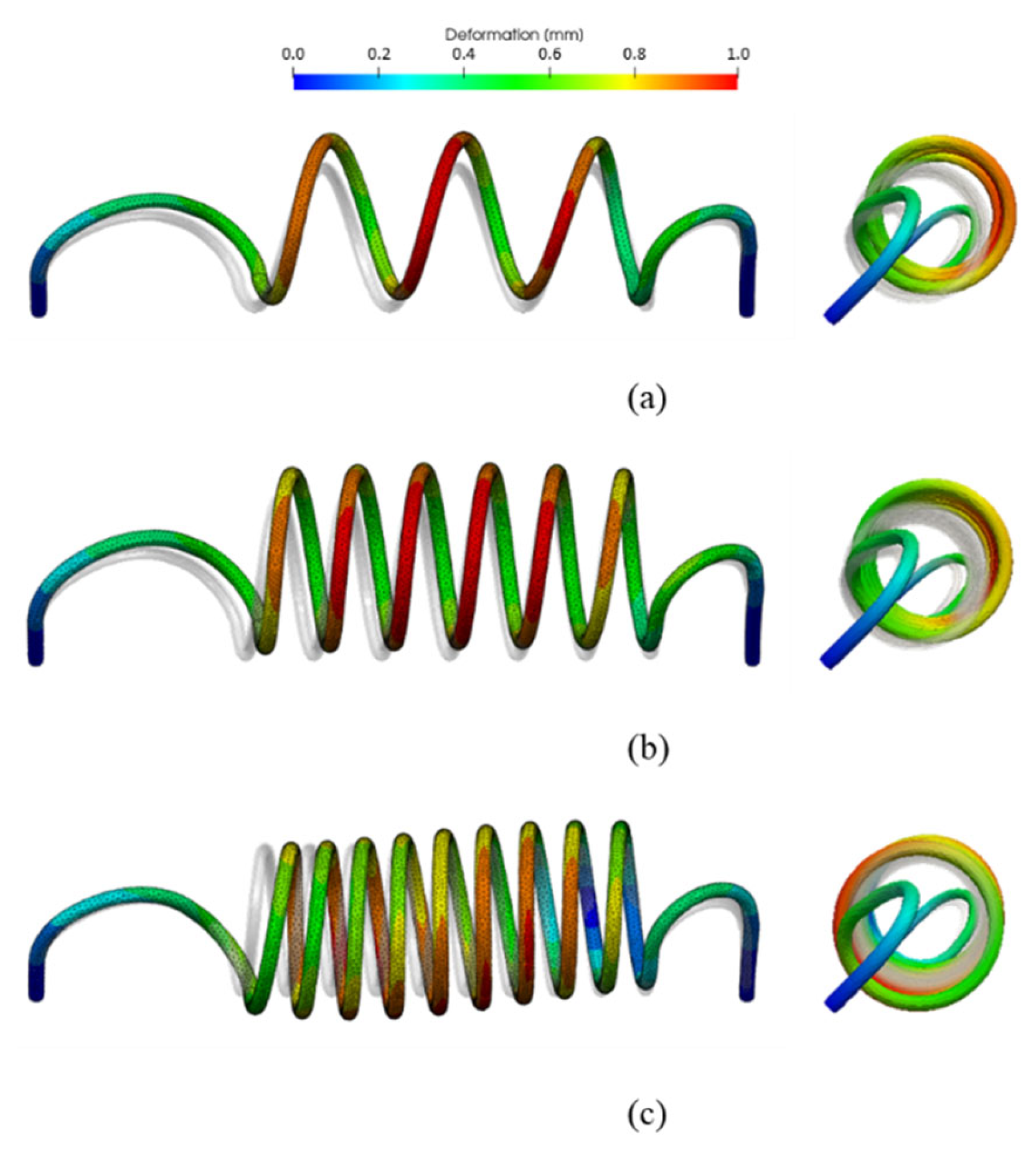
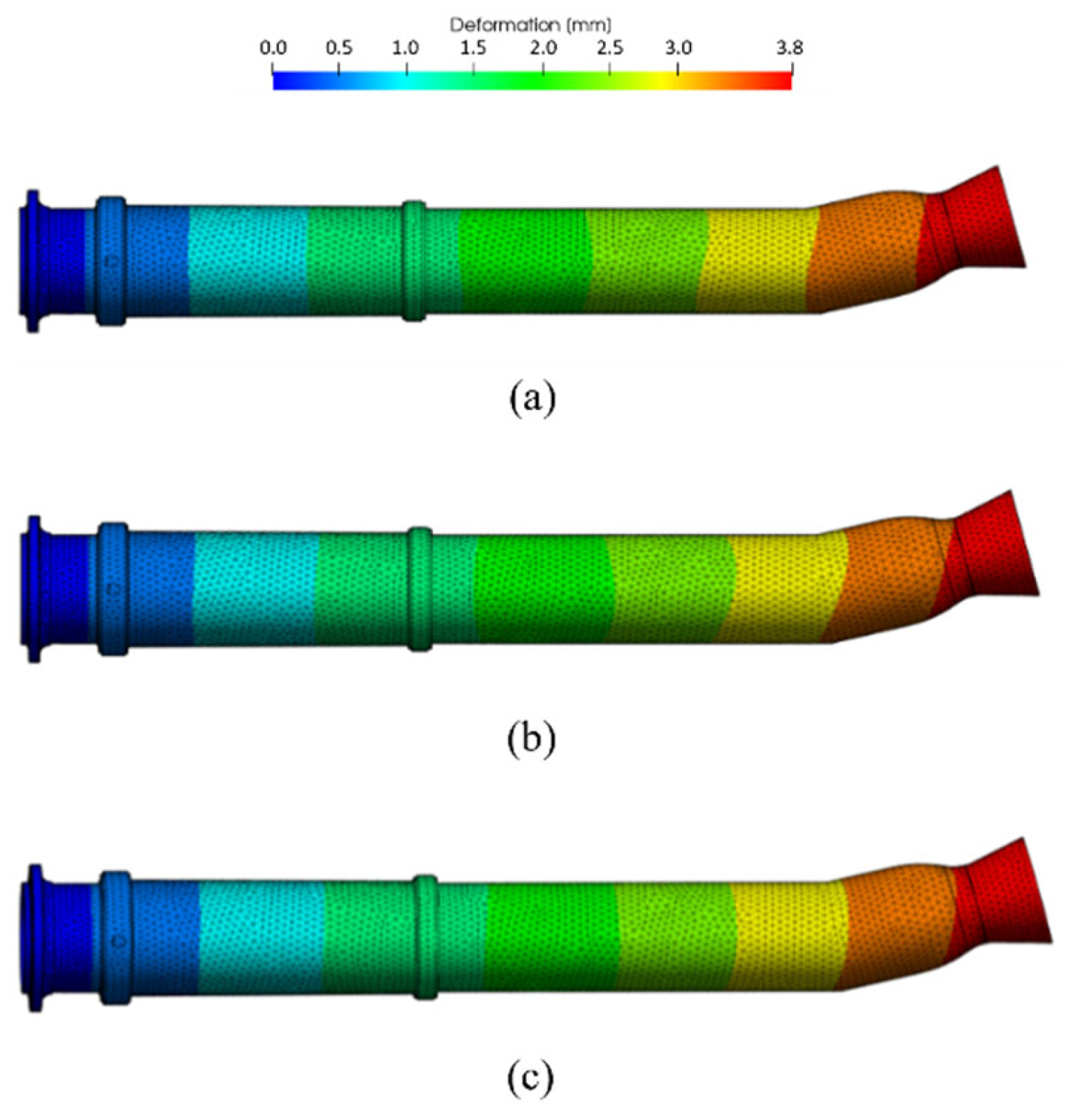
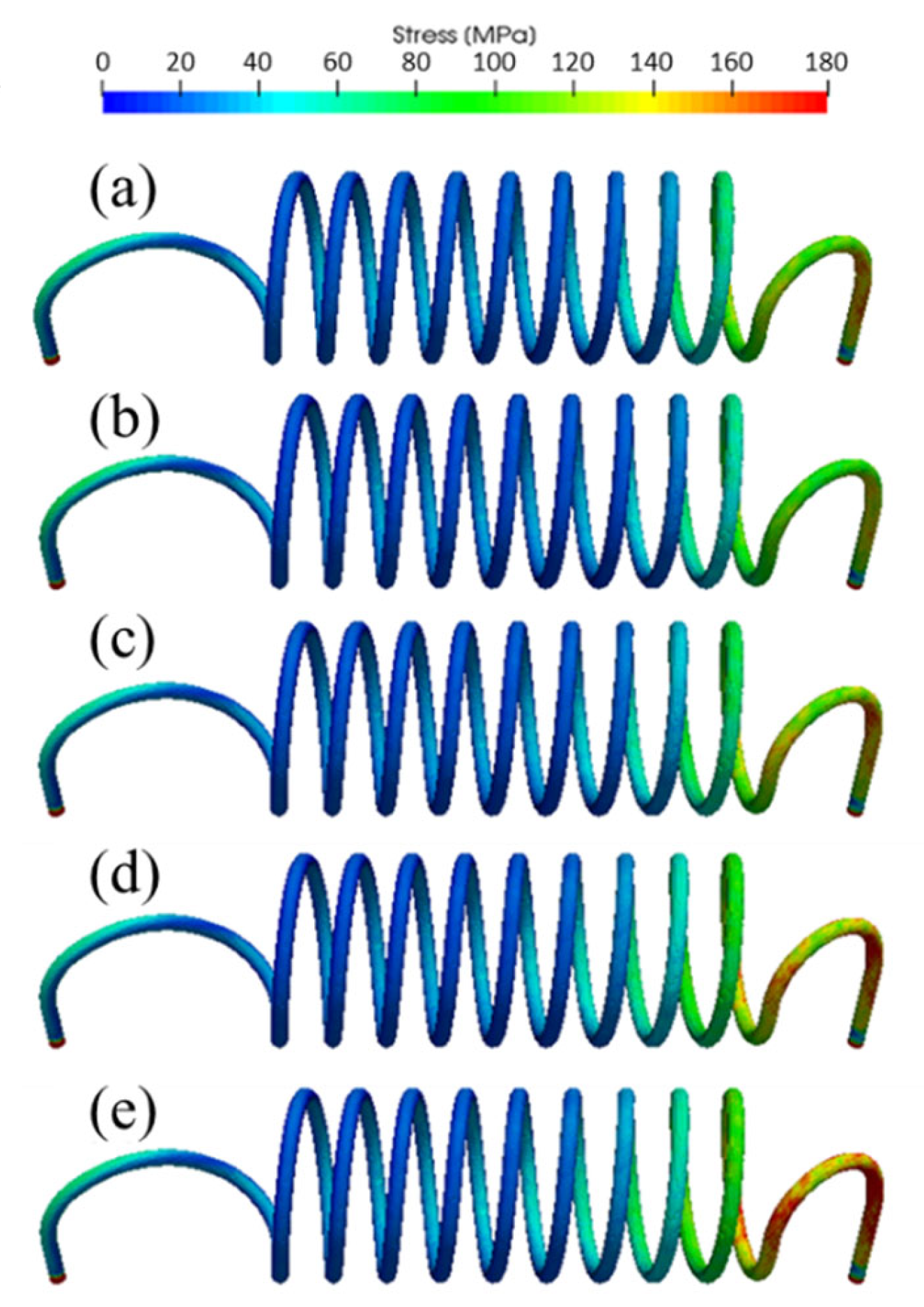
According to the structural analysis of the helically-coiled tube, a maximum deformation of about 1 mm was predicted. This deformation could not make any structural problems, such as physical contact with the inner wall of the heat exchanger. In addition, the safety factors for thermal stress for $N_c$ = 3, 6, and 9 exceed 1, so the structural integrity was acceptable. At the outer casing of the heat exchanger, a maximum deformation of 3.8 mm was predicted near the outlet due to heat transfer from GG gas. However, such deformation would not cause structural problems, such as physical contact with the main nozzle of the liquid rocket engine. Considering this deformation of the outer casing of the heat exchanger, a heat exchanger with a straight body can be designed to generate higher thrust;

(4)

The amount of heat received from the hot GG gas increased with the mass flow rate. However, the temperature difference between the inlet and outlet of helium decreased from 670 K to 654 K. In the case of the highest flow rate (6 g/s), the outlet temperature was 8 K lower than that in the case of the design flow rate and was within the usable range. As the flow rate increased, the pressure drop of helium increased from 0.34 MPa to 2.73 MPa;

(5)

As the mass flow rate increased, the amount of heat received from the high-temperature GG gas tended to increase which caused an increase in the temperature difference on the right side of the helically-coiled tube. Consequently, the thermal stress was higher than that at the design flow rate and the safety factor was less than 1 at a flow rate of 4 g/s or more. Thus, a kind of design limit in safety factor was identified from the design point of view;

(6)

The heat transfer characteristics according to the shape and flow rate changes of the heat exchanger were analyzed using dimensionless numbers. De and Nu increased as the $N_c$ of the coiled tube decreased. Moreover, De and Nu increased with the mass flow rate. The correlation of Nu and De was in the form of an exponential function and a trend was derived from these results. Nu and De showed similar trends at flow rates other than the design flow rate; therefore, their trend was related to $N_c$. This exponential correlation can be used with respect to one of the thermal correlations for the design of the heat exchanger with coiled tubes in open-cycle liquid rocket engines. In the future, the effect of fluid properties, such as viscosity, will be analyzed in terms of the Prandtl number.