Finite Element Method-Based Optimisation of Magnetic Coupler Design for Safe Operation of Hybrid UAVs
Abstract
:1. Introduction
- Performing transient analysis on the dynamically modelled state of the MC;
- Dynamic investigation of the effect of misalignments on the transmitted torque;
- Examination of the MC efficiency depending on the operating speed at a critical angle;
- Exploring the negative torque between the rotors in case of a loss of synchronisation;
- Proposing the correction coefficients to identify the error margin of simulations.
2. Design Considerations
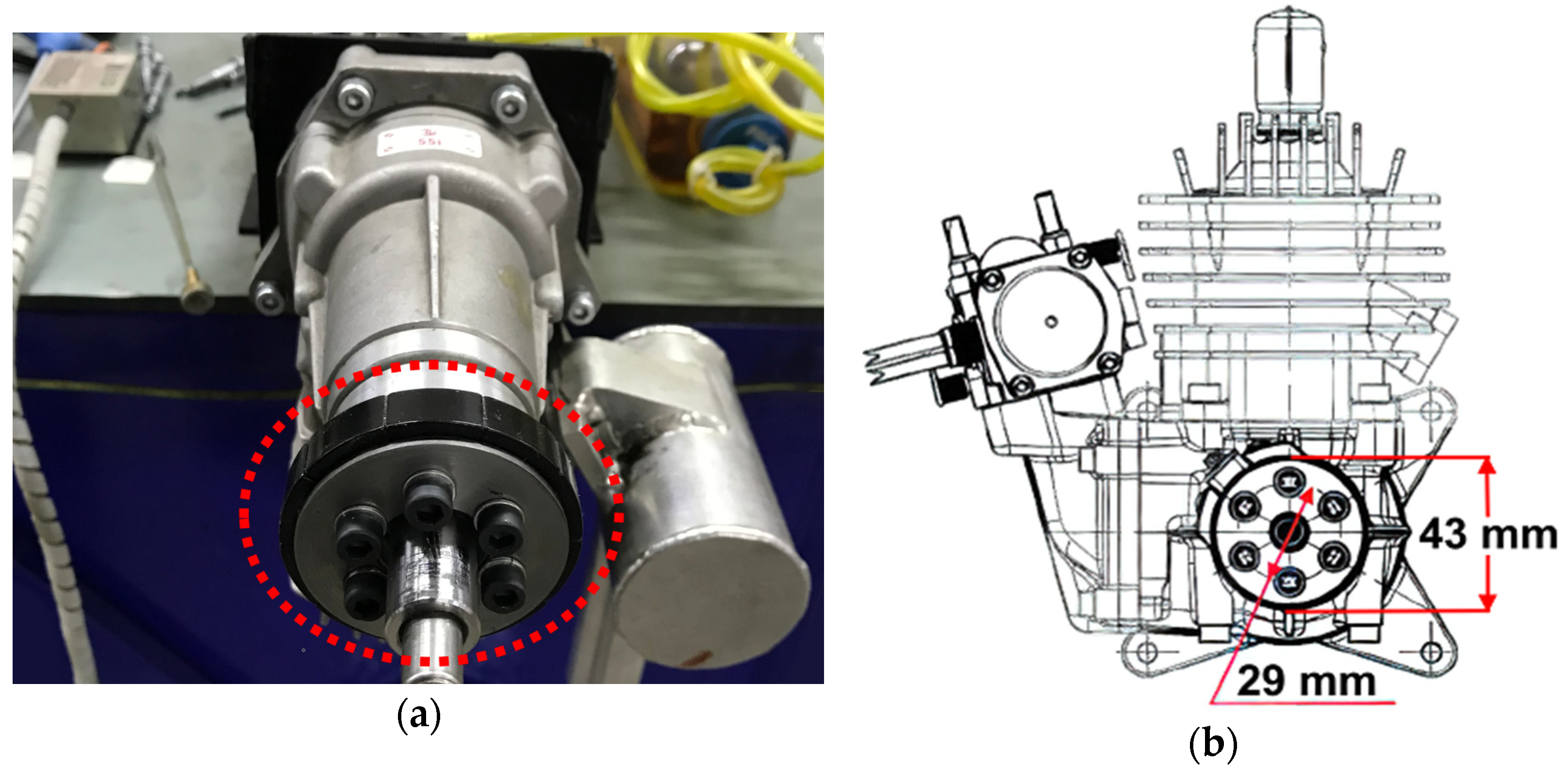
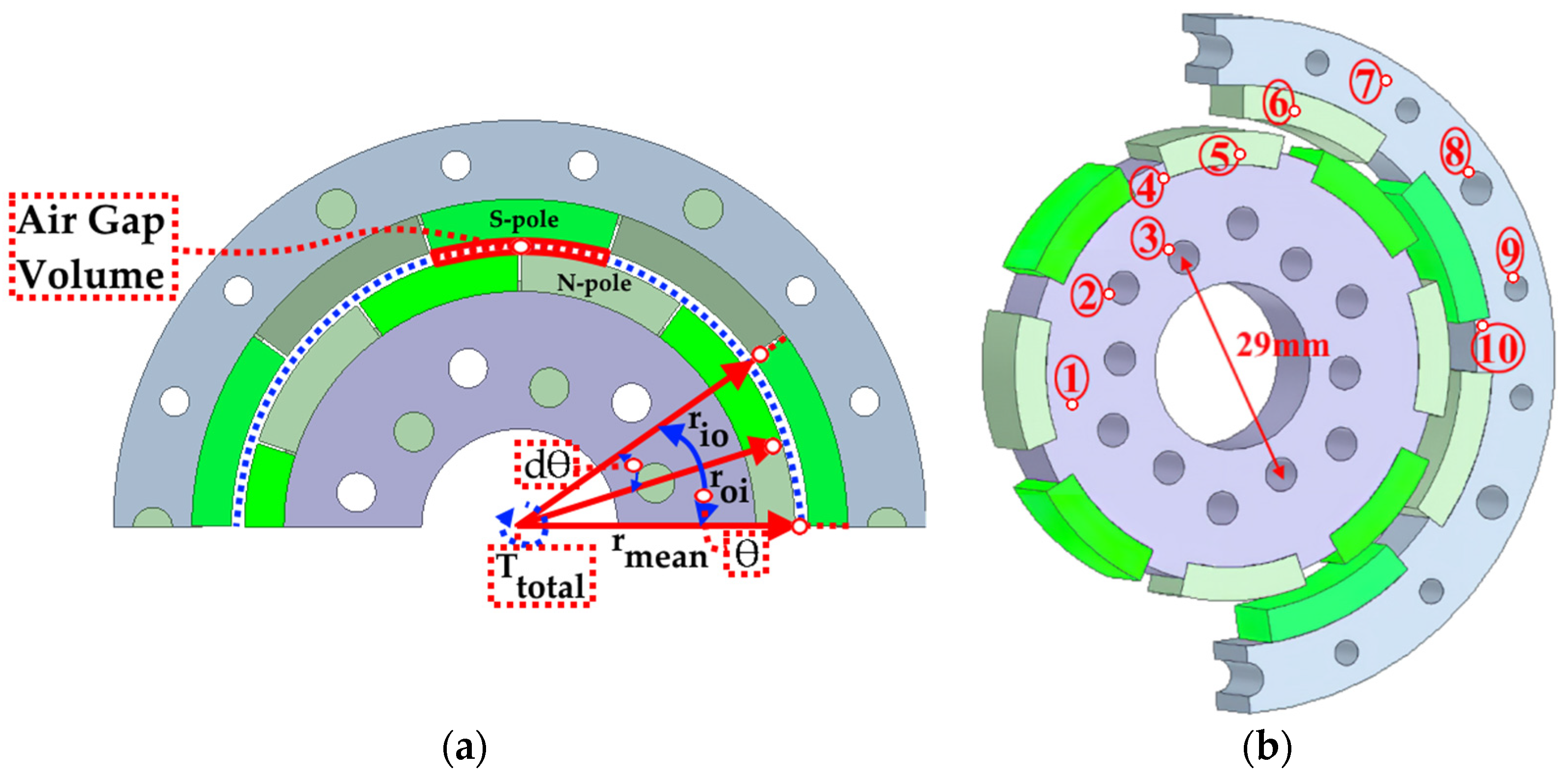
2.1. Determination of the Minimum Outer Diameter of the Inner Rotor
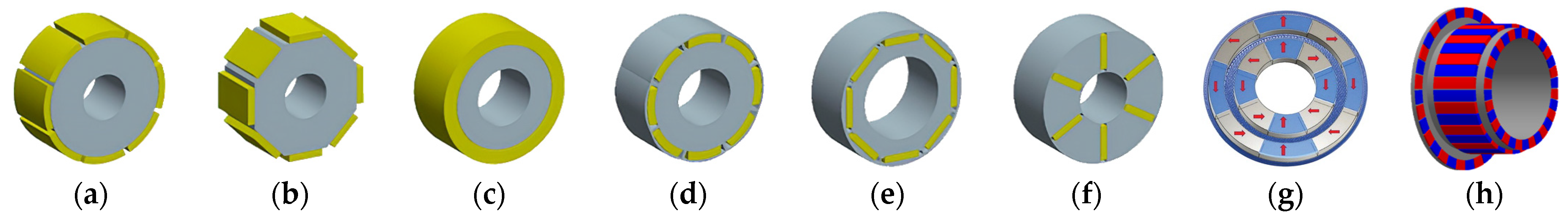
2.2. Selection of Rotor Topology
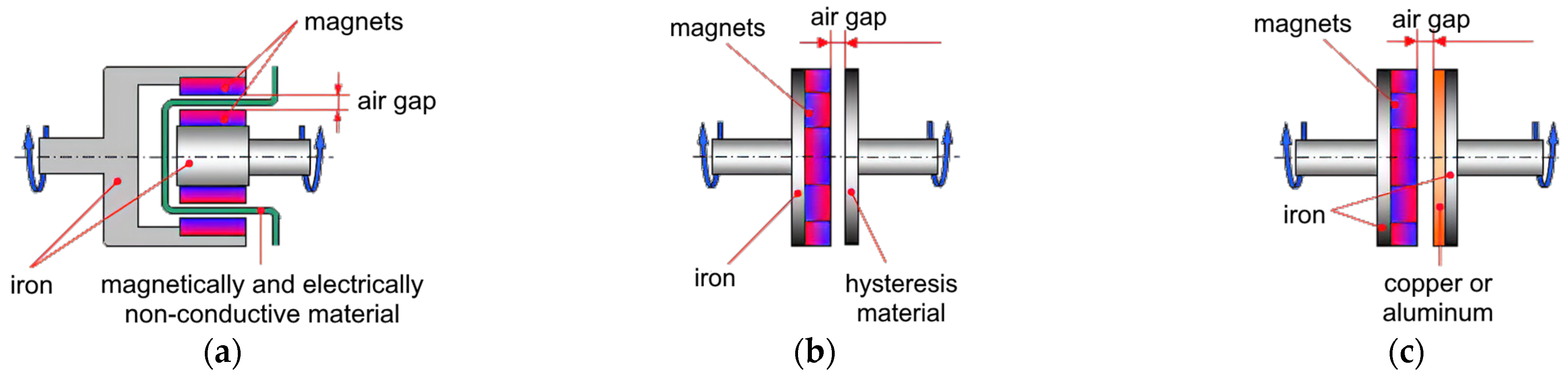
2.3. Materials Overview
3. Design Studies
3.1. Analytical Preliminary Sizing
3.2. Maxwell 2D Static Analyses
3.2.1. Correlation of Effective Air Gap Diameter and Model Length
3.2.2. Investigation of Optimum Pole Number
3.2.3. Effect of Air Gap Clearance on Pullout Torque
3.2.4. Determination of PM Thickness
3.2.5. Determination of the Thickness of Rotor Yokes
3.2.6. Investigation of the PM Embrace and Offset Effect
3.3. Maxwell 2D Transient Analyses
3.3.1. Comparison of Pole Types
3.3.2. Effect of PM Type, Grade, and Temperature on Pullout Torque
3.3.3. Rotor Flux Density and Mesh Distribution
3.3.4. Investigation of Negative Torque at Loss of Synchronisation
3.4. Maxwell 3D Static and Transient Optimetric Analyses
3.4.1. Static Locked-Rotor Torque and Transient Torque Ripple Analyses
3.4.2. Investigation of Different Rotor Materials and Air Gap Flux Density
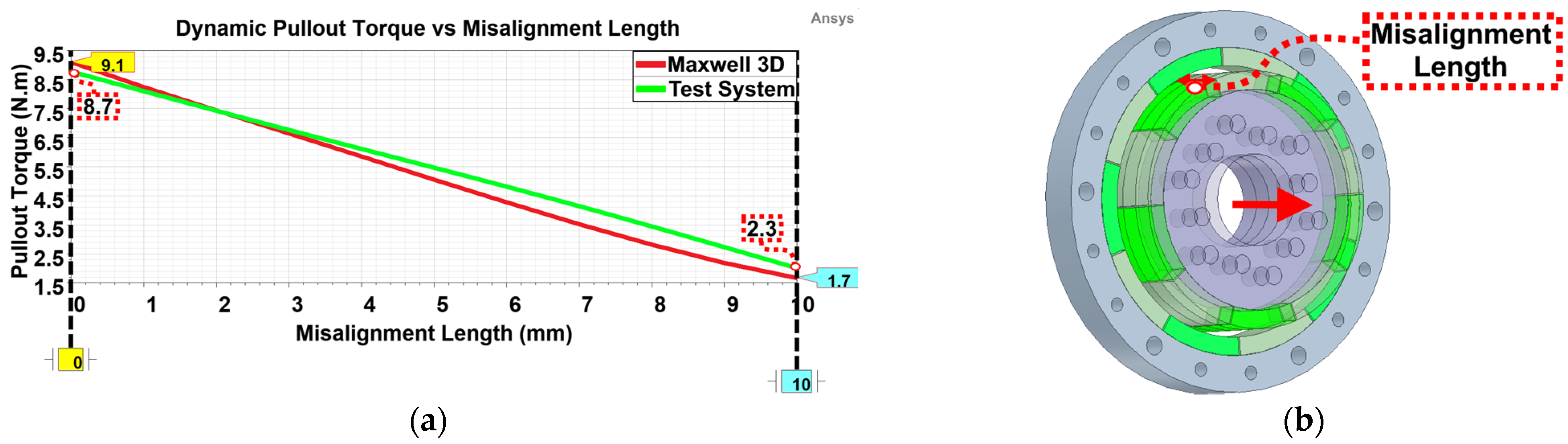
3.4.3. Study of Pullout Torque Depending on Misalignment Length
3.4.4. Magnetic Coupler Efficiency and Induced Eddy-Current Losses on PMs
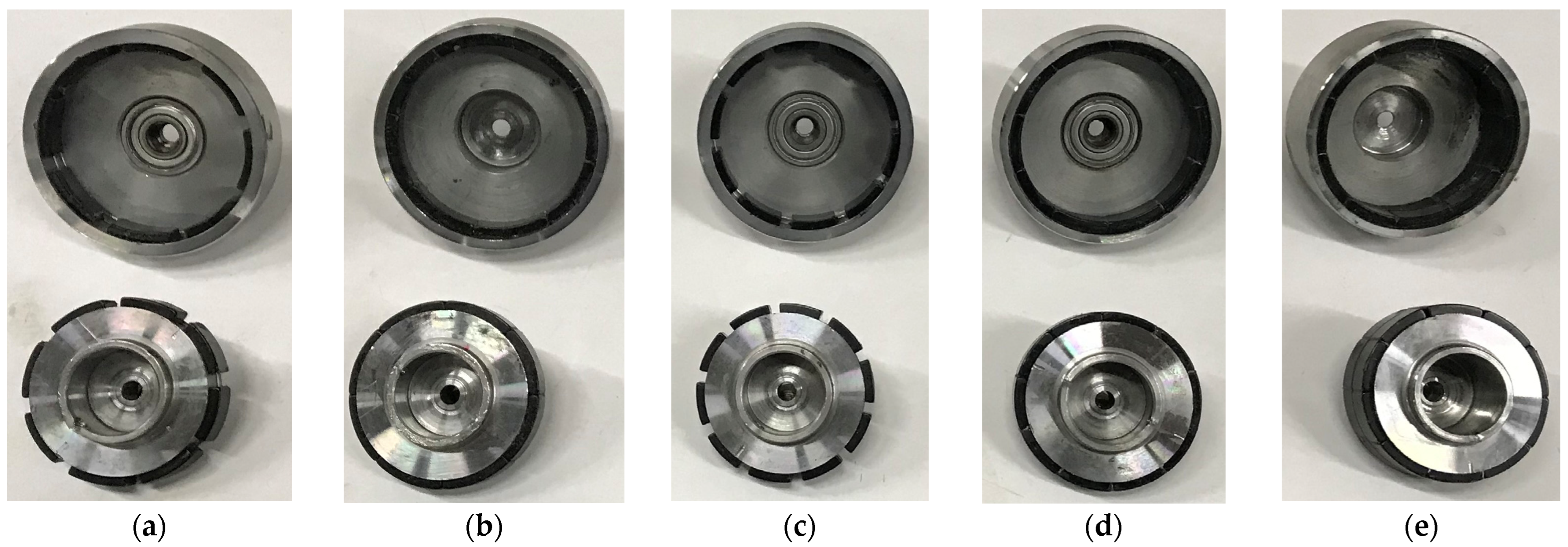
3.5. Summary List of Various MC Designs
4. Results and Discussion
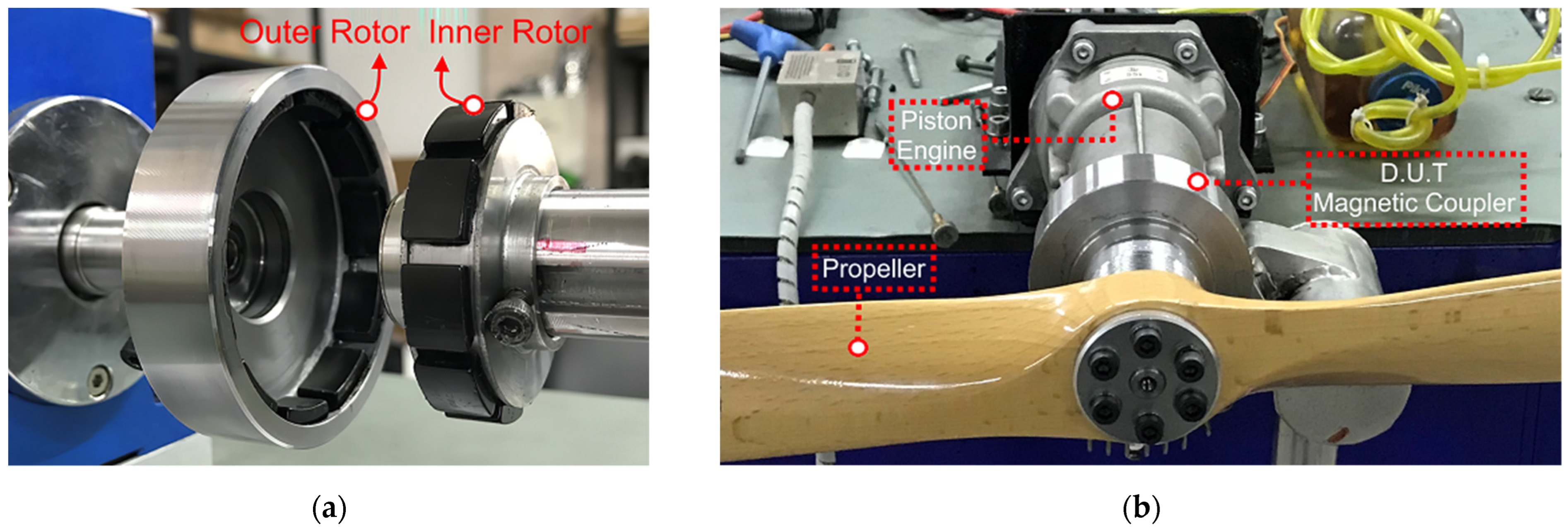
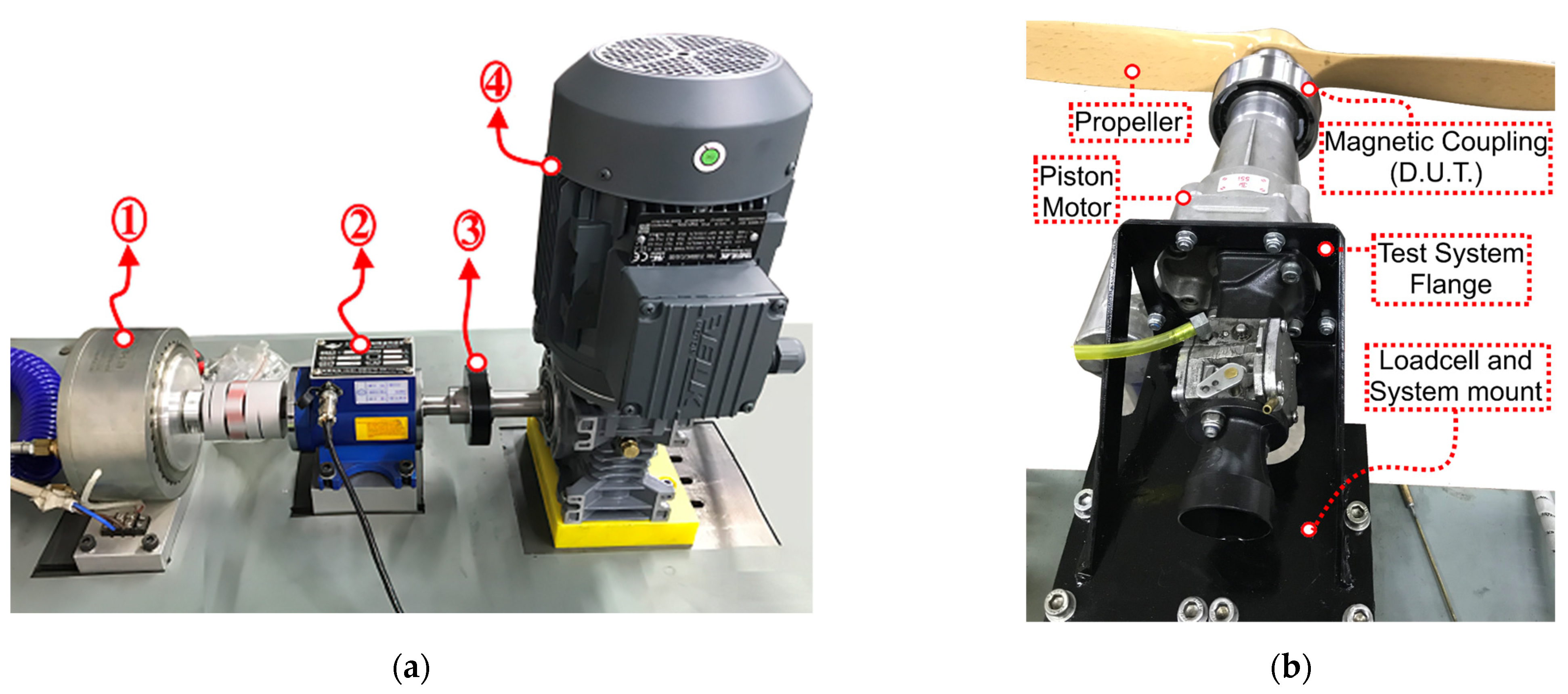
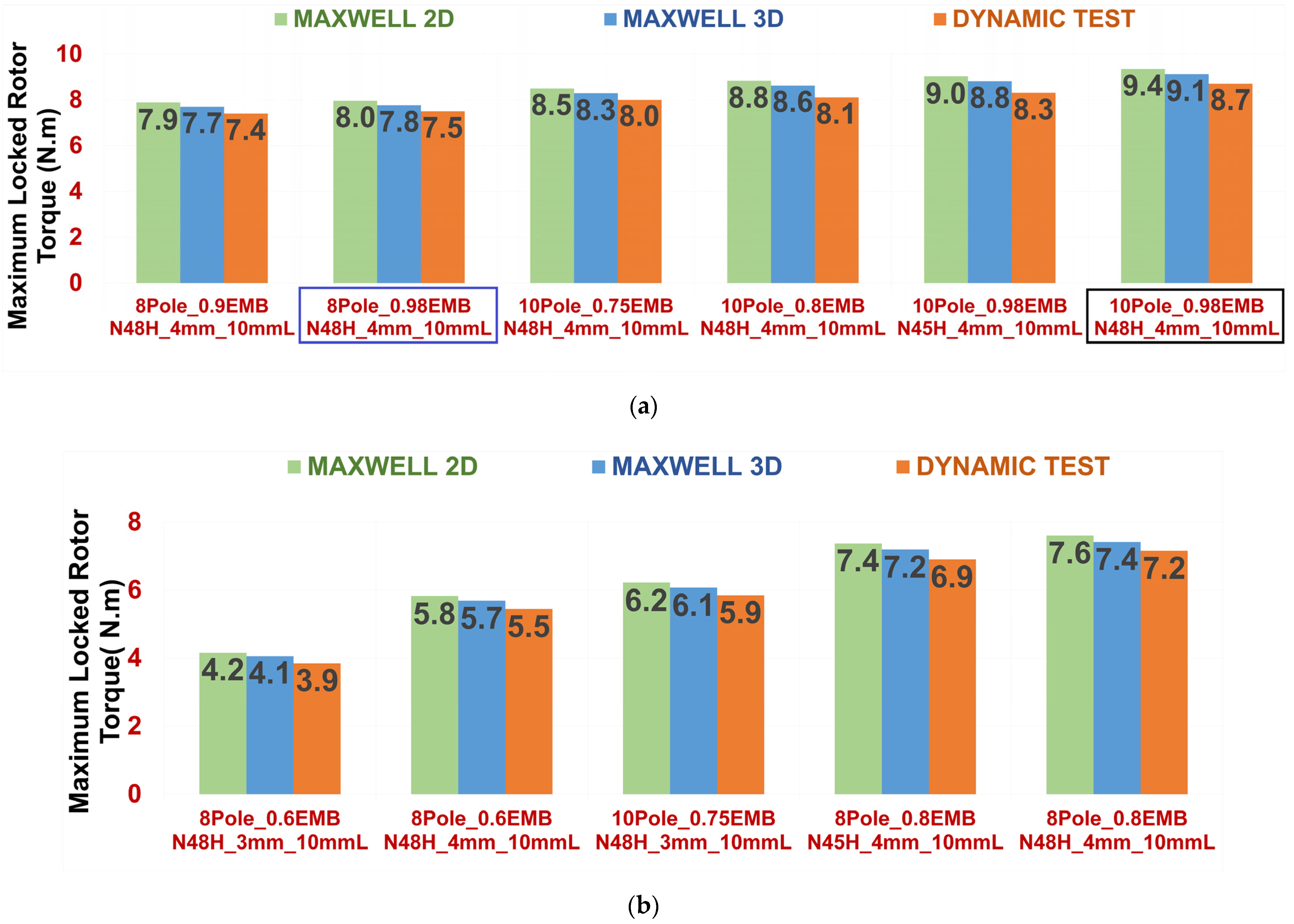
4.1. Locked-Rotor Test Results in Summary
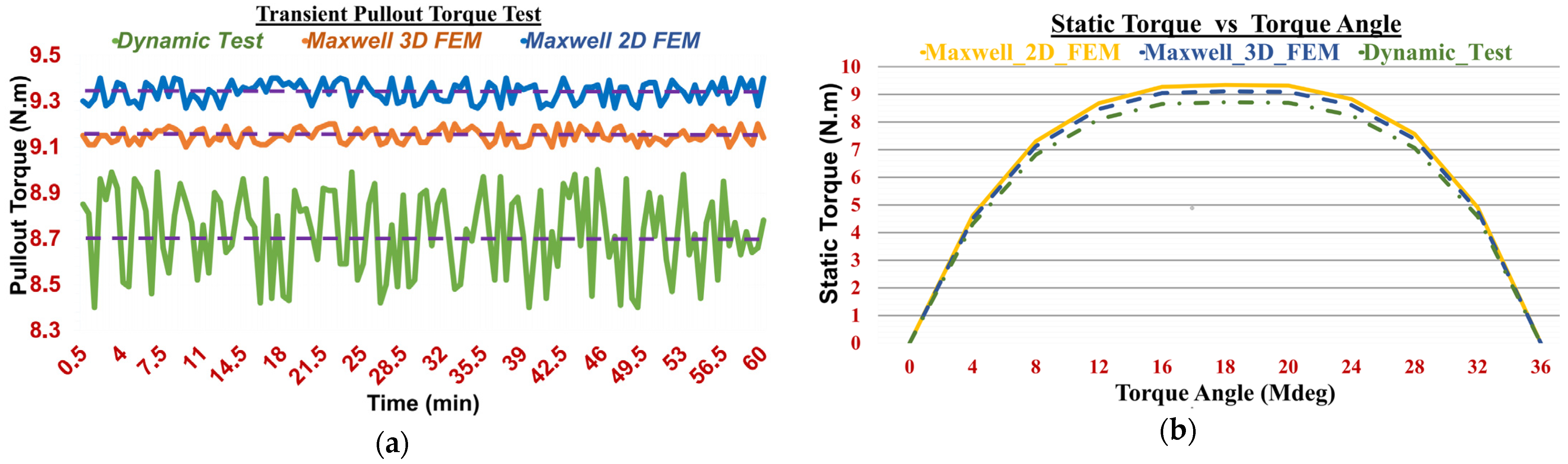
4.2. Investigation of Pullout Torque in Transient and Static Torque versus Torque Angle
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Arslan, S.; Iskender, I.; Navruz, T.S. Optimal Design of an In-Runner Direct-Drive Synchronous Permanent Magnet Starter/Generator for UAVs. In Proceedings of the 2022 International Symposium on Multidisciplinary Studies and Innovative Technologies (ISMSIT), Ankara, Turkey, 20–22 October 2022; pp. 543–548. [Google Scholar] [CrossRef]
- Griffo, A.; Wrobel, R.; Mellor, P.H.; Yon, J.M. Design and Characterization of a Three-Phase Brushless Exciter for Aircraft Starter/Generator. IEEE Trans. Ind. Appl. 2013, 49, 2106–2115. [Google Scholar] [CrossRef]
- Ose-Zala, B.; Pugachov, V. The comparison of active and reactive magnetic couplers. In Proceedings of the 2012 Electric Power Quality and Supply Reliability, Tartu, Estonia, 11–13 June 2012; pp. 1–4. [Google Scholar] [CrossRef]
- Carpentier, A.; Galopin, N.; Chadebec, O.; Meunier, G.; Guérin, C. Application of the virtual work principle to compute magnetic forces with a volume integral method. Int. J. Numer. Model. Electron. Netw. Devices Fields 2013, 27, 418–432. [Google Scholar] [CrossRef]
- Li, K.; Bird, J.Z.; Acharya, V.M. Ideal Radial Permanent Magnet Coupling Torque Density Analysis. IEEE Trans. Magn. 2017, 53, 1–4. [Google Scholar] [CrossRef]
- Ravaud, R.; Lemarquand, G.; Lemarquand, V.; Depollier, C. Torque in permanent magnet couplings: Comparison of uniform and radial magnetization. J. Appl. Phys. 2009, 105, 053904. [Google Scholar] [CrossRef]
- Akcay, Y.; Giangrande, P.; Tweedy, O.; Galea, M. Fast and Accurate 2D Analytical Subdomain Method for Coaxial Magnetic Coupling Analysis. Energies 2021, 14, 4656. [Google Scholar] [CrossRef]
- Eker, M.; Özsoy, M. Investigation of the effect of demagnetization fault at Line Start AF-PMSM with FEM. Acad. Platf. J. Eng. Smart Syst. 2022, 10, 94–100. [Google Scholar] [CrossRef]
- Ziolkowski, M.; Brauer, H. Fast Computation Technique of Forces Acting on Moving Permanent Magnet. IEEE Trans. Magn. 2010, 46, 2927–2930. [Google Scholar] [CrossRef]
- Ose-Zala, B.; Onzevs, O.; Pugachov, V. Formula Synthesis of Maximal Mechanical Torque on Volume for Cylindrical Magnetic Coupler. Electr. Control. Commun. Eng. 2013, 3, 37–43. [Google Scholar] [CrossRef]
- Kang, H.-B.; Choi, J.-Y. Parametric Analysis and Experimental Testing of Radial Flux Type Synchronous Permanent Magnet Coupling Based on Analytical Torque Calculations. J. Electr. Eng. Technol. 2014, 9, 926–931. [Google Scholar] [CrossRef]
- Ose, B.; Pugachov, V. The Influence of Pole Pair Number and Magnets’ Width on Mechanical Torque of Magnetic Coupler with Rounded Permanent Magnets. Sci. J. Riga Tech. Univ. Power Electr. Eng. 2011, 28, 63–66. [Google Scholar] [CrossRef]
- Meng, G.Y.; Niu, Y.H. The Torque Research for Permanent Magnet Coupling Based on Ansoft Maxwell Transient Analysis. Appl. Mech. Mater. 2013, 423, 2014–2019. [Google Scholar] [CrossRef]
- Wang, J.; Lin, H.; Fang, S.; Huang, Y. A General Analytical Model of Permanent Magnet Eddy Current Couplings. IEEE Trans. Magn. 2014, 50, 1–9. [Google Scholar] [CrossRef]
- Kang, H.-B.; Choi, J.-Y.; Cho, H.-W.; Kim, J.-H. Comparative Study of Torque Analysis for Synchronous Permanent Magnet Coupling with Parallel and Halbach Magnetized Magnets Based on Analytical Field Calculations. IEEE Trans. Magn. 2014, 50, 1–4. [Google Scholar] [CrossRef]
- Lubin, T.; Mezani, S.; Rezzoug, A. Simple Analytical Expressions for the Force and Torque of Axial Magnetic Couplings. IEEE Trans. Energy Convers. 2012, 27, 536–546. [Google Scholar] [CrossRef]
- Ge, Y.-J.; Nie, C.-Y.; Xin, Q. A three dimensional analytical calculation of the air-gap magnetic field and torque of coaxial magnetic gears. Prog. Electromagn. Res. 2012, 131, 391–407. [Google Scholar] [CrossRef]
- Niu, S.; Mao, Y. A Comparative Study of Novel Topologies of Magnetic Gears. Energies 2016, 9, 773. [Google Scholar] [CrossRef]
- Jian, L.; Chau, K.-T. Analytical calculation of magnetic field distribution in coaxial magnetic gears. Prog. Electromagn. Res. 2009, 92, 1–16. [Google Scholar] [CrossRef]
- Chau, K.T.; Zhang, D.; Jiang, J.Z.; Liu, C.; Zhang, Y. Design of a Magnetic-Geared Outer-Rotor Permanent-Magnet Brushless Motor for Electric Vehicles. IEEE Trans. Magn. 2007, 43, 2504–2506. [Google Scholar] [CrossRef] [Green Version]
- Jian, L.; Chau, K.T. Design and analysis of a magnetic-geared electronic-continuously variable transmission system using finite element method. Prog. Electromagn. Res. 2010, 107, 47–61. [Google Scholar] [CrossRef]
- Wang, S.; Guo, Y.; Cheng, G.; Li, D. Performance Study of Hybrid Magnetic Coupler Based on Magneto Thermal Coupled Analysis. Energies 2017, 10, 1148. [Google Scholar] [CrossRef]
- Zheng, D.; Wang, D.; Li, S.; Shi, T.; Li, Z.; Yu, L. Eddy current loss calculation and thermal analysis of axial-flux permanent magnet couplers. AIP Adv. 2017, 7, 025117. [Google Scholar] [CrossRef]
- Min, K.-C.; Choi, J.-Y.; Kim, J.-M.; Cho, H.-W.; Jang, S.-M. Eddy-Current Loss Analysis of Noncontact Magnetic Device with Permanent Magnets Based on Analytical Field Calculations. IEEE Trans. Magn. 2015, 51, 1–4. [Google Scholar] [CrossRef]
- Ose-Zala, B.; Jakobsons, E.; Suskis, P. The use of Magnetic Coupler instead of Lever Actuated Friction Clutch for Wind Plant. Elektron. Elektrotechnika 2012, 18, 13–16. [Google Scholar] [CrossRef]
- Li, Y.; Hu, Y.; Song, B.; Mao, Z.; Tian, W. Performance Analysis of Conical Permanent Magnet Couplings for Underwater Propulsion. J. Mar. Sci. Eng. 2019, 7, 187. [Google Scholar] [CrossRef]
- Chau, K.T.; Jiang, C.; Han, W.; Lee, C.H.T. State-of-the-art electromagnetics research in electric and hybrid vehicles (invited paper). Prog. Electromagn. Res. 2017, 159, 139–157. [Google Scholar] [CrossRef]
- Saleh, K.; Sumner, M. Sensorless Speed Control of Five-Phase PMSM Drives in Case of a Single-Phase Open-Circuit Fault. Iran. J. Sci. Technol. Trans. Electr. Eng. 2019, 43, 501–517. [Google Scholar] [CrossRef]
- Niemenmaa, A.; Salmia, L.; Arkkio, A.; Saari, J. Modeling Motion, Stiffness, and Damping of a Permanent-Magnet Shaft Coupling. IEEE Trans. Magn. 2010, 46, 2763–2766. [Google Scholar] [CrossRef]
- Krasilnikov, A.Y.; Krasilnikov, A.A. Calculation of losses in current-conducting screen in sealed machines and devices due to loose packing of magnets in half-clutches of magnetic clutch. Chem. Pet. Eng. 2011, 47, 392–397. [Google Scholar] [CrossRef]
- Mei, Y.; Luo, J. Influence of Eccentric Arc Design at the Rotor on the Electromagnetic Performance of a Permanent-Magnet Synchronous Motor. Iran. J. Sci. Technol. Trans. Electr. Eng. 2022. [Google Scholar] [CrossRef]
- Fontchastagner, J.; Lefevre, Y.; Messine, F. Some Co-Axial Magnetic Couplings Designed Using an Analytical Model and an Exact Global Optimization Code. IEEE Trans. Magn. 2009, 45, 1458–1461. [Google Scholar] [CrossRef]
- Kim, S.H.; Shin, J.W.; Ishiyama, K. Magnetic Bearings and Synchronous Magnetic Axial Coupling for the Enhancement of the Driving Performance of Magnetic Wireless Pumps. IEEE Trans. Magn. 2014, 50, 1–4. [Google Scholar] [CrossRef]
- Cao, W.; Mecrow, B.C.; Atkinson, G.J.; Bennett, J.W.; Atkinson, D.J. Overview of Electric Motor Technologies Used for More Electric Aircraft (MEA). IEEE Trans. Ind. Electron. 2012, 59, 3523–3531. [Google Scholar] [CrossRef]
- Zareb, M.; Nouibat, W.; Bestaoui, Y.; Ayad, R.; Bouzid, Y. Evolutionary Autopilot Design Approach for UAV Quadrotor by Using GA. Iran. J. Sci. Technol. Trans. Electr. Eng. 2020, 44, 347–375. [Google Scholar] [CrossRef]
- Benarous, M.; Trezieres, M. Design of a cost-effective magnetic gearbox for an aerospace application. J. Eng. 2019, 2019, 4081–4084. [Google Scholar] [CrossRef]
- Charpentier, J.; Lemarquand, G. Calculation of ironless permanent magnet couplings using semi-numerical magnetic pole theory method. COMPEL-Int. J. Comput. Math. Electr. Electron. Eng. 2001, 20, 72–89. [Google Scholar] [CrossRef]
- Singh, R.; Lal, R.; Singari, R.; Chaudhary, R. Failure of Piston in IC Engines: A Review. Int. J. Mod. Eng. Res. 2014, 4, 1–10. [Google Scholar]
- Moosavian, A.; Najafi, G.; Ghobadian, B.; Mirsalim, M. The effect of piston scratching fault on the vibration behavior of an IC engine. Appl. Acoust. 2017, 126, 91–100. [Google Scholar] [CrossRef]
- 3W-Modellmotoren GmbH Home Page. Available online: https://3w-modellmotoren.de/produkt/bearcat-f8f-blau/?lang=en (accessed on 29 January 2023).
- IEEE. IEEE Approved Draft Trial-Use Guide for Testing Permanent Magnet Machines. In IEEE P1812/D5; IEEE: New York, NY, USA, 2014; pp. 1–65. [Google Scholar]
- Yang, X.; Pei, X. 15—Hybrid system for powering unmanned aerial vehicles: Demonstration and study cases. In Hybrid Energy Systems Hybrid Technologies for Power Generation; Elsevier: Amsterdam, The Netherlands, 2022; pp. 439–473. [Google Scholar] [CrossRef]
- Valavanis, K.P.; Vachtsevanos, G.J. Handbook of Unmanned Aerial Vehicles; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Easy Access Rules for Unmanned Aircraft Systems (Regulations (EU) 2019/947 and 2019/945). Official Journal of the European Union. 2022. Available online: https://www.easa.europa.eu/en/downloads/110913/en (accessed on 29 January 2023).
- Ose-Zala, B. Design Optimization of Cylindrical Magnetic Coupler Based on Calculations of Magnetic Field. Ph.D. Thesis, Department of Power and Electrical Engineering, Riga Technical University, Riga, Latvia, 2015. [Google Scholar]
- Suti, A.; Di Rito, G.; Galatolo, R. Fault-Tolerant Control of a Dual-Stator PMSM for the Full-Electric Propulsion of a Lightweight Fixed-Wing UAV. Aerospace 2022, 9, 337. [Google Scholar] [CrossRef]
- Quattrocchi, G.; Berri, P.C.; Vedova, M.D.L.D.; Maggiore, P. An Improved Fault Identification Method for Electromechanical Actuators. Aerospace 2022, 9, 341. [Google Scholar] [CrossRef]
- Akcay, Y.; Giangrande, P.; Galea, M. A Novel Magnetic Coupling Configuration for Enhancing the Torque Density. In Proceedings of the 23rd International Conference on Electrical Machines and Systems (ICEMS), Hamamatsu, Japan, 24–27 November 2020; pp. 228–233. [Google Scholar] [CrossRef]
- Ravaud, R.; Lemarquand, V.; Lemarquand, G. Analytical Design of Permanent Magnet Radial Couplings. IEEE Trans. Magn. 2010, 46, 3860–3865. [Google Scholar] [CrossRef]
- Bossavit, A. Virtual power principle and Maxwell’s tensor: Which comes first? COMPEL Int. J. Comput. Math. Electr. Electron. Eng. 2011, 30, 1804–1814. [Google Scholar] [CrossRef]
- Yonnet, J.-P.; Hemmerlin, S.; Rulliere, E.; Lemarquand, G. Analytical calculation of permanent magnet couplings. IEEE Trans. Magn. 1993, 29, 2932–2934. [Google Scholar] [CrossRef]
- Nagrial, M.H.; Rizk, J.; Hellany, A. Design of synchronous torque couplers. World Acad. Sci. Eng. Technol. Int. J. Mech. Mechatron. Eng. 2011, 5, 1319–1324. [Google Scholar] [CrossRef]
- Lin, W.Y.; Kuan, L.P.; Jun, W.; Han, D. Near-Optimal Design and 3-D Finite Element Analysis of Multiple Sets of Radial Magnetic Couplings. IEEE Trans. Magn. 2009, 44, 4747–4753. [Google Scholar] [CrossRef]
- Maxwell Help Overview Statistical Analysis Overview, Release 20.1; Ansys® Electronics: Canonsburg, PA, USA, 2020.
- Ose, B.; Pugachov, V.; Orlova, S.; Vanags, J. The influence of permanent magnets’ width and number on the mechanical torque of a magnetic coupler with rectangular permanent magnets. In Proceedings of the 2011 IEEE International Symposium on Industrial Electronics, Gdansk, Poland, 27–30 June 2011; pp. 761–765. [Google Scholar] [CrossRef]
- Nachouane, A.B.; Abdelli, A.; Friedrich, G.; Vivier, S. Estimation of Windage Losses inside Very Narrow Air Gaps of High Speed Electrical Machines without an Internal Ventilation Using CFD Methods. In Proceedings of the 2016 XXII International Conference on Electrical Machines (ICEM), Lausanne, Switzerland, 4–7 September 2016. [Google Scholar] [CrossRef]
- Suti, A.; Di Rito, G.; Galatolo, R. Novel Approach to Fault-Tolerant Control of Inter-Turn Short Circuits in Permanent Magnet Synchronous Motors for UAV Propellers. Aerospace 2022, 9, 401. [Google Scholar] [CrossRef]
- Bojoi, R.; Cavagnino, A.; Miotto, A.; Tenconi, A.; Vaschetto, S. Radial flux and axial flux PM machines analysis for more electric engine aircraft applications. In Proceedings of the 2010 IEEE Energy Conversion Congress and Exposition, Atlanta, GA, USA, 12–16 September 2010; pp. 1672–1679. [Google Scholar] [CrossRef]
- Ums Quality Electrical Calibration Co., Ltd. Home Page. Available online: http://www.umsankara.com.tr (accessed on 29 January 2023).
- Zipay, J.J.; Modlin, C.T.; Larsen, C.E. The Ultimate Factor of Safety for Aircraft and Spacecraft—Its History, Applications and Misconceptions. In Proceedings of the 57th AIAA/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, San Diego, CA, USA, 4–8 January 2016. [Google Scholar] [CrossRef] [Green Version]


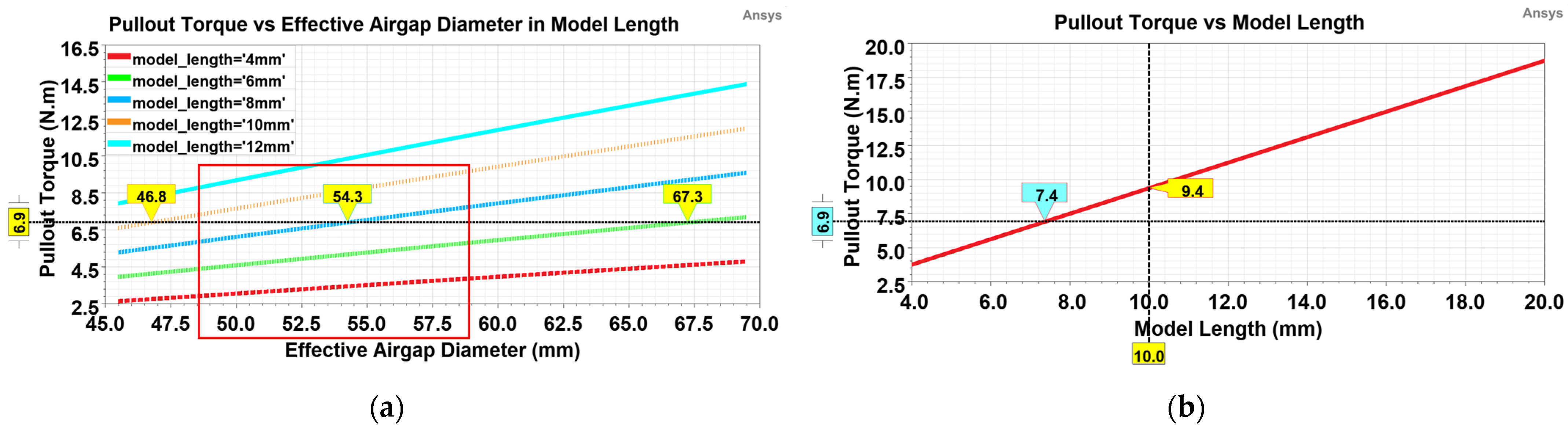





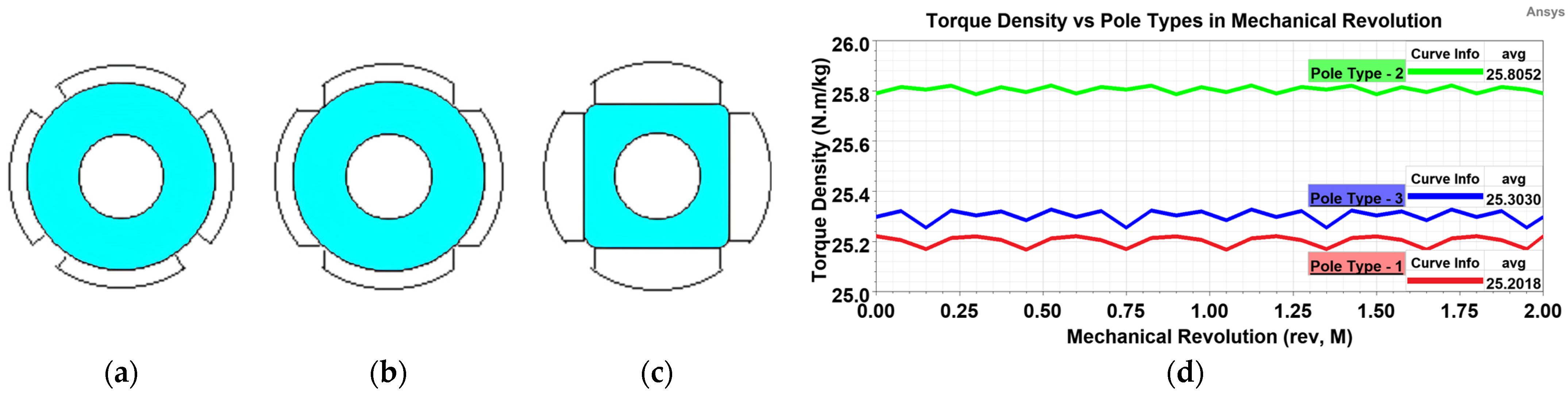

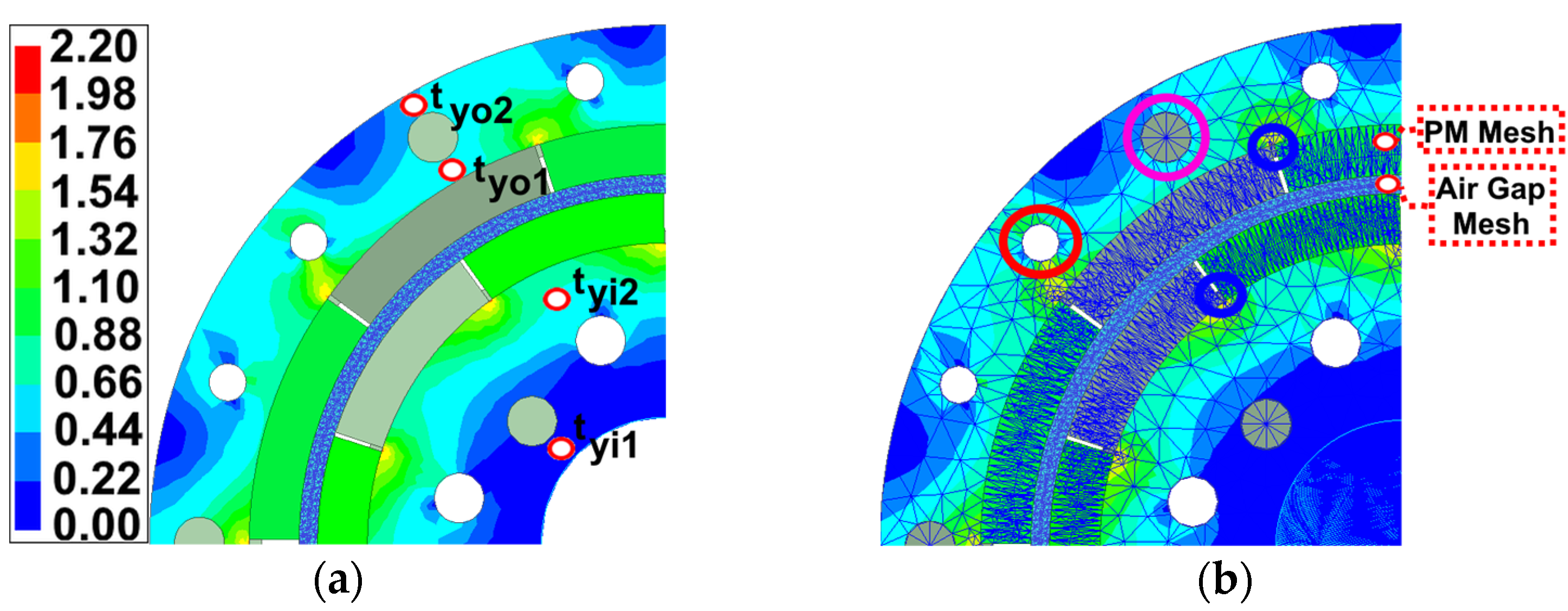
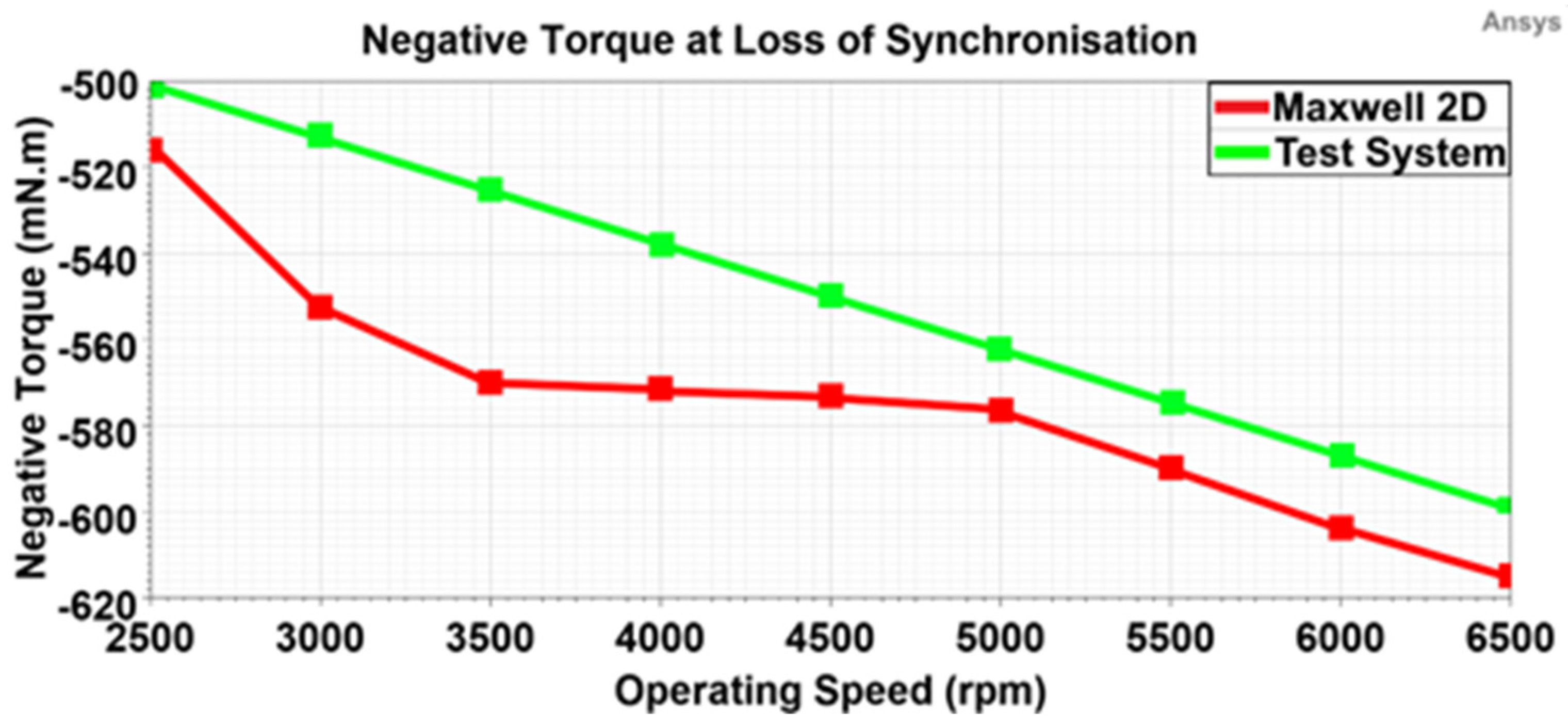



| Parameters | Value |
|---|---|
| Pullout torque, with safety factor and correction coefficient | 6.9 N·m |
| Minimum torque density, required | 18.4 N·m/kg |
| Rated speed | 4500 rpm |
| Operation speed range | 2500–6500 rpm |
| Design Outputs | 10-Pole |
| Air gap volume, minimum required | 1289.5 mm3 |
| Length of the model, based on rmean | 5 mm |
| (θ-dθ), dθ at critical torque angle | 0.314 rad. |
| Critical torque angle | 18 (°M) |
| Design Assumptions | |
| Middle of the air gap radius, rmean | 27.5 mm |
| Average air gap flux density | 0.65 T |
| Air gap length | 1.5 mm |
| Pullout torque, required | 6.9 N·m |
| Pole Number | 8-Pole | 10-Pole * | ||||
|---|---|---|---|---|---|---|
| Embrace | 0.6 | 0.8 | 0.98 | 0.8 | 0.98 * | |
| PM Thickness | 3 mm | 4 mm | 4 mm | 4 mm * | ||
| Grade of PM | N48H | N45H | N48H | N48H | N48H * | |
| Outer diameter of outer rotor | 79 mm | 83 mm | 83 mm * | |||
| Inner diameter of outer rotor | 57 mm | 59 mm | 59 mm * | |||
| Outer diameter of inner rotor | 54 mm | 56 mm | 56 mm * | |||
| Inner diameter of inner rotor | 20 mm | 20 mm | 20 mm * | |||
| Air gap length | 1.5 mm | 1.5 mm | 1.5 mm * | |||
| Effective air gap diameter | 55.5 mm | 57.5 mm | 57.5 mm * | |||
| Model length | 10 mm | 10 mm | 10 mm * | |||
| Total weight (gr) | 320 | 350 | 351 | 370 | 352 | 371 |
| Pullout torque (N·m), dynamic | 3.9 | 6.9 | 7.2 | 7.5 | 8.1 | 8.7 |
| Torque density (N·m/kg) | 12.2 | 19.7 | 20.5 | 20.3 | 23 | 23.45 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arslan, S.; Iskender, I.; Navruz, T.S. Finite Element Method-Based Optimisation of Magnetic Coupler Design for Safe Operation of Hybrid UAVs. Aerospace 2023, 10, 140. https://doi.org/10.3390/aerospace10020140
Arslan S, Iskender I, Navruz TS. Finite Element Method-Based Optimisation of Magnetic Coupler Design for Safe Operation of Hybrid UAVs. Aerospace. 2023; 10(2):140. https://doi.org/10.3390/aerospace10020140
Chicago/Turabian StyleArslan, Sami, Ires Iskender, and Tuğba Selcen Navruz. 2023. "Finite Element Method-Based Optimisation of Magnetic Coupler Design for Safe Operation of Hybrid UAVs" Aerospace 10, no. 2: 140. https://doi.org/10.3390/aerospace10020140
APA StyleArslan, S., Iskender, I., & Navruz, T. S. (2023). Finite Element Method-Based Optimisation of Magnetic Coupler Design for Safe Operation of Hybrid UAVs. Aerospace, 10(2), 140. https://doi.org/10.3390/aerospace10020140






