Simulation of Drilling Temperature Rise in Frozen Soil of Lunar Polar Region Based on Discrete Element Theory
Abstract
:1. Introduction
2. Model
2.1. Heat Transfer Model
2.1.1. Heat Transfer Model between Particles
2.1.2. Heat Transfer Model of Geometry
- The drill tool is discretized into equispaced drill tool elements and segmented into time step;
- In the current time interval, the variations of the drill temperature caused by the heat source at the front of the drill were calculated, and the influence of convective heat transfer and radiation heat dissipation on the heat transfer inside the drill is considered;
- In the current time interval, the original continuous temperature field was no longer continuous because of radiation or convection, and thermal was transferred from the high-temperature element to the low-temperature element inside the drill tool. Herein, this process is referred to as the secondary heat conduction. Moreover, secondary heat conduction was calculated using the central difference method;
- In the subsequent time interval, steps 2 and 3 are repeated;
- End of simulation and output results.
2.2. Discrete Element Model
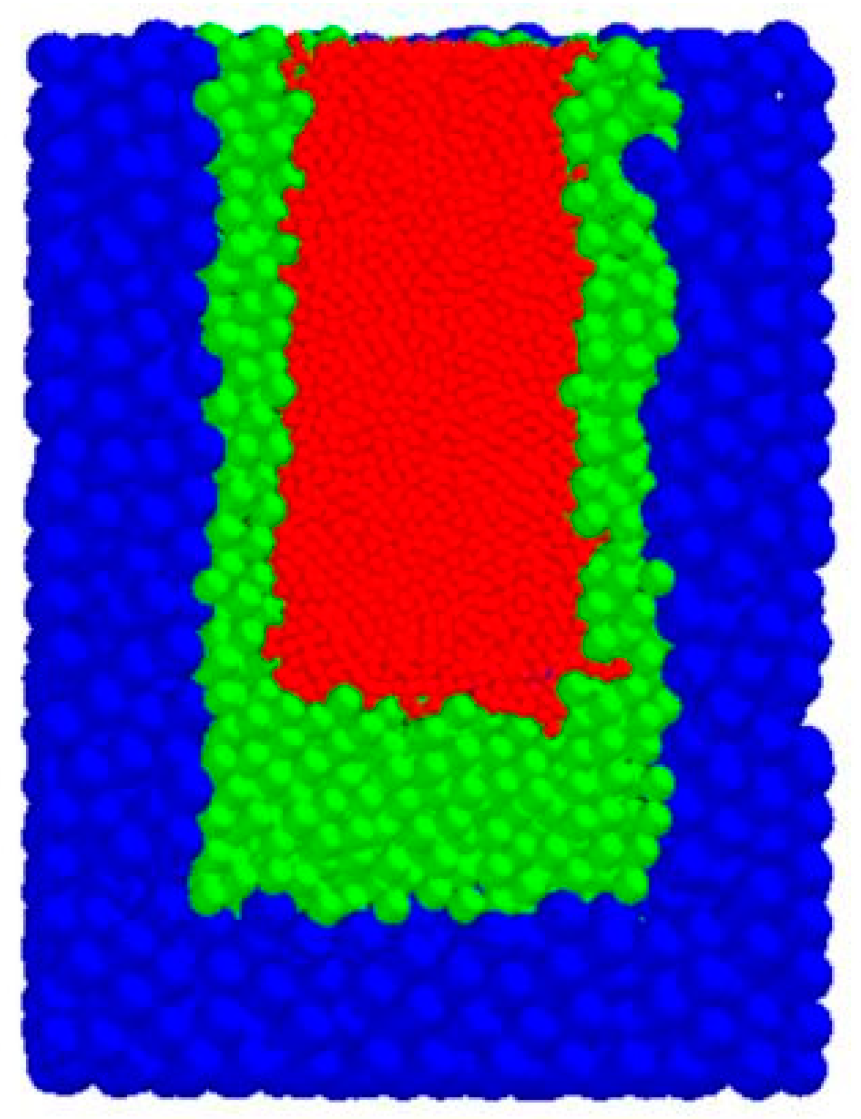
2.2.1. Particle Modeling
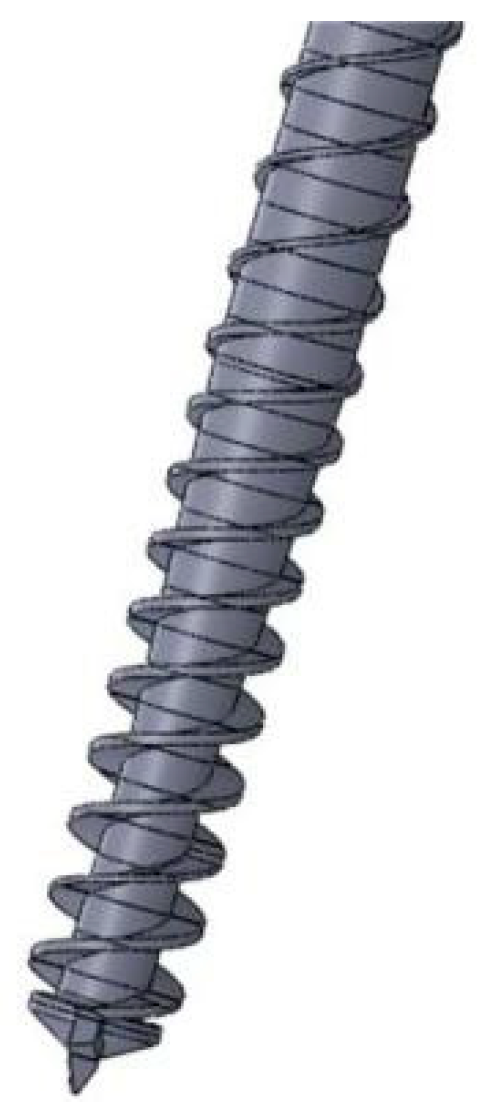
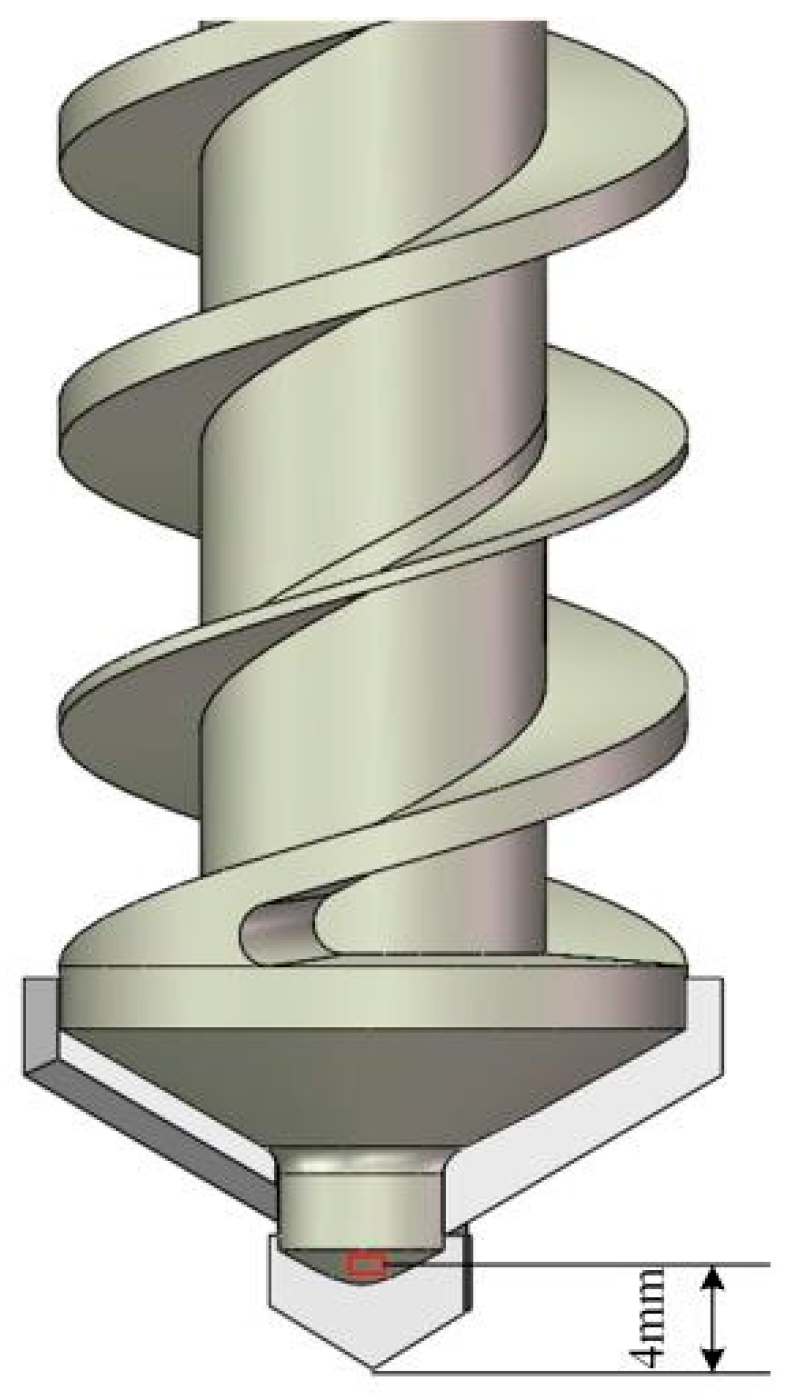
2.2.2. Geometric Modeling
3. Experimental Apparatus and Simulated Frozen Lunar Soil
3.1. Experimental Apparatus
3.2. Simulated Frozen Lunar Soil
- Weigh all kinds of particle size anorthosite and basalt, into the oven for drying (more than 8 h);
- According to the different material different particle size ratio configuration, place into a blender for uniform mixing;
- After the mixing of dry soil, the mixing of water samples should be allocated according to dry soil and different water content.
- After the completion of mixed water configuration, homogenize seal stand for 6 to 8 h;
- Use a press to compact the sample five times to the required compactness;
- Sample the samples after compaction to verify the actual moisture content of the samples after preparation;
- Transfer the sample to the secondary refrigeration freezer (−80 °C) for storage after 6–8 h of primary refrigeration (−30 °C), and the sample needs to undergo secondary refrigeration for 6–8 h before use.
4. Results and Discussion
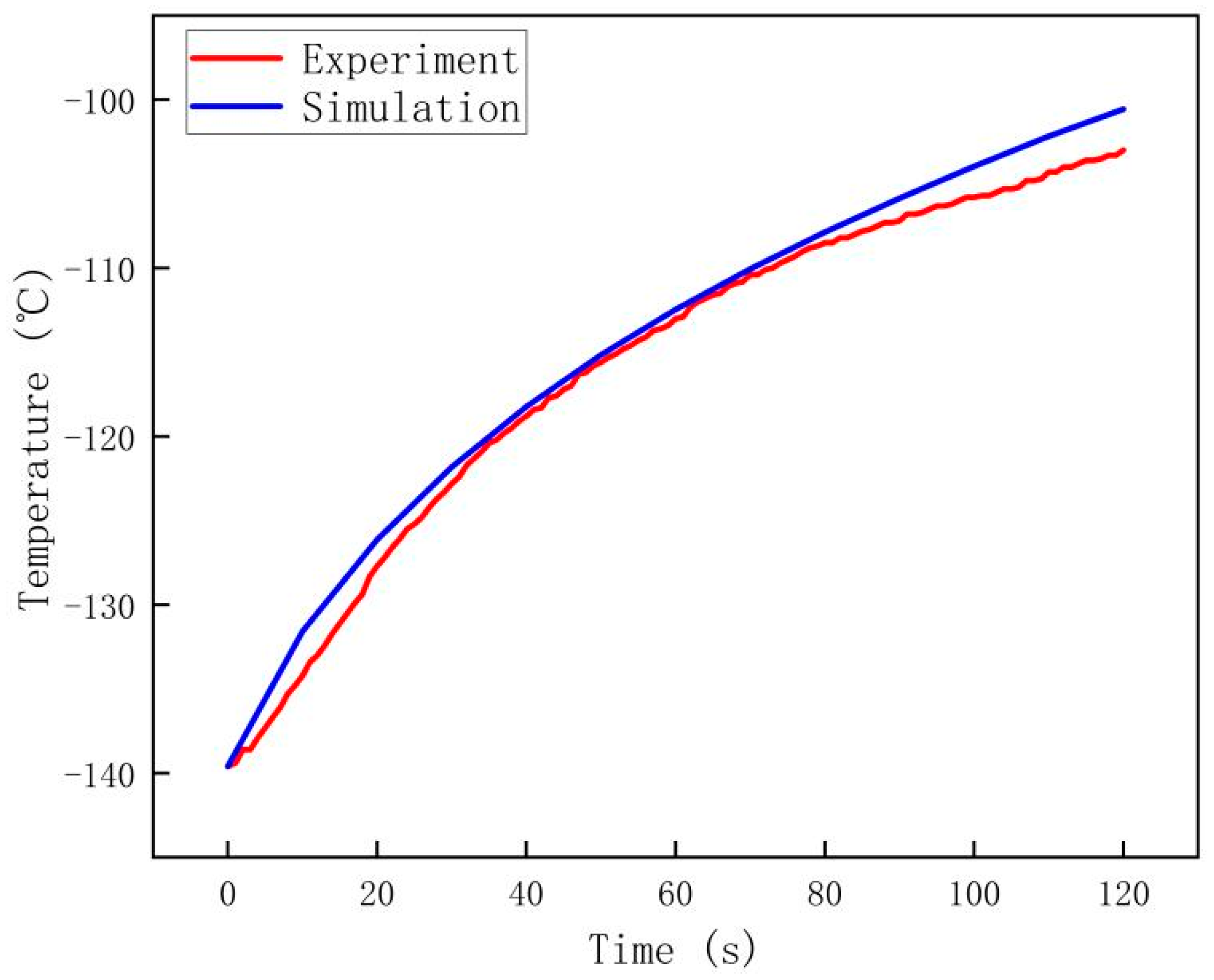
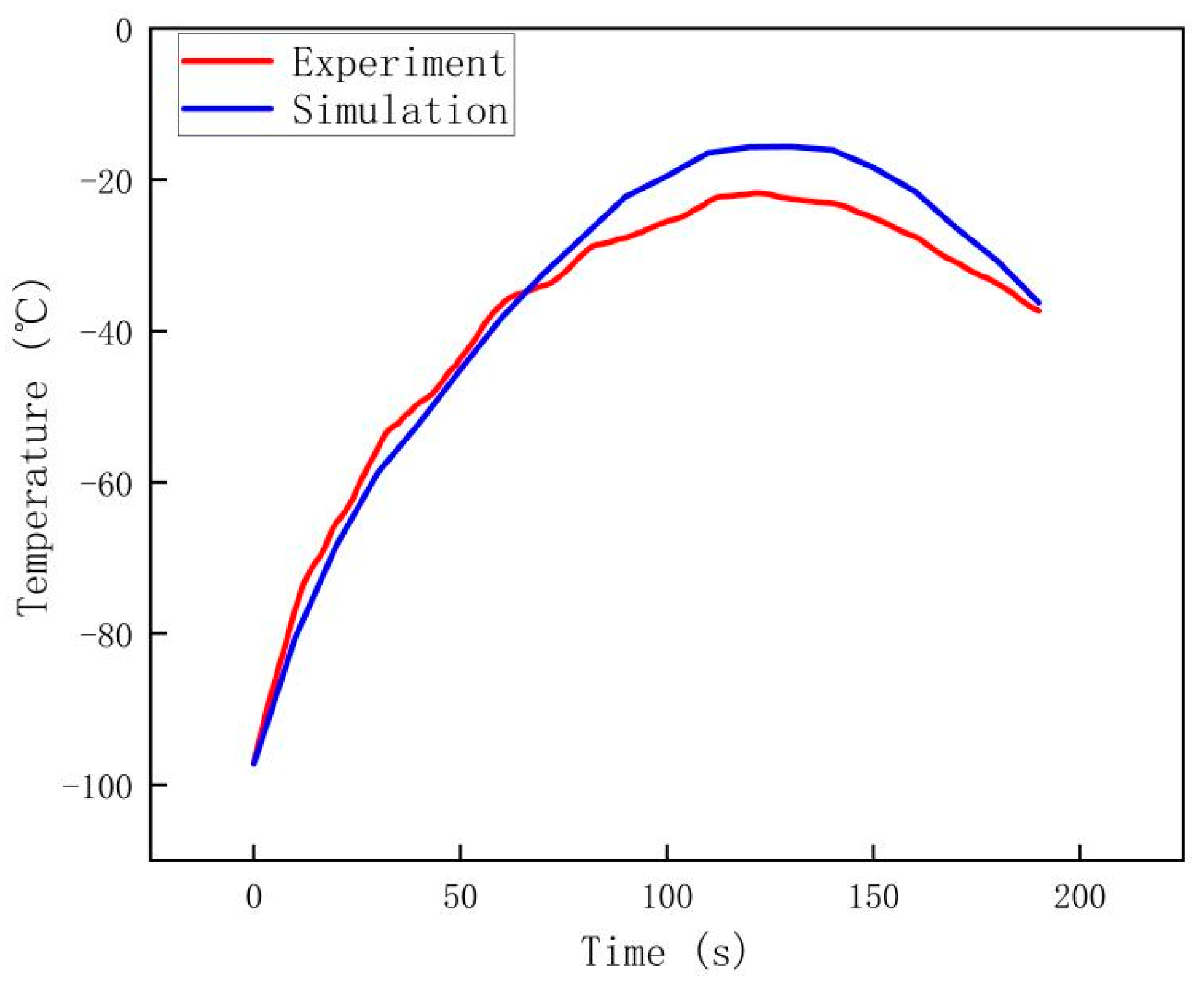
4.1. Drilling Parameters and Experimental Results
4.2. Simulation Parameters and Results
4.2.1. Simulation Parameters
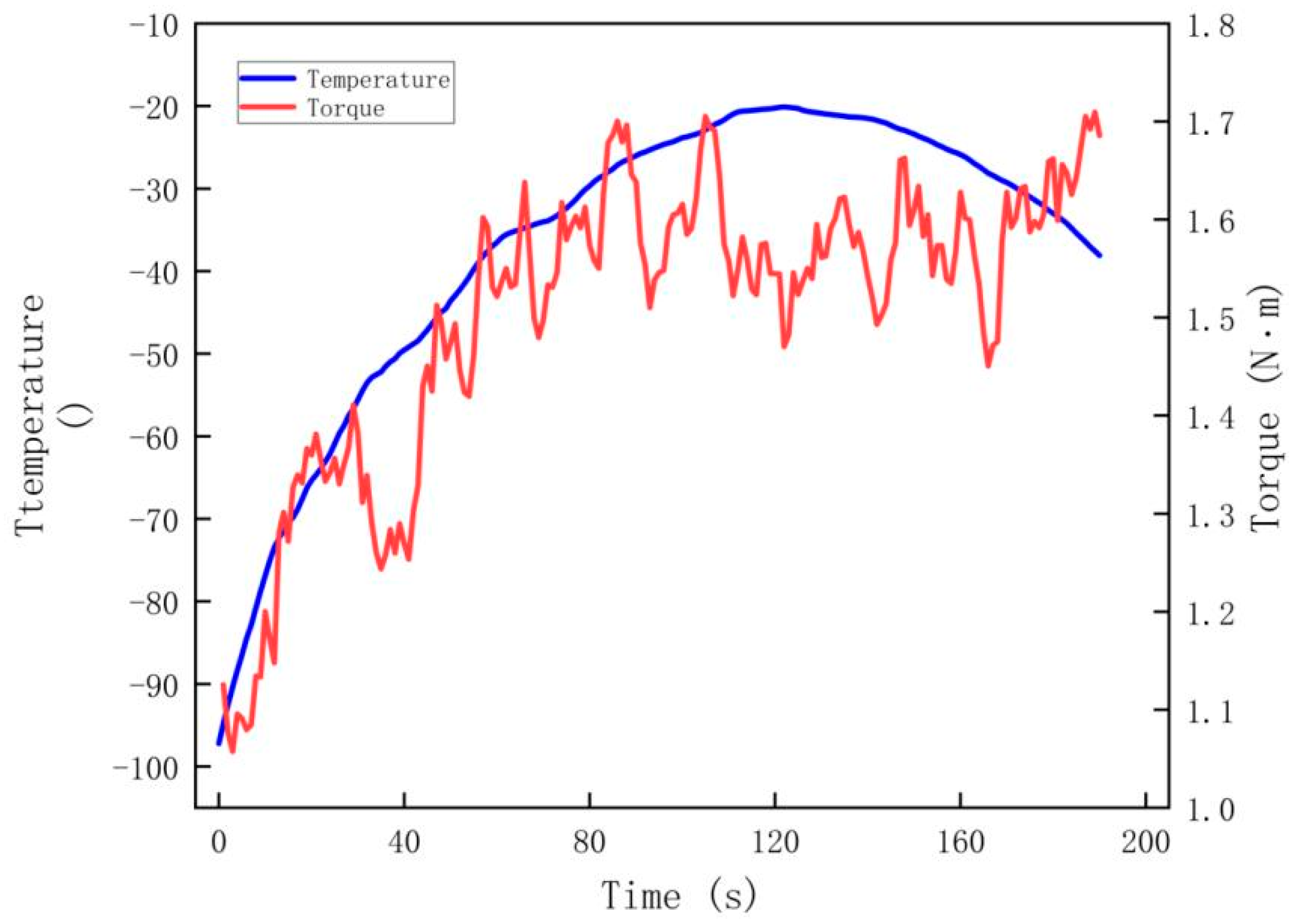
4.2.2. Simulation Results
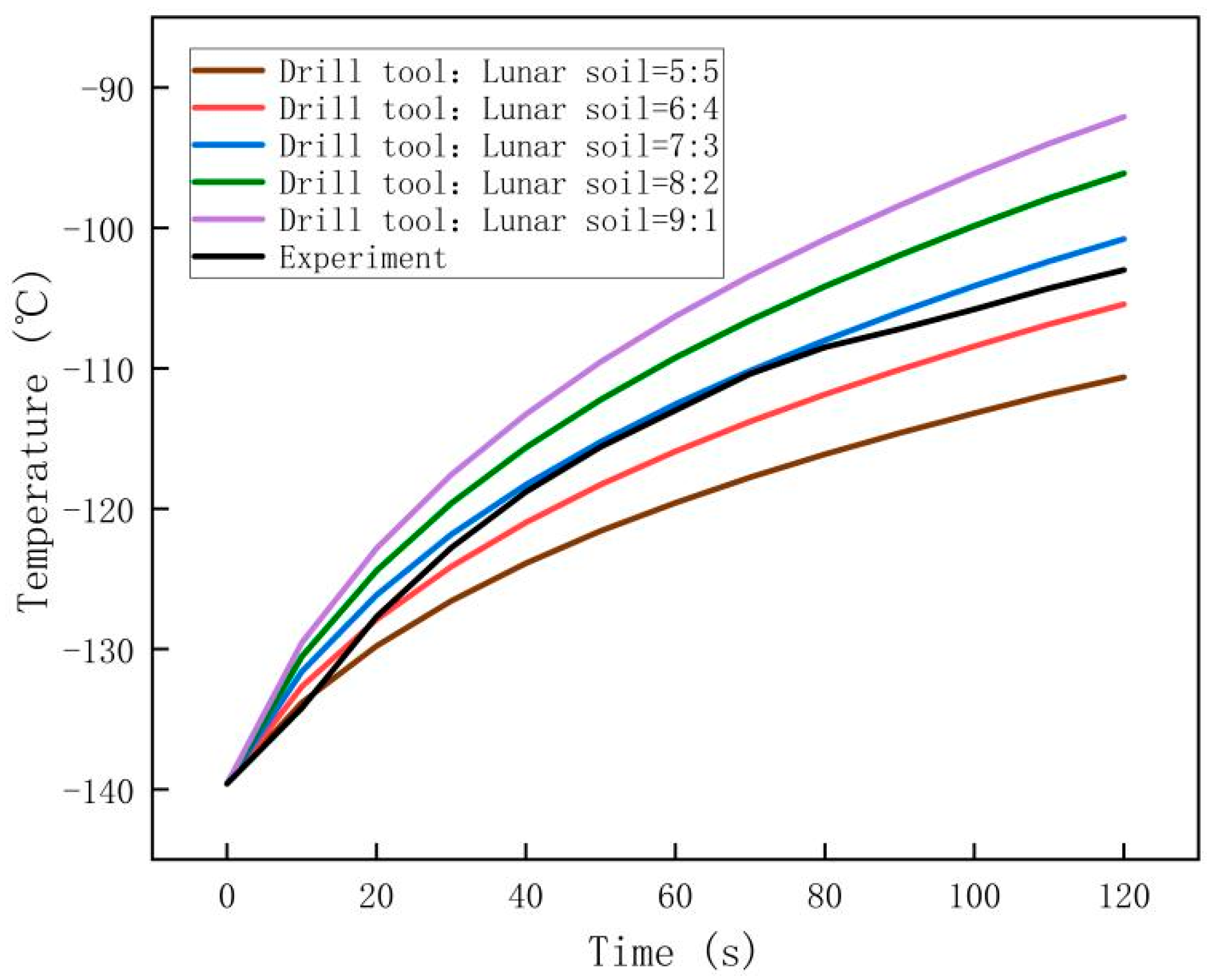
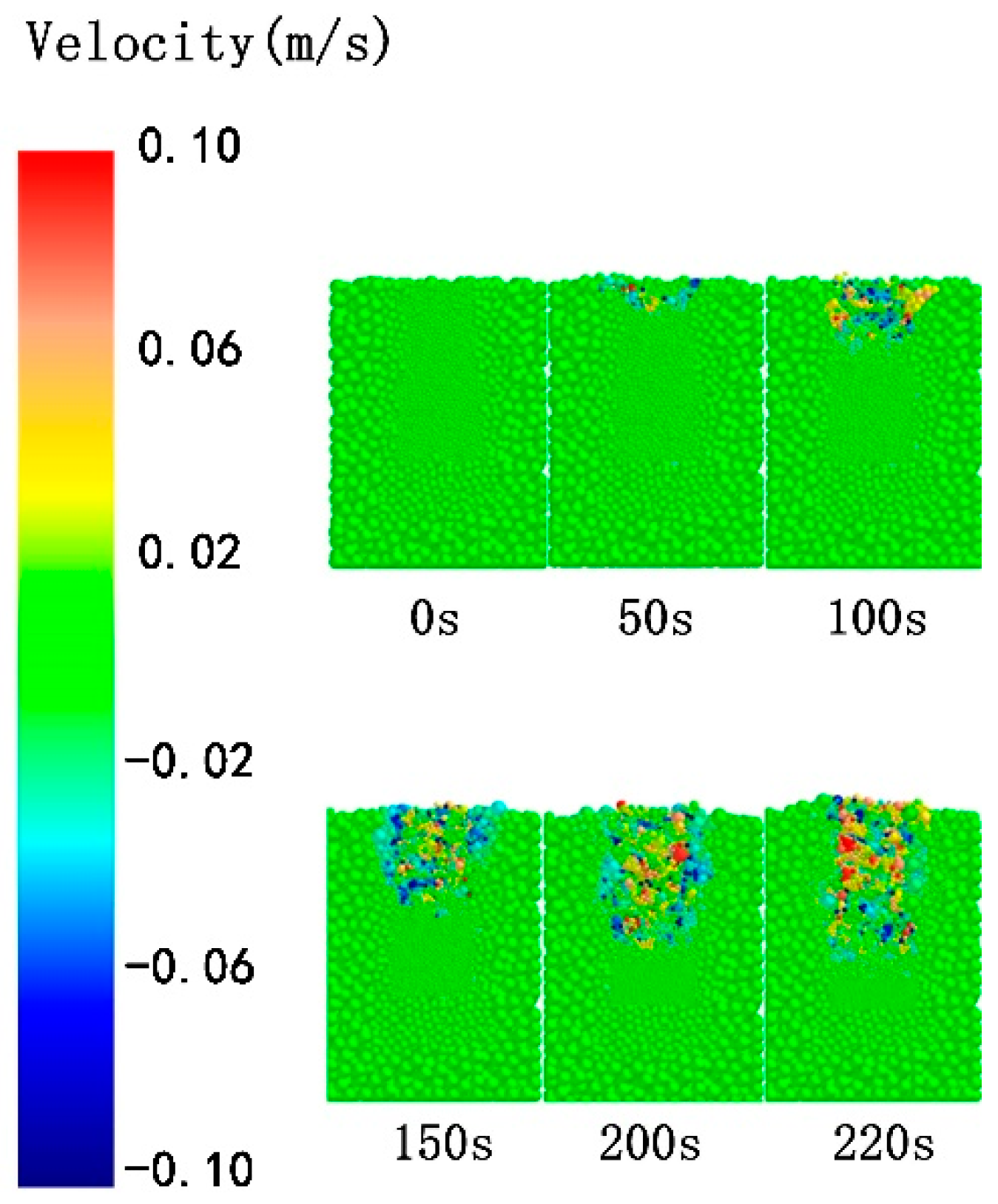
4.3. Analysis and Discussion
5. Conclusions
- The error between the results of the discrete element simulation and experiments in terms of temperature increase was approximately 10%, indicating that the developed model can calculate the increase in drilling tool temperature with a certain applicability in the drilling process.
- Under the drilling conditions and reasonable considerations of this study, the maximum increase in the drill bit temperature was approximately 60 °C.
- The heat distribution ratio between the drill tool and the simulated lunar soil will change during drilling. The results indicated that the current calculation model exhibited a high adaptability, and the calculation results were not invalid due to fluctuations in the distribution ratio.
- In the simulations, the majority of the lunar soil particles near the drill tool traversed along the positive direction of the Z-axis, and the flow of the simulated lunar soil particles could effectively reduce the rate of temperature increase for the drill bit. Only a few particles near the drill bit traversed along the negative Z-axis, which is one of the reasons for the high temperature of the simulated lunar soil particles at the front of the drill tool.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Liu, D.Y.; Zhang, H.; Yang, S.; Yin, C.; Zhang, J.B.; Sun, Q.C.; Lai, X.M. Lunar polar drilling sampling technique. J. Deep Space Explor. 2020, 7, 278–289. (In Chinese) [Google Scholar]
- Liu, D.Y.; Wang, L.S.; Sun, Q.C.; Lai, X.M.; Liu, J.W. Experimental study on simulated lunar soil drilling of frozen soil in lunar polar region. J. Sci. Technol. Eng. 2018, 18, 256–261. (In Chinese) [Google Scholar]
- Wang, C.; Zhang, X.J.; Yao, W. Progress in in-situ development and utilization of water ice resources in lunar polar region. J. Deep Space Explor. 2020, 7, 241–247. (In Chinese) [Google Scholar]
- Stephen, E.W. A mechanistic model for the thermal conductivity of planetary regolith: 1. The effects of particle shape, composition, cohesion, and compression at depth. Icarus 2020, 352, 113964. [Google Scholar]
- Chen, T.; Zhao, Z.; Schwartz, S.R.; Liu, C.S.; Wang, Q. Invariance of conveying capacity for drilling into lunar soil simulant. Adv. Space. Res. 2019, 64, 1816–1824. [Google Scholar] [CrossRef]
- Zhang, T.; Chao, C.Y.; Yao, Z.X.; Xu, K.; Zhang, W.X.; Ding, X.L.; Liu, S.T.; Zhao, Z.; An, Y.H.; Wang, B. The technology of lunar regolith environment construction on Earth. Acta Astronaut. 2020, 178, 216–232. [Google Scholar] [CrossRef]
- Li, Z.W.; Zeng, X.Y.; Wen, T.G.; Zhang, Y.L. Numerical Comparison of Contact Force Models in the Discrete Element Method. J. Aerosp. 2022, 9, 737. [Google Scholar] [CrossRef]
- Vargas, W.L.; McCarthy, J.J. Heat conduction in granular materials. J. AIChE 2001, 47, 1052–1059. [Google Scholar] [CrossRef]
- Vargas, W.L.; McCarthy, J.J. Stress effects on the conductivity of particulate beds. Chem. Eng. Sci. 2002, 57, 3119–3131. [Google Scholar] [CrossRef]
- Vargas, W.L.; McCarthy, J.J. Conductivity of granular media with stagnant interstitial fluids via thermal particle dynamics simulation. Int. J. Heat Mass Transf. 2002, 45, 4847–4856. [Google Scholar] [CrossRef]
- Vargas, W.L.; McCarthy, J.J. Thermal expansion effects and heat conduction in granular materials. Phys. Rev. E 2007, 76, 041301. [Google Scholar] [CrossRef] [PubMed]
- Cui, J.S.; Hou, X.Y.; Wen, G.L.; Liang, Z.W. Thermal simulation of drilling into lunar rock simulant by discrete element method. Acta Astronaut. 2019, 160, 378–387. [Google Scholar] [CrossRef]
- Liu, T.X.; Zhou, J.; Liang, L.; Zhao, Y.; Cao, D.Q. Investigation of effect of stones on lunar regolith drilling using DEM. Comput. Geotech. 2021, 131, 103958. [Google Scholar] [CrossRef]
- Gong, Z.; Wu, Y.; Zhu, Z.G.; Wang, Y.; Liu, Z.Y.; Xu, W.X. DEM and dual-probability-Brownian motion scheme for thermal conductivity of multiphase granular materials with densely packed non-spherical particles and soft interphase networks. Comput. Method. Appl. M.. 2020, 372, 113372. [Google Scholar] [CrossRef]
- Liang, X.; Liu, X.J.; Xia, D.H. Numerical investigation of the gas–solid heat transfer characteristics of packed multi-size particles. Int. J. Heat Mass Tran.. 2020, 149, 119237. [Google Scholar] [CrossRef]
- Wu, H.; Gui, N.; Yang, X.T.; Tu, J.Y.; Jiang, S.Y. A matrix model of particle-scale radiative heat transfer in structured and randomly packed pebble bed. Int. J. Therm. Sci. 2020, 153, 106334. [Google Scholar] [CrossRef]
- Tang, S.; Zheng, B.; Sun, P.; Wang, Q.Z.; Wang, Z.L.; Han, X.B.; Chang, R.Z.; Shen, Y.K.; Liu, Y.Q. Effect of particle characteristic parameters on the heat transfer process of double vacancy particle bed. Int. Commun. Heat Mass Transf. 2020, 119, 104995. [Google Scholar] [CrossRef]
- Lee, D.; Pham, K.; Kang, M.; Park, S.; Choi, H. Stepwise DE/FE combined approach for estimating effective thermal conductivity of frozen spherical particulate media. Comput. Geotech. 2020, 128, 103837. [Google Scholar] [CrossRef]
- Calvet, T.; Vanson, J.M.; Masson, R. A DEM/FFT approach to simulate the effective thermal conductivity of granular media. Int. J. Therm. Sci. 2022, 172, 107339. [Google Scholar] [CrossRef]
- Govender, N.; Cleary, P.W.; Kiani-Oshtorjani, M.; Wilke, D.N.; Wu, C.Y.; Kureck, H. The effect of particle shape on the packed bed effective thermal conductivity based on DEM with polyhedral particles on the GPU. Chem. Eng. Sci. 2020, 219, 115584. [Google Scholar] [CrossRef]
- Xiao, Y.; Ma, G.L.; Nan, B.W.; McCartney, J.S. Thermal Conductivity of Granular Soil Mixtures with Contrasting Particle Shapes. J. Geotech. Geoenviron.. 2020, 146, 06020004. [Google Scholar] [CrossRef] [Green Version]
- Chen, L.; Wang, C.; Moscardini, M.; Kamlah, M.; Liu, S.L. A DEM-based heat transfer model for the evaluation of effective thermal conductivity of packed beds filled with stagnant fluid: Thermal contact theory and numerical simulation. Int. J. Heat Mass Tran.. 2019, 132, 331–346. [Google Scholar] [CrossRef]
- Peeketi, A.R.; Moscardini, M.; Vijayan, A.; Gan, Y.; Kamlah, M.; Annabattula, R.K. Effective thermal conductivity of a compacted pebble bed in a stagnant gaseous environment: An analytical approach together with DEM. Fusion Eng. Des. 2018, 130, 80–88. [Google Scholar] [CrossRef]
- Cui, J.S.; Hou, X.Y.; Deng, Z.Q.; Pan, W.J.; Quan, Q.Q. Prediction of the temperature of a drill in drilling lunar rock simulant in a vacuum. J. Therm. Sci. 2017, 21, 989–1002. [Google Scholar] [CrossRef]
- Cui, J.S. Study on filling model and characteristics of simulated lunar soil. Master. Harbin Harbin Inst. Heilongjiang. 2011. (In Chinese) [Google Scholar]
- Deng, Z.Q.; Cui, J.S.; Hou, X.Y.; Jiang, S.Y. Calibration of Discrete Element Heat Transfer Parameters by Central Composite Design. Chin. J. Mech. Eng.-EN. 2017, 30, 419–427. [Google Scholar] [CrossRef]






| Water Content (wt) | Effective Thermal Conductivity (W/m·K) | Specific Heat Capacity (J/kg·°C) | Shear Modulus (MPa) |
|---|---|---|---|
| 5% | 50.00 | 228.95 | 40.00 |
| 10% | 66.18 | 406.70 | 40.00 |
| Mineral Class @Proportion of | Particle Size Range | Proportion of |
|---|---|---|
| Anorthosite(A) @(70%) | 0.025–0.05 mm | 31.568% |
| 0.05–0.075 mm | 6.797% | |
| 0.075–0.1 mm | 10.545% | |
| 0.25–0.5 mm | 10.545% | |
| 0.5–1 mm | 10.545% | |
| Basalt(B) @(30%) | 0.025–0.05 mm | 13.502% |
| 0.05–0.075 mm | 2.920% | |
| 0.075–0.1 mm | 4.526% | |
| 0.25–0.5 mm | 4.526% | |
| 0.5–1 mm | 4.526% |
| Type | Parameter | ||
|---|---|---|---|
| Particle size range | 0–1 mm | ||
| Sample temperature | 93 K | ||
| Basic mineral | Pure dry soil sample and mixed water sample | ||
| Moisture content | 5wt% | 10wt% | |
| Density (g/cm3) | 1.9 | 1.75 | |
| Measurement result | Effective thermal conductivity(W/(m·K)) | 0.8611 | 1.1397 |
| Specific heat capacity (J/(kg·°C)) | 228.95 | 270.6 | |
| No. | Water Content (wt%) | Rotational Speed (rpm) | Feed Rate (mm/min) | Drilling Duration (s) |
|---|---|---|---|---|
| A1 | 5% | 120 | 0.62 | 120 |
| A2 | 5% | 250 | 12.66 | 220 |
| A3 | 10% | 250 | 5.70 | 190 |
| Parameters of the Particle System | Parameter |
|---|---|
| Effective thermal conductivity of particles (W/m·K) | 50.00 (A1), 50.00 (A1), 66.18 (A1) |
| specific heat capacity of a particle (J/kg·°C) | 228.95 (A1), 228.95 (A2), 406.70 (A3) |
| Particle radius (mm) | 0.8 mm, 1.6 mm, and 2.4 mm |
| Particle density (kg/m3) | 3 × 103 |
| Shear modulus of particles (Pa) | 4 × 107 |
| Poisson’s ratio of particles | 0.25 |
| Thermal conductivity of geometry (W/m·K) | 44.19 |
| Specific heat of geometry (J/kg·°C) | 544.00 |
| Geometric density (kg/m3) | 7.85 × 103 |
| Shear modulus of geometry (Pa) | 8 × 1010 |
| Poisson’s ratio of geometry | 0.25 |
| Particle-particle friction coefficient | 0.50 |
| Particle-geometry friction coefficient | 0.48 |
| Coefficient of restitution | 0.50 |
| Inner diameter of drill pipe (mm) | 12.00 |
| External diameter of drill pipe (mm) | 17.00 |
| Thickness of drill pipe (mm) | 2.50 |
| Cross-sectional area of drill pipe (mm2) | 113.83 |
| Rotational speed of drill (rpm) | 120.00 (A1), 250.00 (A2), 250.00 (A3) |
| Feed rate of the drill (m/s) | 1.03 × 10−5 (A1), 2.10 × 10–4 (A2), 9.50 × 10–5 (A3) |
| Initial temperature (°C) | –139.60 (A1), –149.70 (A2), –97.20 (A3) |
| No. | A1 | A2 | A3 |
|---|---|---|---|
| Temperature increase in experiments (°C) | 36.60 | 58.80 | 59.11 |
| Temperature increase in simulation (°C) | 39.04 | 52.77 | 60.94 |
| Relative error of temperature rise | 6.67% | 10.26% | 3.10% |
| Maximum error of experimental and simulation (°C) | 2.63 | 13.32 | 5.43 |
| Curve-fitting degree | 0.99 | 0.90 | 0.92 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cui, J.; Kui, L.; Zhang, W.; Zhao, D.; Chang, J. Simulation of Drilling Temperature Rise in Frozen Soil of Lunar Polar Region Based on Discrete Element Theory. Aerospace 2023, 10, 368. https://doi.org/10.3390/aerospace10040368
Cui J, Kui L, Zhang W, Zhao D, Chang J. Simulation of Drilling Temperature Rise in Frozen Soil of Lunar Polar Region Based on Discrete Element Theory. Aerospace. 2023; 10(4):368. https://doi.org/10.3390/aerospace10040368
Chicago/Turabian StyleCui, Jinsheng, Le Kui, Weiwei Zhang, Deming Zhao, and Jiaqing Chang. 2023. "Simulation of Drilling Temperature Rise in Frozen Soil of Lunar Polar Region Based on Discrete Element Theory" Aerospace 10, no. 4: 368. https://doi.org/10.3390/aerospace10040368
APA StyleCui, J., Kui, L., Zhang, W., Zhao, D., & Chang, J. (2023). Simulation of Drilling Temperature Rise in Frozen Soil of Lunar Polar Region Based on Discrete Element Theory. Aerospace, 10(4), 368. https://doi.org/10.3390/aerospace10040368






