Gust Alleviation by Active–Passive Combined Control of the Flight Platform and Antenna Array for a Flying Wing SensorCraft
Abstract
:1. Introduction
- Adopting a sensor–integrated design.
- The high–aspect–ratio wing is designed to improve the cruise lift–to–drag ratio and extend the flight time in the mission area.
- (1)
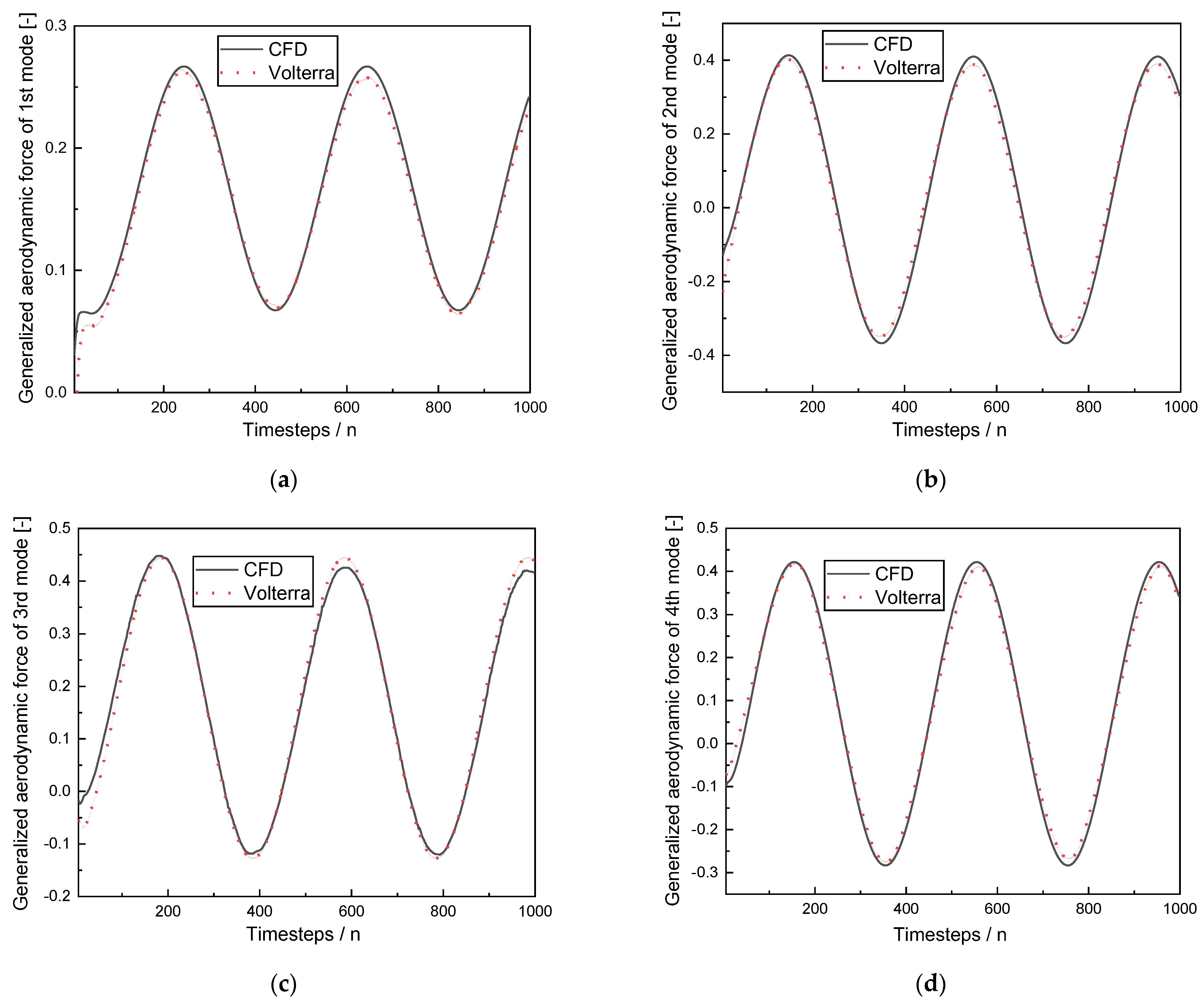
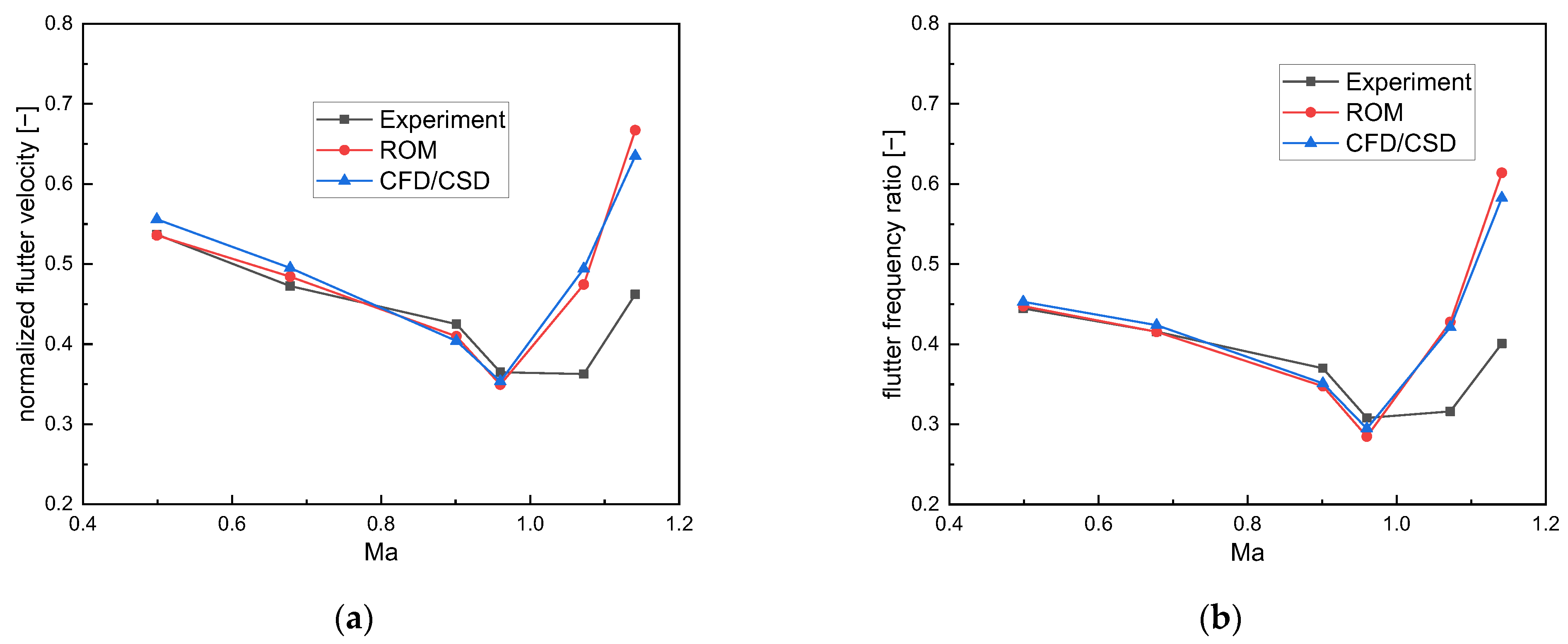
- Based on the Volterra series theory, an unsteady aerodynamic reduced–order model (ROM) is established, which ensures the flow field nonlinearity and reduces computational time. Coupled with the structural dynamics model, the aeroelastic model of the flying wing SensorCraft is obtained. The aeroelastic model of the AGARD445.6 wing verifies the accuracy and ef–ficiency of the aerodynamic reduced–order method.
- (2)
- The wing–conformal antenna array is designed. A method for calculating the far–field pattern of the antenna array based on mode superposition is proposed, which enables fast and quantitative analysis of the electromagnetic performance of antenna arrays under dynamic loads.
- (3)
- The passive wingtip device is designed, and the GLA control method is proposed by combining the LQG/LTR active controller with the passive wing–tip. Compared with the LQG/LTR active GLA method, the influence of the active–passiveactive–passive combined GLA method on the aircraft platform and the conformal antenna is analyzed in detail.
2. Physical Model
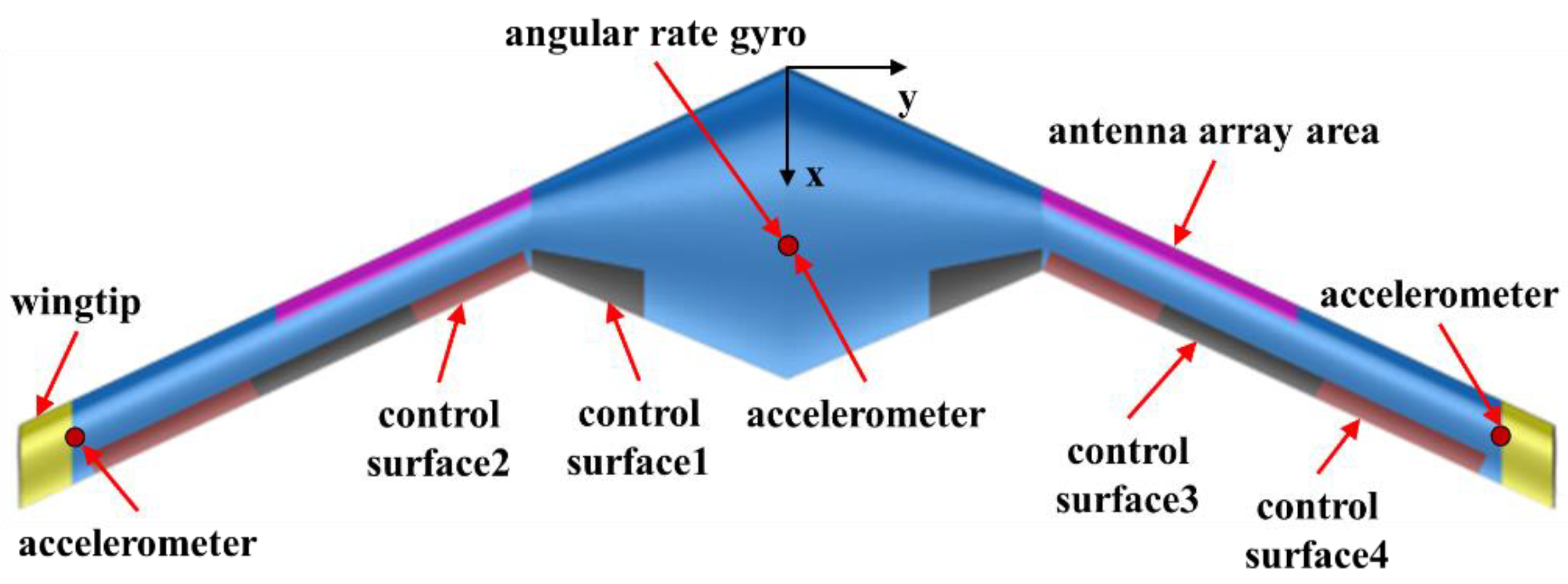
2.1. Flying Wing SensorCraft Model
2.2. Wing–Conformal Antenna Array Model
2.3. Passive Wingtip Device Model
3. Calculation Method
3.1. Reduced–Order Aerodynamic Model Based on the Volterra Series
3.2. Structural Dynamics Model with the Passive Wingtip Device
3.3. Aeroelastic Coupled Model
3.4. Flight Dynamics Model
3.5. Actuator Model
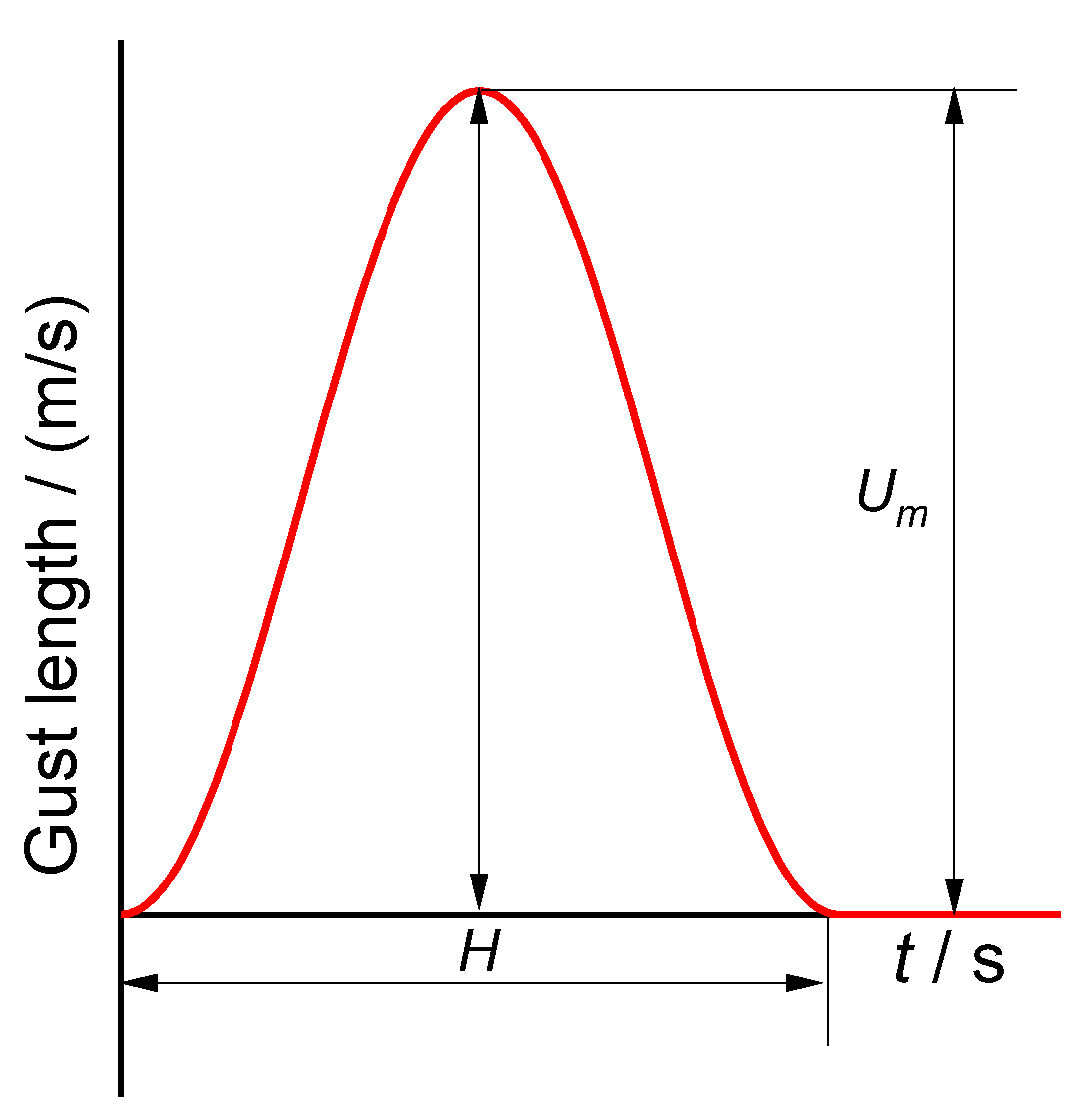
3.6. Discrete Gust Model
3.7. Gust Response Model
3.8. Fast Method of Antenna Array Pattern Based on Modal Superposition
- (1)
- According to the modal method, the node displacement vector of the antenna carrier under dynamic load was obtained, and each array element’s distribution position and deflection angle were calculated. could be expressed as the linear combination of each order vibration mode of the antenna carrier.where is the vibration mode matrix of all nodes of the antenna carrier, and x is the generalized displacement of the mode obtained from Equation (10), which represents the weight of each order vibration mode in the structural deformation .
- (2)
- The pattern of each array element was rotated according to its deflection angle.
- (3)
- The phase difference of each array element was calculated according to its distribution position.
- (4)
- Each array element’s pattern was superimposed to obtain the antenna array’s pattern.
4. Design of the Active Control System
4.1. Active Control Scheme
4.2. LQG/LTR Control Method
- (1)
- Solving the Riccati equation to determine the observer gain Kf.
- (2)
- Designing the optimal control gain Kc and selecting the appropriate weighting matrices Q and R cause the system to approach the open–loop gain of the Kalman filter observer as closely as possible.
5. Verification of the Reduced–Order Aerodynamic Method
6. Analysis of the Active–Passive Combined Gust Load Alleviation
6.1. Structural Modal of the SensorCraft
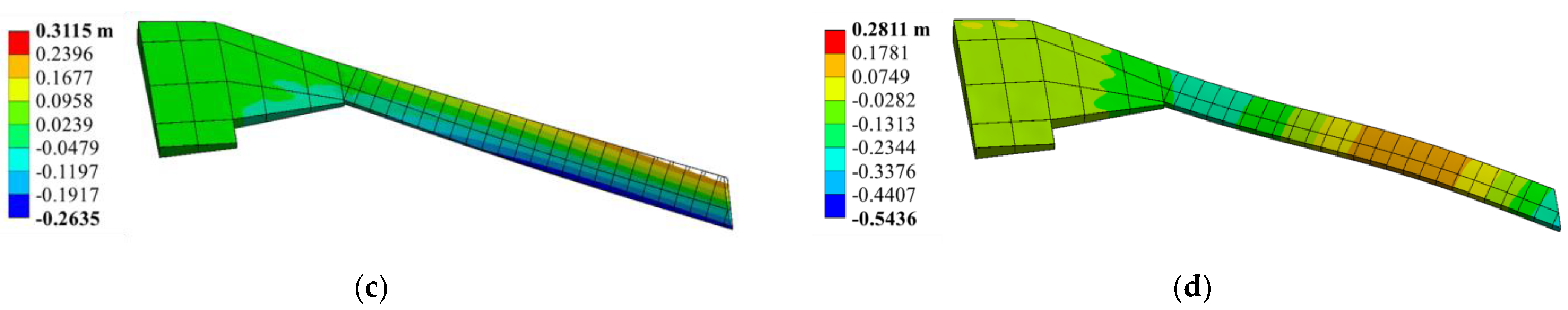
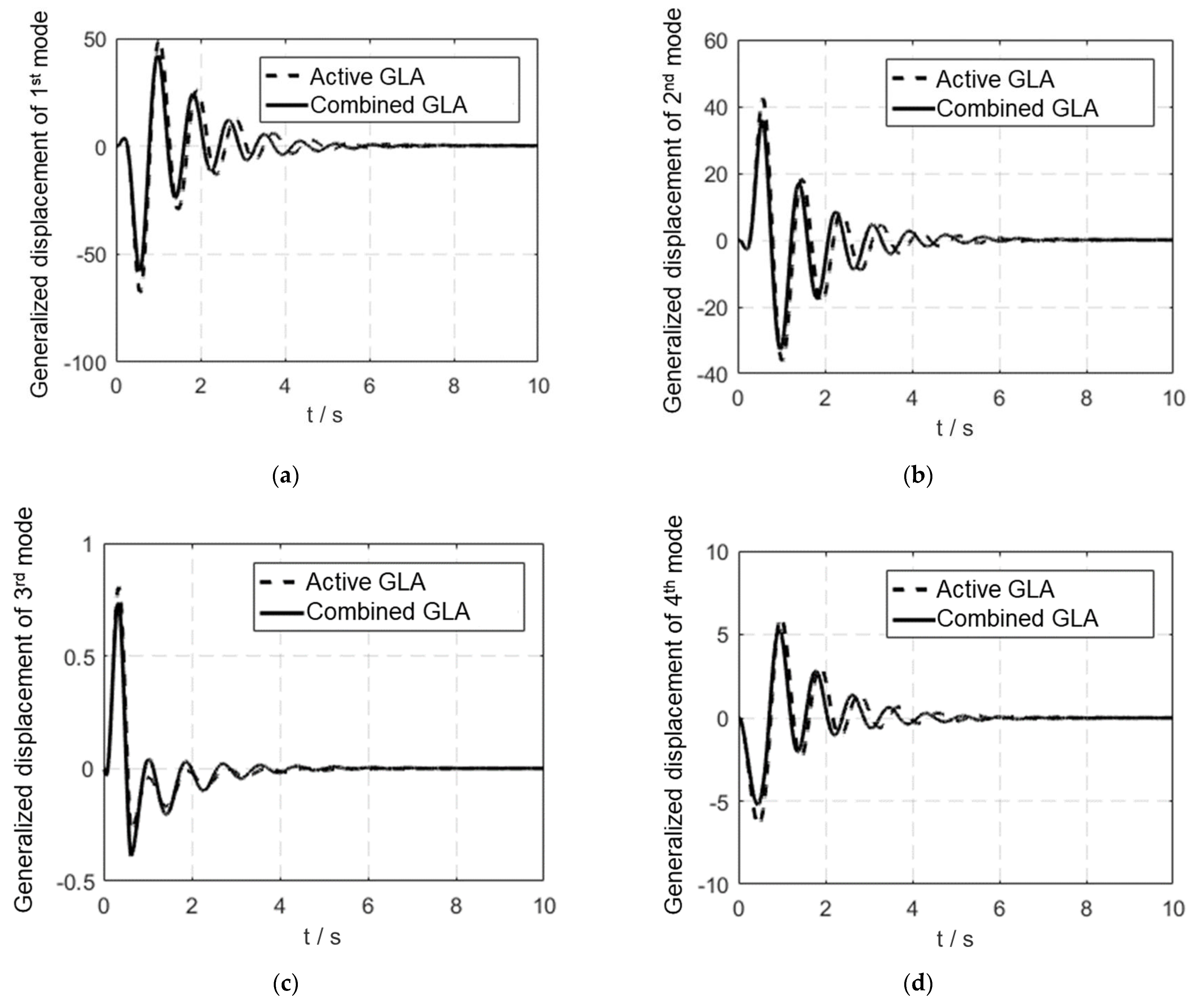
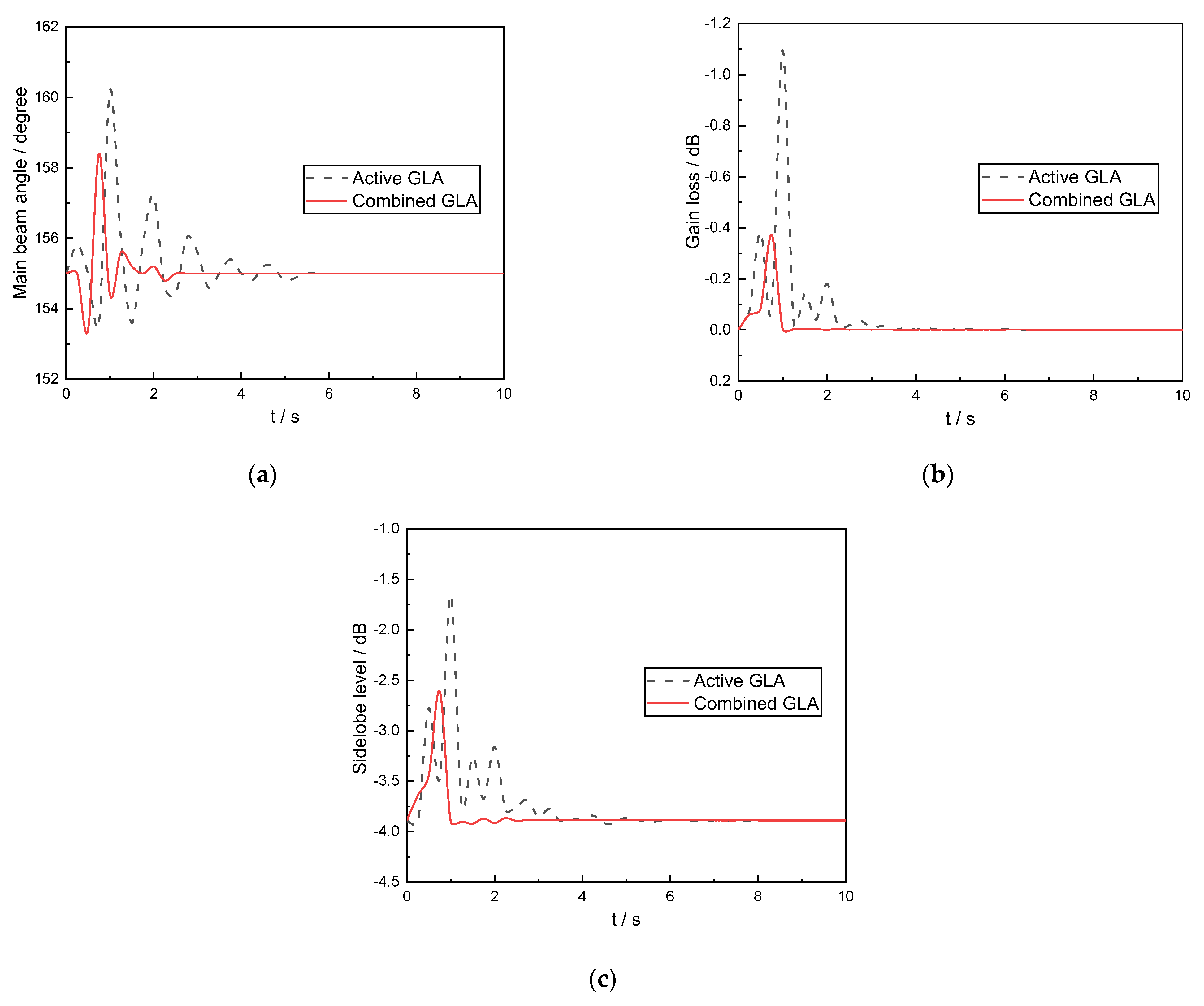
6.2. The Effects of the Active–Passive Combined Method on the Flight Platform
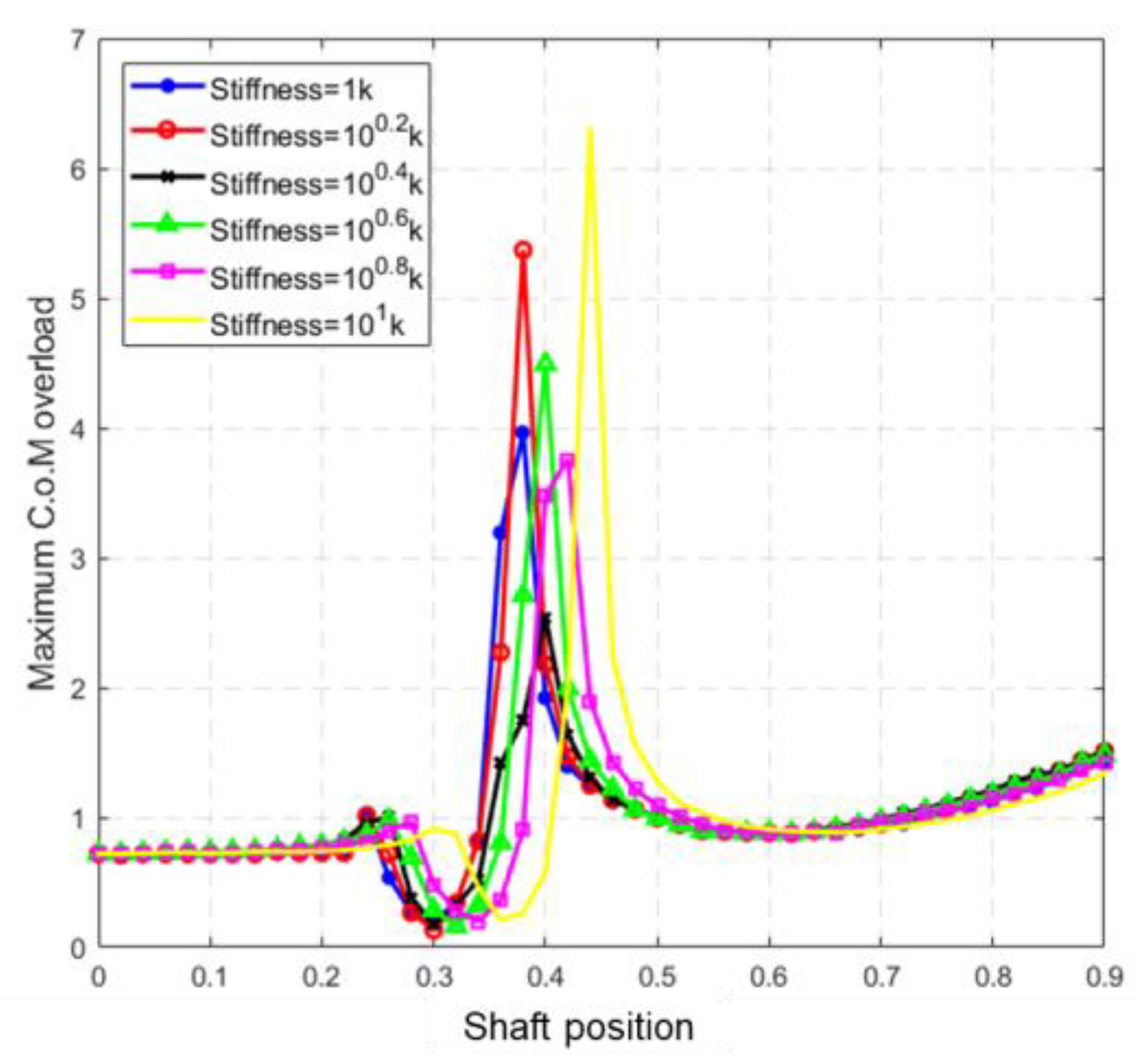
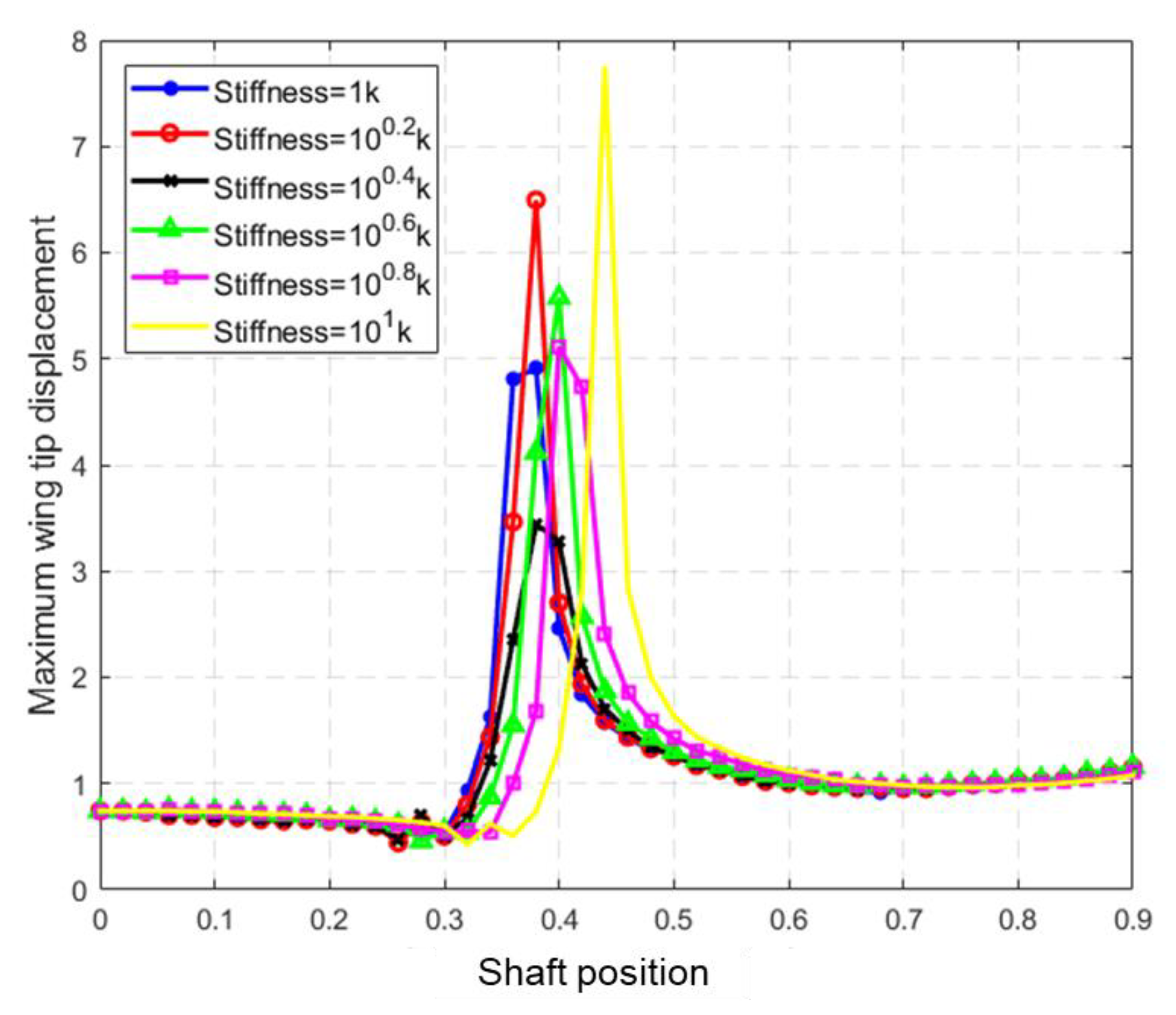
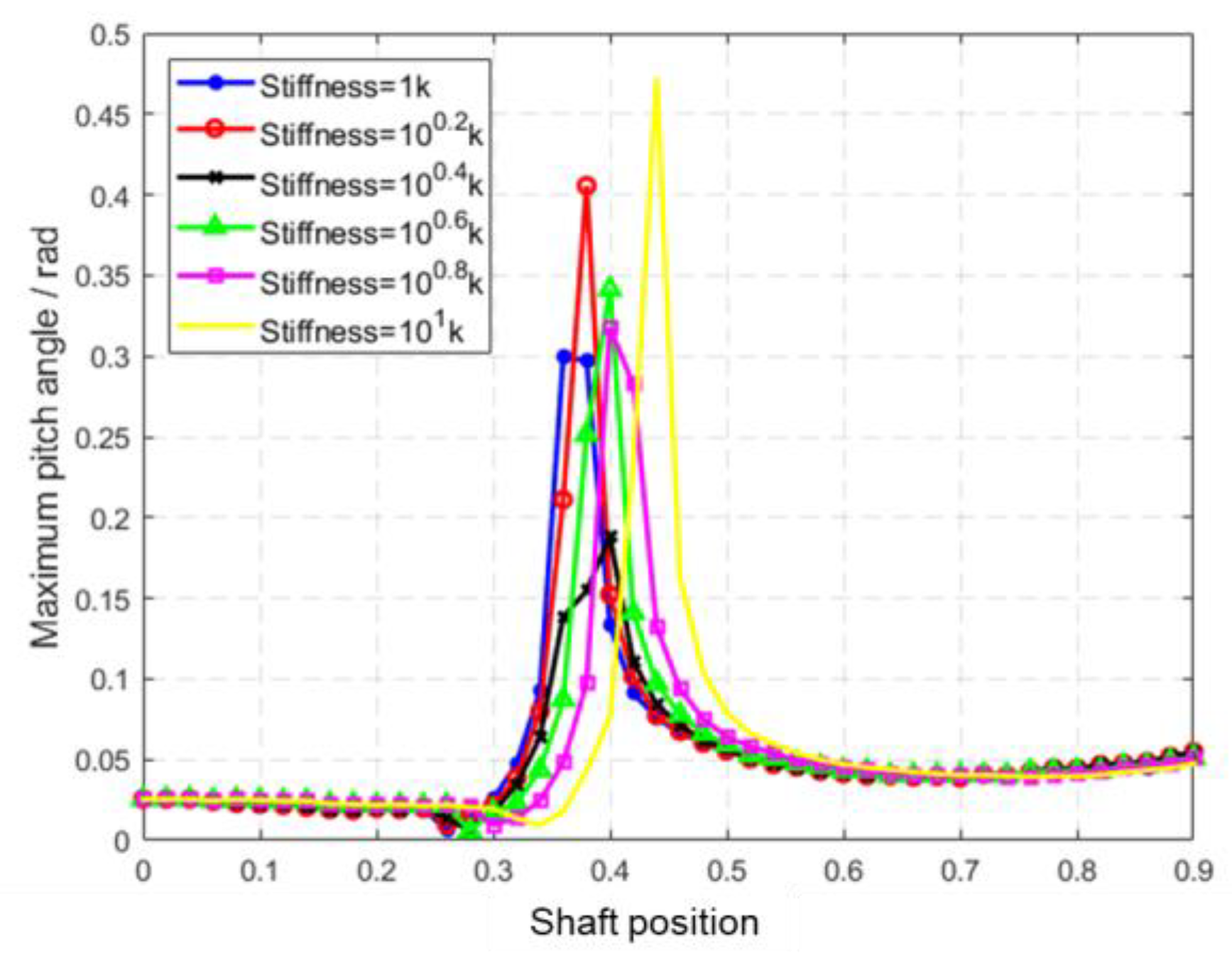
6.3. Influences of the Passive Wingtip Parameters on Gust Response
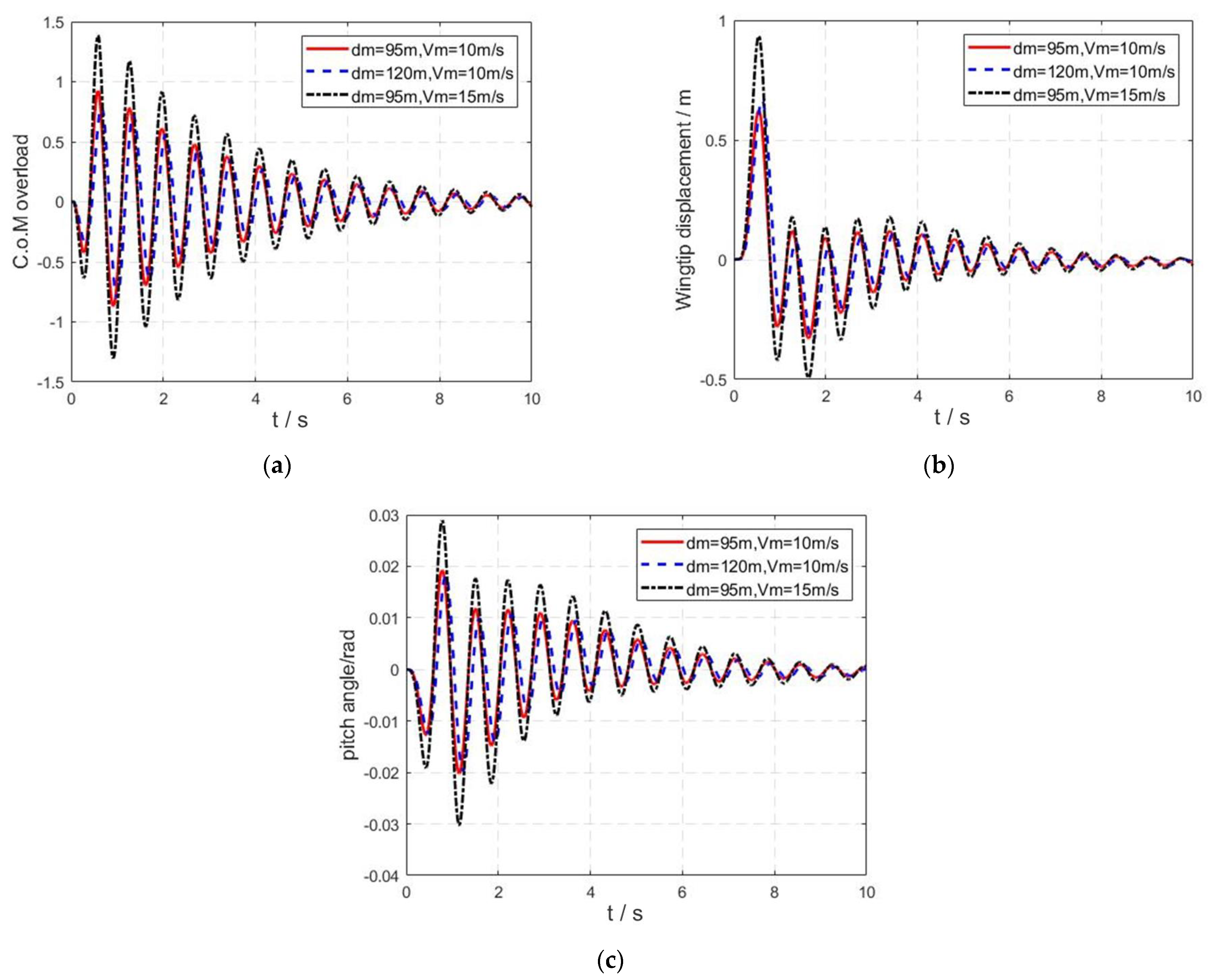
6.4. Gust Responses under Different Gust Conditions
7. Impact of Wing Deformation on the Electromagnetic Performance of the Antenna Array
7.1. The Impact of Static Deformation
7.2. The Impact of Dynamic Deformation
8. Conclusions
- (1)
- The unsteady aerodynamic reduced–order model based on the Volterra series is effective for predicting the modal response of the system, and can be used for rapid analysis of the aeroelastic response of the SensorCraft platform under gust. The far–field pattern method based on modal superposition can be used to rapidly evaluate the electromagnetic performance of conformal arrays under dynamic loads.
- (2)
- Compared with the LQG/LTR active GLA method, the active–passive combined GLA method of “LQG/LTR active controller + passive wingtip” can significantly reduce the peak response of wingtip displacement and pitch angle. The peak response of the inner control surface decreases, and the outer control surface increases under the active–passive combined GLA method. The reason for this is that the outer control surface needs to control both the attitude angle and wingtip displacement, causing a large surface deflection.
- (3)
- As the shaft position of the passive wing moves backward, the peak value of gravity overload reaches the minimum when the shaft position is at 0.3–0.4 c from the leading edge, which is located behind the aerodynamic center, where the wingtip is easier to deflect. The peak values of the wingtip displacement and pitch angle reach the minimum when the shaft of the passive wingtip is located at 0.25–0.35 c from the leading edge. When using a passive wingtip for GLA, it is necessary to conduct a trade–off evaluation of various targets and reasonably define the position of the shaft.
- (4)
- With the increase in gust length, the peak values of gravity center overload, wingtip displacement, and pitch angle increase and decrease. The wingtip displacement peaks when the gust length is approximately 150 m, and the peak value of the pitch angle is achieved when the gust length is about 100 m.
- (5)
- The 1st–order deformation has little impact on the pattern. The effects of the 2nd–4th order deformation on the pattern are mainly reflected in the main beam angle and sidelobe level, and the main lobe gain exhibits little change.
Author Contributions
Funding
Conflicts of Interest
Correction Statement
References
- Cord, T.; Newbern, S. Unmanned air vehicles: New challenges in design. In Proceedings of the Aerospace Conference, Big Sky, MT, USA, 10–17 March 2001. [Google Scholar]
- Hall, J.K.; Clark, C.S. SensorCraft Mission Simulation Study; Air Force Research Laboratory, Wright–Patterson AFB OH Air Vehicles Directorate: Dayton, OH, USA, 2002. [Google Scholar]
- Genello, G.J.; Baldygo, W.J., Jr.; Callahan, M.J. Modeling and simulation for Sensor Craft multi–mission radar. In Proceedings of the Aerospace Conference, Big Sky, MT, USA, 10–17 March 2001. [Google Scholar]
- Smallwood, B.; Canfield, R.; Terzuoli, A. Structurally integrated antennas on a joined–wing aircraft. In Proceedings of the 44th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Norfolk, VA, USA, 7–13 April 2003. [Google Scholar]
- Vartio, E.; Shaw, E.; Vetter, T. Gust load alleviation flight control system design for a SensorCraft vehicle. In Proceedings of the 26th AIAA Applied Aerodynamics Conference, Honolulu, HI, USA, 18–21 August 2008. [Google Scholar]
- Yagil, L.; Raveh, D.E.; Idan, M. Elastic deformations control of highly flexible aircraft in trimmed flight and gust encounter. J. Aircr. 2017, 55, 829–840. [Google Scholar] [CrossRef]
- Fonte, F.; Toffol, F.; Ricci, S. Design of a wing tip device for active maneuver and gust load alleviation. In Proceedings of the 2018 AIAA/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Kissimmee, FL, USA, 8–12 January 2018. [Google Scholar]
- Castrichini, A.; Hodigere Siddaramaiah, V.; Calderon, D.E.; Cooper, J.E.; Wilson, T.; Lemmens, Y. Nonlinear folding wing tips for gust loads alleviation. J. Aircr. 2016, 53, 1391–1399. [Google Scholar] [CrossRef][Green Version]
- Perron, G.; Drela, M. Competition passive gust load alleviation through the bend–twist coupling of composite beams on typical commercial airplane wings. In Proceedings of the 54th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Boston, MA, USA, 8–11 April 2013. [Google Scholar]
- Cooper, J.E.; Miller, S.; Vio, G.A.; Sensburg, O. Optimization of a scaled SensorCraft model with passive gust alleviation. In Proceedings of the 12th AIAA/ISSMO Multidisciplinary Analysis & Optimization Conference, Victoria, TX, USA, 10–12 September 2008. [Google Scholar]
- Cooper, J.E. Structural Design and Analysis of an Aeroelastic Tailoring and Passive Load Alleviation Concept for A Sensor Craft; Manchester University: Manchester, UK; EOARD: London, UK, 2007. [Google Scholar]
- Cooper, J.E.; Chekkal, I.; Cheung, R.; Wales, C.; Allen, N.J.; Lawson, S.; Peace, A.J.; Cook, R.; Standen, P.; Hancock, S.D.; et al. Design of a morphing wingtip. J. Aircr. 2014, 52, 1394–1403. [Google Scholar] [CrossRef][Green Version]
- Guo, S.; Li, D.; Sensburg, O. Optimal design of a passive gust alleviation device for a flying wing aircraft. In Proceedings of the 12th AIAA Aviation Technology, Integration, and Operations (ATIO) Conference and 14th AIAA/ISSMO Multidisciplinary Analysis and Optimization Conference, Indianapolis, IN, USA, 17–19 September 2012. [Google Scholar]
- Guo, S.; Sensburg, O. Passive Gust Alleviation for a Flying Wing Aircraft; Cranfield University: Bedford, UK; AFRL: Dayton, OH, USA, 2013. [Google Scholar]
- Guo, S.; Sensburg, O. Wind Tunnel Model and Test to Evaluate the Effectiveness of a Passive Gust Alleviation Device for a Flying Wing Aircraft; Cranfield University: Bedford, UK; AFRL: Dayton, OH, USA, 2016. [Google Scholar]
- Roberts, R.W. Sensor–Craft Analytical Certification; Air Force Institute of Technology, Wright–Patterson AFB OH School of Engineering and Management: Dayton, OH, USA, 2003; pp. 22–36. [Google Scholar]
- Knott, P. Deformation and Vibration of Conformal Antenna Arrays and Compensation Techniques; Fgan–Fhr Research Institute for High Frequency Physics and Radar Techniques Wachtberg: Waterberg, Germany, 2006. [Google Scholar]
- Lucia, D. The SensorCraft configurations: A non–linear aeroservoelastic challenge for aviation. In Proceedings of the 46th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Materials Conference, Austin, TX, USA, 18–21 April 2005. [Google Scholar]
- Silva, W. Identification of nonlinear aeroelastic systems based on the Volterra theory: Progress and opportunities. Nonlinear Dyn. 2005, 39, 25–62. [Google Scholar] [CrossRef]
- Marzocca, P.; Librescu, L.; Silva, W. Nonlinear open/closed–loop aeroelastic analysis of airfoils via Volterra series. AIAA J. 2004, 42, 673–686. [Google Scholar] [CrossRef]
- Vartio, E.; Shimko, A.; Tilmann, C.; Flick, P. Structural modal control and gust load alleviation for a SensorCraft concept. In Proceedings of the 46th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Austin, TX, USA, 18–21 April 2005. [Google Scholar]
- Song, L.; Lu, S.; Han, C.; Zhou, J.; Huang, J.; Li, P.; Ghorbani, K. Efficient computation of real–time distorted conformal load–bearing antenna structure under dynamic mechanical load based on modal superposition. IEEE J. Multiscale Multiphys. Comput. Tech. 2018, 3, 246–254. [Google Scholar] [CrossRef]
- Gao, C.; Liu, X.; Zhang, W. On the dispersion mechanism of the flutter boundary of the AGARD 445.6 wing. AIAA J. 2021, 8, 2657–2669. [Google Scholar] [CrossRef]
- Yateo, E.C.J. AGARD Standard Aeroelastic Configurations for Dynamic Response Wing 445.6; NASA Langley Research Center: Hampton, VA, USA, 1988.
- Goura, G.; Badcock, K.J.; Woodgate, M.A.; Richards, B.E. Implicit Methods for the Time Marching Analysis of Flutter. Aeronaut. J. 2001, 105, 199–214. [Google Scholar] [CrossRef]










| Compressive Modulus (MPa) | Tensile Modulus (MPa) | Shear Modulus (MPa) |
| 22,000 | 3000 | 3000 |
| Compressive strength (MPa) | Tensile strength (MPa) | Shear strength (MPa) |
| 388 | 540 | 120 |
| Mode Shape | Frequency (Hz) |
|---|---|
| 1st bending mode | 0.763 |
| 2nd bending mode | 4.336 |
| 1st torsion mode | 8.502 |
| 3rd bending mode | 10.559 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hao, S.; Ma, T.; Wang, Y.; Li, H.; Zhao, S.; Tan, P. Gust Alleviation by Active–Passive Combined Control of the Flight Platform and Antenna Array for a Flying Wing SensorCraft
. Aerospace 2023, 10, 511. https://doi.org/10.3390/aerospace10060511Hao S, Ma T, Wang Y, Li H, Zhao S, Tan P. Gust Alleviation by Active–Passive Combined Control of the Flight Platform and Antenna Array for a Flying Wing SensorCraft
. Aerospace. 2023; 10(6):511. https://doi.org/10.3390/aerospace10060511 Chicago/Turabian StyleHao, Shuai, Tielin Ma, Yi Wang, Huadong Li, Shiwei Zhao, and Puxue Tan. 2023. "Gust Alleviation by Active–Passive Combined Control of the Flight Platform and Antenna Array for a Flying Wing SensorCraft
" Aerospace 10, no. 6: 511. https://doi.org/10.3390/aerospace10060511 APA StyleHao, S., Ma, T., Wang, Y., Li, H., Zhao, S., & Tan, P. (2023). Gust Alleviation by Active–Passive Combined Control of the Flight Platform and Antenna Array for a Flying Wing SensorCraft
. Aerospace, 10(6), 511. https://doi.org/10.3390/aerospace10060511




