Cross-Scale Light Absorption Properties of Surface Bionic Microstructures for Spacecraft Stealth
Abstract
:1. Introduction
2. Spacecraft Optical Stealth Characteristics Modeling
2.1. OCS-Based Spacecraft Brightness Magnitude Modeling
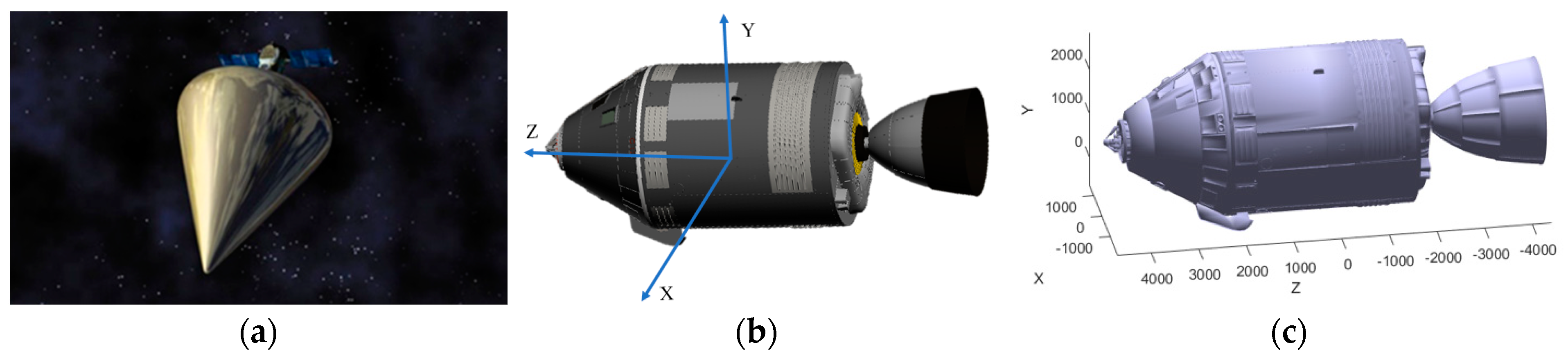
2.2. Spacecraft OCS Calculation Based on Z-Buffer Graphic Blanking
- (1)
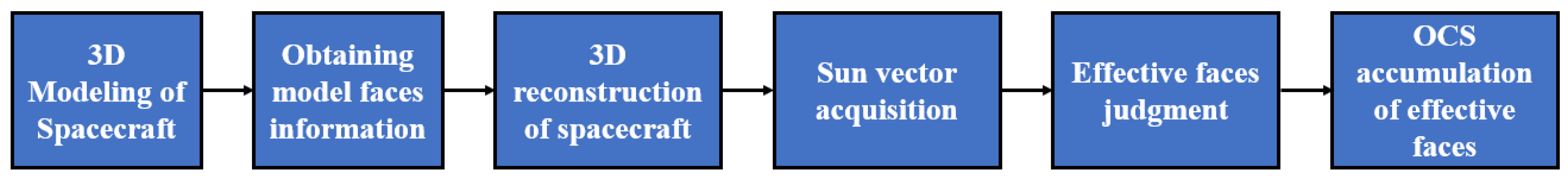
- Spacecraft surface element classification: First, a 3D model with spacecraft surface details and element division of the surface is obtained using 3dmax, the material information of each surface element is confirmed and then, the modified and perfect spacecraft model is exported in OBJ format.
- (2)
- Spacecraft OCS 3D reconstruction: A program is written to read the OBJ format file, which includes the numbers of all surface elements and the coordinates of the three corresponding vertices; the surface element index is adopted to reconstruct the spacecraft model.
- (3)
- Spacecraft sun vector acquisition: Through using the spacecraft orbit information, the solar vector information in the orbital system can be calculated. In this paper, the spacecraft is stable along three axes with respect to the ground, i.e., the coordinates of this system coincide with the coordinates of the orbital system, so the solar vector in the orbital system is also the solar vector in this system. If attitude maneuvers are available, then the orbital solar vector can be easily projected onto the present system by means of the attitude matrix.
- (4)
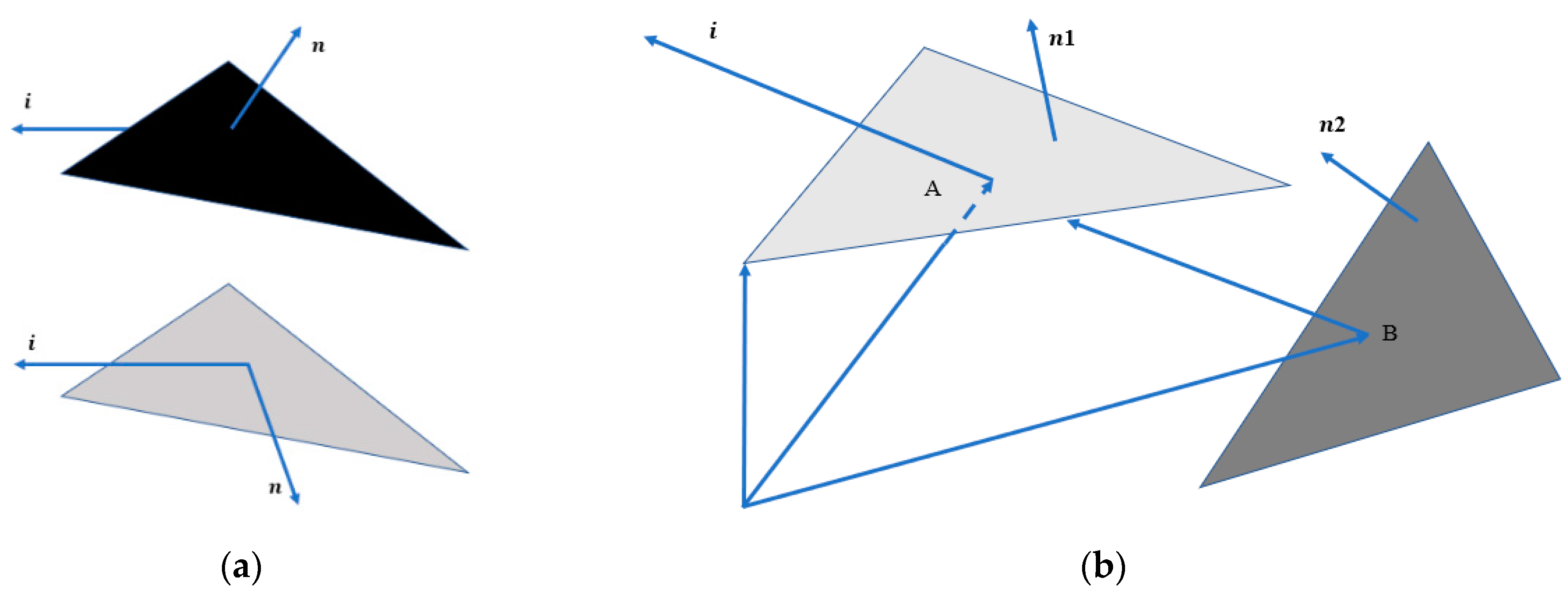
- Effective surface element determination: This is the core step of the spacecraft OCS calculation. Based on the occlusion situation analyzed earlier, the valid face elements are marked based on the vector algorithm and the Z-buffer algorithm, which perform self-occlusion and mutual occlusion checks of the face elements, respectively.
- (5)
- Whole-star OCS calculation: Combining the BRDF model with the light absorption characteristics of the surface microstructure, the OCS of each effective face element is calculated and the whole-star OCS is obtained via accumulation.
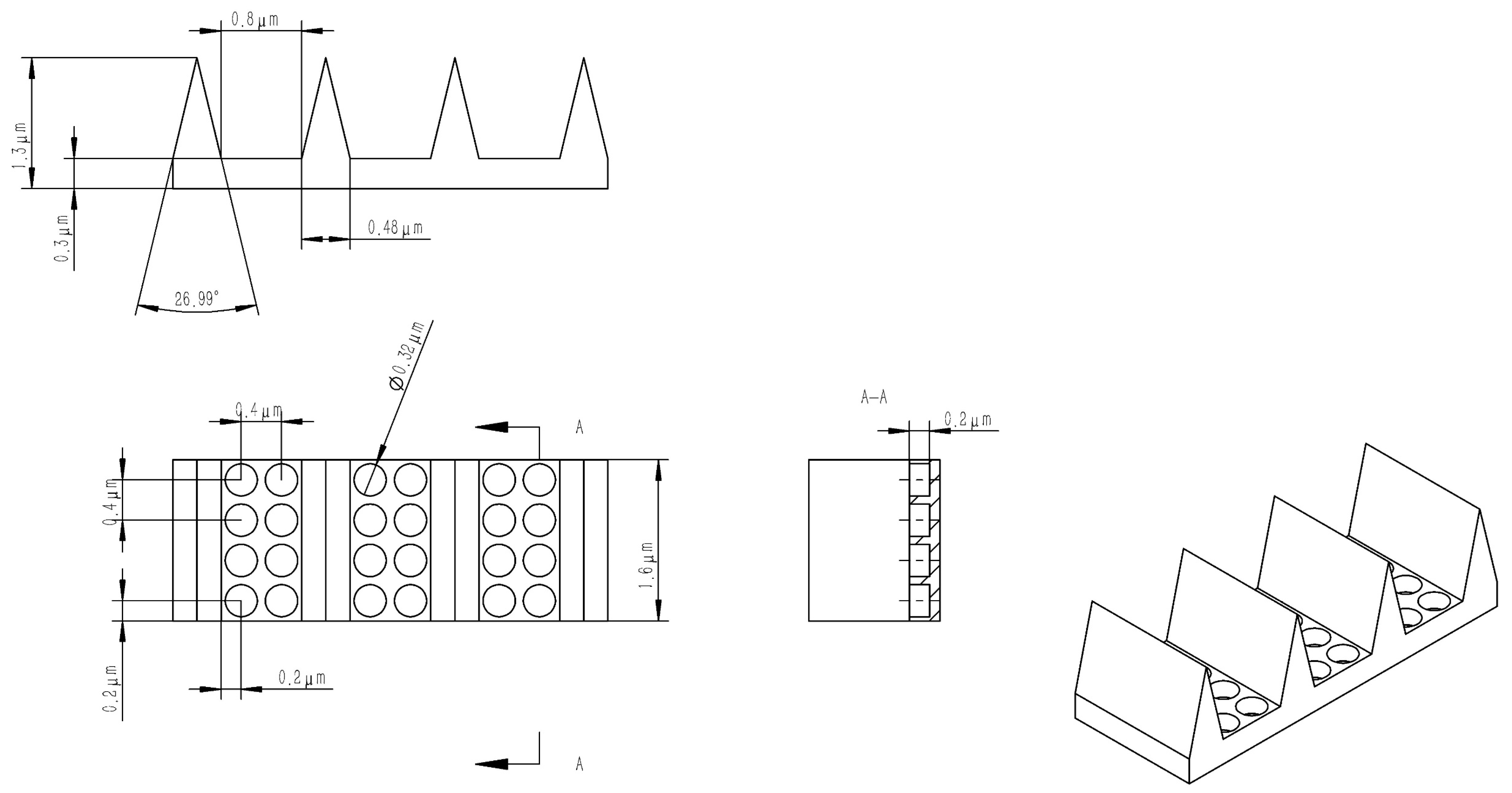
3. Optical Stealth Design Based on Bionic Microstructured Surfaces
- (6)
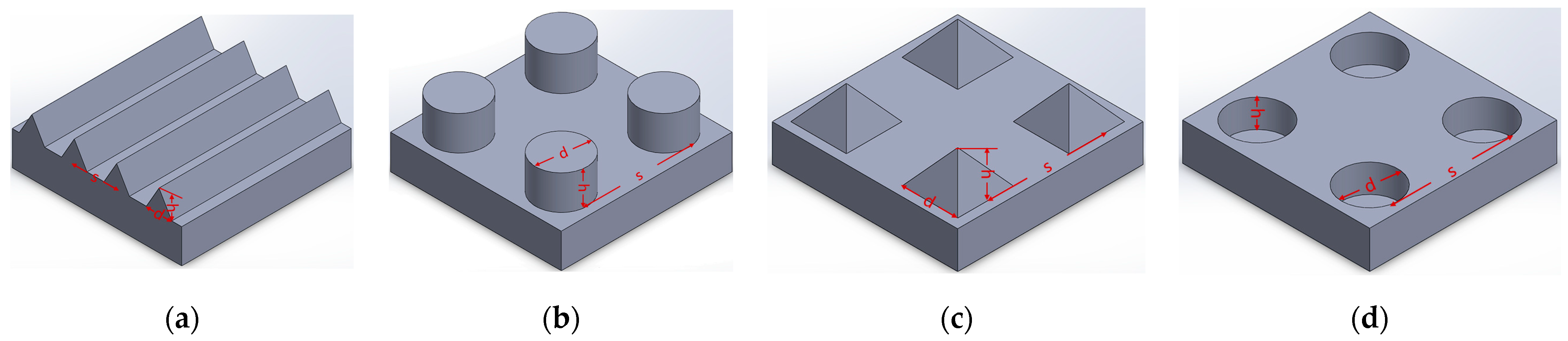
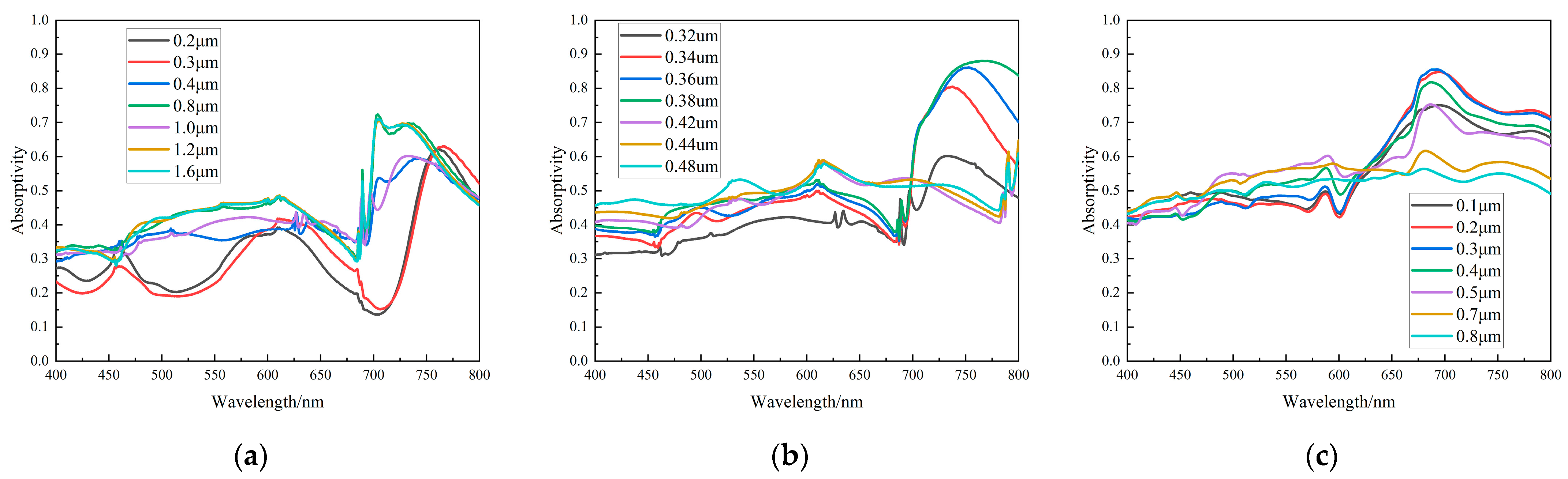
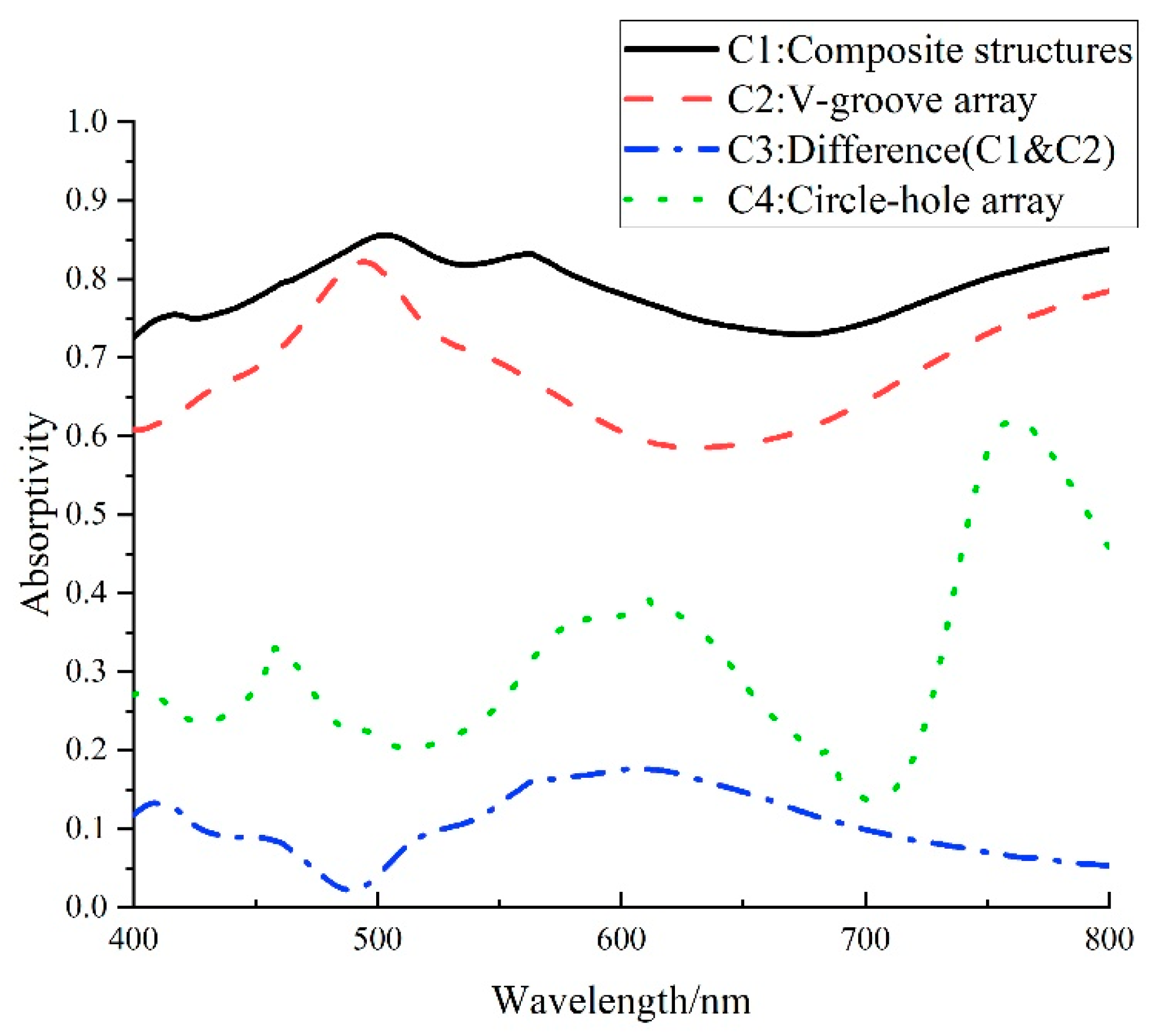
- The bionic microstructure has a good light absorption effect. Comparing the absorption parameters of the four configurations, it can be clearly seen that the V-groove array has the best light absorption effect. Figure 7a shows that the V-groove array can achieve more than 95% absorbance and achieve the stealth effect. The other microstructures can also achieve 80% absorbance through adjusting the parameters.
- (7)
- Microstructures with the same configuration can absorb specific wavelength bands through changing the structural parameters. Figure 7b shows that the V-groove array exhibits peak absorptivity for light in the 450–500 nm and 600 nm to 700 nm bands; Figure 10b shows that the hole array exhibits absorptivity peaks for light in the 700–800 nm band. That is, through changing the structural parameters, the surface of the object can show different colors.
- (8)
- Increasing the density of microstructures per unit area or increasing the height/depth of the structures can improve the light absorption properties. From Figure 7c, Figure 8c, Figure 9c and Figure 10c, it can be seen that when other scale factors are fixed, increasing the structural density can improve the absorption rate; Figure 7a, Figure 8a, Figure 9a and Figure 10a show that increasing the height or depth of the structure increases the light absorption rate.
4. Results and Discussion
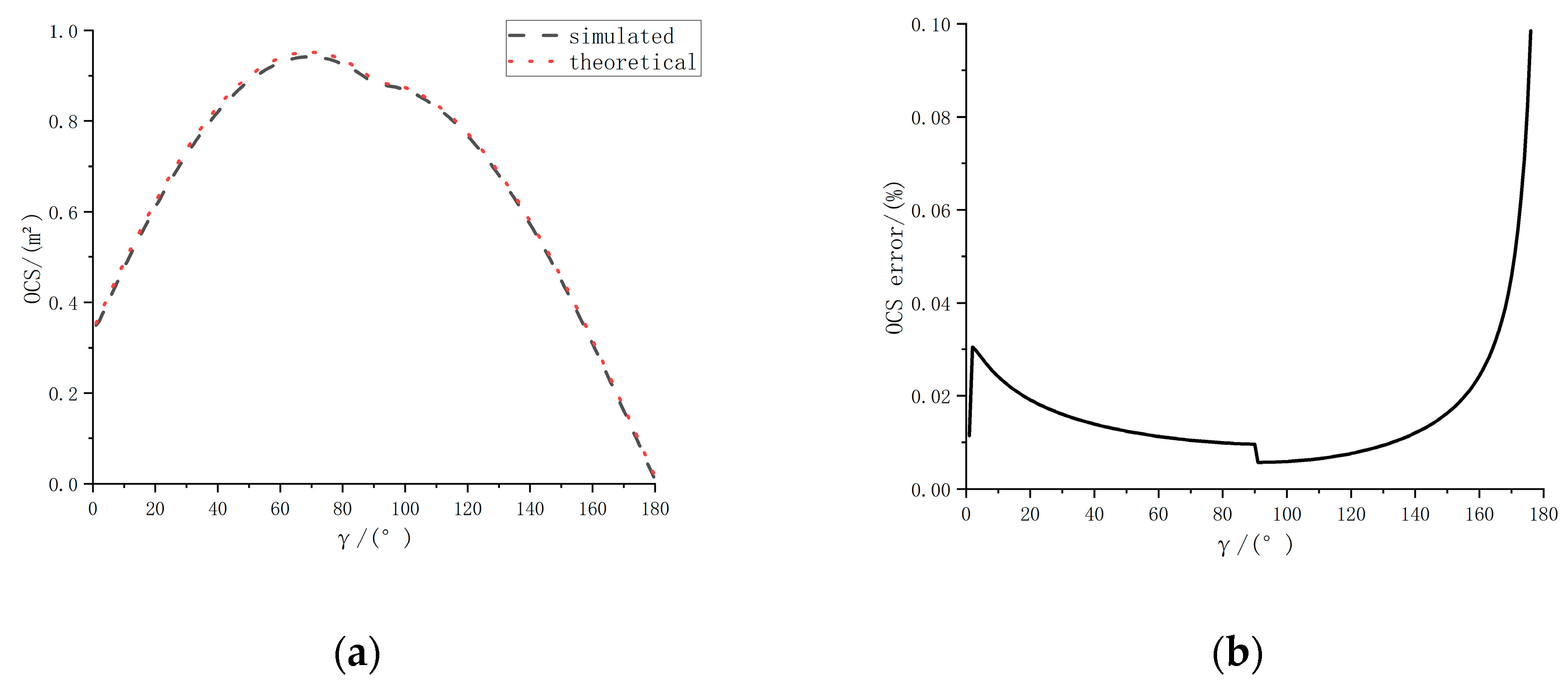
4.1. Verification of the OCS Algorithm Based on Graphic Blanking with a Standard Body
4.2. Analysis of the Optical Stealth Characteristics of Spacecraft Using Biomimetic Microstructured Surfaces
- (1)
- After the stealth structure covering process is performed on the main surface of the spacecraft, the stealth effect is obvious: the darkest magnitude before stealth treatment is 14.6, whereas the darkest magnitude after stealth treatment is 18.71.
- (2)
- The stealth treatment of the spacecraft does not change its brightness pattern: when the angle between the sun vector and the observation vector is within ±5°, the smaller the angle is, the higher the brightness; when the angle between the sun vector and the observation vector exceeds 5°, the larger the angle is, the lower the brightness; and when the sun vector coincides with the x-axis, the spacecraft has the lowest brightness and the largest magnitude.
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhu, D.; Zhang, Z.; Zhao, C.; Liu, Y. A satellite configuration design for optical stealth. Aerosp. Control Appl. 2017, 43, 61–66. [Google Scholar]
- Mehrabi, S.; Bilal, R.; Naveed, M.; Ali, M. Ultra-broadband nanostructured metamaterial absorber based on stacked square-layers of TiN/TiO2. Opt. Mater. Express 2022, 12, 2199–2211. [Google Scholar] [CrossRef]
- Naveed, M.; Bilal, R.; Baqir, M.; Bashir, M.; Ali, M.; Rahim, A. Ultrawideband fractal metamaterial absorber made of nickel operating in the UV to IR spectrum. Opt. Mater. Express 2021, 29, 42911–42923. [Google Scholar] [CrossRef]
- Liu, J.; Chen, W.; Zheng, J.-C.; Chen, Y.-S.; Yang, C.-F. Wide-Angle Polarization-Independent Ultra-Broadband Absorber from Visible to Infrared. Nanomaterials 2020, 10, 27. [Google Scholar] [CrossRef] [Green Version]
- Wang, K.; Wang, C.; Yin, Y.; Chen, K. Modification of Alpigment with graphene for infrared/visual stealth compatiblefabric coating. J. Alloys Compd. 2017, 690, 741–748. [Google Scholar] [CrossRef]
- Ding, F.; Mo, L.; Zhu, J.; He, S. Lithography-free, broadband, omnidirectional, and polarization-insensitive thinoptical absorber. Appl. Phys. Lett. 2015, 106, 061108. [Google Scholar] [CrossRef]
- Rana, A.S.; Mehmood, M.Q.; Jeong, H.; Kim, I.; Rho, J. Tungsten-based ultrathin absorber for visible regime. Sci. Rep. 2018, 8, 2443. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wu, D.; Liu, C.; Liu, Y.; Yu, L.; Yu, Z.; Chen, L.; Ma, R.; Ye, H. Numerical study of an ultra-broadband near-perfect solar absorber in the visible and near-infrared region. Opt. Lett. 2017, 42, 450–453. [Google Scholar] [CrossRef]
- Ghobadi, A.; Hajian, H.; Gokbayrak, M.; Dereshgi, S.A.; Toprak, A.; Butun, B.; Ozbay, E. Visiblelight nearly perfect absorber: An optimum unit cellarrangement for near absolute polarization insensitivity. Opt. Express 2017, 25, 27624–27634. [Google Scholar] [CrossRef]
- Ni, X.; Wong, Z.J.; Mrejen, M.; Wang, Y.; Zhang, X. An ultrathininvisibility skin cloak for visible light. Science 2015, 349, 1310–1314. [Google Scholar] [CrossRef]
- Hsu, L.; Ndao, A.; Kanté, B. Broadband and linearpolarization metasurface carpet cloak in the visible. Opt. Lett. 2019, 44, 2978–2981. [Google Scholar] [CrossRef] [PubMed] [Green Version]
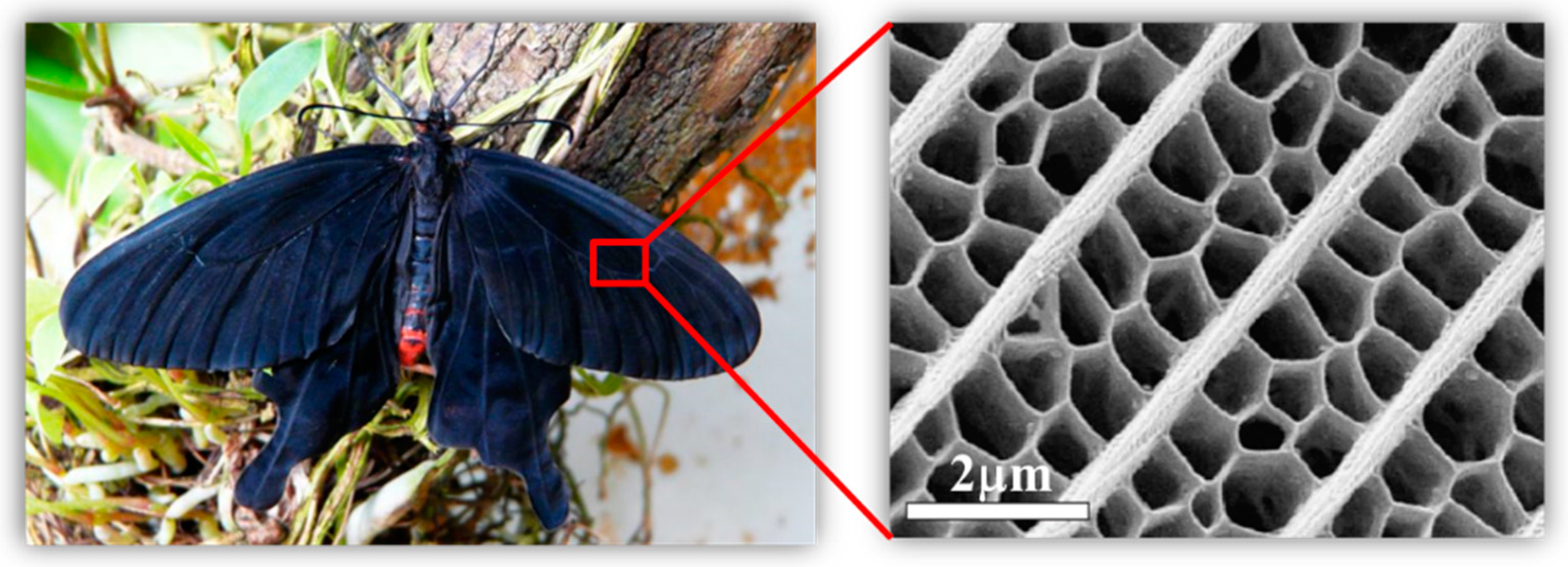
- Fang, J.; Gu, J.; Liu, Q.; Zhang, W.; Su, H.; Zhang, D. Three-dimensional CdS/Au butterfly wing scales with hierarchical rib structures for plasmon-enhanced photocatalytic hydrogen production. ACS Appl. Mater. Interfaces 2018, 10, 19649–19655. [Google Scholar] [CrossRef] [PubMed]
- Can, X.; Yasheng, Z.; Peng, L. Calculation of optical cross section areas of spatial objects based on OpenGL picking technique. Acta Opt. Sin. 2017, 37, 218–227. [Google Scholar]
- Wang, L.; Wu, J.; Yang, X.; Popescu, V. VR Exploration Assistance through Automatic Occlusion Removal. IEEE Trans. Vis. Comput. Graph. 2019, 25, 2083–2092. [Google Scholar] [CrossRef]
- Zhu, S.; Zhang, D.; Chen, Z.; Gu, J.; Li, W.; Jiang, H.; Zhou, G. A simple and effective approach towards biomimetic replication of photonic structures from butterfly wings. Nanotechnology 2009, 20, 315303. [Google Scholar] [CrossRef]
- Wang, W.; Zhang, W.; Fang, X.; Huang, Y.; Liu, Q.; Gu, J.; Zhang, D. Demonstration of higher colour response with ambient refractive index in Papilio blumei as compared to Morpho rhetenor. Sci. Rep. 2014, 4, 5591. [Google Scholar] [CrossRef] [Green Version]
- Liu, H.; Zhao, Q.; Zhou, H.; Ding, J.; Zhang, D.; Zhu, H.; Fan, T. Hydrogen evolution via sunlight water splitting on an artificial butterfly wing architecture. Phys. Chem. Chem. Phys. 2011, 13, 10872–10876. [Google Scholar] [CrossRef]
- Tan, Y.; Gu, J.; Zang, X.; Xu, W.; Shi, K.; Xu, L.; Zhang, D. Versatile fabrication of intact three-dimensional metallic butterfly wing scales with hierarchical sub-micrometer structures. Angew. Chem. Int. Ed. 2011, 50, 8307–8311. [Google Scholar] [CrossRef] [PubMed]
- Tan, Y.; Gu, J.; Xu, W.; Chen, Z.; Liu, D.; Liu, Q.; Zhang, D. Reduction of CuO butterfly wing scales generates Cu SERS substrates for DNA base detection. ACS Appl. Mater. Interfaces 2013, 5, 9878–9882. [Google Scholar] [CrossRef]
- Guan, Y.; Su, H.; Yang, C.; Wu, L.; Chen, S.; Gu, J.; Zhang, W.; Zhang, D. Ordering of hollow Ag-Au nanospheres with butterfly wings as a bio-template. Sci. Rep. 2018, 8, 9261. [Google Scholar] [CrossRef]
- Ahmad, Q.A.; Ehsan, M.I.; Khan, N.; Majeed, A.; Zeeshan, A.; Ahmad, R.; Noori, F.M. Numerical simulation and modeling of a poroelastic media for detection and discrimination of geo-fluids using finite difference method. Alex. Eng. J. 2022, 61, 3447–3462. [Google Scholar] [CrossRef]
- Huang, H. Concept study on satellite stealth. Aerosp. Electron. Warf. 2010, 6. [Google Scholar]







| Material | ρd | ρs | α | a | b |
|---|---|---|---|---|---|
| GaAs | 0.0428 | 23.0067 | 2122.5000 | 2.1124 | 4.8569 |
| Al | 0.0774 | 8.7556 | 389.9936 | 1.6878 | 4.4520 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiu, Y.; Kang, G.; Cheng, X.; Wu, J. Cross-Scale Light Absorption Properties of Surface Bionic Microstructures for Spacecraft Stealth. Aerospace 2023, 10, 561. https://doi.org/10.3390/aerospace10060561
Qiu Y, Kang G, Cheng X, Wu J. Cross-Scale Light Absorption Properties of Surface Bionic Microstructures for Spacecraft Stealth. Aerospace. 2023; 10(6):561. https://doi.org/10.3390/aerospace10060561
Chicago/Turabian StyleQiu, Yuhuan, Guohua Kang, Xunlong Cheng, and Jiaqi Wu. 2023. "Cross-Scale Light Absorption Properties of Surface Bionic Microstructures for Spacecraft Stealth" Aerospace 10, no. 6: 561. https://doi.org/10.3390/aerospace10060561
APA StyleQiu, Y., Kang, G., Cheng, X., & Wu, J. (2023). Cross-Scale Light Absorption Properties of Surface Bionic Microstructures for Spacecraft Stealth. Aerospace, 10(6), 561. https://doi.org/10.3390/aerospace10060561


_Zhu.png)



