A Methodology for Allocating Incremental Resources in Single-Airport Time Slots
Abstract
:1. Introduction
2. Literature Review
3. Single Airport Slot Incremental Resource Allocation Model
3.1. Notion
- A flight time is not a free point in time, but a specific time slot usually of 5 min. Multiple take-off or landing flights can be scheduled within a single time slot [9].
- Aircraft flight times and taxi times within the terminal area are derived from historical data of actual operations and do not take into account aircraft taxiing conflicts or conflicts within the terminal area airspace.
- The time of day allocated to each flight is the slot time, for take-off flights it is the withdrawal block time and for landing flights it is the upper block time.
- The time of an existing flight assignment cannot be adjusted.
3.2. Resource Model
3.2.1. Time Resources
3.2.2. Runway Resource
3.2.3. Corridor Resources
3.2.4. Parking Position Resources
3.3. Task Model
3.3.1. Historical Flight Assignments
3.3.2. New Flight Assignment
3.4. Binding Condition
3.4.1. Time Uniqueness Constraint
3.4.2. Entry and Exit Balance Constraints
3.4.3. Constant Coordination of Parameter Constraints
3.4.4. Runway Operating Capacity Constraints
3.4.5. Corridor Entrance Capacity Constraints
3.4.6. Flight Waveforms Constraints
3.4.7. Total Daily Flight Slots Constraints
3.5. Objective Function
3.6. Solution Algorithm
4. Efficiency Evaluation Indicators
4.1. Flight Delay
4.2. Capacity Fulfillment of Key Resource Points
5. Simulation and Validation of a Single-Airport Slot Increment Model
5.1. Experimental Data
5.2. Computer Simulation and Validation
5.2.1. Arithmetic Example Based on a Single-Airport Slot Incremental Model
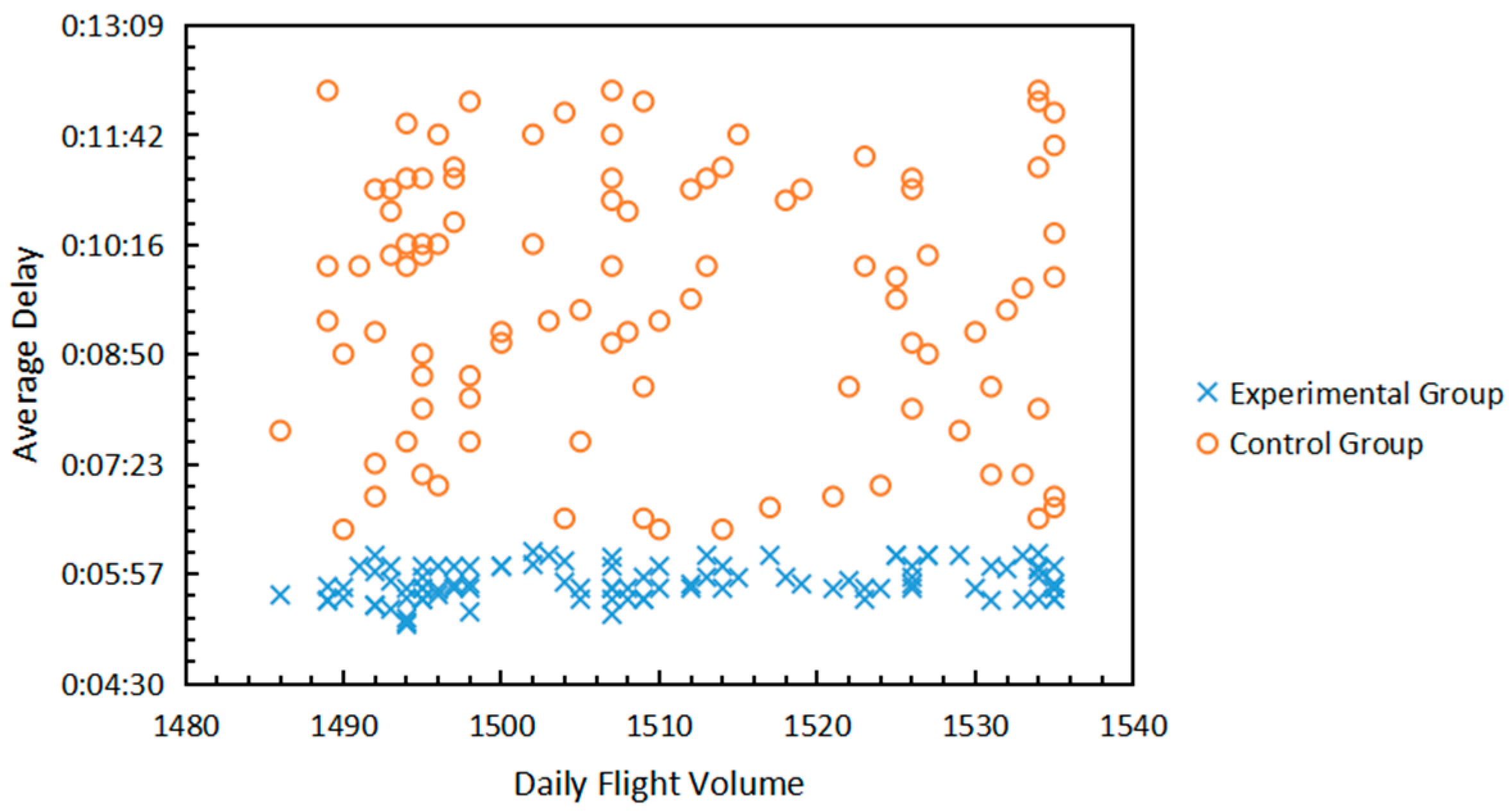
5.2.2. Monte Carlo Simulation Based Validation Algorithms
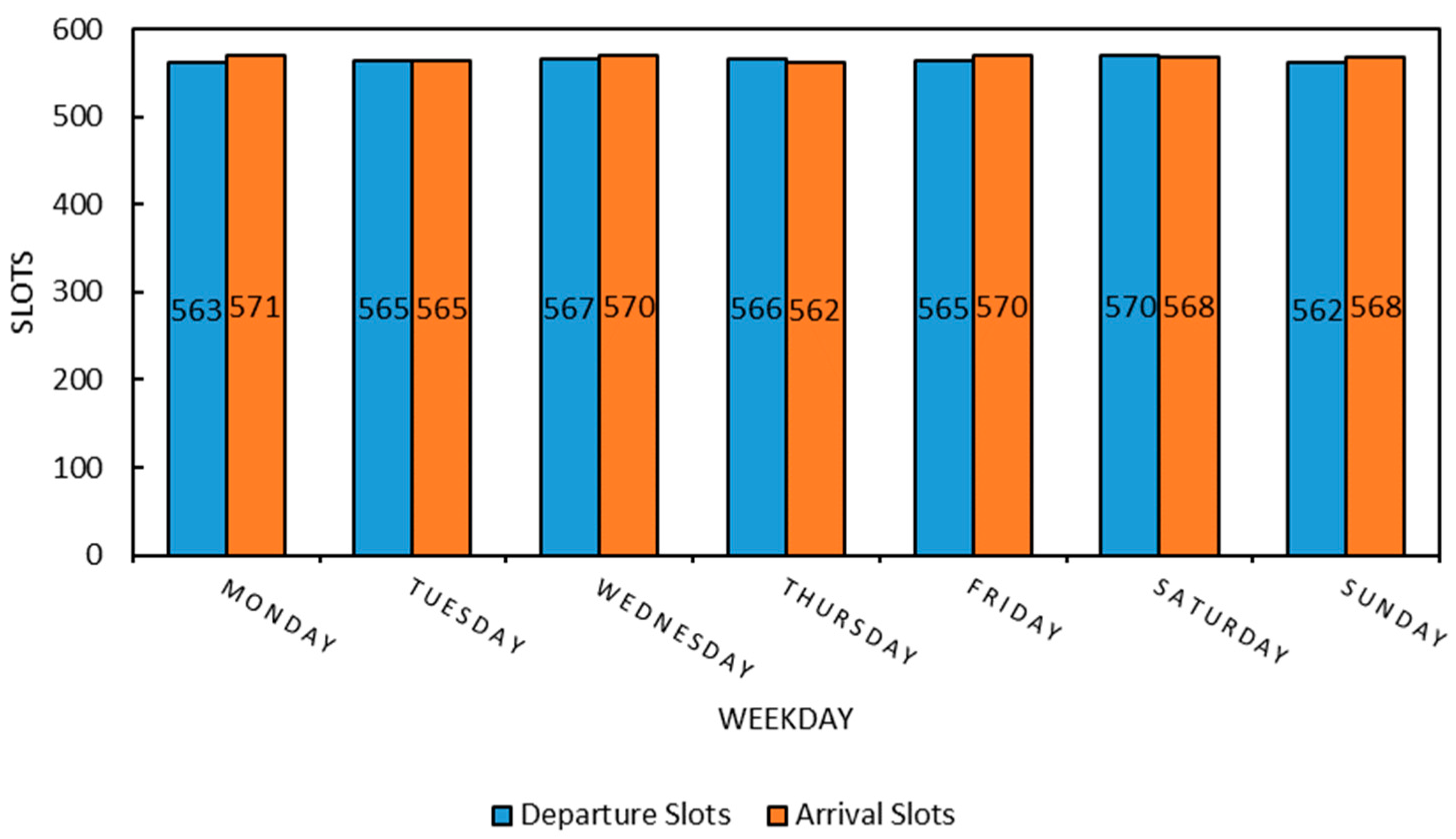
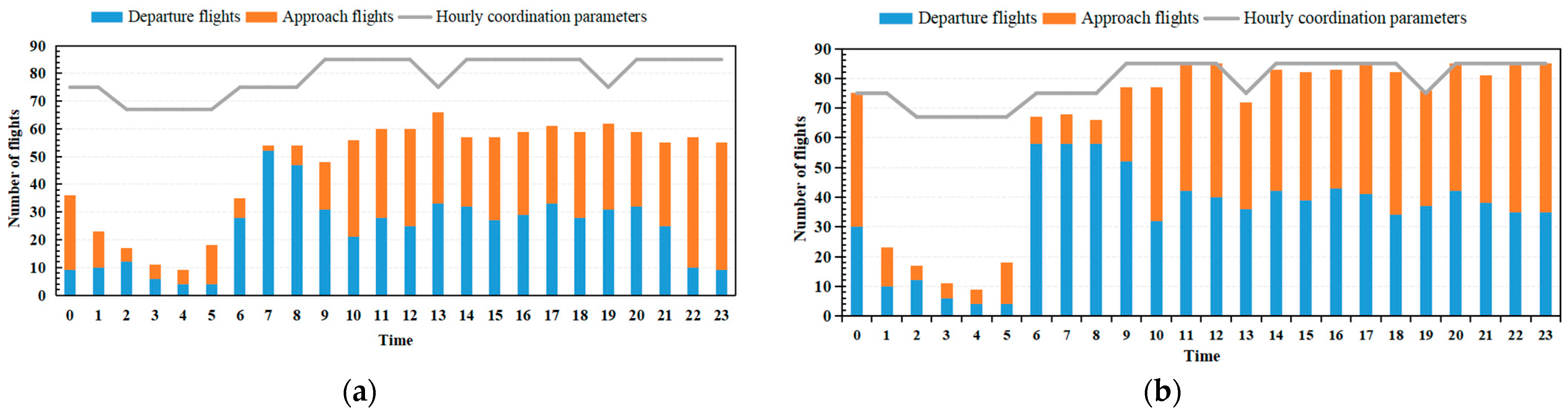
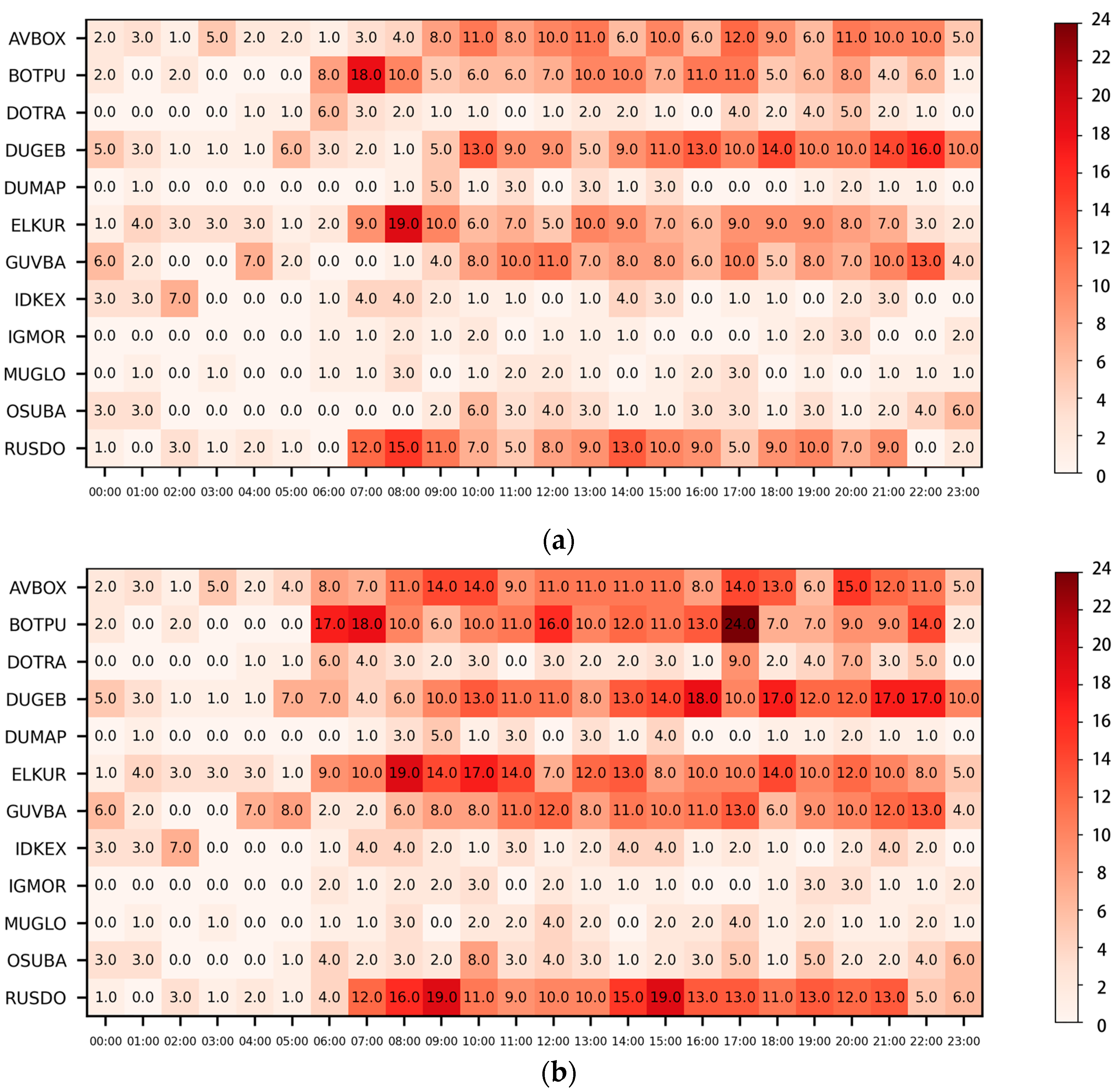
5.3. Results Analysis
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- International Air Transport Association. Worldwide Slot Guidelines, 2022 ed.; International Air Transport Association: Geneva, Switzerland, 2022. [Google Scholar]
- Zografos, K.G.; Madas, M.A.; Androutsopoulos, K.N. Increasing Airport Capacity Utilisation through Optimum Slot Scheduling: Review of Current Developments and Identification of Future Needs. J. Sched. 2017, 20, 3–24. [Google Scholar] [CrossRef]
- Abeyratne, R.I.R. Management of Airport Congestion through Slot Allocation. J. Air Transp. Manag. 2000, 6, 29–41. [Google Scholar] [CrossRef]
- Wang, S.; Hu, M.; Zhao, Z.; Shu, J.; Zhu, X. Research on Flight Schedule Optimization Based on Different Runway Operation Modes. J. Phys. Conf. Ser. 2023, 2491, 012001. [Google Scholar] [CrossRef]
- Odoni, A.R. The Flow Management Problem in Air Traffic Control. In Flow Control of Congested Networks; Odoni, A.R., Bianco, L., Szegö, G., Eds.; Springer: Berlin/Heidelberg, Germany, 1987; pp. 269–288. ISBN 978-3-642-86728-6. [Google Scholar]
- Pellegrini, P.; Castelli, L.; Pesenti, R. Secondary Trading of Airport Slots as a Combinatorial Exchange. Transp. Res. Part E Logist. Transp. Rev. 2012, 48, 1009–1022. [Google Scholar] [CrossRef]
- Zografos, K.G.; Salouras, Y.; Madas, M.A. Dealing with the Efficient Allocation of Scarce Resources at Congested Airports. Transp. Res. Part C Emerg. Technol. 2012, 21, 244–256. [Google Scholar] [CrossRef]
- Corolli, L.; Lulli, G.; Ntaimo, L. The Time Slot Allocation Problem under Uncertain Capacity. Transp. Res. Part C Emerg. Technol. 2014, 46, 16–29. [Google Scholar] [CrossRef]
- Pyrgiotis, N.; Odoni, A. On the Impact of Scheduling Limits: A Case Study at Newark Liberty International Airport. Transp. Sci. 2016, 50, 150–165. [Google Scholar] [CrossRef]
- Ribeiro, N.A.; Jacquillat, A.; Antunes, A.P.; Odoni, A.R.; Pita, J.P. An Optimization Approach for Airport Slot Allocation under IATA Guidelines. Transp. Res. Part B Methodol. 2018, 112, 132–156. [Google Scholar] [CrossRef]
- Androutsopoulos, K.N.; Manousakis, E.G.; Madas, M.A. Modeling and Solving a Bi-Objective Airport Slot Scheduling Problem. Eur. J. Oper. Res. 2020, 284, 135–151. [Google Scholar] [CrossRef]
- Zografos, K.G.; Jiang, Y. A Bi-Objective Efficiency-Fairness Model for Scheduling Slots at Congested Airports. Transp. Res. Part C Emerg. Technol. 2019, 102, 336–350. [Google Scholar] [CrossRef]
- Katsigiannis, F.A.; Zografos, K.G.; Fairbrother, J. Modelling and Solving the Airport Slot-Scheduling Problem with Multi-Objective, Multi-Level Considerations. Transp. Res. Part C Emerg. Technol. 2021, 124, 102914. [Google Scholar] [CrossRef]
- Fairbrother, J.; Zografos, K.G. On the Development of A Fair And Efficient Slot Scheduling Mechanism at Congested Airports. In Proceedings of the 2018 Annual Meeting of the Transportation Research Board (TRB), Washington, DC, USA, 7–11 January 2018. [Google Scholar]
- Jiang, Y.; Zografos, K.G. A Decision Making Framework for Incorporating Fairness in Allocating Slots at Capacity-Constrained Airports. Transp. Res. Part C Emerg. Technol. 2021, 126, 103039. [Google Scholar] [CrossRef]
- Jacquillat, A.; Vaze, V. Interairline Equity in Airport Scheduling Interventions. Transp. Sci. 2018, 52, 941–964. [Google Scholar] [CrossRef]
- Jacquillat, A.; Odoni, A.R. An Integrated Scheduling and Operations Approach to Airport Congestion Mitigation. Oper. Res. 2015, 63, 1390–1410. [Google Scholar] [CrossRef]
- Fairbrother, J.; Zografos, K.G.; Glazebrook, K.D. A Slot-Scheduling Mechanism at Congested Airports That Incorporates Efficiency, Fairness, and Airline Preferences. Transp. Sci. 2020, 54, 115–138. [Google Scholar] [CrossRef]
- Tan, X.; Wang, S.; Zeng, W.; Quan, Z. A Collaborative Optimization Method of Flight Slots Considering Fairness Among Airports. Math. Probl. Eng. 2022, 2022, 1–18. [Google Scholar] [CrossRef]
- Zeng, W.; Ren, Y.; Wei, W.; Yang, Z. A Data-Driven Flight Schedule Optimization Model Considering the Uncertainty of Operational Displacement. Comput. Oper. Res. 2021, 133, 105328. [Google Scholar] [CrossRef]
- Zografos, K.G.; Androutsopoulos, K.N.; Madas, M.A. Minding the Gap: Optimizing Airport Schedule Displacement and Acceptability. Transp. Res. Part A Policy Pract. 2018, 114, 203–221. [Google Scholar] [CrossRef]
- Wang, S.; Yang, L.; Wang, Y.; Cong, W. A Data and Model-Driven Approach to Predict Congestion of Departure Traffic at Airport. In Proceedings of the 2022 Integrated Communication, Navigation and Surveillance Conference (ICNS), Virtual, 5–7 April 2022; IEEE: Dulles, VA, USA, 2022; pp. 1–15. [Google Scholar]





| Set | |
| Set of all original flight times | |
| Set after adding new slots | |
| Set of runway operating modes | |
| Set of runway running patterns | |
| Set of approach runways corresponding to runway patterns | |
| Set of departure runways corresponding to runway pattern | |
| Set of the entrance of the corridor | |
| Ste of parking position | |
| Set of new entry slot | |
| Set of new departure slot | |
| Set of general entry time | |
| Set of total departure time | |
| Set of runway time | |
| Set of corridor entrance time | |
| Parameters | |
| Time zone parameters | |
| Maximum capacity of aircraft that can pass through each corridor entrance per unit time | |
| Estimated arrival flight time | |
| Estimated taxi-out time | |
| Estimated time of arrival | |
| Estimated landing time | |
| Scheduled in-block time | |
| Scheduled off-block time | |
| Estimated take off time | |
| Estimated time of departure | |
| Estimated time of arrival | |
| Average flight time from corridor entrance to landing strip | |
| Approach aircraft landing time at runway | |
| Average taxi-in time from runway to target stop | |
| Approach aircraft in parking position block wheel block time | |
| Average flight time from take-off runway to corridor entrance | |
| Probability parameter for the use of parking positions by approaching aircraft per unit time | |
| Probability parameter for the use of parking positions by departing aircraft per unit of time | |
| 1 h capacity limit parameter | |
| Approach capacity limit parameters | |
| Off-site capacity limiting parameters | |
| 15 min capacity limit parameter | |
| Variables | |
| Every incoming flight task | |
| Every departing flight assignment | |
| Model decision variables, adding an off-site task at hour | |
| Model decision variable, adding an entry task at hour |
| Callsign | Aircraft | Date | Terminal1 | Time1 | Time2 | Terminal2 |
|---|---|---|---|---|---|---|
| CA1638 | 737 | .2..... | ZYTN | 08:45 | 10:35 | ZBAA |
| CA1081 | 77F | .2345.. | ZBAA | 02:00 | 04:20 | ZSPD |
| CA1139 | 321 | 1234567 | ZBAA | 17:00 | 18:50 | ZBYC |
| CA1207 | 319 | 1234567 | ZBAA | 09:55 | 12:25 | ZLLL |
| CA1345 | 330 | 1234567 | ZBAA | 15:30 | 19:35 | ZJSY |
| Type of Result | Average Value | Standard Deviation |
|---|---|---|
| Average delay throughout the day | 0:09:34 | 0:00:45 |
| Average departure delay | 0:14:08 | 0:02:06 |
| Average approach delays | 0:05:39 | 0:00:41 |
| Arithmetic Example | Flight Volume | Average Delay throughout the Day |
|---|---|---|
| Base case | 1128 | 0:03:59 |
| Model increment example | 1509.84 | 0:05:52 |
| Random increment example | 1509.84 | 0:09:34 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, S.; Hu, M.; Chang, Z.; Zhu, X. A Methodology for Allocating Incremental Resources in Single-Airport Time Slots. Aerospace 2023, 10, 772. https://doi.org/10.3390/aerospace10090772
Wang S, Hu M, Chang Z, Zhu X. A Methodology for Allocating Incremental Resources in Single-Airport Time Slots. Aerospace. 2023; 10(9):772. https://doi.org/10.3390/aerospace10090772
Chicago/Turabian StyleWang, Shuce, Minghua Hu, Zhening Chang, and Xuhao Zhu. 2023. "A Methodology for Allocating Incremental Resources in Single-Airport Time Slots" Aerospace 10, no. 9: 772. https://doi.org/10.3390/aerospace10090772
APA StyleWang, S., Hu, M., Chang, Z., & Zhu, X. (2023). A Methodology for Allocating Incremental Resources in Single-Airport Time Slots. Aerospace, 10(9), 772. https://doi.org/10.3390/aerospace10090772





