Method for Underground Motion Using Vibration-Induced Ground Resistance Changes for Planetary Exploration
Abstract
:1. Introduction
1.1. Importance of Space Development and Current Situation of Extraterrestrial Body Exploration
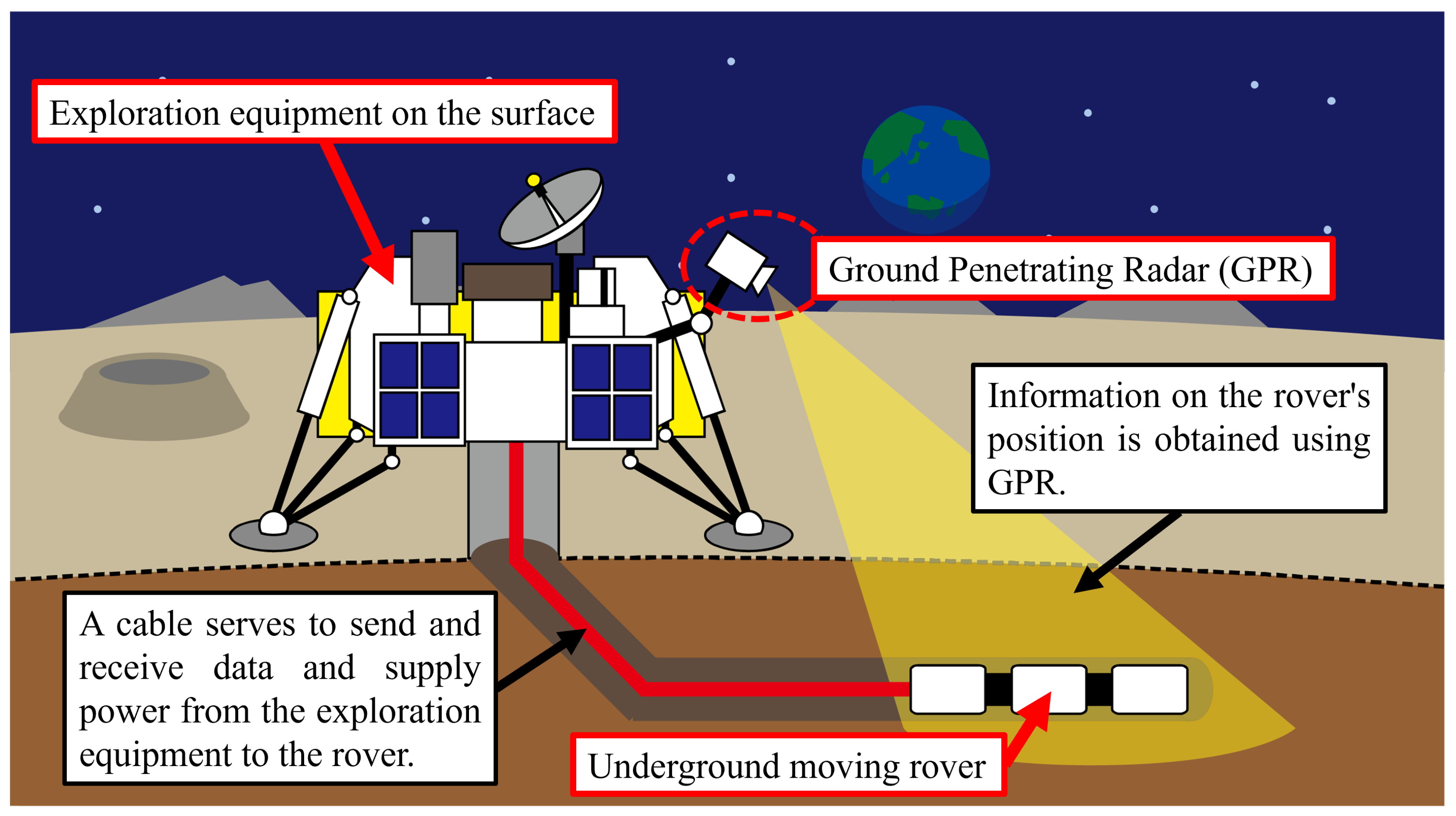
1.2. Importance of Underground Exploration
1.3. Study Overview
2. Proposal of Underground Movement Using Vibration
2.1. Underground Movement of Organisms
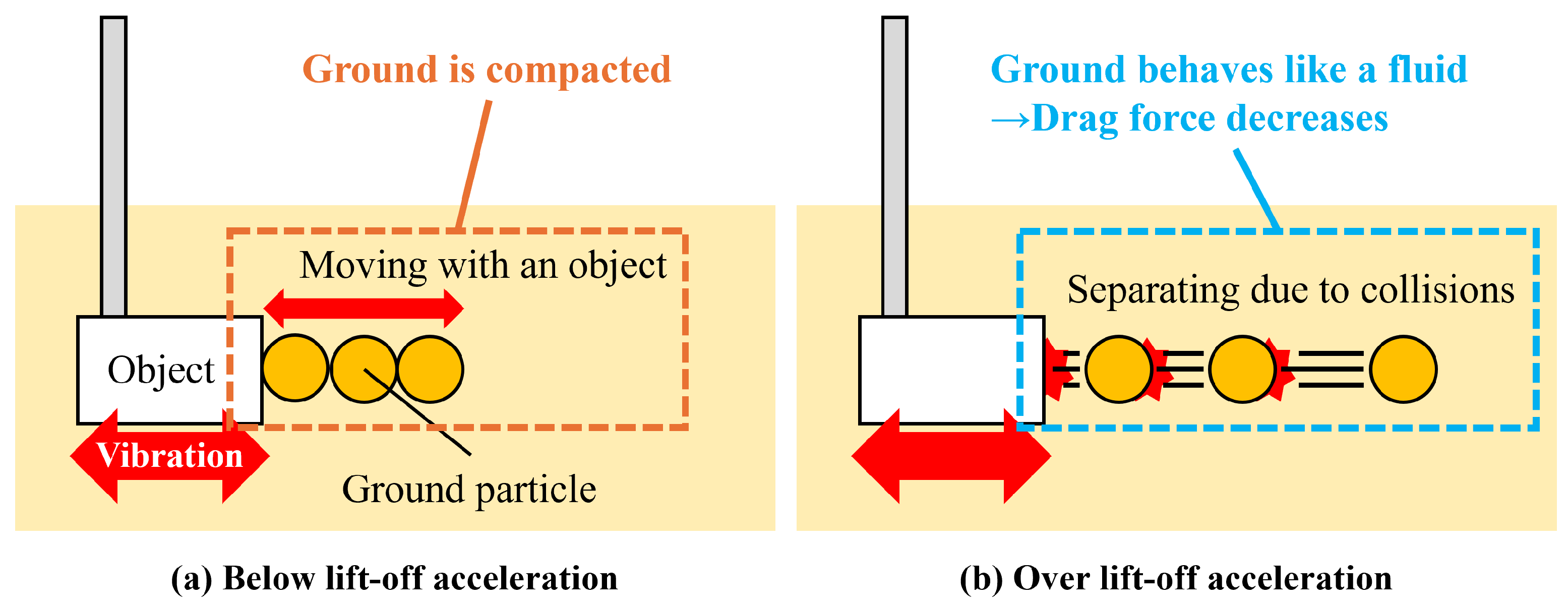
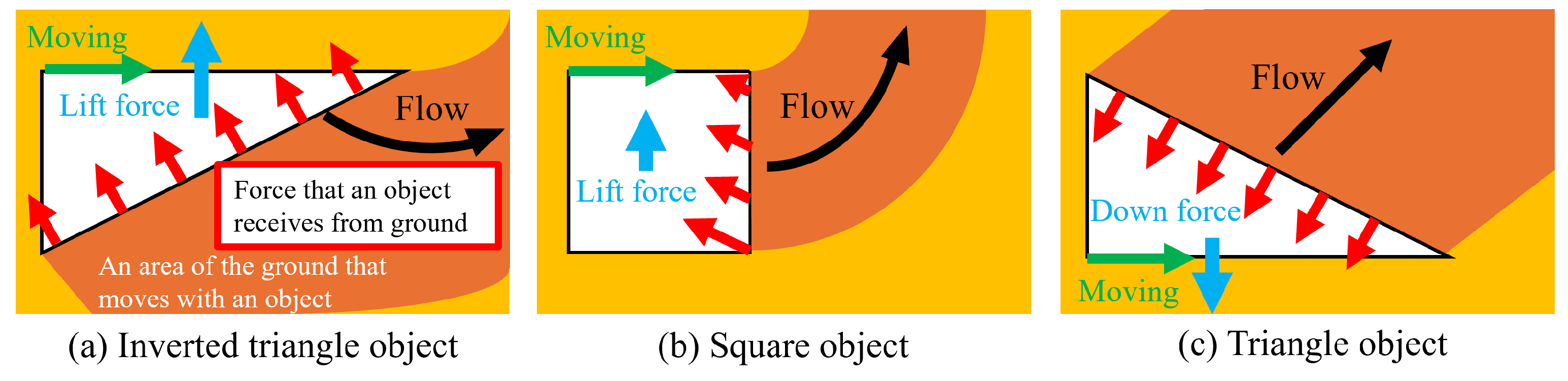
2.2. Changes in the Ground by Vibration

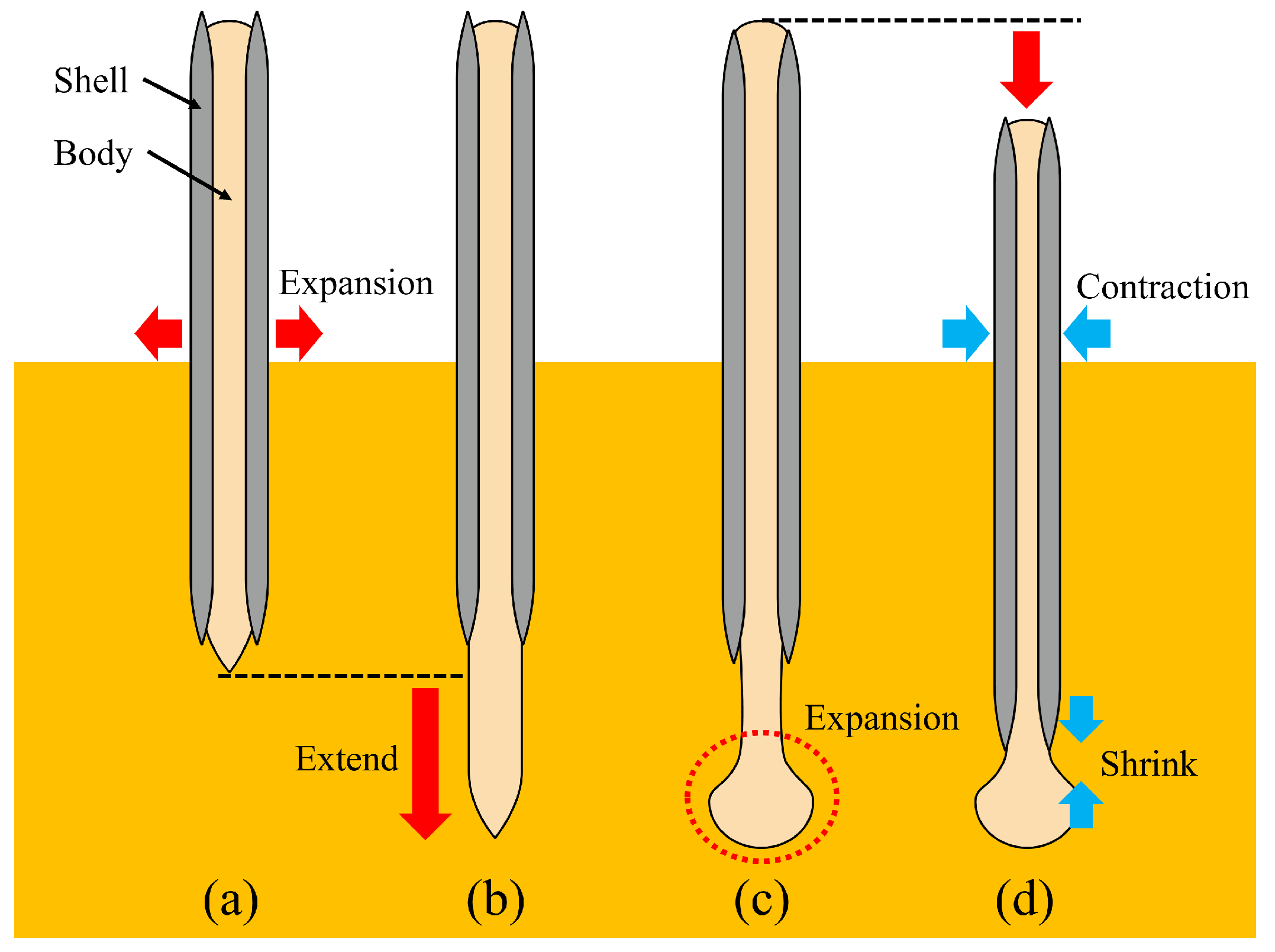
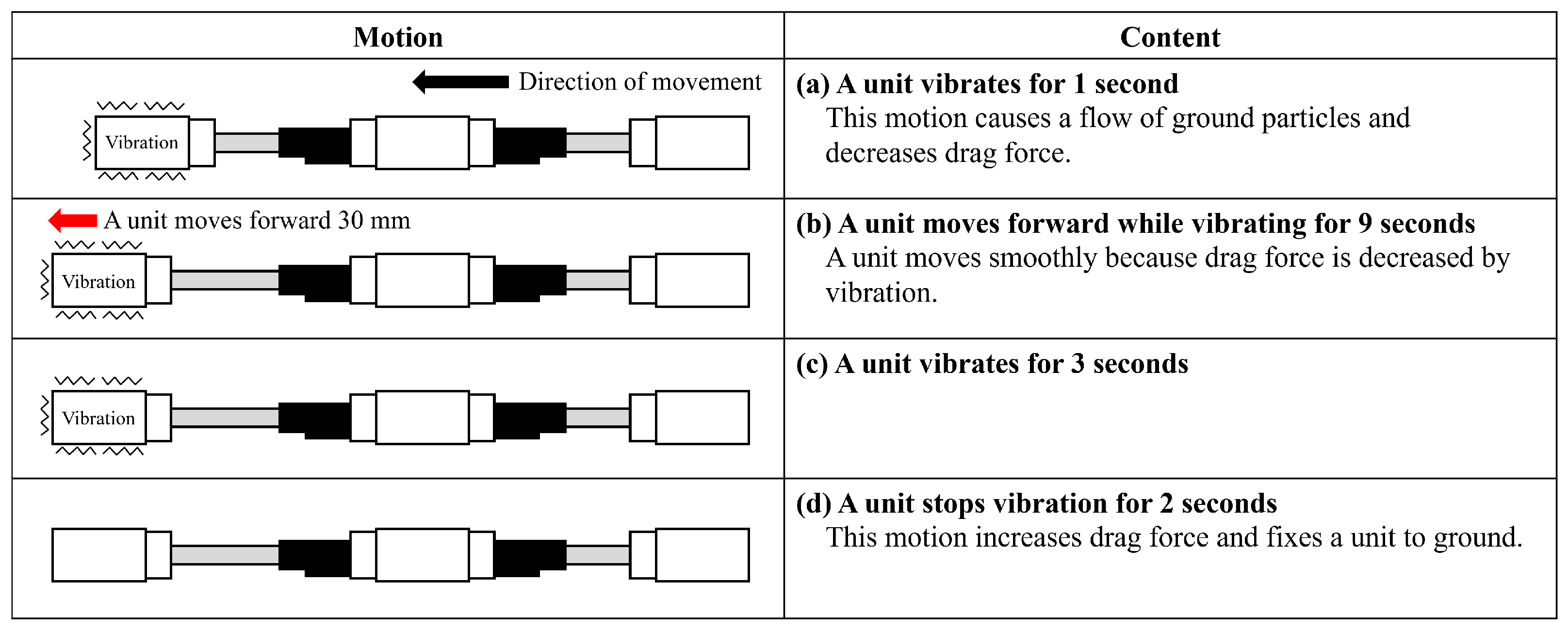
2.3. Proposal of a Testbed That Moves Underground
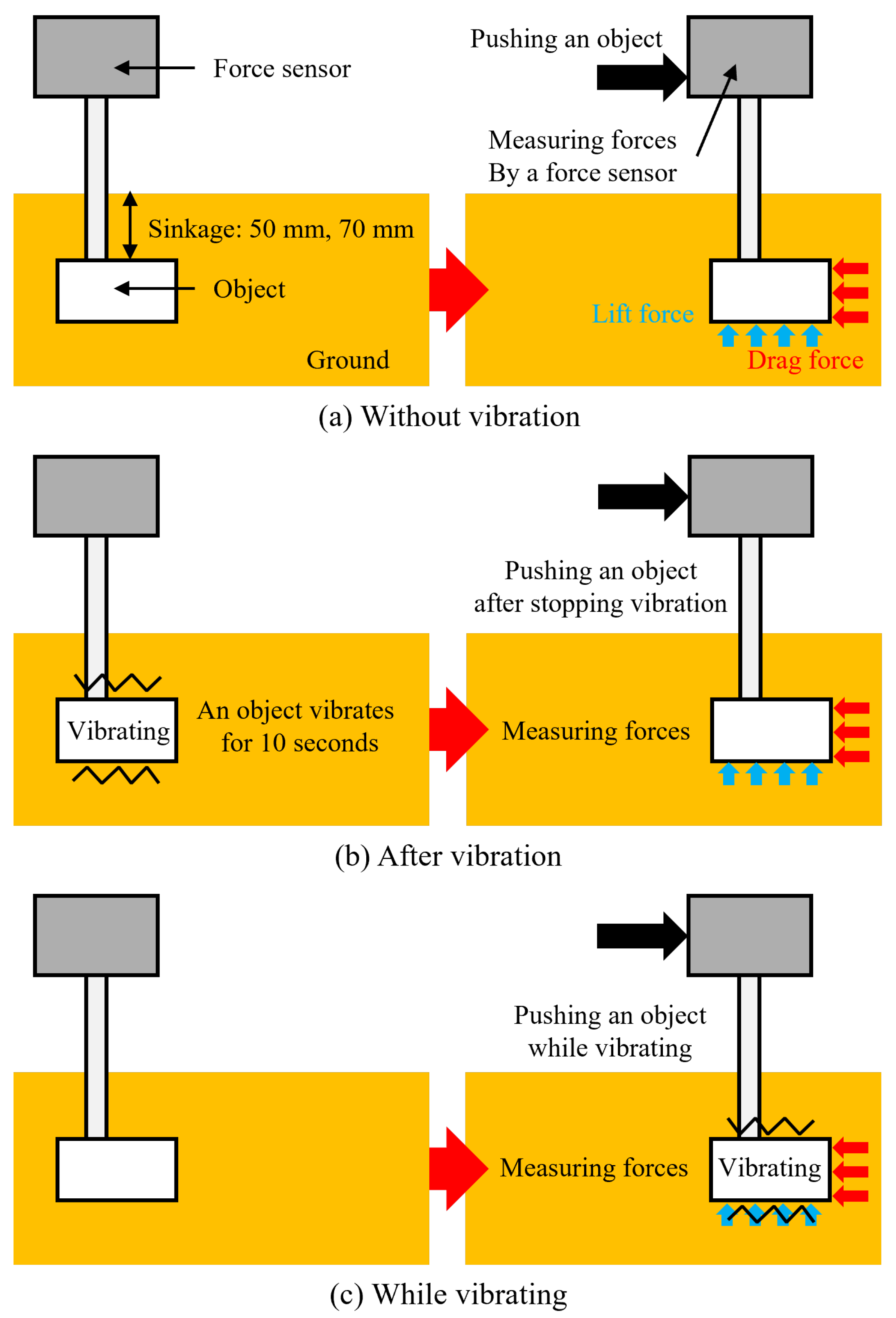
3. Measurement of Forces in the Ground
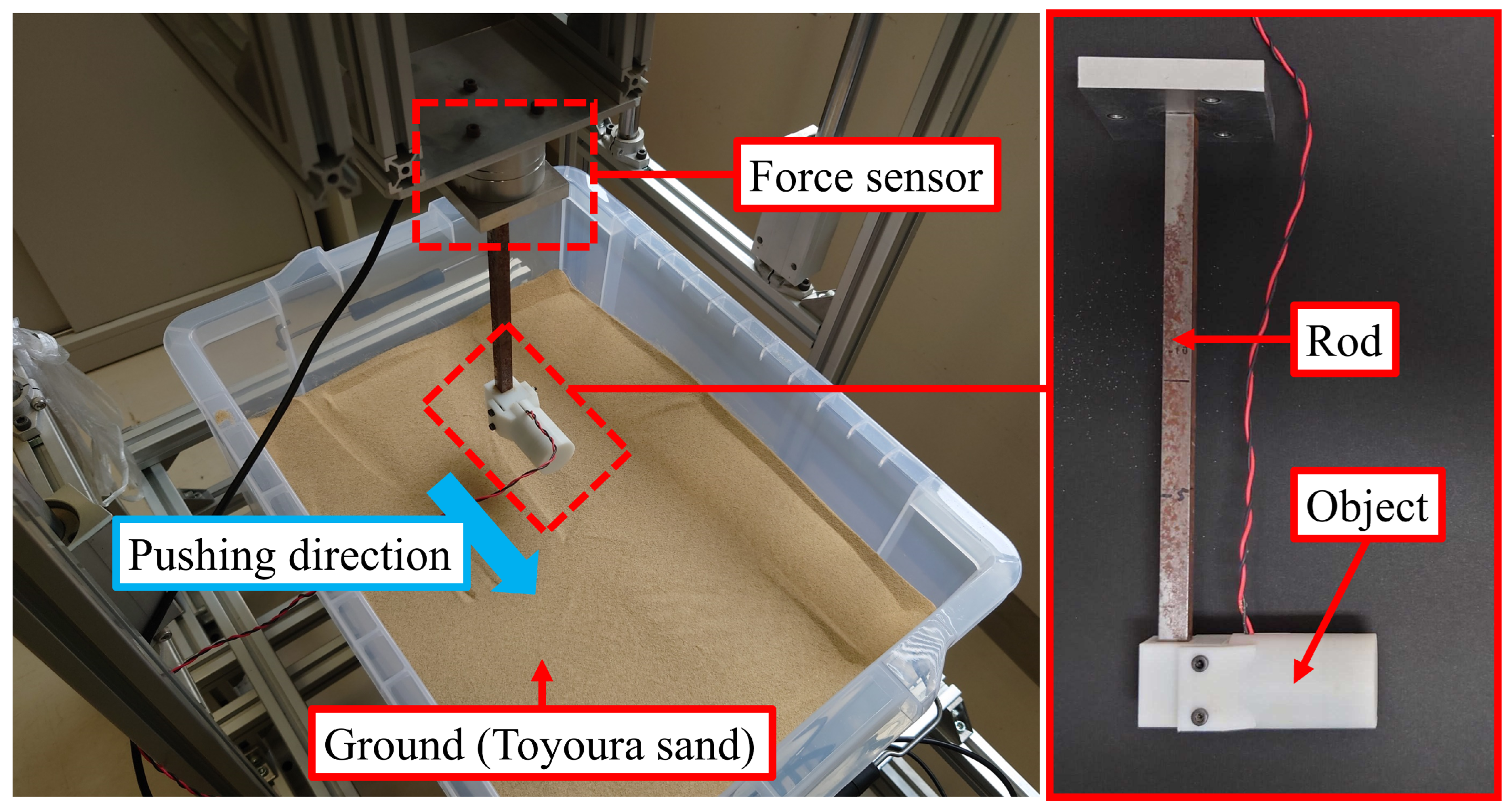
3.1. Experimental Methods
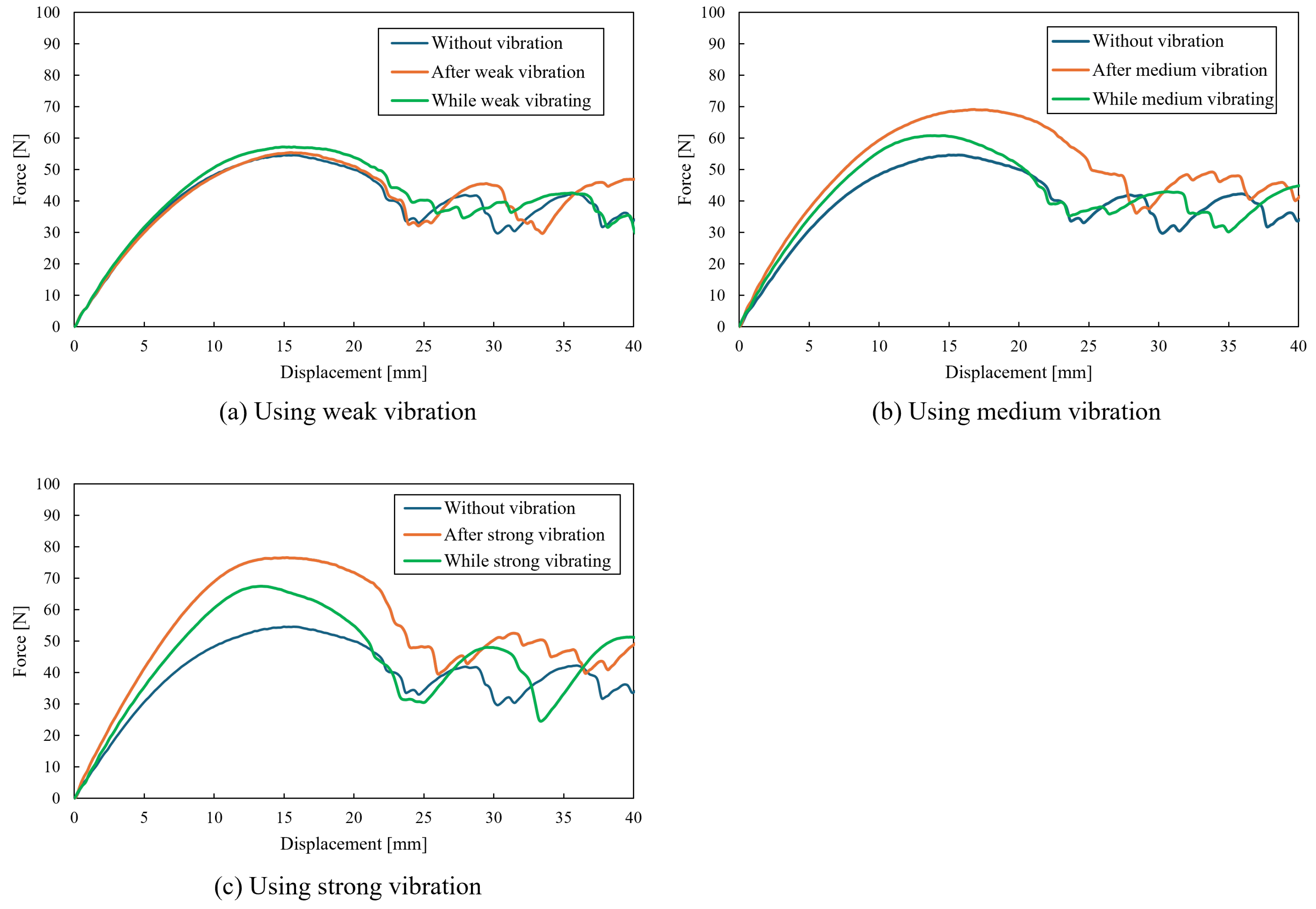
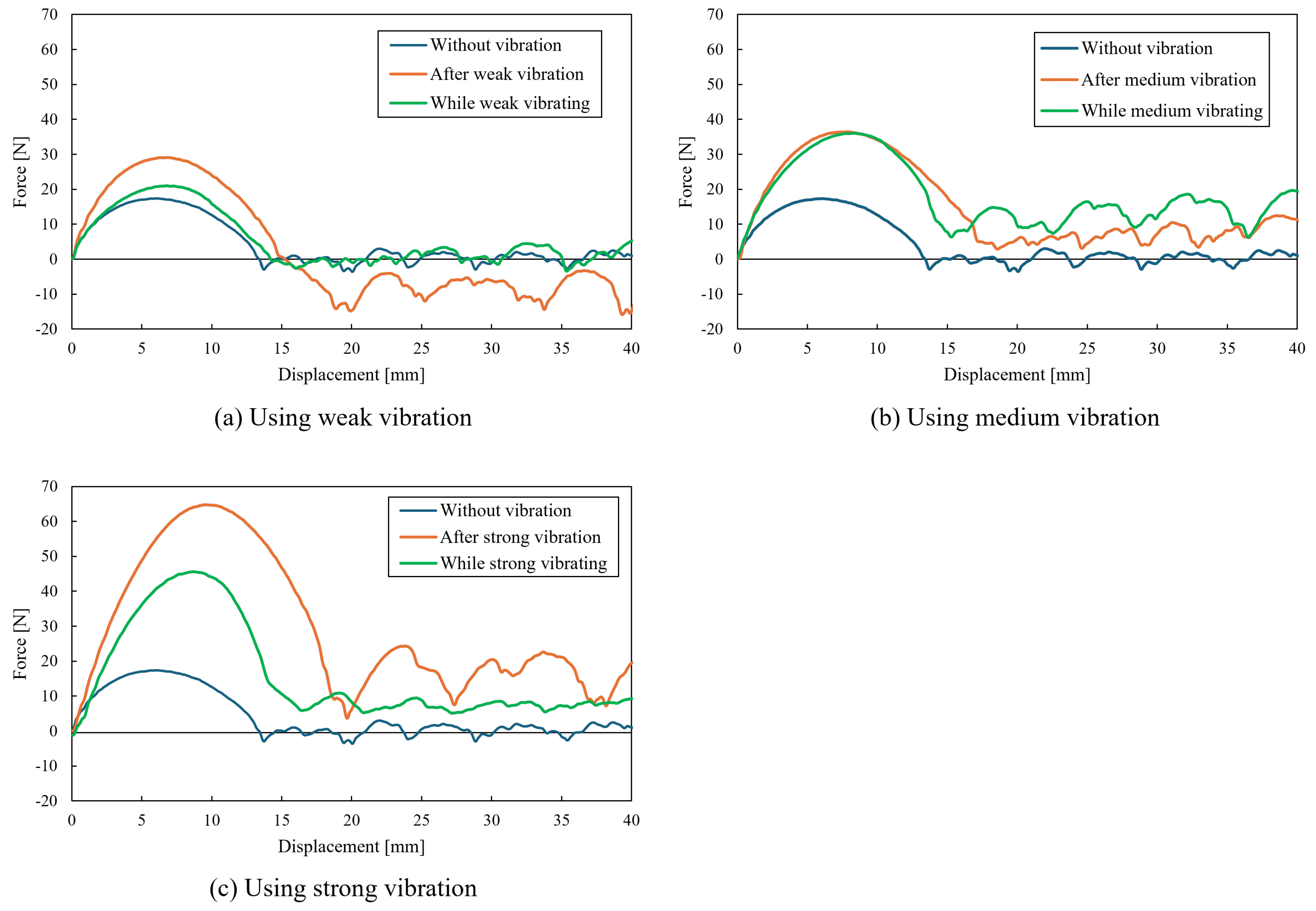
3.2. Experimental Results and Discussion
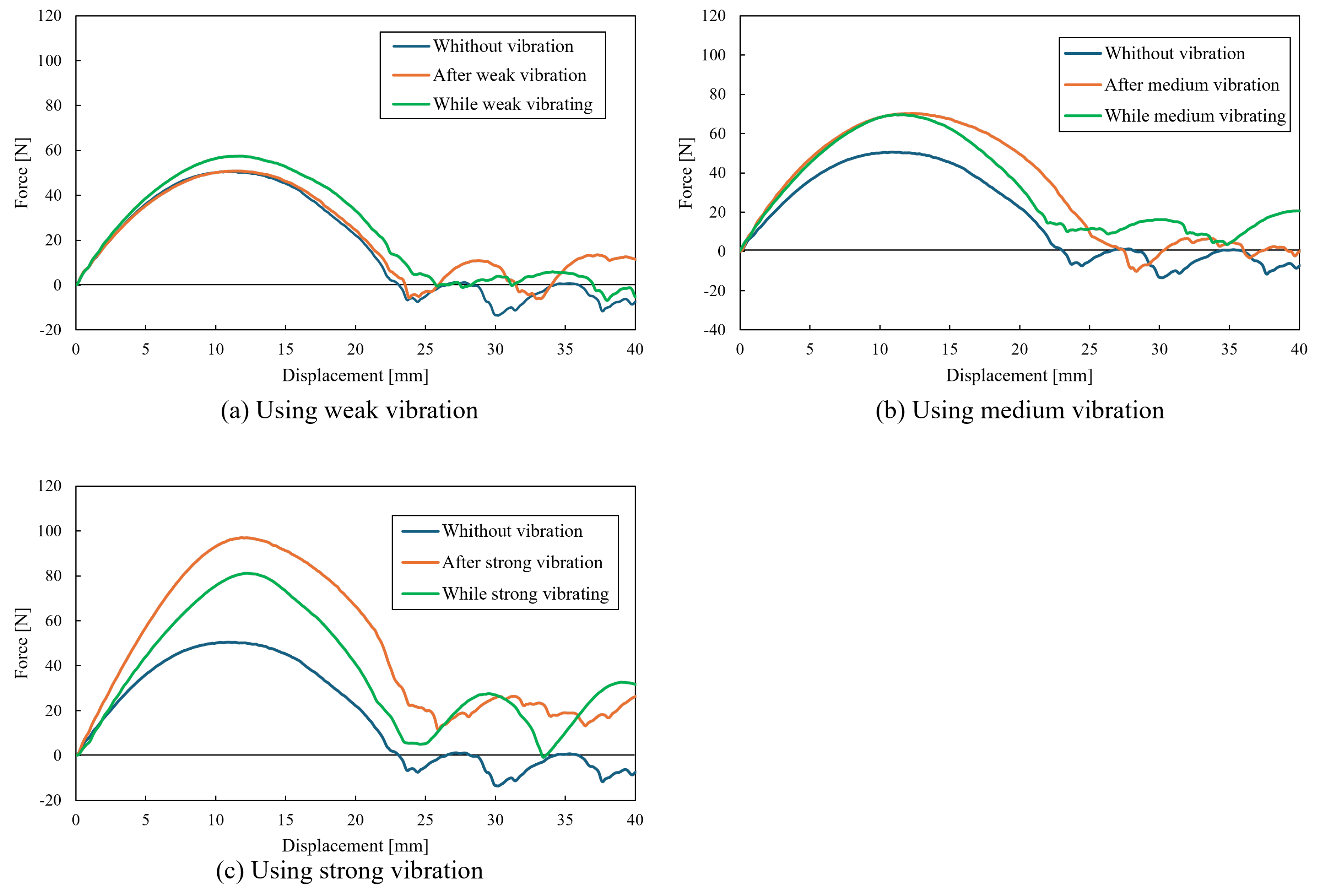
3.2.1. Drag Force Results
3.2.2. Lift Force Results
3.2.3. Proposal of Underground Motion Method Using Vibration
4. Verification of the Underground Motion Method
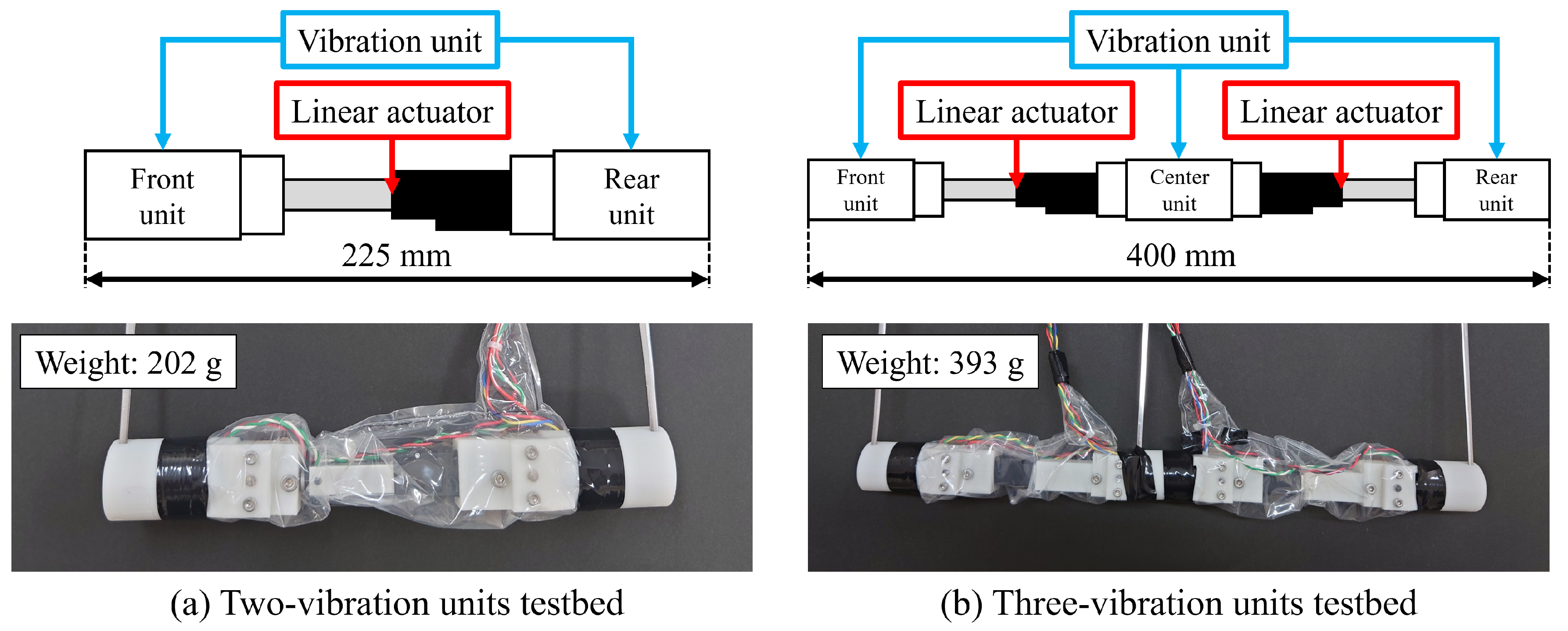
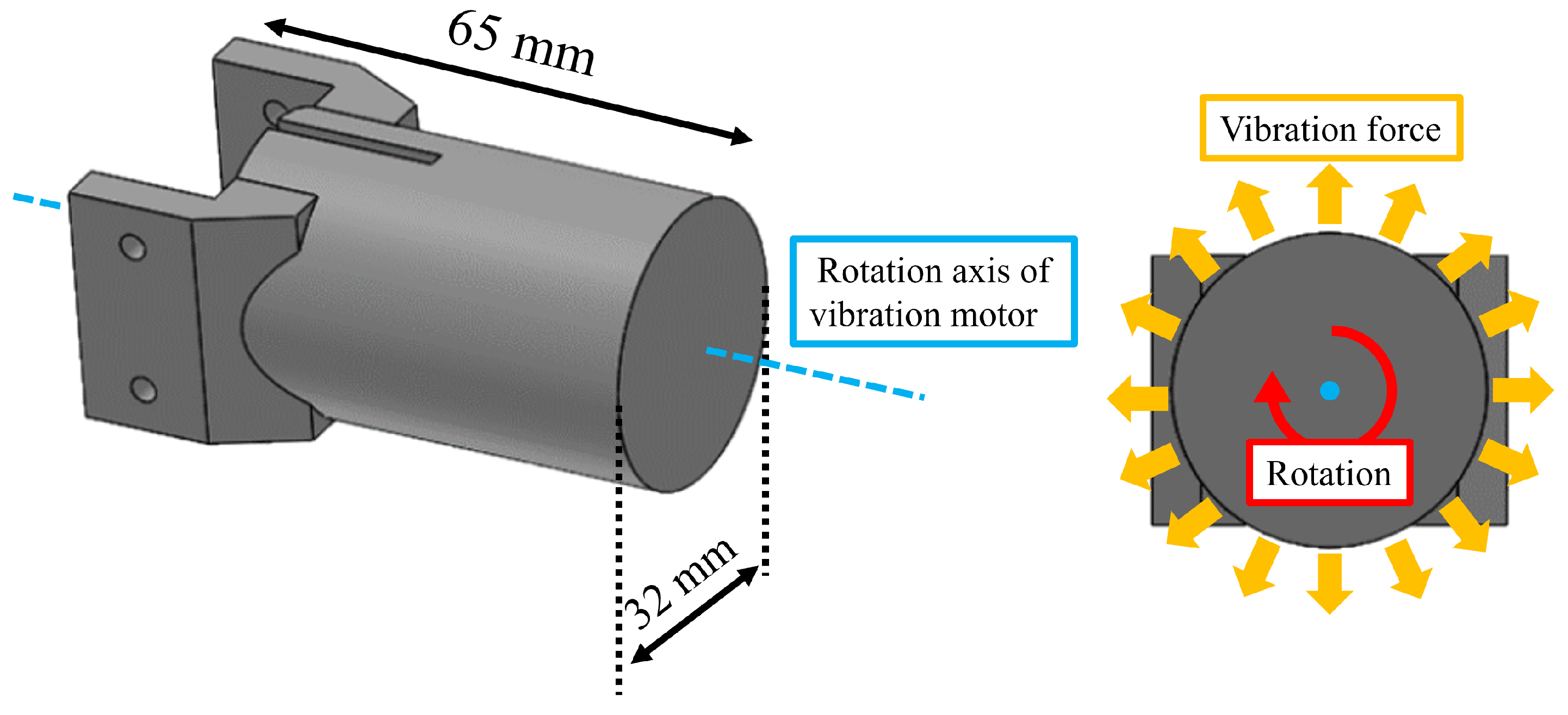
4.1. Experimental Methods
4.2. Experimental Results and Discussion
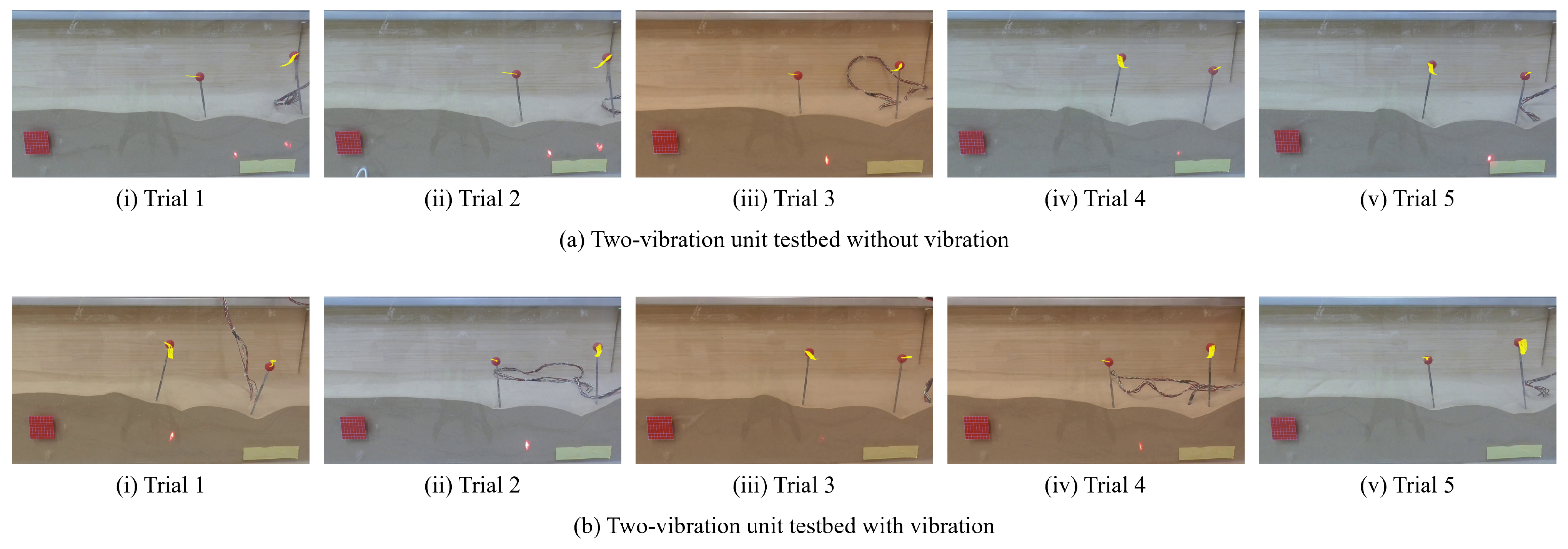
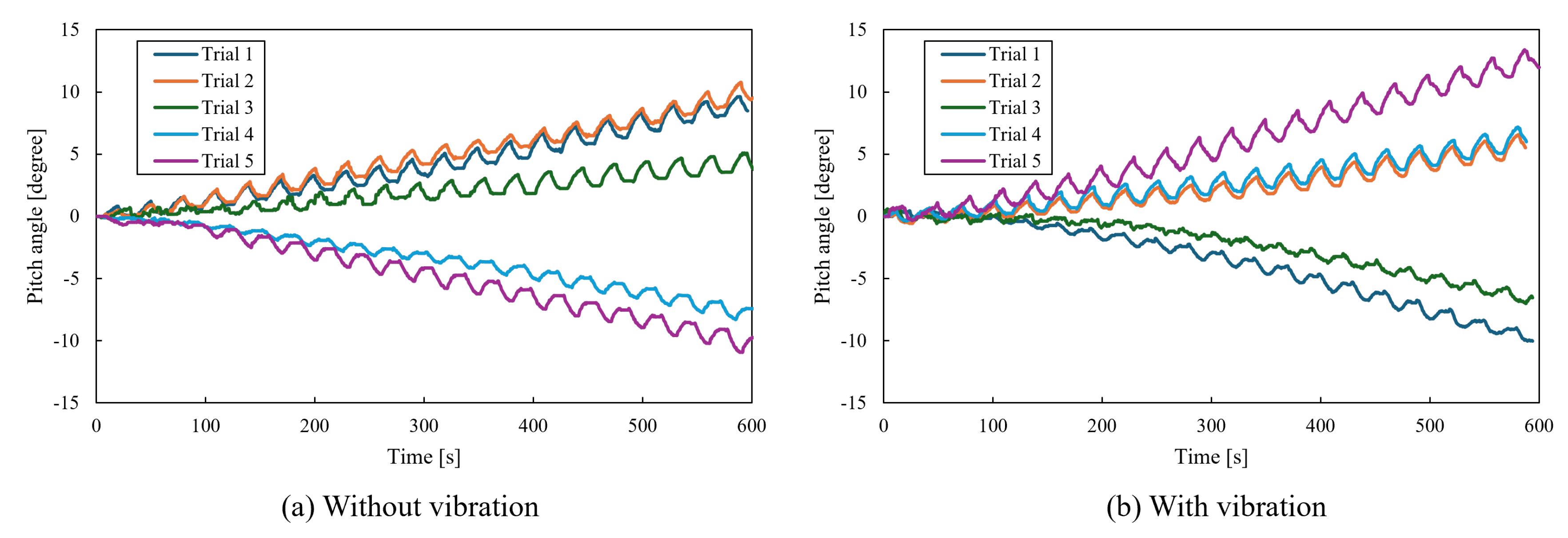
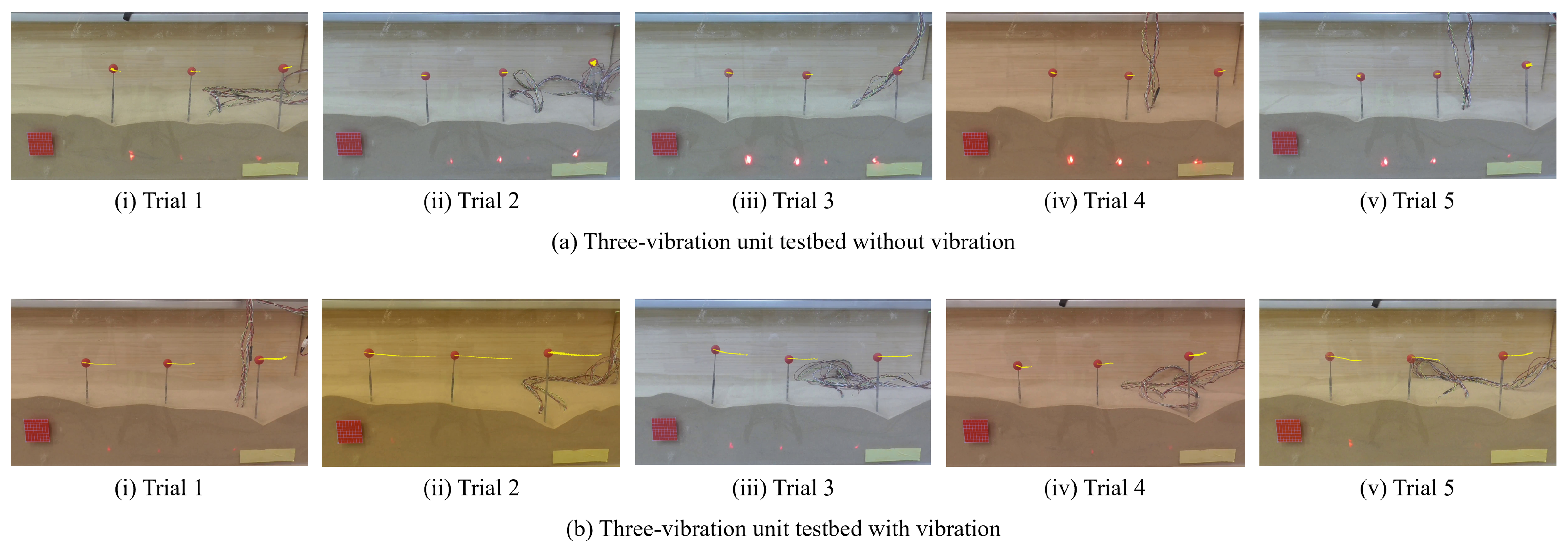
4.2.1. Results for Two-Vibration Unit Testbed
4.2.2. Results for the Three-Vibration Unit Testbed
4.2.3. Investigation of Relationship between Mobility and Strength of Vibration
4.2.4. Discussion of Slip Rate
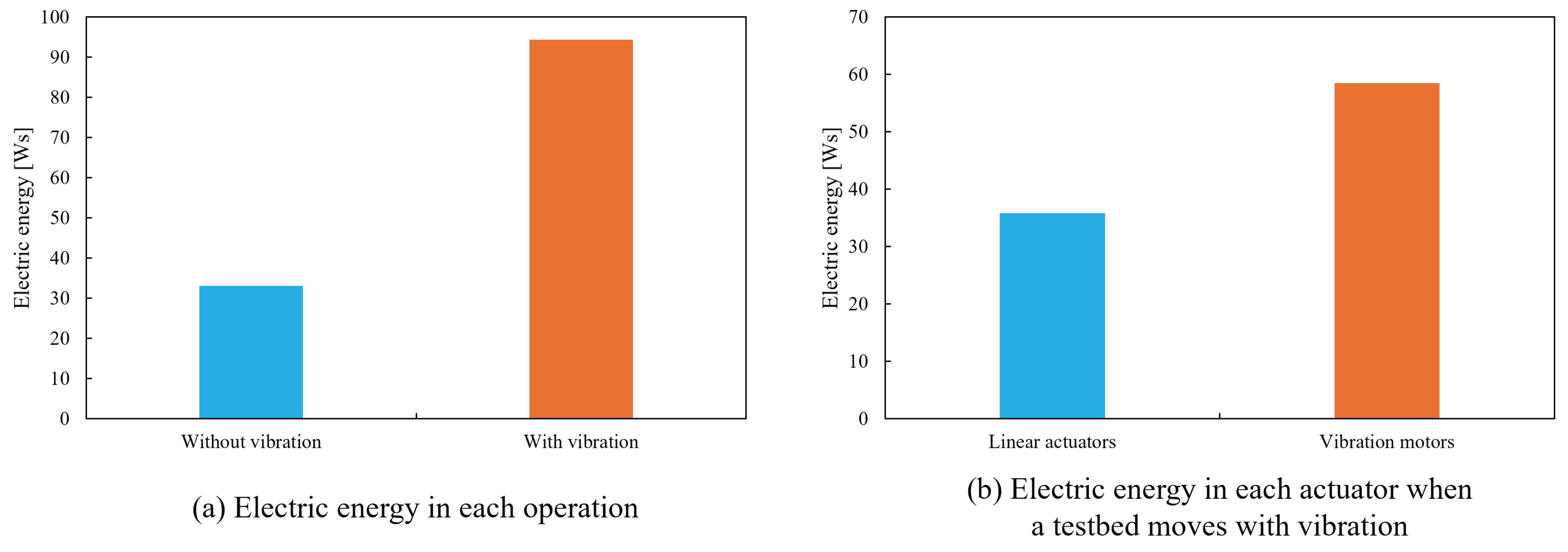
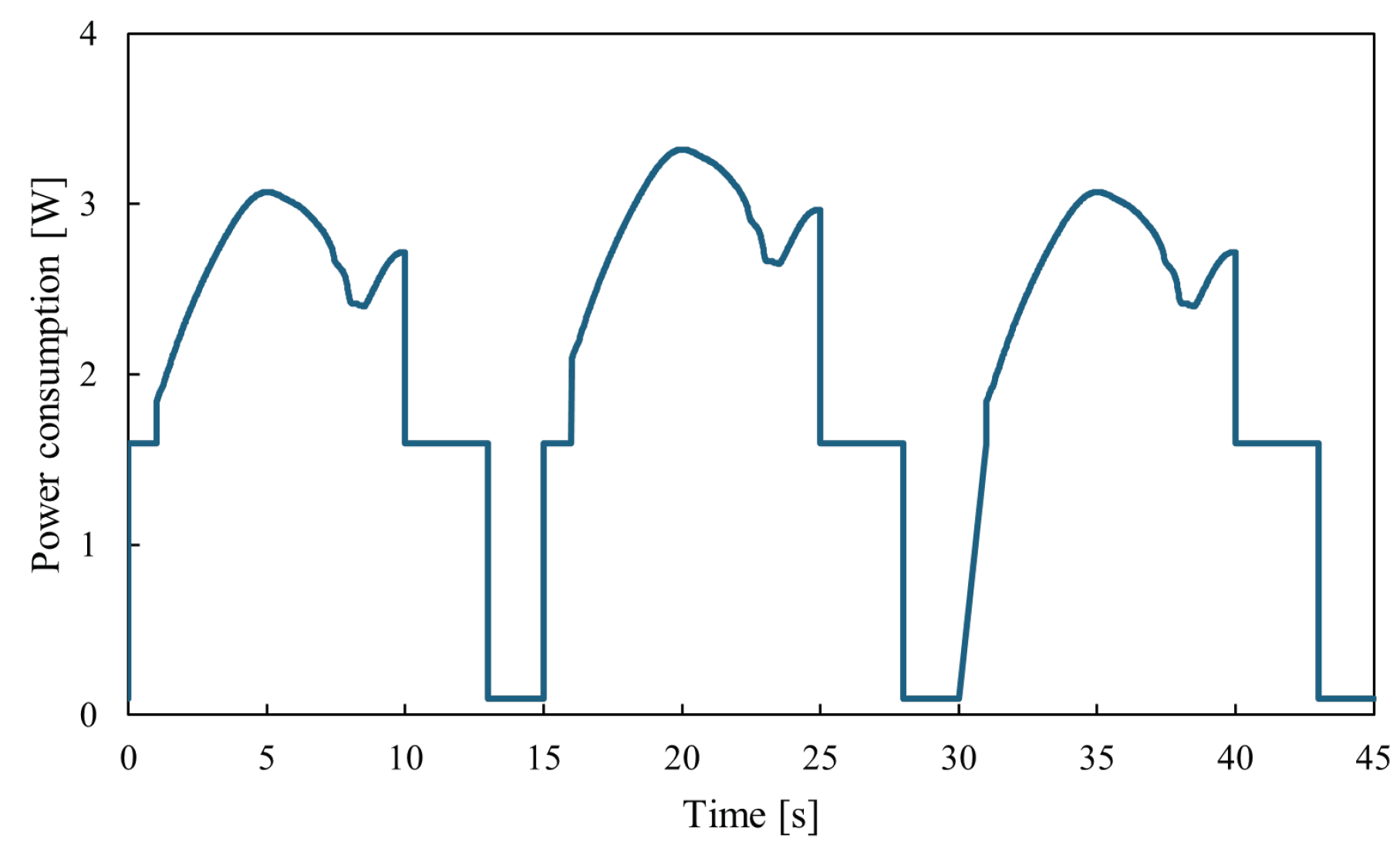
4.2.5. Discussion of Energy Efficiency
5. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| NASA | National Aeronautics and Space Administration |
| ISRO | Indian Space Research Organisation |
| JAXA | Japan Aerospace Exploration Agency |
| LUPEX | Lunar Polar Exploration Mission |
| MMX | Martian Moons eXploration |
| UGV | Unmanned Ground Vehicle |
| SLIM | Smart Lander for Investigating Moon |
| GPR | Ground Penetrating Radar |
| LPR | Luna Penetrating Radar |
| DEM | Discrete Element Method |
| NMR | Nuclear Magnetic Resonance |
| PIV | Particle Image Velocimetry |
| RPS | Rotations Per Second |
Appendix A. Measurement of the Ground Forces with Rod Only
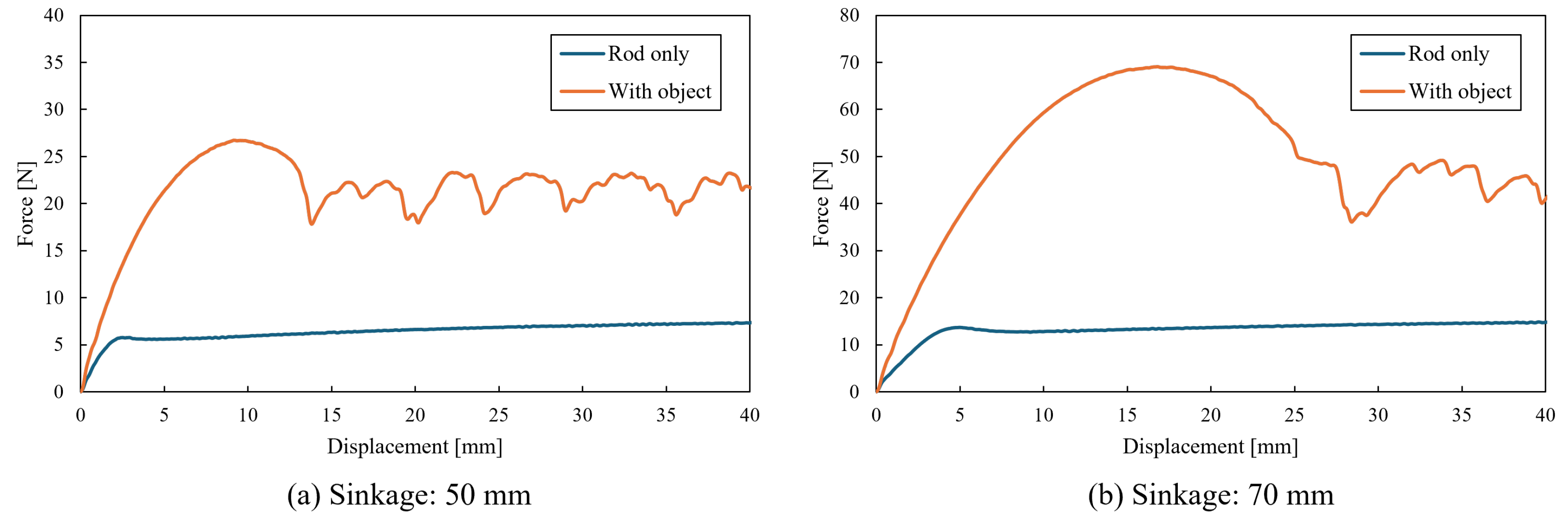
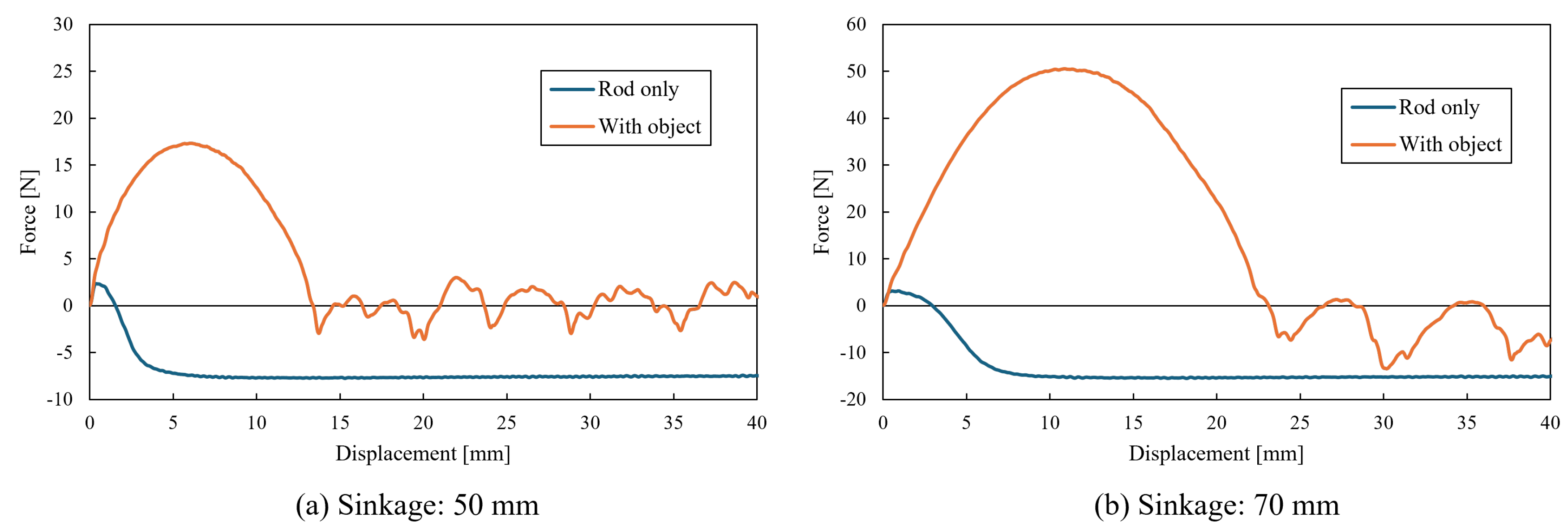
| Experimental Condition | Force in a Rod Only [N] | Force with an Object [N] |
|---|---|---|
| Drag force, sinkage of a rod: 50 mm | 7.36 | 26.7 |
| Drag force, sinkage of a rod: 70 mm | 14.8 | 69.1 |
| Lift force, sinkage of a rod: 50 mm | 2.36 | 17.3 |
| Lifr force, sinkage of a rod: 70 mm | 3.19 | 50.5 |
Appendix B. Measurement of Vibration Parameters
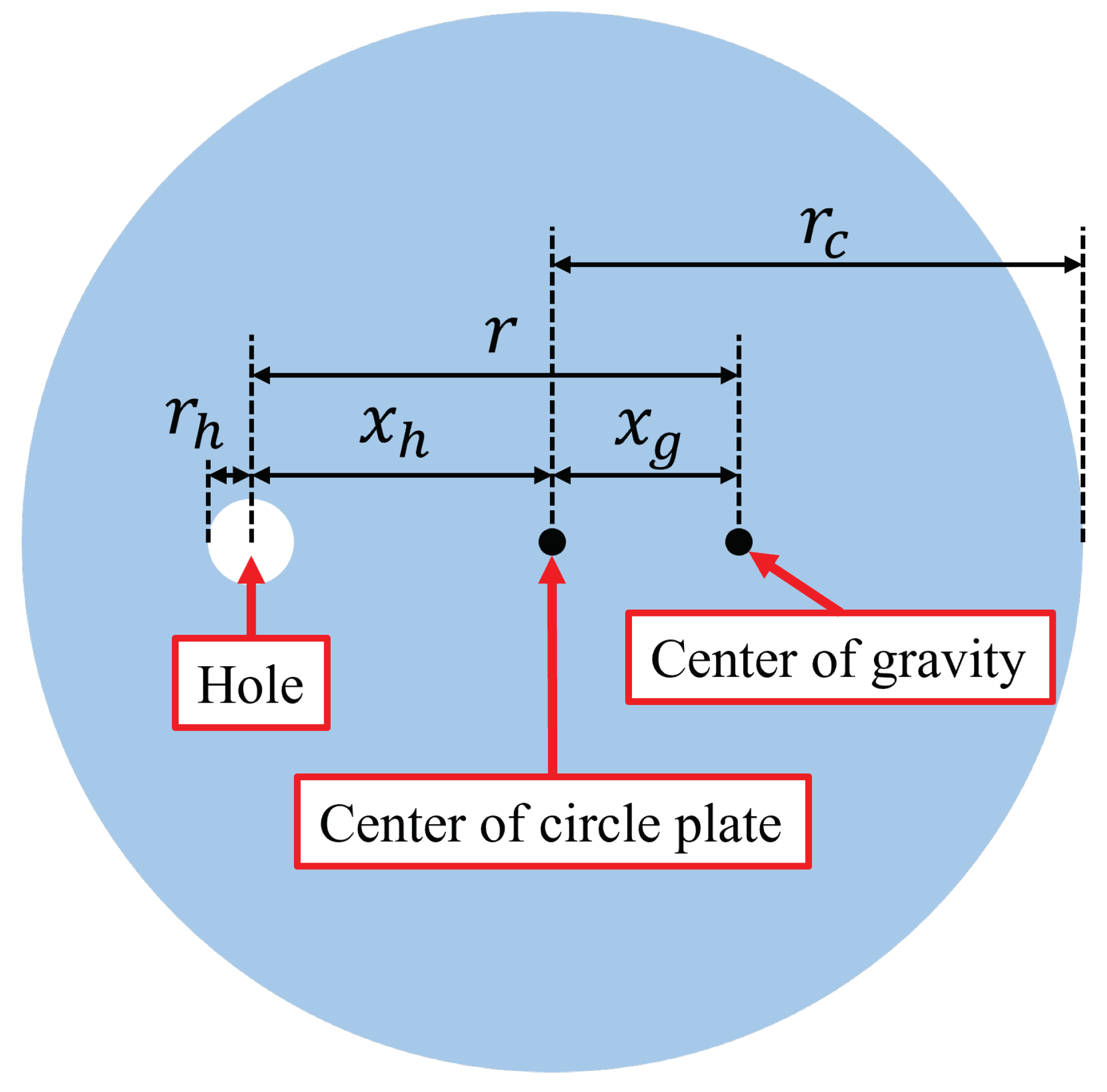
| Parameter | Value |
|---|---|
| Distance from center of circle plate to center of gravity | [m] |
| Distance from center of circle plate to center of hole | [m] |
| Radius of hole | [m] |
| Radius of circle plate | [m] |
| Rotation radius of an unbalanced load r | [m] |
| Mass of an unbalanced load m | [kg] |
References
- Wang, T.; Quan, Q.; Xu, Y.; Yu, H.; Tang, D.; Deng, Z. Study the influence of anchoring parameters on asteroid drilling anchoring with discrete element simulation. Adv. Spa. Res. 2023, 71, 816–828. [Google Scholar] [CrossRef]
- Hein, M.A.; Matheson, R.; Fries, D. A techno-economic analysis of asteroid mining. Act. Astr. 2020, 168, 104–115. [Google Scholar] [CrossRef]
- Sommariva, A.; Gori, L.; Chizzolini, B.; Pianorsi, M. The economics of moon mining. Act. Astr. 2020, 170, 712–718. [Google Scholar] [CrossRef]
- Qiao, L.; Chen, J.; Xu, L.; Wan, S.; Cao, H.; Li, B.; Ling, Z. Geology of the Chang’e-5 landing site: Constraints on the sources of samples returned from a young nearside mare. Icarus 2021, 364, 114480. [Google Scholar] [CrossRef]
- Liu, J.; Liu, B.; Ren, X.; Li, C.; Shu, R.; Guo, L.; Yu, S.; Zhou, Q.; Liu, D.; Zeng, X.; et al. Evidence of water on the lunar surface from Chang’E-5 in-situ spectra and returned samples. Nat. Commun. 2022, 13, 3119. [Google Scholar] [CrossRef]
- NASA, MARS. 2020 Mission Perseverance Rover. Available online: https://mars.nasa.gov/mars2020/mission/overview/ (accessed on 26 August 2024).
- JAXA, International Space Exploration, Lunar Polar Exploration (LUPEX). Available online: https://www.exploration.jaxa.jp/e/program/lunarpolar/index.html (accessed on 26 August 2024).
- JAXA, International Space Exploration, Martian Moons eXploration (MMX). Available online: https://www.exploration.jaxa.jp/e/program/index.html#mmx (accessed on 26 August 2024).
- Touhoku University, Startup Incubation Center. Available online: https://startup.tohoku.ac.jp/achievement/interview-kisuitech/ (accessed on 26 August 2024).
- JAXA, International Space Exploration, Smart Lander for Investigating Moon (SLIM). Available online: https://www.exploration.jaxa.jp/e/program/index.html#slim (accessed on 26 August 2024).
- NASA, Gateway. Available online: https://www.nasa.gov/mission/gateway/ (accessed on 26 August 2024).
- JAXA, Hayabusa2 Extended Mission. Available online: https://www.hayabusa2.jaxa.jp/en/ (accessed on 26 August 2024).
- NASA, Weather on the Moon. Available online: https://science.nasa.gov/moon/weather-on-the-moon/ (accessed on 26 August 2024).
- Notsu, R.; Nagano, H.; Ogawa, H. A proposal of lunar long duration method (Fabrication of lunar environment simulator and experimental validation). Tran. JSME 2014, 80, TEP0199. [Google Scholar] [CrossRef]
- Nakatake, T.; Konno, M.; Mizushima, A.; Yamada, Y.; Nakamura, T.; Kubota, T. Soil-Circulating System for a Peristaltic-Type Lunar Excavation Robot. J. Rob. Soc. Jpn. 2017, 35, 230–238. [Google Scholar] [CrossRef]
- Nicholas, D.N.; Karsai, A.; Cooper, M.M.; Aydin, O.Y.; Aydin, E.; Goldman, I.D.; Hawkes, W.E. Controlling subterranean forces enables a fast, steerable, burrowing soft robot. Sci. Robot. 2021, 6, eabe2922. [Google Scholar] [CrossRef]
- Nesnas, A.D.I.; Kerber, L.; Sellar, G.; Balint, T.; Denevi, B.; Parness, J.A.; Kornfeld, P.R.; Smith, M.; McGarey, P.; Brown, T.; et al. Moon Diver: Exploring a pit’s exposed strata to understand lunar volcanism. Act. Astr. 2023, 211, 163–176. [Google Scholar] [CrossRef]
- Yuan, Z.; Mu, R.; Zhao, H.; Wang, K. Predictive Model of a Mole-Type Burrowing Robot for Lunar Subsurface Exploration. Aerospace 2023, 10, 190. [Google Scholar] [CrossRef]
- Winter, G.A.; Deits, H.L.R.; Dorsch, S.D.; Slocum, H.A.; Hosoi, E.A. Razor clam to RoboClam: Burrowing drag reduction mechanisms and their robotic adaptation. Bioinspir. Biomim. 2014, 9, 036009. [Google Scholar] [CrossRef] [PubMed]
- Cerkvenik, U.; Dodou, D.; Leeuwen, L.J.; Gussekloo, S.W.S. Functional principles of steerable multi-element probes in insects. Bio. Rev. 2019, 94, 555–574. [Google Scholar] [CrossRef] [PubMed]
- Watanabe, T.; Iizuka, K. Proposal of walking to reduce slipping behavior using compaction effect of loose soil caused by a propagation of vibration for small light lunar planetary exploration rovers with legs. Trans. JSME 2020, 86, 886. [Google Scholar] [CrossRef]
- Baumgartner, W.; Fidler, F.; Weth, A.; Habbecke, M.; Jakob, P.; Butenweg, C.; Bohme, W. Investigating the Locomotion of the Sandfish in Desert Sand Using NMR-Imaging. PLoS ONE 2008, 3, e3309. [Google Scholar] [CrossRef] [PubMed]
- Watanabe, T.; Iizuka, K. Experimental investigation of relationship between bearing capacity and vibration parameters for planetary exploration legged rovers. ROBOMECH J. 2023, 10, 27. [Google Scholar] [CrossRef]
- Yeomans, B.; Saaj, M.C.; Winnendael, V.M. Walking planetary rovers - Experimental analysis and modelling of leg thrust in loose granular soils. J Terramech. 2013, 50, 107–120. [Google Scholar] [CrossRef]
- King, H.R.; Susante, V.P.; Gefreh, A.M. Analytical models and laboratory measurements of the soil-tool interaction force to push a narrow tool through JSC-1A lunar simulant and Ottawa sand at different cutting depths. J. Terramech. 2011, 48, 85–95. [Google Scholar] [CrossRef]
- Kobayashi, T.; Fujiwara, Y.; Yamakawa, J.; Yasufuku, N.; Omine, K. Mobility performance of a rigid wheel in low gravity environments. J. Terramech. 2010, 47, 261–274. [Google Scholar] [CrossRef]
- Ono, S.; Namikawa, S.; Yoshida, K. Analysis of soil flow and traction mechanics for lunar rovers over different types of soils using particle image velocimetry. J. Terramech. 2021, 95, 89–100. [Google Scholar] [CrossRef]
- Oe, A.; Nishida, S.; Nakatani, S. Study of passive steering mechanism for small Mars surface exploration rovers. J. Terramech. 2024, 112, 35–43. [Google Scholar] [CrossRef]
- Wada, S.; Kouda, M.; Enami, A. Experimental study on passive earth pressure Part 1 The experimental device and an example of the passive earth pressure tests by the device. J. Str. Con. Eng. Arc. Ins. Japan 1998, 63, 69–76. [Google Scholar] [CrossRef]
- Watanabe, T.; Iizuka, K. Observation of Movement of Ground Particles Given Vibration When Rod Is Dragged. Int. J. Eng. Technol. 2022, 14, 1–8. [Google Scholar] [CrossRef]
- Arnold, N.E. Identifying the effects of history on adaptation: Origins of different sand-diving techniques in lizards. J. Zoo. 1995, 235, 351–388. [Google Scholar] [CrossRef]
- Ding, Y.; Gravish, N.; Goldman, I.D. Drag Induced Lift in Granular Media. Phys. Rev. Lett. 2011, 106, 028001. [Google Scholar] [CrossRef] [PubMed]
- Maladen, D.R.; Umbanhowar, B.P.; Ding, Y.; Masse, A.; Goldman, I.D. Granular lift forces predict vertical motion of a sand-swimming robot. In Proceedings of the 2011 IEEE International Conference on Robotics and Automation, Shanghai, China, 9–13 May 2011. [Google Scholar] [CrossRef]
- SAHRP, News Release, January 2024. Available online: https://corporate.jp.sharp/news/240129-b.html (accessed on 26 August 2024).











| Item | Condition (Value) |
|---|---|
| Linear actuator | L12-30-210-S (Actuonix Motion Devices Inc., Saanichton, BC, Canada) |
| Vibration motor | TP-2528C-24 (Three Peace Co., Ltd., Tokyo, Japan) |
| Speed of linear actuator | 3.34 mm/s |
| Length that linear actuator extends | 30 mm |
| Vibration | Supply Voltage [V] | Vibration Force (Error) [N] | Frequency (Error) [Hz] |
|---|---|---|---|
| Weak | 10 | 1.09 () | 70 () |
| Medium | 20 | 5.20 () | 154 () |
| Strong | 30 | 11.9 () | 233 () |
| Item | Condition (Value) |
|---|---|
| Number of trials | 5 |
| Sinkage of the object | 50 mm, 70 mm |
| Pushing speed | 4.17 mm/s |
| Pushing time | 10 s |
| Type of sand | Toyoura sand (Toyoura Keiseki Kogyo Co., Ltd., Shimonoseki, Japan) |
| Vibration motor | TP-2528C-24 (Three Peace Co., Ltd., Tokyo, Japan) |
| Force sensor | PFS055YA251U6 (Leptrino Co., Ltd., Saku, Japan) |
| Item | Condition (Value) |
|---|---|
| Number of trials | 5 |
| Sinkage of testbed | 100 mm |
| Movement pattern | With and without vibration |
| Type of sand | Toyoura sand |
| Generated Electricity in One Solar Panel [W] | Size of One Solar Panel [mm] |
|---|---|
| 20.9 | Length: 297, Width: 271, Height: 0.25 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Watanabe, T.; Kobayashi, K.; Hiramoto, K.; Iizuka, K. Method for Underground Motion Using Vibration-Induced Ground Resistance Changes for Planetary Exploration. Aerospace 2024, 11, 811. https://doi.org/10.3390/aerospace11100811
Watanabe T, Kobayashi K, Hiramoto K, Iizuka K. Method for Underground Motion Using Vibration-Induced Ground Resistance Changes for Planetary Exploration. Aerospace. 2024; 11(10):811. https://doi.org/10.3390/aerospace11100811
Chicago/Turabian StyleWatanabe, Tomohiro, Koya Kobayashi, Kazuhiko Hiramoto, and Kojiro Iizuka. 2024. "Method for Underground Motion Using Vibration-Induced Ground Resistance Changes for Planetary Exploration" Aerospace 11, no. 10: 811. https://doi.org/10.3390/aerospace11100811
APA StyleWatanabe, T., Kobayashi, K., Hiramoto, K., & Iizuka, K. (2024). Method for Underground Motion Using Vibration-Induced Ground Resistance Changes for Planetary Exploration. Aerospace, 11(10), 811. https://doi.org/10.3390/aerospace11100811






