Numerical Study on Far-Field Noise Characteristic Generated by Wall-Mounted Swept Finite-Span Airfoil within Transonic Flow
Abstract
:1. Introduction
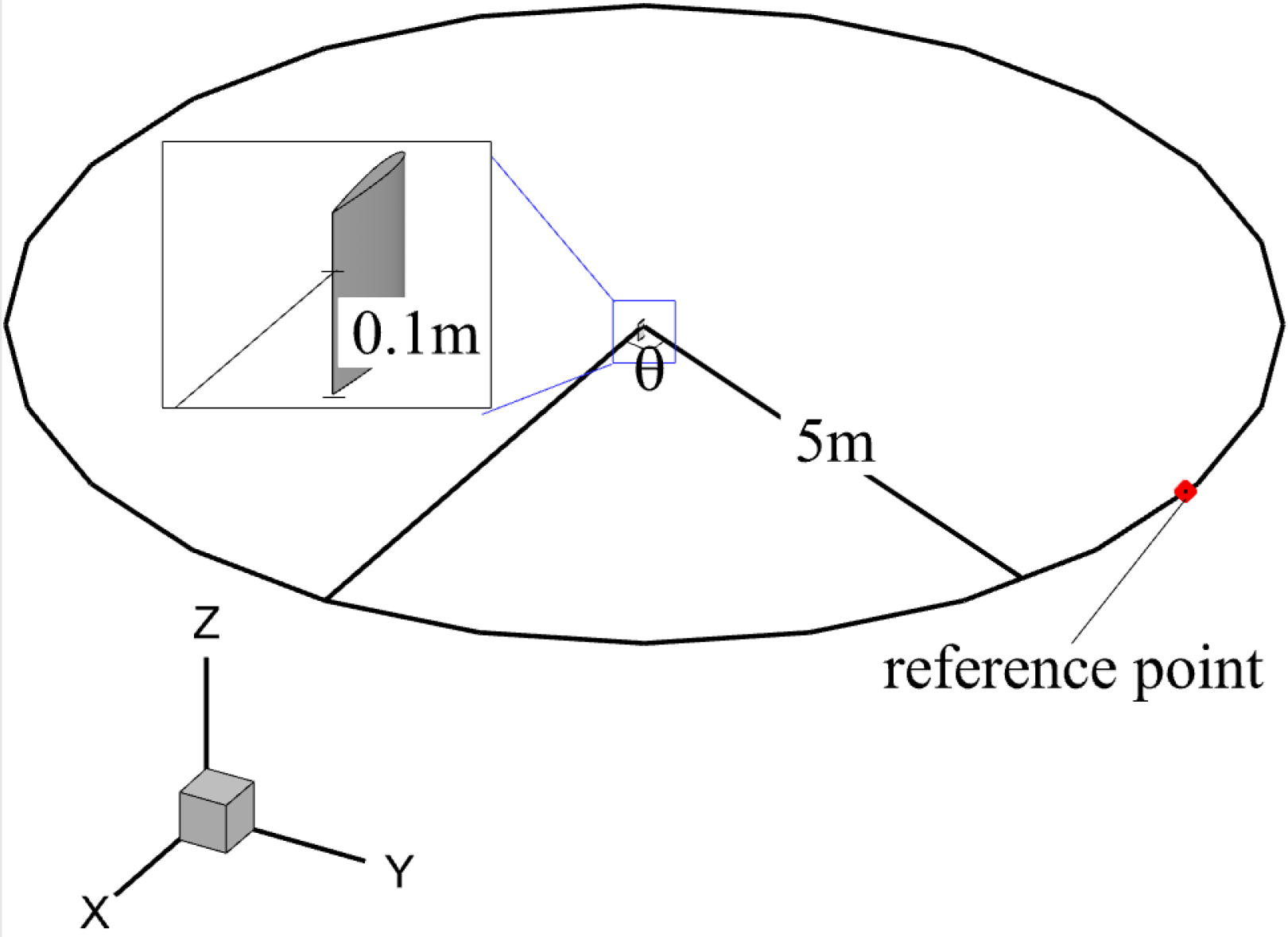
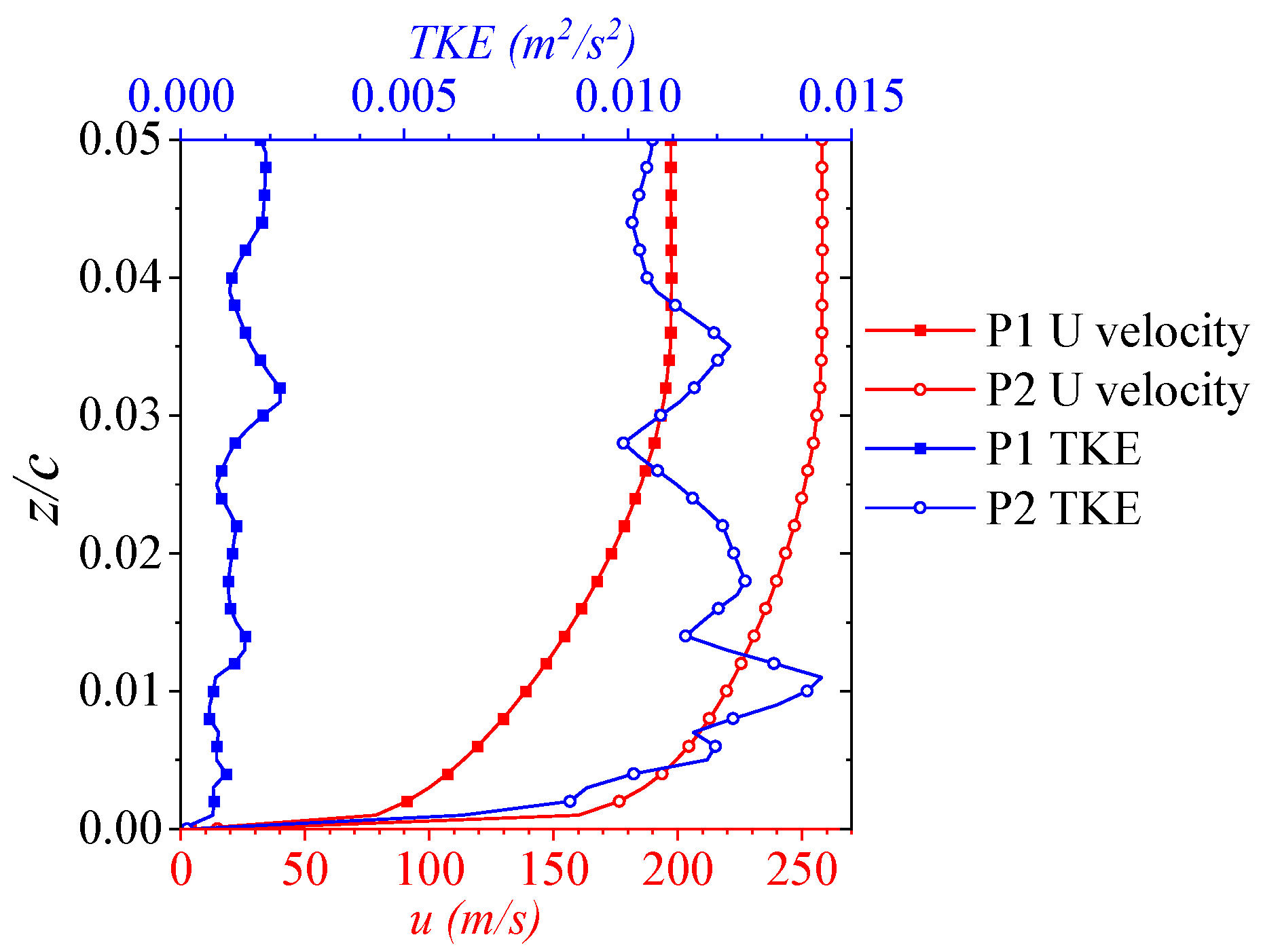
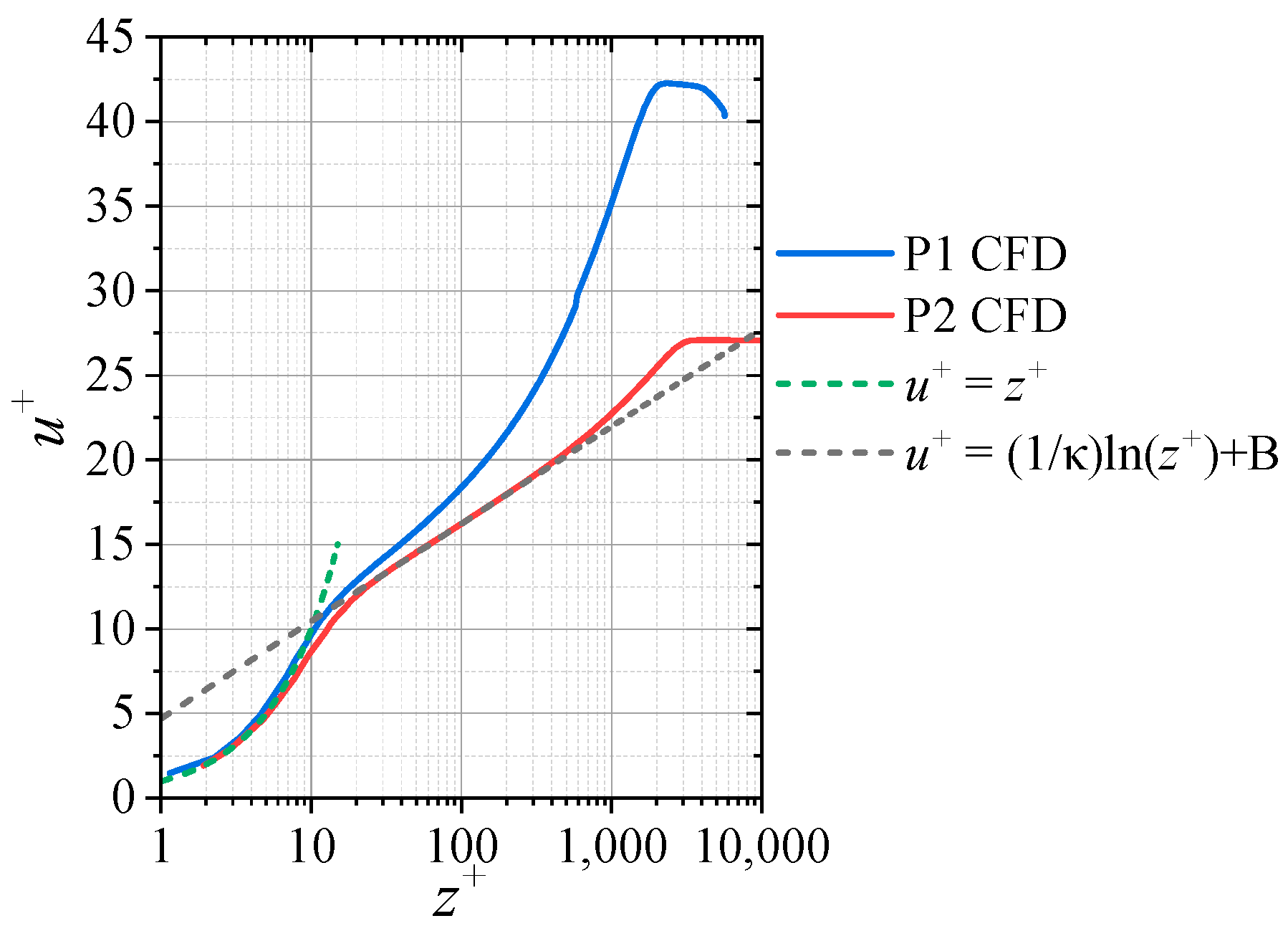
2. Model Setup and Methodology Validation
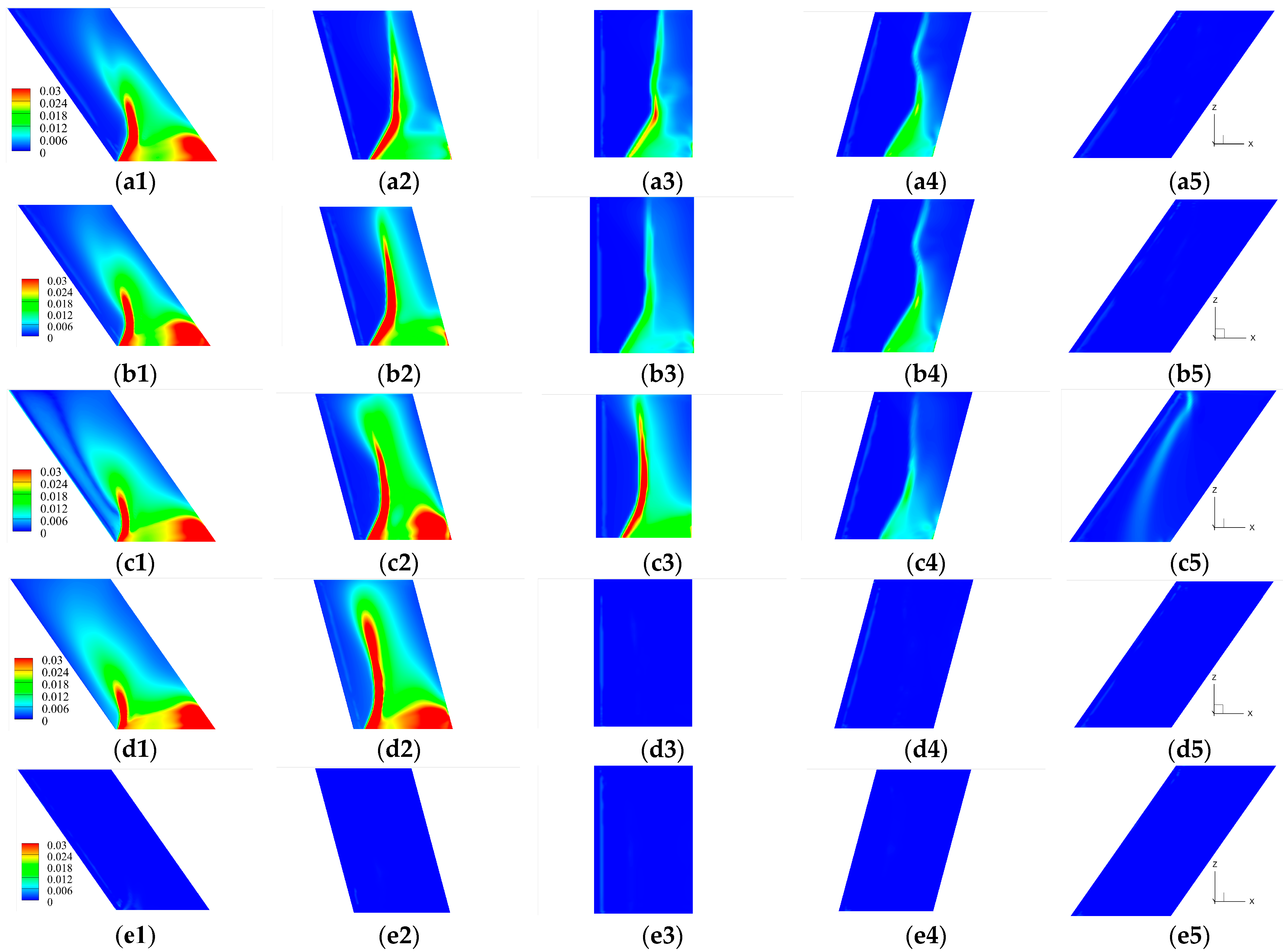
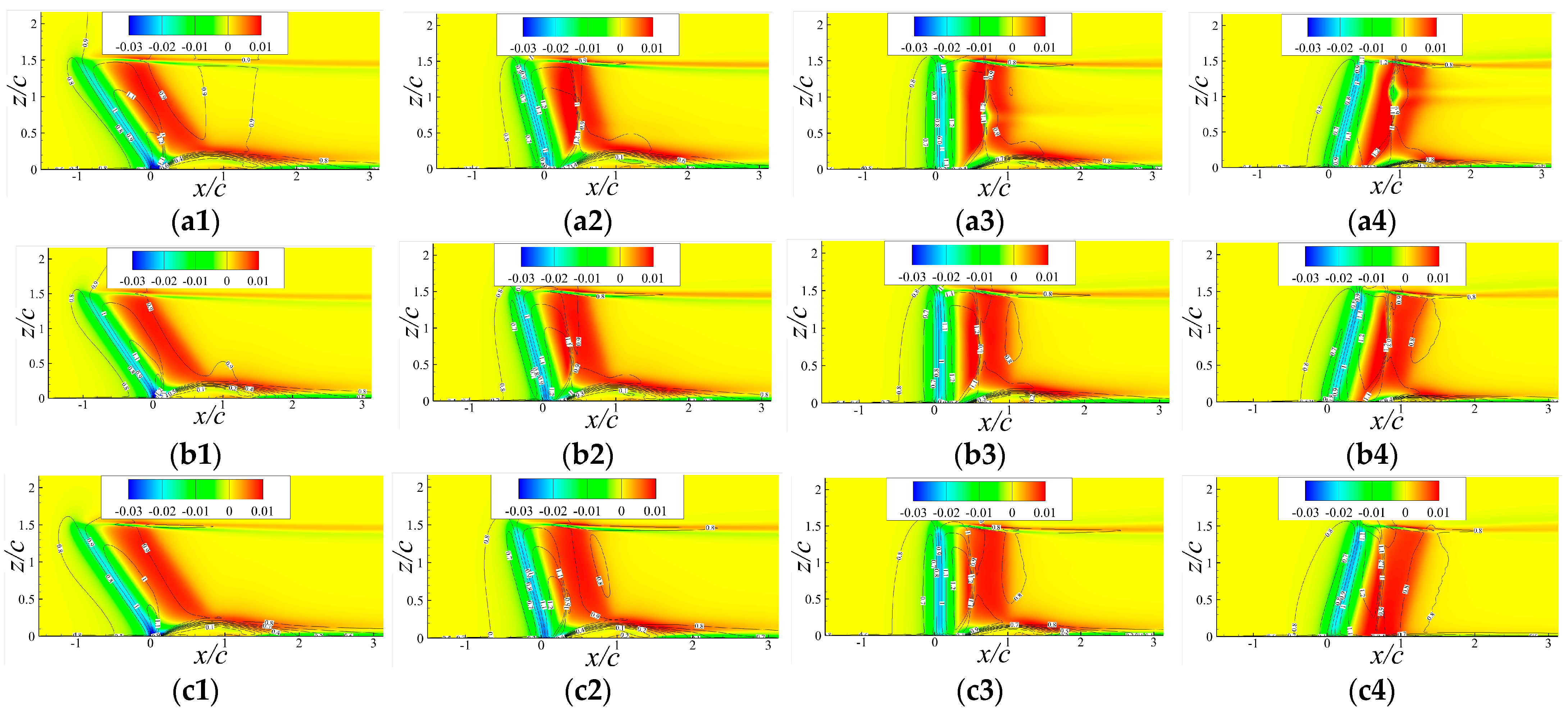
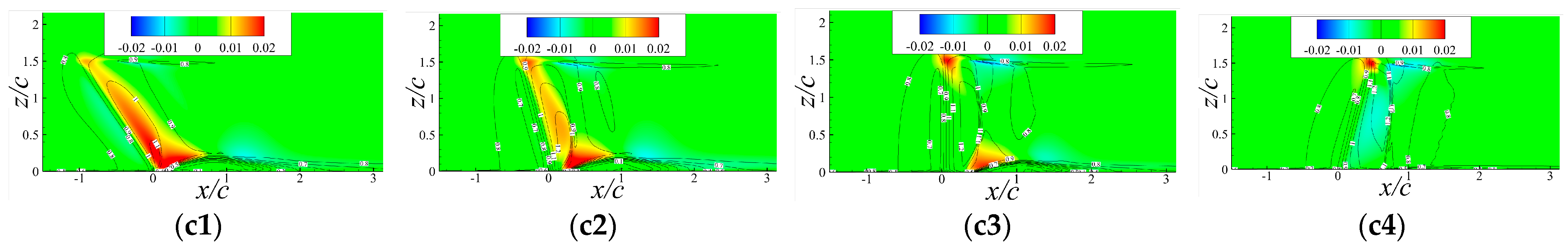
3. The Effect of Sweep Angle on Shock Waves Position, Pressure Fluctuations, and Bottom Boundary Layer
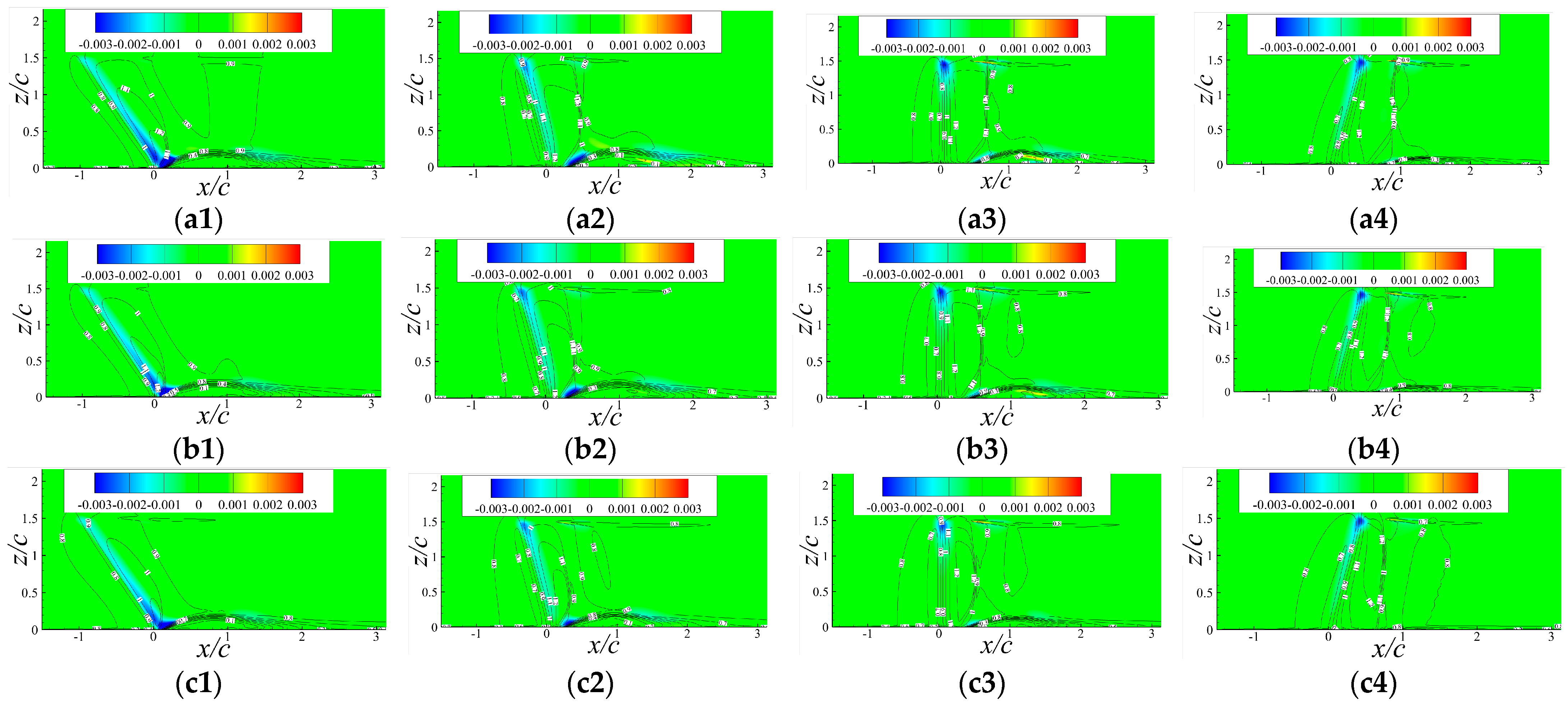
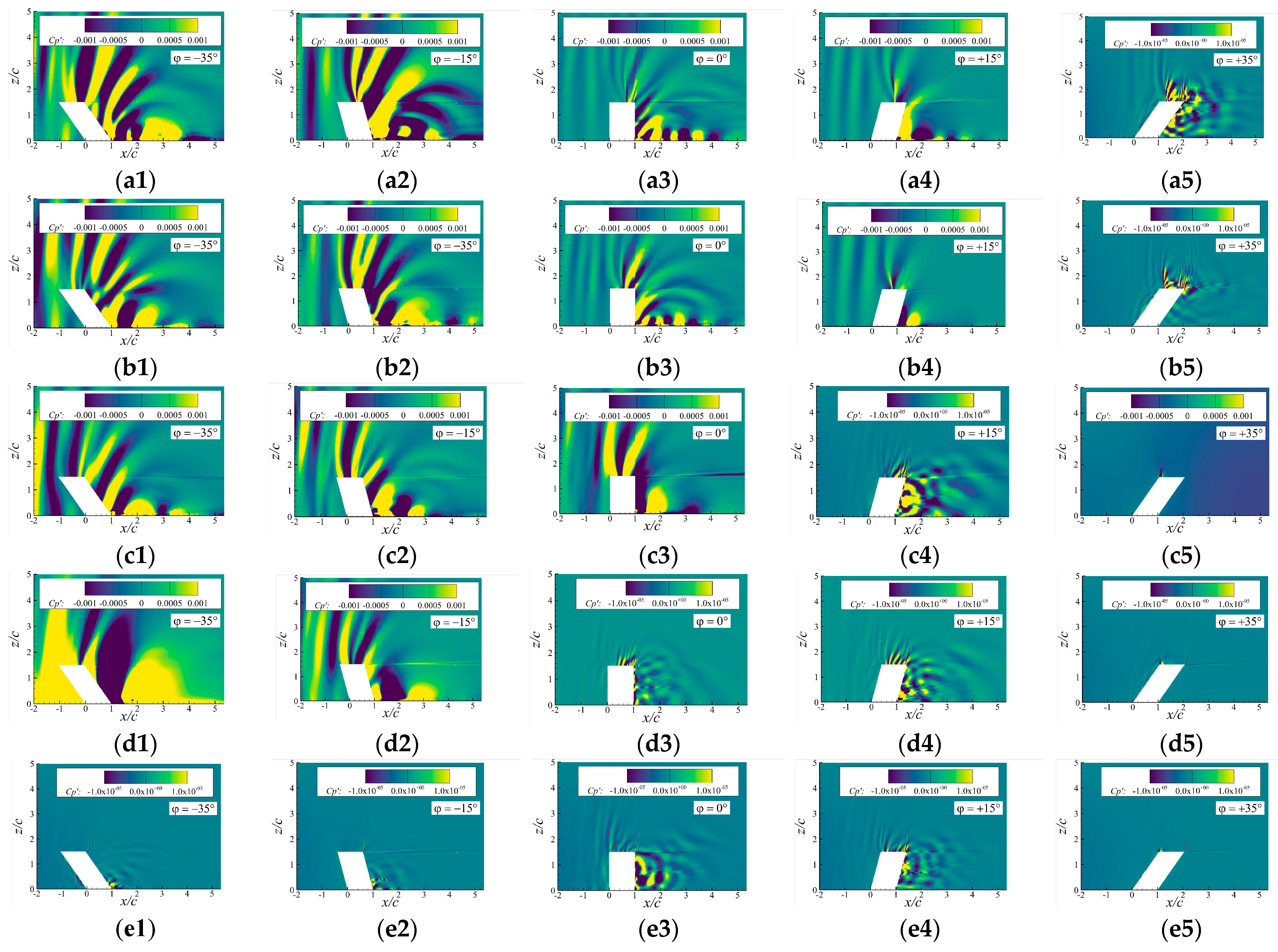
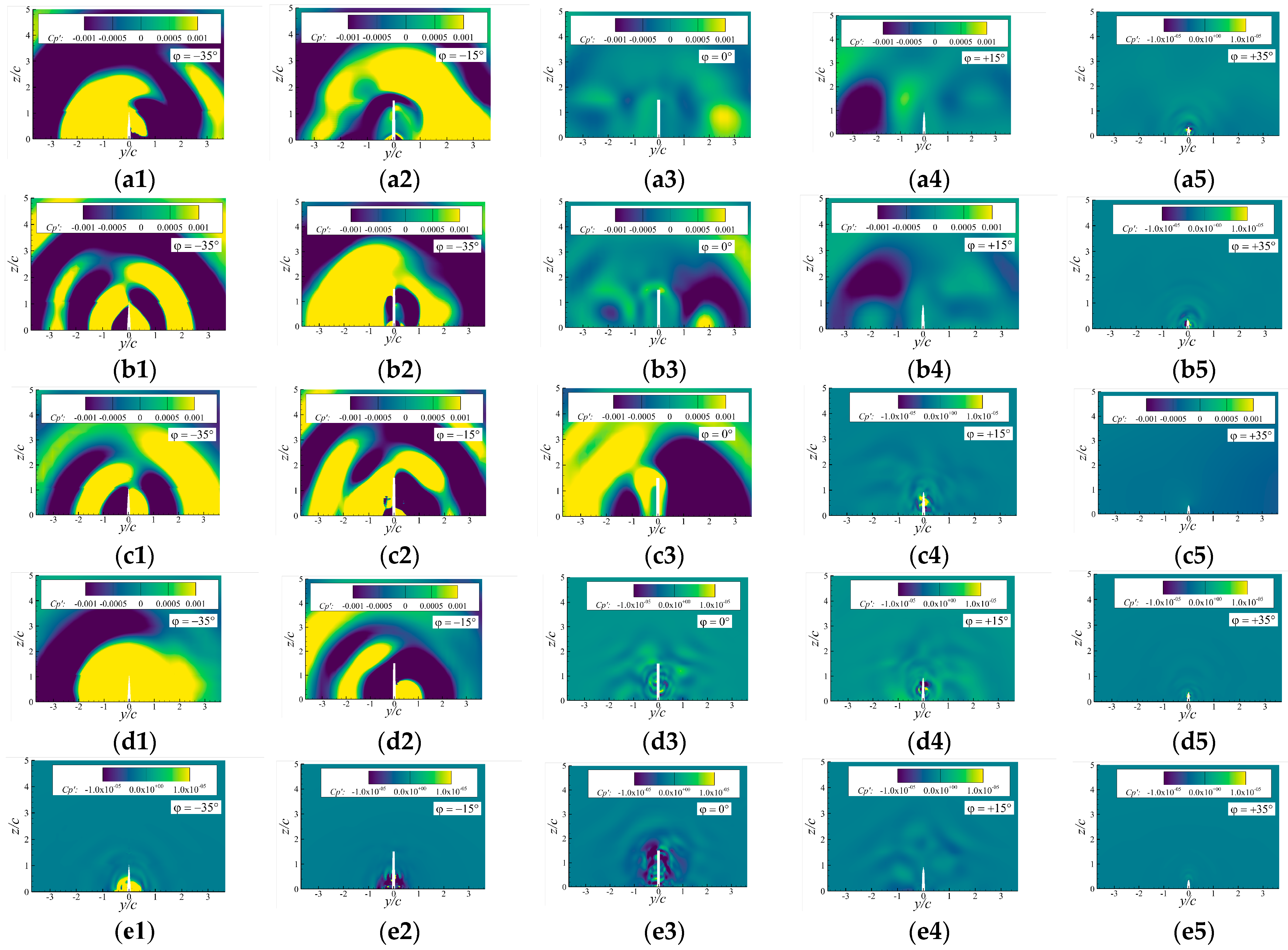
4. Pressure Wave Propagation of Near Field
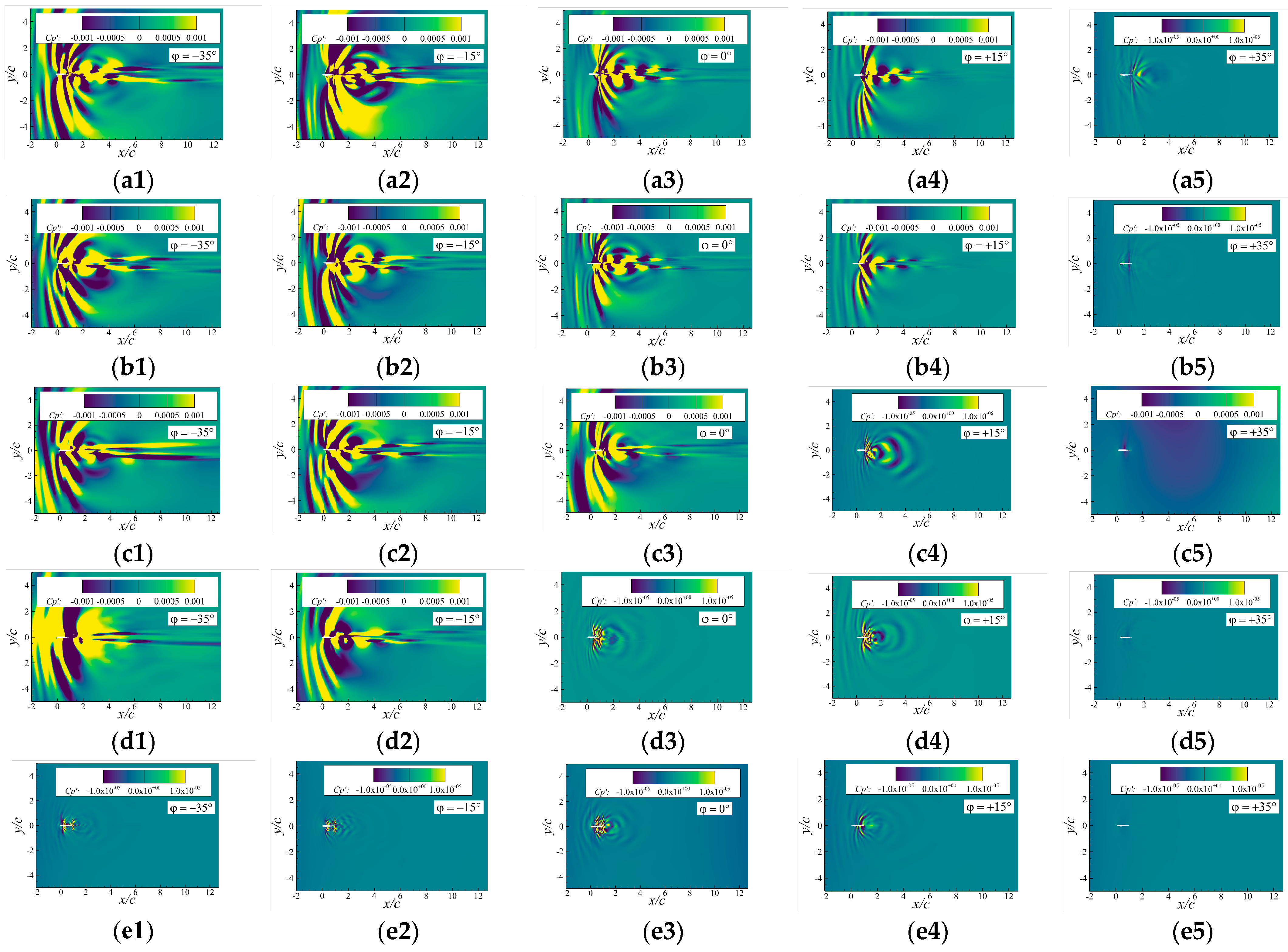
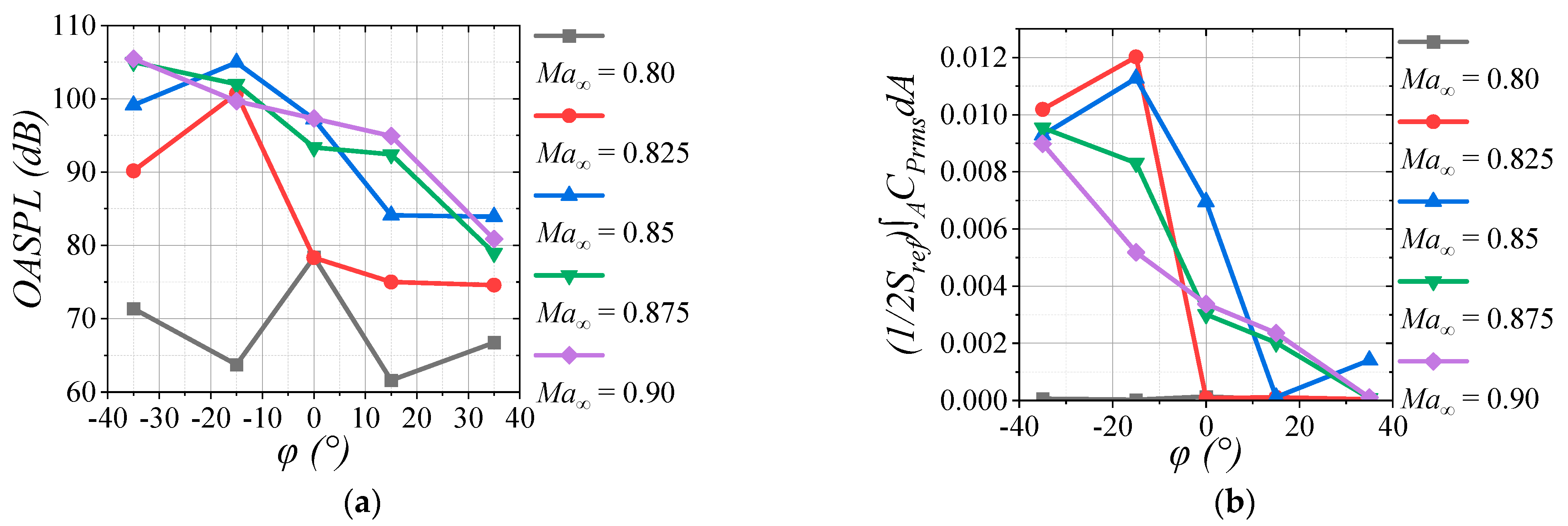
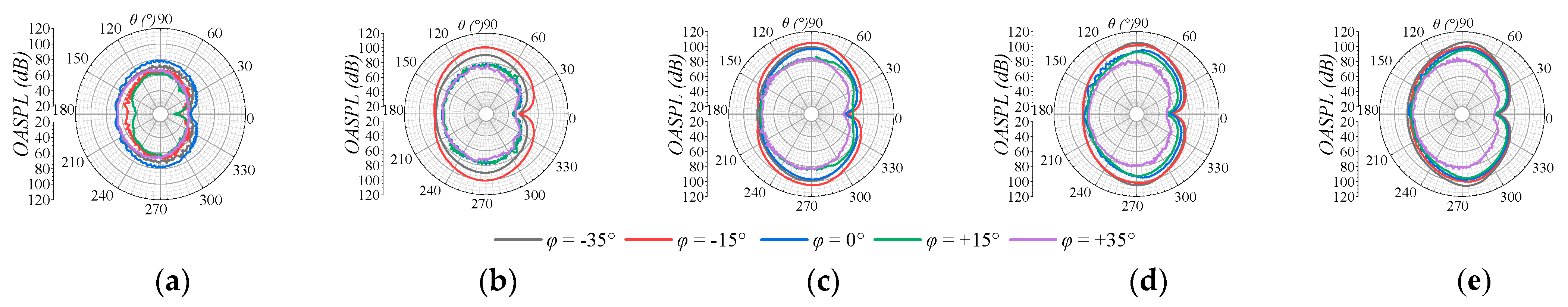
5. Far-Field Noise Directivity
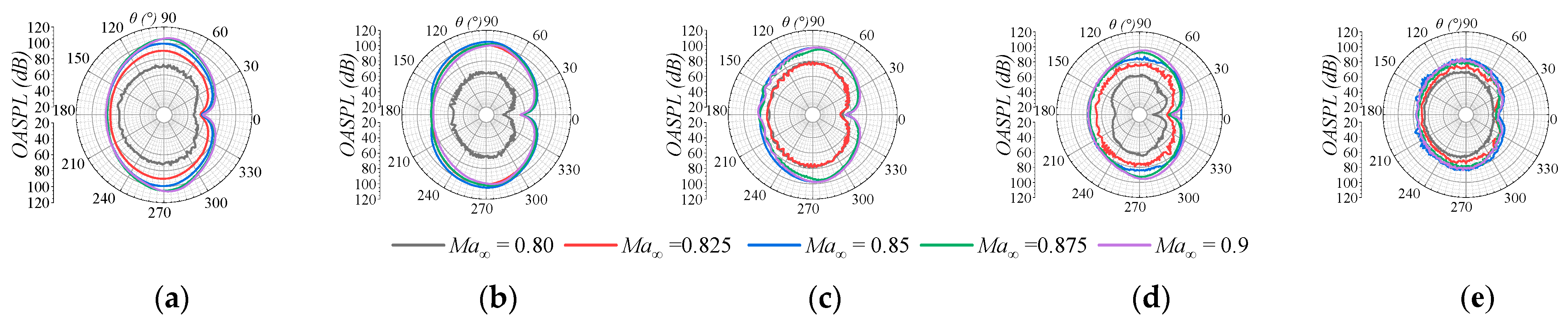
5.1. Effect of Forward and Backward Sweep Angles on Far-Field Noise Directivity
5.2. Effect of Forward and Backward Sweep Angles on Far-Field Noise Directivity
6. Conclusions
- The airfoil surface pressure fluctuation caused by the motion of the shock wave position in the junction area occurs at ≥ 0.825 for the airfoils with = −35° and −15°, at ≥ 0.85 for the airfoils with = 0°, and at ≥ 0.875 for the airfoils with = +15°. When = +35°, the airfoils do not produce flow separation at the junction. Instead, there is only a relatively weak pressure fluctuation at the tip area at = 0.85 for the airfoil of = +35°.
- When shock wave motion and boundary layer separation occur at the junction, pressure waves propagate to the side and upstream, while pressure waves propagate downstream due to vortex shedding on the bottom. When the flow at the junction is stable, turbulence occurs downstream of the entire span for the straight airfoil, in the root area for the forward-swept airfoil, and at the tip for the backward-swept airfoil.
- The pressure fluctuation on the airfoil surface is the primary source of far-field noise generated by a wall-mounted finite-span airfoil in transonic conditions. The far-field directivity of this noise exhibits obvious dipole noise characteristics, and the curve is very smooth. In addition, the amplitude of far-field noise is positively correlated with normal Mach number and the absolute values of sweep angle.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hu, N.; Buchholz, H.; Herr, M. Contributions of different aeroacoustic sources to aircraft cabin noise. In Proceedings of the 19th AIAA/CEAS Aeroacoustics Conference, Berlin, Germany, 27–29 May 2013. [Google Scholar] [CrossRef]
- Havrilesko, B.R.; Hassan, M.; Denney, R.K.; Taiw, J.C. En route jet aircraft noise analysis. In Proceedings of the AIAA/3AF Aircraft Noise and Emissions Reduction Symposium, Atlanta, GA, USA, 16–20 June 2014. [Google Scholar] [CrossRef]
- Moshkov, P. Contributions of different sources to cabin noise of a superjet 100 in cruise flight condition. In Proceedings of the AIAA AVIATION 2021 FORUM, Virtual Event, 2–6 August 2021. [Google Scholar] [CrossRef]
- Mehta, R. Effect of wing nose shape on the flow in a wing body junction. Aeronaut. J. 1984, 88, 456. [Google Scholar] [CrossRef]
- Simpson, R.L. Junction flows. Annu. Rev. Fluid Mech. 2001, 33, 415–443. [Google Scholar] [CrossRef]
- Giuni, M.; Green, R.B. Vortex formation on squared and rounded tip. Aerosp. Sci. Technol. 2013, 29, 191–199. [Google Scholar] [CrossRef]
- Karakus, C.; Akilli, H.; Sahin, B. Formation, structure, and development of the near-field wing tip vortices. Proc. Inst. Mech. Eng. Part G-J. Aerosp. Eng. 2008, 222, 13–22. [Google Scholar] [CrossRef]
- Moreau, D.J.; Brooks, L.A.; Doolan, C.J. Broadband trailing edge noise from a sharp-edged strut. J. Acoust. Soc. Am. 2011, 129, 2820–2829. [Google Scholar] [CrossRef]
- Arbey, H.; Bataille, J. Noise generated by airfoil profiles placed in a uniform laminar flow. J. Fluid Mech. 1983, 134, 33–47. [Google Scholar] [CrossRef]
- Moreau, D.J.; Prime, Z.; Porteous, R.; Doolan, C.J.; Valeau, V. Flow-induced noise of a wall-mounted finite airfoil at low-to-moderate Reynolds number. J. Sound Vib. 2014, 333, 6924–6941. [Google Scholar] [CrossRef]
- Moreau, D.J.; Doolan, C.J. Tonal noise production from a wall-mounted finite airfoil. J. Sound Vib. 2016, 363, 199–224. [Google Scholar] [CrossRef]
- Brooks, T.F.; Hodgson, T.H. Trailing edge noise prediction from measured surface pressures. J. Sound Vib. 1981, 78, 69–117. [Google Scholar] [CrossRef]
- Nash, E.C.; Lowson, M.V.; McAlpine, A. Boundary-layer instability noise on aerofoils. J. Fluid Mech. 1999, 382, 27–61. [Google Scholar] [CrossRef]
- McAlpine, A.; Nash, E.C.; Lowson, M.V. On the generation of discrete frequency tones by the flow around an aerofoil. J. Sound Vib. 1999, 222, 753–779. [Google Scholar] [CrossRef]
- Moreau, D.J.; Doolan, C.J. An Investigation of the Tonal Noise Produced by a Wall-Mounted Finite Airfoil at Angle of Attack, 22nd ed.; AIAA, Ed.; AIAA/CEAS: Lyon, France, 2016. [Google Scholar] [CrossRef]
- Geyer, T.F.; Moreau, D.J. A study of the effect of airfoil thickness on the tonal noise generation of finite, wall-mounted airfoils. Aerosp. Sci. Technol. 2021, 115, 106768. [Google Scholar] [CrossRef]
- Moreau, D.J.; Geyer, T.F.; Doolan, C.J.; Sarradj, E. Camber effects on the tonal noise and flow characteristics of a wall-mounted finite airfoil. In Proceedings of the 23rd AIAA/CEAS Aeroacoustics Conference, Denver, Colorado, 5–9 June 2017; pp. 2017–3172. [Google Scholar] [CrossRef]
- Moreau, D.J.; Geyer, T.F.; Doolan, C.J.; Sarradj, E. Surface curvature effects on the tonal noise of a wall-mounted finite airfoil. J. Acoust. Soc. Am. 2018, 143, 3460–3473. [Google Scholar] [CrossRef]
- Zhang, T.; Geyer, T.F.; Silva, C.; Fischer, J.R.; Doolan, C.J.; Moreau, D.J. Experimental investigation of tip vortex formation noise produced by wall-mounted finite airfoils. J. Aerosp. Eng. 2021, 34, 4021079. [Google Scholar] [CrossRef]
- Giez, J.; Vion, L.; Roger, M.; Moreau, S. Effect of the edge and tip vortex on airfoil self-noise and Turbulence Impingement Noise. In Proceedings of the 22nd AIAA/CEAS Aeroacoustics Conference, Lyon, France, 30 May–1 June 2016; AIAA Paper. pp. 2016–2996. [Google Scholar] [CrossRef]
- Yakhina, G.R.; Roger, M.; Finez, A.; Bouley, S.; Baron, V.; Moreau, S.; Giez, J. Localization of swept free-tip airfoil noise sources by microphone array processing. AIAA J. 2020, 58, 3414–3425. [Google Scholar] [CrossRef]
- Grasso, G.; Roger, M.; Moreau, S. Effect of sweep angle and of wall-pressure statistics on the free-field directivity of airfoil trailing-edge noise. In Proceedings of the 25th AIAA/CEAS Aeroacoustics Conference, Delft, The Netherlands, 20–23 May 2019; AIAA Paper. pp. 2019–2612. [Google Scholar] [CrossRef]
- Grasso, G.; Roger, M.; Moreau, S. Analytical model of the source and radiation of sound from the trailing edge of a swept airfoil. J. Sound Vib. 2021, 493, 115838. [Google Scholar] [CrossRef]
- Koch, R.; Sanjosé, M.; Moreau, S. Acoustic investigation of the transonic RAE 2822 airfoil with large-eddy simulation. In Proceedings of the 28th AIAA/CEAS Aeroacoustics Conference, Southampton, UK, 14–17 June 2022; AIAA Paper. pp. 2022–2816. [Google Scholar] [CrossRef]
- Alguacil, A.; Becherucci, L.; Sanjosé, M.; Moreau, S. Broadband noise of the transonic RAE 2822 Airfoil. In Proceedings of the AIAA AVIATION Forum, San Diego, CA, USA, 12–16 June 2023; AIAA Paper. pp. 2023–3635. [Google Scholar] [CrossRef]
- Zhang, Q.; Gao, C.; Zhou, F.; Yang, D.; Zhang, W. Study on flow noise characteristic of transonic deep buffeting over an airfoil. Phys. Fluids 2023, 35, 046109. [Google Scholar] [CrossRef]
- Zhong, S.Y.; Zhang, X.; Gill, J.; Fattah, R.; Sun, Y.H. A numerical investigation of the airfoil-gust interaction noise in transonic flows: Acoustic processes. J. Sound Vib. 2018, 425, 239–256. [Google Scholar] [CrossRef]
- Zhong, S.Y.; Zhang, X.; Gill, J.; Fattah, R.; Sun, Y.H. Geometry effect on the airfoil-gust interaction noise in transonic flows. Aerosp. Sci. Technol. 2019, 92, 181–191. [Google Scholar] [CrossRef]
- Ruban, A.I.; Bernots, T.; Kravtsova, M.A. Linear and nonlinear receptivity of the boundary layer in transonic flows. J. Fluid Mech. 2016, 786, 154–189. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, J.; Qiao, L.; Zhang, Y.; Junqiang Bai, J. Improved Amplification Factor Transport Transition Model for Transonic Boundary Layers. AIAA J. 2023, 61, 2022–4030. [Google Scholar] [CrossRef]
- Clemens, N.T.; Narayanaswamy, V. Low-frequency unsteadiness of shock wave/turbulent boundary layer interactions. Annu. Rev. Fluid Mech. 2014, 46, 469–492. [Google Scholar] [CrossRef]
- Gaitonde, D.V. Progress in shock wave/boundary layer interactions. Prog. Aeosp. Sci. 2015, 72, 80–99. [Google Scholar] [CrossRef]
- Gaitonde, D.V.; Adler, M.C. Dynamics of three-dimensional shock-wave/boundary-layer interactions. Annu. Rev. Fluid Mech. 2023, 55, 291–321. [Google Scholar] [CrossRef]
- Tô, J.B.; Simiriotis, N.; Marouf, A.; Szubert, D.; Asproulias, I.; Zilli, D.M.; Hoarau, Y.; Hunt, J.C.R.; Braza, M. Effects of vibrating and deformed trailing edge of a morphing supercritical airfoil in transonic regime by numerical simulation at high Reynolds number. J. Fluids Struct. 2019, 91, 102595. [Google Scholar] [CrossRef]
- Szulc, O.; Doerffer, P.; Flaszynski, P.; Suresh, T. Numerical modelling of shock wave-boundary layer interaction control by passive wall ventilation. Comput. Fluids 2020, 200, 104435. [Google Scholar] [CrossRef]
- Poplingher, L.; Raveh, D.E. Comparative Modal Study of the Two-Dimensional and Three-Dimensional Transonic Shock Buffet. AIAA J. 2023, 61, 2022–2483. [Google Scholar] [CrossRef]
- D’Aguanno, A.; Schrijer, F.F.J.; Oudheusden, B.W. Finite-Wing and Sweep Effects on Transonic Buffet Behavior. AIAA J. 2022, 60, 6715–6725. [Google Scholar] [CrossRef]
- Gleize, V.; Dumont, A.; Mayeur, J.; Destarac, D. RANS simulations on TMR test cases and M6 wing with the Onera elsA flow solver. Turbulent Flow. In Proceedings of the Solutions for NACA 0012 and Other Test Cases from the Turbulence Model Resource Website: Residual and Grid Convergence II, Kissimmee, FL, USA, 5–9 January 2015; AIAA Paper. pp. 1745–2015. [Google Scholar] [CrossRef]
- Mayeur, J.; Dumont, A.; Gleize, V.; Destarac, D. RANS simulations on TMR 3D test cases with the Onera elsA flow solver. In Proceedings of the Special Session: Evaluation of RANS Solvers on Benchmark Aerodynamic Flows II, San Diego, CA, USA, 4–8 January 2016; AIAA Paper. pp. 1357–2016. [Google Scholar] [CrossRef]










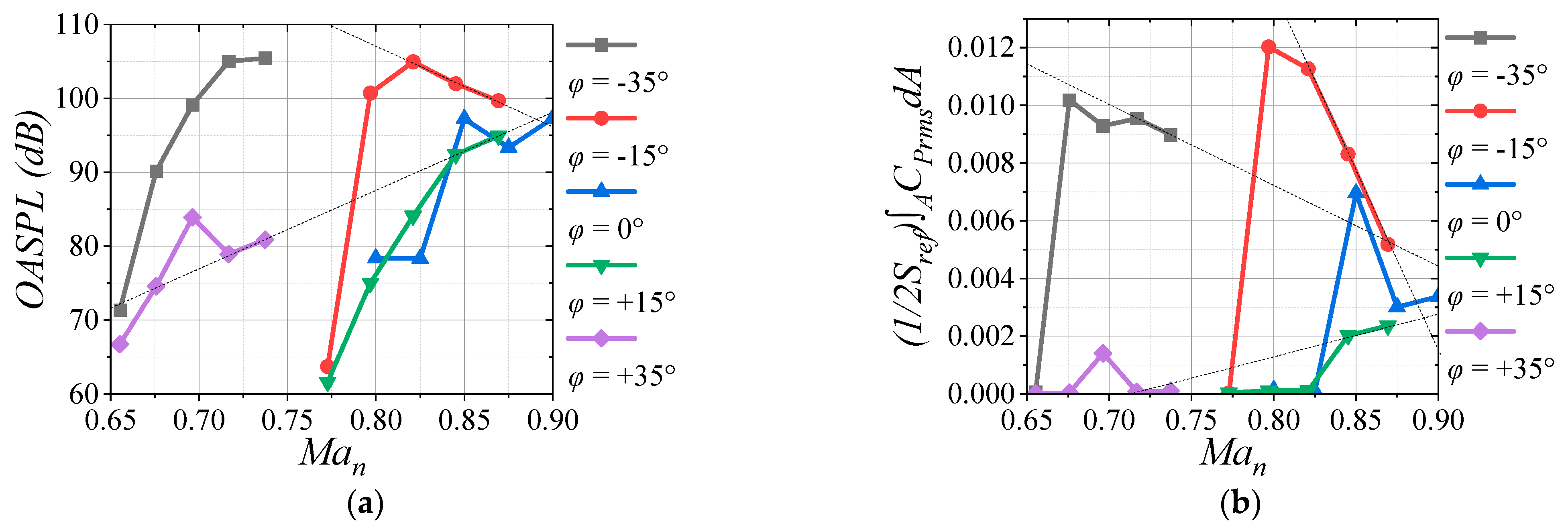
| Type 1 | Supplier | Aircraft | ||||
|---|---|---|---|---|---|---|
| S65-8280-18 BA | Sensor Systems Inc., Chatsworth, CA, USA | +46° | 0.694659 | 1.09 | ||
| 20-200-20 BA | Chelton Ltd., Marlow, UK | +45° | 0.707107 | 1.16 | ||
| 110-337 BA | ACR Artex, Fort Lauderdale, FL, USA | +38° | 0.788011 | 0.83 | ||
| ANT500 BA | Mc Murdo Group, Lanham, MD, USA | +37° | 0.798636 | 1.15 | ||
| S65-8262-305 BA | Sensor Systems Inc., Chatsworth, CA, USA | +36° | 0.809017 | 1.4 | ||
| 20-200-F18LP BA | Chelton Ltd., Marlow, UK | +30° | 0.866025 | 1.89 | ||
| 21-30-176 BA | Cooper Antennas Ltd., Marlow, UK | +30° | 0.866025 | 1.2 | ||
| 0851HLAI PT | Aero-Instruments, Depew, NY, USA | A320 | 0.785 | −25° | 0.906308 | 1 |
| 0851HT1AI PT | AeroControlex, South Euclid, OH, USA | B737 | 0.74 | −25° | 0.906308 | 1 |
| 0856AE19AI PT | AeroControlex, South Euclid, OH, USA | B737NG | 0.785 | −35° | 0.819152 | 1.5 |
| 0851FJ1AI PT | Aero-Instruments, Depew, NY, USA | B757 | 0.8 | −35° | 0.819152 | 0.7 |
| PT | -- | B747 | 0.85 | −45° | 0.707107 | 1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, R.; Liu, P.; Zhang, J.; Guo, H. Numerical Study on Far-Field Noise Characteristic Generated by Wall-Mounted Swept Finite-Span Airfoil within Transonic Flow. Aerospace 2024, 11, 645. https://doi.org/10.3390/aerospace11080645
Jiang R, Liu P, Zhang J, Guo H. Numerical Study on Far-Field Noise Characteristic Generated by Wall-Mounted Swept Finite-Span Airfoil within Transonic Flow. Aerospace. 2024; 11(8):645. https://doi.org/10.3390/aerospace11080645
Chicago/Turabian StyleJiang, Runpei, Peiqing Liu, Jin Zhang, and Hao Guo. 2024. "Numerical Study on Far-Field Noise Characteristic Generated by Wall-Mounted Swept Finite-Span Airfoil within Transonic Flow" Aerospace 11, no. 8: 645. https://doi.org/10.3390/aerospace11080645
APA StyleJiang, R., Liu, P., Zhang, J., & Guo, H. (2024). Numerical Study on Far-Field Noise Characteristic Generated by Wall-Mounted Swept Finite-Span Airfoil within Transonic Flow. Aerospace, 11(8), 645. https://doi.org/10.3390/aerospace11080645






