Calibration of a Load Measurement System for an Unmanned Aircraft Composite Wing Based on Fibre Bragg Gratings and Electrical Strain Gauges
Abstract
1. Introduction
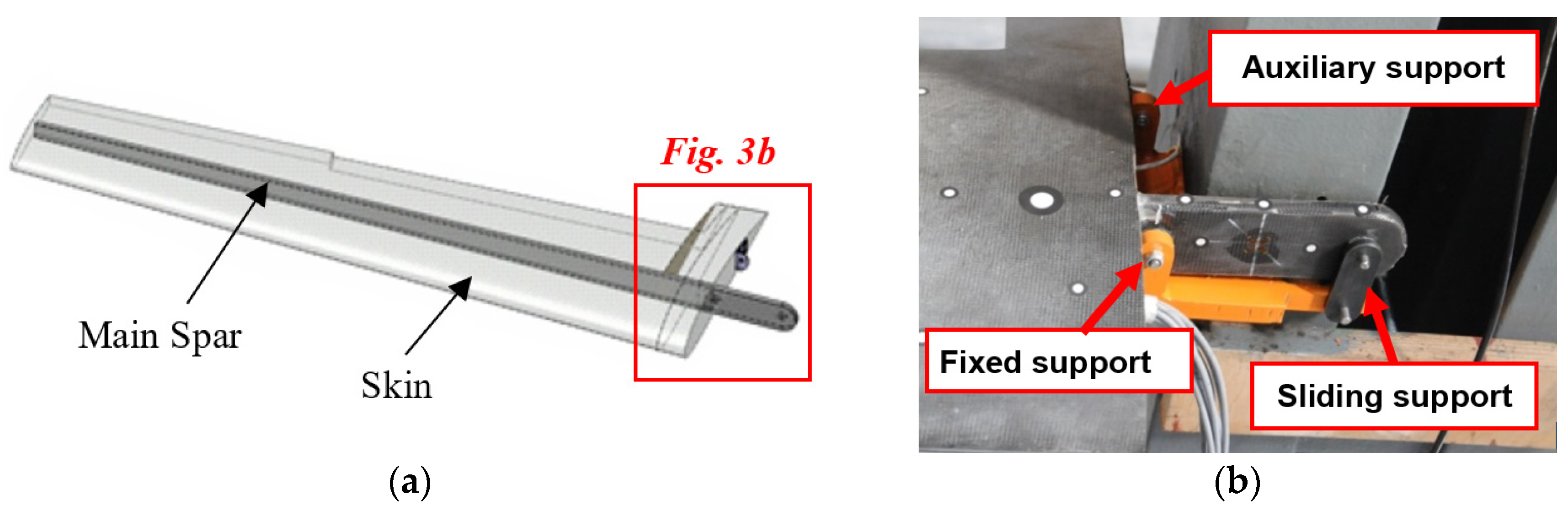
2. Tested Structure
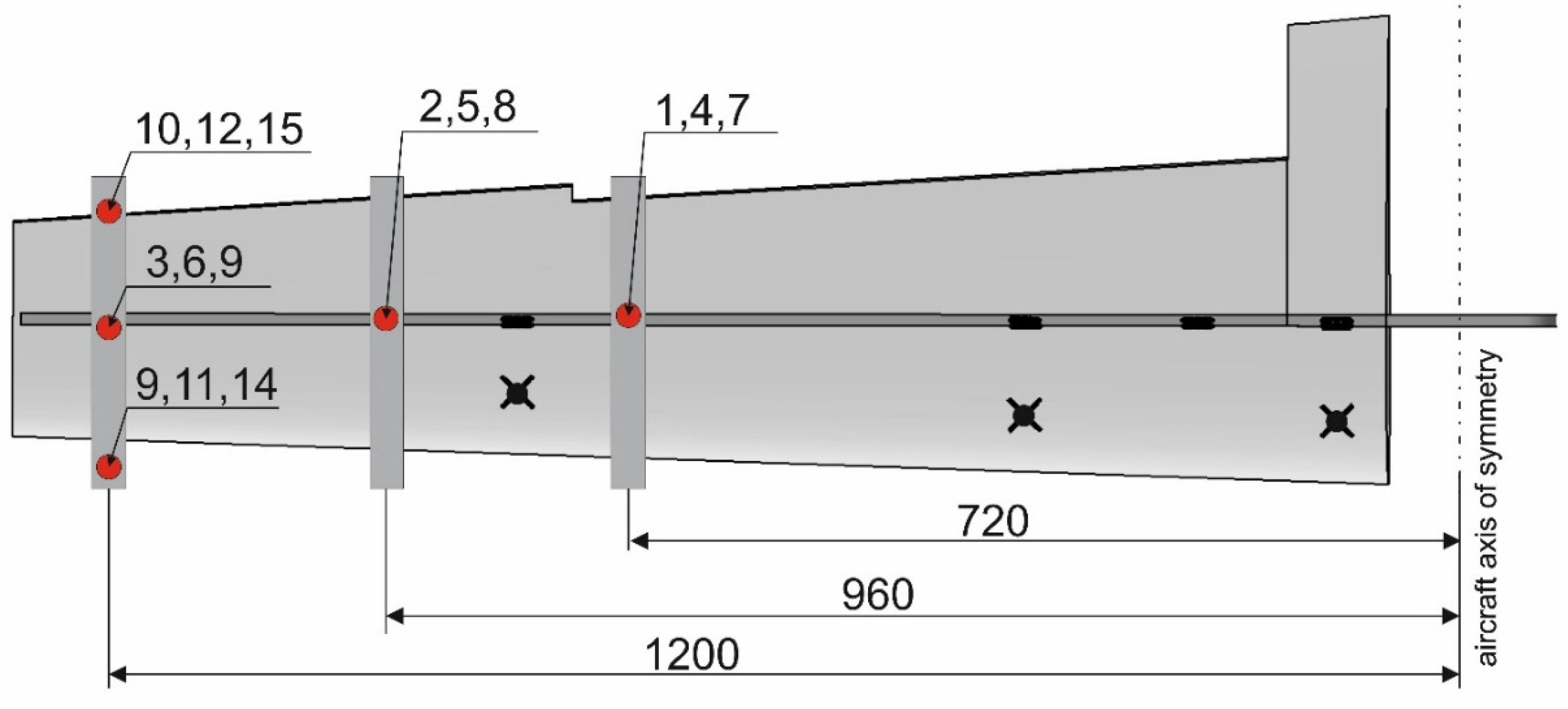
3. Measurement System
4. Measurement System Calibration
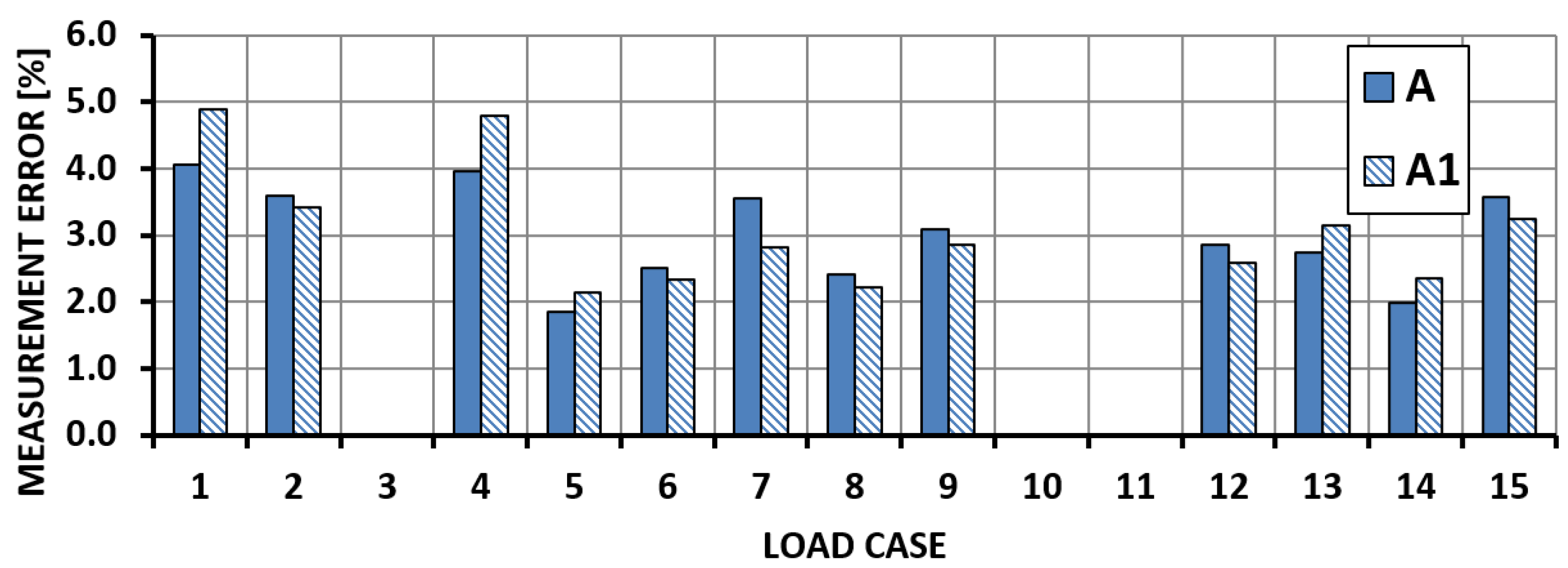
5. Measurement Results
5.1. Calibration Cases
- Calibration with the use of one full measurement system: System A
- Calibration with the use of only the bridges for the measurement of shear force and bending moment: System B
- Calibration with the use of individual bridges—individual measurements of shear force, bending moment and torsional moment: System C
5.2. System Calibration for Resistance Strain Gauges (Sections II and III)
5.3. System Calibration for BRAGG FIBRE STRAIN GAUGES (OPT Section)
6. Summary and Conclusions
Funding
Conflicts of Interest
References
- Schrenk, O. A Simple Approximation Method for Obtaining the Spanwise Lift Distribution. J. R. Aeronaut. Soc. 1941, 45, 331–336. [Google Scholar] [CrossRef]
- Aoki, Y.; Ishikawa, T.; Takeda, S.-I.; Hayakawa, Y.; Harada, A.; Kikukawa, H. Fatigue test of lightweight composite wing structure. Int. J. Fatigue 2006, 28, 1109–1115. [Google Scholar] [CrossRef]
- Baraniecki, R.; Kaniewska, M.; Leski, A. Fatigue Life Assessment of Selected Structural Elements of MI-24 Helicopter. Fatigue Aircr. Struct. 2010, 2010, 5–13. [Google Scholar] [CrossRef][Green Version]
- Diamanti, K.; Soutis, C. Structural health monitoring techniques for aircraft composite structures. Prog. Aerosp. Sci. 2010, 46, 342–352. [Google Scholar] [CrossRef]
- Reymer, P. Validation of the FEM model of the Mi-24 tail boom and vertical stabilizer. J. KONES Powertrain Transp. 2011, 18. [Google Scholar]
- Takeda, S.-I.; Aoki, Y.; Ishikawa, T.; Takeda, N.; Kikukawa, H. Structural health monitoring of composite wing structure during durability test. Compos. Struct. 2007, 79, 133–139. [Google Scholar] [CrossRef]
- Brzoska, Z. Statyka i Stateczność Konstrukcji Prętowych i Cienkościennych; PWN: Warsaw, Poland, 1965. [Google Scholar]
- Megson, T.H.G. Introduction to Aircraft Structural Analysis, 2nd ed.; Butterworth-Heinemann: Oxford, UK, 2014. [Google Scholar]
- Skopinski, T.; Aiken, W.; Huston, W. Calibration of Strain-Gauge Installation in Aircraft Structures for the Measurements of Flight Loads; NACA Technical Note 2993; NACA: Washington, DC, USA, 1953. [Google Scholar]
- Eckstorm, C. Flight Loads Measurements Obtained from Calibrated Strain-Gage Bridges Mounted Externally on Skin of Low-Aspect-Ratio Wing; NASA TN D-8349; NASA: Washington, DC, USA, 1976. [Google Scholar]
- Hovell, P.; Webber, D.; Roberts, T. The use of Calibrated Strain Gauges for Flight Load Determination; Aeronautical Research Council Technical Report 1041; NASA: Washington, DC, USA, 1969. [Google Scholar]
- Jenkins, J.; DeAngelis, V. A Summary of Numerous Strain-Gauge Load Calibrations on Aircraft Wings and Tails in a Technology Format; NASA Technical Memorandum 4804; NASA: Washington, DC, USA, 1997. [Google Scholar]
- Lokos, W.; Stauf, R. Strain-Gage Calibration Parametric Study; NASA TM 212853; NASA: Washington, DC, USA, 2004. [Google Scholar]
- Monaghan, R.; Fields, R. Experiments to Study Strain-Gage Load Calibrations on Wing Structure at Elevated Temperatures; NASA TN D-7390; NASA: Washington, DC, USA, 1973. [Google Scholar]
- Bao, P.; Yuan, M.; Dong, S.; Song, H.; Xue, J. Fiber Bragg grating sensor fatigue crack real-time monitoring based on spectrum cross-correlation analysis. J. Sound Vib. 2013, 332, 43–57. [Google Scholar] [CrossRef]
- Grouve, W.; Warnet, L.; de Boer, A.; Akkerman, R.; Vlekken, J. Delamination detection with fibre Bragg gratings based on dynamic behavior. Compos. Sci. Technol. 2008, 68, 2418–2424. [Google Scholar] [CrossRef]
- Takeda, N.; Okabe, Y.; Kuwahara, J.; Kojima, S.; Ogisu, T. Development of smart composite structures with small-diameter fiber Bragg grating sensors for damage detection: Quantitative evaluation of delamination length in CFRP laminates using Lamb wave sensing. Compos. Sci. Technol. 2005, 65, 2575–2587. [Google Scholar] [CrossRef]
- Mulle, M.; Zitoune, R.; Collombet, F.; Robert, L.; Grunevald, Y.-H. Embedded FBGs and 3-D DIC for the stress analysis of a structural specimen subjected to bending. Compos. Struct. 2009, 91, 48–55. [Google Scholar] [CrossRef]
- Rao, Y. Recent progress in applications of in-fibre Bragg grating sensors. Opt. Lasers Eng. 1999, 31, 297–324. [Google Scholar] [CrossRef]
- Antonucci, V.; Esposito, M.; Ricciardi, M.; Giordano, M.; Zarrelli, M. Strain monitoring of composite elements by fibre Bragg grating sensors during a quasi-static indentation. Compos. Part B Eng. 2014, 56, 34–41. [Google Scholar] [CrossRef]
- Minakuchi, S.; Takeda, N.; Takeda, S.-I.; Nagao, Y.; Franceschetti, A.; Liu, X. Life cycle monitoring of large-scale CFRP VARTM structure by fiber-optic-based distributed sensing. Compos. Part A Appl. Sci. Manuf. 2011, 42, 669–676. [Google Scholar] [CrossRef]
- Papantoniou, A.; Rigas, G.; Alexopoulos, N.D.; Rigas, G.-P. Assessment of the strain monitoring reliability of fiber Bragg grating sensor (FBGs) in advanced composite structures. Compos. Struct. 2011, 93, 2163–2172. [Google Scholar] [CrossRef]
- Iadicicco, A.; Natale, D.; Di Palma, P.; Spinaci, F.; Apicella, A.; Campopiano, S. Strain Monitoring of a Composite Drag Strut in Aircraft Landing Gear by Fiber Bragg Grating Sensors. Sensors 2019, 19, 2239. [Google Scholar] [CrossRef] [PubMed]
- Orkisz, M.; Święch, Ł.; Zacharzewski, J. Fatigue tests of motor glider wing’s composite spar. Maint. Reliab. 2012, 14, 228–232. [Google Scholar]
- Ramly, R.; Kuntjoro, W.; Rahman, M.K.A. Using Embedded Fiber Bragg Grating (FBG) Sensors in Smart Aircraft Structure Materials. Procedia Eng. 2012, 41, 600–606. [Google Scholar] [CrossRef]
- Tserpes, K.I.; Karachalios, V.; Giannopoulos, I.; Prentzias, V.; Ruzek, R. Strain and damage monitoring in CFRP fuselage panels using fiber Bragg grating sensors. Part I: Design, manufacturing and impact testing, Compos. Struct. 2014, 107, 726–736. [Google Scholar]
- Růžek, R.; Kudrna, P.; Kadlec, M.; Karachalios, V.; Tserpes, K. Strain and damage monitoring in CFRP fuselage panels using fiber Bragg grating sensors. Part II: Mechanical testing and validation. Compos. Struct. 2014, 107, 737–744. [Google Scholar] [CrossRef]
- Ryu, C.; Lee, J.; Kim, C.; Hong, C. Buckling behaviour monitoring of composite wing box using multiplexed and multi-channeled built-in fiber Bragg grating strain sensor. NDT&E Int. 2008, 41, 534–543. [Google Scholar]
- Ma, Z.; Chen, X. Fiber Bragg Gratings Sensors for Aircraft Wing Shape Measurement: Recent Applications and Technical Analysis. Sensors 2018, 19, 55. [Google Scholar] [CrossRef] [PubMed]
- Nicolas, M.; Sullivan, R.W.; Richards, W.L. Large Scale Applications Using FBG Sensors: Determination of In-Flight Loads and Shape of a Composite Aircraft Wing. Aerospace 2016, 3, 18. [Google Scholar] [CrossRef]












| SECTION III | SECTION II | OPT SECTION | |||||
|---|---|---|---|---|---|---|---|
| Load Case | V | M | T | M | T | M | T |
| [N] | [Nm] | [Nm] | [Nm] | [Nm] | [Nm] | [Nm] | |
| 1 | 1.0 V | 1.0 M | 0.0 | 0.5 M | 0.0 | 0.8 M | 0.0 |
| 2 | 1.0 V | 1.4 M | 0.0 | 1.0 M | 0.0 | 1.2 M | 0.0 |
| 3 | 1.0 V | 1.8 M | 0.0 | 1.3 M | 0.0 | 1.6 M | 0.0 |
| 4 | 1.6 V | 1.6 M | 0.0 | 0.8 M | 0.0 | 1.2 M | 0.0 |
| 5 | 1.6 V | 2.2 M | 0.0 | 1.5 M | 0.0 | 1.9 M | 0.0 |
| 6 | 1.6 V | 2.8 M | 0.0 | 2.1 M | 0.0 | 2.5 M | 0.0 |
| 7 | 2.2 V | 2.2 M | 0.0 | 1.1 M | 0.0 | 1.7 M | 0.0 |
| 8 | 2.2 V | 3.8 M | 0.0 | 2.8 M | 0.0 | 3.4 M | 0.0 |
| 9 | 2.2 V | 3.1 M | 0.0 | 2.0 M | 0.0 | 2.6 M | 0.0 |
| 10 | 1.0 V | 1.8 M | 0.2 M | 1.3 M | 0.2 M | 1.6 M | 0.2 M |
| 11 | 1.0 V | 1.4 M | −0.2 M | 1.0 M | −0.2 M | 1.2 M | −0.2 M |
| 12 | 1.6 V | 2.8 M | 0.4 M | 2.1 M | 0.4 M | 2.5 M | 0.4 M |
| 13 | 1.6 V | 2.2 M | −0.4 M | 1.5 M | −0.4 M | 1.9 M | −0.4 M |
| 14 | 2.2 V | 3.8 M | 0.5 M | 2.8 M | 0.5 M | 3.4 M | 0.5 M |
| 15 | 2.2 V | 3.1 M | −0.5 M | 2.0 M | −0.5 M | 2.6 M | −0.5 M |
| SECTION III | SECTION II | |||
|---|---|---|---|---|
| Load Case | A | C | A | C |
| [%] | [%] | [%] | [%] | |
| 10 | 9 | 16 | 3 | 109 |
| 11 | 9 | 73 | 6 | 52 |
| 12 | 3 | 13 | 2 | 109 |
| 13 | 2 | 77 | 3 | 53 |
| 14 | 3 | 12 | 2 | 109 |
| 15 | 0 | 78 | 4 | 54 |
| average | 4.33 | 44.83 | 3.33 | 81.00 |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Święch, Ł. Calibration of a Load Measurement System for an Unmanned Aircraft Composite Wing Based on Fibre Bragg Gratings and Electrical Strain Gauges. Aerospace 2020, 7, 27. https://doi.org/10.3390/aerospace7030027
Święch Ł. Calibration of a Load Measurement System for an Unmanned Aircraft Composite Wing Based on Fibre Bragg Gratings and Electrical Strain Gauges. Aerospace. 2020; 7(3):27. https://doi.org/10.3390/aerospace7030027
Chicago/Turabian StyleŚwięch, Łukasz. 2020. "Calibration of a Load Measurement System for an Unmanned Aircraft Composite Wing Based on Fibre Bragg Gratings and Electrical Strain Gauges" Aerospace 7, no. 3: 27. https://doi.org/10.3390/aerospace7030027
APA StyleŚwięch, Ł. (2020). Calibration of a Load Measurement System for an Unmanned Aircraft Composite Wing Based on Fibre Bragg Gratings and Electrical Strain Gauges. Aerospace, 7(3), 27. https://doi.org/10.3390/aerospace7030027





