Numerical and Experimental Investigation of Oil-Guiding Splash Lubrication in Light Helicopter’s Reducers
Abstract
:1. Introduction
2. CFD Methodology
2.1. Governing Equation
2.2. CFD Modeling
2.2.1. Oil-Guiding Cylinder and Oil-Guiding Splash Lubrication System
2.2.2. Flow Monitoring Plane
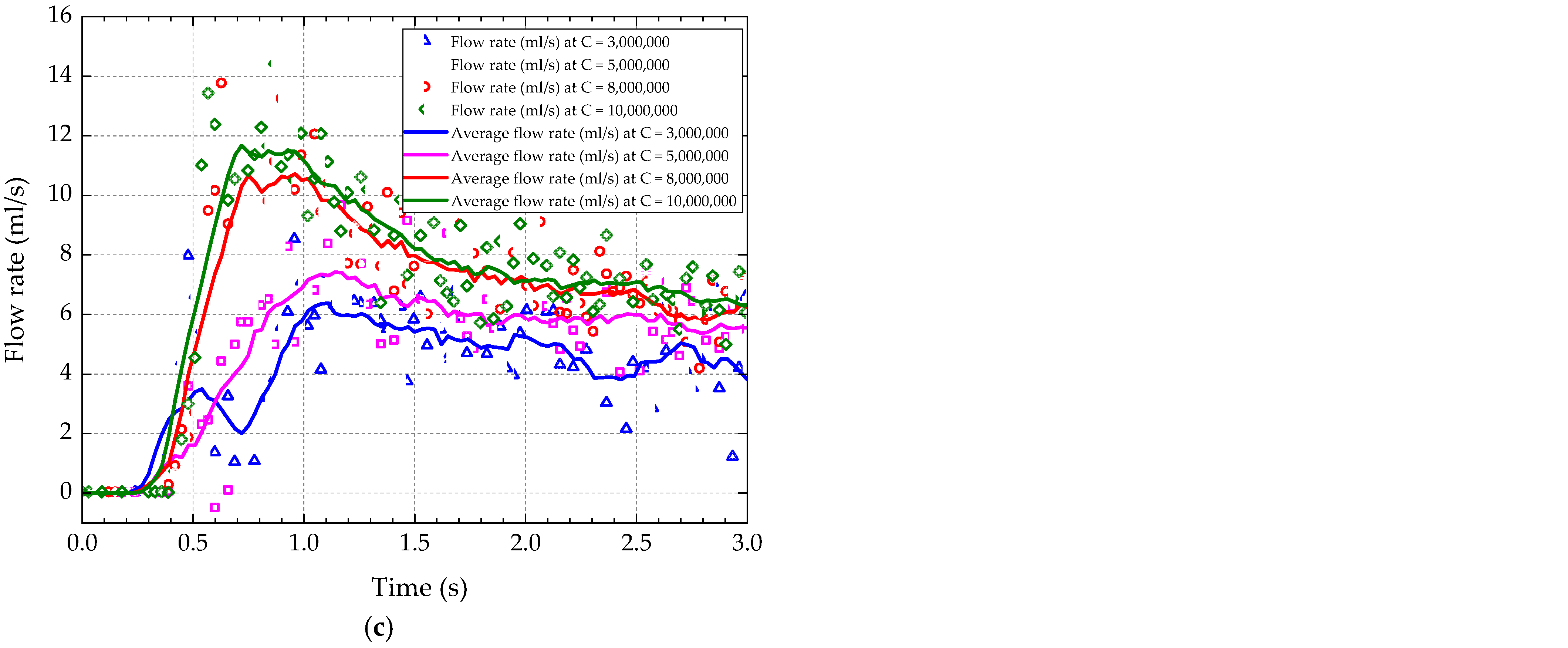
2.3. CFD Grid Technology
3. Numerical Results
3.1. Rotational Speed
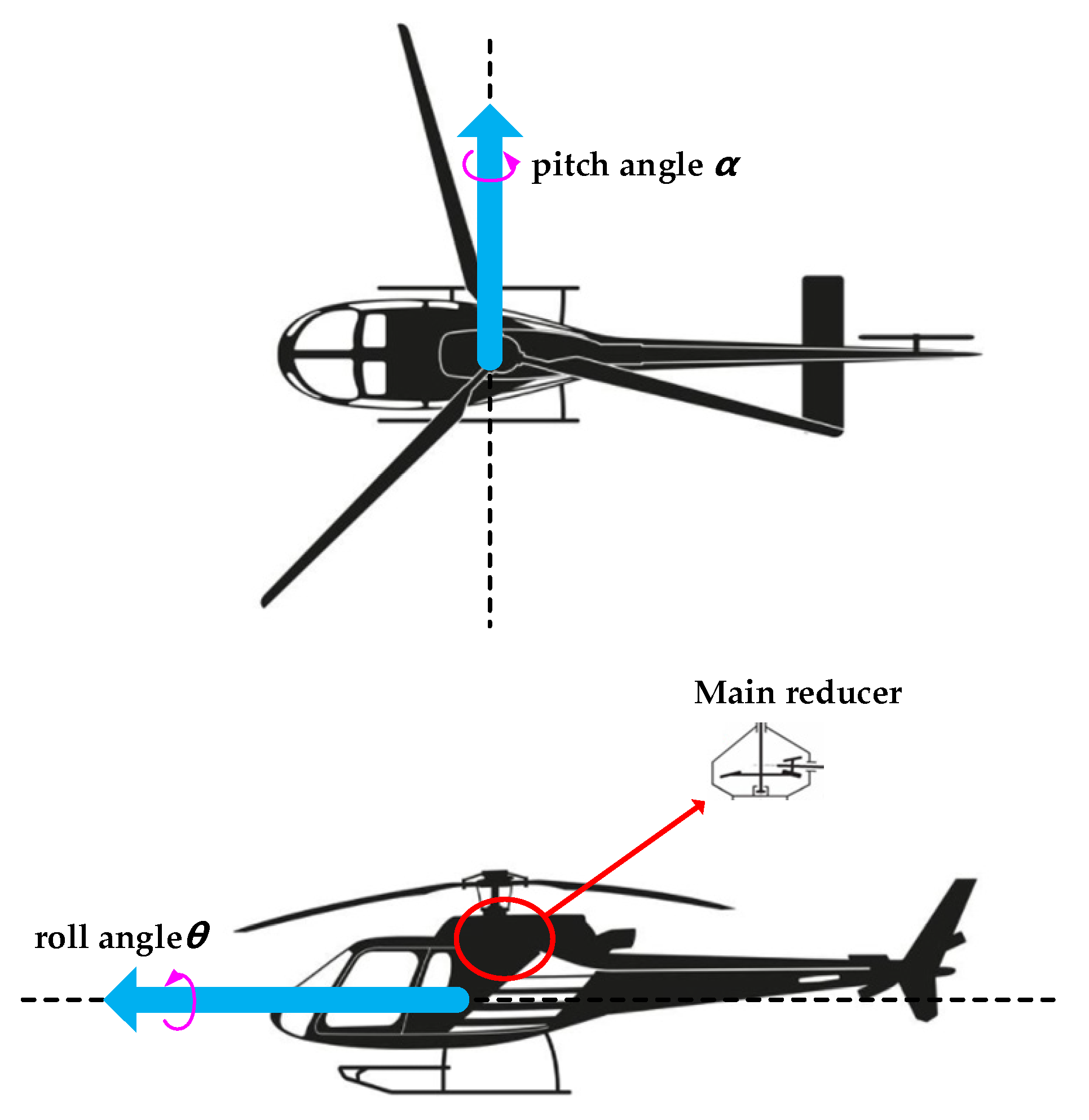
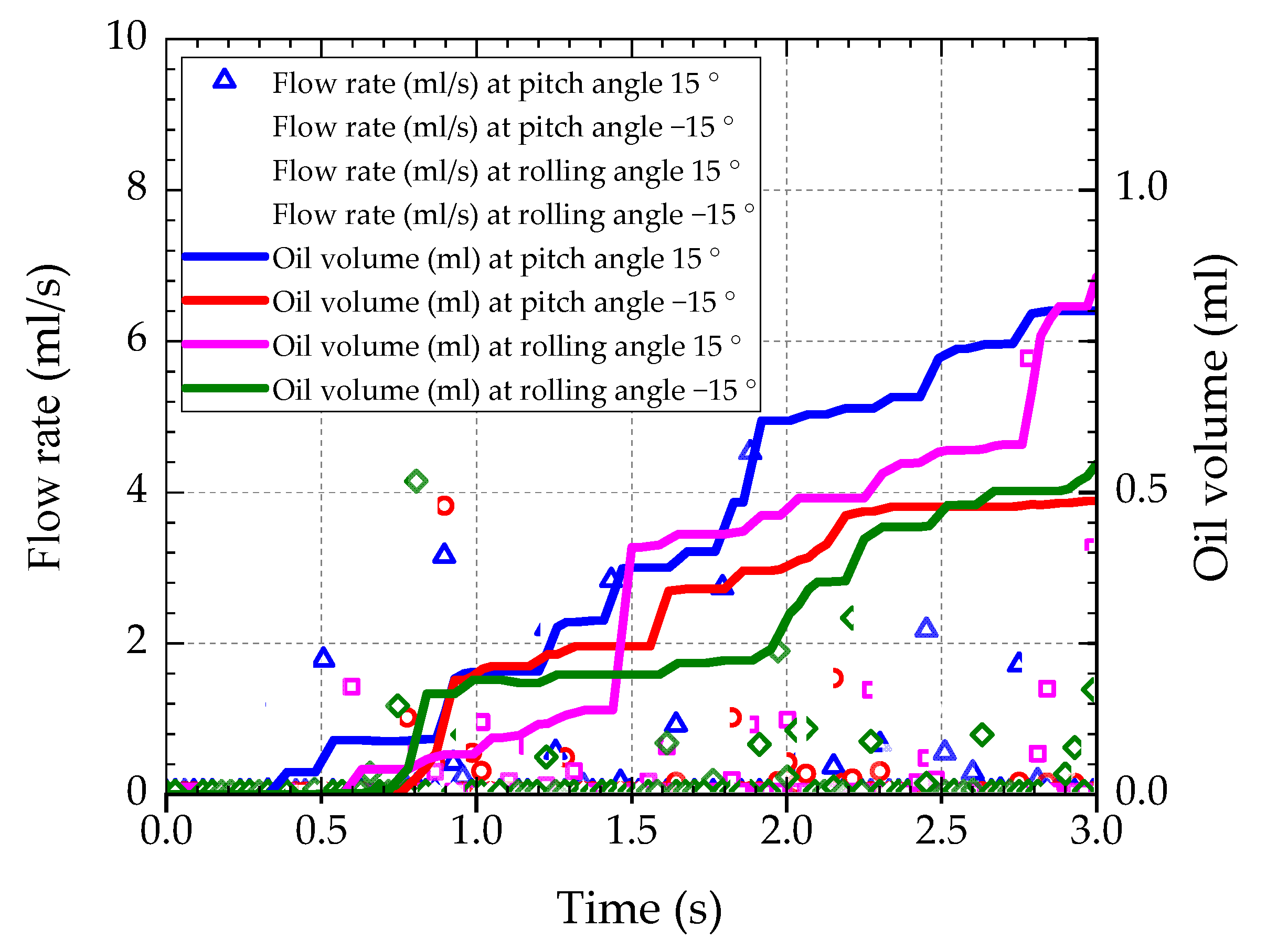
3.2. Flight Inclination
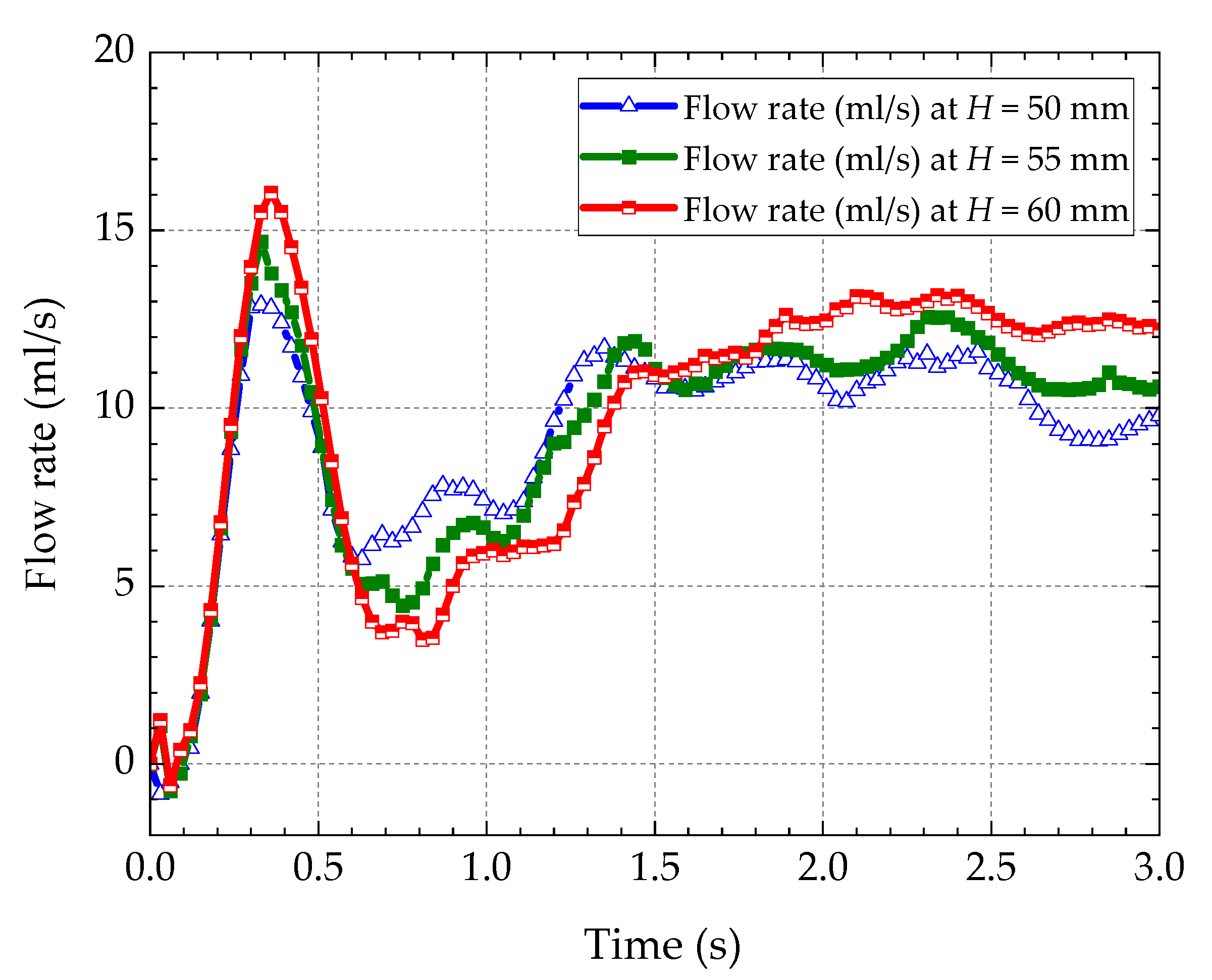
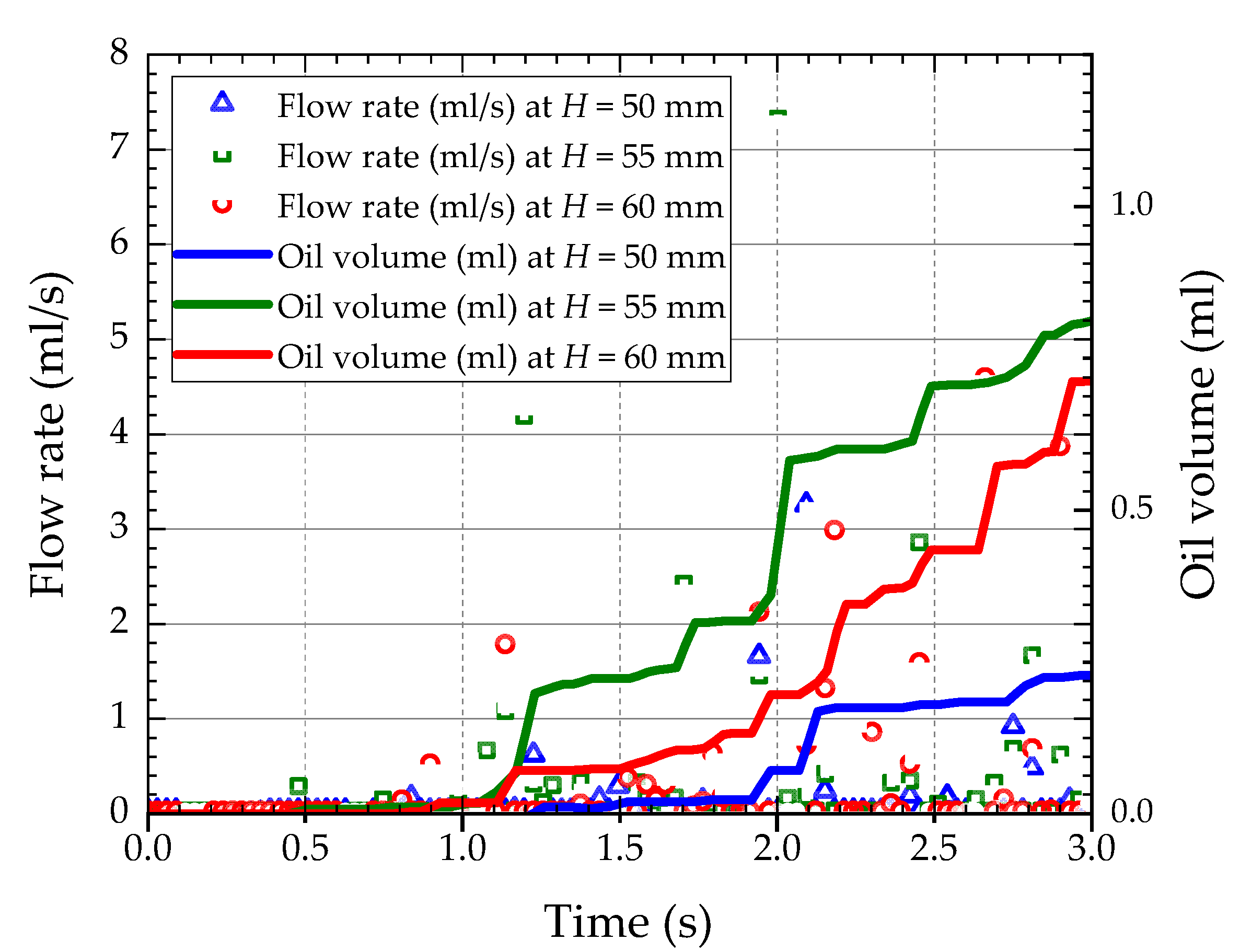
3.3. Oil Level
4. Experimental Validation
4.1. Test Rig
4.2. Experimental Results
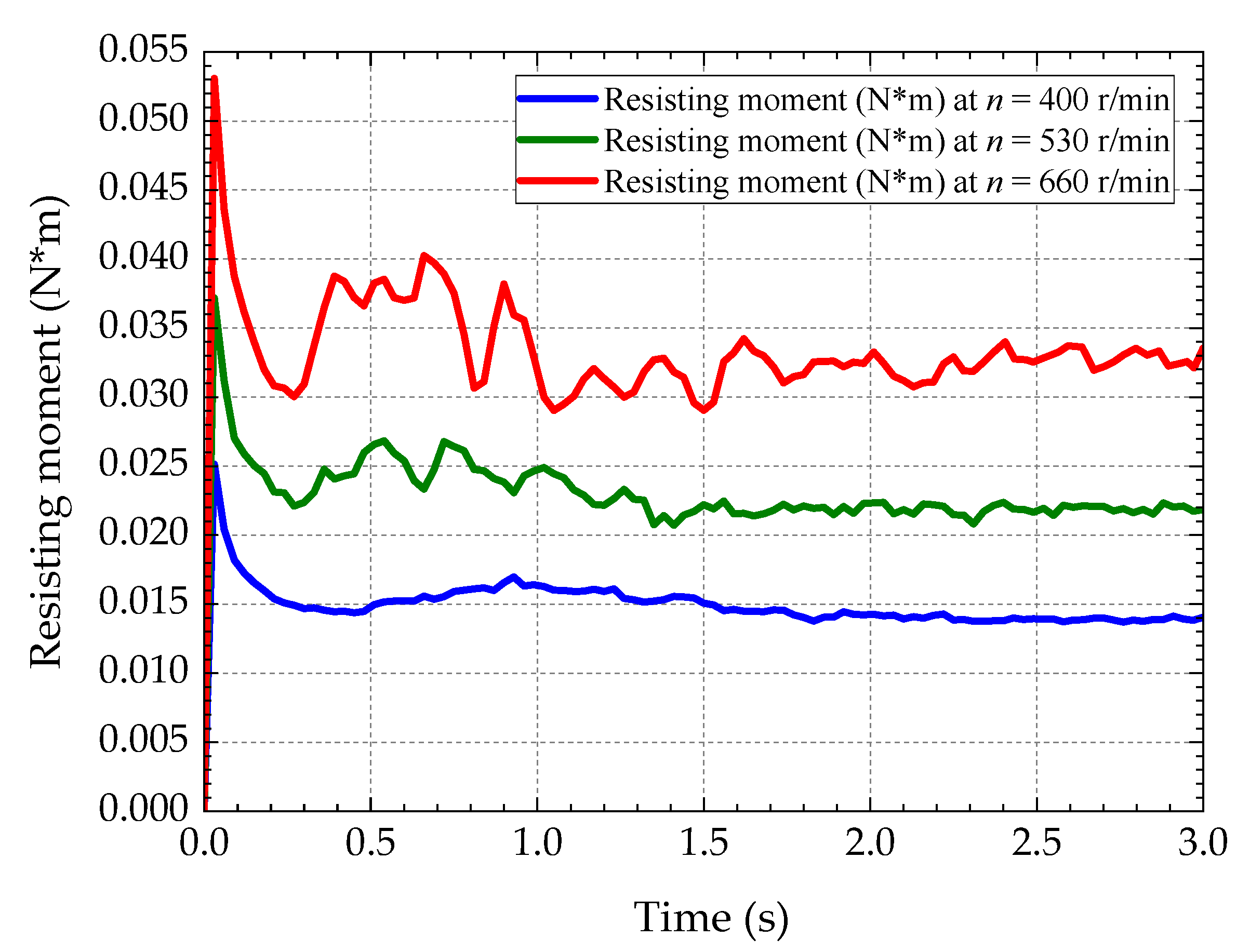
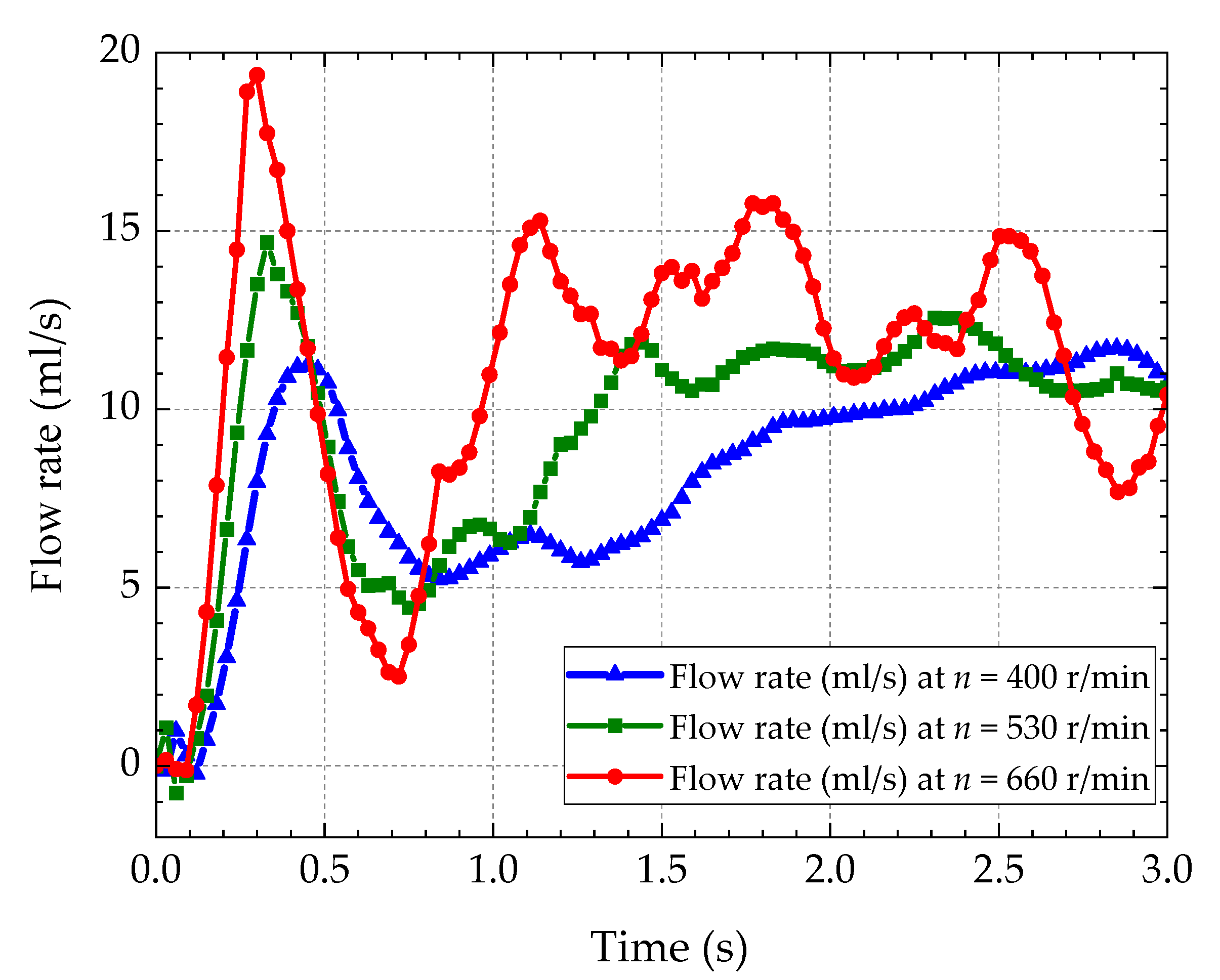
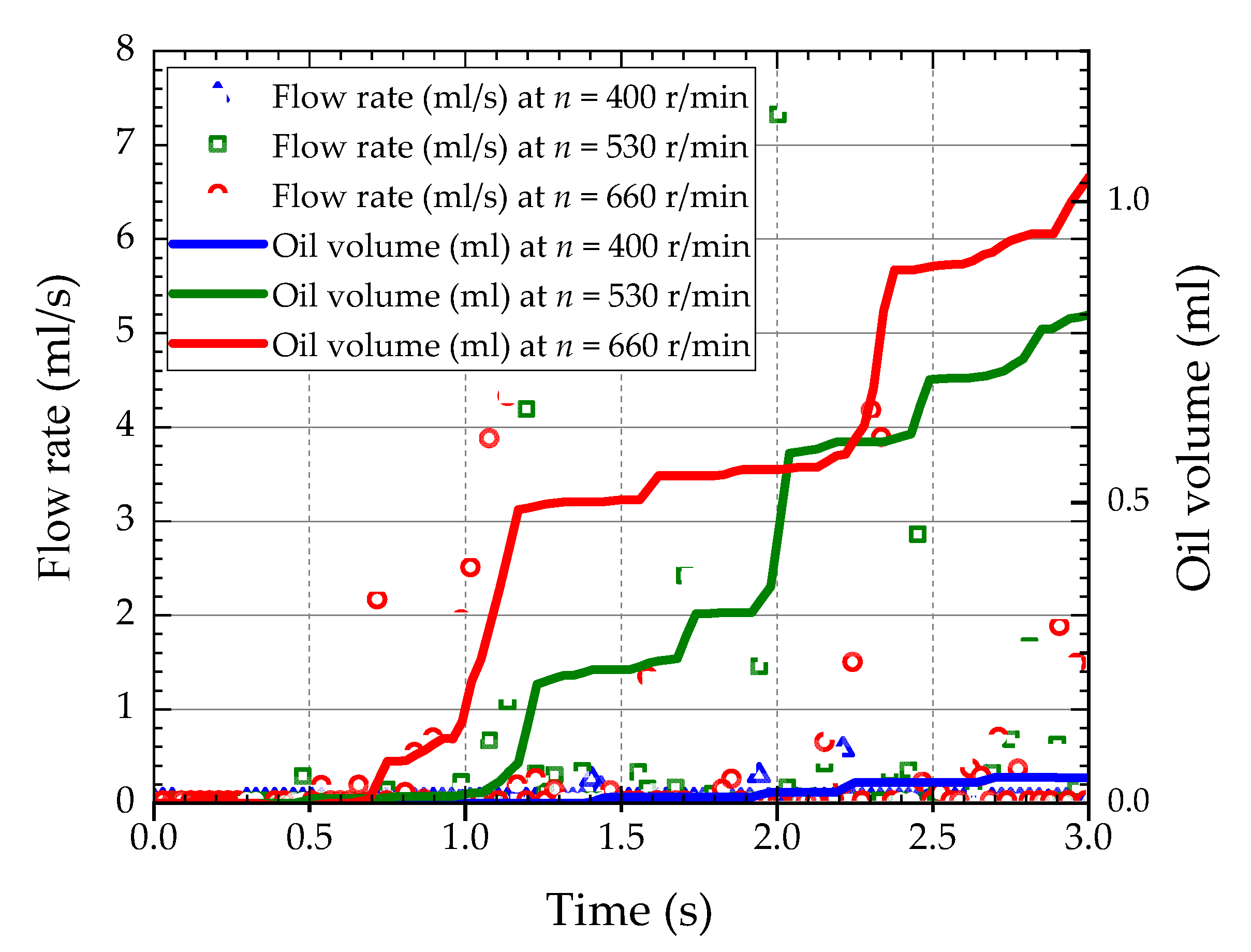
4.2.1. Rotational Speed
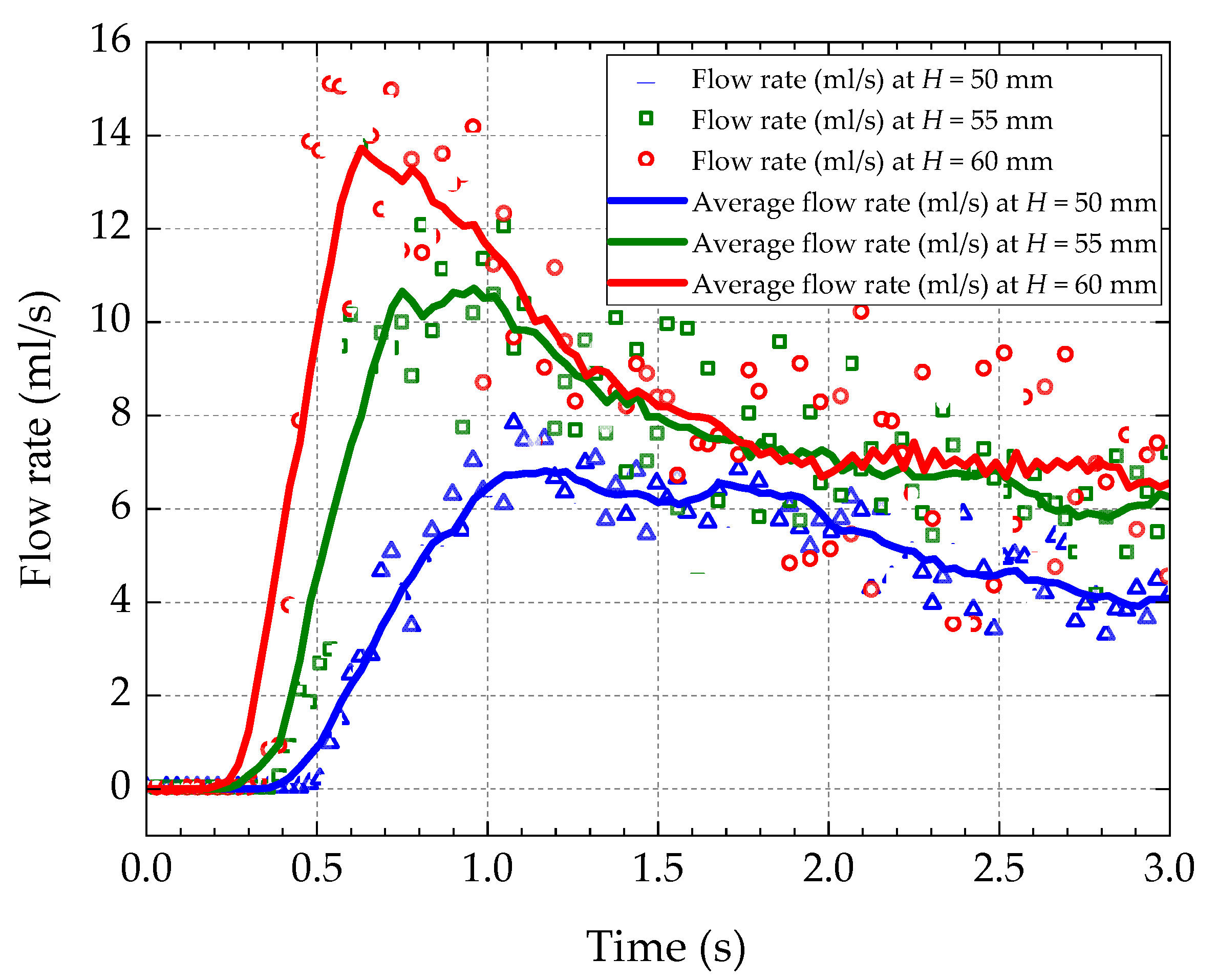
4.2.2. Oil Level
5. Conclusions
- The increase in rotational speed makes the flow rate of baseline testing rise, and the amount of oil splashed to the oil-collecting pipe is greatly increased, resulting in a larger increase in oil volume. There is a critical speed between 400 r/min and 530 r/min, beyond which the oil can climb to the top of the oil-guiding cylinder in large quantities; if not, the continuous oil supply cannot be realized.
- The amount of oil passing through the target area increases with the oil level. The higher the oil level height, the more oil is splashed into the oil-collecting tube from the gear surface. From the oil supply at the top of the oil-guiding cylinder, the oil supply increases when the oil level rises, but the amount of oil is not significantly elevated. At this point, the amount of oil flowing through the oil-jet hole has reached saturation point. Balancing the resisting moment and the flow rate, the oil level height H = 55 mm is the best choice.
- The difference in flow rate caused by the pitch angle and the roll angle is mainly due to gravity and rotation direction. When the direction of oil throwing is inclined to the gravity direction, the splash of oil volume is higher. When the rotor shaft is rotating, the splash of oil volume is higher as the oil-jet hole moves from high to low than that of moving from low to high.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Seetharaman, S.; Kahraman, A.; Moorhead, M.D.; Petry-Johnson, T.T. Oil Churning Power Losses of a Gear Pair: Experiments and Model Validation. J. Tribol. 2009, 131, 022202. [Google Scholar] [CrossRef]
- Neurouth, A.; Changenet, C.; Ville, F.; Octrue, M.; Tinguy, E. Experimental Investigations to Use Splash Lubrication for High-Speed Gears. J. Tribol. 2017, 139, 061104. [Google Scholar] [CrossRef]
- Casseau, V.; Espinoza, D.E.R.; Scanlon, T.J.; Brown, R.E. A Two-Temperature Open-Source CFD Model for Hypersonic Reacting Flows, Part Two: Multi-Dimensional Analysis. Aerospace 2016, 3, 45. [Google Scholar] [CrossRef] [Green Version]
- Youssefi, M.R.; Knight, D. Assessment of CFD Capability for Hypersonic Shock Wave Laminar Boundary Layer Interactions. Aerospace 2017, 4, 25. [Google Scholar] [CrossRef]
- Samad, A.; Villeneuve, E.; Morency, F.; Volat, C. A Numerical and Experimental Investigation of the Convective Heat Transfer on a Small Helicopter Rotor Test Setup. Aerospace 2021, 8, 53. [Google Scholar] [CrossRef]
- Moshammer, T.; Mayr, F.; Kargl, K.; Honeger, C. Simulation of Oil Flow in Gear Box Housing. SAE Tech. Pap. Ser. 2006, 1, 1574. [Google Scholar] [CrossRef]
- Concli, F.; Torre, A.D.; Gorla, C.; Montenegro, G. A New Integrated Approach for the Prediction of the Load Independent Power Losses of Gears: Development of a Mesh-Handling Algorithm to Reduce the CFD Simulation Time. Adv. Tribol. 2016, 2016, 2957151. [Google Scholar] [CrossRef]
- Concli, F.; Gorla, C. Numerical modeling of the power losses in geared transmissions: Windage, churning and cavitation simulations with a new integrated approach that drastically reduces the computational effort. Tribol. Int. 2016, 103, 58–68. [Google Scholar] [CrossRef]
- Concli, F.; Gorla, C. Numerical modeling of the churning power losses in planetary gearboxes: An innovative partitioning-based meshing methodology for the application of a computational effort reduction strategy to complex gearbox configurations. Lubr. Sci. 2017, 29, 455–474. [Google Scholar] [CrossRef]
- Liu, H.; Jurkschat, T.; Lohner, T.; Stahl, K. Determination of oil distribution and churning power loss of gearboxes by finite volume CFD method. Tribol. Int. 2017, 109, 346–354. [Google Scholar] [CrossRef]
- Liu, H.; Arfaoui, G.; Stanić, M.; Montigny, L.; Jurkschat, T.; Lohner, T.; Stahl, K. Numerical modelling of oil distribution and churning gear power losses of gearboxes by smoothed particle hydrodynamics. Proc. Inst. Mech. Eng. Part J. J. Eng. Tribol. 2018, 233, 74–86. [Google Scholar] [CrossRef]
- Liu, H.; Jurkschat, T.; Lohner, T.; Stahl, K. Detailed Investigations on the Oil Flow in Dip-Lubricated Gearboxes by the Finite Volume CFD Method. Lubricants 2018, 6, 47. [Google Scholar] [CrossRef] [Green Version]
- Hu, X.; Jiang, Y.; Luo, C.; Feng, L.; Dai, Y. Churning power losses of a gearbox with spiral bevel geared transmission. Tribol. Int. 2019, 129, 398–406. [Google Scholar] [CrossRef]
- Jiang, Y.; Hu, X.; Hong, S.; Li, P.; Wu, M. Influences of an oil guide device on splash lubrication performance in a spiral bevel gearbox. Tribol. Int. 2019, 136, 155–164. [Google Scholar] [CrossRef]
- Hu, X.; Wang, A.; Li, P.; Wang, J. Influence of dynamic attitudes on oil supply for bearings and churning power losses in a splash lubricated spiral bevel gearbox. Tribol. Int. 2021, 159, 106951. [Google Scholar] [CrossRef]
- Changenet, C.; Velex, P. A Model for the Prediction of Churning Losses in Geared Transmissions—Preliminary Results. J. Mech. Des. 2006, 129, 128–133. [Google Scholar] [CrossRef]
- Changenet, C.; Velex, P. Housing Influence on Churning Losses in Geared Transmissions. J. Mech. Des. 2008, 130, 062603. [Google Scholar] [CrossRef]
- Changenet, C.; Leprince, G.; Ville, F.; Velex, P. A Note on Flow Regimes and Churning Loss Modeling. J. Mech. Des. 2011, 133, 121009. [Google Scholar] [CrossRef]
- Quiban, R.; Changenet, C.; Marchesse, Y.; Ville, F.; Belmonte, J. Churning losses of spiral bevel gears at high rotational speed. Proc. Inst. Mech. Eng. Part J. J. Eng. Tribol. 2019, 234, 172–182. [Google Scholar] [CrossRef]
- Dai, Y.; Ma, F.; Zhu, X.; Ouyang, B. Development of an analytical model to estimate the churning power losses of a spiral bevel gear. Tribol. Int. 2020, 151, 106536. [Google Scholar] [CrossRef]
- Lu, F.; Wang, M.; Bao, H.; Huang, W.; Zhu, R. Churning power loss of the intermediate gearbox in a helicopter under splash lubrication. Proc. Inst. Mech. Eng. Part J. J. Eng. Tribol. 2021. [Google Scholar] [CrossRef]
- Wang, Y.; Niu, W.; Wei, S.; Song, G. Influence of spin flow on lubricating oil jet—Design method of oil spray parameters to high speed spur gears. Tribol. Int. 2015, 92, 290–300. [Google Scholar] [CrossRef]
- Wang, Y.; Song, G.; Niu, W.; Chen, Y. Influence of oil injection methods on the lubrication process of high speed spur gears. Tribol. Int. 2018, 121, 180–189. [Google Scholar] [CrossRef]
- Wang, Y.; Song, G.; Niu, W.; Chen, Y. Optimized design of spray parameters of oil jet lubricated spur gears. Tribol. Int. 2018, 120, 149–158. [Google Scholar] [CrossRef]
- Dai, Y.; Wu, W.; Zhou, H.B.; Zhang, J.; Ma, F.Y. Numerical Simulation and Optimization of Oil Jet Lubrication for Rotorcraft Meshing Gears. Int. J. Simul. Model. 2018, 17, 318–326. [Google Scholar] [CrossRef]
- Dai, Y.; Jia, J.; Ouyang, B.; Bian, J. Determination of an Optimal Oil Jet Nozzle Layout for Helical Gear Lubrication: Mathematical Modeling, Numerical Simulation, and Experimental Validation. Complexity 2020, 2020, 2187027. [Google Scholar] [CrossRef]
- Dai, Y.; Ma, F.; Zhu, X.; Su, Q.; Hu, X. Evaluation and optimization of the oil jet lubrication performance for orthogonal face gear drive: Modelling, simulation and experimental validation. Energies 2019, 12, 1935. [Google Scholar] [CrossRef] [Green Version]
- Zhu, X.; Dai, Y.; Ma, F.; Ouyang, B. Mathematical modeling and numerical simulation for determining an optimized oil jet layout for spiral bevel gear lubrication. Proc. Inst. Mech. Eng. Part J. J. Eng. Tribol. 2021, 235, 611–628. [Google Scholar] [CrossRef]
- Fondelli, T.; Andreini, A.; Da Soghe, R.; Facchini, B.; Cipolla, L. Numerical Simulation of Oil Jet Lubrication for High Speed Gears. Int. J. Aerosp. Eng. 2015, 2015, 752457. [Google Scholar] [CrossRef]
- Fondelli, T.; Andreini, A.; Da Soghe, R.; Facchini, B.; Cipolla, L. Volume of fluid (VOF) analysis of oil-jet lubrication for high-speed spur gears using an adaptive meshing approach. In Proceedings of the ASME Turbo Expo 2015: Turbine Technical Conference and Exposition, Montreal, QC, Canada, 15–19 June 2015. [Google Scholar] [CrossRef]
- Massini, D.; Fondelli, T.; Facchini, B.; Tarchi, L.; Leonardi, F. High Speed Visualizations of oil Jet Lubrication for Aero-engine Gearboxes. Energy Procedia 2016, 101, 1248–1255. [Google Scholar] [CrossRef]
- Yin, M.; Xu, L.J.; Dai, Y.; Yang, D.; Zhu, X. Flow Characteristics of Oil-Guiding Splash Lubrication: Simulation and Experiment Studies. Int. J. Simul. Model. 2021, 20, 363–374. [Google Scholar] [CrossRef]
- Wang, T.; Guo, Y.; Wang, G.; Wang, X. Detection and analysis of metal scrap in lubricating oil based on wear fault of spindle bearing of turboshaft engine. Vibroeng. Procedia 2021, 38, 32–37. [Google Scholar] [CrossRef]
- Wróblewski, P. Analysis of Torque Waveforms in Two-Cylinder Engines for Ultralight Aircraft Propulsion Operating on 0W-8 and 0W-16 Oils at High Thermal Loads Using the Diamond-Like Carbon Composite Coating. SAE Int. J. Engines 2021, 15, 2022. [Google Scholar] [CrossRef]
- Wróblewski, P.; Koszalka, G. An Experimental Study on Frictional Losses of Coated Piston Rings with Symmetric and Asymmetric Geometry. SAE Int. J. Engines 2021, 14, 853–866. [Google Scholar] [CrossRef]

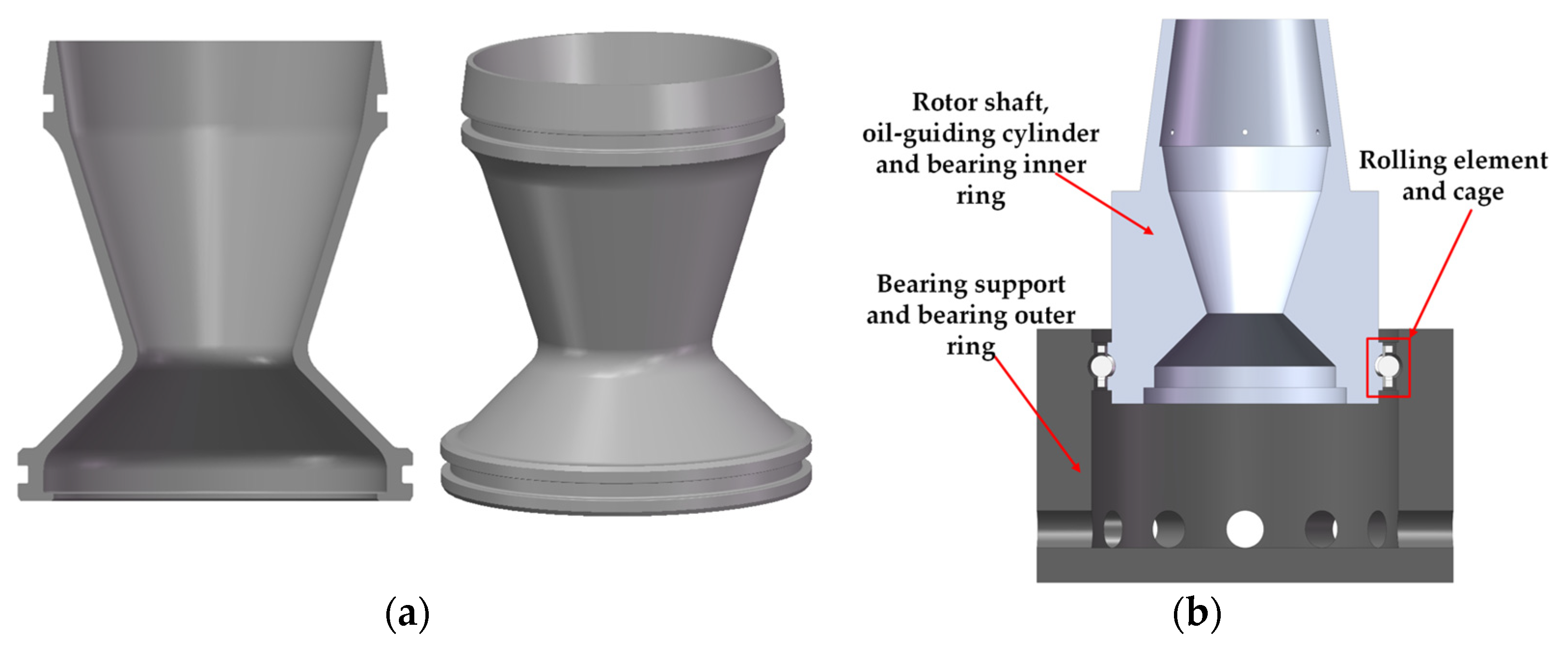





| Monitoring Planes | x-Direction (mm) | y-Direction (mm) | z-Direction (mm) |
|---|---|---|---|
| 1 | x = 70 | yL = −15, yH = 15 | zL = 105, zH = 135 |
| 2 | xL = −33, xH = 33 | yL = −33, yH = 33 | z = 113 |
| 3 | x = −70 | yL = −8, yH = 8 | zL = −38, zH = −22 |
| Rotating Speed (r/min) | Oil Volume of Monitoring Plane 1 (mL) | Oil Volume of Monitoring Plane 2 (mL) | Oil Volume of Monitoring Plane 3 (mL) |
|---|---|---|---|
| 400 | 0.043 | 0.11 | 24.40 |
| 530 | 0.82 | 19.66 | 28.24 |
| 660 | 1.04 | 28.97 | 33.10 |
| Parameters | Total Oil Volume (mL) | Direction of Tilt |
|---|---|---|
| α = 15°, θ = 0° | 0.80041 | Spin-in side |
| α = −15°, θ = 0° | 0.48555 | Spin-out side |
| α = 0°, θ = 0° | 0.81169 | Level |
| α = 0°, θ = 15° | 0.85675 | Spin-in side |
| α = 0°, θ = −15° | 0.54695 | Spin-out side |
| Oil Level (mm) | Oil Volume of Monitoring Plane 1 (mL) | Oil Volume of Monitoring Plane 2 (mL) | Oil Volume of Monitoring Plane 3 (mL) |
|---|---|---|---|
| 50 | 0.23 | 13.12 | 27.68 |
| 55 | 0.81 | 19.66 | 28.24 |
| 60 | 0.71 | 22.91 | 29.19 |
| Rotating Speed n (r/min) | RunningTime (min) | Experimental Oil Volume (mL) | Baseline Testing Oil Volume (mL) | Target Oil Volume (mL) | Numerical Oil Volume (mL) | Error |
|---|---|---|---|---|---|---|
| 400 | 5 | 0.5 | 0.4 | 0.1 | 0.12 | 20.0% |
| 530 | 5 | 4.4 | 1.9 | 2.5 | 2.16 | 13.6% |
| 660 | 5 | 10.2 | 7.7 | 2.5 | 2.27 | 9.2% |
| Oil Level H (mm) | Running Time (min) | Experimental Oil Volume (mL) | Baseline Testing Oil Volume (mL) | Target Oil Volume (mL) | Numerical Oil Volume (mL) | Error |
|---|---|---|---|---|---|---|
| 45 | 5 | 0.7 | 0.6 | 0.1 | 0.09 | 10.0% |
| 55 | 5 | 4.4 | 1.9 | 2.5 | 2.16 | 13.6% |
| 65 | 5 | 17.3 | 14.2 | 3.1 | 3.49 | 12.6% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yin, M.; Chen, X.; Dai, Y.; Yang, D.; Xu, L.; Zhu, X. Numerical and Experimental Investigation of Oil-Guiding Splash Lubrication in Light Helicopter’s Reducers. Aerospace 2021, 8, 345. https://doi.org/10.3390/aerospace8110345
Yin M, Chen X, Dai Y, Yang D, Xu L, Zhu X. Numerical and Experimental Investigation of Oil-Guiding Splash Lubrication in Light Helicopter’s Reducers. Aerospace. 2021; 8(11):345. https://doi.org/10.3390/aerospace8110345
Chicago/Turabian StyleYin, Mei, Xi Chen, Yu Dai, Duan Yang, Lanjin Xu, and Xiang Zhu. 2021. "Numerical and Experimental Investigation of Oil-Guiding Splash Lubrication in Light Helicopter’s Reducers" Aerospace 8, no. 11: 345. https://doi.org/10.3390/aerospace8110345
APA StyleYin, M., Chen, X., Dai, Y., Yang, D., Xu, L., & Zhu, X. (2021). Numerical and Experimental Investigation of Oil-Guiding Splash Lubrication in Light Helicopter’s Reducers. Aerospace, 8(11), 345. https://doi.org/10.3390/aerospace8110345







