Aspect Ratio Driven Relationship between Nozzle Internal Flow and Supersonic Jet Mixing
Abstract
1. Introduction
2. Methodology
2.1. Physics Setup
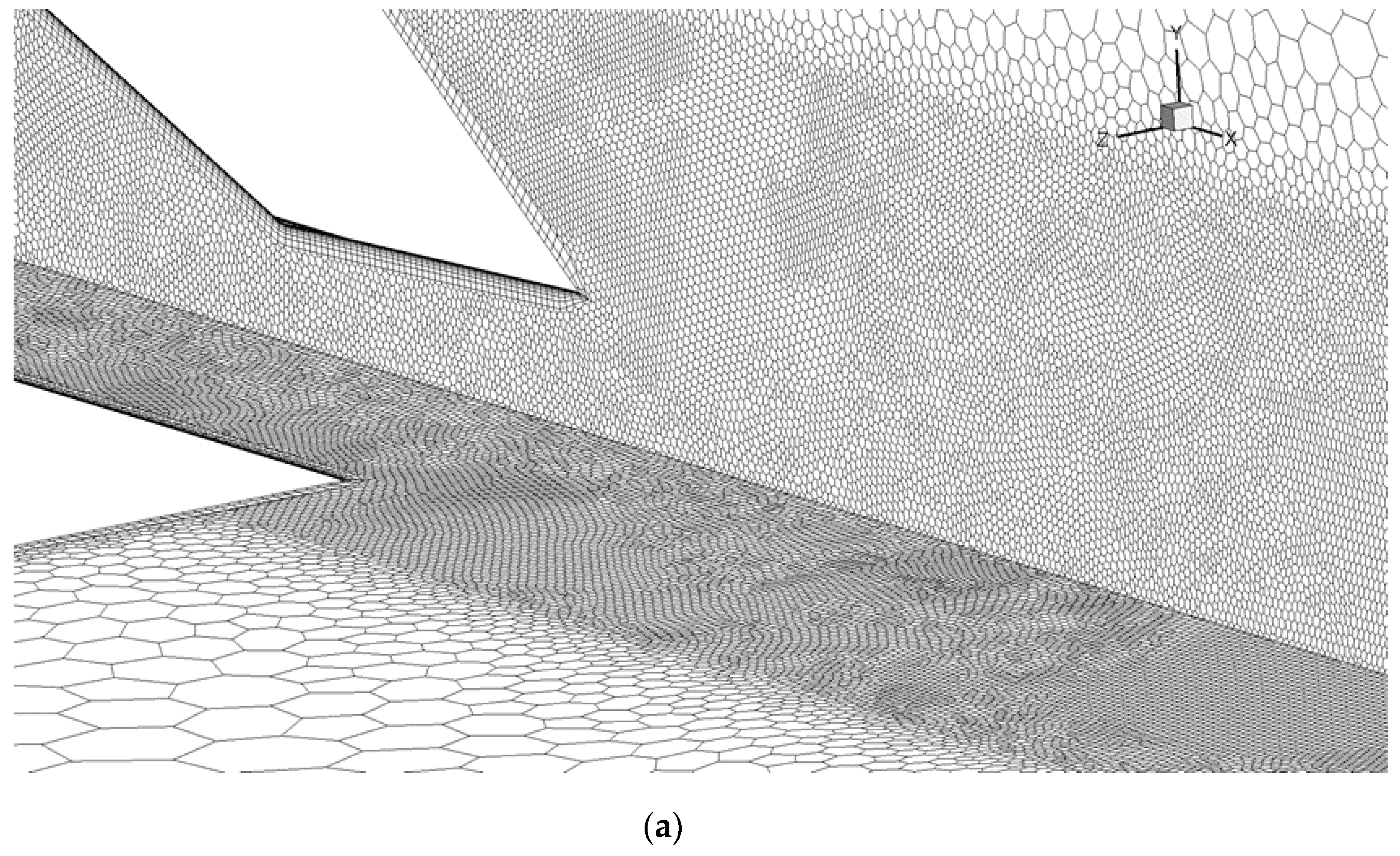
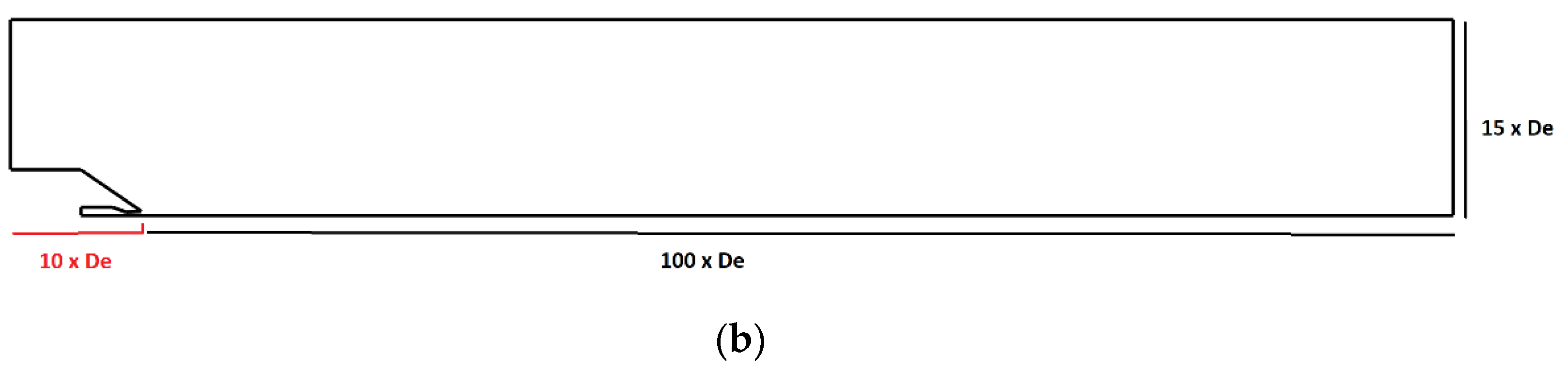
2.2. Computational Domain, Mesh, and Boundary Conditions
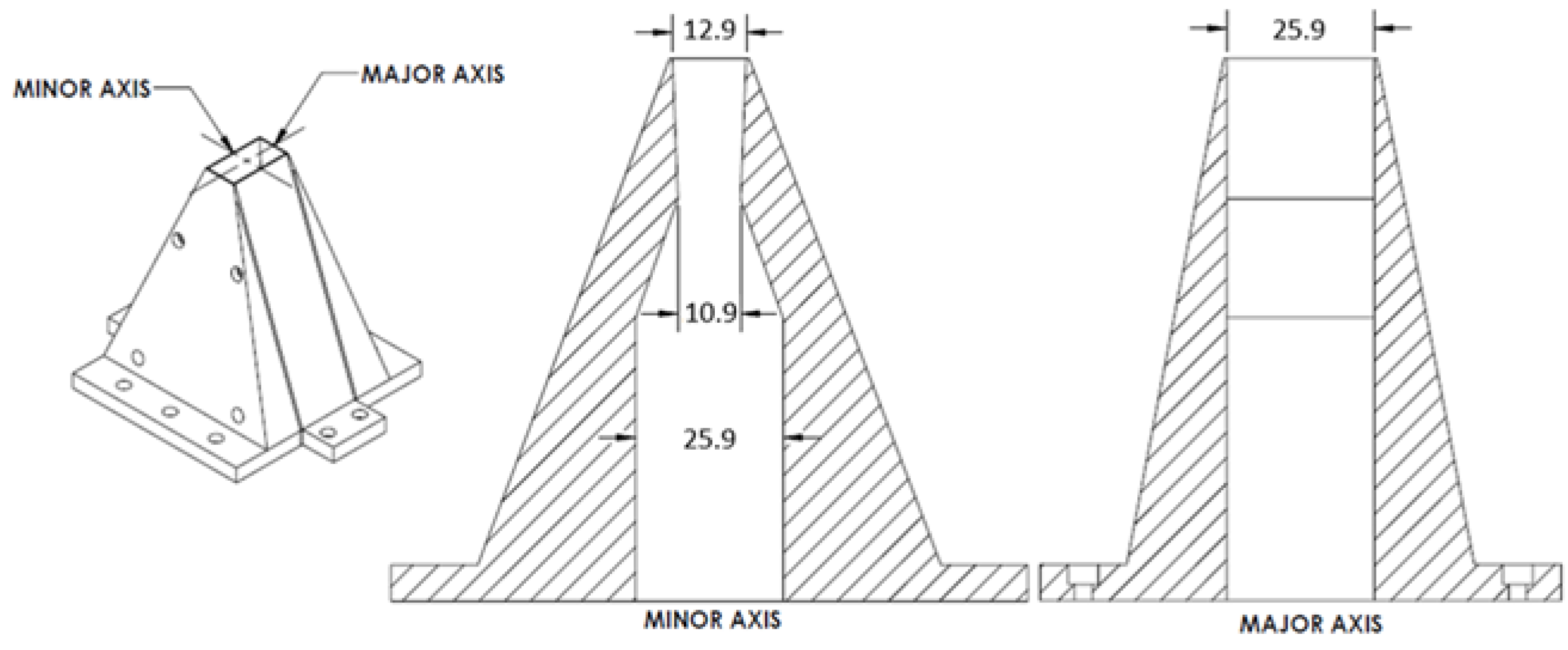
2.3. Nozzle Geometries and CFD Cases
Validation and Verification
3. Results
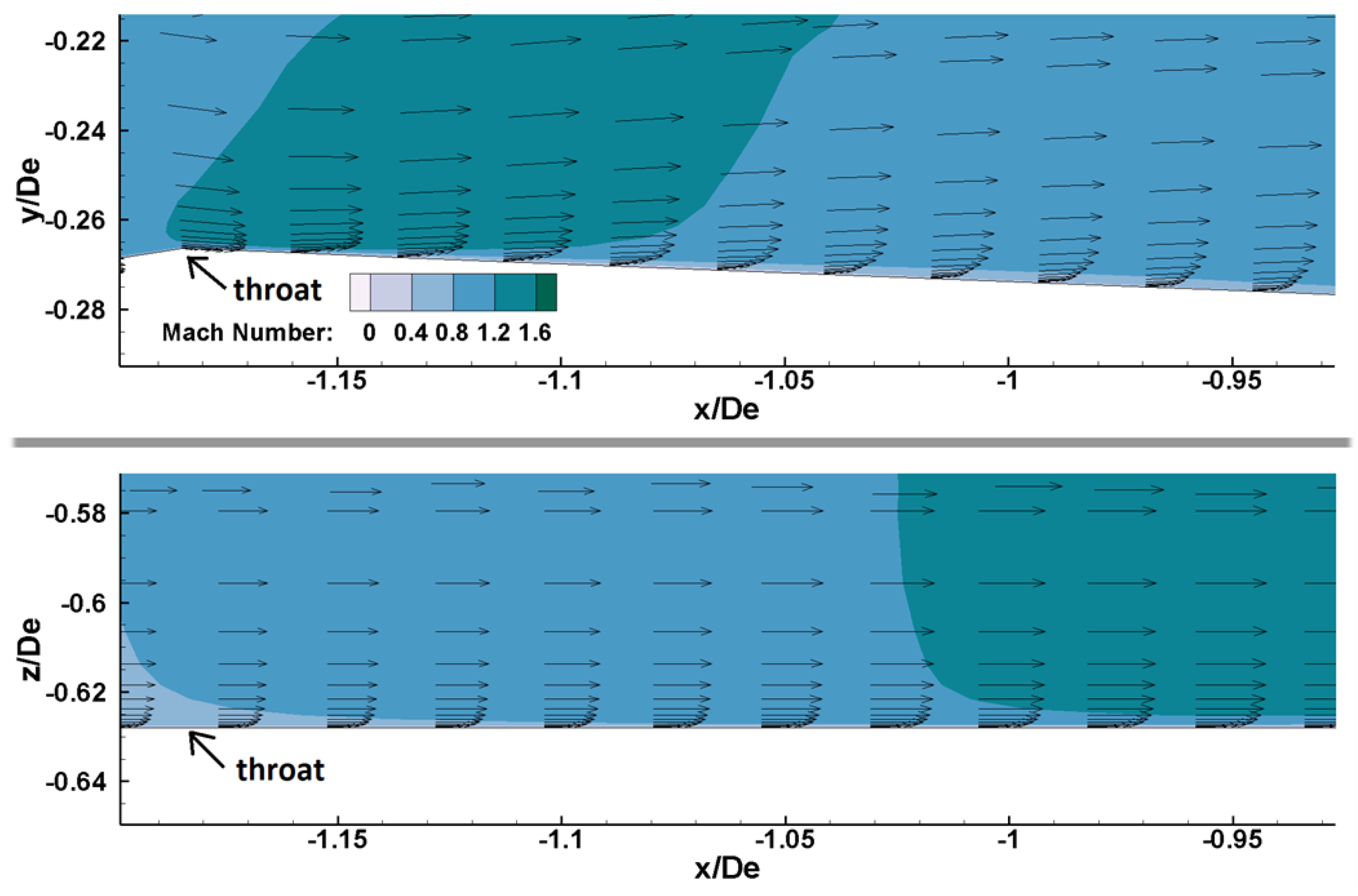
3.1. Aspect Ratio and Shock Formation
3.2. Nozzle-Exit Properties and Potential Core
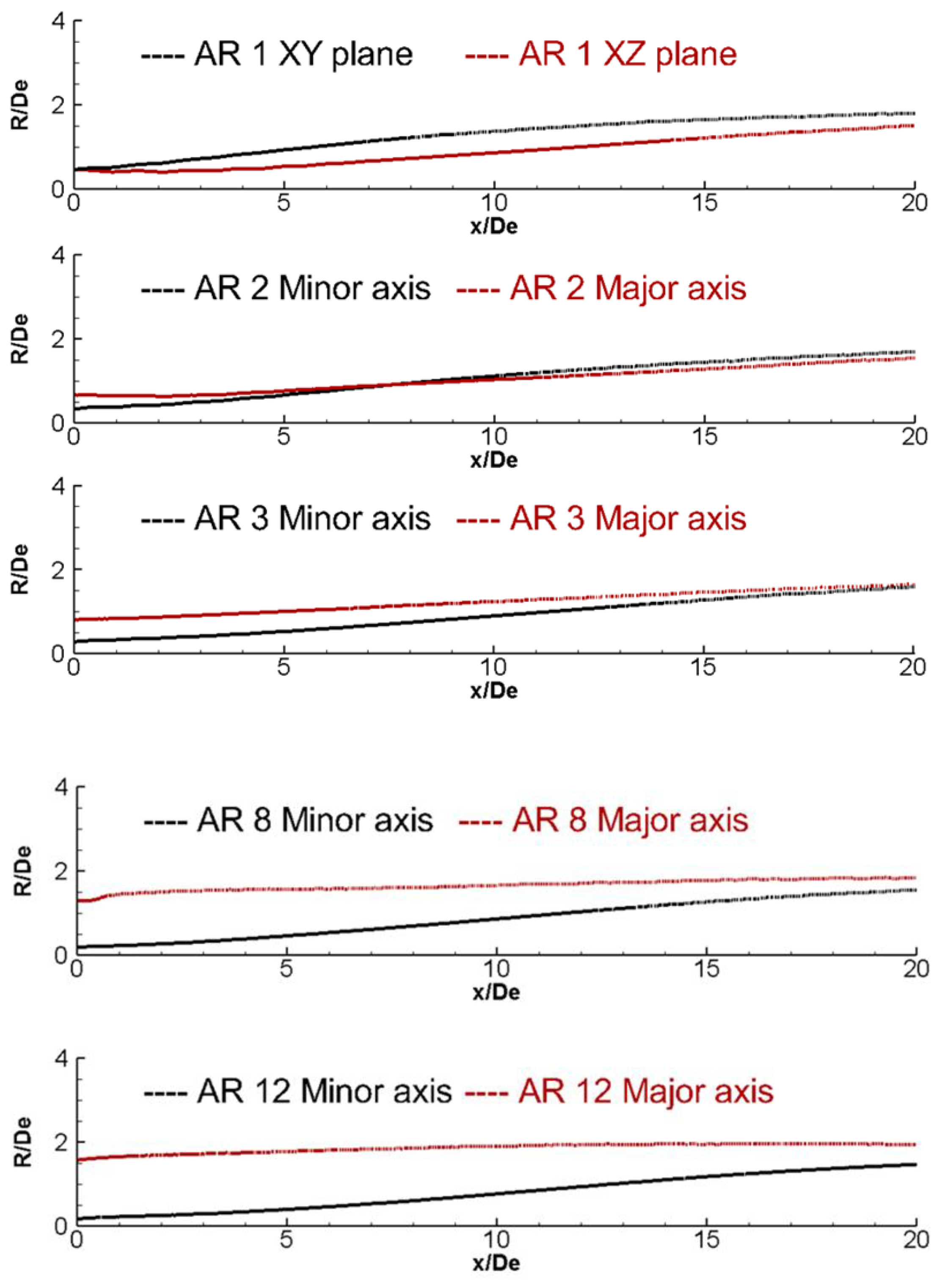
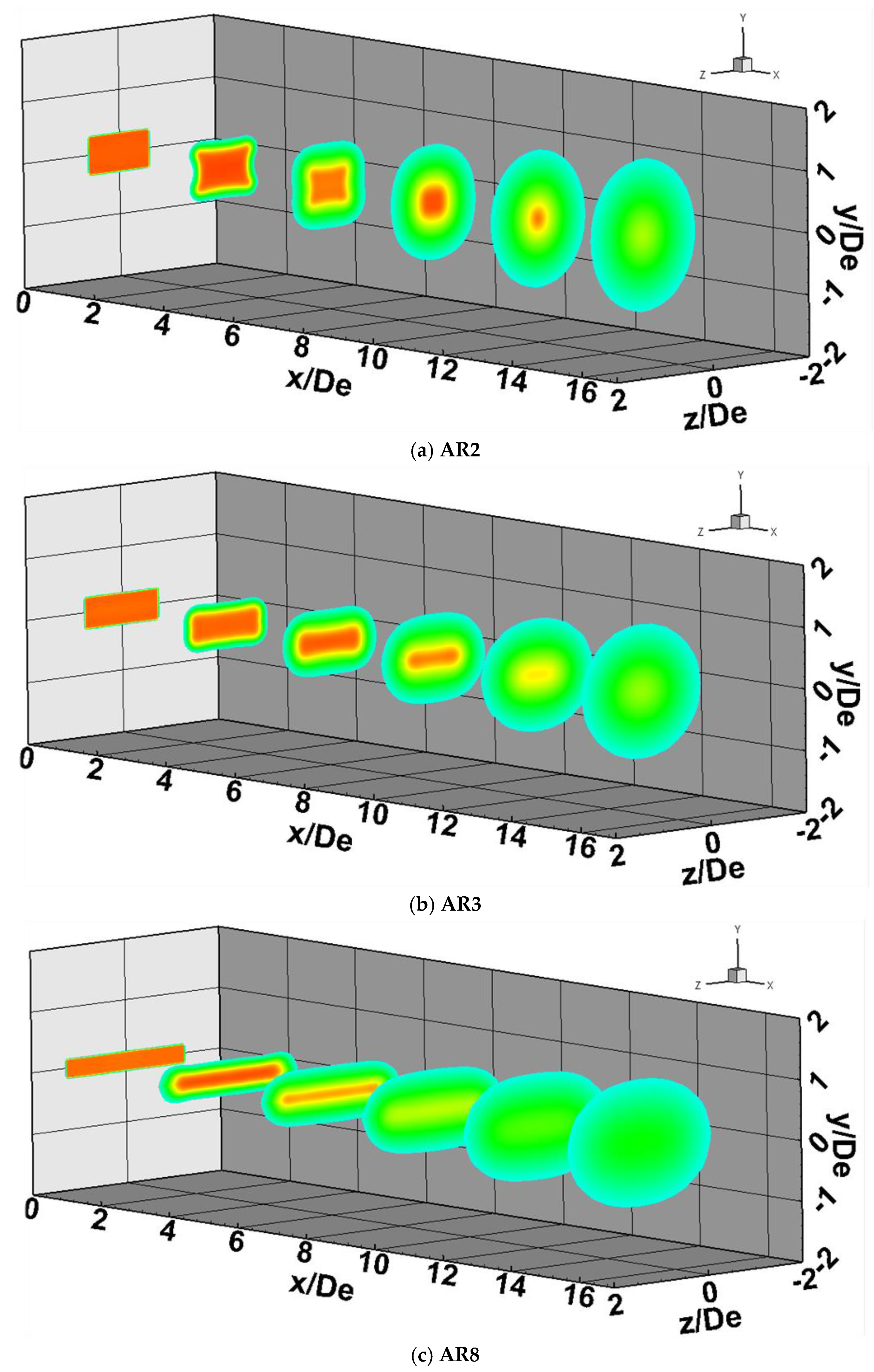
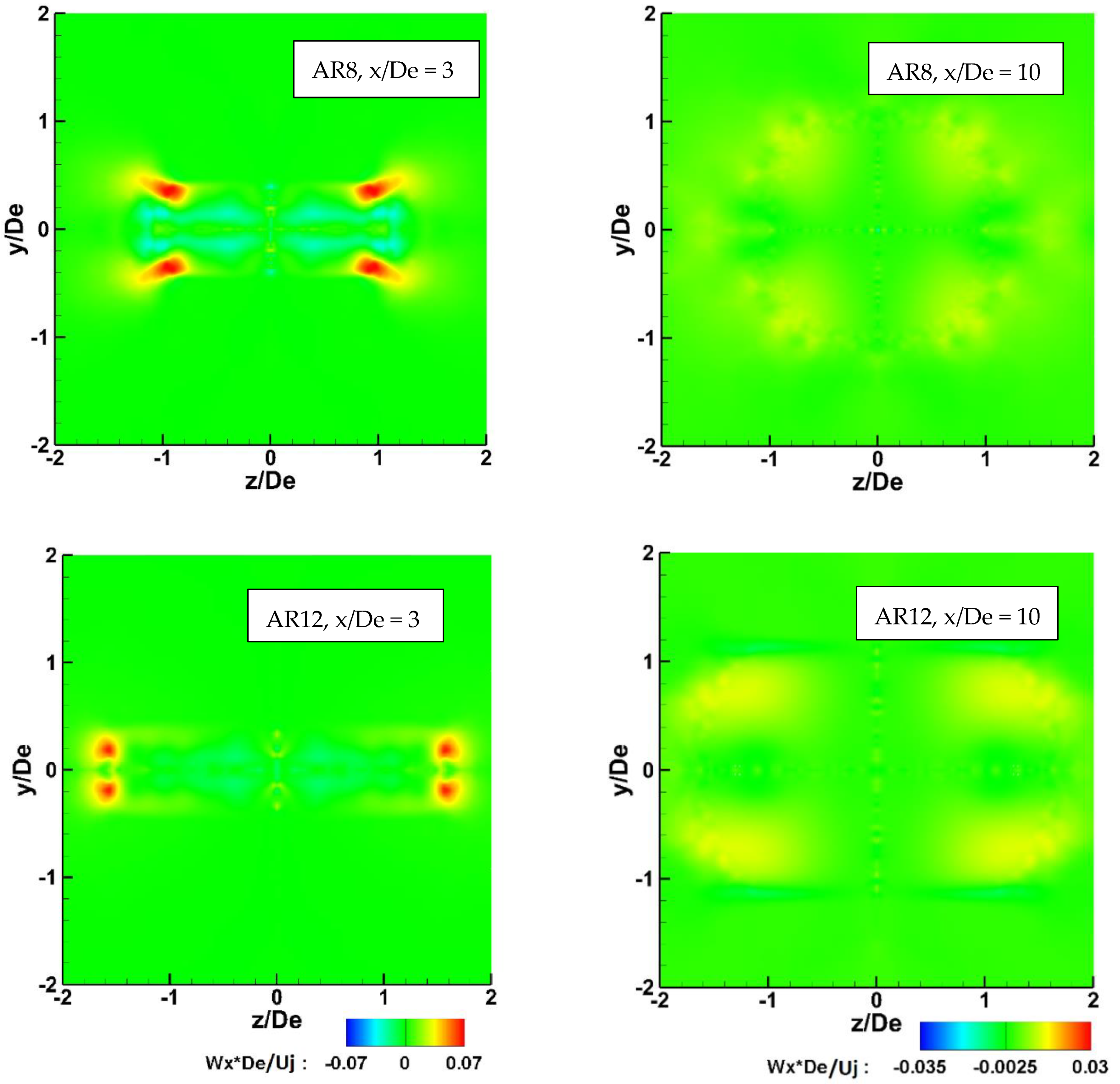
3.3. Jet Mixing, Axis Switching and Streamwise Vorticity
4. Conclusions and Future Work
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
Abbreviations
| AR | Aspect ratio |
| CFD | Computational fluid dynamics |
| LES | Large eddy simulation |
| RANS | Reynolds-averaged Navier−Stokes |
| R | Radial distance |
| SST | Shear stress transport |
| TKE | Turbulent kinetic energy |
| TR | Temperature ratio |
| U | Velocity in X direction |
| W | Vorticity |
Nomenclature
| Aexit | Nozzle-exit area |
| Cd | Discharge coefficient |
| De | Nozzle-exit equivalent diameter |
| Uj | Jet velocity at nozzle exit |
| Wx | Streamwise vorticity |
| x | Streamwise location in X direction |
| y | Location in Y direction |
| z | Location in Z direction |
References
- Zaman, K.B.M.Q. Axis switching and spreading of an asymmetric jet: The role of coherent structure dynamics. J. Fluid Mech. 1996, 316, 1–27. [Google Scholar] [CrossRef]
- Zaman, K.B.M.Q. Spreading Characteristics and Thrust of Jets from Asymmetric Nozzles. In Proceedings of the 34th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 15–18 January 1995; p. 200. [Google Scholar]
- Zaman, K.B.M.Q.; Steffen, C.J.; Reddy, D.R. Entrainment and Spreading Characteristics of Jets from Asymmetric Nozzles. In Proceedings of the 4th Shear Flow Conference, Snowmass Village, CO, USA, 29 June–2 July 1997. [Google Scholar]
- Gutmark, E.; Schadow, K.C.; Bicker, C.J. Near acoustic field and shock structure of rectangular supersonic jets. AIAA J. 1990, 28, 1163–1170. [Google Scholar] [CrossRef]
- Krothapalli, A.; Baganoff, D.; Karamcheti, K. On the mixing of a rectangular jet. J. Fluid Mech. 1981, 107, 201–220. [Google Scholar] [CrossRef]
- Mora, P. Investigation of the Noise Radiation from Heated Supersonic Jets. Ph.D. Thesis, University of Cincinnati, Cincinnati, OH, USA, 2016. [Google Scholar]
- Baier, F.; Mora, P.; Gutmark, E.; Kailasanath, K. Flow Measurements from a Supersonic Rectangular Nozzle Exhausting over a Flat Surface. In Proceedings of the 55th AIAA Aerospace Sciences Meeting, Grapevine, TX, USA, 9–13 January 2017. [Google Scholar]
- Brown, C.; Dippold, V. Jet-Surface Interaction–High Aspect Ratio Nozzle Test Nozzle Design and Preliminary Results. In Proceedings of the Acoustics Working Group Meeting, Cleveland, OH, USA, 20–21 October 2015. [Google Scholar]
- Viswanath, K.; Johnson, R.; Corrigan, A.; Kailasanath, K.; Mora, P.; Baier, F.; Gutmark, E. Noise Characteristics of a Rectangular vs. Circular Nozzle for Ideally Expanded Jet Flow. In Proceedings of the 54th AIAA Aerospace Sciences Meeting, San Diego, CA, USA, 4–8 January 2016. [Google Scholar]
- Munday, D.; Gutmark, E.; Liu, J.; Kailasanath, K. Flow Structure of Supersonic Jets from Conical Cd Nozzles. In Proceedings of the 39th AIAA Fluid Dynamics Conference, San Antonio, TX, USA, 22–25 June 2009. [Google Scholar]
- Fontaine, R.; Elliott, G.; Austin, J.; Freund, J. Very near-nozzle shear-layer turbulence and jet noise. J. Fluid Mech. 2015, 770, 27–51. [Google Scholar] [CrossRef]
- Heeb, N.; Mora, P.; Gutmark, E.; Kailasanath, K. Investigation of the Noise from a Rectangular Supersonic Jet. In Proceedings of the 19th AIAA/CEAS Aeroacoustics Conference, Berlin, Germany, 27–29 May 2013. [Google Scholar]
- Dippold, V., III. Design and Analyses of High Aspect Ratio Nozzles for Distributed Propulsion Acoustic Measurements. In Proceedings of the 34th AIAA Applied Aerodynamics Conference, Washington, DC, USA, 13–17 June 2016. [Google Scholar]
- Liu, J.; Kailasanath, K.; Ramamurti, R.; Munday, D.; Gutmark, E.; Lohner, R. Large-eddy simulations of a supersonic jet and its near-field acoustic properties. AIAA J. 2009, 47, 1849–1865. [Google Scholar] [CrossRef]
- Frate, F.; Bridges, J. Extensible Rectangular Nozzle Model System. In Proceedings of the 49th AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aerospace Exposition, Orlando, FL, USA, 4–7 January 2011; p. 975. [Google Scholar]
- Behrouzi, P.; McGuirk, J. Underexpanded jet development from a rectangular nozzle with Aft-Deck. AIAA J. 2015, 53, 5. [Google Scholar] [CrossRef][Green Version]
- Chen, N.; Huidan, Y. Mechanism of axis switching in low aspect-ratio rectangular jets. Int. J. Comput. Math. Appl. 2014, 67, 437–444. [Google Scholar] [CrossRef]
- Pereira, O.; Rodríguez, A.; Calleja-Ochoa, A.; Celaya, A.; Lopez de Lacalle, L.N.; Fernandez-Valdivielso, A.; Gonzalez, H. Simulation of cryo-cooling to improve super alloys cutting tools. Int. J. Precis. Eng. Manuf. Green Technol. 2021. [Google Scholar] [CrossRef]
- Trumper, M.; Behrouzi, P.; McGuirk, J. Influence of nozzle exit conditions on the near-field development of high subsonic and underexpanded axisymmetric jets. Aerospace 2018, 5, 35. [Google Scholar] [CrossRef]
- Georgiadis, N.J.; DeBonis, J. Navier–stokes analysis methods for turbulent jet flows with application to aircraft exhaust nozzles. Prog. Aerosp. Sci. 2006, 42, 377–418. [Google Scholar] [CrossRef]
- Bhide, K.; Siddappaji, K.; Abdallah, S. A Combined Effect of Wall Curvature and Aspect Ratio on the Performance of Rectangular Supersonic Nozzles. In Proceedings of the 6th International Conference on Jets, Wakes and Separated Flows, Cincinnati, OH, USA, 9–12 October 2017. [Google Scholar]
- Bhide, K.; Siddappaji, K.; Abdallah, S. Influence of fluid–thermal–structural interaction on boundary layer flow in rectangular supersonic nozzles. Aerospace 2018, 5, 33. [Google Scholar] [CrossRef]
- Bhide, K. On Shock-Boundary Layer Interactions-A Multiphysics Approach. Master’s Thesis, University of Cincinnati, Cincinnati, OH, USA, 2018. [Google Scholar]
- Greitzer, E.; Tan, C.; Graf, M. Internal Flow: Concepts and Applications; Cambridge University Press: Cambridge, UK, 2007; Volume 3. [Google Scholar]
- Star-CCM+ User Guide. Available online: https://docs.sw.siemens.com/documentation/external/PL20190509110447511/en-US/starccm/starccm/index.html#page/connect%2Fsplash.html (accessed on 28 February 2021).
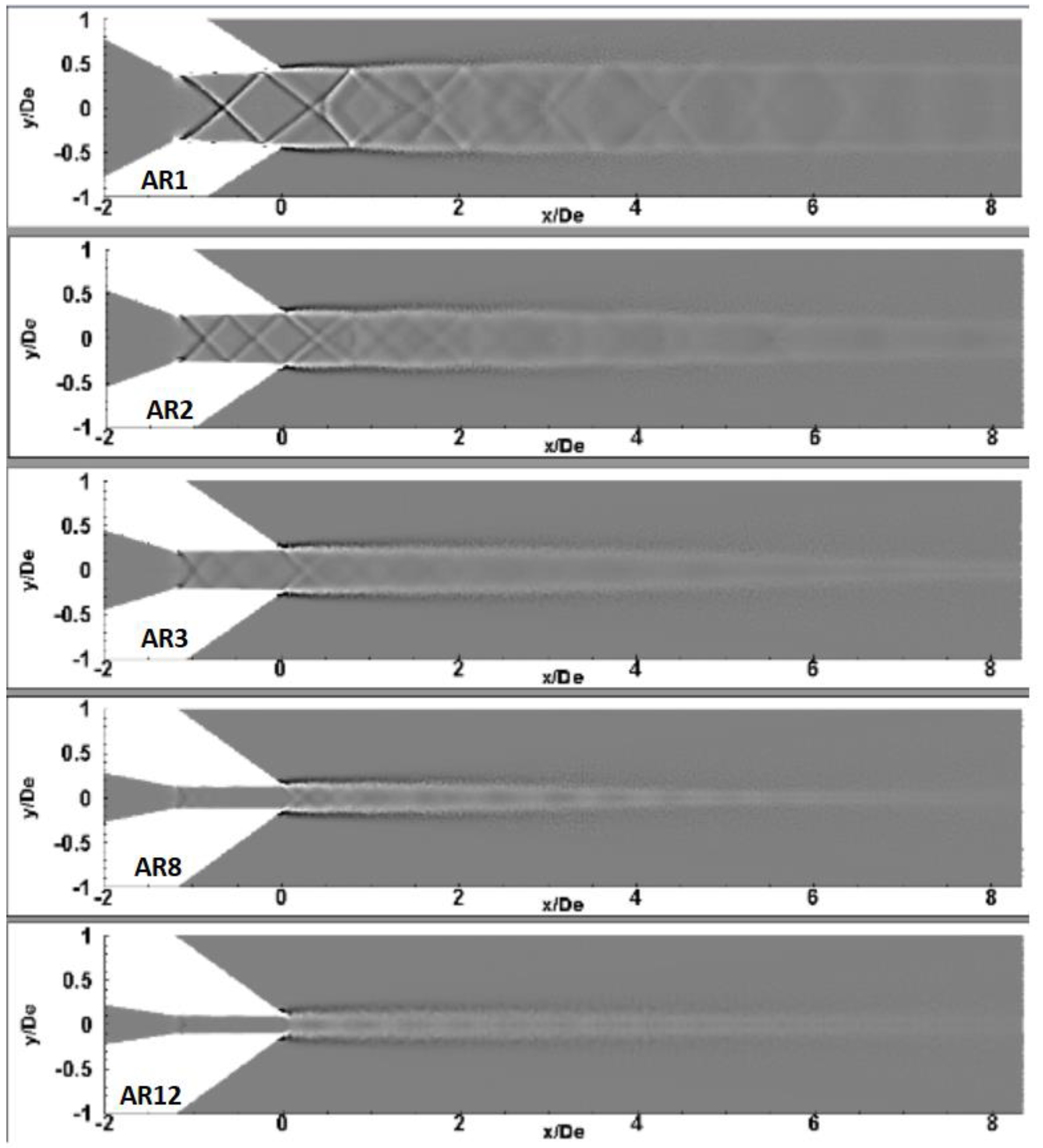
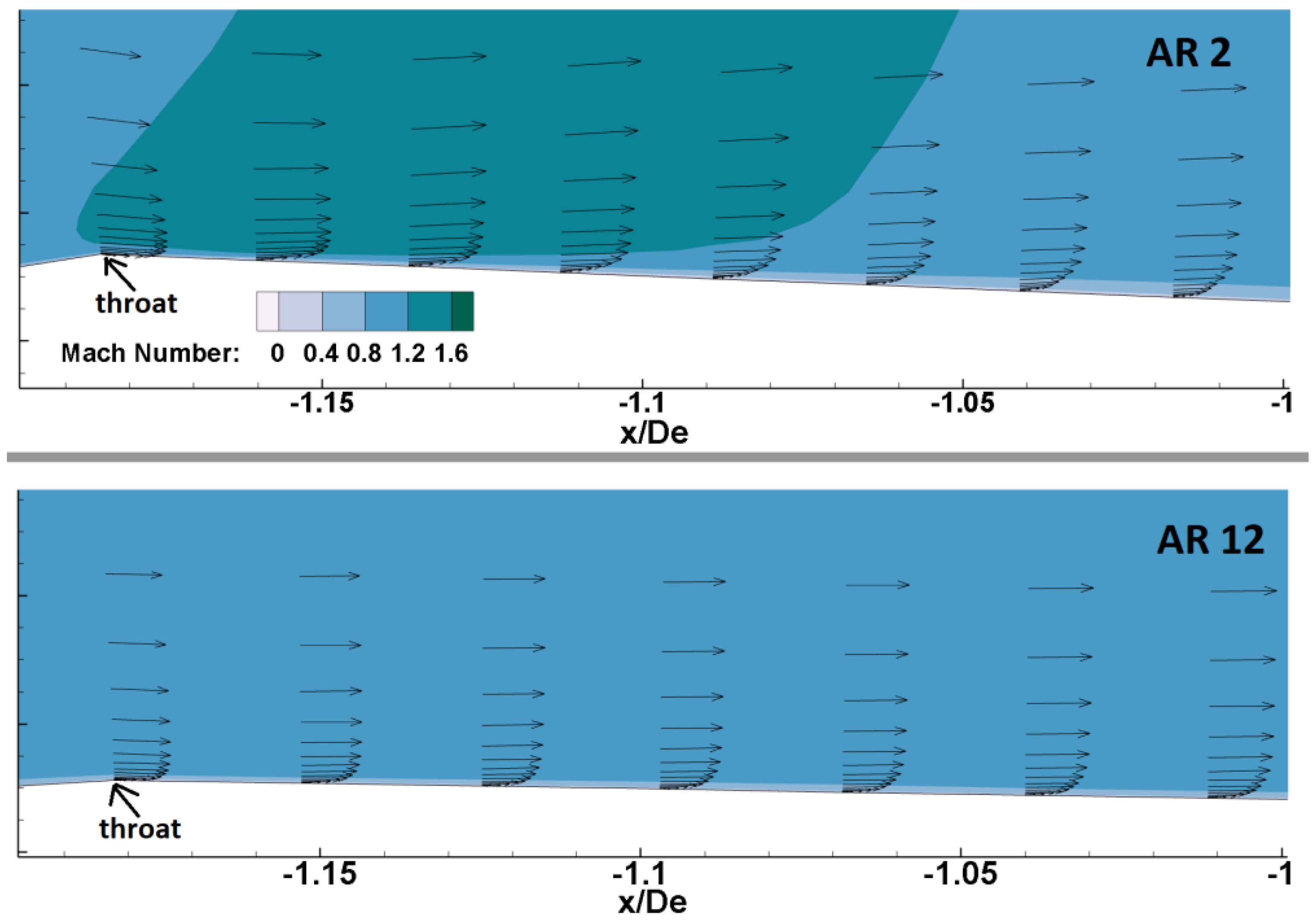


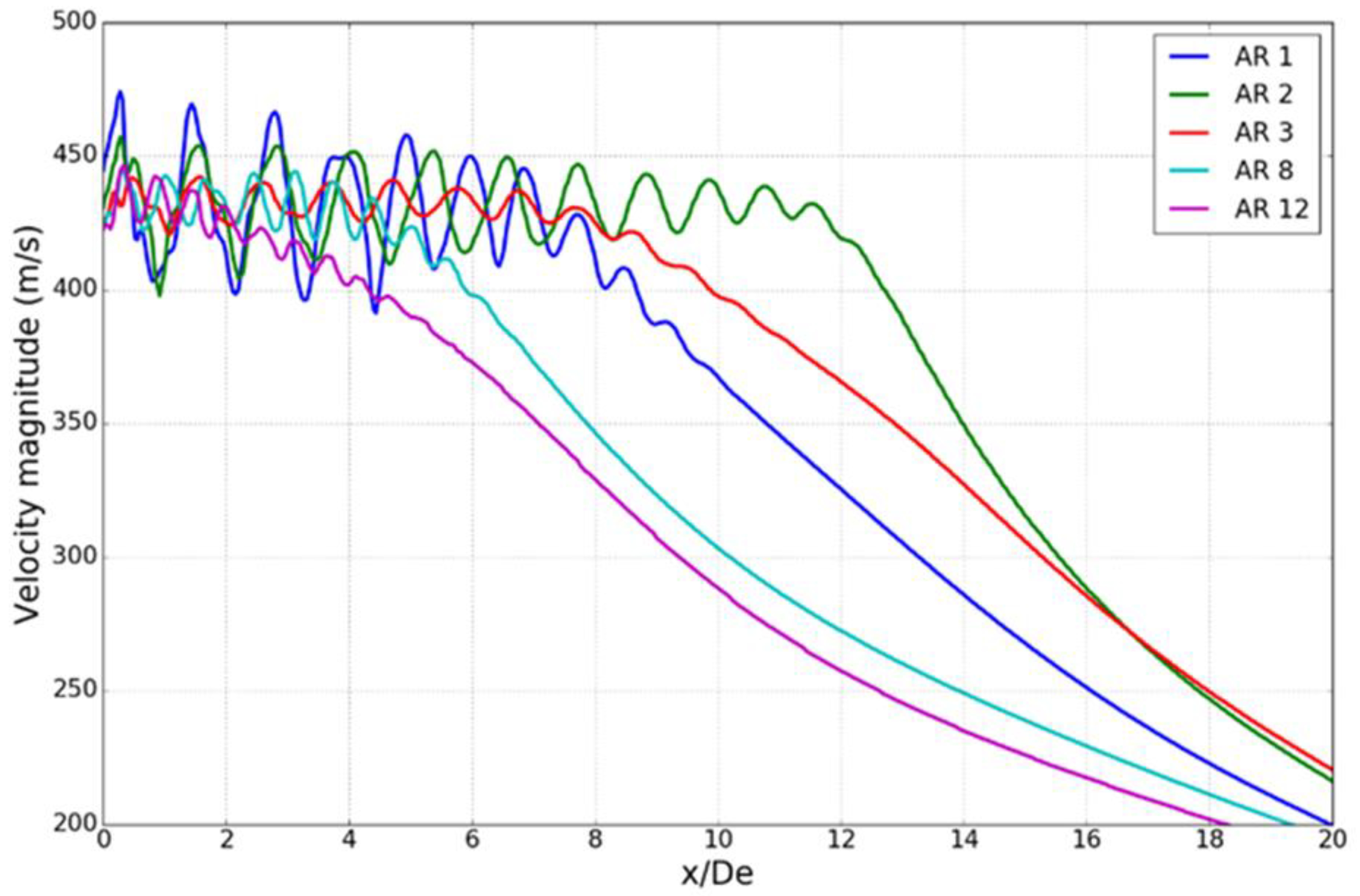
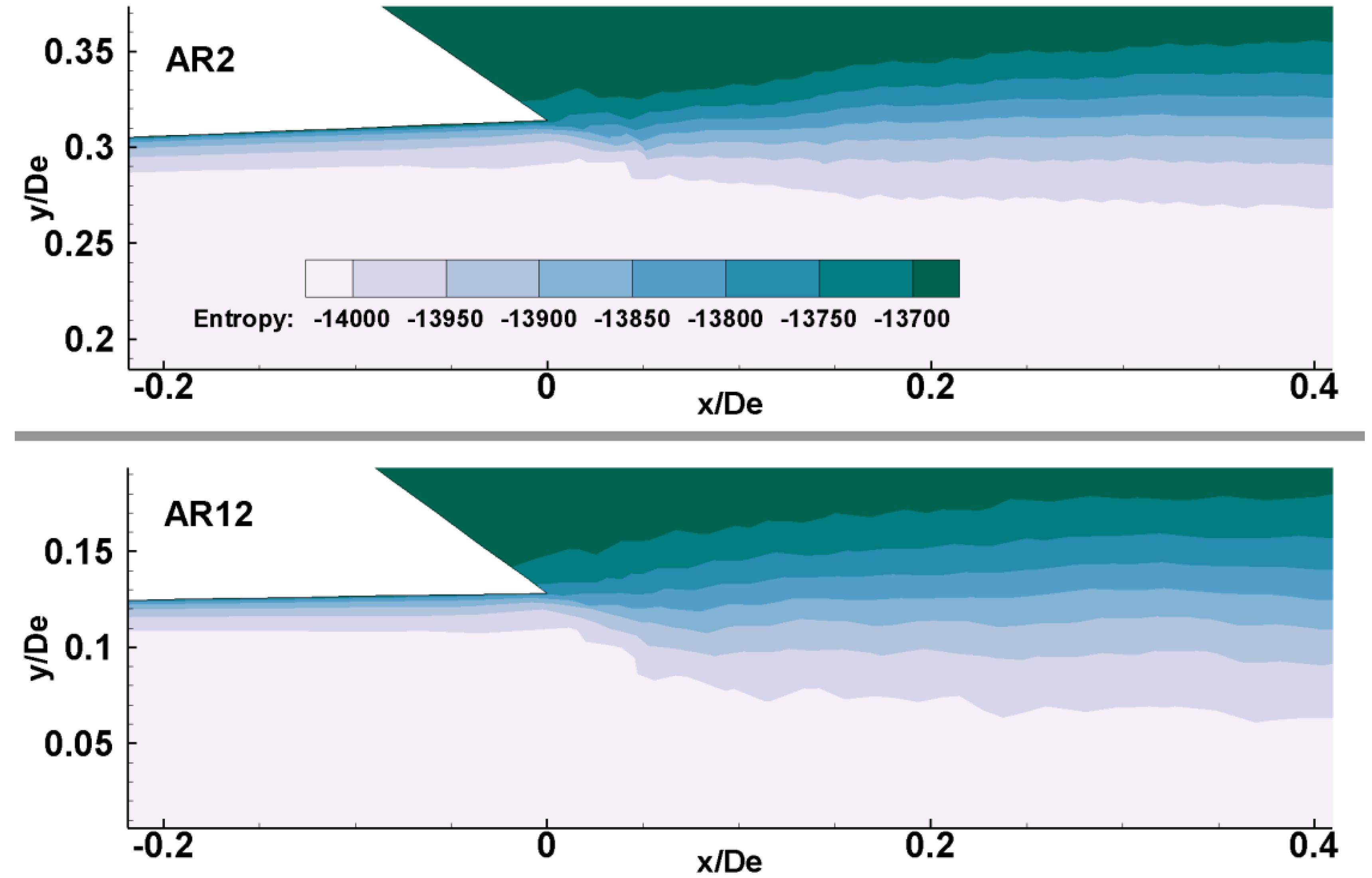
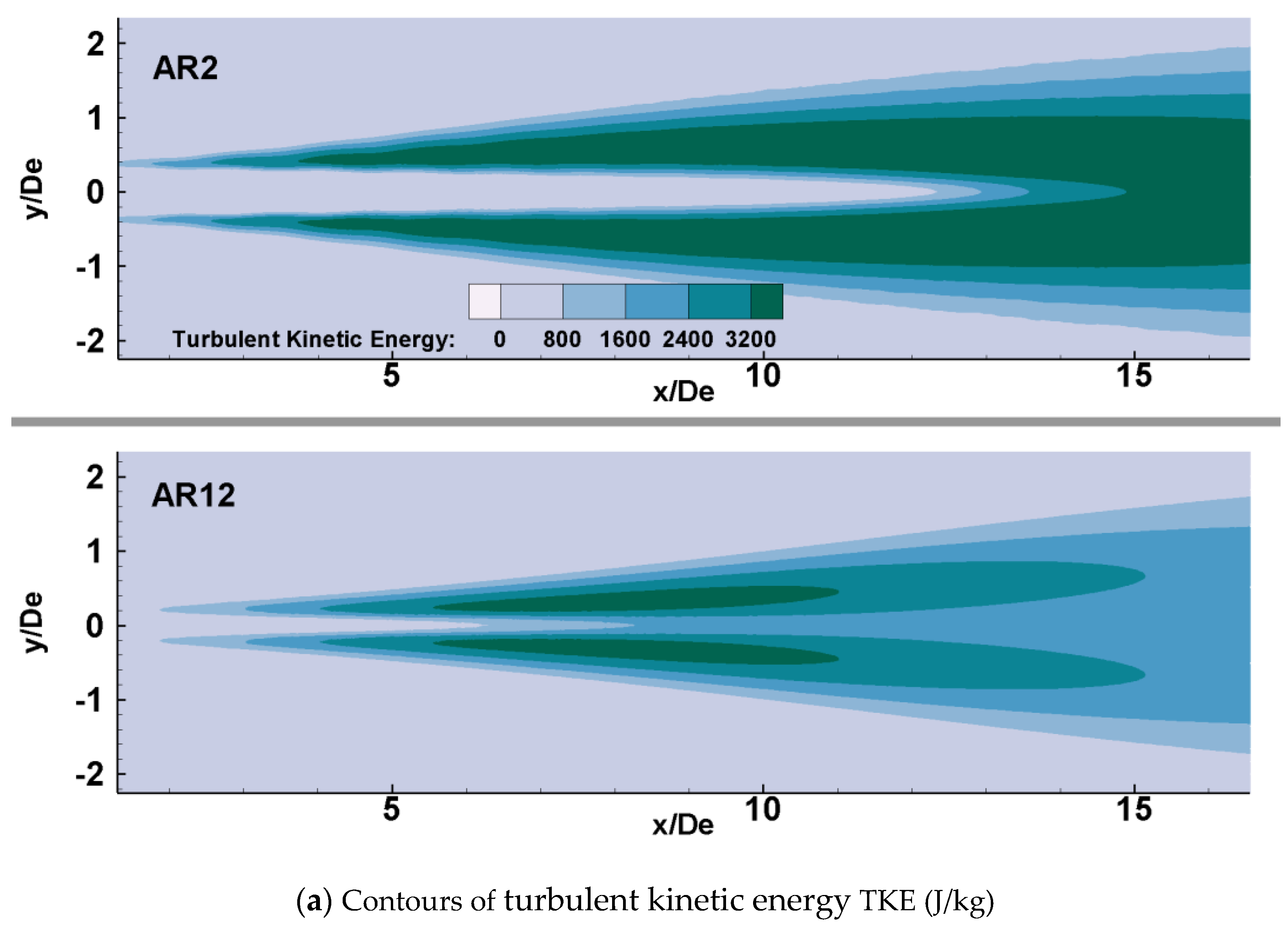



| Aspect Ratio | Abbreviation |
|---|---|
| 1 | AR1 |
| 2 | AR2 |
| 3 | AR3 |
| 8 | AR8 |
| 12 | AR12 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bhide, K.; Siddappaji, K.; Abdallah, S. Aspect Ratio Driven Relationship between Nozzle Internal Flow and Supersonic Jet Mixing. Aerospace 2021, 8, 78. https://doi.org/10.3390/aerospace8030078
Bhide K, Siddappaji K, Abdallah S. Aspect Ratio Driven Relationship between Nozzle Internal Flow and Supersonic Jet Mixing. Aerospace. 2021; 8(3):78. https://doi.org/10.3390/aerospace8030078
Chicago/Turabian StyleBhide, Kalyani, Kiran Siddappaji, and Shaaban Abdallah. 2021. "Aspect Ratio Driven Relationship between Nozzle Internal Flow and Supersonic Jet Mixing" Aerospace 8, no. 3: 78. https://doi.org/10.3390/aerospace8030078
APA StyleBhide, K., Siddappaji, K., & Abdallah, S. (2021). Aspect Ratio Driven Relationship between Nozzle Internal Flow and Supersonic Jet Mixing. Aerospace, 8(3), 78. https://doi.org/10.3390/aerospace8030078






