Numerical Investigation on Hybrid Rocket Motors with Star-Segmented Rotation Grain
Abstract
:1. Introduction
2. Model Description
2.1. Geometry Models
2.2. Numerical Models
2.2.1. Gas Governing Equations
2.2.2. Turbulence Model
2.2.3. Chemical Reaction Model
2.2.4. Fluid–Solid Coupling and Fuel Regression Rate Model
2.2.5. Boundary Conditions
2.3. Model Validation
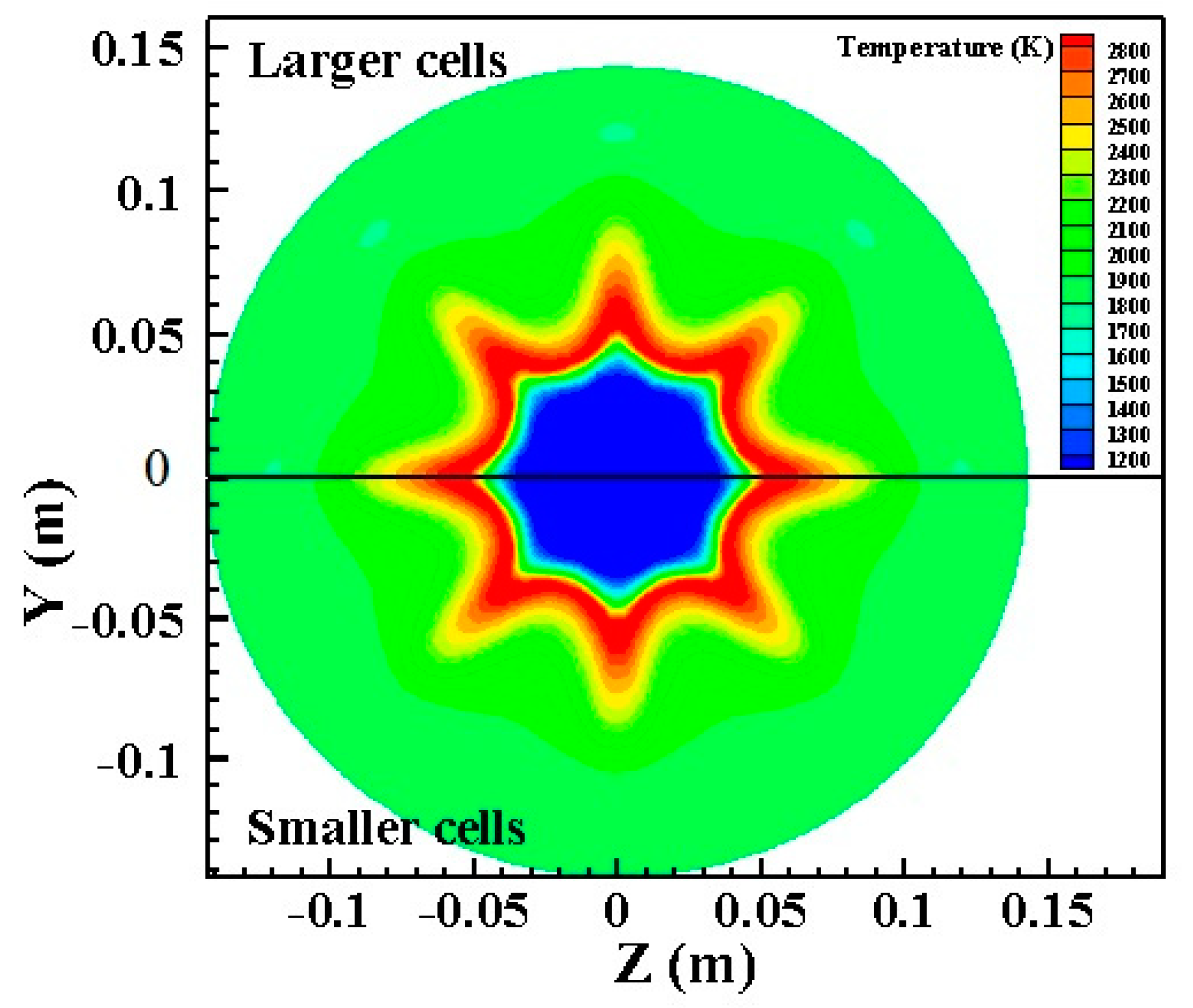
2.3.1. Grid Independence Verification
2.3.2. Numerical Model Verification
3. Results and Discussion
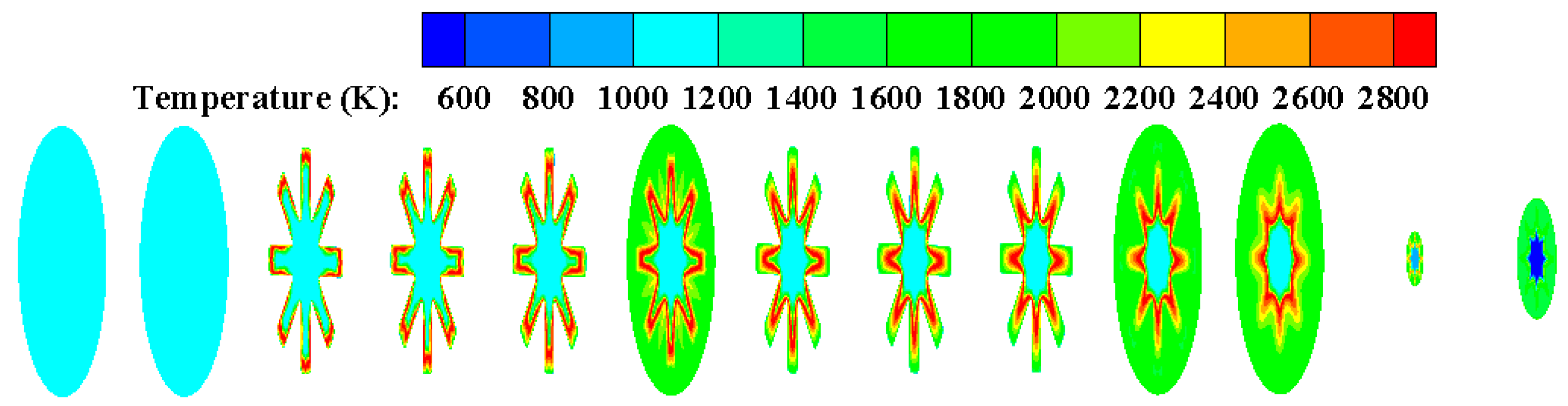
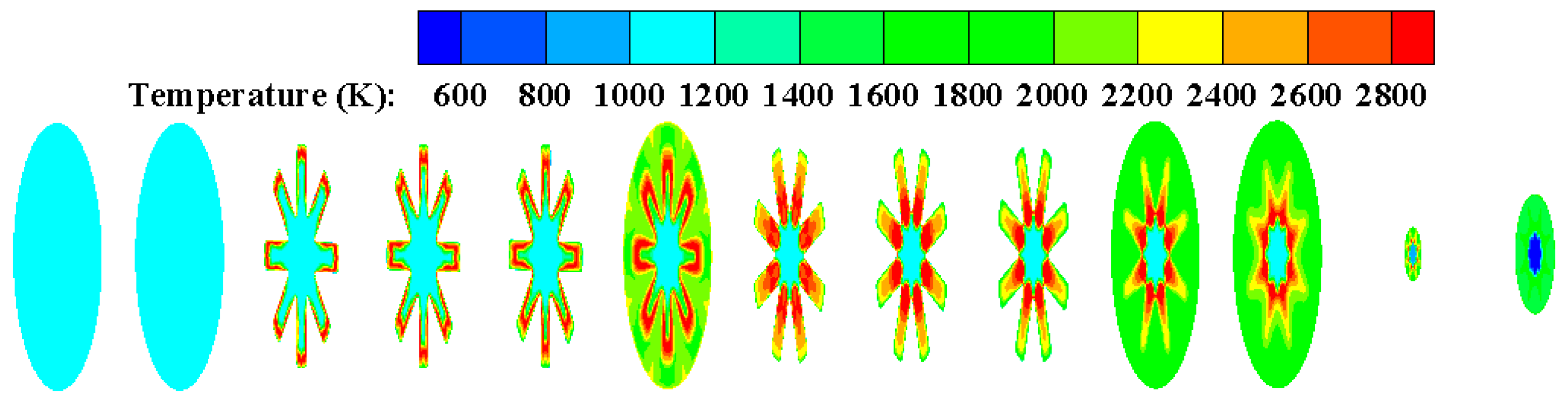
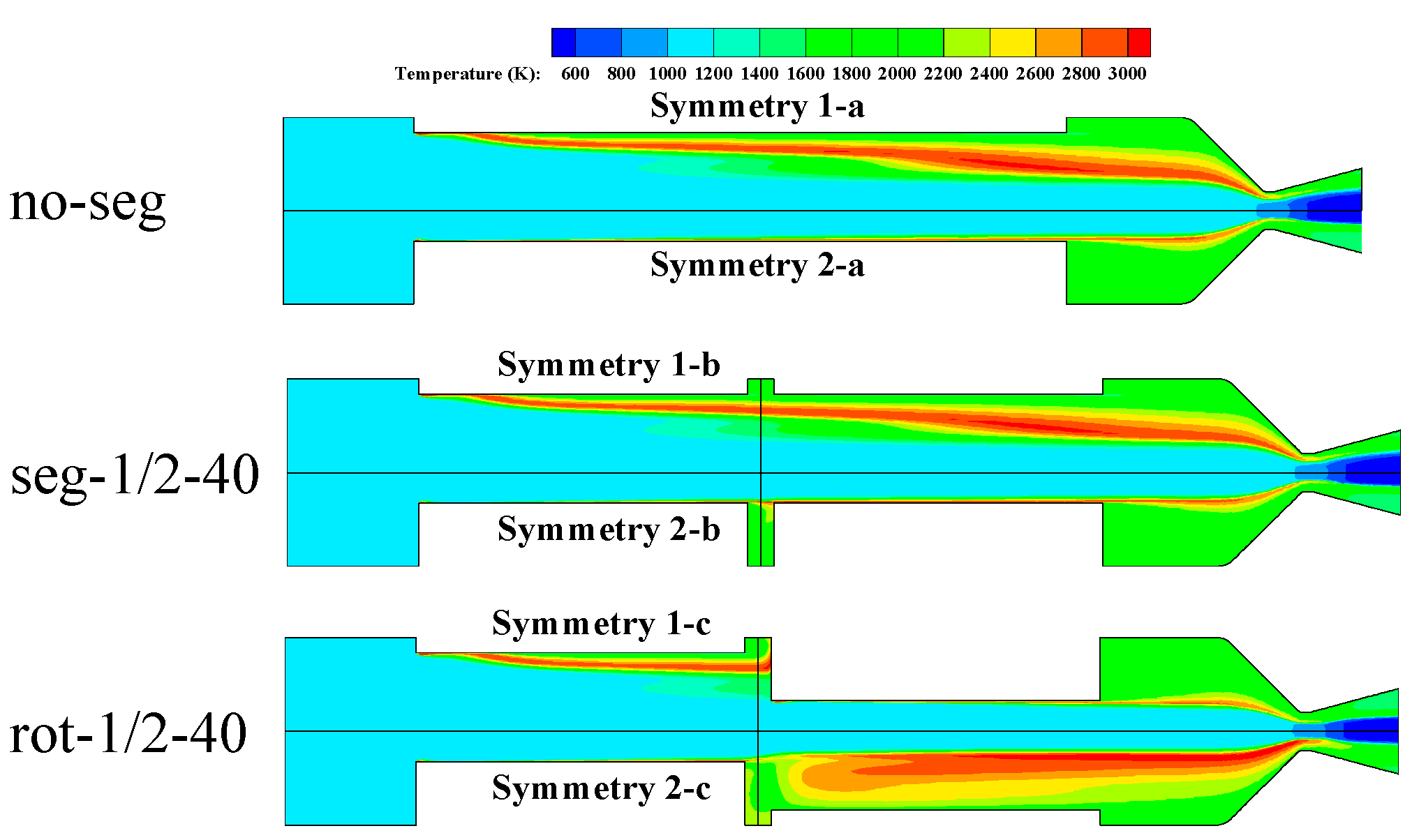
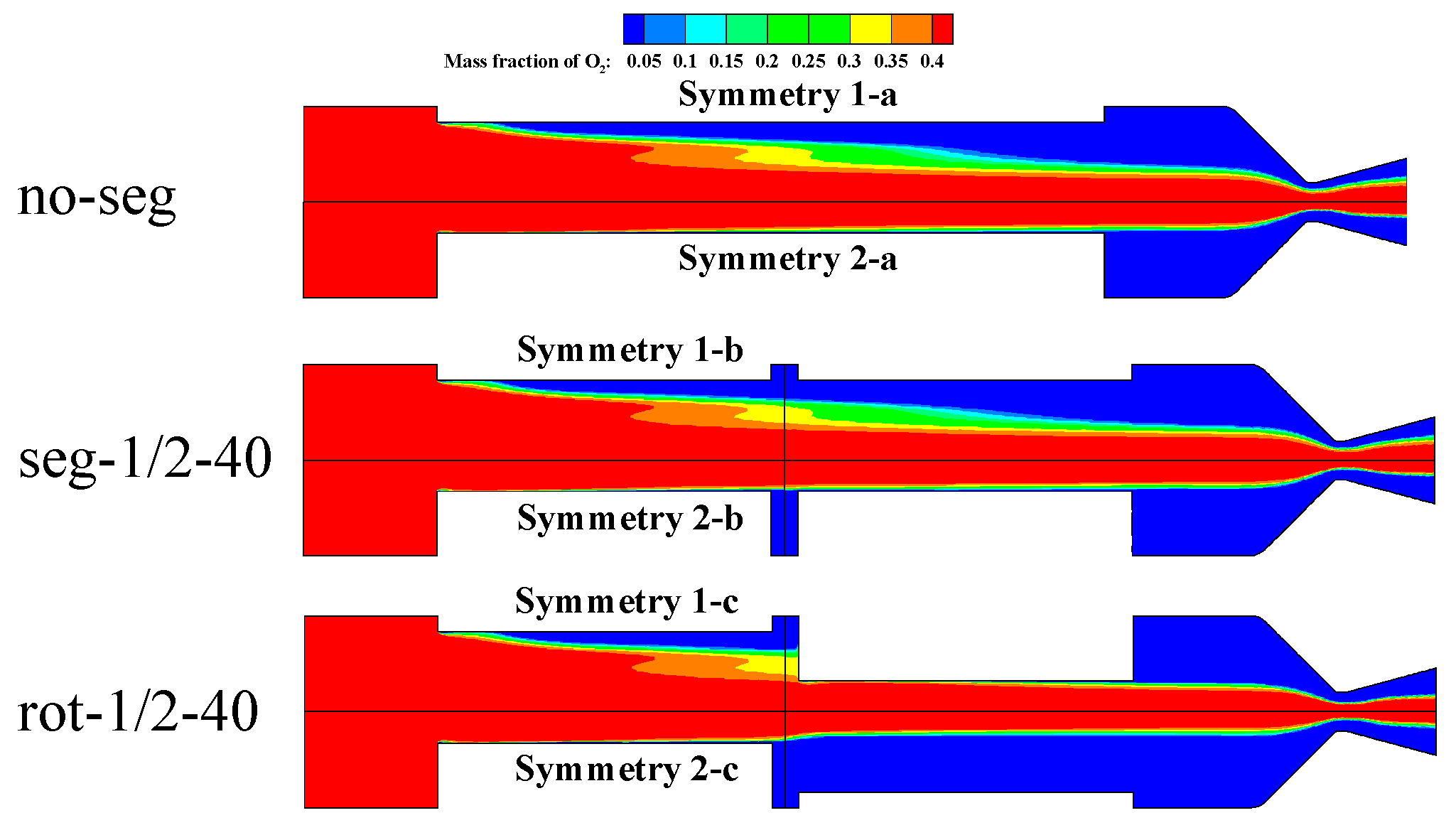
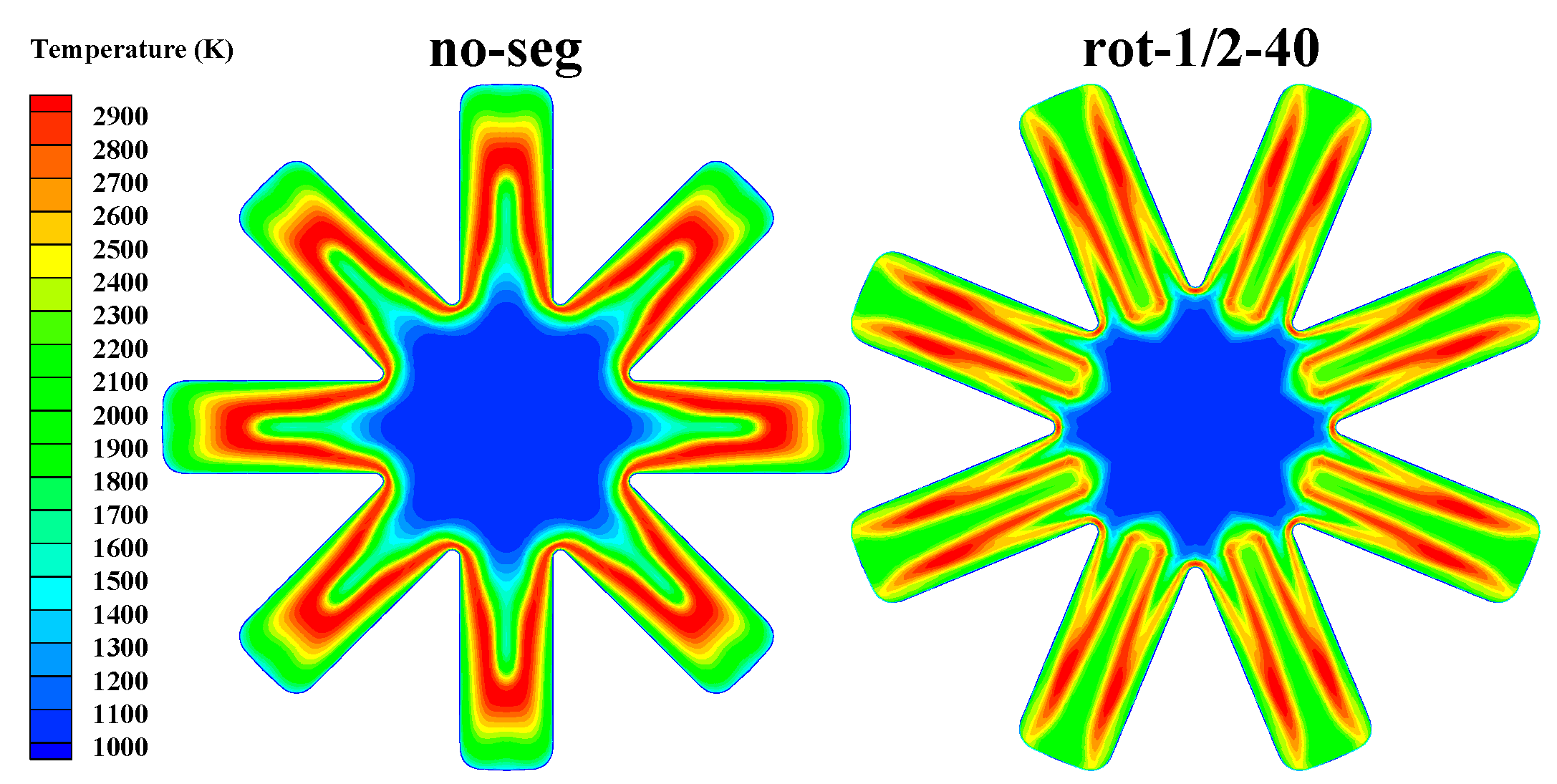
3.1. Combustion Flow Field Characteristics
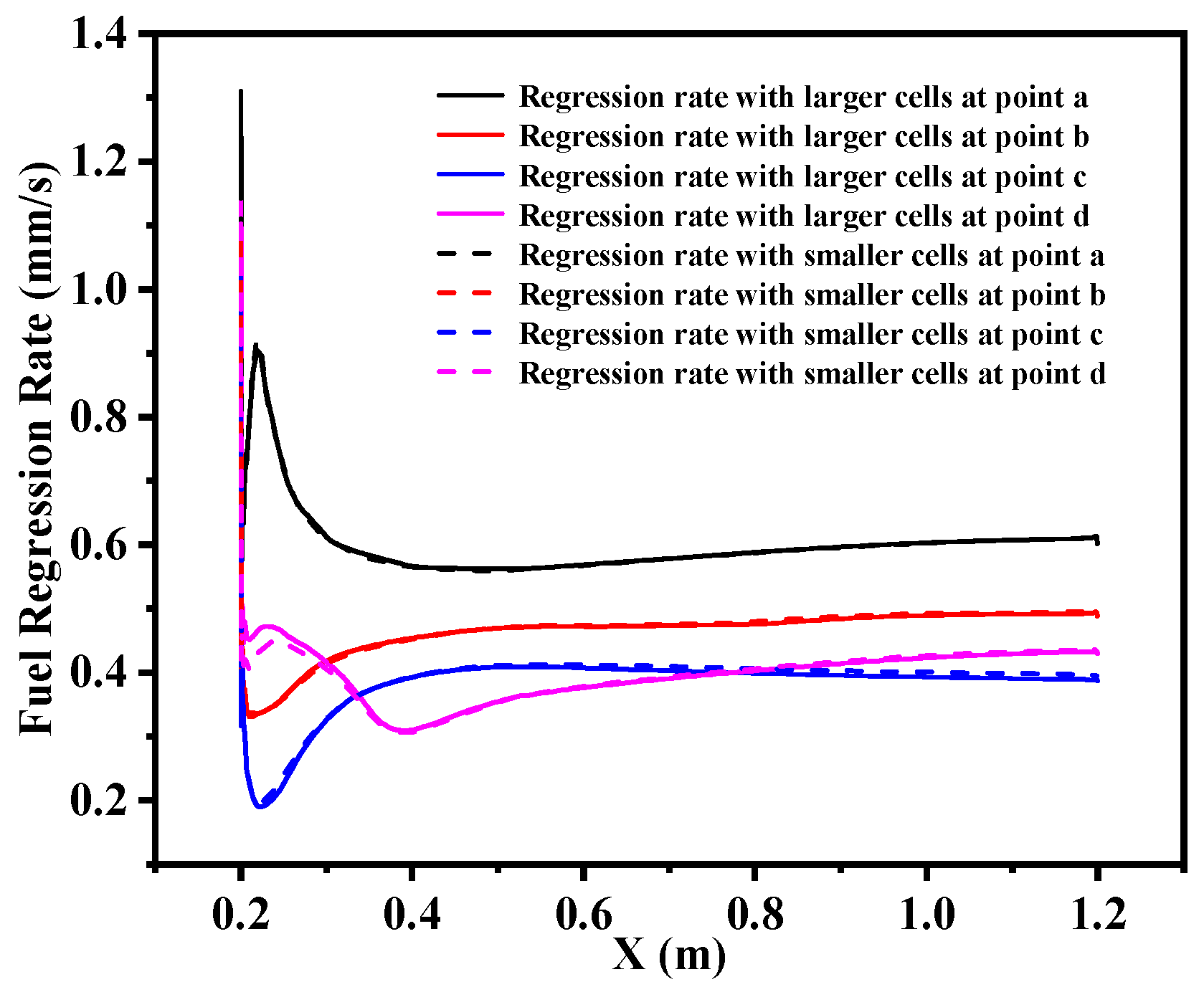
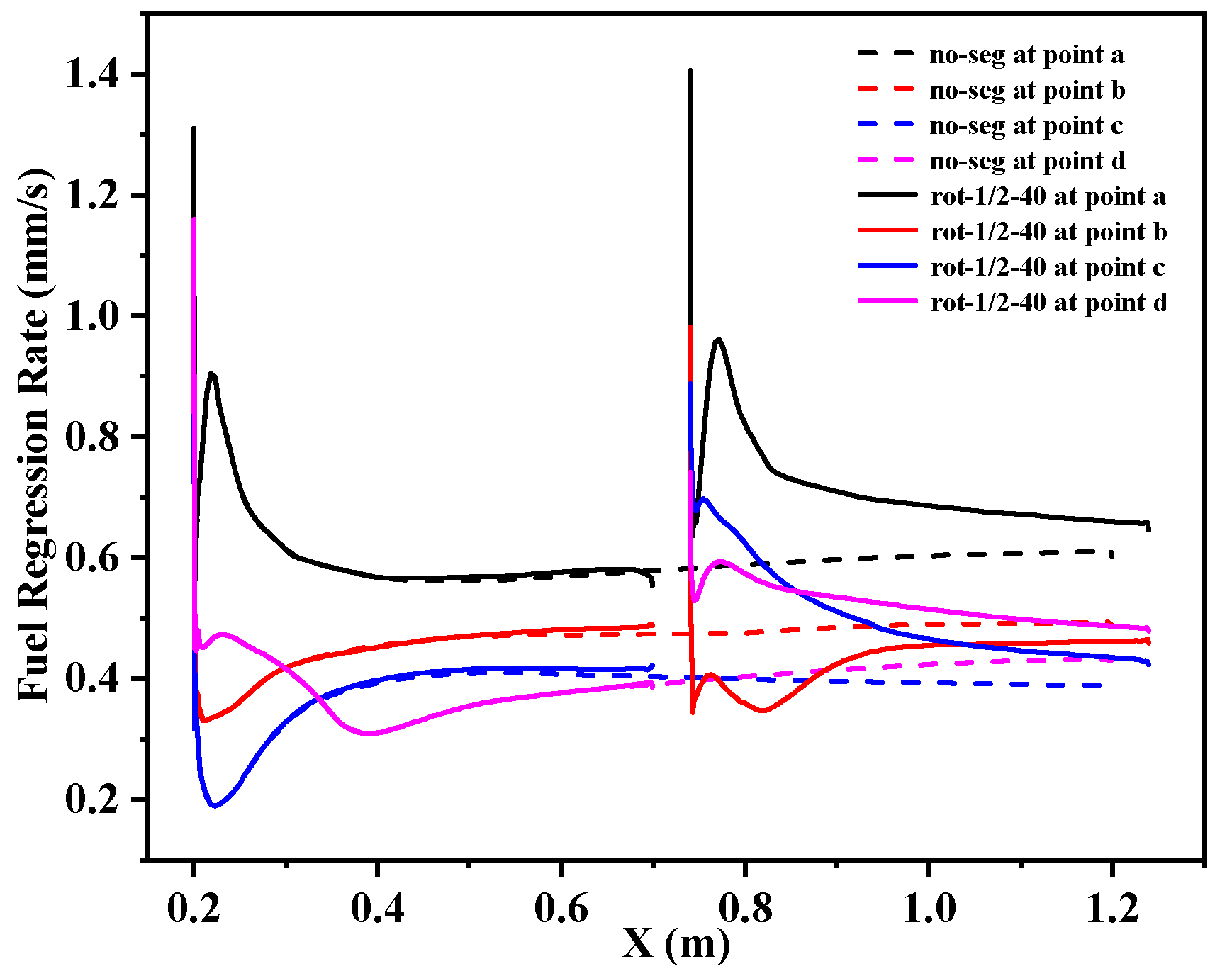
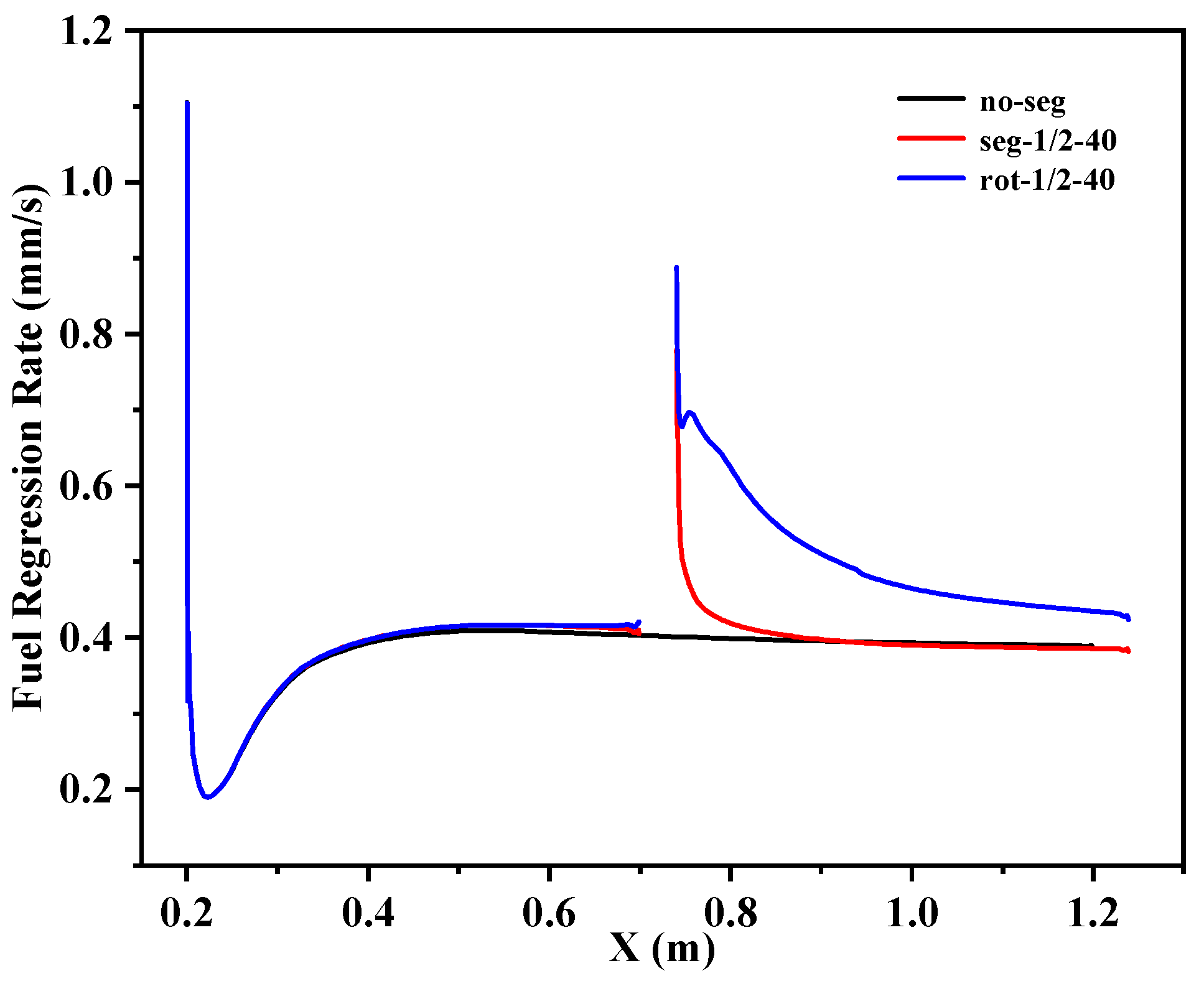
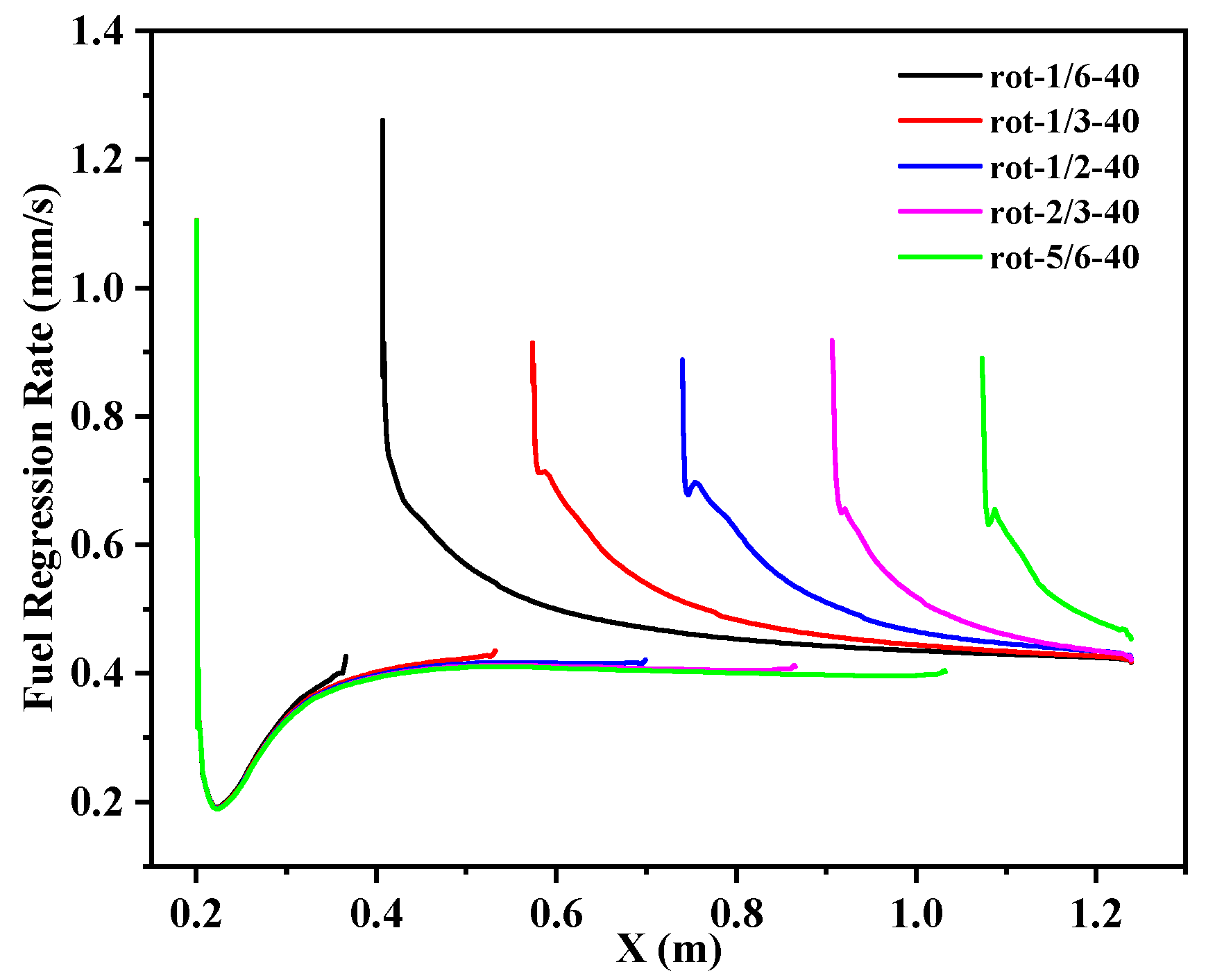
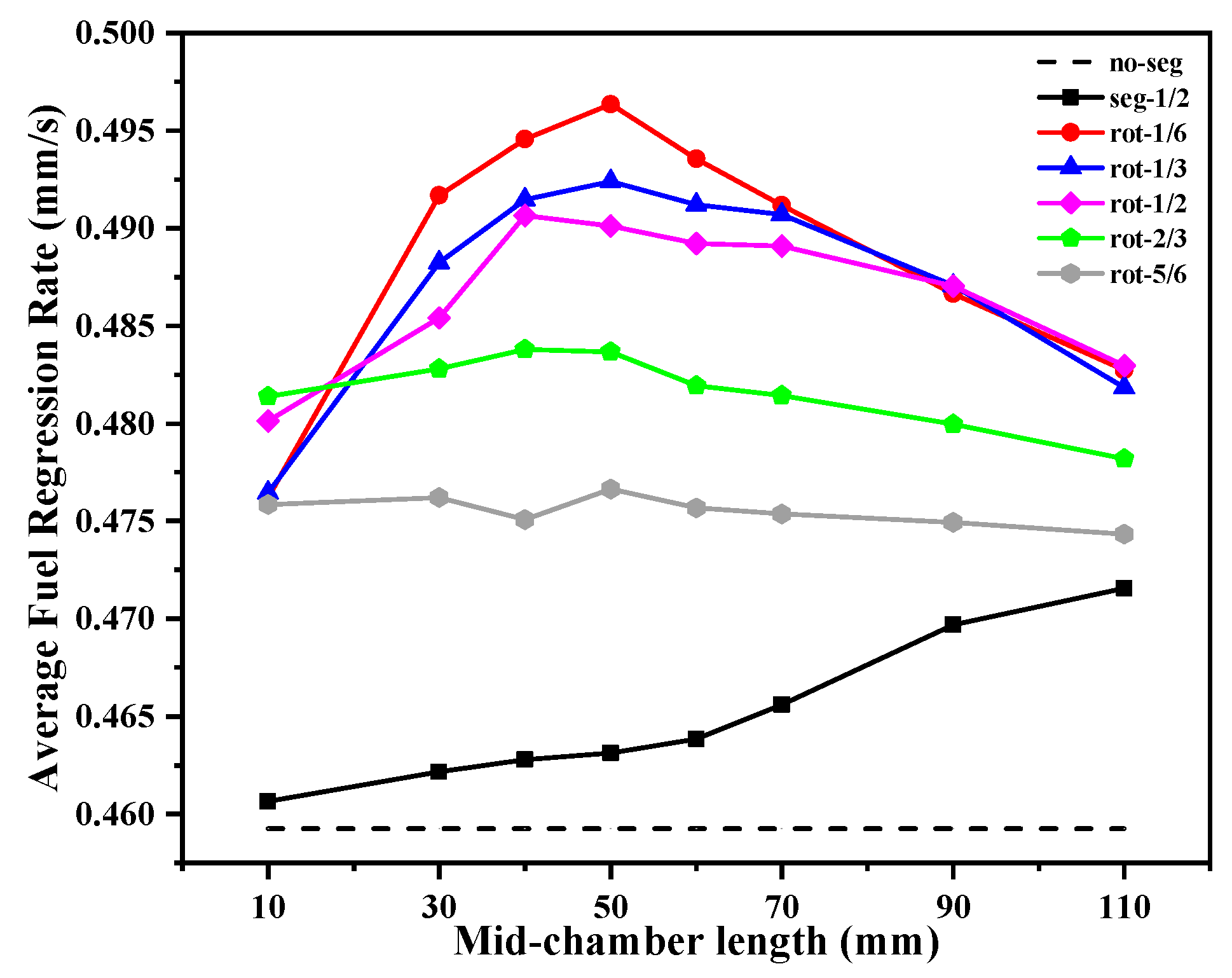
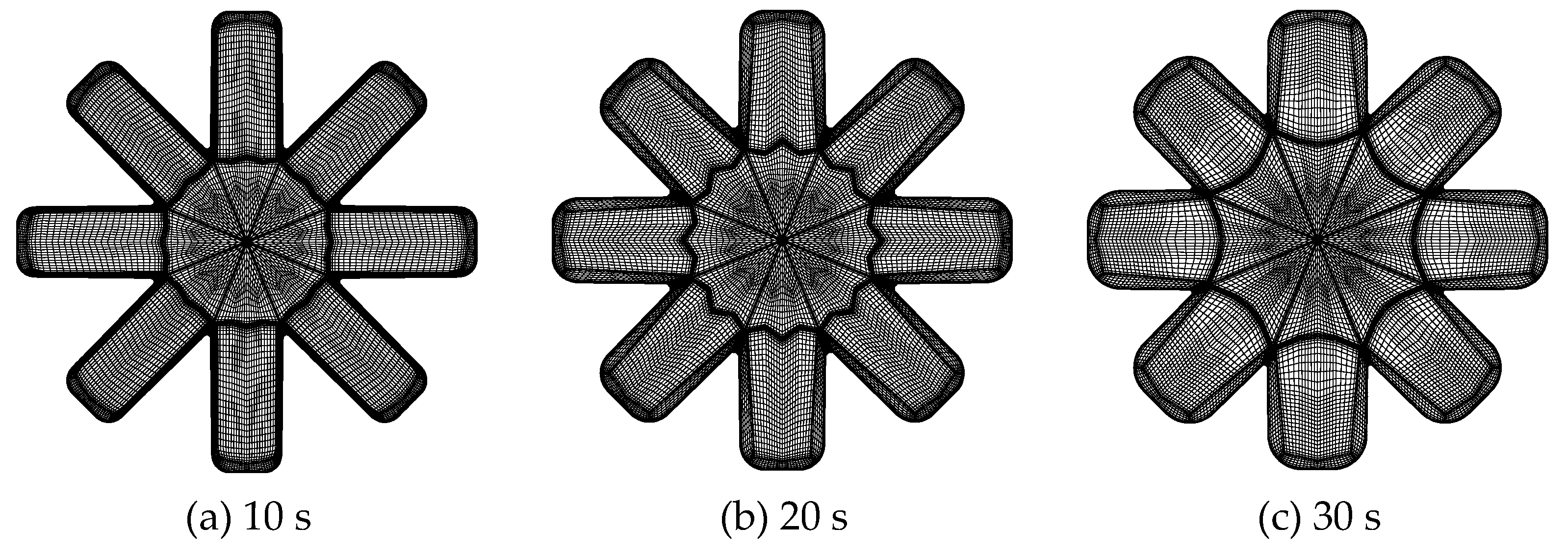
3.2. Regression Rates
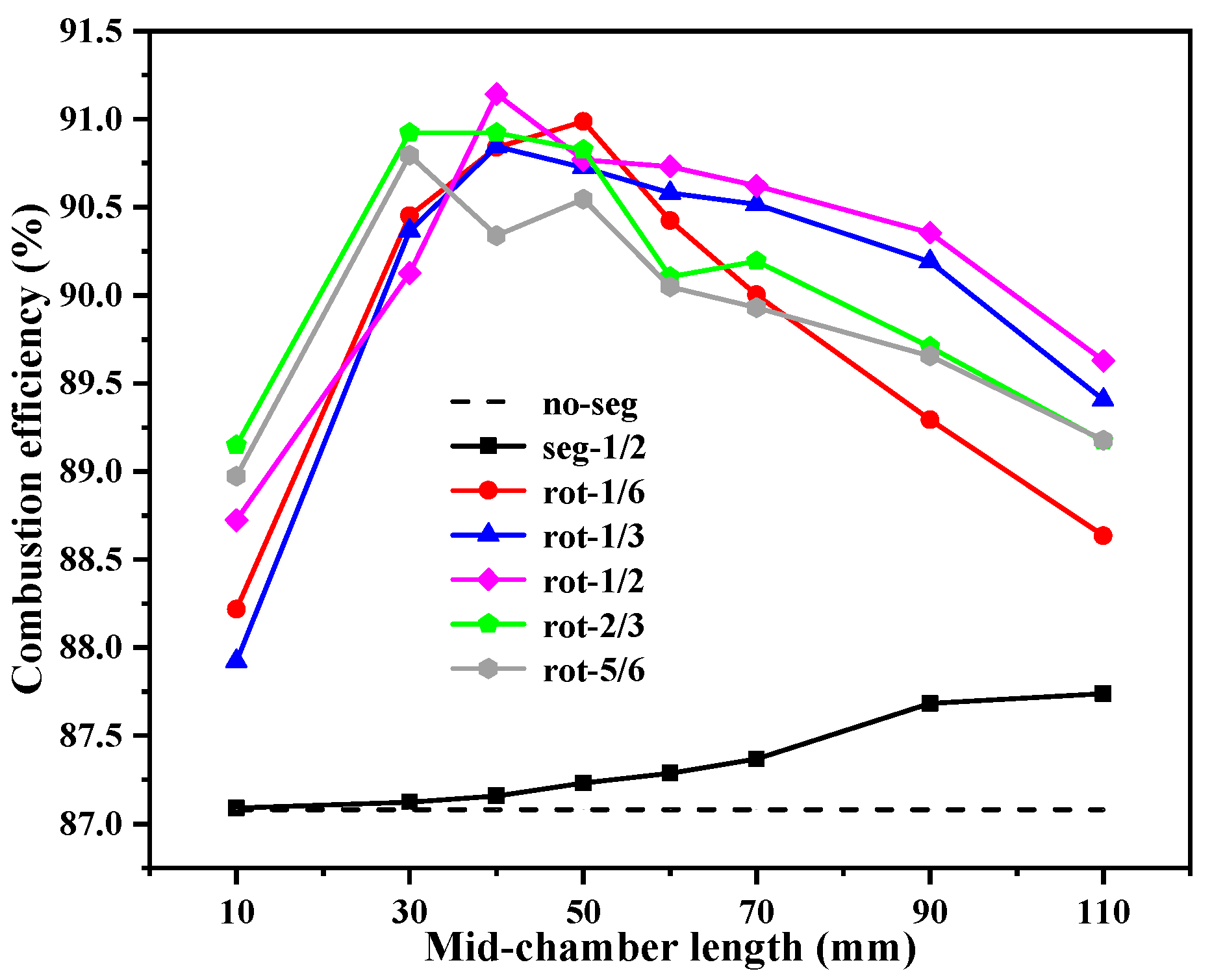
3.3. Combustion Efficiency
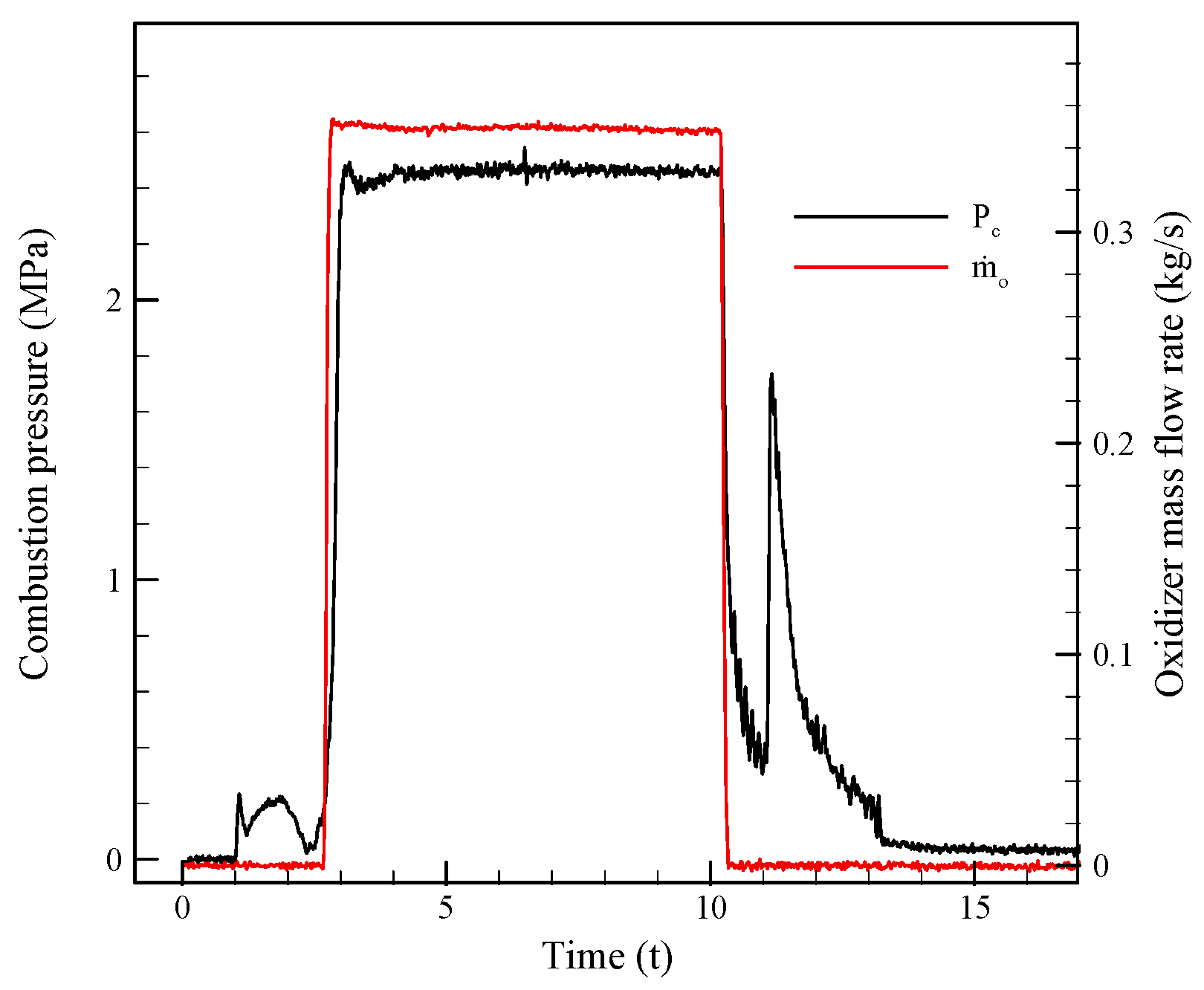
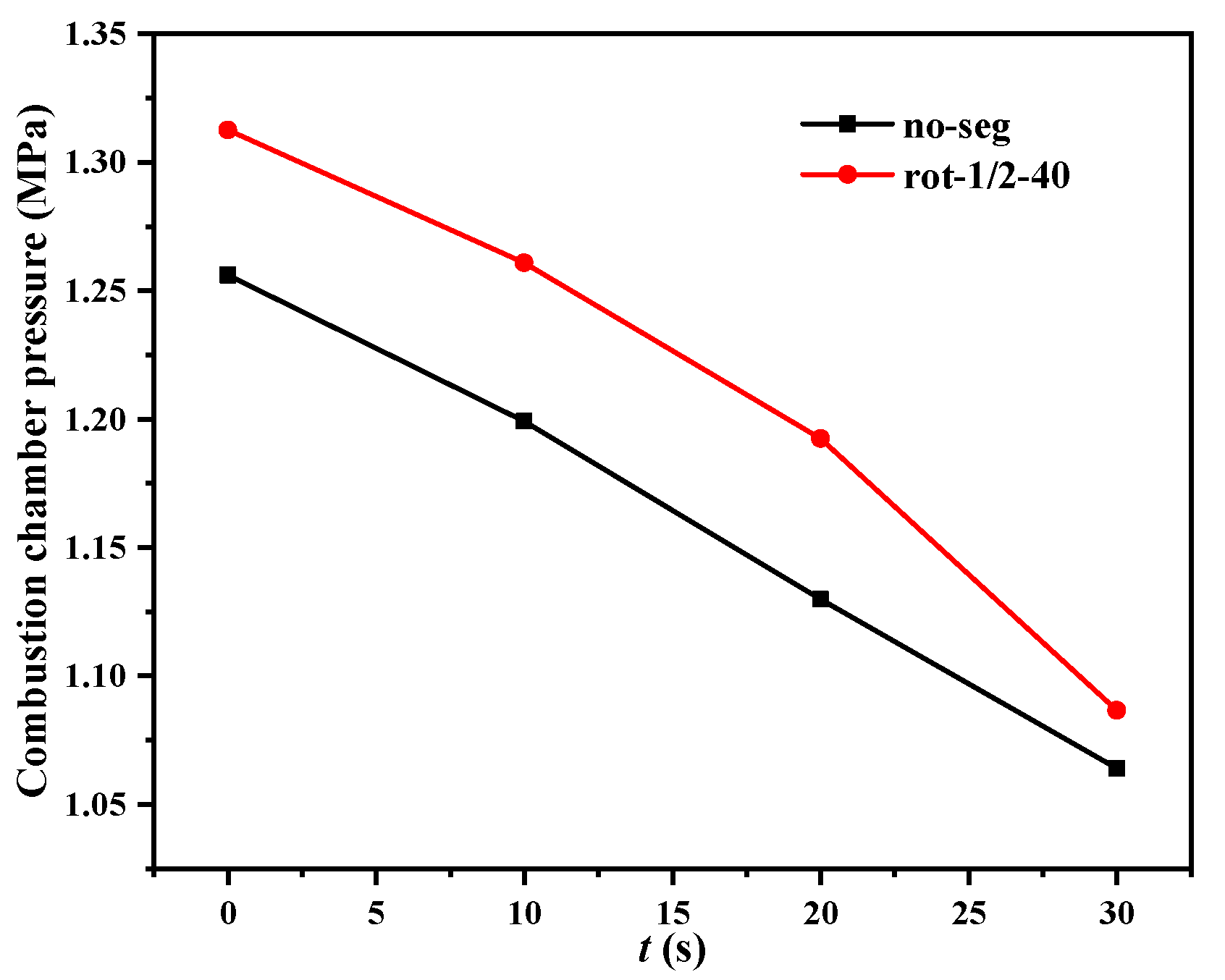
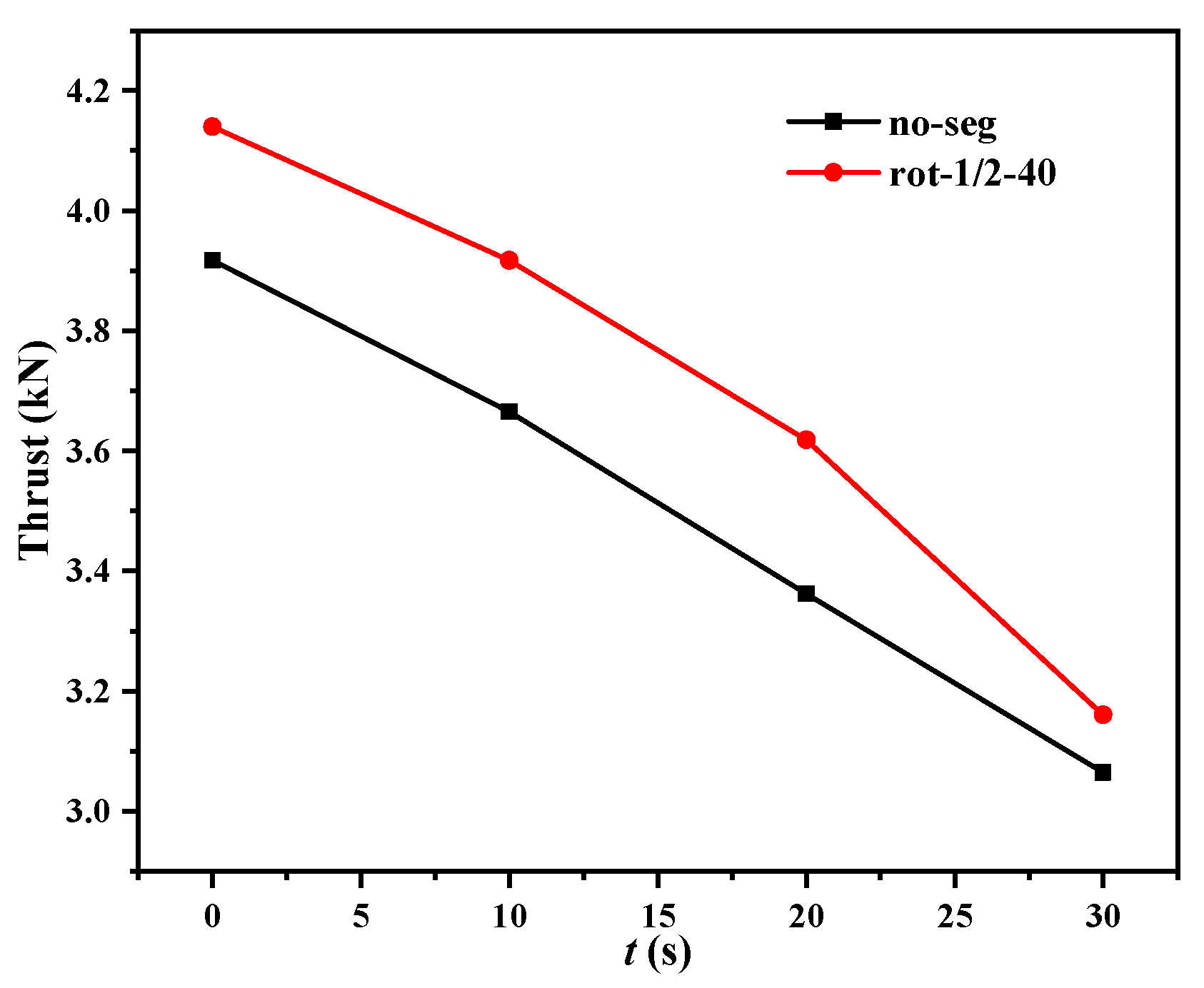
3.4. Full-Time Motor Performances
4. Conclusions
- The segmented rotation grain configuration has little influence on the combustion flow field in fore-section, while the flow field in the aft-section is disturbed by the front-end face of the aft-section grain. The flame in the aft-section shifts towards the central core flow and the oxidizer in the slot of the star in the aft-section is depleted more. The highest temperature in case rot-1/2-40 increases by about 83 K in comparison with that in case no-seg.
- The fuel regression rate distributions along the motor axis in the fore-section grain are only slightly affected by the segmented rotation grain configuration, while the segmented rotation grain configuration can enhance the average fuel regression rates in the aft-section grain. When the segmented position increases (from 1/6 to 5/6), the average regression rate in the fore-section grain increases gradually. The average regression rates in fore-section grain vary little with the increase in the mid-chamber length. As for cases with the rotation angle of 22.5°, the average regression rate in the aft-section grain rises with the increase in segmented position. The average regression rate in aft-section grain in case rot-5/6-10 is the highest, which increases by 25.04% compared with that in case no-seg. The average fuel regression rate of the entire grain in case rot-1/6-50 is the highest, with a maximum regression rate of 0.4963 mm/s.
- The segmented rotation grain configuration has a positive influence on the combustion efficiency. For cases with the rotation angle of 22.5°, the general change tendency of combustion efficiency is that it increases at first and then declines with the increase in mid-chamber length. When the mid-chamber length is not larger than 30 mm, the cases with the segmented position of 2/3 achieve higher combustion efficiency. While the mid-chamber length is greater than 60 mm, the cases with the segmented position of 1/2 reach higher combustion efficiency. In comparison with the base case, the combustion efficiency in case rot-1/2-40 increases 4.06%.
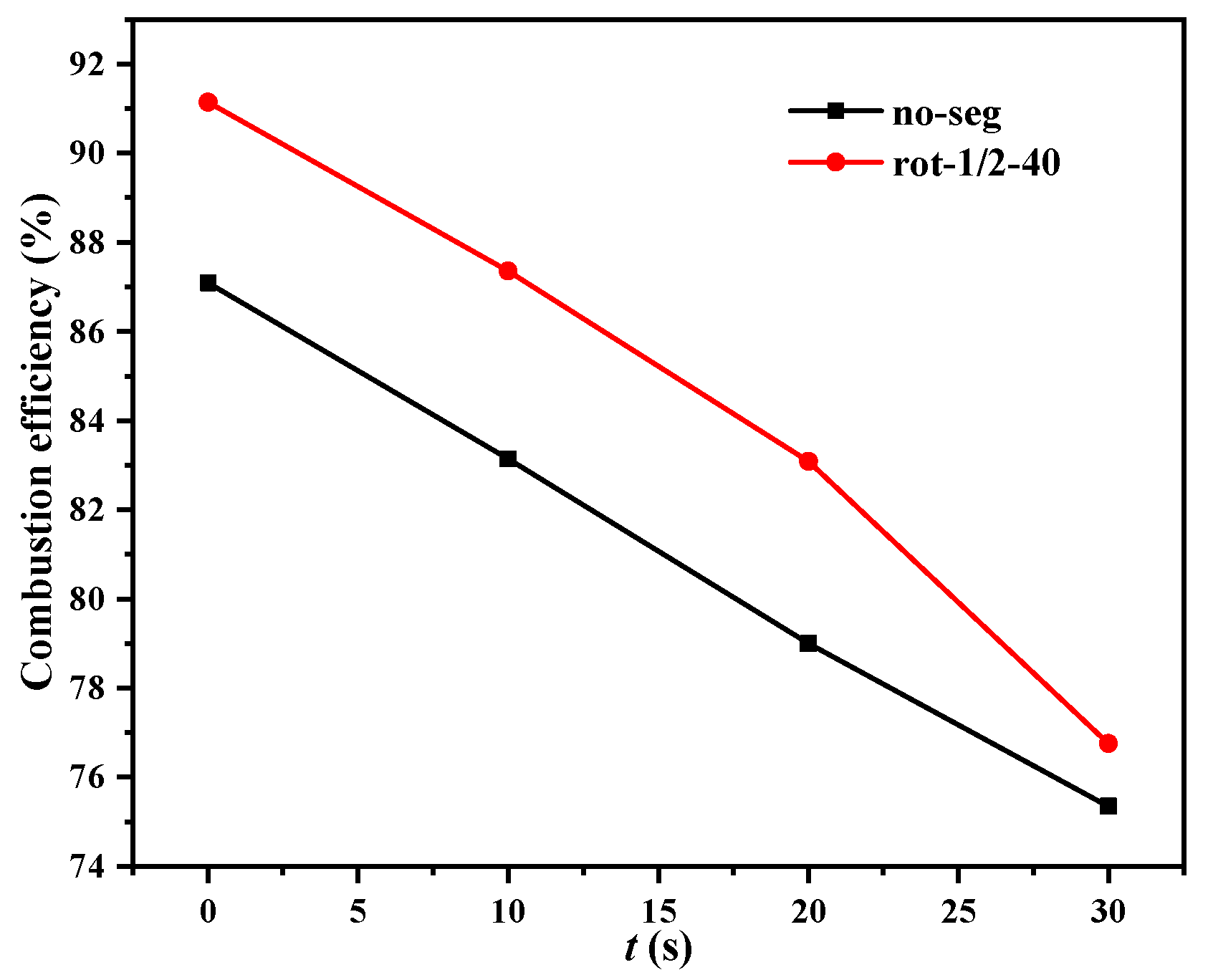
- The combustion chamber pressure, thrust, average fuel regression rate, and combustion efficiency decrease with the increase in the working time, and they are improved by adopting segmented rotation grain configuration during the entire period of operation. The combustion efficiency increases by 1.40–4.21% during the motor operation.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Di Martino, G.D.; Carmicino, C.; Mungiguerra, S.; Savino, R. The Application of Computational Thermo-Fluid-Dynamics to the Simulation of Hybrid Rocket Internal Ballistics with Classical or Liquefying Fuels: A Review. Aerospace 2019, 6, 56. [Google Scholar] [CrossRef] [Green Version]
- Oztan, C.; Coverstone, V. Utilization of additive manufacturing in hybrid rocket technology: A review. Acta Astronaut. 2020, 180, 130–140. [Google Scholar] [CrossRef]
- Rashkovskiy, S.A.; Yakush, S.E. Numerical simulation of low-melting temperature solid fuel regression in hybrid rocket engines. Acta Astronaut. 2020, 176, 710–716. [Google Scholar] [CrossRef]
- Vignesh, B.; Kumar, R. Effect of multi-location swirl injection on the performance of hybrid rocket motor. Acta Astronaut. 2020, 176, 111–123. [Google Scholar] [CrossRef]
- Dinesh, M.; Rajput, S.S.; Kumar, R. Protrusion effect on the performance of hybrid rocket with liquefying and non-liquefying fuels. Acta Astronaut. 2021, 178, 536–547. [Google Scholar] [CrossRef]
- Okninski, A. On use of hybrid rocket propulsion for suborbital vehicles. Acta Astronaut. 2018, 145, 1–10. [Google Scholar] [CrossRef]
- Yun, Y.; Seo, J.; Park, K.; Huh, J.; Lim, J.; Kwon, S. Integration validation of key components for small sounding rockets. Aerosp. Sci. Technol. 2020, 100, 105823. [Google Scholar] [CrossRef]
- Ahn, B.; Huh, J.; Bhosale, V.K.; Kwon, S. Three-Dimensionally Printed Polylactic Acid as Solid Fuel for Hydrogen Peroxide Hybrid Rockets. J. Propuls. Power 2021, 37, 171–175. [Google Scholar] [CrossRef]
- Davydenko, N.A.; Gollender, R.G.; Gubertov, A.M.; Mironov, V.V.; Volkov, N.N. Hybrid rocket engines: The benefits and prospects. Aerosp. Sci. Technol. 2007, 11, 55–60. [Google Scholar] [CrossRef]
- Zhu, H.; Tian, H.; Cai, G. Hybrid uncertainty-based design optimization and its application to hybrid rocket motors for manned lunar landing. Chin. J. Aeronaut. 2017, 30, 719–725. [Google Scholar] [CrossRef]
- Tian, H.; Li, X.; Yu, N.; Cai, G. Numerical and experimental investigation on the effects of aft mixing chamber diaphragm in hybrid rocket motor. Sci. China Technol. Sci. 2013, 56, 2721–2731. [Google Scholar] [CrossRef]
- Li, X.; Tian, H.; Cai, G. Numerical analysis of fuel regression rate distribution characteristics in hybrid rocket motors with different fuel types. Sci. China Technol. Sci. 2013, 56, 1807–1817. [Google Scholar] [CrossRef]
- Li, X.; Tian, H.; Yu, N.; Cai, G. Three-Dimensional Numerical Simulation of Two-Phase Flow in Hybrid Rocket Motor. In Proceedings of the 49th AIAA/ASME/SAE/ASEE Joint Propulsion Conference, San Jose, CA, USA, 14–17 July 2013. [Google Scholar]
- Lee, C.; Na, Y.; Lee, G. The Enhancement of Regression Rate of Hybrid Rocket Fuel by Helical Grain Configuration and Swirl Flow. In Proceedings of the 41st AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Tucson, AZ, USA, 10–13 July 2005. [Google Scholar]
- Whitmore, S.A.; Walker, S.D.; Merkley, D.P.; Sobbi, M. High regression rate hybrid rocket fuel grains with helical port struc-tures. J. Propuls. Power. 2015, 31, 1727–1738. [Google Scholar] [CrossRef] [Green Version]
- Zhang, S.; Hu, F.; Zhang, W. Numerical investigation on the regression rate of hybrid rocket motor with star swirl fuel grain. Acta Astronaut. 2016, 127, 384–393. [Google Scholar] [CrossRef]
- Tian, H.; Li, Y.; Li, C.; Sun, X. Regression rate characteristics of hybrid rocket motor with helical grain. Aerosp. Sci. Technol. 2017, 68, 90–103. [Google Scholar] [CrossRef]
- Tian, H.; He, L.; Zhu, H.; Wang, P.; Xu, X. Numerical and experimental investigation on hybrid rocket motor with two-hole segmented rotation grain. Aerosp. Sci. Technol. 2019, 92, 820–830. [Google Scholar] [CrossRef]
- Tian, H.; Duan, Y.; Zhu, H. Three-dimensional numerical analysis on combustion performance and flow of hybrid rocket motor with multi-segmented grain. Chin. J. Aeronaut. 2020, 33, 1181–1191. [Google Scholar] [CrossRef]
- Betelin, V.B.; Kushnirenko, A.G.; Smirnov, N.N.; Nikitin, V.F.; Tyurenkova, V.V.; Stamov, L.I. Numerical investigations of hybrid rocket engines. Acta Astronaut. 2018, 144, 363–370. [Google Scholar] [CrossRef]
- Mangeot, A.; William-Louis, M.; Gillard, P. Static and moving solid/gas interface modeling in a hybrid rocket engine. Acta Astronaut. 2018, 148, 89–98. [Google Scholar] [CrossRef]
- Zhu, H.; Li, M.; Tian, H.; Wang, P.; Yu, N.; Cai, G. Numerical and experimental investigations on injection effects of orifice injector plate in hybrid rocket motors. Acta Astronaut. 2019, 162, 275–283. [Google Scholar] [CrossRef]
- Shih, T.-H.; Liou, W.W.; Shabbir, A.; Yang, Z.; Zhu, J. A new k-ϵ eddy viscosity model for high reynolds number turbulent flows. Comput. Fluids 1995, 24, 227–238. [Google Scholar] [CrossRef]
- Chiaverini, M.J.; Harting, G.C.; Lu, Y.-C.; Kuo, K.K.; Peretz, A.; Jones, H.S.; Wygle, B.S.; Arves, J.P. Pyrolysis Behavior of Hybrid-Rocket Solid Fuels Under Rapid Heating Conditions. J. Propuls. Power 1999, 15, 888–895. [Google Scholar] [CrossRef]
- Venkateswaran, S.; Merkle, C. Size Scale-up in Hybrid Rocket Motors. In Proceedings of the 34th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 15–18 January 1996. [Google Scholar]
- Magnussen, B.F.; Hjertager, B.H. On mathematical modeling of turbulent combustion with special emphasis on soot formation and combustion. Symp. (Int.) Combust. 1977, 16, 719–729. [Google Scholar] [CrossRef]
- Cohen, N.S.; Fleming, R.W.; Derr, R.L. Role of Binders in Solid Propellant Combustion. AIAA J. 1974, 12, 212–218. [Google Scholar] [CrossRef] [Green Version]
- Sun, X.; Tian, H.; Cai, G. Diameter and position effect determination of diaphragm on hybrid rocket motor. Acta Astronaut. 2016, 126, 325–333. [Google Scholar] [CrossRef]
- Tian, H.; Jiang, X.; Yu, R.; Zhu, H.; Zhang, Y.; Cai, G. Numerical analysis of the hybrid rocket motor with axial injection based on oxidizer flow distribution. Acta Astronaut. 2022, 192, 245–257. [Google Scholar] [CrossRef]
- Cai, G.; Cao, B.; Zhu, H.; Tian, H.; Ma, X. Parametric investigation of secondary injection in post-chamber on combustion performance for hybrid rocket motor. Acta Astronaut. 2017, 140, 427–438. [Google Scholar] [CrossRef]
- Tian, H.; Yu, R.; Li, C.; Zhao, S.; Zhu, H. Numerical and experimental study of the thermochemical erosion of a graphite nozzle in a hybrid rocket motor with a star grain. Acta Astronaut. 2018, 155, 10–22. [Google Scholar] [CrossRef]
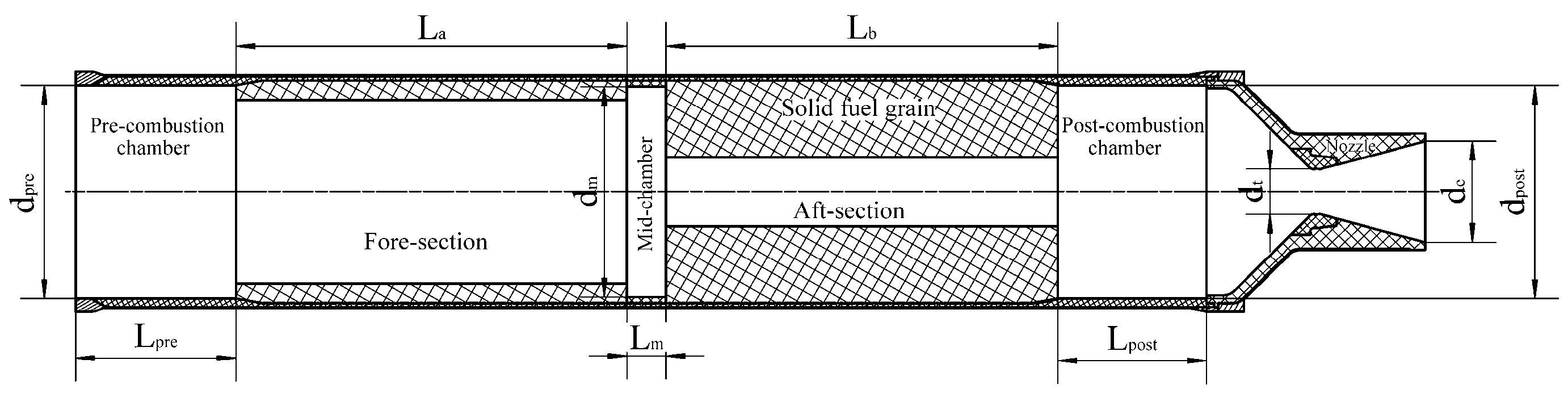
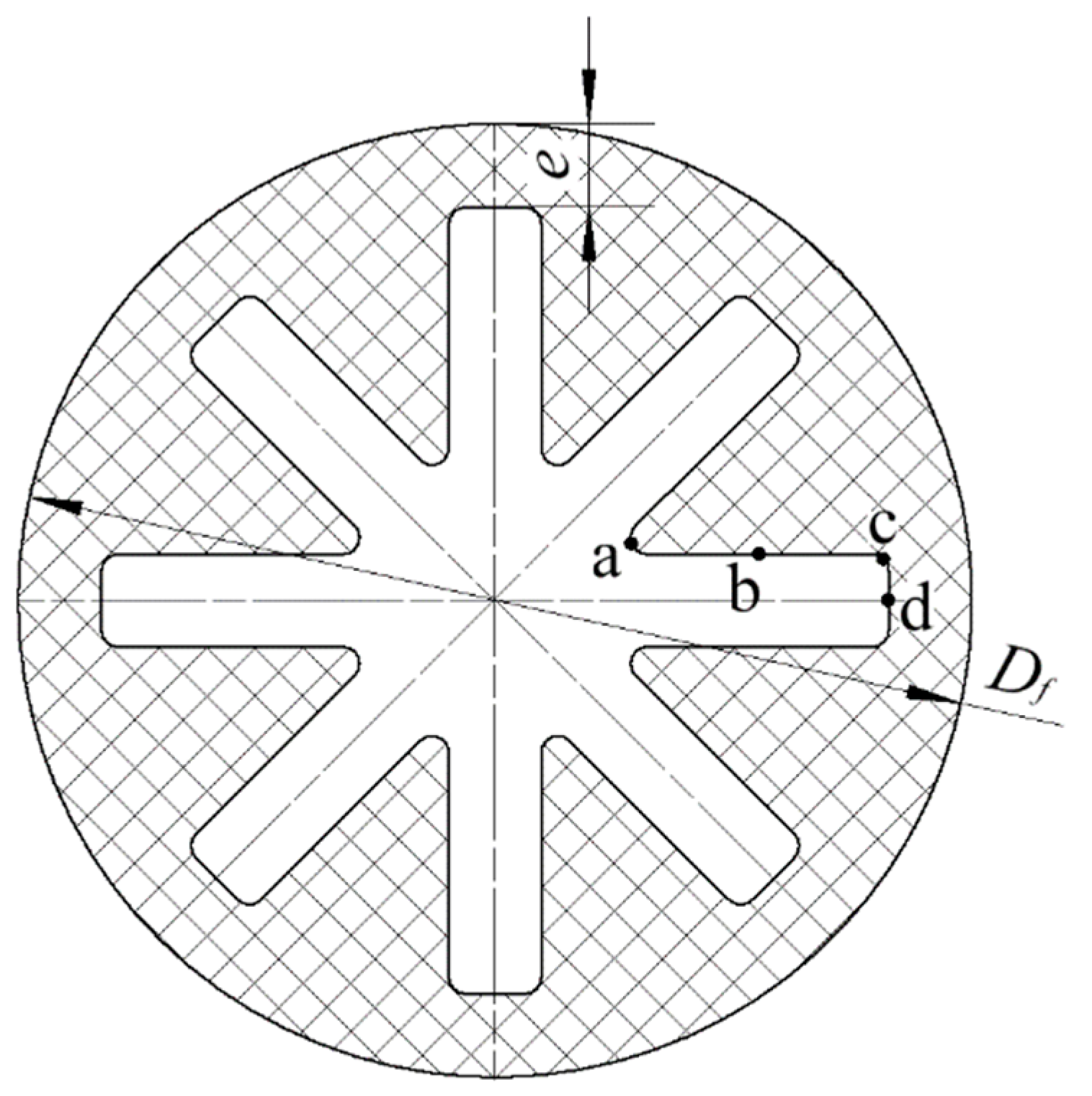
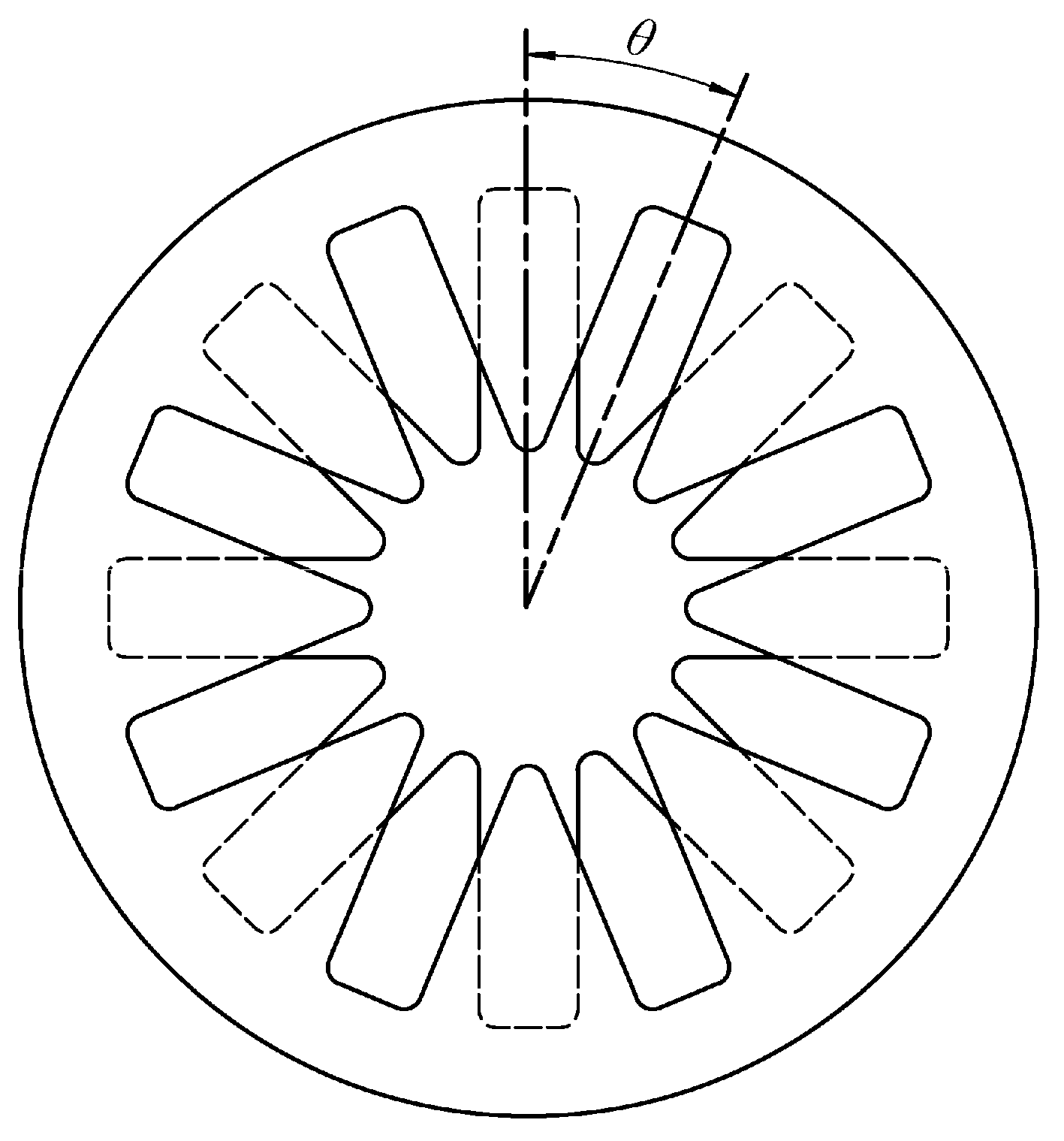
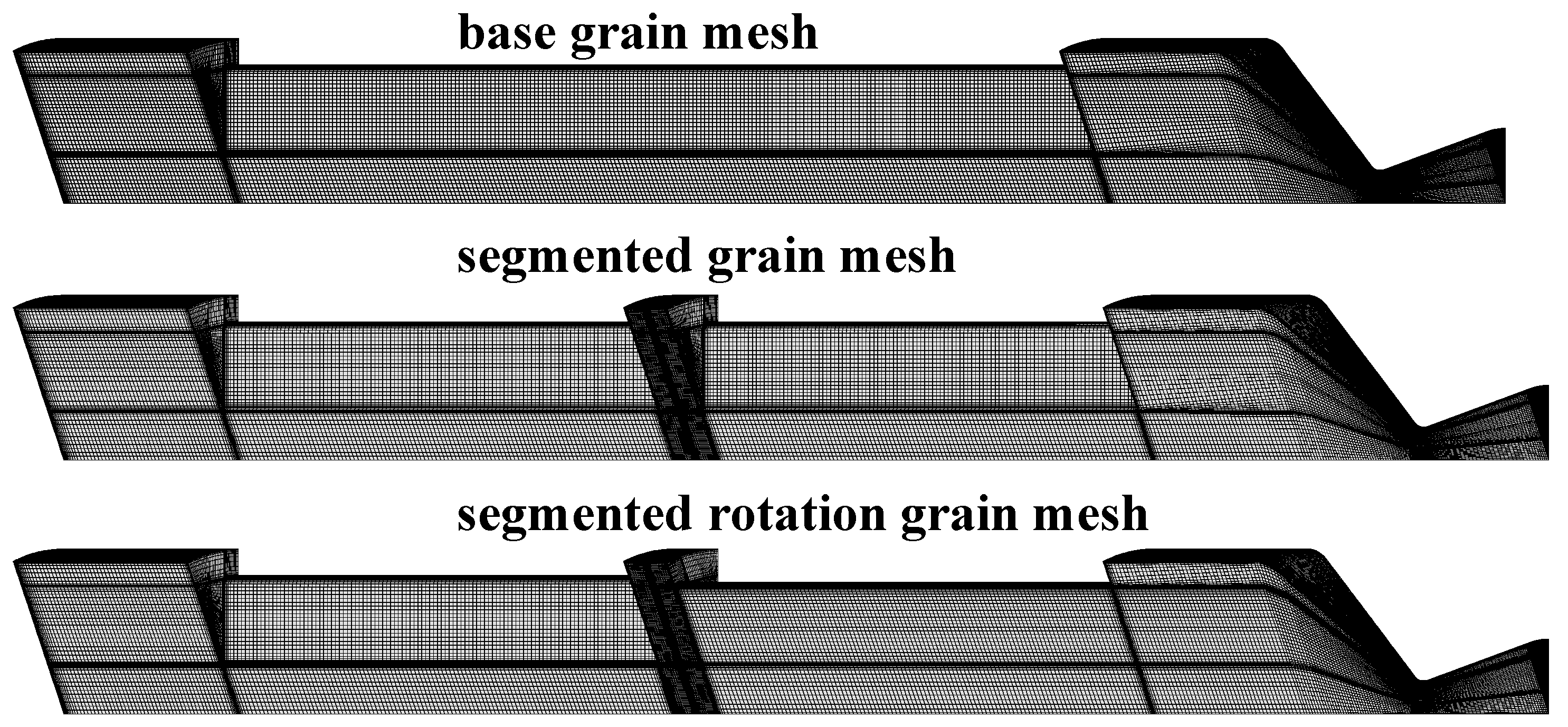
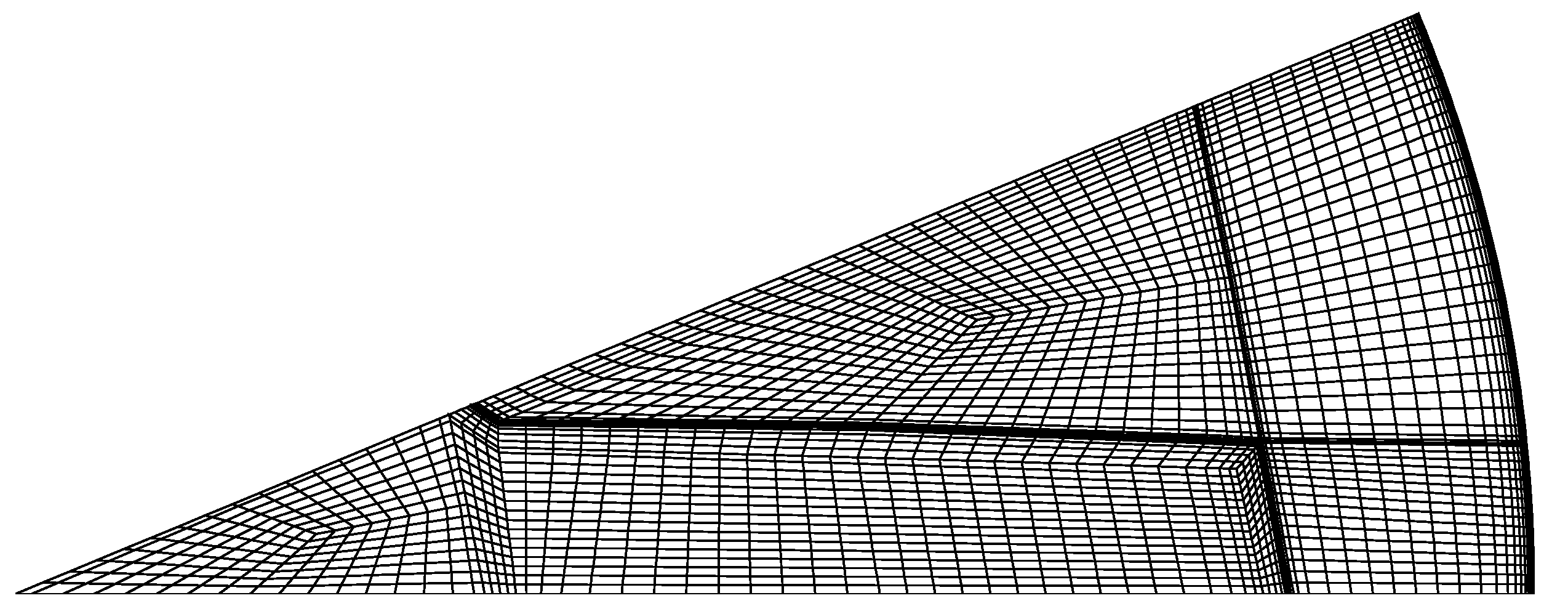
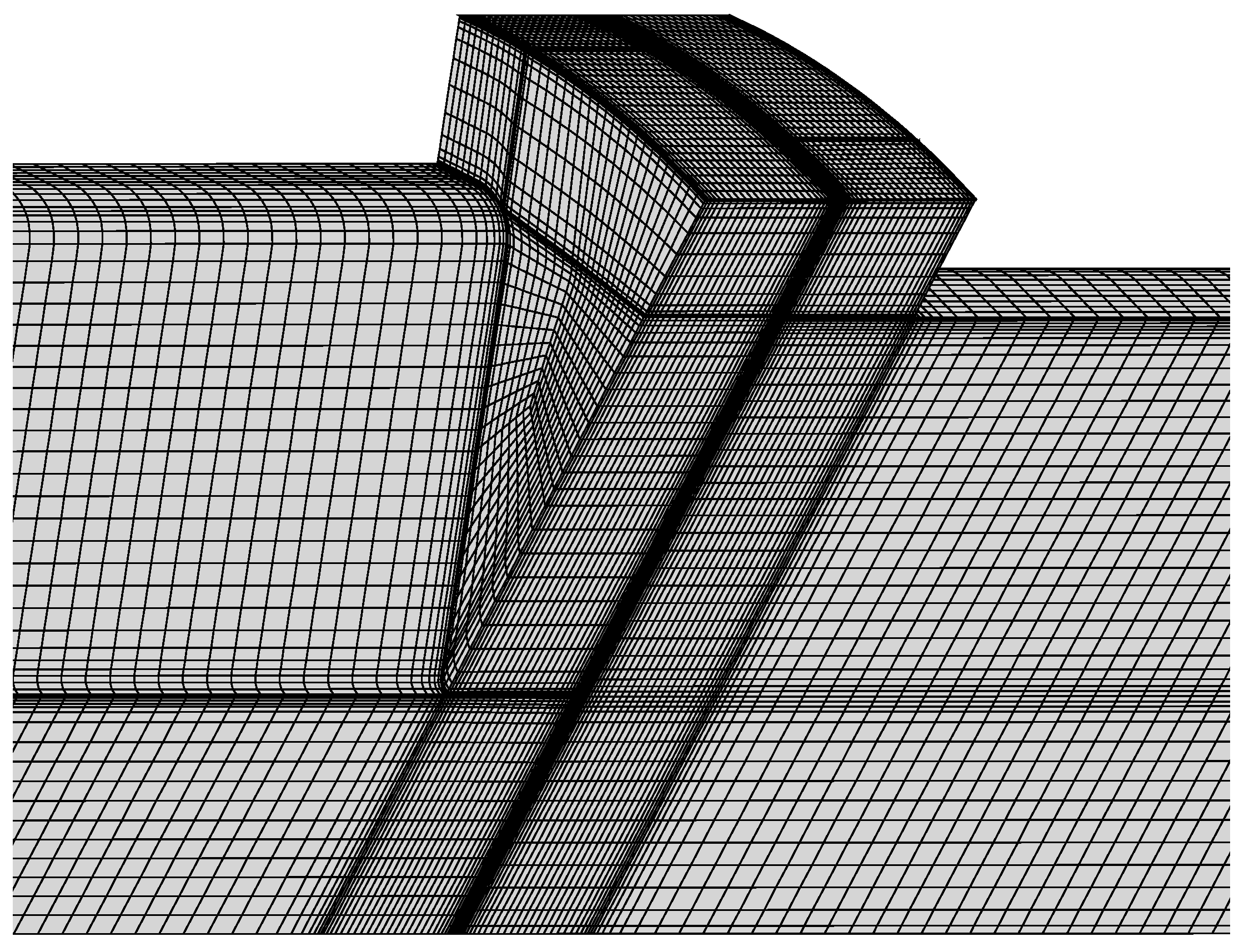
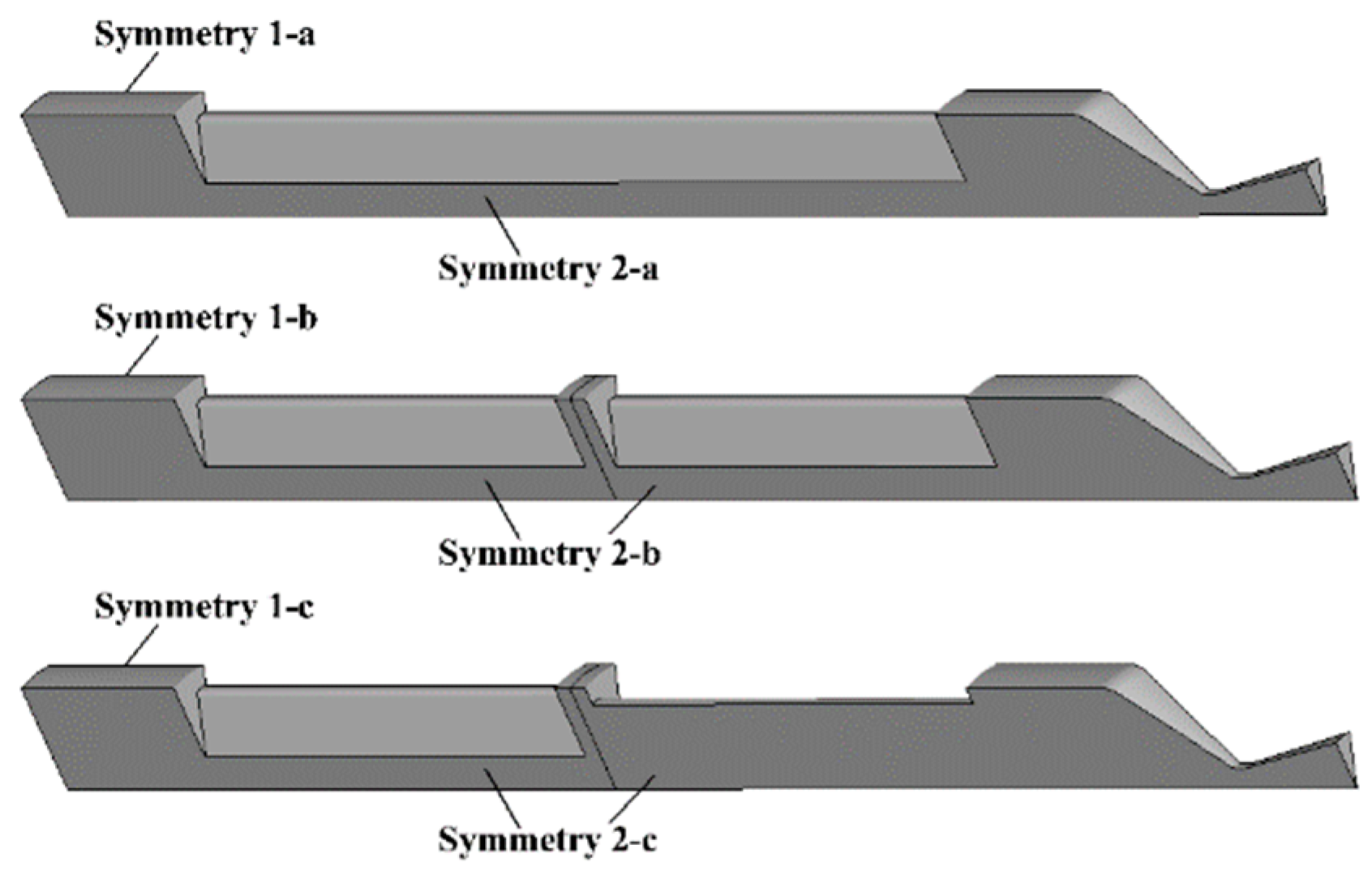










| Name | Values |
|---|---|
| Pre-combustion chamber inner diameter dpre (mm) | 286 |
| Pre-combustion chamber length Lpre (mm) | 200 |
| Fuel grain star number | 8 |
| Fuel grain outer diameter Df (mm) | 285 |
| Fuel grain thickness e (mm) | 25 |
| Rotation angle of two segmented grains θ (°) | 22.5 |
| Mid-chamber inner diameter dm (mm) | 286 |
| Total grain length La+Lb (mm) | 1000 |
| Post-combustion chamber inner diameter dpost (mm) | 286 |
| Post-combustion chamber length Lpost (mm) | 200 |
| Nozzle throat diameter dt (mm) | 58 |
| Nozzle exit diameter de (mm) | 129.69 |
| Nozzle convergence half-angle (degree) | 45 |
| Nozzle divergence half-angle (degree) | 15 |
| Case | Lm (mm) | θ (°) | La (mm) | Lb (mm) | Case | Lm (mm) | θ(°) | La (mm) | Lb (mm) |
|---|---|---|---|---|---|---|---|---|---|
| no-seg | 0 | - | 1000 | 0 | |||||
| seg-1/2-10 | 10 | 0 | 500 | 500 | rot-1/2-10 | 10 | 22.5 | 500 | 500 |
| seg-1/2-30 | 30 | 0 | 500 | 500 | rot-1/2-30 | 30 | 22.5 | 500 | 500 |
| seg-1/2-40 | 40 | 0 | 500 | 500 | rot-1/2-40 | 40 | 22.5 | 500 | 500 |
| seg-1/2-50 | 50 | 0 | 500 | 500 | rot-1/2-50 | 50 | 22.5 | 500 | 500 |
| seg-1/2-60 | 60 | 0 | 500 | 500 | rot-1/2-60 | 60 | 22.5 | 500 | 500 |
| seg-1/2-70 | 70 | 0 | 500 | 500 | rot-1/2-70 | 70 | 22.5 | 500 | 500 |
| seg-1/2-90 | 90 | 0 | 500 | 500 | rot-1/2-90 | 90 | 22.5 | 500 | 500 |
| seg-1/2-110 | 110 | 0 | 500 | 500 | rot-1/2-110 | 110 | 22.5 | 500 | 500 |
| rot-1/6-10 | 10 | 22.5 | 166.67 | 833.33 | rot-2/3-10 | 10 | 22.5 | 666.67 | 333.33 |
| rot-1/6-30 | 30 | 22.5 | 166.67 | 833.33 | rot-2/3-30 | 30 | 22.5 | 666.67 | 333.33 |
| rot-1/6-40 | 40 | 22.5 | 166.67 | 833.33 | rot-2/3-40 | 40 | 22.5 | 666.67 | 333.33 |
| rot-1/6-50 | 50 | 22.5 | 166.67 | 833.33 | rot-2/3-50 | 50 | 22.5 | 666.67 | 333.33 |
| rot-1/6-60 | 60 | 22.5 | 166.67 | 833.33 | rot-2/3-60 | 60 | 22.5 | 666.67 | 333.33 |
| rot-1/6-70 | 70 | 22.5 | 166.67 | 833.33 | rot-2/3-70 | 70 | 22.5 | 666.67 | 333.33 |
| rot-1/6-90 | 90 | 22.5 | 166.67 | 833.33 | rot-2/3-90 | 90 | 22.5 | 666.67 | 333.33 |
| rot-1/6-110 | 110 | 22.5 | 166.67 | 833.33 | rot-2/3-110 | 110 | 22.5 | 666.67 | 333.33 |
| rot-1/3-10 | 10 | 22.5 | 333.33 | 666.67 | rot-5/6-10 | 10 | 22.5 | 833.33 | 166.67 |
| rot-1/3-30 | 30 | 22.5 | 333.33 | 666.67 | rot-5/6-30 | 30 | 22.5 | 833.33 | 166.67 |
| rot-1/3-40 | 40 | 22.5 | 333.33 | 666.67 | rot-5/6-40 | 40 | 22.5 | 833.33 | 166.67 |
| rot-1/3-50 | 50 | 22.5 | 333.33 | 666.67 | rot-5/6-50 | 50 | 22.5 | 833.33 | 166.67 |
| rot-1/3-60 | 60 | 22.5 | 333.33 | 666.67 | rot-5/6-60 | 60 | 22.5 | 833.33 | 166.67 |
| rot-1/3-70 | 70 | 22.5 | 333.33 | 666.67 | rot-5/6-70 | 70 | 22.5 | 833.33 | 166.67 |
| rot-1/3-90 | 90 | 22.5 | 333.33 | 666.67 | rot-5/6-90 | 90 | 22.5 | 833.33 | 166.67 |
| rot-1/3-110 | 110 | 22.5 | 333.33 | 666.67 | rot-5/6-110 | 110 | 22.5 | 833.33 | 166.67 |
| Parameters | Value (T < 722 K) | Value (T > 722 K) |
|---|---|---|
| Pre-exponential factor A (m/s) | 3.9640 | 0.01104 |
| Activation energy E (J/mol) | 55,803.0 | 20,523.8 |
| Main Parameters | Test Data | Simulation Results | Error |
|---|---|---|---|
| (kg/s) | 0.351 | 0.351 | - |
| (MPa) | 2.449 | 2.344 | 4.29% |
| (mm/s) | 0.753 | 0.764 | 1.46% |
| (m/s) | 1507.01 | 1464.23 | 2.84% |
| Case | (mm/s) | (mm/s) | (mm/s) | Case | (mm/s) | (mm/s) | (mm/s) |
|---|---|---|---|---|---|---|---|
| no-seg | - | - | 0.4593 | ||||
| seg-1/2-10 | 0.4474 | 0.4739 | 0.4607 | rot-1/2-10 | 0.4479 | 0.5124 | 0.4801 |
| seg-1/2-30 | 0.4484 | 0.4759 | 0.4622 | rot-1/2-30 | 0.4488 | 0.5220 | 0.4854 |
| seg-1/2-40 | 0.4486 | 0.4770 | 0.4628 | rot-1/2-40 | 0.4486 | 0.5326 | 0.4906 |
| seg-1/2-50 | 0.4484 | 0.4778 | 0.4631 | rot-1/2-50 | 0.4486 | 0.5317 | 0.4901 |
| seg-1/2-60 | 0.4485 | 0.4792 | 0.4639 | rot-1/2-60 | 0.4481 | 0.5303 | 0.4892 |
| seg-1/2-70 | 0.4484 | 0.4829 | 0.4656 | rot-1/2-70 | 0.4486 | 0.5296 | 0.4891 |
| seg-1/2-90 | 0.4481 | 0.4913 | 0.4697 | rot-1/2-90 | 0.4485 | 0.5256 | 0.4870 |
| seg-1/2-110 | 0.4486 | 0.4945 | 0.4716 | rot-1/2-110 | 0.4486 | 0.5173 | 0.4830 |
| rot-1/6-10 | 0.4134 | 0.4888 | 0.4762 | rot-2/3-10 | 0.4527 | 0.5387 | 0.4814 |
| rot-1/6-30 | 0.4177 | 0.5065 | 0.4917 | rot-2/3-30 | 0.4537 | 0.5410 | 0.4828 |
| rot-1/6-40 | 0.4181 | 0.5099 | 0.4946 | rot-2/3-40 | 0.4539 | 0.5436 | 0.4838 |
| rot-1/6-50 | 0.4182 | 0.5120 | 0.4963 | rot-2/3-50 | 0.4538 | 0.5434 | 0.4837 |
| rot-1/6-60 | 0.4177 | 0.5087 | 0.4936 | rot-2/3-60 | 0.4539 | 0.5381 | 0.4819 |
| rot-1/6-70 | 0.4173 | 0.5060 | 0.4912 | rot-2/3-70 | 0.4537 | 0.5369 | 0.4814 |
| rot-1/6-90 | 0.4178 | 0.5004 | 0.4867 | rot-2/3-90 | 0.4538 | 0.5322 | 0.4800 |
| rot-1/6-110 | 0.4178 | 0.4957 | 0.4827 | rot-2/3-110 | 0.4538 | 0.5271 | 0.4782 |
| rot-1/3-10 | 0.4373 | 0.4960 | 0.4764 | rot-5/6-10 | 0.4561 | 0.5743 | 0.4758 |
| rot-1/3-30 | 0.4381 | 0.5133 | 0.4883 | rot-5/6-30 | 0.4572 | 0.5714 | 0.4762 |
| rot-1/3-40 | 0.4382 | 0.5181 | 0.4915 | rot-5/6-40 | 0.4572 | 0.5647 | 0.4751 |
| rot-1/3-50 | 0.4376 | 0.5198 | 0.4924 | rot-5/6-50 | 0.4571 | 0.5742 | 0.4766 |
| rot-1/3-60 | 0.4382 | 0.5177 | 0.4912 | rot-5/6-60 | 0.4571 | 0.5685 | 0.4757 |
| rot-1/3-70 | 0.4382 | 0.5170 | 0.4907 | rot-5/6-70 | 0.4569 | 0.5677 | 0.4754 |
| rot-1/3-90 | 0.4378 | 0.5117 | 0.4871 | rot-5/6-90 | 0.4570 | 0.5646 | 0.4749 |
| rot-1/3-110 | 0.4382 | 0.5036 | 0.4818 | rot-5/6-110 | 0.4569 | 0.5613 | 0.4743 |
| Case | O/F | |||||
|---|---|---|---|---|---|---|
| no-seg | 1.2561 | 3.201 | 2.6208 | 1266.26 | 1454.09 | 87.08 |
| seg-1/2-10 | 1.2562 | 3.192 | 2.6226 | 1265.54 | 1453.16 | 87.09 |
| seg-1/2-30 | 1.2567 | 3.182 | 2.6246 | 1265.03 | 1451.99 | 87.12 |
| seg-1/2-40 | 1.2571 | 3.177 | 2.6256 | 1265.02 | 1451.42 | 87.16 |
| seg-1/2-50 | 1.2582 | 3.177 | 2.6256 | 1266.08 | 1451.42 | 87.23 |
| seg-1/2-60 | 1.2589 | 3.169 | 2.6271 | 1266.11 | 1450.50 | 87.29 |
| seg-1/2-70 | 1.2600 | 3.158 | 2.6295 | 1266.05 | 1449.11 | 87.37 |
| seg-1/2-90 | 1.2643 | 3.129 | 2.6351 | 1267.64 | 1445.70 | 87.68 |
| seg-1/2-110 | 1.2650 | 3.118 | 2.6375 | 1267.22 | 1444.31 | 87.74 |
| rot-1/6-10 | 1.2716 | 3.088 | 2.6438 | 1270.76 | 1440.50 | 88.22 |
| rot-1/6-30 | 1.3025 | 2.991 | 2.6648 | 1291.41 | 1427.74 | 90.45 |
| rot-1/6-40 | 1.3078 | 2.975 | 2.6684 | 1294.95 | 1425.54 | 90.84 |
| rot-1/6-50 | 1.3098 | 2.965 | 2.6705 | 1295.87 | 1424.25 | 90.99 |
| rot-1/6-60 | 1.3019 | 2.978 | 2.6675 | 1289.51 | 1426.08 | 90.42 |
| rot-1/6-70 | 1.2961 | 2.995 | 2.6638 | 1285.54 | 1428.32 | 90.00 |
| rot-1/6-90 | 1.2863 | 3.021 | 2.6580 | 1278.59 | 1431.91 | 89.29 |
| rot-1/6-110 | 1.2771 | 3.048 | 2.6521 | 1272.29 | 1435.45 | 88.63 |
| rot-1/3-10 | 1.2673 | 3.087 | 2.6439 | 1266.44 | 1440.41 | 87.92 |
| rot-1/3-30 | 1.3016 | 3.013 | 2.6598 | 1292.96 | 1430.80 | 90.37 |
| rot-1/3-40 | 1.3082 | 2.992 | 2.6645 | 1297.14 | 1427.89 | 90.84 |
| rot-1/3-50 | 1.3064 | 2.988 | 2.6654 | 1294.97 | 1427.36 | 90.73 |
| rot-1/3-60 | 1.3044 | 2.993 | 2.6642 | 1293.60 | 1428.12 | 90.58 |
| rot-1/3-70 | 1.3035 | 2.997 | 2.6633 | 1293.15 | 1428.63 | 90.52 |
| rot-1/3-90 | 1.2992 | 3.021 | 2.6581 | 1291.36 | 1431.80 | 90.19 |
| rot-1/3-110 | 1.2883 | 3.051 | 2.6514 | 1283.74 | 1435.88 | 89.40 |
| rot-1/2-10 | 1.2786 | 3.066 | 2.6483 | 1275.62 | 1437.76 | 88.72 |
| rot-1/2-30 | 1.2984 | 3.031 | 2.6559 | 1291.64 | 1433.14 | 90.13 |
| rot-1/2-40 | 1.3125 | 2.996 | 2.6635 | 1302.00 | 1428.55 | 91.14 |
| rot-1/2-50 | 1.3072 | 3.000 | 2.6627 | 1297.10 | 1429.02 | 90.77 |
| rot-1/2-60 | 1.3067 | 3.004 | 2.6617 | 1297.11 | 1429.63 | 90.73 |
| rot-1/2-70 | 1.3053 | 3.012 | 2.6600 | 1296.45 | 1430.64 | 90.62 |
| rot-1/2-90 | 1.3016 | 3.021 | 2.6579 | 1293.81 | 1431.92 | 90.35 |
| rot-1/2-110 | 1.2914 | 3.044 | 2.6530 | 1286.14 | 1434.94 | 89.63 |
| rot-2/3-10 | 1.2846 | 3.057 | 2.6503 | 1280.66 | 1436.56 | 89.15 |
| rot-2/3-30 | 1.3101 | 3.047 | 2.6523 | 1305.01 | 1435.33 | 90.92 |
| rot-2/3-40 | 1.3100 | 3.039 | 2.6541 | 1304.07 | 1434.27 | 90.92 |
| rot-2/3-50 | 1.3086 | 3.041 | 2.6536 | 1302.92 | 1434.55 | 90.82 |
| rot-2/3-60 | 1.2984 | 3.053 | 2.6512 | 1293.91 | 1436.01 | 90.10 |
| rot-2/3-70 | 1.2997 | 3.057 | 2.6503 | 1295.70 | 1436.56 | 90.19 |
| rot-2/3-90 | 1.2928 | 3.065 | 2.6486 | 1289.64 | 1437.61 | 89.71 |
| rot-2/3-110 | 1.2852 | 3.076 | 2.6461 | 1283.27 | 1439.09 | 89.17 |
| rot-5/6-10 | 1.2826 | 3.093 | 2.6427 | 1282.26 | 1441.16 | 88.97 |
| rot-5/6-30 | 1.3088 | 3.088 | 2.6438 | 1307.92 | 1440.52 | 90.80 |
| rot-5/6-40 | 1.3023 | 3.095 | 2.6423 | 1302.18 | 1441.43 | 90.34 |
| rot-5/6-50 | 1.3052 | 3.086 | 2.6441 | 1304.18 | 1440.34 | 90.55 |
| rot-5/6-60 | 1.2980 | 3.091 | 2.6431 | 1297.51 | 1440.92 | 90.05 |
| rot-5/6-70 | 1.2964 | 3.095 | 2.6422 | 1296.31 | 1441.47 | 89.93 |
| rot-5/6-90 | 1.2924 | 3.098 | 2.6416 | 1292.67 | 1441.81 | 89.66 |
| rot-5/6-110 | 1.2856 | 3.103 | 2.6405 | 1286.38 | 1442.48 | 89.18 |
| Case | (kN) | |||
|---|---|---|---|---|
| no-seg-0s | 2.0000 | 0.6238 | 1.2561 | 3.9169 |
| no-seg-10s | 2.0000 | 0.5445 | 1.1993 | 3.6653 |
| no-seg-20s | 2.0000 | 0.4287 | 1.1299 | 3.3617 |
| no-seg-30s | 2.0000 | 0.3556 | 1.0639 | 3.0649 |
| rot-1/2-40-0s | 2.0000 | 0.6665 | 1.3125 | 4.1397 |
| rot-1/2-40-10s | 2.0000 | 0.5847 | 1.2607 | 3.9176 |
| rot-1/2-40-20s | 2.0000 | 0.4589 | 1.1923 | 3.6184 |
| rot-1/2-40-30s | 2.0000 | 0.3671 | 1.0867 | 3.1609 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, H.; Jiang, X.; Lu, Y.; Liang, Y.; Zhu, H.; Cai, G. Numerical Investigation on Hybrid Rocket Motors with Star-Segmented Rotation Grain. Aerospace 2022, 9, 585. https://doi.org/10.3390/aerospace9100585
Tian H, Jiang X, Lu Y, Liang Y, Zhu H, Cai G. Numerical Investigation on Hybrid Rocket Motors with Star-Segmented Rotation Grain. Aerospace. 2022; 9(10):585. https://doi.org/10.3390/aerospace9100585
Chicago/Turabian StyleTian, Hui, Xianzhu Jiang, Yudong Lu, Yu Liang, Hao Zhu, and Guobiao Cai. 2022. "Numerical Investigation on Hybrid Rocket Motors with Star-Segmented Rotation Grain" Aerospace 9, no. 10: 585. https://doi.org/10.3390/aerospace9100585
APA StyleTian, H., Jiang, X., Lu, Y., Liang, Y., Zhu, H., & Cai, G. (2022). Numerical Investigation on Hybrid Rocket Motors with Star-Segmented Rotation Grain. Aerospace, 9(10), 585. https://doi.org/10.3390/aerospace9100585






