Dynamic Response of Structurally Reinforced Wing Leading Edge against Soft Impact
Abstract
:1. Introduction
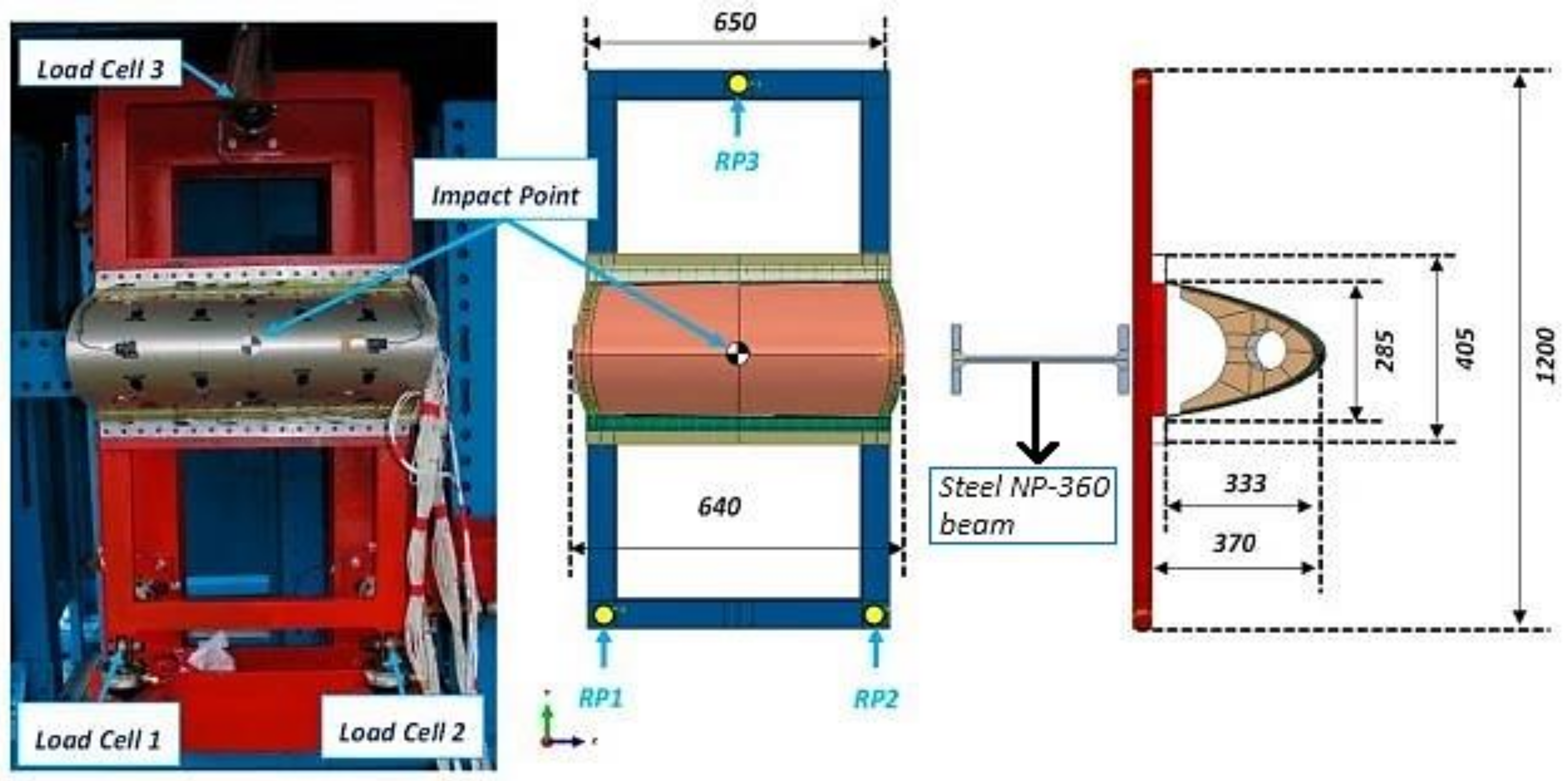
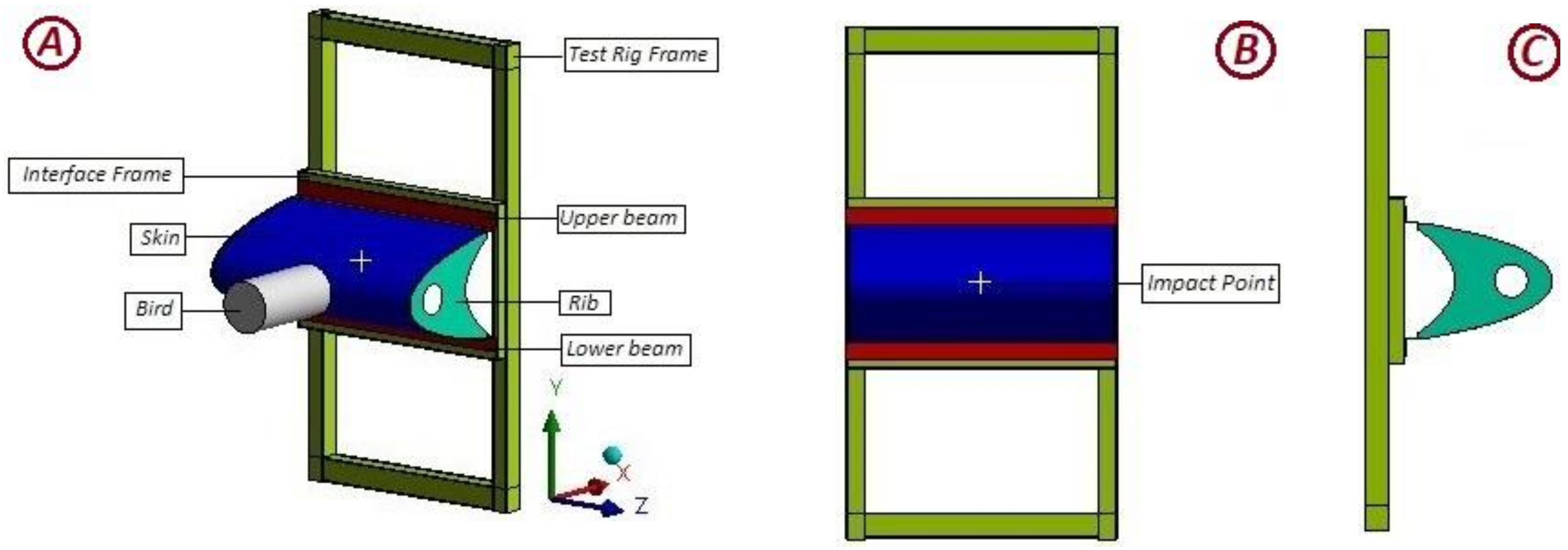
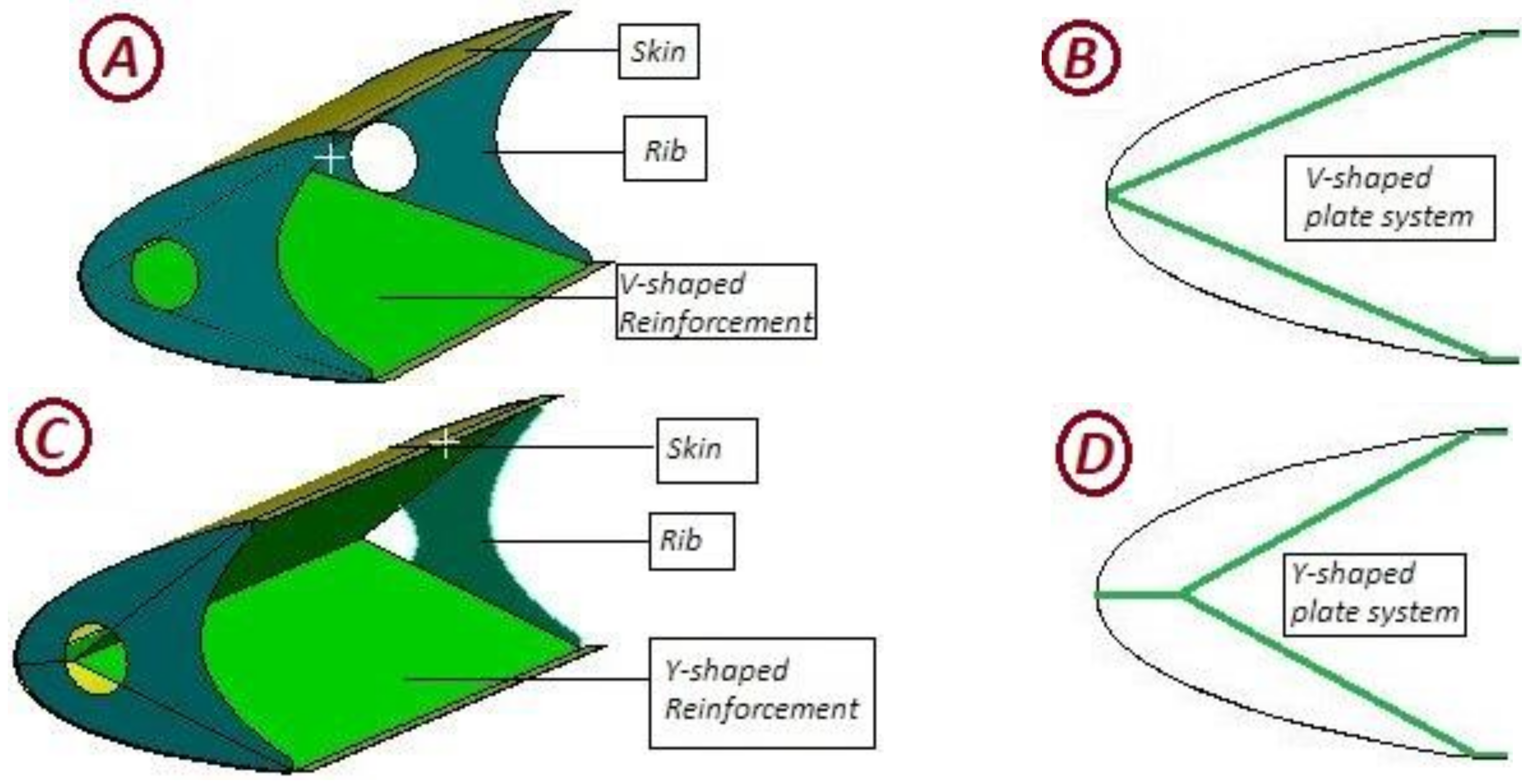
2. Test Article Design
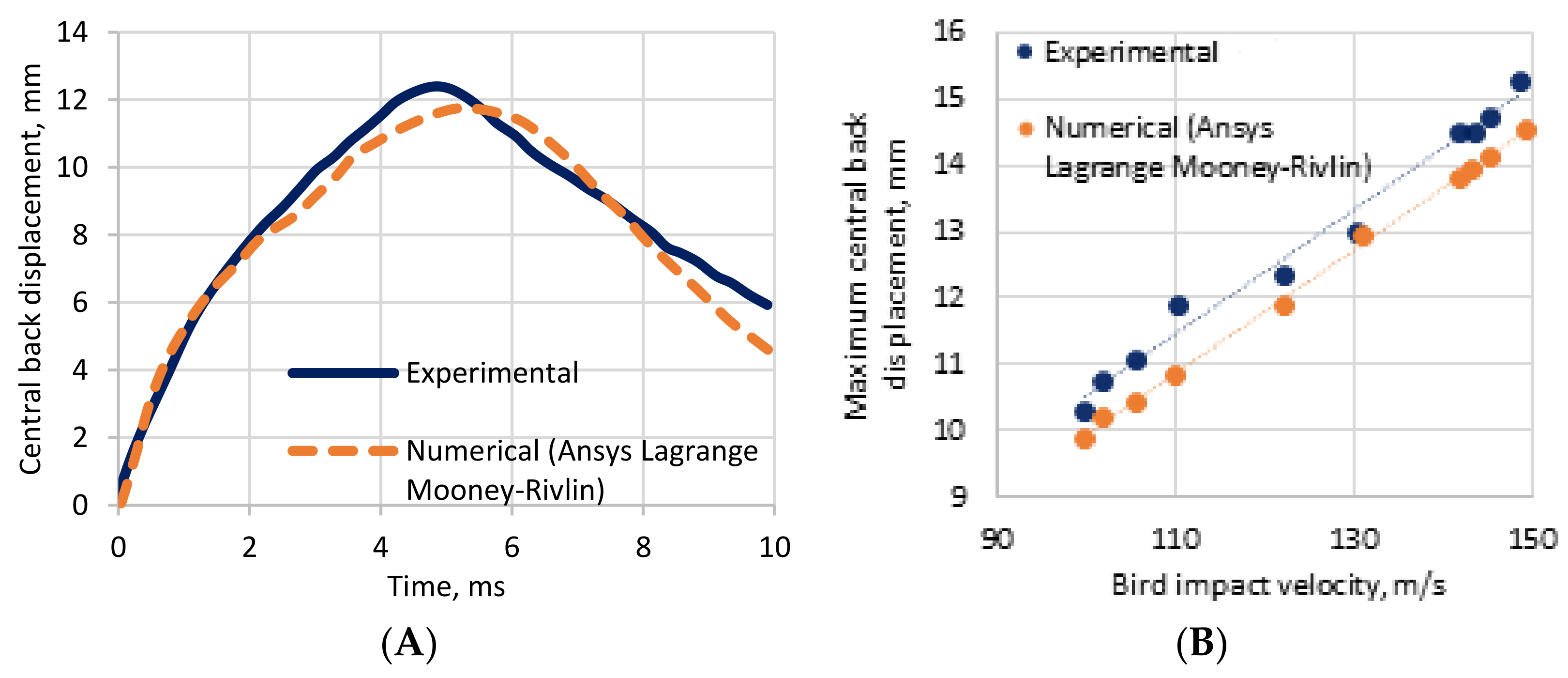
3. Bird Material Model
4. Numerical Outcomes
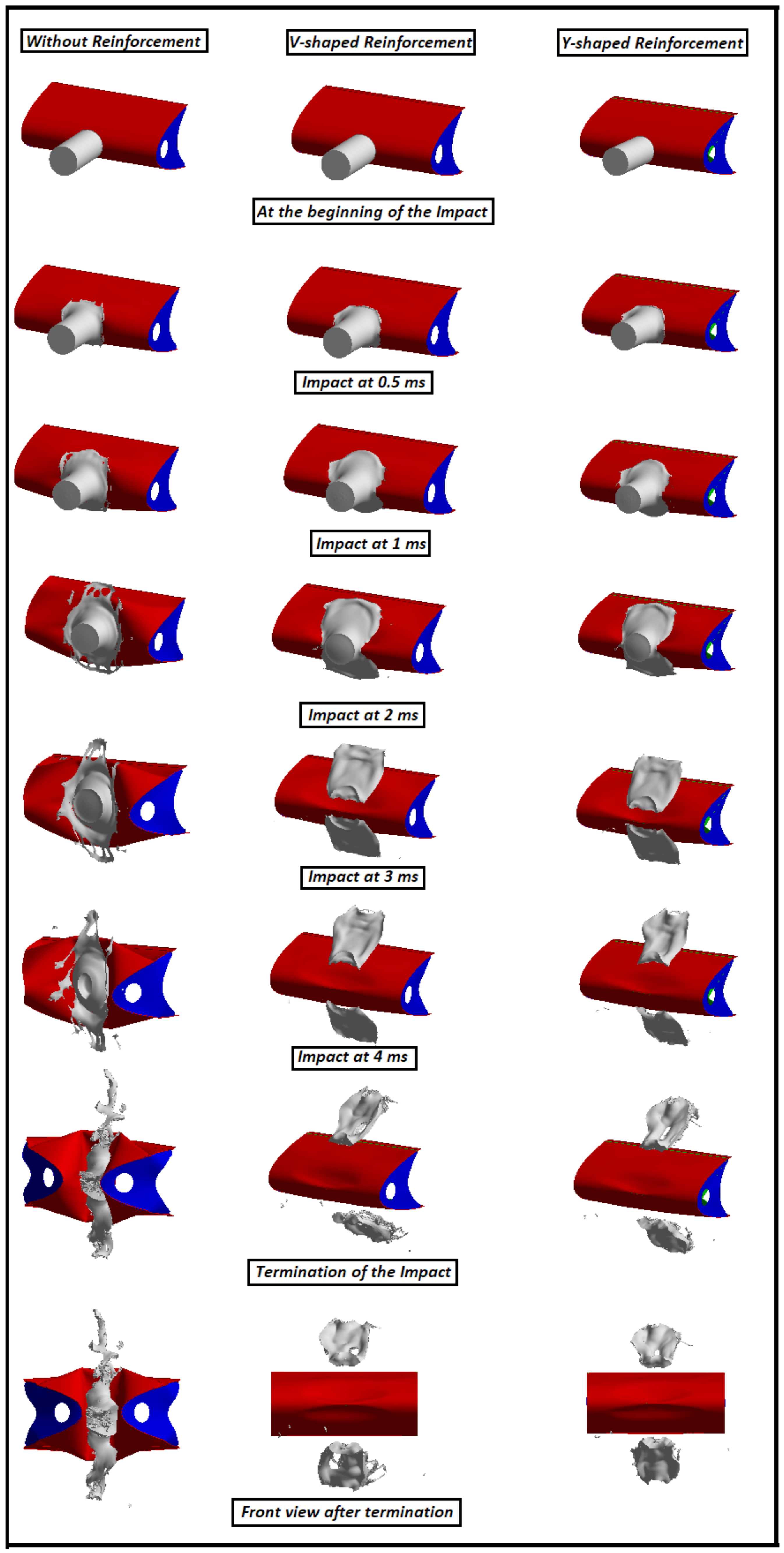
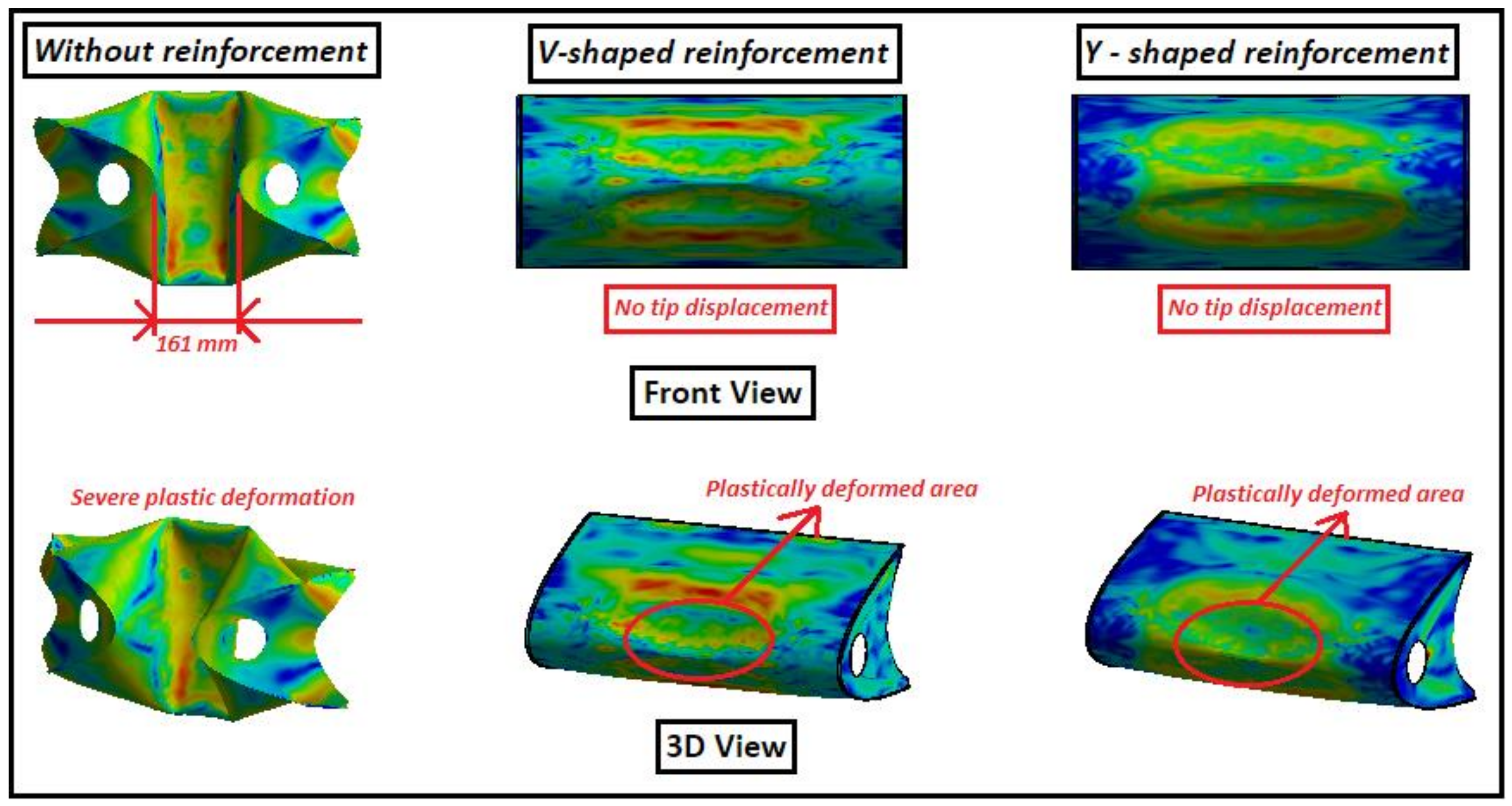
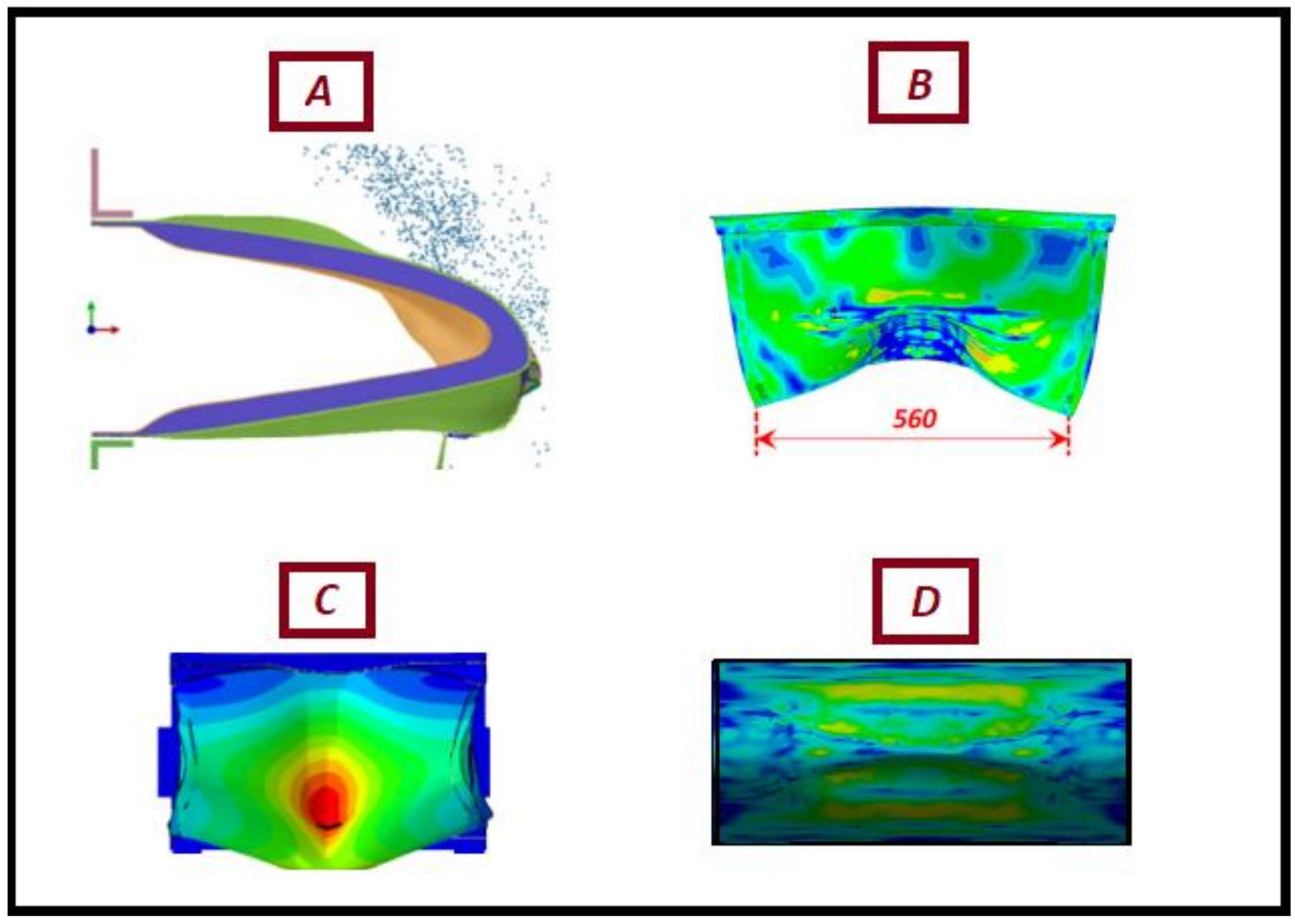
4.1. Bird Strike Impact Events
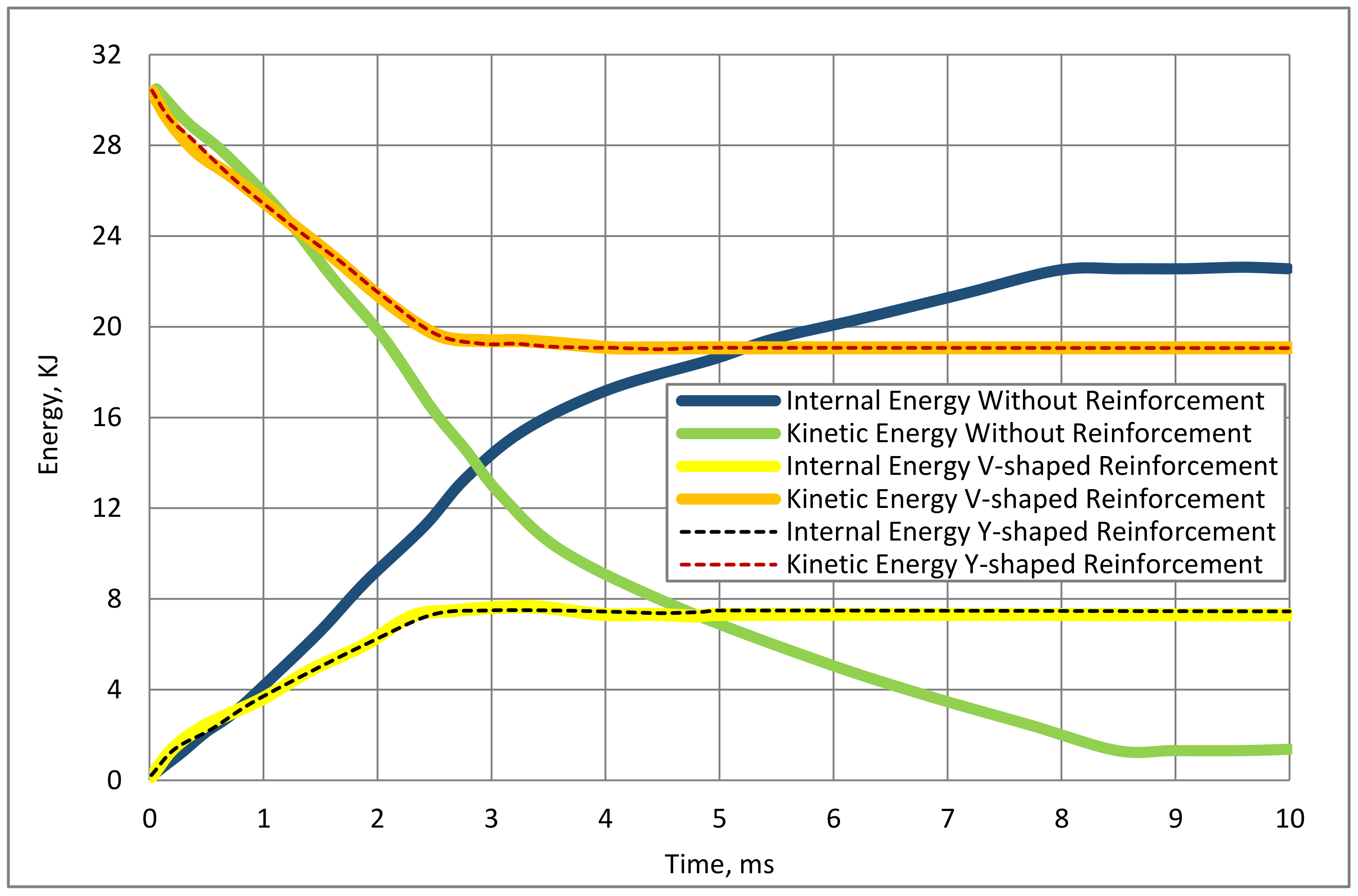
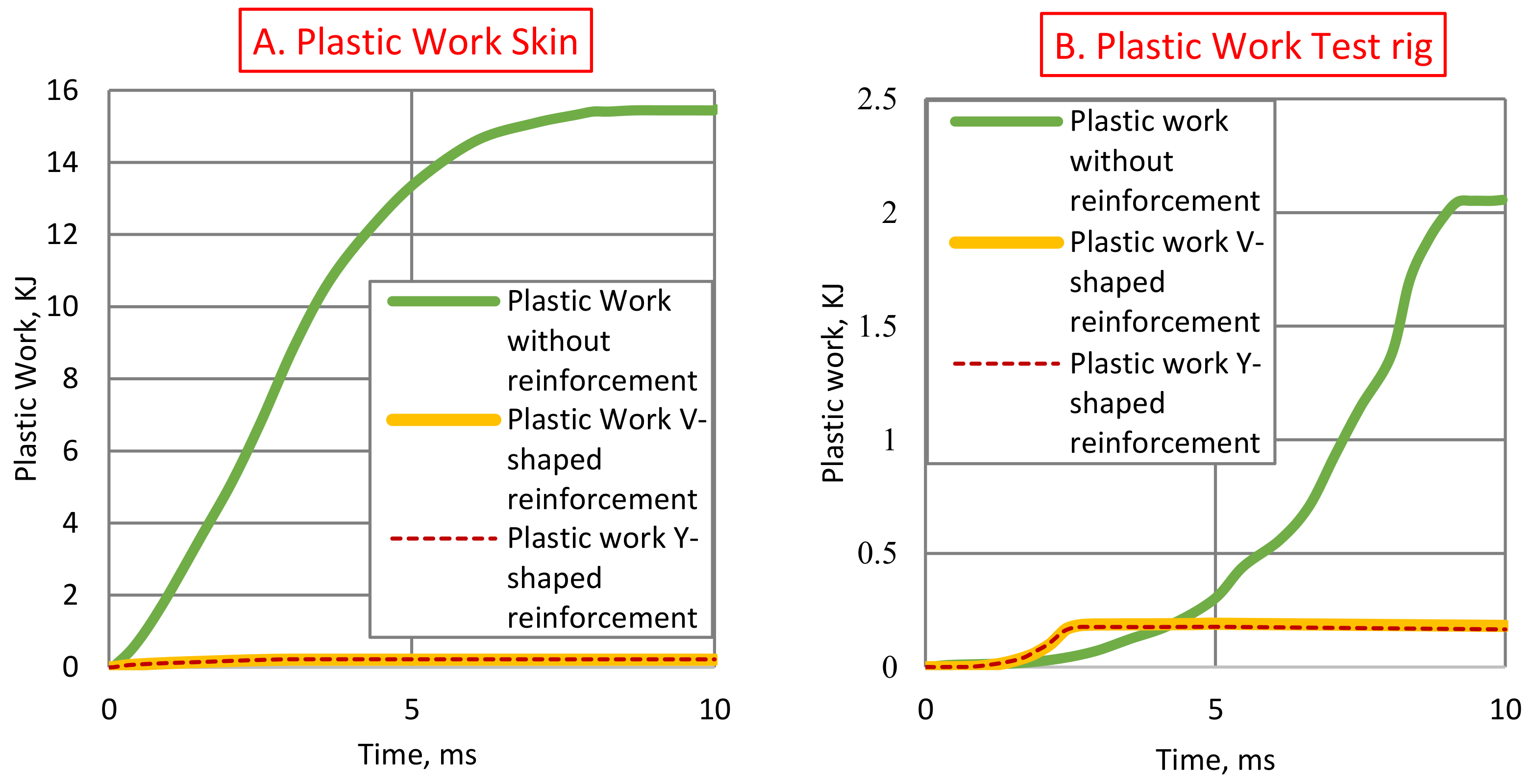
4.2. Energy Summary
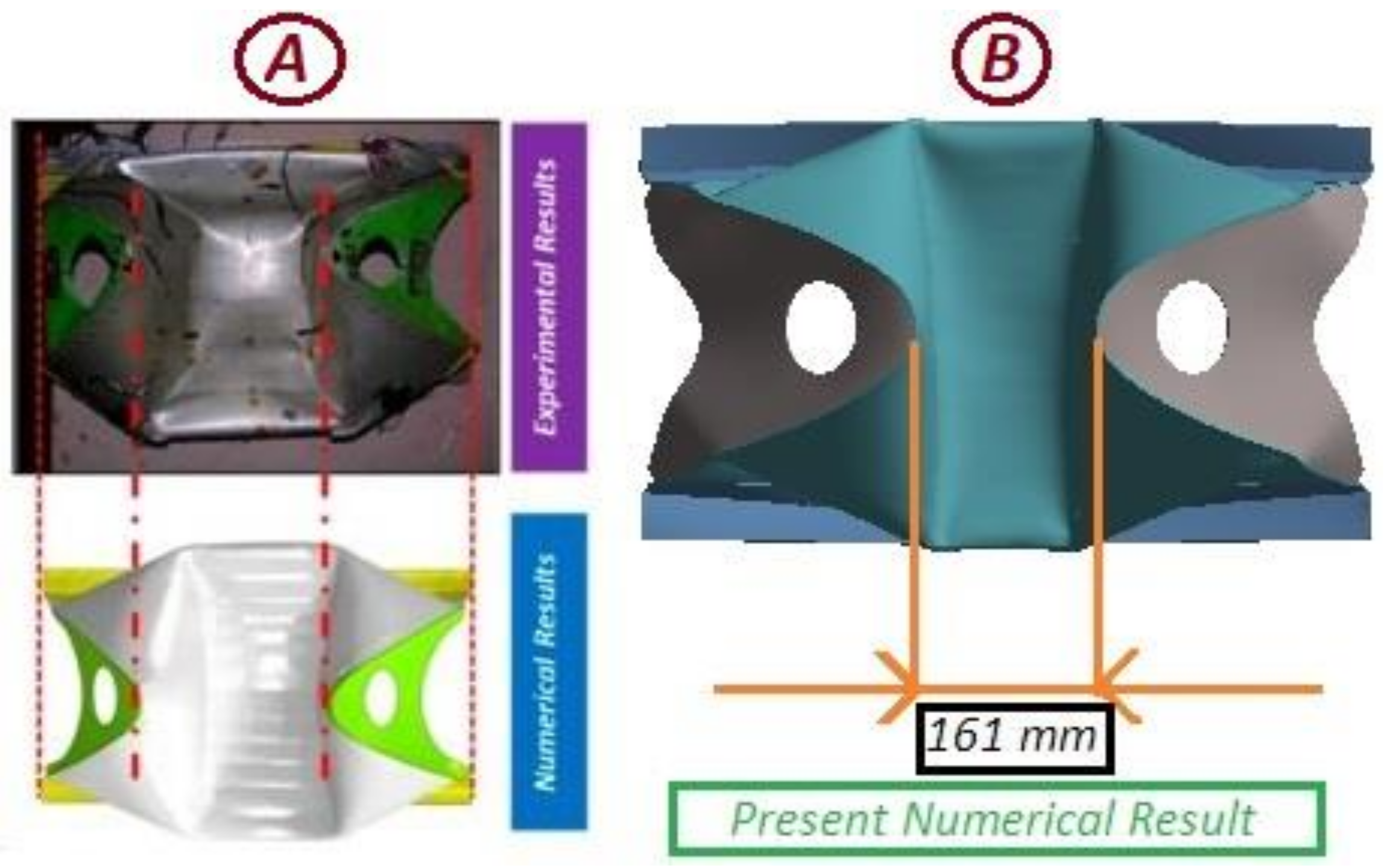
4.3. Deformed Configuration
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- FAA Foreign Object Debris Program. Available online: https://www.faa.gov/airports/airport_safety/fod/ (accessed on 3 March 2022).
- Mao, R.; Meguid, S.; Ng, T. Transient three dimensional finite element analysis of a bird striking a fan blade. Int. J. Mech. Mater. Design 2008, 4, 79–96. [Google Scholar] [CrossRef] [Green Version]
- Plassard, F.; Hereil, P.; Joseph, P.; Mespoulet, J. Experimental and numerical study of a bird strike against a windshield. EPJ Web Conf. 2015, 94, 01051. [Google Scholar] [CrossRef] [Green Version]
- Wilbeck, J.S.; Barber, J.P. Bird impact loading. Shock Vib. Bull. 1978, 48, 115–122. [Google Scholar]
- Impact Behavior of Low Strength Projectiles. Available online: https://apps.dtic.mil/sti/citations/ADA060423 (accessed on 3 March 2022).
- Guida, M.; Marulo, F.; Meo, M.; Riccio, M. Analysis of Bird Impact on a Composite Tailplane Leading Edge. Appl. Compos. Mater. 2008, 15, 241–257. [Google Scholar] [CrossRef] [Green Version]
- Guida, M.; Marulo, F.; Polito, T.; Meo, M.; Riccio, M. Design and Testing of a Fiber-Metal-Laminate Bird-Strike-Resistant Leading Edge. J. Aircr. 2009, 46, 2121–2129. [Google Scholar] [CrossRef] [Green Version]
- Guida, M.; Marulo, F.; Meo, M.; Russo, S. Experimental Tests Analysis of Fiber Metal Laminate under Birdstrike. Mech. Adv. Mater. Struct. 2012, 19, 376–395. [Google Scholar] [CrossRef] [Green Version]
- Georgiadis, S.; Gunnion, A.; Thomson, R.; Cartwright, B. Bird-strike simulation for certification of the Boeing 787 composite moveable trailing edge. Compos. Struct. 2008, 86, 258–268. [Google Scholar]
- Orlando, S.; Marulo, F.; Guida, M.; Timbrato, F. Bird strike assessment for a composite wing flap. Int. J. Crashworthiness 2017, 23, 219–235. [Google Scholar] [CrossRef]
- Liu, J.; Li, Y.; Gao, X. Bird strike on a flat plate: Experiments and numerical simulations. Int. J. Impact Eng. 2014, 70, 21–37. [Google Scholar] [CrossRef]
- Grimaldi, A.; Sollo, A.; Guida, M.; Marulo, F. Parametric study of a SPH high velocity impact analysis—A birdstrike windshield application. Compos. Struct. 2013, 96, 616–630. [Google Scholar] [CrossRef]
- Zhang, Z.; Li, L.; Zhang, D. Effect of arbitrary yaw/pitch angle in bird strike numerical simulation using SPH method. Aerosp. Sci. Technol. 2018, 81, 284–293. [Google Scholar] [CrossRef]
- Badshah, S.; Naeem, A.; Farhan Rafique, A.; Ul Haq, I.; Abdullah Malik, S. Numerical Study on the Critical Frequency Response of Jet Engine Rotors for Blade-Off Conditions against Bird Strike. Appl. Sci. 2019, 9, 5568. [Google Scholar] [CrossRef] [Green Version]
- Wu, B.; Lin, J.; Hedayati, R.; Zhang, G.; Zhang, J.; Zhang, L. Dynamic Responses of the Aero-Engine Rotor System to Bird Strike on Fan Blades at Different Rotational Speeds. Appl. Sci. 2021, 11, 8883. [Google Scholar] [CrossRef]
- Zhou, Y.; Sun, Y.; Huang, T. SPH-FEM Design of Laminated Plies under Bird-Strike Impact. Aerospace 2019, 6, 112. [Google Scholar] [CrossRef] [Green Version]
- Zhou, Y.; Sun, Y.; Huang, T. Bird-Strike Resistance of Composite Laminates with Different Materials. Materials 2019, 13, 129. [Google Scholar] [CrossRef] [Green Version]
- Kreculj, D.; Rasuo, B. Impact damage modeling in laminated composite aircraft structures. In Sustainable Composites for Aerospace Applications; Woodhead Publishing: Cambridge, UK, 2018; pp. 125–153. [Google Scholar]
- Kreculj, D.; Rasuo, B. Review of Impact Damages Modelling in Laminated Composite Aircraft Structures, 1st ed.; Tehnicki Vjesnik; University of Osijek: Osijek, Croatia, 2013. [Google Scholar]
- Hasan, M. Interface Failure of Heated GLARETM Fiber–Metal Laminates under Bird Strike. Aerospace 2020, 7, 28. [Google Scholar] [CrossRef] [Green Version]
- Reglero, J.; Rodríguez-Pérez, M.; Solórzano, E.; de Saja, J. Aluminium foams as a filler for leading edges: Improvements in the mechanical behaviour under bird strike impact tests. Mater. Design 2011, 32, 907–910. [Google Scholar] [CrossRef]
- Liu, J.; Li, Y.; Yu, X.; Tang, Z.; Gao, X.; Lv, J.; Zhang, Z. A novel design for reinforcing the aircraft tail leading edge structure against bird strike. Int. J. Impact Eng. 2017, 105, 89–101. [Google Scholar] [CrossRef]
- Qiu, J.; Wang, D.; Liu, C.; Chen, L.; Huang, H.; Sun, Q. Dynamic response of bird strike on honeycomb-based sandwich panels of composite leading edge. Int. J. Crashworthiness 2020, 26, 424–437. [Google Scholar] [CrossRef]
- Yu, Z.; Xue, P.; Yao, P.; Zahran, M. Novel Design of Wing Leading Edge against Birdstrike. J. Aerosp. Eng. 2020, 33, 04020009. [Google Scholar] [CrossRef]
- Di Caprio, F.; Sellitto, A.; Saputo, S.; Guida, M.; Riccio, A. A Sensitivity Analysis of the Damage Behavior of a Leading-Edge Subject to Bird Strike. Appl. Sci. 2020, 10, 8187. [Google Scholar] [CrossRef]
- Slimane, S.; Slimane, A.; Guelailia, A.; Boudjemai, A.; Kebdani, S.; Smahat, A.; Mouloud, D. Hypervelocity impact on honeycomb structure reinforced with bi-layer ceramic/aluminum facesheets used for spacecraft shielding. Mech. Adv. Mater. Struct. 2021, 1–19. [Google Scholar] [CrossRef]
- Meguid, S.; Mao, R.; Ng, T. FE analysis of geometry effects of an artificial bird striking an aeroengine fan blade. Int. J. Impact Eng. 2008, 35, 487–498. [Google Scholar] [CrossRef]
- Heimbs, S. Computational methods for bird strike simulations: A review. Comput. Struct. 2011, 89, 2093–2112. [Google Scholar] [CrossRef]
- Hedayati, R.; Sadighi, M. Bird Strike: An Experimental, Numerical and Theoretical Investigation, 1st ed.; Woodhead Publishing: Cambridge, UK; pp. 113–135.
- Hedayati, R.; Jahanbakhshi, M. Finite element analysis of an aluminum airplane stabilizer against birdstrike. J. Braz. Soc. Mech. Sci. Eng. 2015, 38, 317–326. [Google Scholar] [CrossRef]
- Zhou, J. Experimental and Numerical Investigation of Soft Impact Loading on Aircraft Materials. Ph.D. Thesis, Imperial College, London, UK, 2018. [Google Scholar]
- Hedayati, R.; Ziaei-Rad, S. Effect of Impact Orientation on Bird Strike Analysis. Int. J. Veh. Struct. Syst. 2011, 3, 184–191. [Google Scholar] [CrossRef]
- McCallum, S.; Shoji, H.; Akiyama, H. Development of an advanced multi-material bird-strike model using the smoothed particle hydrodynamics method. Int. J. Crashworthiness 2013, 18, 579–597. [Google Scholar] [CrossRef]
- Di Caprio, F.; Cristillo, D.; Saputo, S.; Guida, M.; Riccio, A. Crashworthiness of wing leading edges under bird impact event. Compos. Struct. 2019, 216, 39–52. [Google Scholar] [CrossRef]
- Azeem Aslam, M.; Ke, Z.; Rayhan, S.; Faizan, M.; Mamuda Bello, I. An investigation of soft impacts on selected aerospace grade alloys based on Johnson-Cook Material Model. J. Phys. Conf. Ser. 2020, 1707, 012008. [Google Scholar] [CrossRef]
- Ansys Explicit Library; Ansys: Canonsburg, PA, USA, 2021.
- Aslam, M.; Rayhan, S.; Ke, Z.; Yu, W. Ballistic gelatin Lagrange Mooney-Rivlin material model as a substitute of bird in finite element bird strike case studies. Lat. Am. J. Solids Struct. 2020, 17, 1–15. [Google Scholar] [CrossRef]
- Zhou, J.; Liu, J.; Zhang, X.; Yan, Y.; Jiang, L.; Mohagheghian, I.; Dear, J.; Charalambides, M. Experimental and numerical investigation of high velocity soft impact loading on aircraft materials. Aerosp. Sci. Technol. 2019, 90, 44–58. [Google Scholar] [CrossRef]


| Parameters | Aluminum-2024-T3 | Aluminum-7075-T6 |
|---|---|---|
| Density, ρ (kg m−3) | 2700 | 2700 |
| Poisson’s ratio, υ | 0.3 | 0.3 |
| Young’s modulus, E (GPa) | 70 | 70 |
| Yield stress, A (MPa) | 352 | 520 |
| Strain hardening modulus, B (MPa) | 440 | 477 |
| Strain hardening exponent, n | 0.42 | 0.52 |
| Reference strain rate, ε0 (s−1) | 3.3 × 10−4 | 5 × 10−4 |
| Strain rate coefficient, C | 0.0083 | 0.001 |
| Thermal softening exponent, m | 1.7 | 1 |
| Reference temperature, T0 (°K) | 293 | 293 |
| Melting temperature, Tmelt (°K) | 775 | 893 |
| Specific heat (J/kg-°K) | 900 | 910 |
| Density, ρ (kg m−3) | Material Constant, C0 (MPa) | Material Constant, C10 (MPa) |
|---|---|---|
| 968 | 0.218 | 0.0805 |
| Wing Model | Skin, KJ | Ribs, KJ | Reinforcement, KJ | Test Rig, KJ |
|---|---|---|---|---|
| No reinforcement | 17 | 0.33 | - | 3.1 |
| V-shaped reinforcement | 2.8 | 0.06 | 0.324 | 0.61 |
| Y-shaped reinforcement | 2.93 | 0.06 | 0.3 | 0.55 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aslam, M.A.; Rayhan, S.B.; Zhang, K. Dynamic Response of Structurally Reinforced Wing Leading Edge against Soft Impact. Aerospace 2022, 9, 260. https://doi.org/10.3390/aerospace9050260
Aslam MA, Rayhan SB, Zhang K. Dynamic Response of Structurally Reinforced Wing Leading Edge against Soft Impact. Aerospace. 2022; 9(5):260. https://doi.org/10.3390/aerospace9050260
Chicago/Turabian StyleAslam, Muhammad Azeem, Saiaf Bin Rayhan, and Ke Zhang. 2022. "Dynamic Response of Structurally Reinforced Wing Leading Edge against Soft Impact" Aerospace 9, no. 5: 260. https://doi.org/10.3390/aerospace9050260
APA StyleAslam, M. A., Rayhan, S. B., & Zhang, K. (2022). Dynamic Response of Structurally Reinforced Wing Leading Edge against Soft Impact. Aerospace, 9(5), 260. https://doi.org/10.3390/aerospace9050260






