Conceptual Design of a Hybrid Hydrogen Fuel Cell/Battery Blended-Wing-Body Unmanned Aerial Vehicle—An Overview
Abstract
:1. Introduction
2. Pre-Conceptual Phase
2.1. Educational Aspects
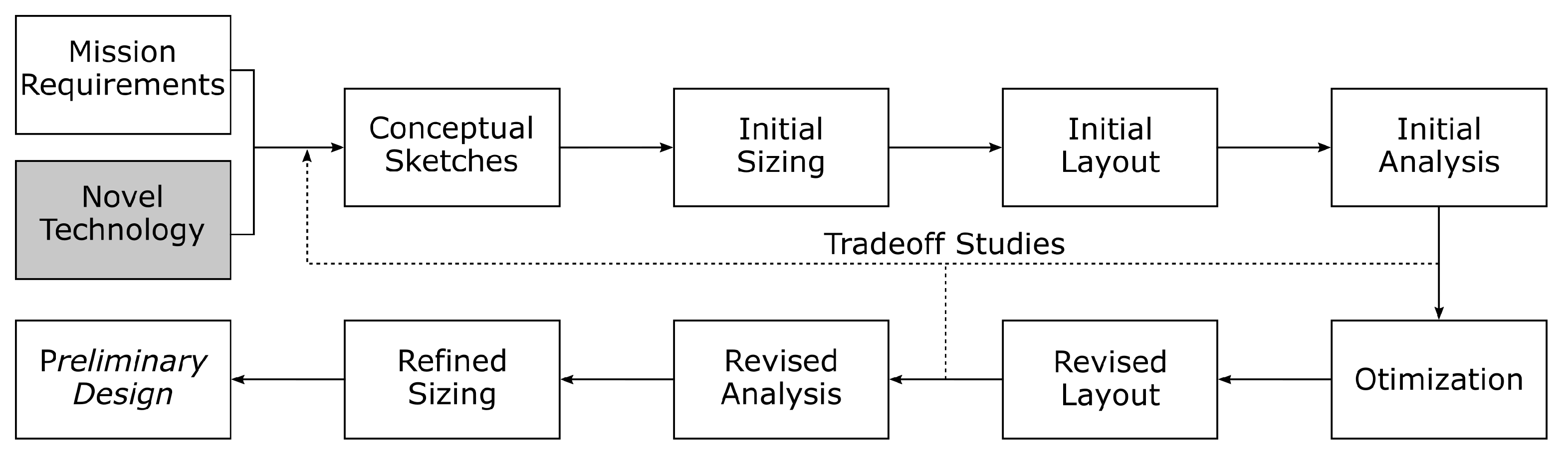
2.2. Design Methodology
2.3. Mission Specifications and Design Parameters
3. Initial Sizing
3.1. Weight Estimation
- The approach takes the aspects of electric or hybrid-electric fuel cell-based propulsion into consideration;
- The breakdown of the weight components is more detailed, as in lighter aircraft it is more critical to differentiate the weight of subsystems and avionics from the generic empty weight, as they might have more significant impact in terms of weight fractions.
3.2. Aerodynamic Sizing
4. Conceptual Design Phase
4.1. Configuration Layout
4.2. Aerodynamic Analysis Methodology
4.2.1. Low-Fidelity Numerical Methods
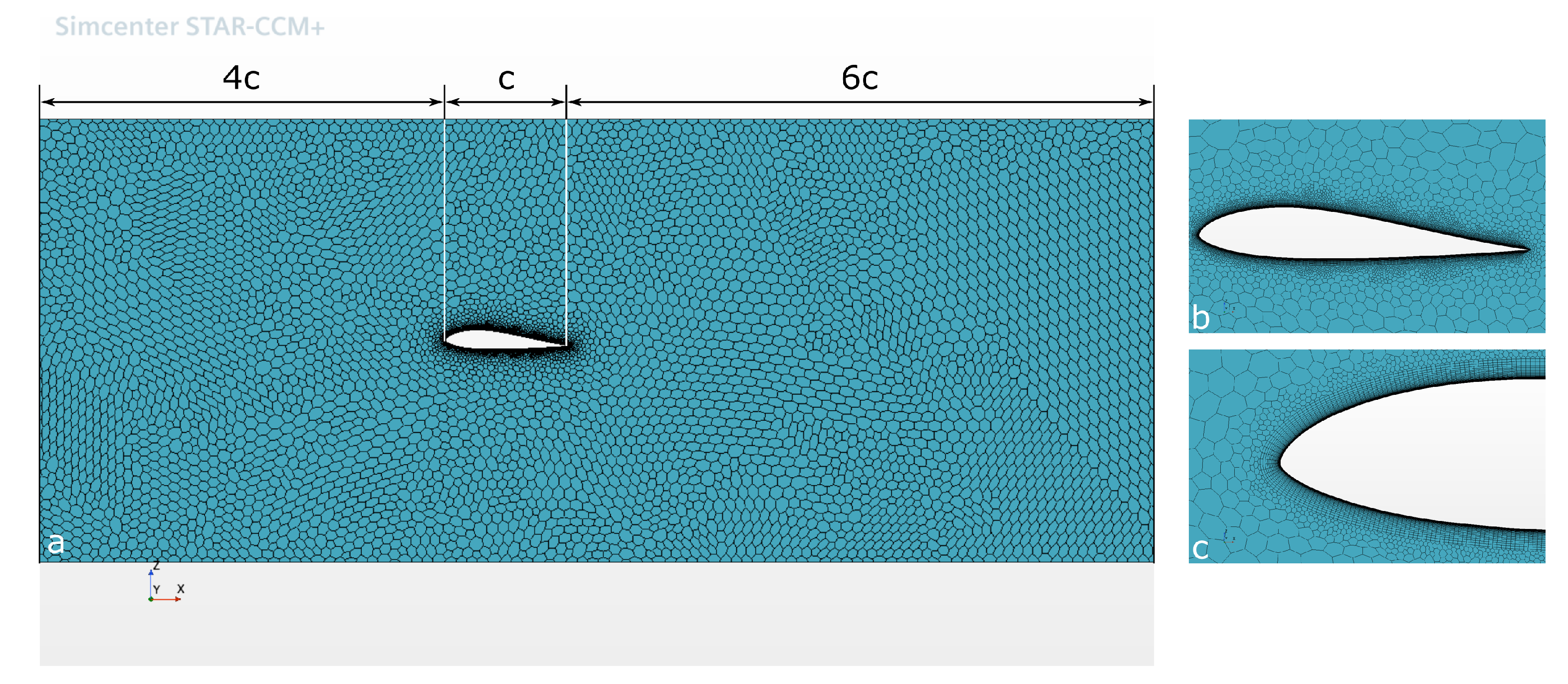
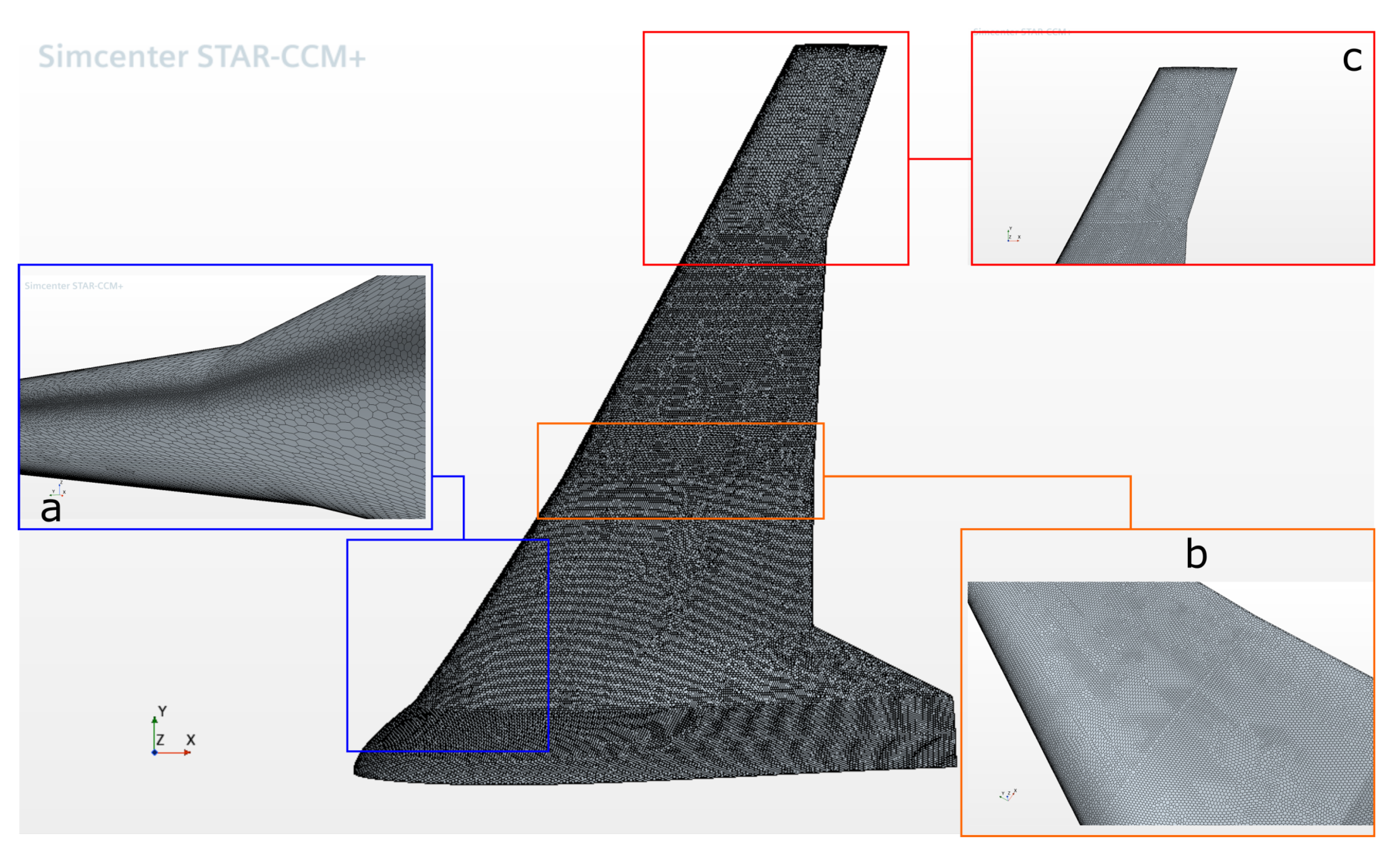
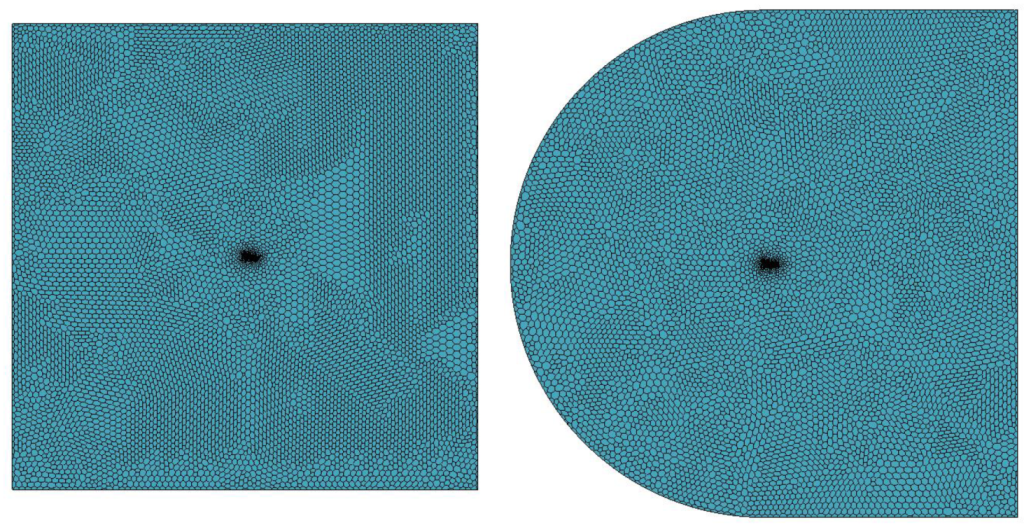
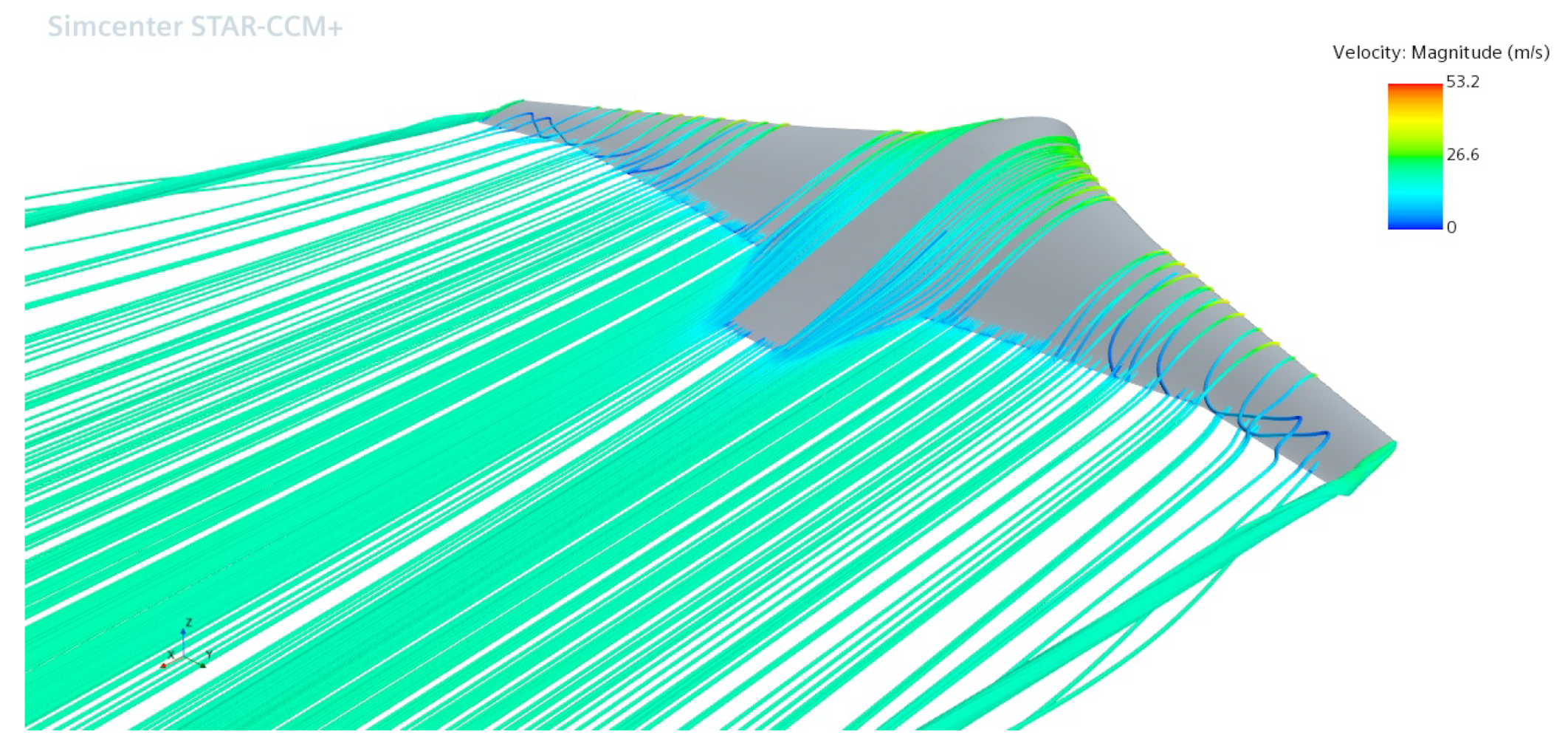
4.2.2. CFD Methodology
- The wing minimum surface size, which in practice defines the wing leading edge smallest surface cell dimension;
- The wing target surface size, i.e., the upper and lower wing surface cell maximum dimension;
- The external domain boundaries target surface size, i.e., the far-field maximum cell surface dimension;
- The characteristic cell dimension within the cone-delimited wingtip mesh region.
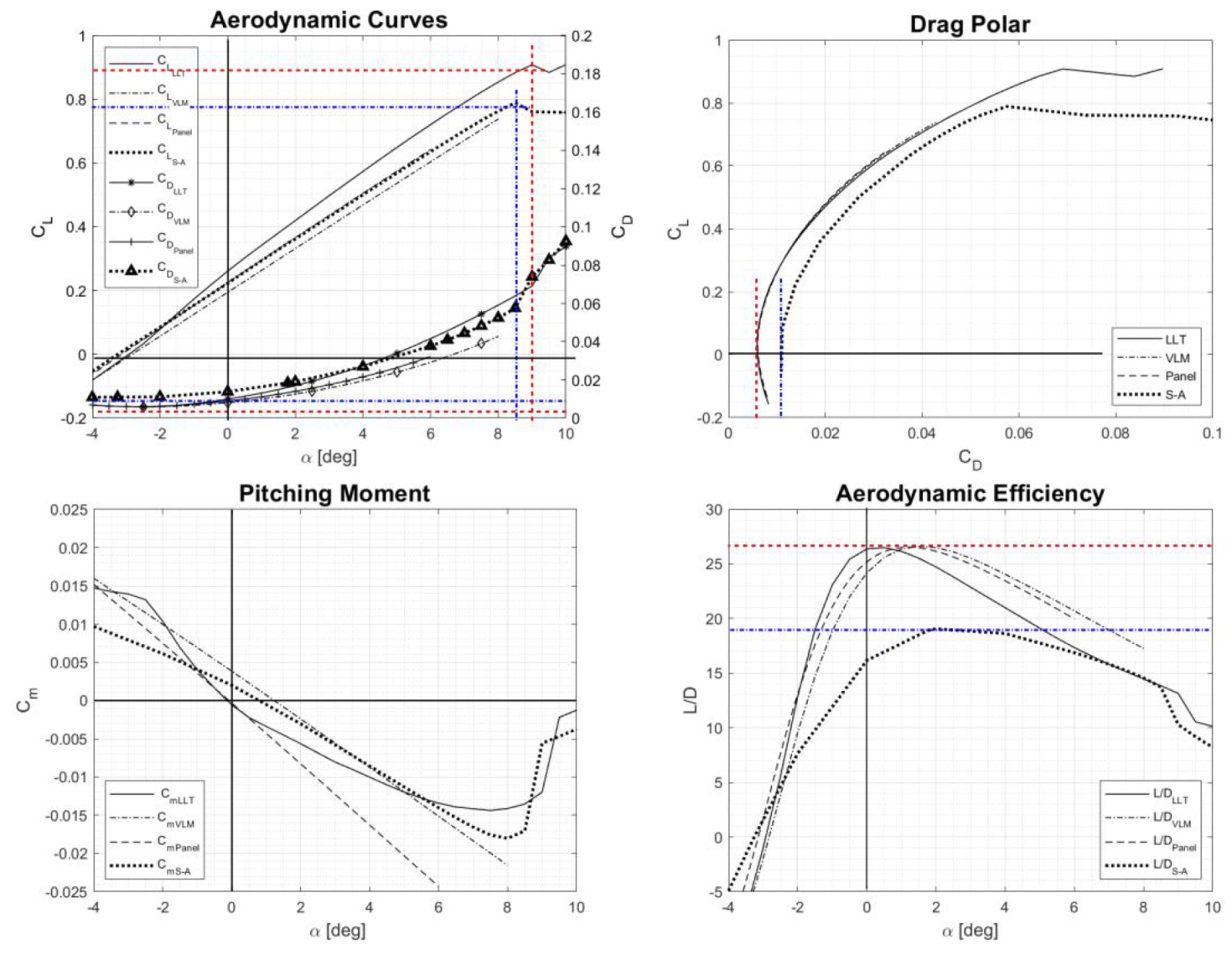
4.3. Aerodynamic Performance
5. Power Requirements and Battery Definition
5.1. Power Required Analysis
5.2. Hybrid Hydrogen Fuel Cell/Battery System
6. Future Work
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Centracchio, F.; Rossetti, M.; Iemma, U. Approach to the weight estimation in the conceptual design of hybrid-electric-powered unconventional regional aircraft. J. Adv. Transp. 2018, 2018, 1–15. [Google Scholar] [CrossRef] [Green Version]
- Hordé, T.; Achard, P.; Metkemeijer, R. PEMFC Application for aviation: Experimental and numerical study of sensitivity to altitude. Int. J. Hydrogen Energy 2012, 37, 10818–10829. [Google Scholar] [CrossRef]
- Winter, M.; Brodd, R.J. What Are Batteries, Fuel Cells, and Supercapacitors? J. Chem. Rev. 2003, 104, 4245–4269. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Baroutaji, A.; Wilberforce, T.; Ramadan, M.; Ghani Olabi, A. Comprehensive investigation on hydrogen and fuel cell technology in the aviation and aerospace sectors. Renew. Sustain. Energy Rev. 2019, 106, 31–40. [Google Scholar] [CrossRef] [Green Version]
- Peters, R.; Samsun, R.C. Evaluation of multifunctional fuel cell systems in aviation using a multistep process analysis methodology. Appl. Energy 2013, 111, 46–63. [Google Scholar] [CrossRef]
- Guida, D.; Muntillo, M. Design methodology for a PEM fuel cell power system in a more electrical aircraft. Appl. Energy 2017, 192, 446–456. [Google Scholar] [CrossRef]
- Romeo, G.; Borello, F.; Correa, G. Setup and test flights of all-electric two-seater aeroplane powered by fuel cells. J. Aircr. 2011, 48, 1331–1341. [Google Scholar] [CrossRef]
- Romeo, G.; Borello, F.; Correa, G.; Cestino, E. ENFICA-FC: Design of transport aircraft powered by fuel cell & flight test of zero emission 2-seater aircraft powered by fuel cells fueled by hydrogen. Int. J. Hydrogen Energy 2013, 28, 469–479. [Google Scholar]
- Barrett, S. ZeroAvia completes first hydrogen-electric passenger plane flight. Fuel Cells Bull. 2020, 6. [Google Scholar]
- Gong, A.; Verstraete, D. Fuel cell propulsion in small fixed-wing unmanned aerial vehicles: Current status and research needs. Int. J. Hydrogen Energy 2017, 42, 21311–21333. [Google Scholar] [CrossRef]
- Swider-Lyons, K.E.; MacKrell, J.A.; Rodgers, J.A.; Page, G.S.; Schuette, M.; Stroman, R.O. Hydrogen fuel cell propulsion for long endurance small UAVs. In Proceedings of the Name of the Conference, AIAA Centennial of Naval Aviation Forum “100 Years of Achievement and Progress”, Virginia Beach, VA, USA, 21–22 September 2011. [Google Scholar]
- Stroman, R.O.; Schuette, M.W.; Swider-Lyons, K.; Rodgers, J.A.; Edwards, D.J. Liquid hydrogen fuel system design and demonstration in a small long endurance air vehicle. Int. J. Hydrogen Energy 2014, 39, 11279–11290. [Google Scholar] [CrossRef]
- Bradley, T.H.; Moffitt, B.A.; Mavris, D.M.; Parekh, D.E. Development and experimental characterization of a fuel cell powered aircraft. J. Power Sources 2007, 39, 11279–11290. [Google Scholar] [CrossRef]
- Rhoads, G.D.; Wagner, N.A.; Taylor, B.J.; Keen, D.B.; Bradley, T.H. Design and flight test results for a 24 h fuel cell unmanned air vehicle. In Proceedings of the 8th Annual International Energy Conversion Engineering Conference, Nashville, TN, USA, 25–28 July 2010. [Google Scholar]
- Herwerth, C.; Chiang, C.; Ko, A.; Matsuyama, S.; Choi, S.B.; Mirmirani, M.; Gamble, D.; Paul, R.; Sanchez, V.; Arena, A.; et al. Development of a Small Long Endurance Hybrid PEM Fuel Cell Powered UAV; Aerospace Technology Conference and Exposition, SAE Technical Paper; SAE International: Warrendale, PE, USA, 2007. [Google Scholar]
- Gong, A.; Palmer, J.L.; Brian, G.; Harvey, J.R.; Verstraete, D. Performance of a hybrid, fuel-cell-based power system during simulated small unmanned aircraft missions. Int. J. Hydrogen Energy 2016, 41, 11418–11426. [Google Scholar] [CrossRef]
- Gang, B.G.; Kim, H.; Kwon, S. Ground simulation of a hybrid power strategy using fuel cells and solar cells for high-endurance unmanned aerial vehicles. Energy 2017, 141, 1547–1554. [Google Scholar] [CrossRef]
- Kim, K.; Kim, T.; Kwon, S. Fuel cell systems with sodium borohydride as hydrogen source for unmanned aerial vehicles. J. Power Sources 2011, 196, 9069–9075. [Google Scholar] [CrossRef]
- Lapena-Rey, N.; Blanco, J.A.; Ferreyra, E.; Lemus, J.L.; Pereira, S.; Serrot, E. A fuel cell powered unmanned aerial vehicle for low altitude surveillance missions. Int. J. Hydrogen Power 2017, 42, 6926–6940. [Google Scholar] [CrossRef]
- Gadalla, M.; Zafar, S. Analysis of a hydrogen fuel cell-PV power system for small UAV. Int. J. Hydrogen Energy 2016, 41, 6422–6432. [Google Scholar] [CrossRef]
- Renau, J.; Lozano, A.; Barroso, J.; Miralles, J.; Martín, J.; Sánchez, F.; Barreras, F. Use of fuel cell stacks to achieve high altitudes in light unmanned aerial vehicles. Int. J. Hydrogen Energy 2015, 40, 14573–14583. [Google Scholar] [CrossRef] [Green Version]
- Depcik, C.; Cassady, T.; Collicott, B.; Burugupally, S.P.; Li, X.; Alam, S.S.; Arandia, J.R.; Hobeck, J. Comparison of lithium ion batteries, hydrogen fueled combustion engines, and a hydrogen fuel cell in powering a small unmanned aerial vehicle. Energy Convers. Manag. 2020, 207, 112514. [Google Scholar] [CrossRef]
- Verstraete, D.; Lehmkuehler, K.; Wong, K.C. Design of a Fuell Cell Powered Blended Wing Body UAV. In Proceedings of the ASME 2012 International Mechanical Engineering Conference & Exposition, IMECE 2012, Houston, TX, USA, 9–15 November 2012. IMECE2012-88871. [Google Scholar]
- Özbek, E.; Yalin, G.; Ekici, S.; Karakoc Hikmet, T. Evaluation of design methodology, limitations, and iterations of a hydrogen fuelled hybrid fuel cell mini UAV. Energy 2020, 213, 118757. [Google Scholar] [CrossRef]
- Savvaris, A.; Xie, Y.; Malandrakis, K.; Lopez, M.; Tsourdos, A. Development of a fuel cell hybrid-powered unmanned aerial vehicle. In Proceedings of the 24th Mediterranean Conference on Control and Automation (MED), Athens, Greece, 21–24 June 2016. [Google Scholar]
- Project for an Unmanned Airplane with Zero Emissions to cross the South Atlantic. Available online: https://www.isae-supaero.fr/en/news/project-for-an-unmanned-airplane-with-zero-emissions-to-cross-the-south/ (accessed on 2 March 2021).
- De Wagter, C.; Remes, B.; Smeur, E.; van Tienen, F.; Ruijsink, R.; van Hecke, K.; van der Horst, E. The NederDrone: A hybrid lift, hybrid energy hydrogen UAV. Int. J. Hydrogen Energy 2021, 46, 16003–16018. [Google Scholar] [CrossRef]
- Project Phoenix. Available online: https://aerodelft.nl/project-phoenix/ (accessed on 12 May 2022).
- DELAIR. Available online: https://delair.aero/delair-commercial-drones/hydrone/ (accessed on 7 September 2021).
- Guynn, M.D.; Freeh, J.E.; Olson, E.D. Evaluation of a Hydrogen Fuel Cell Powered Blended-Wing-Body Aircraft Concept for Reduced Noise and Emissions. In NASA/TM-2004-212989; National Aeronautics and Space Administration: Washington, DC, USA, 2004. [Google Scholar]
- ZEROe, towards the World’s First Zero-Emission Commercial Aircraft. Available online: https://www.airbus.com/innovation/zero-emission/hydrogen/zeroe.html (accessed on 7 September 2021).
- Reist, T.A.; Zingg, D.W. Aerodynamic design of blended wing body and lifting-fuselage aircraft. In Proceedings of the 34th AIAA Applied Aerodynamics Conference, Session: Innovative Aerodynamic Concepts & Designs, Washington, DC, USA, 13–17 June 2016. AIAA 2016-3874. [Google Scholar]
- Bradley, K.R. A sizing methodology for the conceptual design of blended-wing-body transports. In NASA/CR-2004-213016; National Aeronautics and Space Administration: Washington, DC, USA, 2004. [Google Scholar]
- Crawley, E.F.; Malmqvist, J.; Östlund, S.; Brodeur, D.R.; Edström, K. Rethinking Engineering Education. The CDIO Approach, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Raymer, D.P. Aircraft Design: A Conceptual Approach, 6th ed.; AIAA Educational Series; AIAA: Reston, VA, USA, 2018; pp. 27–94; discussion 834–838. [Google Scholar]
- Panagiotou, P.; Fotiadis-Karras, S.; Yakinthos, K. Conceptual design of a Blended Wing Body MALE UAV. Aerosp. Sci. Technol. 2018, 73, 32–47. [Google Scholar] [CrossRef]
- HES Energy Systems. Available online: https://www.hes.sg/ (accessed on 7 September 2021).
- Intelligent Energy. Available online: https://www.intelligent-energy.com/ (accessed on 7 September 2021).
- Gundlach, J. Designing Unmanned Aircraft Systems: A Comprehensive Approach, 3rd ed.; AIAA Educational Series; AIAA: Reston, VA, USA, 2012. [Google Scholar]
- Liebeck, R.H. Design of the blended wing body subsonic transport. J. Aircr. 2004, 41, 10–25. [Google Scholar] [CrossRef] [Green Version]
- Qin, N.; Vavalle, A.; Le Moigne, A.; Laban, M.; Hackett, K.; Weinerfelt, P. Aerodynamic considerations of blended wing body aircraft. Prog. Aerosp. Sci. 2004, 40, 321–343. [Google Scholar] [CrossRef]
- Qin, N.; Vavalle, A.; Le Moigne, A. Spanwise lift distribution for blended wing body aircraft. J. Aircraft 2005, 42, 356–365. [Google Scholar] [CrossRef]
- Airfoil Tools. Available online: http://airfoiltools.com/search/index (accessed on 7 September 2021).
- Drela, M. XFOIL: An Analysis and Design System for Low Reynolds Number Airfoils. In Lectures Noted in Engineering, Proceedings of the Conference in Low Reynolds Number Aerodynamics, Notre Dame, IN, USA, 5–7 June 1989; Mueller, T.J., Ed.; Springer: Berlin, Germany, 1989. [Google Scholar]
- Anderson, J.D. Aircraft Performance and Design; McGraw-Hill Int. Editions: Singapore, 1999. [Google Scholar]
- Pamadi, B.N. Performance, Stability, Dynamics and Control of Airplanes; AIAA Educational Series; AIAA: Reston, VA, USA, 1998. [Google Scholar]
- Panagiotou, P.; Yakinthos, K. Parametric aerodynamic study of Blended-Wing-Body platforms at low subsonic speeds for UAV applications. In Proceedings of the 35th AIAA Applied Aerodynamics Conference, AIAA AVIATION Forum, Denver, CO, USA, 5–9 June 2017. AIAA 2017-3737. [Google Scholar]
- Panagiotou, P.; Kaparos, P.; Salpingidou, C.; Yakinthos, K. Aerodynamic design of a MALE UAV. Aerosp. Sci. Technol. 2016, 50, 127–138. [Google Scholar] [CrossRef]
- Koster, J.; Balaban, S.; Hillery, D.; Hmbargar, C.; Nasso, D.; Serani, E.; Velazco, A. Design of a Blended Wing Body UAS with Hybrid Propulsion. In Proceedings of the ASME 2011 International Mechanical Engineering Congress & Exposition, IMECE2011, Denver, CO, USA, 11–17 November 2011. IMECE2011-62126. [Google Scholar]
- McDonald, R.A.; Gloudemans, J.R. Open Vehicle Sketch Pad: An Open Source Parametric Geometry and Analysis Tool for Conceptual Aircraft Design. In Proceedings of the AIAA SCITECH 2022 Forum, San Diego, CA, USA, 3–7 January 2022. [Google Scholar]
- xflr5. Available online: http://www.xflr5.tech/xflr5.htm (accessed on 7 September 2021).
- Tomac, M.; stenfelt, G. Predictions of stability and control for a flying wing. Aerosp. Sci. Technol. 2014, 39, 179–186. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef] [Green Version]
- Douvi, E.C.; Tsavalos, I.A.; Margaris, P.D. Evaluation of the turbulence models for the simulation of the flow over a National Advisory Committee for Aeronautics (NACA) 0012 airfoil. J. Mech. Eng. Res. 2012, 4, 100–111. [Google Scholar]
- Menter, F.R.; Kuntz, M.; Langtry, R. Ten Years of Industrial Experience with the SST Turbulence Model. Turbul. Heat Mass Transf. 2003, 4, 625–632. [Google Scholar]
- Spalart, P.R.; Rumsey, C.L. Effective inflow conditions for Turbulence Models in Aerodynamic calculations by Spalart and Rumsey. AIAA J. 2007, 45, 2544–2553. [Google Scholar] [CrossRef]
- Goetten, F.; Finger, D.F.; Marino, M.; Bil, C. A revire of guidelines and best practices for subsonic aerodynamic simulations unsing RANS CFD. In Proceedings of the Asia Pacific International Symposium on Aerospace Technology, Gold Coast, Australia, 4–6 December 2019; ISBN 978-1-925627-40-4. [Google Scholar]
- Versteeg, H.; Malalasekera, W. An Introduction to Computational Fluid Dynamics: The Finite Volume Method, 2nd ed.; Pearson: London, UK, 2007. [Google Scholar]
- Chiche, A.; Lindbergh, G.; Stenius, I.; Lagergren, C. A Strategy for Sizing and Optimizing the Energy System on Long-Range AUVs. IEEE J. Ocean. Eng. 2021, 1132–1143. [Google Scholar] [CrossRef]
- Chiche, A.; Andruetto, C.; Lagergren, C.; Lindbergh, G.; Stenius, I.; Peretti, L. Feasibility and impact of a Swedish fuel cell-powered rescue boat. Ocean. Eng. 2021, 234, 109259. [Google Scholar] [CrossRef]
- Wikner, E.; Thiringer, T. Extending Battery Lifetime by Avoiding High SOC. Appl. Sci. 2018, 8, 1–16. [Google Scholar] [CrossRef] [Green Version]
- Sturm, J.; Frank, A.; Rheinfeld, A.; Erhard, S.V.; Jossen, A. Impact of electrode and cell design on fast charging capabilities of cylindrical lithium-ion batteries. J. Electrochem. Soc. 2020, 167, 130505. [Google Scholar] [CrossRef]





| Aircraft | Mass [kg] | Wing Span [m] | Cruise Velocity [m/s] | Aspect Ratio | Fuel Cell Power [W] |
|---|---|---|---|---|---|
| Bradley et al. [13] | 16.4 | 6.58 | 14.5 | 23 | 500 |
| Rhoads et al. [14] | 13.4 | 5.54 | - | 22.76 | 600 |
| Swider-Lyons et al. [11] | 16 | 5.18 | 13.89 | 17 | 550 |
| Renau et al. [22] | 16 | 4 | - | - | 700 |
| Savvaris et al. [25] | 13.04 | - | - | 8.5 | 500 |
| Lapena-Rey et al. [19] | 11 | 4.7 | - | 22 | 500 |
| Özbek et al. [24] | 6.5 | 3 | 16 | 9.76 | 250 |
| Item | Mass [g] |
|---|---|
| Energy and Storage | |
| Fuel Cell | 810 |
| Hybrid Battery | 230 |
| Hydrogen Mass | 80 |
| Hydrogen Storage | 1400 |
| Regulator | 250 |
| Extra Battery | 2000 |
| Avionics | |
| Pixhawk 4 | 20 |
| Holybro GPS Module | 30 |
| Holybro Telemetry | 30 |
| Propulsion | |
| Emax Gran Turbo Motor | 140 |
| APC Propeller | 25 |
| Brushless ESC | 95 |
| Servos | 50 |
| Subsystems | |
| Tricycle Landing Gear | 420 |
| Symbol | WF | W [N] |
|---|---|---|
| 1.000 | 245.3 | |
| 0.565 | 138.65 | |
| 0.075 | 18.4 | |
| 0.095 | 23.3 | |
| 0.050 | 12.3 | |
| 0.055 | 13.5 | |
| 0.160 | 39.2 |
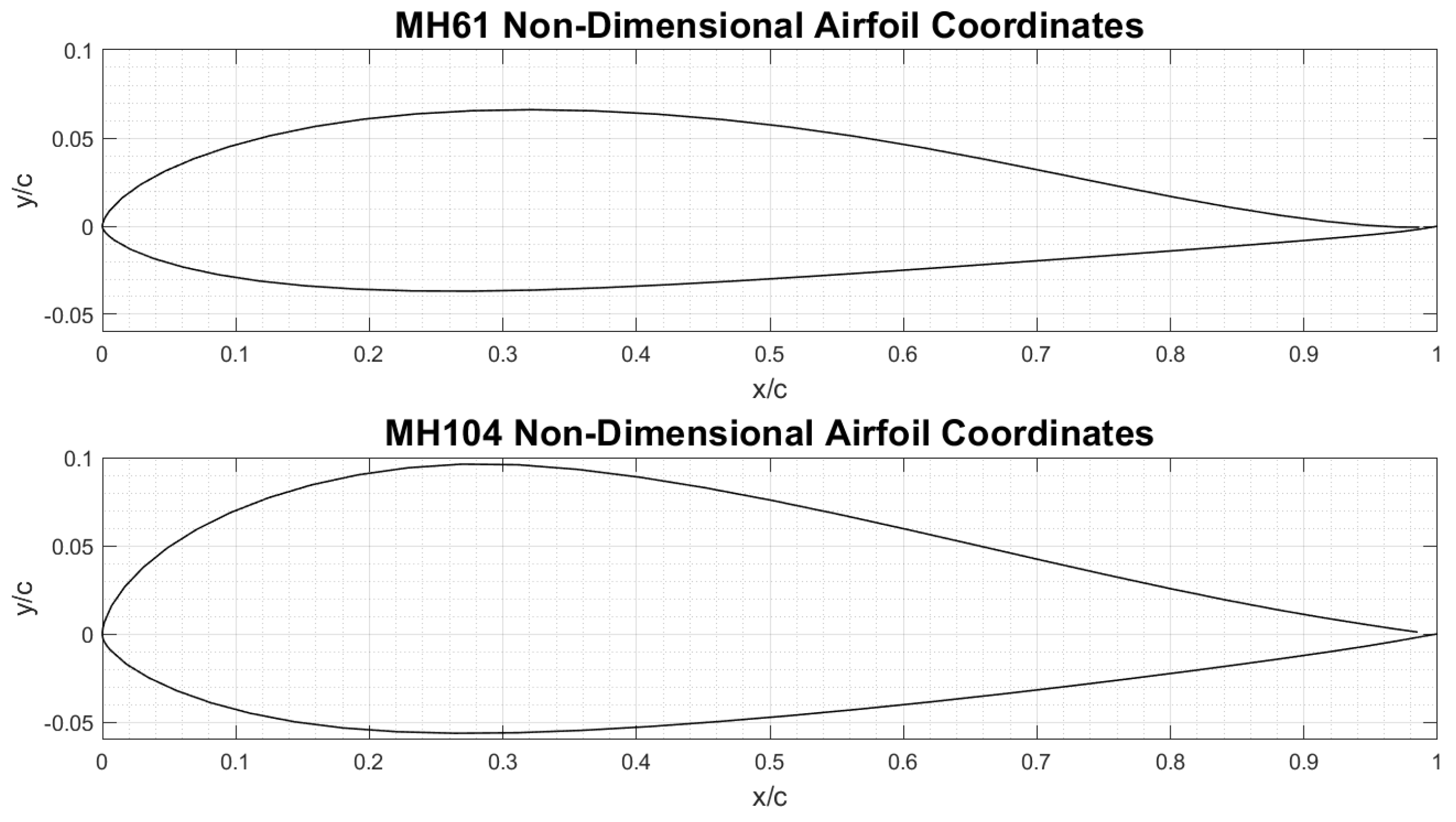
| Aircraft | Airfoil | (t/c) | Max Camber | ||
|---|---|---|---|---|---|
| Section | Section | % | %c | % | %c |
| Fuselage | MH104 | 15.2 | 26.4 | 1.9 | 31.1 |
| Wings | MH61 | 10.2 | 27.6 | 1.4 | 37.3 |
| Parameter | Symbol | Unit | |
|---|---|---|---|
| Aspect Ratio | AR | 5.47 | [-] |
| Planform Area | S | 2.92 | [m] |
| Wing Sweep | 30 | [] | |
| Wing Loading | W/S | 83.9 | [N/m] |
| Stall Speed | 12 | [m/s] | |
| Max. Sectonal Lift Coefficient | 1.22 | [-] | |
| Max. Lift Coefficient | 0.95 | [-] | |
| Drag Coefficient | 0.078 | [-] | |
| Zero-Lift Drag Coefficient | 0.015 | [-] | |
| Oswald Efficiency Factor | e | 0.83 | [-] |
| Width | Length | Height | Weight | |
|---|---|---|---|---|
| [mm] | [mm] | [mm] | [kg] | |
| Fuel Cell Stack | 170 | 200 | 110 | 1.0 |
| 3 L Composite Tank | 122 | 400 | 122 | 2.3 |
| Parameter | Symbol | Unit | |
|---|---|---|---|
| Wing Span | 4 | 4 | [m] |
| Centre Chord | 1.7 | [m] | |
| Root Chord | 1.29 | [m] | |
| Tip Chord | 0.25 | [m] | |
| Aspect Ratio | AR | 5.57 | [-] |
| Planform Area | S | 2.87 | [m] |
| Wing Sweep (avg) | 30 | [] | |
| Wing Loading | W/S | 85.5 | [N/m] |
| Oswald Efficiency Facort | e | 0.88 | [-] |
| Domain | Domain | Domain | Domain | |||
|---|---|---|---|---|---|---|
| Shape | [] | Length | Width | Height | [-] | [-] |
| Box | 2 | 80 | 40 | 30 | 0.354 | 0.0193 |
| Bullet | 2 | 40 radius | [-] | [-] | 0.354 | 0.0193 |
| 4c–6c Box | 2 | [-] | [-] | [-] | 0.362 | 0.0190 |
| Box | 10 | 80 | 40 | 30 | 0.709 | 0.0935 |
| Bullet | 10 | 40 radius | [-] | [-] | 0.727 | 0.0937 |
| 4c–6c Box | 10 | [-] | [-] | [-] | 0.725 | 0.0958 |
| Simulation | Cell | Wing | Wing | Wing Tip | External Domain |
|---|---|---|---|---|---|
| Reference | Count | MSS | TSS | Cone Cell | DBoundaries TSS |
| 4(PL2) | 4.7 × 10 | 1 mm | 7.5 mm | 30 mm | 150 mm |
| Parameter | Unit | VLM2 | Non-Linear | Panel | CFD | Initial |
|---|---|---|---|---|---|---|
| LLT | Method | - | Sizing | |||
| a | [] | 0.068 | 0.079 | 0.071 | 0.068 | - |
| [-] | - | 0.90 | - | 0.789 | 0.95 | |
| [] | - | 9 | - | 8.5 | - | |
| [-] | 0.008 | 0.009 | 0.009 | 0.011 | 0.015 | |
| [-] | 0.004 | 0.000 | 0.009 | 0.002 | - | |
| [] | −0.0031 | −0.0026 | −0.0039 | −0.0024 | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suewatanakul, S.; Porcarelli, A.; Olsson, A.; Grimler, H.; Chiche, A.; Mariani, R.; Lindbergh, G. Conceptual Design of a Hybrid Hydrogen Fuel Cell/Battery Blended-Wing-Body Unmanned Aerial Vehicle—An Overview. Aerospace 2022, 9, 275. https://doi.org/10.3390/aerospace9050275
Suewatanakul S, Porcarelli A, Olsson A, Grimler H, Chiche A, Mariani R, Lindbergh G. Conceptual Design of a Hybrid Hydrogen Fuel Cell/Battery Blended-Wing-Body Unmanned Aerial Vehicle—An Overview. Aerospace. 2022; 9(5):275. https://doi.org/10.3390/aerospace9050275
Chicago/Turabian StyleSuewatanakul, Siwat, Alessandro Porcarelli, Adam Olsson, Henrik Grimler, Ariel Chiche, Raffaello Mariani, and Göran Lindbergh. 2022. "Conceptual Design of a Hybrid Hydrogen Fuel Cell/Battery Blended-Wing-Body Unmanned Aerial Vehicle—An Overview" Aerospace 9, no. 5: 275. https://doi.org/10.3390/aerospace9050275
APA StyleSuewatanakul, S., Porcarelli, A., Olsson, A., Grimler, H., Chiche, A., Mariani, R., & Lindbergh, G. (2022). Conceptual Design of a Hybrid Hydrogen Fuel Cell/Battery Blended-Wing-Body Unmanned Aerial Vehicle—An Overview. Aerospace, 9(5), 275. https://doi.org/10.3390/aerospace9050275








