Mean Reversion Lessens Mean Blur: Evidence from the S&P Composite Index
Abstract
1. Introduction
2. Literature Review
3. Data Set
4. Preliminary Analysis
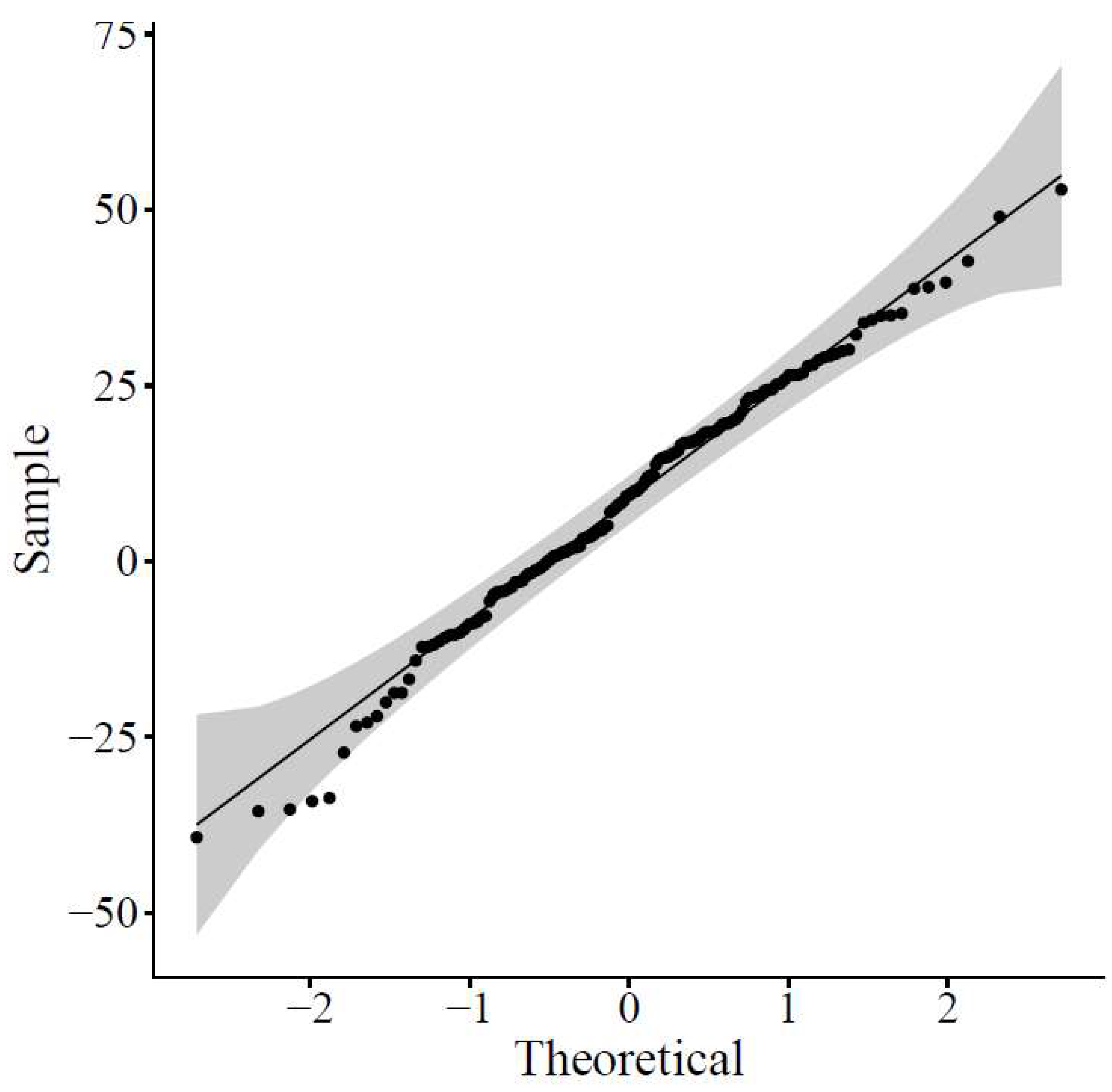
4.1. Aggregational Normality
4.2. Serial Correlation

4.3. Rescaled Range Analysis
5. Statistical Findings
5.1. Mean Blur
5.2. Stationarity Tests
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| 1 | As remarked by an anonymous reviewer, the above-mentioned unit root tests may be misleading whenever data are affected by structural breaks. According to the Zivot-Andrews test, the null hypothesis of a unit root is rejected once more with a confidence level of 0.99 for real rates of logarithmic return. A structural break occurring in July 2000 has been detected, which is in line with Table 5 in Nguyen et al. (2022). The same authors summarize the relevant econometric literature. |
References
- Black, Fischer. 1993. Estimating expected return. Financial Analysts Journal 49: 36–38. [Google Scholar] [CrossRef]
- Buffett, Warren, and Carol Loomis. 2001. Warren Buffett on the Stock Market. Fortune 144: 40–46. [Google Scholar]
- Campbell, John Y., and Robert J. Shiller. 1998. Valuation ratios and the long-run stock market outlook. Journal of Portfolio Management 24: 11–26. [Google Scholar] [CrossRef]
- Campbell, John Y., Andrew W. Lo, and A. Craig MacKinlay. 1997. The Econometrics of Financial Markets. Princeton: Princeton University Press. [Google Scholar]
- Corazza, Marco, and Anastasios G. Malliaris. 2002. Multifractality in foreign currency markets. Multinational Finance Journal 6: 387–401. [Google Scholar] [CrossRef]
- Cornell, Bradford. 2010. Economic growth and equity investing. Financial Analysts Journal 66: 54–64. [Google Scholar] [CrossRef]
- Dunbar, Nicholas, and Nick Dunbar. 2001. Inventing Money. The Story of Long-Term Capital Management and the Legends behind it. Chichester: Wiley. [Google Scholar]
- Fama, Eugene F., and Kenneth R. French. 1988a. Permanent and temporary components of stock prices. Journal of Political Economy 96: 246–73. [Google Scholar] [CrossRef]
- Fama, Eugene F., and Kenneth R. French. 1988b. Dividend yields and expected stock returns. Journal of Financial Economics 22: 3–25. [Google Scholar] [CrossRef]
- Golez, Benjamin, and Peter Koudijs. 2018. Four centuries of return predictability. Journal of Financial Economics 127: 248–63. [Google Scholar] [CrossRef]
- Haugen, Robert A. 1997. Modern Investment Theory, 4th ed. Upper Saddle River: Prentice-Hall. [Google Scholar]
- Hurst, Harold E. 1956. The problem of long-term storage in reservoirs. Hydrological Sciences Journal 1: 13–27. [Google Scholar] [CrossRef]
- Hurst, Harold Edwin. 1951. The long-term storage capacity of reservoirs. Transactions of the American Society of Civil Engineers 116: 770–99. [Google Scholar] [CrossRef]
- Lo, Andrew W., and A. Craig MacKinlay. 1988. Stock market prices do not follow random walks: Evidence from a simple specification test. Review of Financial Studies 1: 41–66. [Google Scholar] [CrossRef]
- Loomis, Carol, and Warren Buffett. 1999. Mr. Buffett on the Stock Market. Fortune 140: 110–15. [Google Scholar]
- Luenberger, David G. 2014. Investment Science, 2nd ed. New York: Oxford University Press. [Google Scholar]
- Maginn, John L., Donald L. Tuttle, Dennis W. McLeavey, and Jerald E. Pinto. 2007. The portfolio management process and the investment policy statement. In Managing Investment Portfolios. A Dynamic Process, 3rd ed. Edited by John L. Maginn, Donald L. Tuttle, Dennis W. McLeavey and Jerald E. Pinto. Hoboken: Wiley, pp. 1–19. [Google Scholar]
- Nguyen, James, Wei-Xuan Li, and Clara Chia-Sheng Chen. 2022. Mean Reversions in Major Developed Stock Markets: Recent Evidence from Unit Root, Spectral and Abnormal Return Studies. Journal of Risk and Financial Management 15: 162. [Google Scholar] [CrossRef]
- Peters, Edgar E. 1994. Fractal Market Analysis. New York: Wiley. [Google Scholar]
- Poterba, James M., and Lawrence H. Summers. 1988. Mean reversion in stock prices: Evidence and implications. Journal of Financial Economics 22: 27–59. [Google Scholar] [CrossRef]
- Royal Swedish Academy of Sciences. 2013. Trendspotting in Asset Markets. Available online: https://www.nobelprize.org/uploads/2018/06/popular-economicsciences2013.pdf (accessed on 4 March 2022).
- Sharpe, William F., Peng Chen, Jerald. E. Pinto, and Dennis. W. McLeavey. 2007. Asset allocation. In Managing Investment Portfolios. A Dynamic Process, 3rd ed. Edited by John L. Maginn, Donald L. Tuttle, Dennis W. McLeavey and Jerald E. Pinto. Hoboken: Wiley, pp. 230–327. [Google Scholar]
- Shiller, Robert J. 2015. Irrational Exuberance, 3rd ed. Princeton: Princeton University Press. [Google Scholar]
- Siegel, Jeremy J. 2014. Stocks for the Long Run, 5th ed. New York: McGraw-Hill. [Google Scholar]
- Spierdijk, Laura, and Jacob A. Bikker. 2017. Mean reversion in stock prices: Implications for long-term investors. In Pension Fund Economics and Finance. Efficiency, Investments and Risk-Taking. Edited by Jacob Bikker. London: Routledge. [Google Scholar]
- Summers, Lawrence H. 1986. Does the stock market rationally reflect fundamental values? Journal of Finance 41: 591–601. [Google Scholar] [CrossRef]
- von Hippel, Paul T. 2005. Mean, median, and skew: Correcting a textbook rule. Journal of Statistics Education 13. [Google Scholar] [CrossRef]
- Working, Holbrook. 1960. Note on the correlation of first differences of averages in a random chain. Econometrica 28: 916–18. [Google Scholar] [CrossRef]


| Linear Rate | Logarithmic Rate | |
|---|---|---|
| Worst rate | −26.19% | −30.36% |
| Median | 0.94% | 0.94% |
| Best rate | 52.43% | 42.15% |
| Negative rates | 39.21% | 39.21% |
| Mean | 0.65% | 0.56% |
| Standard deviation | 4.10% | 4.08% |
| Skewness | 0.58 | −0.36 |
| Kurtosis excess | 18.24 | 11.38 |
| Linear Rate | Logarithmic Rate | |
|---|---|---|
| Worst rate | −39.29% | −49.90% |
| Median | 10.01% | 9.54% |
| Best rate | 53.25% | 42.69% |
| Negative rates | 30.87% | 30.87% |
| Mean | 8.55% | 6.73% |
| Standard deviation | 17.98% | 17.63% |
| Skewness | −0.29 | −0.83 |
| Kurtosis excess | 0.03 | 0.92 |
| Rate | Jarque-Bera | Shapiro-Wilk |
|---|---|---|
| Linear | 0.3494 | 0.5082 |
| Logarithmic | 3.741 × 10−6 | 0.00021 |
| Real Linear | 0.3595 | 0.3214 |
| Real Logarithmic | 2.501 × 10−5 | 0.0001341 |
| 1872–2020 | 1872–1935 | 1936–2020 | |
|---|---|---|---|
| Worst rate | −39.29% | −36.66% | −39.29% |
| Median | 10.01% | 4.90% | 11.98% |
| Best rate | 53.25% | 53.25% | 49.16% |
| Negative rates | 30.87% | 32.81% | 29.41% |
| Mean | 8.55% | 8.61% | 8.51% |
| Standard deviation | 17.98% | 18.70% | 17.53% |
| Skewness | −0.29 | −0.04 | −0.52 |
| Kurtosis excess | 0.03 | 0.01 | 0.10 |
| 1872–2020 | 1872–1911 | 1912–1970 | 1971–2020 | |
|---|---|---|---|---|
| Worst rate | −39.29% | −27.94% | −36.66% | −39.29% |
| Median | 10.01% | 7.96% | 7.45% | 12.29% |
| Best rate | 53.25% | 40.53% | 53.25% | 34.99% |
| Negative rates | 30.87% | 27.50% | 35.59% | 28.00% |
| Mean | 8.55% | 8.67%% | 8.82% | 8.14% |
| Standard deviation | 17.98% | 14.27% | 21.39% | 16.51% |
| Skewness | −0.29 | −0.04 | −0.09 | −0.94 |
| Kurtosis excess | 0.03 | −0.05 | −0.55 | 0.69 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Buzzacchi, L.; Ghezzi, L. Mean Reversion Lessens Mean Blur: Evidence from the S&P Composite Index. Int. J. Financial Stud. 2023, 11, 22. https://doi.org/10.3390/ijfs11010022
Buzzacchi L, Ghezzi L. Mean Reversion Lessens Mean Blur: Evidence from the S&P Composite Index. International Journal of Financial Studies. 2023; 11(1):22. https://doi.org/10.3390/ijfs11010022
Chicago/Turabian StyleBuzzacchi, Luigi, and Luca Ghezzi. 2023. "Mean Reversion Lessens Mean Blur: Evidence from the S&P Composite Index" International Journal of Financial Studies 11, no. 1: 22. https://doi.org/10.3390/ijfs11010022
APA StyleBuzzacchi, L., & Ghezzi, L. (2023). Mean Reversion Lessens Mean Blur: Evidence from the S&P Composite Index. International Journal of Financial Studies, 11(1), 22. https://doi.org/10.3390/ijfs11010022






