Optimal Timing Fault Tolerant Control for Switched Stochastic Systems with Switched Drift Fault
Abstract
:1. Introduction
2. Problem Formulation
3. The Main Results
3.1. Single Switching
3.2. Multi-Switchings
3.3. Optimal Fault Tolerant Algorithm
4. Simulation
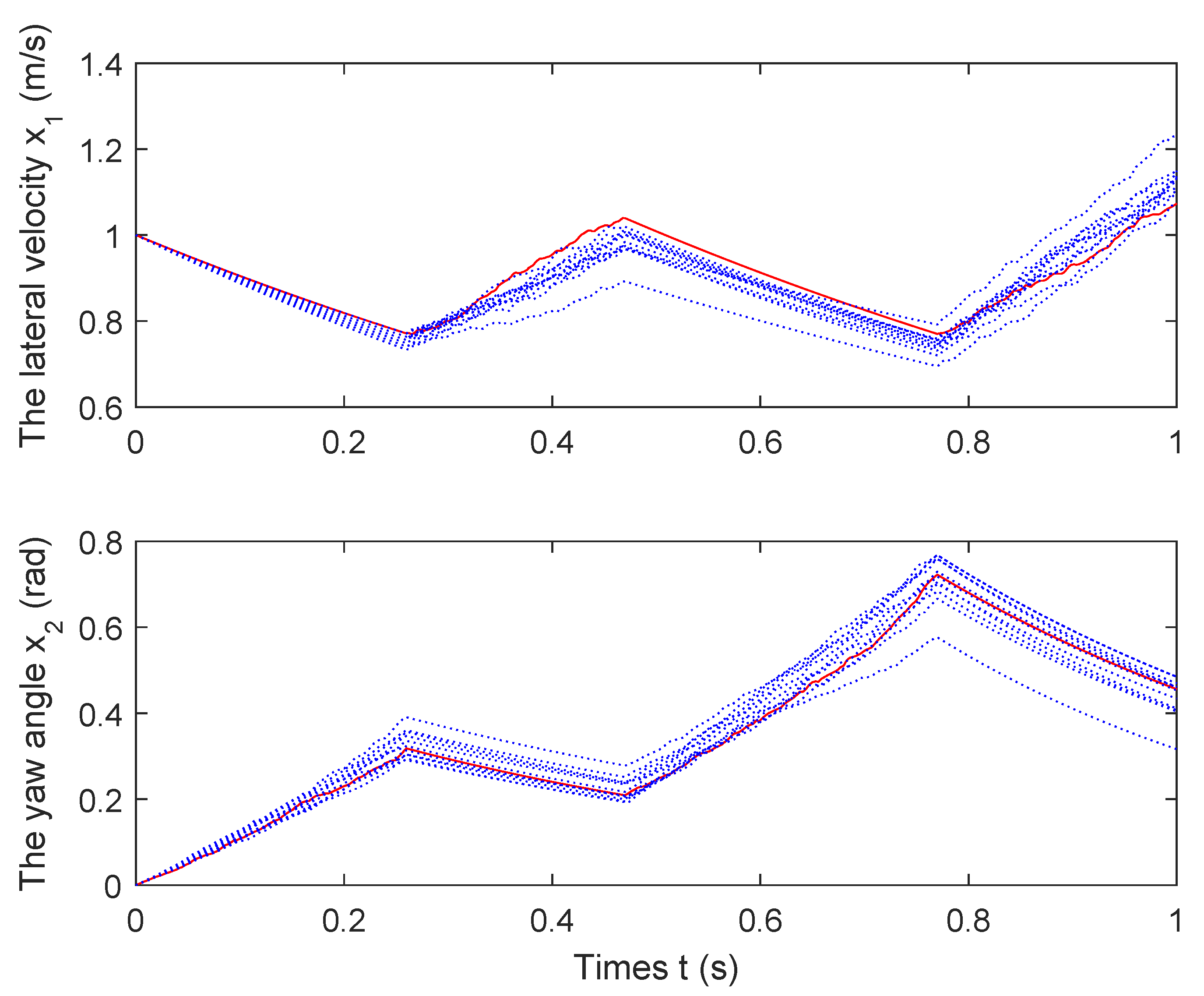
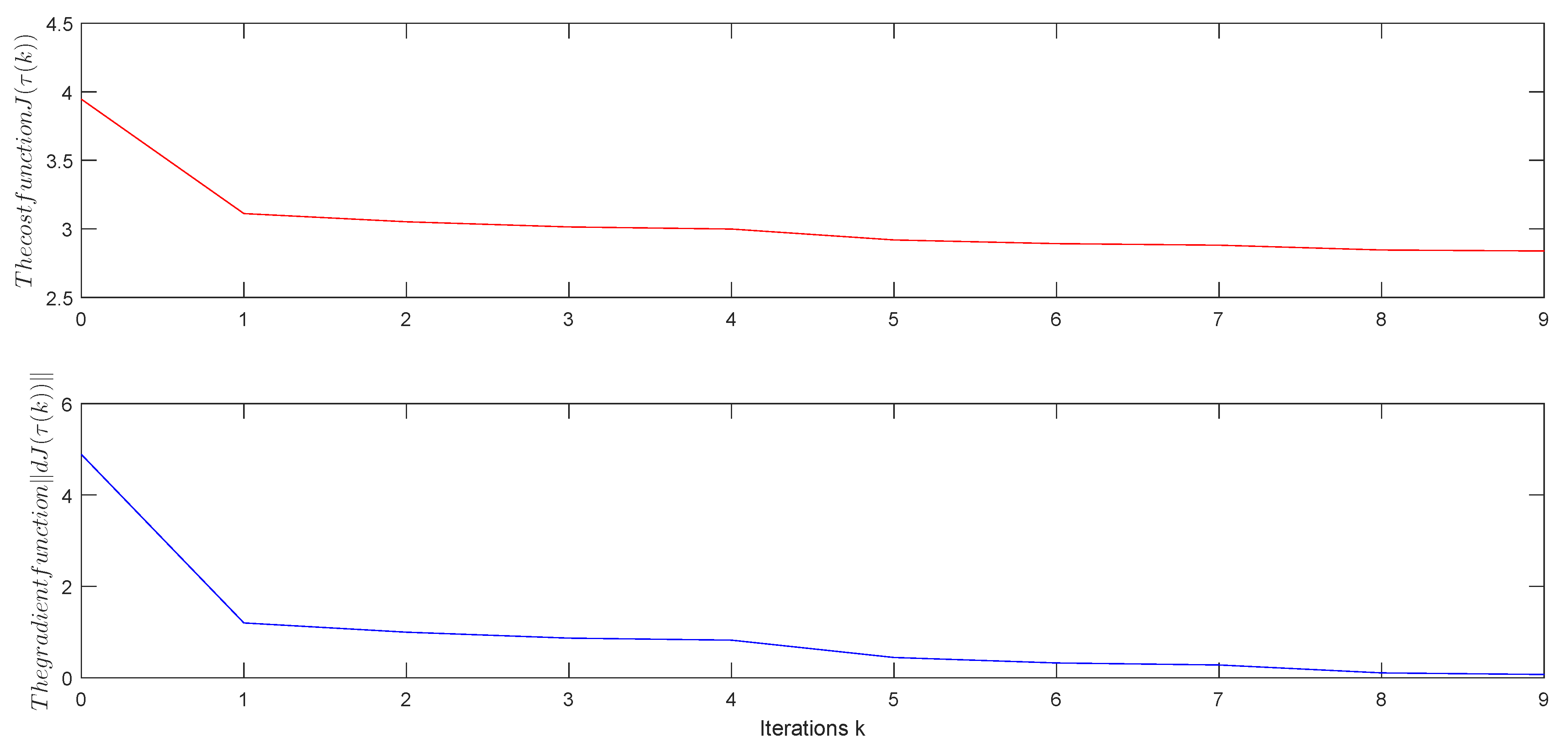
4.1. Practical Example
4.2. Numerical Example
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Geromel, J.C.; Colaneri, P. Stability and stabilization of discrete time switched systems. Int. J. Control 2006, 79, 719–728. [Google Scholar] [CrossRef]
- Wang, B.; Zhu, Q. Stability analysis of semi-Markov switched stochastic systems. Automatica 2018, 94, 72–80. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhu, Q. Stabilization by delay feedback control for highly nonlinear switched stochastic systems with time delays. Int. J. Robust Nonlinear Control 2021, 31, 3070–3089. [Google Scholar] [CrossRef]
- Fan, L.; Zhu, Q. Mean square exponential stability of discrete-time Markov switched stochastic neural networks with partially unstable subsystems and mixed delays. Inf. Sci. 2021, 580, 243–259. [Google Scholar] [CrossRef]
- Bilbao, J.; Bravo, E.; García, O.; Rebollar, C.; Varela, C. Optimising energy management in hybrid microgrids. Mathematics 2022, 10, 214. [Google Scholar] [CrossRef]
- Ozkan, L.; Kothare, M.V. Stability analysis of a multi-model predictive control algorithm with application to control of chemical reactors. J. Process. Control 2006, 16, 81–90. [Google Scholar] [CrossRef]
- Hamouda, M.; Menaem, A.A.; Rezk, H.; Ibrahim, M.N.; Számel, L. Comparative evaluation for an improved direct instantaneous torque control strategy of switched reluctance motor drives for electric vehicles. Mathematics 2021, 9, 302. [Google Scholar] [CrossRef]
- Gao, F.; Wu, Y.; Zhang, Z. Global fixed-time stabilization of switched nonlinear systems: A time-varying scaling transformation approach. IEEE Trans. Circuits Syst. II Express Briefs 2019, 66, 1890–1894. [Google Scholar] [CrossRef]
- Zhu, F.; Antsaklis, P.J. Optimal control of hybrid switched systems: A brief survey. Discret. Event Dyn. Syst. 2015, 25, 345–364. [Google Scholar] [CrossRef]
- Li, S.; Qiu, J.; Ji, H.; Zhu, K.; Li, J. Piezoelectric vibration control for all-clamped panel using DOB-based optimal control. Mechatronics 2011, 21, 1213–1221. [Google Scholar] [CrossRef]
- Wu, X.; Zhang, K.; Cheng, M. Optimal control of constrained switched systems and application to electrical vehicle energy management. Nonlinear Anal. Hybrid Syst. 2018, 30, 171–188. [Google Scholar] [CrossRef]
- Fu, J.; Zhang, C. Optimal control of path-constrained switched systems with guaranteed feasibility. IEEE Trans. Autom. Control 2021, 67, 1342–1355. [Google Scholar] [CrossRef]
- Bai, Q.; Zhu, W. Event-triggered impulsive optimal control for continuous-time dynamic systems with input time-delay. Mathematics 2022, 10, 279. [Google Scholar] [CrossRef]
- Raisch, J.; O’Young, S.D. Discrete approximation and supervisory control of continuous systems. IEEE Trans. Autom. Control 1998, 43, 569–573. [Google Scholar] [CrossRef]
- Dong, J.; Yang, G.H. Robust static output feedback control synthesis for linear continuous systems with polytopic uncertainties. Automatica 2013, 49, 1821–1829. [Google Scholar] [CrossRef]
- Liu, T.; Qu, X.; Tan, W. Online optimal control for wireless cooperative transmission by ambient RF powered sensors. IEEE Trans. Wirel. Commun. 2020, 19, 6007–6019. [Google Scholar] [CrossRef]
- Stellato, B.; Oberblobaum, S.; Goulart, P.J. Second-order switching time optimization for switched dynamical systems. IEEE Trans. Autom. Control. 2017, 62, 5407–5414. [Google Scholar] [CrossRef] [Green Version]
- Seatzu, C.; Corona, D.; Giua, A.; Bemporad, A. Optimal control of continuous-time switched affine systems. IEEE Trans. Autom. Control 2006, 51, 726–741. [Google Scholar] [CrossRef]
- Azimi, M.; Sharifi, G. A hybrid control scheme for attitude and vibration suppression of a flexible spacecraft using energy-based actuators switching mechanism. Aerosp. Sci. Technol. 2018, 82, 140–148. [Google Scholar] [CrossRef]
- Yan, B.; Shi, P.; Lim, C.C.; Wu, C.; Shi, Z. Optimally distributed formation control with obstacle avoidance for mixed–order multi-agent systems under switching topologies. IET Control Theory Appl. 2018, 12, 1853–1863. [Google Scholar] [CrossRef]
- Ding, K.; Zhu, Q. Extended dissipative anti-disturbance control for delayed switched singular semi-Markovian jump systems with multi-disturbance via disturbance observer. Automatica 2021, 128, 109556. [Google Scholar] [CrossRef]
- Wu, K.; Yu, J.; Sun, C. Global robust regulation control for a class of cascade nonlinear systems subject to external disturbance. Nonlinear Dyn. 2017, 90, 1209–1222. [Google Scholar] [CrossRef]
- Zhang, M.; Zhu, Q. Stability analysis for switched stochastic delayed systems under asynchronous switching: A relaxed switching signal. Int. J. Robust Nonlinear Control 2020, 30, 8278–8298. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, K.; Li, S.; Wei, H. Optimal timing control of discrete-time linear switched stochastic systems. Int. J. Control Autom. Syst. 2014, 12, 769–776. [Google Scholar] [CrossRef]
- Huang, R.; Zhang, J.; Lin, Z. Optimal control of discrete-time bilinear systems with applications to switched linear stochastic systems. Syst. Control. Lett. 2016, 94, 165–171. [Google Scholar] [CrossRef]
- Liu, X.; Li, S.; Zhang, K. Optimal control of switching time in switched stochastic systems with multi-switching times and different costs. Int. J. Control 2017, 90, 1604–1611. [Google Scholar] [CrossRef]
- Zhu, C.; Li, C.; Chen, X.; Zhang, K.; Xin, X.; Wei, H. Event-triggered adaptive fault tolerant control for a class of uncertain nonlinear systems. Entropy 2020, 22, 598. [Google Scholar] [CrossRef]
- Zhang, Y.; Jiang, J. Bibliographical review on reconfigurable fault-tolerant control systems. Annu. Rev. Control 2008, 32, 229–252. [Google Scholar] [CrossRef]
- Yu, X.; Jiang, J. A survey of fault-tolerant controllers based on safety-related issues. Annu. Rev. Control 2015, 39, 46–57. [Google Scholar] [CrossRef]
- Zhu, C.; Zhang, K.; Xin, X.; Gao, F.; Wei, H. Event-triggered adaptive fixed-time output feedback fault tolerant control for perturbed planar nonlinear systems. Int. J. Robust Nonlinear Control 2021, 31, 6934–6952. [Google Scholar] [CrossRef]
- Zhu, Q.; Cao, J. Exponential stability of stochastic neural networks with both Markovian jump parameters and mixed time delays. IEEE Trans. Syst. Man Cybern. Part B Cybern. 2010, 41, 341–353. [Google Scholar]
- Zhang, D.; Yu, L. Fault-tolerant control for discrete-time switched linear systems with time-varying delay and actuator saturation. J. Optim. Theory Appl. 2012, 153, 157–176. [Google Scholar] [CrossRef]
- Liu, J.; Zhang, K.; Sun, C.; Wei, H. Robust optimal control of switched autonomous systems. IMA J. Math. Control. Inf. 2016, 33, 173–189. [Google Scholar] [CrossRef]
- Guan, Y.; Yang, H.; Jiang, B. Fault-tolerant control for a class of switched parabolic systems. Nonlinear Anal. Hybrid Syst. 2019, 32, 214–227. [Google Scholar] [CrossRef]
- Peng, S.T. On one approach to constraining the combined wheel slip in the autonomous control of a 4ws4wd vehicle. IEEE Trans. Control. Syst. Technol. 2006, 15, 168–175. [Google Scholar] [CrossRef]
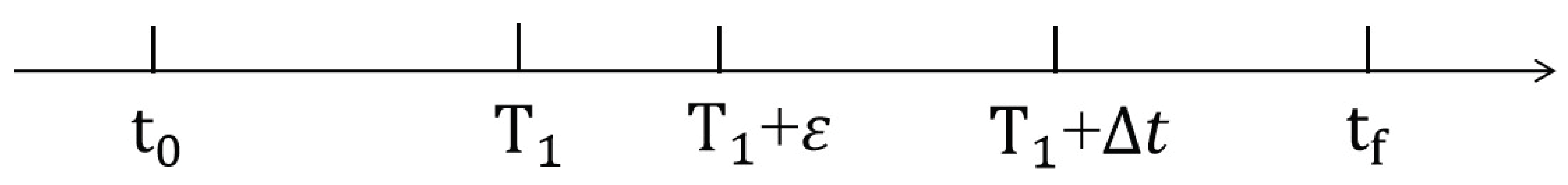



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, C.; He, L.; Zhang, K.; Sun, W.; He, Z. Optimal Timing Fault Tolerant Control for Switched Stochastic Systems with Switched Drift Fault. Mathematics 2022, 10, 1880. https://doi.org/10.3390/math10111880
Zhu C, He L, Zhang K, Sun W, He Z. Optimal Timing Fault Tolerant Control for Switched Stochastic Systems with Switched Drift Fault. Mathematics. 2022; 10(11):1880. https://doi.org/10.3390/math10111880
Chicago/Turabian StyleZhu, Chenglong, Li He, Kanjian Zhang, Wei Sun, and Zengxiang He. 2022. "Optimal Timing Fault Tolerant Control for Switched Stochastic Systems with Switched Drift Fault" Mathematics 10, no. 11: 1880. https://doi.org/10.3390/math10111880
APA StyleZhu, C., He, L., Zhang, K., Sun, W., & He, Z. (2022). Optimal Timing Fault Tolerant Control for Switched Stochastic Systems with Switched Drift Fault. Mathematics, 10(11), 1880. https://doi.org/10.3390/math10111880





