Problem-Based Learning and Applied Mathematics
Abstract
:1. Introduction
2. Problem-Based Learning in Higher Education and Mathematical Modeling
Principles of PBL Methodology
- The role of the tutor as a facilitator of learning;
- The responsibilities of students to be self-directed and self-regulated in their learning;
- The essential elements in the design of poorly structured learning problems as the driving force for research.
- Students must take responsibility for their own learning;
- Problem simulations used in PBL must be poorly structured and allow free interrogation;
- Learning must be integrated from a wide range of disciplines or subjects;
- Collaboration is essential;
- What students learn during their self-directed learning must be applied back to the problem with reanalysis and resolution;
- A final analysis of what has been learned from work with the problem and a discussion of what concepts and principles have been learned are essential;
- Self-assessment and peer review must be carried out at the end of each problem and at the end of each course unit;
- The activities carried out in PBL must be those that are conducive to value the ones in the real world;
- Students exams must measure student progress toward the PBL goals;
- PBL must be the pedagogical base of the curriculum and not be part of a didactic curriculum.
3. Materials and Methods
3.1. Modeling the Pedagogical Activity
- Q1.
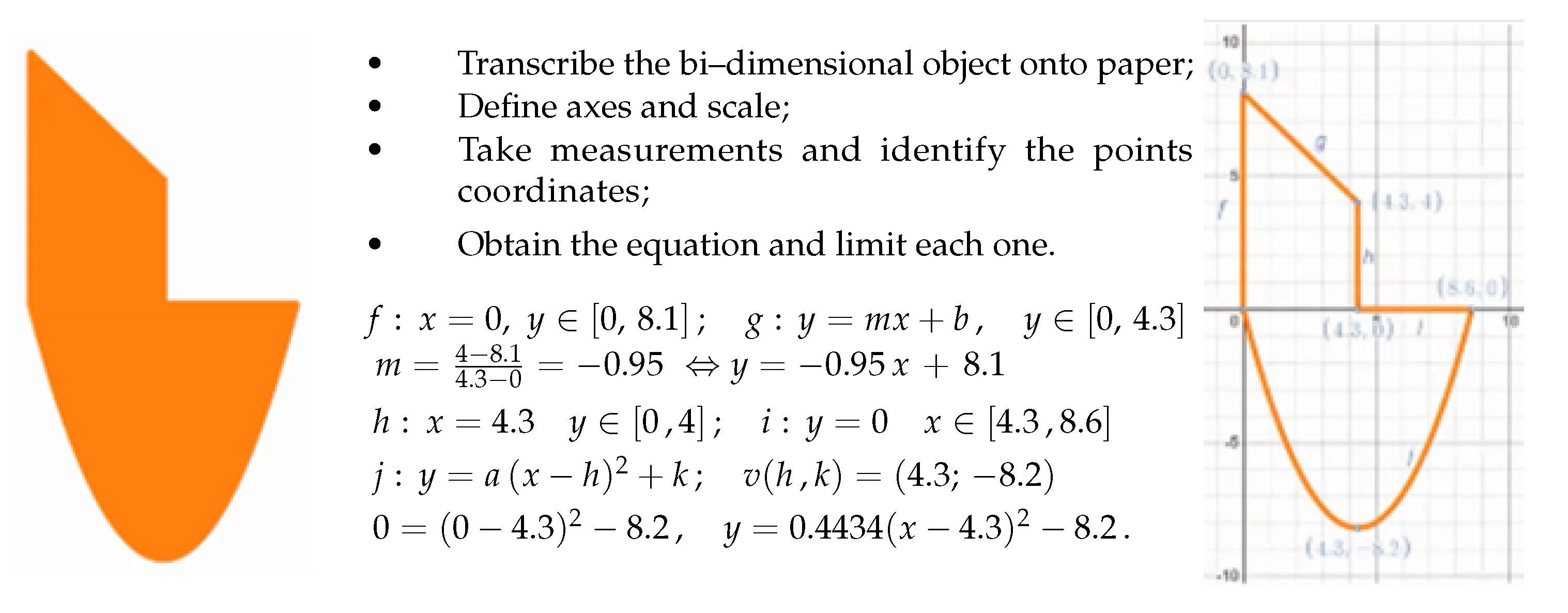
- How should the object be placed in a bi-dimensional frame?
- Q2.
- What are the algebraic expressions of the functions involved (at the boundary of the object)?
- Q3.
- What is the object’s surface area (in square centimeters’)?
- Q4.
- A variation on the proposed challenge.
- Q4.
- Note: Please keep in mind that you can use a ruler.
- Q1.
- What scales were used for the axes? Why?
- Q2.
- Was it obvious that by defining the figure’s boundary, the graphical representation of the functions and their analytic expressions were obtained?
- Q3.
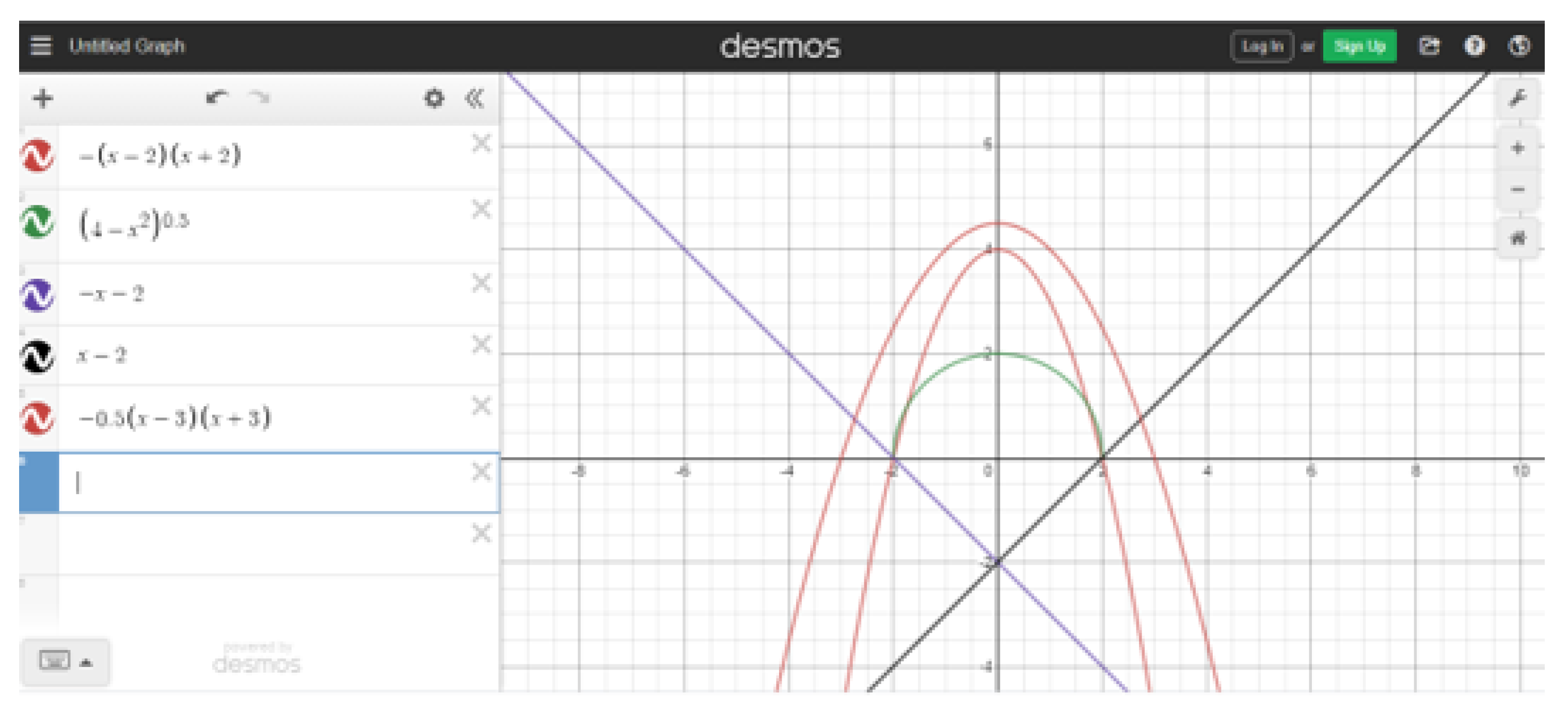
- Was Desmos used to confirm the existence of the desired functions?
- Q4.
- Was the area calculated directly or as the sum/difference of two areas? How did that come across?
- Q5.
- Are you certain that the functions chosen best approximate the desired surface boundary? Why?
- Q6.
- Did you mention that by moving the referential, the area obtained remains the same?
- Q7.
- What effect did the activity have on you? At least three items must be specified.
3.2. Research Methodology and Tools
4. Results
4.1. Answers to Questions of the Pedagogical Challenge
- Enable understanding of the values used in the calculation of algebraic expressions of boundary functions;
- The figure is divided in half;
- The OX axis divided the triangle’s semi-circumference (figures identified as being part of the object);
- The bi-dimensional object’s longest side was parallel to one of the axes;
- The parabola is identified as part of the boundary, with an upward concavity.
4.2. Answers to the Reflection Questions
5. Data Analysis and Discussion
6. Conclusions
- Determined the algebraic expressions for functions;
- Improved their ability to recognize and apply functions;
- Consolidated previously learned material to determine where more study time is needed;
- Learned how to decompose a figure and determine its various areas to improve critical thinking skills;
- Discovered how satellite images are used to calculate area in architecture;
- Determined the irregular areas of real-life objects;
- Recognized and used helpful available software;
- Solved a problem and use concepts learned in class.
- Felt a better understanding of the content and concepts after completing the challenge.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Barrows, H.S.; Tamblyn, R.M. Problem-Based Learning; Springer: New York, NY, USA, 1980. [Google Scholar]
- Gijbels, D.; Dochy, F.; Van den Bossche, P.; Segers, M. Effects of problem-based learning: A metaanalysis from the angle of assessment. Rev. Educ. Res. 2005, 75, 27–61. [Google Scholar] [CrossRef] [Green Version]
- Regehr, G.; Norman, G.R. Issues in cognitive psychology: Implications for professional education. Acad. Med. 1996, 71, 988–1001. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, H.G. Foundations of problem-based learning: Some explanatory notes. Med. Educ. 1993, 27, 422–432. [Google Scholar] [CrossRef] [PubMed]
- Derry, S.J.; Hmelo-Silver, C.E.; Nagarajan, A.; Chernobilsky, E.; Beitzel, B. Cognitive transfer revisited: Can we exploit new media to solve old problems on a large scale? J. Educ. Res. 2006, 35, 145–162. [Google Scholar] [CrossRef]
- Hmelo-Silver, C. Knowledge recycling: Crisscrossing the landscape of educational psychology in a problem-based learning course for pre-service teachers. J. Excell. Coll. Teach. 2000, 11, 41–56. [Google Scholar]
- McPhee, A. Problem-based learning in initial teacher education: Taking the agenda forward. J. Educ. Enq. 2002, 3, 60–78. [Google Scholar]
- Woods, D.R. Problem-based learning for large classes in chemical engineering. In Bringing Problem-Based Learning to Higher Education: Theory and Practice; Wilkerson, L., Gijselaers, W.H., Eds.; Jossey-Bass: San Francisco, CA, USA, 1996; pp. 91–99. [Google Scholar]
- Stinson, J.E.; Milter, R.G. Problem-based learning in business administration education: Curriculum design and implementation issues. In Bringing Problem-Based Learning to Higher Education: Theory and Practice; Wilkerson, L., Gijselaers, W.H., Eds.; Jossey-Bass: San Francisco, CA, USA, 1996; pp. 33–42. [Google Scholar]
- Walker, A.E.; Leary, H. A problem-based learning meta-analysis: Differences across problem types, implementation types, disciplines, and assessment levels. Interdiscip. J. Probl.-Based Learn. 2009, 3, 12–43. [Google Scholar] [CrossRef]
- Hmelo-Silver, C.E.; Derry, S.J.; Bitterman, A.H.; Hatrak, N. Targeting transfer in a STELLAR PBL course for pre-service teachers. Interdiscip. J. Probl.-Based Learn. 2009, 3, 24–42. [Google Scholar] [CrossRef]
- Goodnough, K. Issues in modified problem-based learning: A self-study in pre-service science teacher education. Can. J. Sci. Math. Technol. Educ. 2005, 5, 289–306. [Google Scholar] [CrossRef]
- Mursid, R.; Saragih, A.H.; Hartono, R. The effect of the blended project-based learning model and creative thinking ability on engineering students learning outcomes. Int. J. Educ. Math. Sci. Technol. 2022, 10, 218–235. [Google Scholar] [CrossRef]
- Piñeiro, J.L.; Chapman, O.; Castro-Rodríguez, E.; Castro, E. Prospective Elementary Teachers’ Pedagogical Knowledge for Mathematical Problem Solving. Mathematics 2021, 9, 1811. [Google Scholar] [CrossRef]
- Goti, A.; Akyazi, T.; de la Calle, A.; Oyarbide-Zubillaga, A.; Alberdi, E. Validation of Real Case Solving (RCS) Methodology as an Efficient Engineering Learning Tool. J. Open Innov. Technol. Mark. Complex. 2020, 6, 198. [Google Scholar] [CrossRef]
- Tang, S.; Long, M.; Tong, F.; Wang, Z.; Zhang, H.; Sutton-Jones, K.L. A Comparative Study of Problem-Based Learning and Traditional Approaches in College English Classrooms: Analyzing Pedagogical Behaviors Via Classroom Observation. Behav. Sci. 2020, 10, 105. [Google Scholar] [CrossRef]
- Piñero Charlo, J.C. Educational Escape Rooms as a Tool for Horizontal Mathematization: Learning Process Evidence. Educ. Sci. 2020, 10, 213. [Google Scholar] [CrossRef]
- Pinho, L.A.; Mota, F.B.; Conde, M.V.F.; Alves, L.A.; Lopes, R.M. Mapping Knowledge Produced on Problem-Based Learning between 1945 and 2014: A Bibliometric Analysis. Creat. Educ. 2015, 6, 576–584. [Google Scholar] [CrossRef] [Green Version]
- Nunes, S.; Oliveira, T.A.; Oliveira, A. Problem Based Learning—A Brief Review. In Proceedings of the International Conference on Numerical Analysis and Applied Mathematics (ICNAAM-2016), Rhodes, Greece, 19–25 September 2016; Simos, T., Tsitouras, C., Eds.; Volume 1863. [Google Scholar]
- Alves, A.; Moreira, F.; Fernandes, S.; Leão, C.P.; Sousa, R. PBL in the first year of an industrial engineering and management program: A journey of continuous improvement. In Proceedings of the PAEE/ALE’2017, 9th International Symposium on Project Approaches in Engineering Education (PAEE), University of Brasília, Brasília, Brazil, 11 December 2017; Lima, R.M., Aquere, A.L., Mello, J., Eds.; pp. 44–51. [Google Scholar]
- Duch, B.J.; Groh, S.E.; Allen, D.E. Why problem-based learning? A case study of institutional change in undergraduate education. In The Power of Problem-Based Learning; Duch, B., Groh, S., Allen, D., Eds.; Stylus: Sterling, VA, USA, 2001; pp. 3–11. [Google Scholar]
- Savery, J.R. Overview of Problem-Based Learning: Definitions and Distinctions. Interdiscip. J. Probl.-Based Learn. 2006, 1, 9–20. [Google Scholar] [CrossRef] [Green Version]
- Barrows, H.S. The Tutorial Process; Southern Illinois University School of Medicine: Springfield, MO, USA, 1988. [Google Scholar]
- Dooley, L.M. Case Study Research and Theory Building. Adv. Dev. Hum. Resour. 2002, 4, 335–354. [Google Scholar] [CrossRef]
- Darling-Hammond, L.; Flook, L.; Cook-Harvey, C.; Barron, B.; Osher, D. Implications for educational practice of the science of learning and development. Appl. Dev. Sci. 2020, 24, 97–140. [Google Scholar] [CrossRef] [Green Version]
- Mirzaei, F.; Phang, F.A.; Kashefi, H. Measuring Teachers Reflective Thinking Skills. Procedia-Soc. Behav. Sci. 2014, 141, 640–647. [Google Scholar] [CrossRef] [Green Version]
- Safarie, M.; Tarlani-aliabadi, H. Who Practices What? A Correlational Study of Personality Type and Teaching Reflection. Procedia-Soc. Behav. Sci. 2014, 98, 1606–1640. [Google Scholar] [CrossRef] [Green Version]
- Soisangwarn, A.; Wongwanich, S. Promoting the reflective teacher through peer coaching to improve teaching skills. Procedia-Soc. Behav. Sci. 2014, 116, 2504–2511. [Google Scholar] [CrossRef] [Green Version]
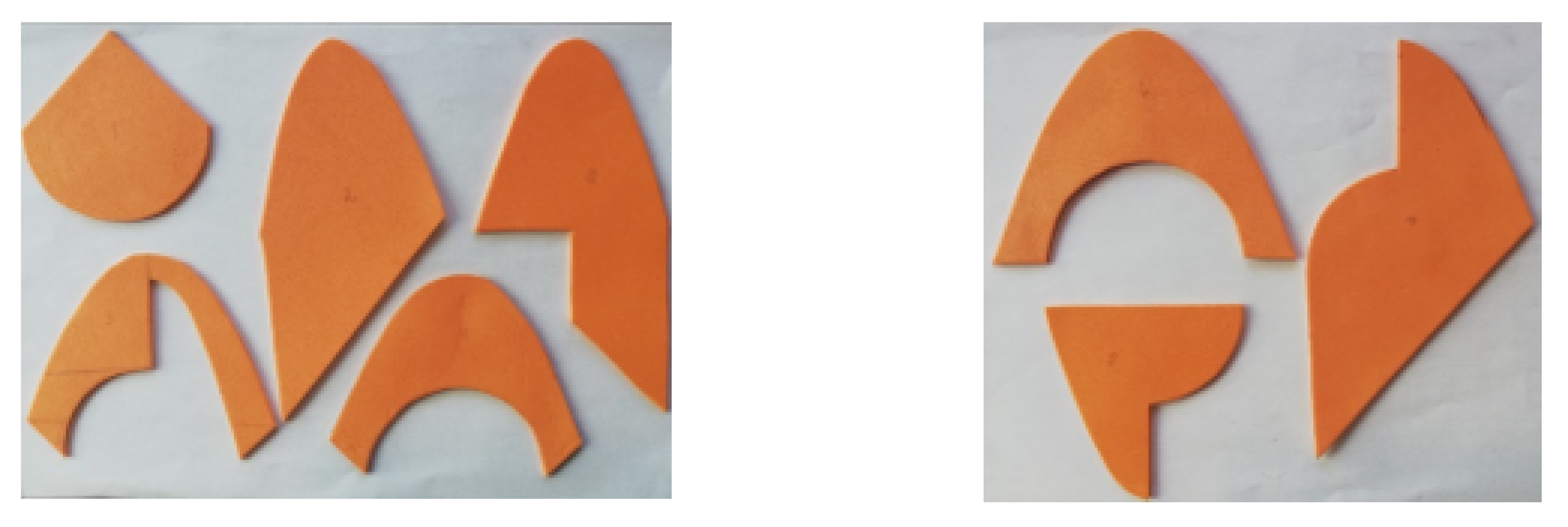

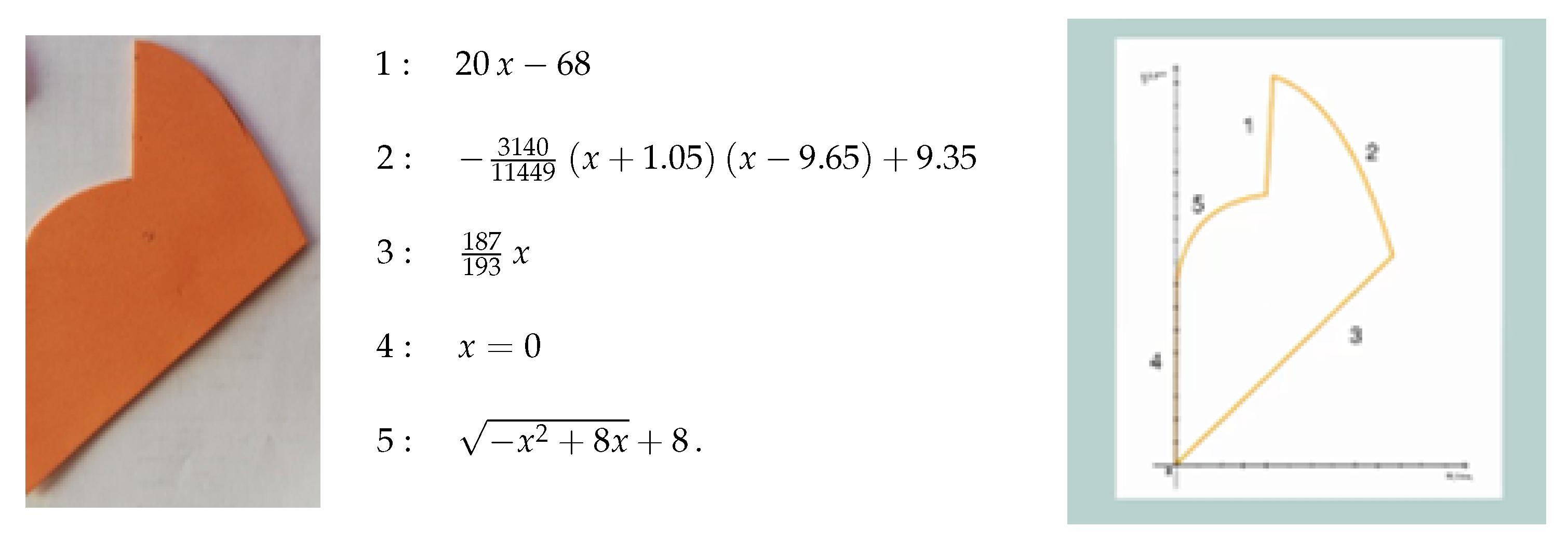
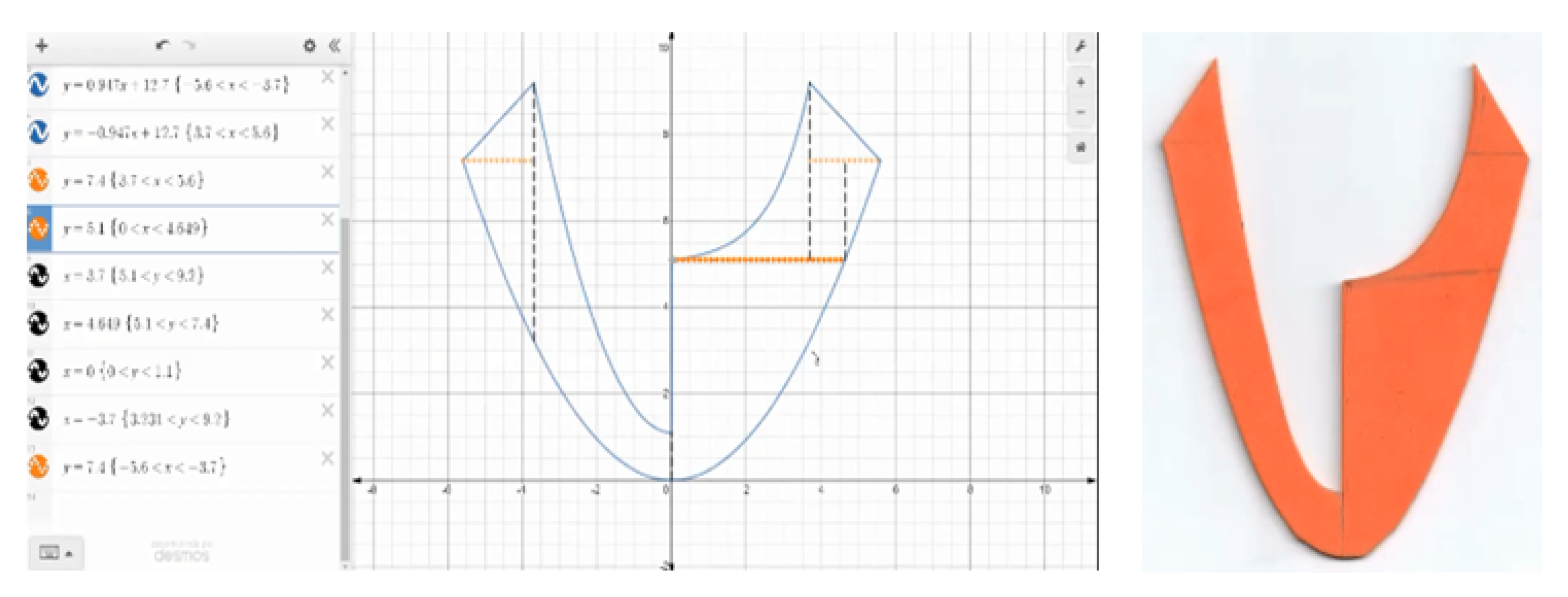
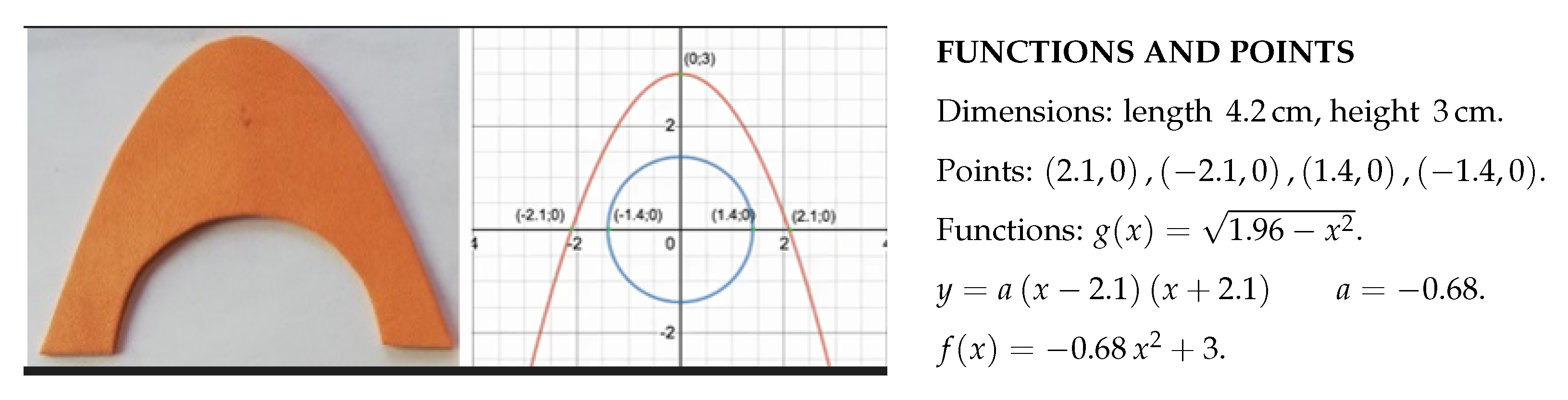




| Pedagogical Task | Curricular Contents (to Apply) | Scientific Knowledge (to Discover) | Skills (to Develop) |
|---|---|---|---|
| Pegagogical Challenge | Graphical representation of a function based on its algebraic expression. | The decision to place the piece in a bi-dimensional reference is critical for reducing/increasing the difficulty of algebraic function determination. | Retrieve the piece sketch, use graphical software (Desmos). |
| In Search of a Bi-Dimensional Geometric Model | Use integral calculus to calculate the area of a surface. | Option to model a surface’s boundary using algebraic expressions of functions. | Extract the numerical calculations, use integral calculus software (Symbolab). |
| It is worth noting that some quadratic functions have a graphical representation that is similar to that of a semi circumference. | |||
| Modeling involves some degree of inaccuracy. |
| . |
| Questions | Students Responses |
|---|---|
| Q1. What scales were used for the axes? Why? | After measuring the dimensions of the object with a ruler, scale in centimeters; Desmos uses a centimeter as a unit of measurement, so it is in square centimeters; Scale in centimeters after noticing that every two squares of a graded sheet measure one centimeter; In the frame, used a one-centimeter scale. |
| Q2. Was it obvious that by defining the figure’s boundary, the graphical representation of the functions and their analytic expressions were obtained? | Yes, some students said. Others responded no, because it was noticed after observing the piece’s position in the reference frame, not immediately. |
| Q3. Was Desmos used to confirm the existence of the desired functions? | Only a group of students had not made it. Desmos was mentioned as an important tool for work resolution in general. |
| Q4. Was the area calculated directly or as the sum/difference of two areas? How did that come across? | The piece position in the axis revealed that the simplest way to calculate area was to sum the areas; A difference in areas necessitated the removal of a portion of the function; A sum of areas, considering the piece’s decomposition as a triangle and a semi-circle; The area was calculated in a straightforward manner; As the sum and difference of two areas. The area of a quarter of a circle was calculated first, and then, it was subtracted from the area of a rectangle (not shown in the figure), and the two areas were added; It was calculated as the sum of areas because it was impossible to compute directly. |
| Q5. Are you certain that the functions chosen best approximate the desired surface boundary? Why? | Yes, in general, but there were difficulties determining the function part of the figure, which could be a parabola or a circumference. |
| Q6. Did you mention that by moving the referential, the area obtained remains the same? | With the exception of one group of students, everyone else answered positively. |
| Q7. What effect did the activity have on you? At least three items must be specified. | By participating in this activity, we were able to answer a persistent question in a practical way: how to calculate the areas of non-rectilinear and flat figures. It was difficult to find the expressions of the lines, but finding the expression of the parabola was even more difficult. It was an intriguing task; I discovered new methods for calculating area, improved my understanding of integrals and anti-derivatives, and became acquainted with useful free software; The ability to apply classroom knowledge to a problem was validated, the ability to recognize and work with functions improved, and this task allowed for better consolidation of the learned contents in order to identify where more time in study was required; Understanding how to decompose a figure and obtain the respective areas, application of area definition through integrals, and resolution of figure areas by integrals are all critical developments; Improved understanding of definite integrals, how to calculate irregular areas of real objects, and how to find function expressions; The discovery of another method for calculating areas aided in integral training; Improved understanding of class material and recognition of potential practical applications. |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rézio, S.; Andrade, M.P.; Teodoro, M.F. Problem-Based Learning and Applied Mathematics. Mathematics 2022, 10, 2862. https://doi.org/10.3390/math10162862
Rézio S, Andrade MP, Teodoro MF. Problem-Based Learning and Applied Mathematics. Mathematics. 2022; 10(16):2862. https://doi.org/10.3390/math10162862
Chicago/Turabian StyleRézio, Sofia, Marina Pedro Andrade, and Maria Filomena Teodoro. 2022. "Problem-Based Learning and Applied Mathematics" Mathematics 10, no. 16: 2862. https://doi.org/10.3390/math10162862
APA StyleRézio, S., Andrade, M. P., & Teodoro, M. F. (2022). Problem-Based Learning and Applied Mathematics. Mathematics, 10(16), 2862. https://doi.org/10.3390/math10162862








