Power-Law Nanofluid Flow over a Stretchable Surface Due to Gyrotactic Microorganisms
Abstract
:1. Introduction
2. Governing Equations
3. Numerical Solution and Code Validation
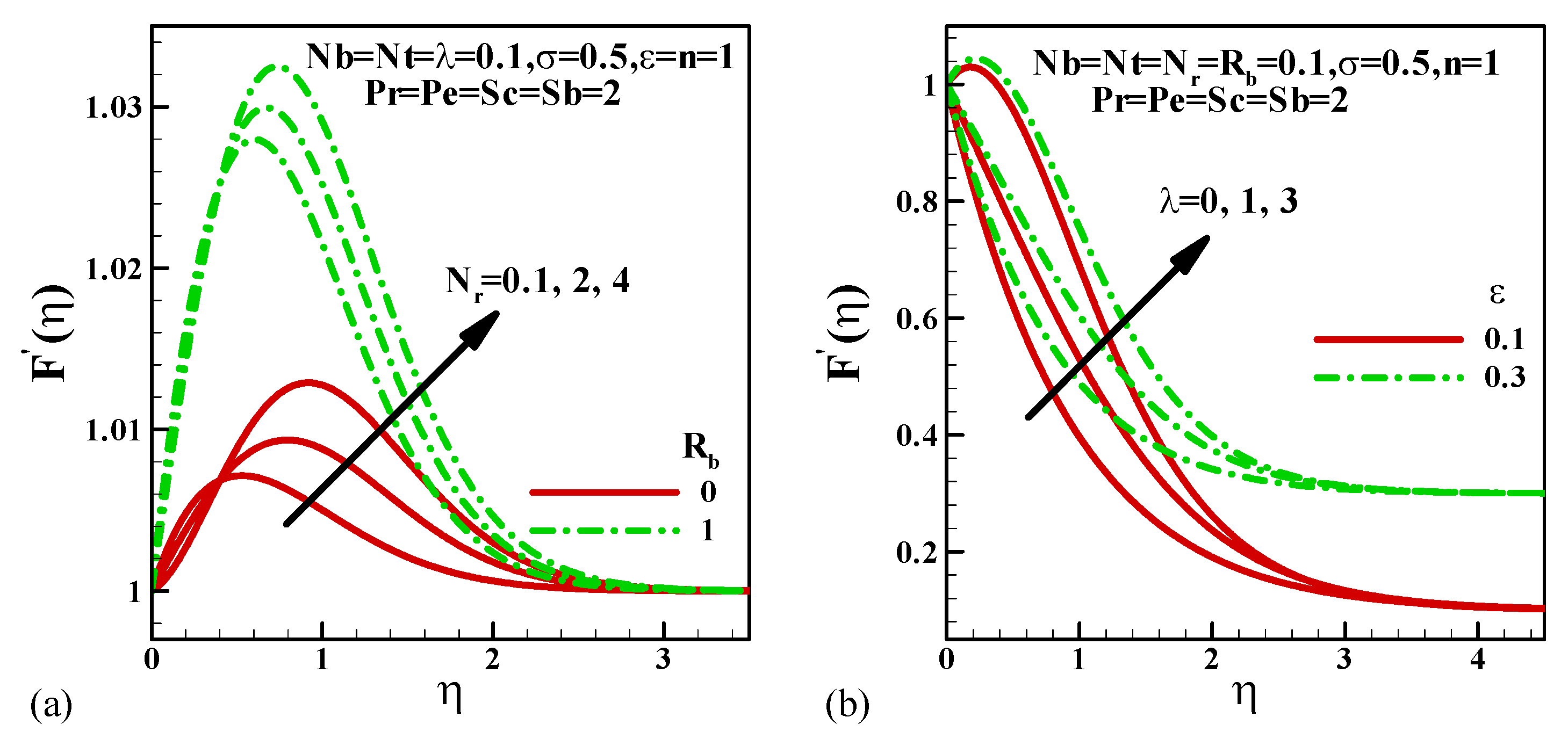
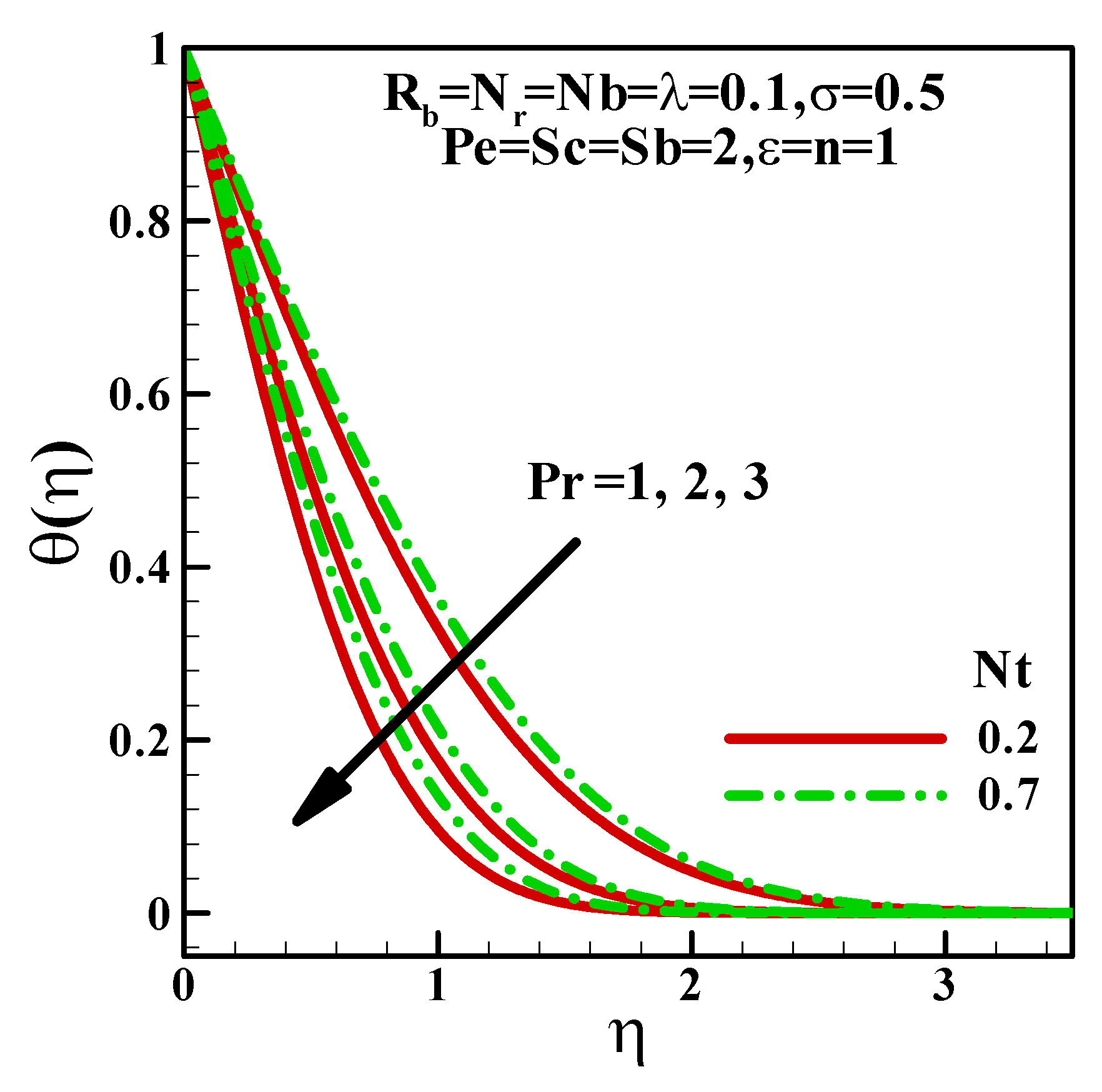
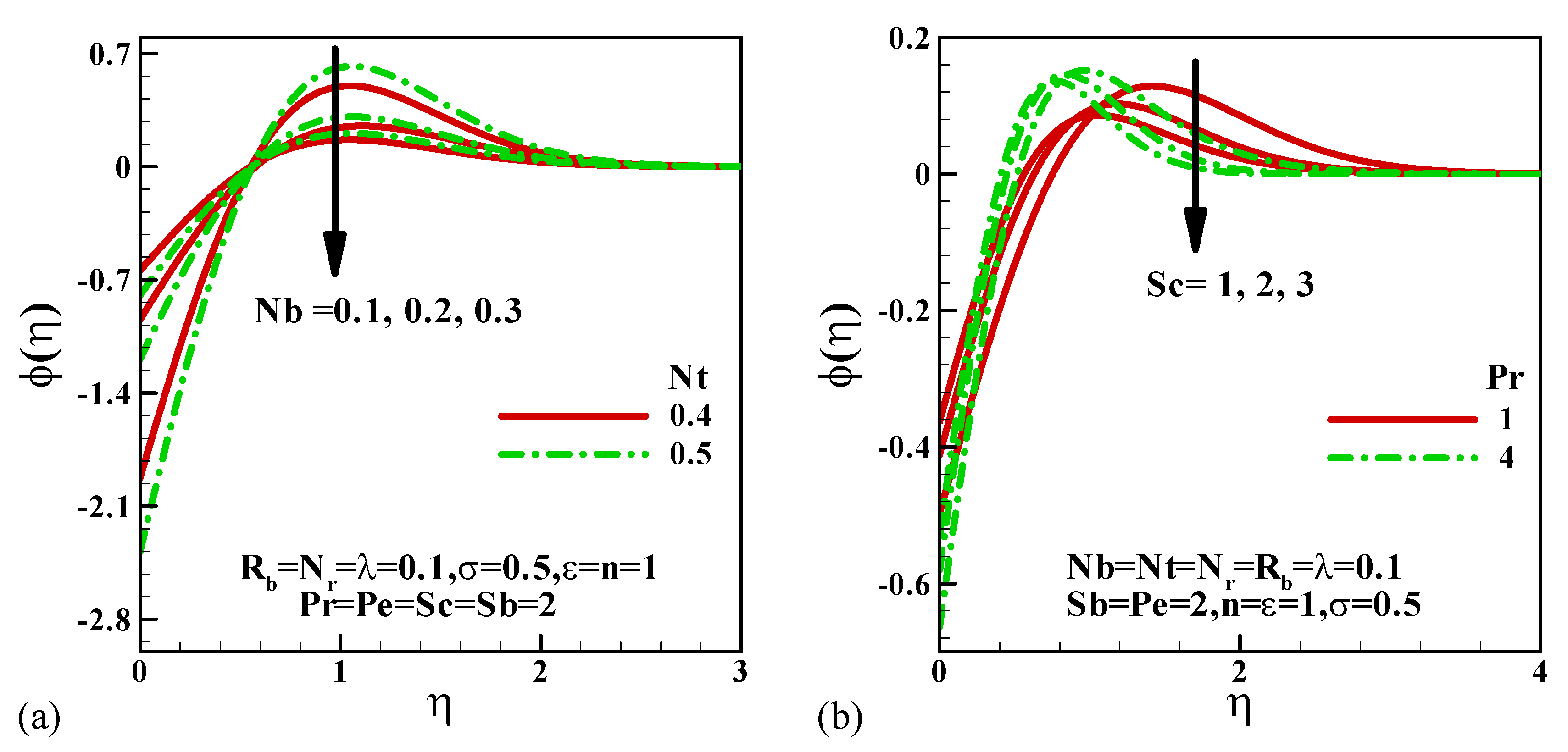
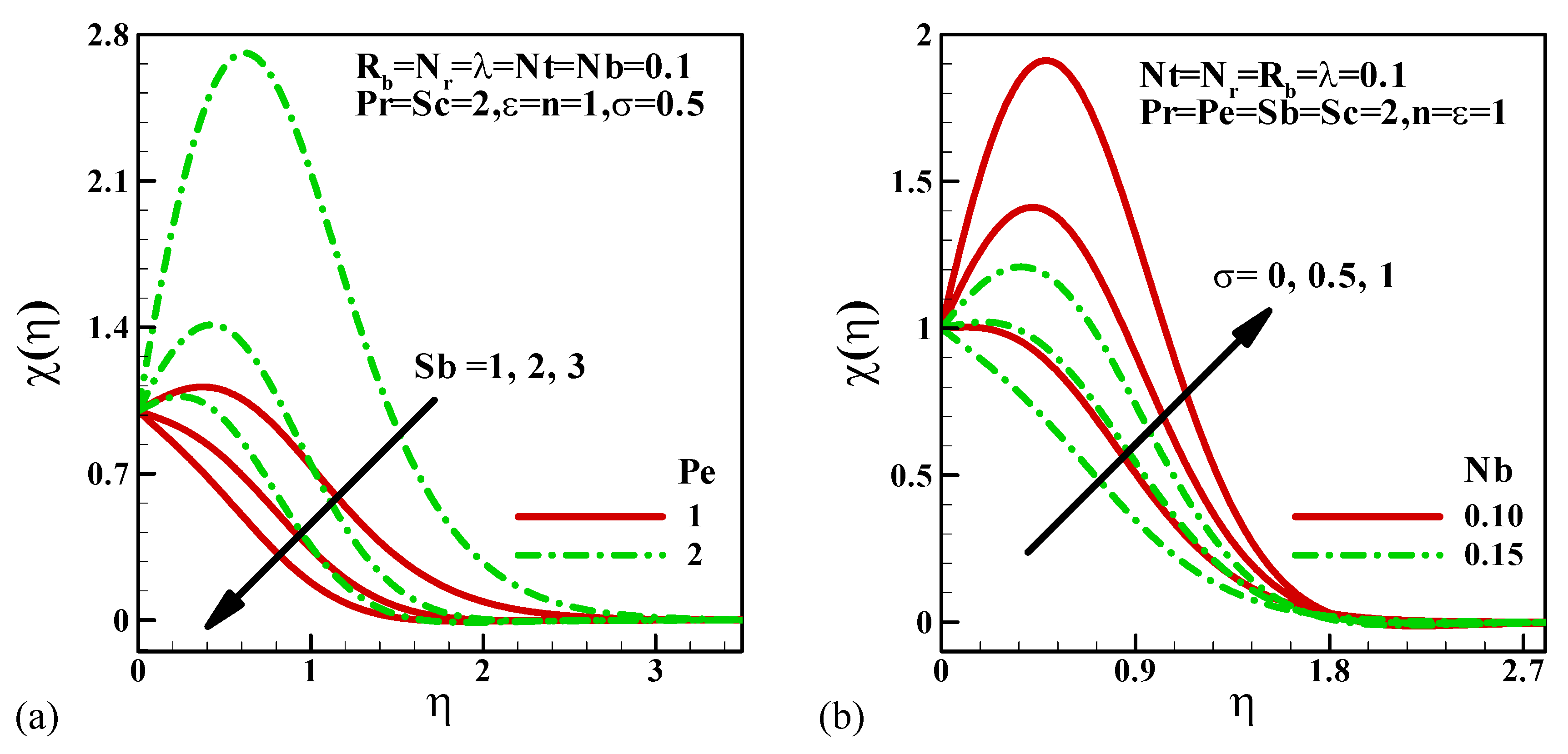
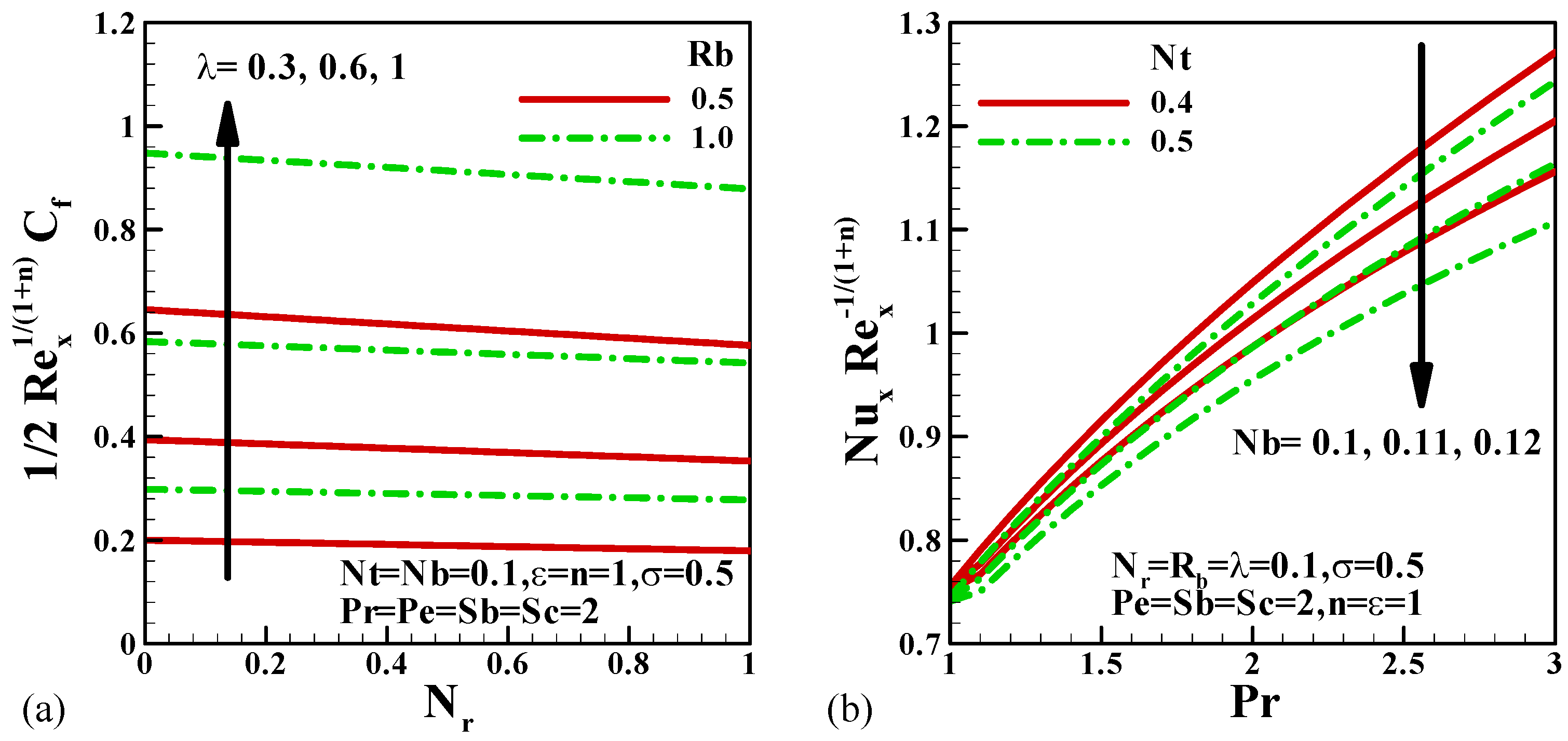
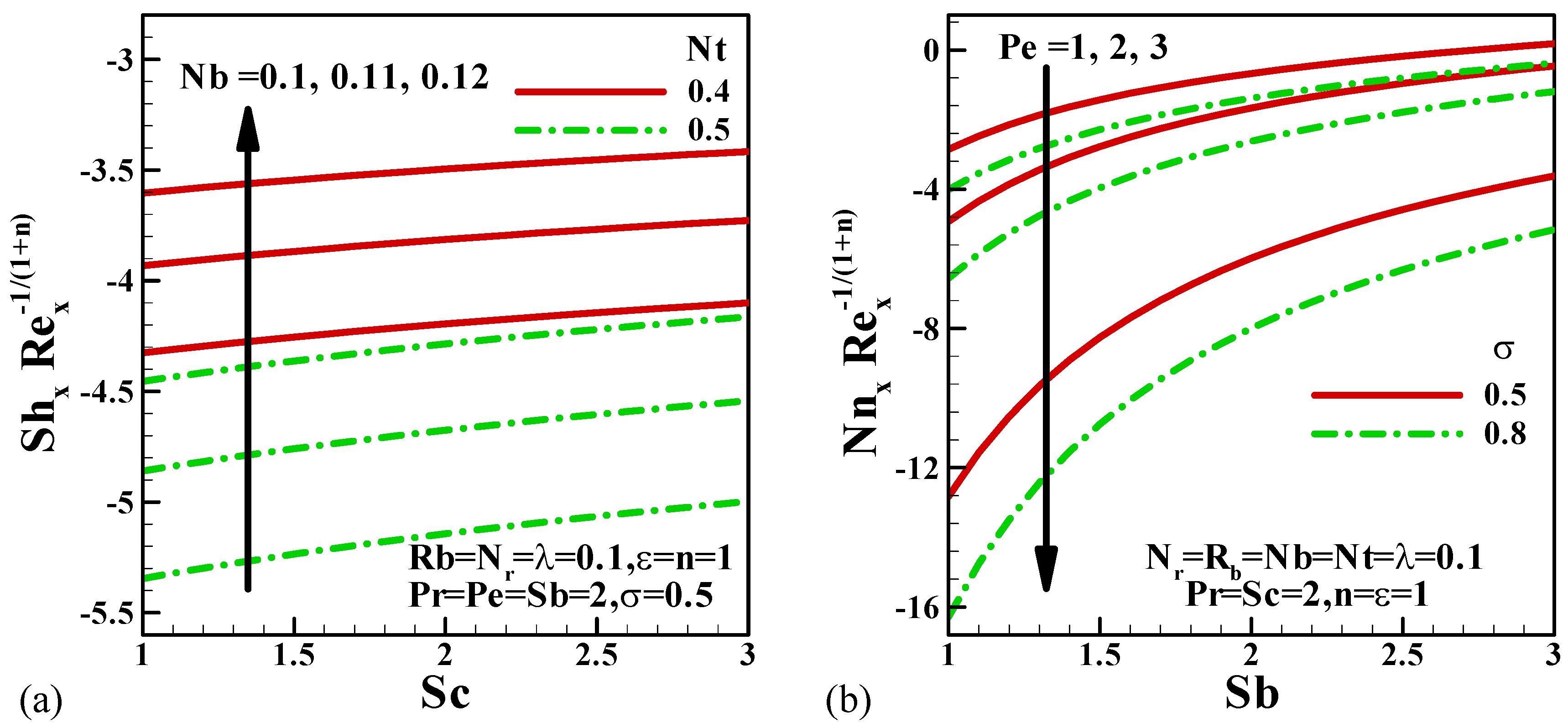
4. Results and Discussion
5. Conclusions
- Besides the bioconvection Schmidt number, all other parameters enhance the dimensionless velocity inside the boundary layer.
- Thermal boundary layer thickness decreases with the increasing Prandtl number.
- The nanofluid parameters generate non-uniform nanoparticle distribution throughout the domain.
- Dimensionless motile microbe density decreases as the bio-convection Schmidt number rises, but rises when the Peclet number falls.
- Skin friction is slightly reduced by the buoyancy parameter, but is slightly increased by the dimensionless mixed convection parameter and the bioconvection Rayleigh number.
- Nanofluid parameters reduce the Nusselt number, while the Prandtl number enhances it.
- Schmidt number and Brownian motion parameter both significantly boost the Sherwood number. Thermophoresis, on the other hand, tends to lower the Sherwood number.
- The bioconvection Peclet number and bioconvection constant contribute to slowing down the rate of mass transfer in motile microorganisms.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| a and c | positive constants |
| C | concentration |
| Cf | local skin-friction coefficient |
| Dn | diffusivity of the microorganisms |
| DB | Brownian diffusion coefficient |
| DT | thermophoretic diffusion coefficient of the microorganisms |
| F′ | dimensionless velocity |
| g | gravitational acceleration |
| Grx | local Grashof number |
| Sb | bioconvection Schmidt number |
| Sc | Schmidt number |
| K | consistency coefficient |
| kf | thermal conductivity |
| N | density of motile microorganisms |
| n | power-law fluid |
| Nb | Brownian motion number |
| Nr | buoyancy ratio parameter |
| Nt | thermophoresis number |
| Nux | Nusselt number |
| Nnx | density number |
| Pe | bioconvectionPéclet number |
| Pr | Prandtl number |
| q | wall heat flux |
| Rb | bioconvection Rayleigh number |
| Rex | local Reynolds number |
| T | temperature |
| u and v | dimensionless velocity component in the x-direction |
| Uw(x) | stretching velocity |
| U∞(x) | free stream velocity |
| wc | maximum cell swimming speed |
| x | streamwise coordinate |
| y | transverse coordinate |
| Greek Symbols | |
| α | thermal diffusivity |
| β | coefficient of thermal expansion |
| γ | average volume of a microorganism |
| σ | bioconvection constant |
| η | pseudo-similarity variable |
| θ | dimensionless temperature |
| φ | nanoparticle volume fraction |
| ψ | non-dimensional stream function |
| λ | mixed convection parameter |
| ratio of velocity parameter | |
| χ | dimensionless density of motile microorganisms |
| μ | dynamic viscosity |
| ν | kinematic viscosity |
| ρf | density of the fluid |
| ρf∞ | density of the base fluid |
| ρp | density of the particles |
| ρm∞ | density of the microorganism |
| (ρc)f | heat capacity of the fluid |
| (ρc)p | effective heat capacity of the nanoparticle material |
| ρ | density |
| Subscripts | |
| w | condition at the wall |
| ∞ | condition at infinity |
References
- Choi, S.U.S. Enhancing thermal conductivity of fluids with nanoparticles. In Proceedings of the 1995 ASME International Mechanical Engineering Congress and Exposition, San Francisco, CA, USA, 12–17 November 1995; pp. 99–105. [Google Scholar]
- Das, K.; Putra, N.; Thiesen, P.; Roetzel, W. Temperature dependence of thermal conductivity enhancement for nanofluid. J. Heat Transf. 2003, 125, 567–574. [Google Scholar] [CrossRef]
- Gorla, R.S.R.; El-Kabeir, S.M.M.; Rashad, A.M. Heat transfer in the boundary layer on a stretching circular cylinder in a nanofluid. J. Thermophys. Heat Transf. 2011, 25, 183–186. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Rashad, A.M. Natural convection from a vertical permeable cone in nanofluid saturated porous media for uniform heat and nanoparticles volume fraction fluxes. Int. J. Numer. Methods Heat Fluid Flow 2012, 22, 1073–1085. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Rashad, M.; Gorla, R.S.R. Non-Similar Solutions for Mixed Convection along with a Wedge Embedded in a Porous Medium Saturated by a Non-Newtonian Nanofluid: Natural Convection Dominated Regime. Int. J. Numer. Methods Heat Fluid Flow 2014, 24, 1471–1786. [Google Scholar] [CrossRef]
- Buongiorno, J. Convective transport in nanofluids. ASME J. Heat Transf. 2006, 128, 240–250. [Google Scholar] [CrossRef]
- Khan, W.A.; Rashad, A.M.; Abdou, M.M.M.; Tlili, I. Natural Bioconvection Flow of A Nanofluid Containing Gyrotactic Microorganisms About A Truncated Cone. Eur. J. Mech./B Fluids 2019, 75, 133–142. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Nabwey, H.A.; Abdelrahman, Z.M.A.; Rashad, A.M. Mixed Bioconvective Flow Over a Wedge in Porous Media Drenched with a Nanofluid. J. Nanofluids 2020, 9, 24–35. [Google Scholar] [CrossRef]
- Nabwey, H.A.; El-Kabeir, S.M.M.; Rashad, A.M.; Abdou, M.M.M. Viscous Dissipation and Joule Heating Effects on MHD Bioconvection Flow of a Nanofluid Containing Gyrotactic Microorganisms over a Vertical Isothermal Cone. J. Nanofluids 2020, 9, 242–255. [Google Scholar] [CrossRef]
- Nabwey, H.A.; El-Kabeir, S.M.M.; Rashad, A.M.; Abdou, M.M.M. Gyrotactic Microorganisms Mixed Convection Flow of Nanofluid over a Vertically Surfaced Saturated Porous Media. Alex. Eng. J. 2021, 61, 1804–1822. [Google Scholar] [CrossRef]
- Irvine, T.F.; Karni, J. Non-Newtonian fluid flow and heat transfer. In Handbook of Single-Phase Convective Heat Transfer; Kakac, S., Shah, R.K., Aung, W., Eds.; Wiley: New York, NY, USA, 1987; pp. 20.1–20.57. [Google Scholar]
- Schowalter, W.R. The application of boundary-layer theory to power-law pseudoplastic fluids: Similar solutions. AICHE J. 1960, 6, 24–28. [Google Scholar] [CrossRef]
- Acrivos, A.; Shah, M.J.; Petersen, E.E. Momentum and heat transfer in laminar boundary layer flows of non-Newtonian fluids past external surfaces. AICHE J. 1960, 6, 312–318. [Google Scholar] [CrossRef]
- Rashad, A.M.; EL-Hakiem, M.A.; Abdou, M.M.M. Natural convection boundary layer of a non-Newtonian fluid about a permeable vertical cone embedded in a porous medium saturated with a nanofluid. Comput. Math. Appl. 2011, 62, 3140–3151. [Google Scholar] [CrossRef]
- Rashad, A.M.; Chamkha, A.J.; Abdou, M.M.M. Mixed Convection Flow Of Non-Newtonian Fluid From Vertical Surface Saturated In A Porous Medium Filled with A Nanofluid. J. Appl. Fluid Mech. 2013, 6, 301–309. [Google Scholar]
- Chamkha, A.J.; Abbasbandy, S.; Rashad, A.M. Non-Darcy Natural Convection Flow of a Non-Newtonian Nanofluid over a Cone Saturated in a Porous Medium with Uniform Heat and Volume Fraction Fluxes. Int. J. Numer. Methods Heat Fluid Flow 2015, 25, 422–437. [Google Scholar] [CrossRef]
- Nabwey, H.A.; Boumazgour, M.; Rashad, A.M. Group Method Analysis of Mixed Convection Stagnation-Point Flow Of Non-Newtonian Nanofluid over a Vertical Stretching Surface. Indian J. Phys. 2017, 91, 731–742. [Google Scholar] [CrossRef]
- Platt, J.R. Bioconvection Patterns in Cultures of Free-Swimming Organisms. Science 1961, 133, 1766–1767. [Google Scholar] [CrossRef]
- Kuznetsov, A.V.; Avramenko, A.A. Effect of small particles on the stability of bioconvection in a suspension of gyrotactic microorganisms in a layer of finite depth. Int. Commun. Heat Mass Transf. 2004, 31, 1–10. [Google Scholar] [CrossRef]
- Kuznetsov, A.V. The onset of nanofluidbioconvection in a suspension containing both nanoparticles and gyrotactic microorganisms. Int. Commun. Heat Mass Transf. 2010, 37, 1421–1425. [Google Scholar] [CrossRef]
- Waqas, H.; Khan, S.U.; Hassan, M.; Bhatti, M.M.; Imran, M. Analysis of the bioconvection flow of modified second-grade nanofluid containing gyrotactic microorganisms and nanoparticles. J. Mol. Liq. 2019, 291, 111231. [Google Scholar] [CrossRef]
- Khan, W.A.; Uddin, M.J.; Ismail, A.I. Free convection of non-Newtonian nanofluids in porous media with gyrotactic microorganisms. Transp. Porous Media 2013, 97, 241–252. [Google Scholar] [CrossRef]
- Khan, W.A.; Rashad, A.M. Combined effects of radiation and chemical reaction on heat and mass transfer by MHD stagnation-point flow of a Micropolar fluid towards a stretching surface. J. Niger. Math. Soc. 2017, 36, 219–238. [Google Scholar]
- Beg, O.A.; Uddin, M.J.; Khan, W.A. Bioconvective Non-Newtonian Nanofluid Transport in Porous Media Containing Micro-Organisms in a Moving Free Stream. J. Mech. Med. Biol. 2015, 15, 5. [Google Scholar] [CrossRef]
- Ishak, A.; Nazar, R.; Pop, I. Mixed convection boundary layers in the stagnation point flow toward a stretching vertical sheet. Meccanica 2006, 41, 509–518. [Google Scholar] [CrossRef]
- Kuznetsov, A.V.; Nield, D.A. Natural convective boundary-layer flow of a nanofluid past a vertical plate: A revised model. Int. J. Therm. Sci. 2014, 77, 126–129. [Google Scholar] [CrossRef]
- Mahapatra, T.R.; Nandy, S.K.; Gupta, A.S. Magnetohydrodynamic stagnation-point flow of a power-law fluid towards a stretching surface. Int. J. Non-Linear Mech. 2009, 44, 123–128. [Google Scholar] [CrossRef]
- Khan, M.; Malik, R.; Munir, A.; Khan, W.A. Flow and heat transfer to Siskonanofluid over a nonlinear stretching sheet. PLoS ONE 2015, 10, e0125683. [Google Scholar]


| Ishak et al. [10] | Khan and Rashad [25] | Present Results | |
|---|---|---|---|
| 0.1 | −0.9694 | −0.96939 | −0.969386154 |
| 0.2 | −0.9181 | −0.91811 | −0.918107089 |
| 0.5 | −0.6673 | −0.66726 | −0.667263673 |
| 2 | 2.0175 | 2.017503 | 2.0175028007 |
| 3 | 4.7294 | 4.729282 | 11.751990603 |
| Pr | Wang [23] | Gorla and Sidawi [24] | Khan and Pop [8] | Present Results |
|---|---|---|---|---|
| 0.07 | 0.0656 | 0.0656 | 0.0663 | 0.0659 |
| 0.2 | 0.1691 | 0.1691 | 0.1691 | 0.1690 |
| 0.7 | 0.4539 | 0.5349 | 0.4539 | 0.4539 |
| 2.0 | 0.9113 | 0.9113 | 0.9113 | 0.9113 |
| 7.0 | 1.8954 | 1.8905 | 1.8954 | 1.8954 |
| 20.0 | 3.3539 | 3.3539 | 3.3539 | 3.3539 |
| 70.0 | 6.4622 | 6.4622 | 6.4621 | 6.4622 |
| n | Mahapatra et al. [27] | Present Results | Mahapatra et al. [27] | Present Results |
|---|---|---|---|---|
| ε = 1.1 | ε = 1.5 | |||
| 0.4 | 0.1035 | 0.1043 | 1.2019 | 1.2020 |
| 0.6 | 0.1193 | 0.1238 | 0.1691 | 1.0170 |
| 0.8 | 0.1407 | 0.14210 | 0.9434 | 0.9431 |
| 1.0 | 0.1643 | 0.16412 | 0.9095 | 0.9089 |
| 1.2 | 0.1888 | 0.18871 | 0.8937 | 0.8932 |
| 1.5 | 0.2257 | 0.22504 | 0.8853 | 0.8840 |
| Khan et al. [28] | Present Results | |
|---|---|---|
| 0.7 | 0.4539 | 0.45445 |
| 2 | 0.9113 | −0.91135 |
| 7 | 1.8954 | −1.89540 |
| 20 | 3.3539 | −3.35391 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nabwey, H.A.; A. Khan, W.; Rashad, A.M.; Mabood, F.; Salah, T. Power-Law Nanofluid Flow over a Stretchable Surface Due to Gyrotactic Microorganisms. Mathematics 2022, 10, 3285. https://doi.org/10.3390/math10183285
Nabwey HA, A. Khan W, Rashad AM, Mabood F, Salah T. Power-Law Nanofluid Flow over a Stretchable Surface Due to Gyrotactic Microorganisms. Mathematics. 2022; 10(18):3285. https://doi.org/10.3390/math10183285
Chicago/Turabian StyleNabwey, Hossam A., Waqar A. Khan, A. M. Rashad, Fazal Mabood, and Taha Salah. 2022. "Power-Law Nanofluid Flow over a Stretchable Surface Due to Gyrotactic Microorganisms" Mathematics 10, no. 18: 3285. https://doi.org/10.3390/math10183285
APA StyleNabwey, H. A., A. Khan, W., Rashad, A. M., Mabood, F., & Salah, T. (2022). Power-Law Nanofluid Flow over a Stretchable Surface Due to Gyrotactic Microorganisms. Mathematics, 10(18), 3285. https://doi.org/10.3390/math10183285








