Analysis of Tutoring in the Professional Development of STEM Teachers
Abstract
:1. Introduction
2. Literature Review
3. Conceptual Framework
4. Learning Design
5. Materials and Methods
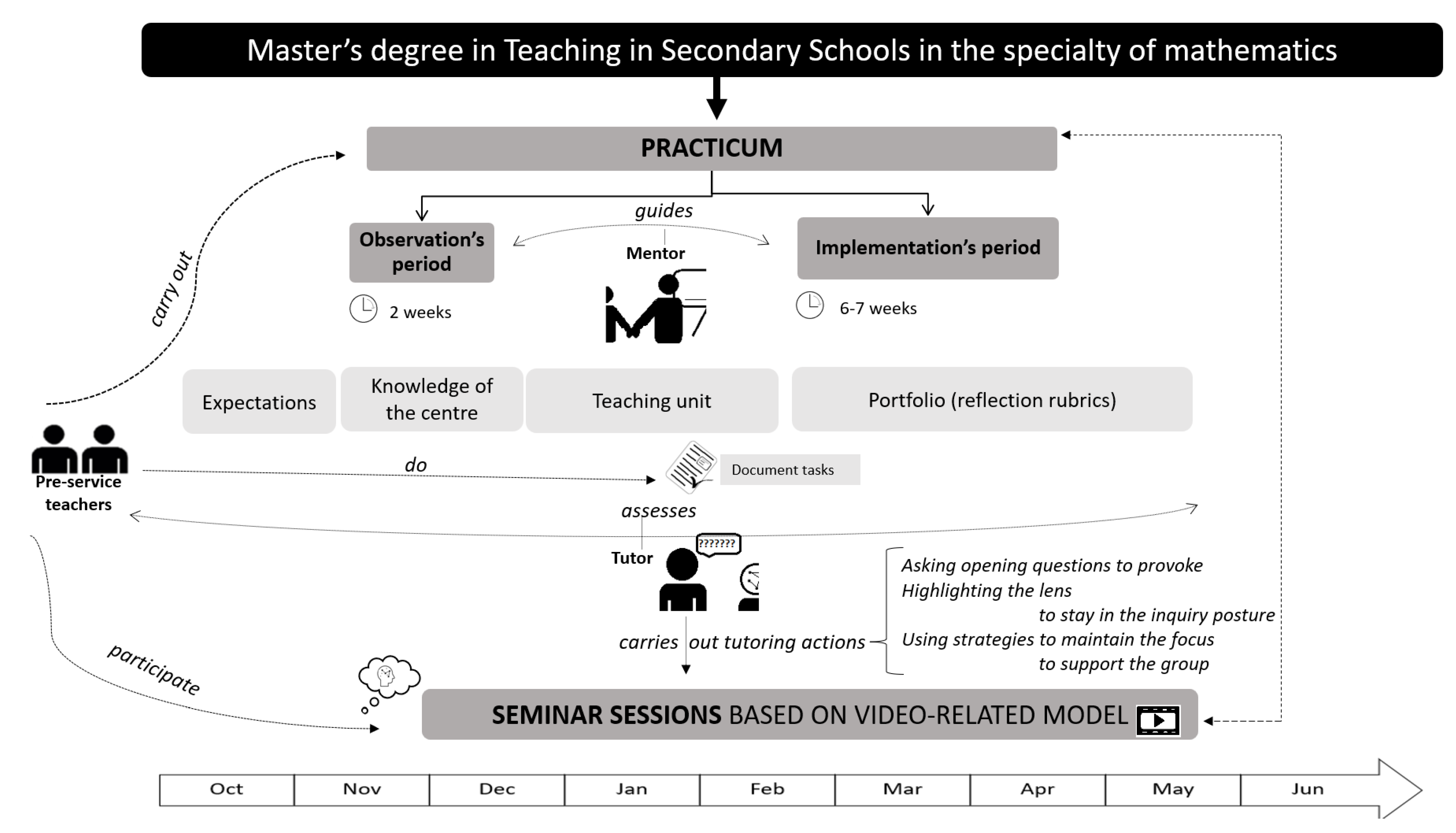
5.1. Context and Participants
5.2. Design, Data Collection, and Analysis
- Defining units of analysis of the transcripts: the interventions of future teachers were segmented according to each moment selected to comment.
- Coding the interventions segmented according to the five lenses.
6. Results
6.1. Changes in the Content and Form of the Participants
6.2. Changes in the Speech and Language of the Participants
6.3. Account off Narrative
6.4. Account for Narrative
6.5. Self-Regulation and Metacognition
6.6. Student’s Mathematical Thinking
6.7. Tasks and Mathematical Content
6.8. Role of the Teacher
6.9. Classroom Discourse
7. Discussions
8. Conclusions
9. Limitations and Discussing the Future Work
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mason, D. Researching Your Own Practice: The Discipline of Noticing, 6th ed.; Routledge: London, UK, 2002. [Google Scholar]
- Santagata, R.; Guarino, J. Using video to teach future teachers to learn from teaching. ZDM Math. Educ. 2011, 43, 133–145. [Google Scholar] [CrossRef]
- Santagata, R.; Yeh, C. Learning to teach mathematics and to analyze teaching effectiveness: Evidence from video- and practice- based approach. J. Math. Teach. Educ. 2014, 17, 491–514. [Google Scholar] [CrossRef]
- Van Es, E.A.; Sherin, M.G. Mathematics teachers learning to notice in the context of a video club. Teach. Teach. Educ. 2008, 24, 244–276. [Google Scholar] [CrossRef]
- Gaudin, C.; Chaliès, S. Video viewing in teacher education and professional development: A literature review. Educ. Res. Rev. 2015, 16, 41–67. [Google Scholar] [CrossRef]
- Karsenty, R.; Arcavi, A. Mathematics, lenses, and videotapes: A framework and a language for developing reflective practices of teaching. J. Math. Teach. Educ. 2017, 20, 433–455. [Google Scholar] [CrossRef]
- Wang, J.; Hartley, K. Video Technology as a Support for Teacher Education Reform. J. Tech. Teach. Educ. 2003, 11, 105–138. [Google Scholar]
- Sherin, M.G.; van Es, E.A. Effects of Video Club Participation on Teachers’ Professional Vision. J. Teach. Educ. 2009, 60, 20–37. [Google Scholar] [CrossRef]
- Nemirovsky, R.; Galvis, A. Facilitating Grounded Online Interactions in Video-Case-Based Teacher Professional Development. J. Sci. Educ. Tech. 2004, 13, 67–79. [Google Scholar] [CrossRef]
- Ding, L.; Domínguez, H. Opportunities to notice: Chinese prospective teachers noticing students’ ideas in a distance formula lesson. J. Math. Teach. Educ. 2016, 19, 325–347. [Google Scholar] [CrossRef]
- Kleinknecht, M.; Schneider, J. What do teachers think and feel when analyzing videos of themselves and other teachers teaching? Teach. Teach. Educ. 2013, 33, 13–23. [Google Scholar] [CrossRef]
- Seidel, T.; Stürmer, K.; Blomberg, G.; Kobarg, M.; Schwindt, K. Teacher learning from analysis of videotaped classroom situations: Dies it make a difference whether teachers observe their own teaching or that of others? Teach. Teach. Educ. 2011, 27, 259–267. [Google Scholar] [CrossRef]
- Zhang, M.; Lundeberg, M.; Eberhardt, J. Strategic facilitation of problem-based discussion for teacher professional development. J. Learn. Sci. 2011, 20, 342–394. [Google Scholar] [CrossRef]
- Kay, R. Exploring the use of video podcasts in education: A comprehensive review of the literature. Comp. Hum. Behav. 2012, 28, 820–831. [Google Scholar] [CrossRef]
- Coles, A. Mathematics teachers learning with video: The role, for the didactician, of a heightened listening. ZDM Math. Educ. 2014, 46, 267–278. [Google Scholar] [CrossRef]
- Linares, S. El Desarrollo de la competencia docente “mirar profesionalmente” la enseñanza-aprendizaje de las matemáticas (The Development of the teaching competence “noticing skill” at the teaching-learning of mathematics). Educ. Rev. 2013, 50, 117–133. [Google Scholar] [CrossRef]
- Fortuny, J.M.; Rodríguez, R. Aprender a mirar con sentido: Facilitar la interpretación de las interacciones en el aula (Learning to notice with meaning: Facilitating the interpretation of interactions in the classroom). Av. Inv. Educ. Matem. 2012, 1, 23–37. [Google Scholar]
- Santagata, R.; König, J.; Scheiner, T.; Nguyen, H.; Adleff, A.K.; Yang, X.; Kaiser, G. Mathematics teacher learning to notice: A systematic review of studies of video-based programs. ZDM-Math. Educ. 2021, 53, 119–134. [Google Scholar] [CrossRef]
- Couso, D.; Grimalt-Álvaro, C.; Simarro, C. Problematizing STEM integration from an epistemological and identity perspective. In Controversial Issues and Social Problems for an Integrated Disciplinary Teaching; Ortega Sánchez, D., Ed.; Springer International Publishing: Berlin, Germany, 2022. [Google Scholar]
- Barth-Cohen, L.; Little, A.; Abrahamson, D. Building reflective practices in a pre-service Math and Science Teacher Education Course that focuses on qualitative video analysis. J. Sci Teach. Educ. 2018, 29, 1–19. [Google Scholar] [CrossRef]
- Roller, S. What they notice in video: A study of prospective secondary mathematics teachers learning to teach. J. Math. Teach. Educ. 2016, 19, 477–498. [Google Scholar] [CrossRef]
- Van Es, E.A.; Tunney, J.; Goldsmith, L.; Seago, N. A framework for the facilitation of teachers’ analysis of video. J. Teach. Educ. 2014, 65, 340–356. [Google Scholar] [CrossRef]
- Arya, P.; Christ, T.; Chiu, M.M. Facilitation and Teacher Behaviors: An Analysis of Literacy Teachers’ Video-Case Discussions. J. Teach. Educ. 2013, 65, 111–127. [Google Scholar] [CrossRef]
- Castro, A.; Amador, J.; Bragelman, J. Facilitating video-based discussions to support prospective teacher noticing. J. Math. Behav. 2019, 54, 1–18. [Google Scholar] [CrossRef]
- Coles, A. Facilitating the use of video with teachers of mathematics: Learning from staying with the detail. Int. J. STEAM Educ. 2019, 6, 1–13. [Google Scholar] [CrossRef]
- Karsenty, R.; Pöhler, B.; Schwarts, G.; Prediger, S.; Arcavi, A. Processes of decision-making by mathematics PD facilitators: The role of resources, orientations, goals, and identities. J. Math. Teach. Educ. 2021, 1–25. [Google Scholar] [CrossRef]
- Beisiegel, M.; Mitchell, R.; Hill, H.C. The design of video-based professional development: An exploratory experiment intended to identify effective features. J. Teach. Educ. 2018, 69, 69–89. [Google Scholar] [CrossRef]
- Borko, H.; Jacobs, J.; Eiteljorg, E.; Pittman, M. Video as a tool for fostering productive discussions in mathematics professional development. Teach. Educ. 2008, 24, 417–436. [Google Scholar] [CrossRef]
- Kang, H.; van Es, E.A. Articulating Design Principles for Productive Use of Video in Preservice Education. J. Teach. Educ. 2019, 70, 250–287. [Google Scholar] [CrossRef]
- Tekkumru-Kisa, M.; Stein, M.K. A framework planning and facilitating video-based professional development. Int. J. STEM Educ. 2017, 4, 28. [Google Scholar] [CrossRef]
- Star, J.R.; Strickland, S.K. Learning to observe using video to improve preservice mathematics teachers’ ability to notice. J. Math. Teach. Educ. 2008, 11, 107–125. [Google Scholar] [CrossRef]
- Breda, A.; Pino-Fan, L.; Font, V. Meta didactic-mathematical knowledge of teachers: Criteria for the reflection and assessment on teaching practice. Eurasia J. Math. Sci. Technol. Educ. 2017, 13, 1893–1918. [Google Scholar] [CrossRef]
- Schön, D.A. The Reflective Practitioner: How Professionals Think in Action; Basic Books: New York, NY, USA, 1983. [Google Scholar]
- Perrenoud, P. Desarrollar la Práctica Reflexiva en el Oficio de Enseñar (Developing Reflective Practice in the Teaching Profession). Profesionalización y Razón Pedagógica; Graó: Barcelona, Spain, 2007. [Google Scholar]
- Jorba, J.; Sanmartí, N. Enseñar, Aprender y Evaluar: Un Proceso de Evaluación Continua (Teaching, Learning and Assessing: A Process of Continuous Evaluation); Ministerio de Educación y Cultura: Barcelona, Spain, 1994; Available online: https://sede.educacion.gob.es/publiventa/descarga.action?f_codigo_agc=20572 (accessed on 30 June 2021).
- Pearson, P.D.; Roehler, L.R.; Dole, J.A.; Duffy, G.G. Developing Expertise in Reading Comprehension; McGraw-Hill: New York, NY, USA, 1992. [Google Scholar]
- Sanmartí, N. Evaluar Para Aprender. 10 Ideas Clave; Graó: Barcelona, Spain, 2007. [Google Scholar]
- Schraw, G.; Crippen, K.J.; Hartley, K. Promoting Self-Regulation in Science Education: Metacognition as Part of a Broader Perspective on Learning. Res. Sci. Educ. 2006, 36, 111–139. [Google Scholar] [CrossRef]
- Sherin, M.; Jacobs, V.; Philipp, R. Mathematics Teacher Noticing. Seeing through Teachers’ Eyes; Routledge: New York, NY, USA, 2010. [Google Scholar]
- Hernández, R.; Fernández, C.; Baptista, P. Metodología de la Investigación (Research Methodology), 6th ed.; McGrawHill: Ciudad de México, Mexico, 2014. [Google Scholar]
- Elliot, J. La Investigación Acción en Educación (Action Research in Education); Morata: Madrid, Spain, 1990. [Google Scholar]
- Molina, M.; Castro, E.; Molina, J.L.; Castro, E. Un acercamiento a la investigación de diseño a través de los experimentos de enseñanza (An approach to design research through teaching experiments). Ense. Cienc. 2011, 29, 75–88. [Google Scholar] [CrossRef] [Green Version]
- Nurick, Y.; Arcavi, A.; Karsenty, R. How can a setting influence one’s reflection? In Proceedings of the 45th Conference of the International Group for the Psychology of Mathematics Education, Alicante, Spain, 18–23 July 2022; Fernández, C., Llinares, S., Gutiérrez, A., Planas, N., Eds.; PME: Alicante, Spain, 2022; Volume 3, pp. 259–266. [Google Scholar]
- Fortuny, J.M.; Recio, T.; Richard, P.R.; Roanes-Lozano, E. Análisis del discurso de los profesores en formación en un contexto de innovación pedagógica en geometría (Analysis of the discourse of teachers in training in a context of pedagogical innovation in geometry). Ann. Didac. Sci. Cogn. 2021, 26, 195–220. [Google Scholar]
- Smith, M.; Stein, M.K. The Five Practices for Orchestrating Productive Mathematics Discussions, 2nd ed.; NCTM: Reston, VA, USA, 2011. [Google Scholar]
- National Council of Teachers of Mathematics (NCTM). Principios y Estándares para la Educación Matemática; Thales: Sevilla, Spain, 2000. [Google Scholar]
- Schwarts, G.; Coles, A.; Arcavi, A. Leading mathematics teacher discussions during professional development: Challenges, opportunities, and discussion sense. F Learn. Math 2022, 42, 53–59. [Google Scholar]
- Llinares, S.; Ivars, P.; Buforn, À.; Groenwald, C. “Mirar profesionalmente” las situaciones de enseñanza: Una competencia basada en el conocimiento (“Noticing” teaching situations professionally: A knowledge-based competence). In Investigación Sobre el Profesor de Matemáticas: Práctica de Aula, Conocimiento, Competencia y Desarrollo Profesional; Badillo, E., Climent, N., Fernández, C., González, M.T., Eds.; Ediciones Universidad de Salamanca: Salamanca, Spain, 2019; pp. 177–192. [Google Scholar]
- Karsenty, R. Professional development of mathematics teachers: Through the lens of the camera. In Proceedings of the 13th International Congress on Mathematical Education, Hamburg, Germany, 24–31 July 2016; Kaiser, G., Forgasz, H., Graven, M.A., Kuzniak, E., Simmt, B., Eds.; Springer: Hamburg, Germany, 2018; pp. 269–288. [Google Scholar]
- Clarke, D. Facilitating reflection and action: The possible contribution of video to mathematics teacher education. In Proceedings of the 2nd Symposium on Video Resources for Mathematics Teacher Development, Weizmann Institute of Science, Rehovot, Israel, 7 January 2014. [Google Scholar]
- European Commission. Communication from the Commission to the European Parliament, the Council, the European Economic and Social Committee and the Committee of the Regions “Next Steps for a Sustainable European Future”. 2016. Available online: https://eur-lex.europa.eu/legal-content/EN/TXT/?uri=COM%3A2016%3A739%3AFIN (accessed on 20 August 2022).
- Karsenty, R.; Arcavi, A.; Nurick, Y. Video-based peer discussions as sources for knowledge growth of secondary teachers. In Proceedings of the CERME 9—Ninth Congress of the European Society for Research in Mathematics Education, Prague, Czech Republic, 4–8 February 2015; pp. 2825–2832. [Google Scholar]
- Schwarts, G.; Karsenty, R. “Can this happen only in Japan?”: Mathematics teachers reflect on a videotaped lesson in a cross-cultural context. J. Math. Teach. Educ. 2020, 23, 527–554. [Google Scholar] [CrossRef]

| Lens | Description | Example of Questions |
|---|---|---|
| Student’s mathematical thinking | Cognitive aspects. Identifying evidence of a student’s mathematical thinking and interpreting their mathematical understanding. | Describe what you think the student did to solve the problem. How do you interpret the student’s answer in terms of their mathematical knowledge? What mathematical difficulties do you identify in students? |
| Role of the teacher | Affective and ecological aspects. Approach to the teacher’s role in the interaction with students: asking questions, listening to comments, managing discussions, delegating responsibilities in the knowledge generation process, feedback, etc. | Describe the way the teacher responds and manages the class. Interpret the strategies used by the teacher during the course of the activity. How does the teacher resolve conflicts? |
| Classroom discourse | Interactive aspects. Focus on classroom interactions that promote mathematical learning opportunities for students. | Identify and describe a specific moment in the classroom discourse in which specific aspects of mathematics are worked on. Identifies and interprets with a theoretical foundation key moments of the discourse regarding the learning of mathematical content. What other learning opportunities have the different solutions to the problem provided the students? |
| Tasks | Mediation aspects. Analysis of the mathematical tasks or activities proposed to students to achieve the learning objectives. | Identifies and interprets with a theoretical foundation the key activities of the session that lead to the achievement of the mathematical learning objectives.What are the main mathematical ideas behind the task that students are expected to understand? What are the strengths and weaknesses of the task? How could it be improved to move towards the learning objectives? |
| Mathematical content | Epistemic aspects. Enumeration and analysis of the mathematical contents of the episode (school mathematics). | Identify the mathematics contained in this episode (concepts, procedures, linguistic elements, and properties). It evaluates whether the mathematical contents are worked on adequately. Do you identify in the teacher ambiguities in the meaning of the mathematical elements that emerge? Which is? How could they be avoided? |
| I1 | [FT]: Here I suggested to Mia that she go out and do her homework on the board instead of me doing it: “Mia, can you do it on the board?” She: “What number?” And me: “20”. And she went out and wrote the answer on the board. She did really well, and I was surprised. |
| I2 | [T]: Why were you surprised? |
| I3 | [FT]: I didn’t expect them to listen. She said, I don’t remember who she addressed, but we assume it was to John: “John, what are the divisors of 20?” And John answered him. I liked. |
| I1 | [T]: Whatever, in the written part. Sure, I believe it’s an important part and then we can start to formalize. |
| I2 | [FT]: Yes, yes, the idea was that … that is, I missed this part. Well, I basically did it. It was, like at the end of the session, I made the connection with the ideas of all the children, but it’s not the idea, or at least it shouldn’t have been like that. But an alternative would have been, which I already did in another session, and it turned out quite well, but not in this one, which was to let the students explain as if they were teachers, that is, the idea is for them to stand up, go to the blackboard with different colors, different tools and explain, in writing and orally, what they have to do to reach the solution. In other words, here the idea would have been: “Toni, okay, you have explained it very well, now you get up to the blackboard and go and explain it to everyone”. |
| I1 | [FT]: Yes, I noticed it in the videorecordings. The word “yes” and the word “okay”. |
| I2 | [T]: You see […]. This is already a significant part of change. That thanks to the videorecording a modification occurs. |
| I3 | [FT]: I have noticed these two things: that I talk a lot and that I repeat those words a lot. |
| I4 | [T]: It’s usual. We all have tics; what happens is that you have to be aware of it. |
| I5 | [FT]: Today I realized in class that I was about to say “okay”, but I stopped, that is, I noticed it and said: “you were about to say…“ |
| I1 | [G]: Sara did this. So, what has she done? She turns 3 × 3 red. 6 × 3 gives 18 and 8 × 3 gives 24. She’s done it two ways, the 3 and the 6. And the 6, she’s realized that 3 and 3 are 6, and so 4 of those red squares will make one of the 6 and it is fixed that you only need to take the 3 and the 6 as well. She says: “And 6 × 4 gives 24 and 6 × 3 gives 18”. |
| I2 | [T]: Did it just happen to her? |
| I3 | [G]: No, to more people. Here we have Nia. She did it like this (shows the girl’s evidence on the board). I used the 5 NCTM practices. The anticipation, I did the work with 4 different forms. A first form, which I think is perhaps the most typical, is me trying, but without making any sense. This was done, for example, by Diana. The other way is I look at the side that measures 18 and I overlap only the side, not my whole rectangle and see if my side fits. If it also fits on both sides, I understand that it works. The third way is, from this second, to vary and say: oh, okay, what does it have to do with the dividers on the sides. This is the third form that I wrote. In other words, here you already realize that it has to do with the dividers and it’s not like I’m checking, oh yes, yes look, it works, period. It works, why does it work? Oh well, I noticed that they are the dividers. Noah and Sara did this, they noticed and told me. (Search.) OK, here’s the selecting and sequencing part. I select which students I want to speak and sequence which forms I want them to come out first. |
| I1 | [T]: Now, if you could only make one comment about what you have prepared, what would you like to teach us? |
| I2 | [A]: Since Martin and Anna were clear, so let them be the ones who tried to convince the others, instead of… |
| I3 | [T]: OK |
| I4 | [A]: Take advantage of the fact that they are clear and that they explain it. I choose, because I know that Aleix has come to see that they are proportional and that Judith, almost links it to the fact that since the angle coincides, everything grows in the same way, I want these two to act as a bridge for me so that let’s go little by little. |
| I5 | [T]: But that’s very good. |
| I6 | [A]: But then what scares me about using Martin and Anna is that if they are also so clear, in the end we change one transmitter of knowledge, which would be me, for two which are them. In other words, I don’t know to what extent they will promote that other really become convinced of this or it will simply be: “no because having the same angle, they are proportional”. |
| I7 | [T]: Yes, but making an idea verbalize to another person increases the value of the idea to the person who verbalizes it. In other words, what changes is the epistemic value of the knowledge, they have more… If you have the opportunity for them to explain it, they have to do some mental actions that increase their understanding. In other words, what do Martin, and Anna get from me? If they already know, they understand it much better. |
| I8 | [T]: Is it the same, Gregory? |
| I9 | [G]: I think it is not the same. It increases the value, it’s peer-to-peer learning, it’s not the same. When a colleague explains something to you, it doesn’t have the same effect as if the teacher explains it to you. And this is particularly important. |
| I10 | [A]: Okay, but still… |
| I11 | [F]: One thing is the teacher, and the other is the students and the role of persuasion that one student has over another is often much higher than the role that the teacher has with respect to the student. It’s what he calls a teaching contract. It is already assumed that the teacher knows it and must teach it well and, therefore, the student listens to, but when another colleague, who has no obligation, tells him that this is so, the value that the other receives is much higher. It makes you put in a higher cognitive effort. |
| I1 | [T]: Stop, stop here (In Gregory’s videorecording). Why didn’t you show the floor tiles? |
| I2 | [G]: It’s an applet they have and it is used to see if it completes or not… |
| I3 | [T]: Why didn’t you give them cardboard and tile? You make 1 × 1, 2 × 2, 3 × 3 tiles…you work the area. And then, well, it’s like the Cuisenaire rods, you see how, which is the square and why some don’t. And even overlap one with the other and of course, that’s what he said, they go with the tablets just by moving the mouse. It’s like what I said to the teacher that “What have they learned?” “Move the mouse”. |
| I1 | [A]: In the middle of TU, I think I could have changed and done a couple of days of exercises, doing definitions and practice and then being able to go back to… |
| I2 | [G]: And why haven’t you done it? What’s the point of ending… |
| I3 | [T]: No, no, he has made up his mind that if this is the TU, this must be done. |
| I4 | [A]: We talked to my mentor, and he told me that it is also a way of learning, that is to say, that you made a mistake in this sense, that you thought that, perhaps the students were more capable or had many more facilities than they really have, and this is also a way to learn. Then he told me to continue with this TU as I had planned it and that nothing happened, but to do this reflection, especially important for me I would consider it in a different way, here, in this activity maybe that would change, maybe he wouldn’t have done it that way…that he thought of it this way, for the sake of improvement, to change the TU he had in mind. And that he considered that the TU itself has enjoyable content and is well structured. The only thing, the “timing” perhaps. |
| I5 | [T]: I think it is also important to reformulate because of course, the law is there, and you have planned, but at a given moment you have to know that those students you have in class…because as much as he wants to advance, if he is advancing on something that… let’s see, that, if they are not finding out, it will not help at all. You have to find that balance, but to reformulate, perhaps, at a certain moment, to say, this is my plan, and this is important. This is my plan, but I had to change this. Why I have had to change or how I have adapted, because in the end you have to adapt to them going up, but that happens to us at all educational levels. |
| I1 | [T]: (To Alex) You see his board, what do you see? |
| I2 | [A]: Everything is very symbolic… |
| I3 | [T]: In other words, if this were an advertisement that has to communicate something, the visual language is extremely poor. It’s your scheme (Gregory’s). Gregory, it doesn’t have to be your scheme, it has to be like an advertisement, it has to adapt to others. That’s what Alex meant. Or am I interpreting it wrong? |
| I4 | [G]: No, no. It is symbolic and since he is already truly clear about what the divisors are, which means that they are common, he makes the list of 18 and 24, see which ones are common and that’s it. But this is my… |
| I5 | [T]: In other words, on the board, it should not be your personal scheme, but it should be something to communicate to others. As Alex says, it’s too formal. |
| I6 | [G]: Yes, yes. The next class, this homework, which is the same but with different numbers, I told Sara to go out and do it. I mean, she was the one who wrote on the board and…no photo, but it was impressive. They paid more attention to her than to me. |
| I1 | [T]: Let’s see. Why are they divisors of 30? |
| I2 | [G]: The 1 and the 30? |
| I3 | [T]: No, you notice that, as a teacher, you say: “They are divisors of 30”, in other words, you are affirming something. I would write and then ask why they are divisors of 30. |
| I4 | [A]: I think that in this episode they told you, 1 × 30 gives 30, and then you keep pointing 1 × 30 = 30. |
| I5 | [T]: Yes, but notice that it says: “they are divisors of 30”. “Maria, 1 and 30 are divisors of 30, why?” |
| I6 | [A]: But I understand that this is a reminder, right? The teacher has already institutionalized the divider. And that they told you in the 1 × 30 form. 1 and 30 are numbers that multiply to 30 and therefore divide 30. |
| I7 | [T]: That! I want them to tell me all this. |
| I8 | [G]: Then let Alex come to class and sit down, because I wouldn’t have anyone but him. |
| I9 | [T]: Because then you are validating the knowledge. Otherwise, if you say they are dividers, it remains that the teacher said so. And then, you don’t make the children, in this case, make it explicit. In other words, it is to take advantage of… |
| I1 | [A]: And then what is also true is that David begins by asking that he has not understood the problem. Then we explain it to him and him himself… |
| I2 | [T]: Do you explain it to him, or do you have Raul explain it to him? |
| I3 | [A]: No, I explained it. |
| I4 | [T]: You always must let the students do it. |
| I5 | [A]: Yes, yes, I agree, but… |
| I6 | [T]: Don’t you mind? |
| I7 | [A]: No, no, I just haven’t thought about it. At that point … you’re like chatting with David you know? David says to you “but is that because I don’t want Messi to shoot?” and you say “No, no, Messi kicks, as if it were Pepe, what you want is to make it difficult for him.” It’s like you should stop 3’’ and say “Ok Raul…” |
| I8 | [T]: Here is something difficult. Suppose you have a class with 22 students like Gregory has. Then you have to put 22 threads of wool between you and the students and between them, that the conversation is not a thread and you forget about the other threads, but it is a global conversation, it is a network that must pass from one to the others, that is, you can stop at David, but immediately you have to keep in mind, which is difficult, the other 21… |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ricart, M.; Estrada, A.; Fortuny, J.M. Analysis of Tutoring in the Professional Development of STEM Teachers. Mathematics 2022, 10, 3331. https://doi.org/10.3390/math10183331
Ricart M, Estrada A, Fortuny JM. Analysis of Tutoring in the Professional Development of STEM Teachers. Mathematics. 2022; 10(18):3331. https://doi.org/10.3390/math10183331
Chicago/Turabian StyleRicart, Maria, Assumpta Estrada, and Josep Maria Fortuny. 2022. "Analysis of Tutoring in the Professional Development of STEM Teachers" Mathematics 10, no. 18: 3331. https://doi.org/10.3390/math10183331
APA StyleRicart, M., Estrada, A., & Fortuny, J. M. (2022). Analysis of Tutoring in the Professional Development of STEM Teachers. Mathematics, 10(18), 3331. https://doi.org/10.3390/math10183331







