Optical Solitons with the Complex Ginzburg–Landau Equation with Kudryashov’s Law of Refractive Index

Abstract

In this paper, the optical solitons for the complex Ginzburg–Landau equation with Kudryashov’s law of refractive index are established. An improved modified extended tanh–function technique is used to extract numerous solutions. Bright and dark solitons, as well as singular soliton solutions, are achieved. In addition, as the modulus of ellipticity approaches unity or zero, solutions are formulated in terms of Jacobi’s elliptic functions, which provide solitons and periodic wave solutions.

1. Introduction

The non-linear Schrödinger equation (NLSE) is one of the most significant underlying mathematical models in optics with several applications in transmission and the design of various non-linear photonic devices. Several models followed by NLSE describe the dynamics of soliton transmission over trans-continental distances. The Gabitov–Turitsyn equation (GTE) describes the slow dynamics of pulses in a dispersion-managed, non-linear system, which is governed solely by an averaged dispersion and non-linearity [1,2]. The Fokas–Lenells equation was derived as a model to describe femtosecond pulse propagation through a single-mode optical silica fiber [3]. The complex Ginzburg–Landau (CGL) equation is one of the most widely researched non-linear models in physics, appearing in the context of soliton propagation dynamics in the telecommunications sector and in non-linear optics. It describes, on a qualitative, and often on a quantitative, level, a wide variety of phenomena from non-linear waves to second-order phase transitions, from superconductivity, superfluidity, and Bose–Einstein condensation to liquid crystals and strings in field theory [4,5,6,7,8,9]. Several articles have investigated different modified versions of this model [10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25]. Cubic–quartic optical soliton perturbation with a CGL equation having ten different forms of non-linear refractive index have been obtained using the enhanced Kudryashov method [19,20]. Highly dispersive optical solitons for the CGL equation with six non-linear forms have been extracted by a modified version of Kudryashov’s method [21]. Dark-singular hybrid optical solitons with a fractional CGL equation have been obtained using an extended Jacobi elliptic function expansion algorithm [22]. Optical solitons in birefringent fibers with a CGL equation having Hamiltonian perturbations have been recovered using a modified extended direct algebraic method [23].

In this study, we implement an improved, modified extended tanh–function (IMETF) method to investigate the optical solitons of the CGL equation with Kudryashov’s law of refractive index, which is considered as [24]

$$i{\psi }_t+a{\psi }_{xx}+\left(b_1{\left|\psi \right|}^{-4n}+b_2{\left|\psi \right|}^{-2n}+b_3{\left|\psi \right|}^{2n}+b_4{\left|\psi \right|}^{4n}\right)\psi +\frac{1}{{\left|\psi \right|}^2{\psi }^{*}}\left\{{\alpha \left|\psi \right|}^2{\left({\left|\psi \right|}^2\right)}_{xx}-\beta {\left({\left|\psi \right|}^2\right)}_x^2\right\}+\gamma \psi =0.$$

(1)

Here, $\psi (x,t)$ is a complex–valued function that represent the non-linear wave profile with the spatial and temporal coordinates x and t. The first term is the linear temporal evolution, while a is the coefficient of the chromatic dispersion. Here, $b_j$, for $1\le j\le 5$, stand for the self–phase modulation structure. $\alpha$ and $\beta$ give additional non-linearities for the CGL equation and $\gamma$ accounts for the coefficient of the detuning parameter.

The version of CGLE studied comes with Kudryashov’s extended version of generalized anti-cubic law non-linearity, which is the generalization of the well-known CGLE. Other non–Kerr laws of non-linearity have been studied in the literature. Setting $b_1=b_2=0$, $n=\frac{1}{2}$ in (1), yields the quadratic–cubic law, while setting $b_1=b_2=0,n=1$ gives the cubic–quintic law; bright and dark solitons are recovered for the last two non-linear forms in [11]. For $b_1=b_2=0$, we reach the dual–power law, which retrieved a dark soliton solution [13], while at $b_2=0,n=1$, the generalized anti–cubic law is given with dark, singular solitons and combo-optical solitons [25]. With the aid of the IMETF algorithm, we extract bright, dark solitons and singular soliton solutions along with solutions formulated in terms of Jacobi’s elliptic functions.

2. IMETF Procedure

Consider a non-linear evolution equation of the form,

$$F\left(\phi ,{\phi }_t,{\phi }_x,{\phi }_{xx},{\phi }_{xt},...\right)=0,$$

(2)

Here, $\phi =\phi (x,t)$ is an unknown function, F is a polynomial in $\phi$ and its several partial derivatives ${\phi }_t,{\phi }_x$ with respect to $t,x$, respectively, in which the non-linear terms and the highest order derivatives are involved.

• Step-1: Use a wave transformation

$$\phi (x,t)=U\left(\xi \right),\xi =k(x-vt),$$

(3)

where $k,v$ are real constants to be determined. Upon this transformation, (2) is reduced to

$$P\left(U,-kv{U}^{\prime },k{U}^{\prime },k^2{U}^{″},...\right)=0,$$

(4)

where P is a polynomial in $U\left(\xi \right)$ and its derivatives.

• Step-2: Assume that the solution of Equation (4) can be expressed as [26,27]

$$U\left(\xi \right)=\sum _{i=0}^N{\alpha }_i{\mathrm{\Phi }}^i+\sum _{i=1}^N{\beta }_i{\mathrm{\Phi }}^{-i},$$

(5)

where $\mathrm{\Phi }$ satisfies

$${\mathrm{\Phi }}^{\prime }=\pm \sqrt{{\delta }_0+{\delta }_1\mathrm{\Phi }+{\delta }_2{\mathrm{\Phi }}^2+{\delta }_3{\mathrm{\Phi }}^3+{\delta }_4{\mathrm{\Phi }}^4}.$$

(6)

This equation gives a wide variety of solutions [27].

• Step-3: The integer number N in Equation (5) is determined by balancing the highest-order derivatives and the non-linear terms in Equation (4).
• Step-4: In Equation (4), substitute solution (5) together with (6). As a result, we obtain a polynomial of $\mathrm{\Phi }$. We gather all terms of the same powers in the generated polynomial and equate them to zero to construct a system of algebraic equations that can be solved using Mathematica to identify the unknown parameters. $k,v,{\alpha }_0,{\alpha }_i$ and ${\beta }_i(i=1,2,...)$ to obtain the solutions of Equation (2).

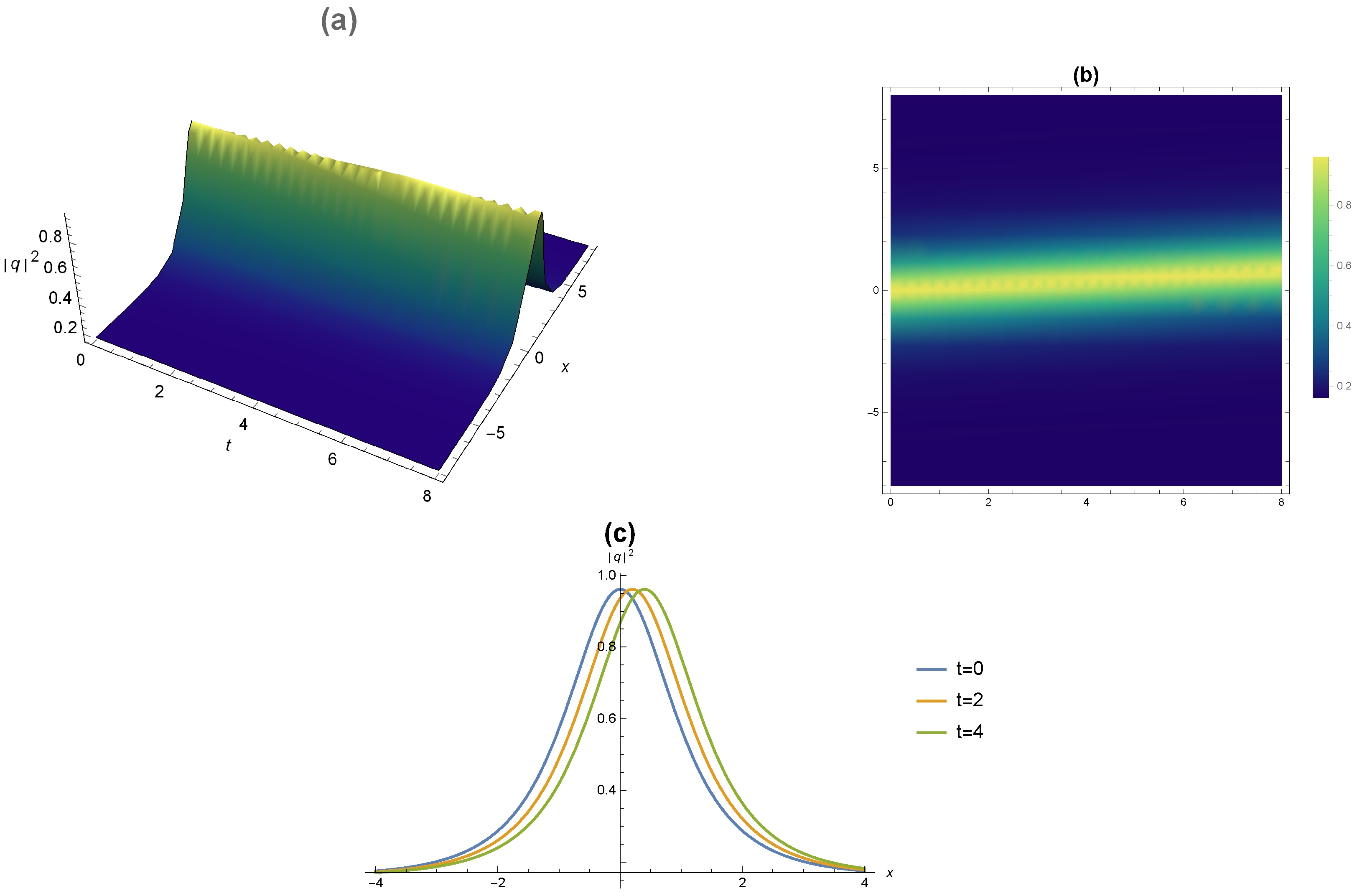
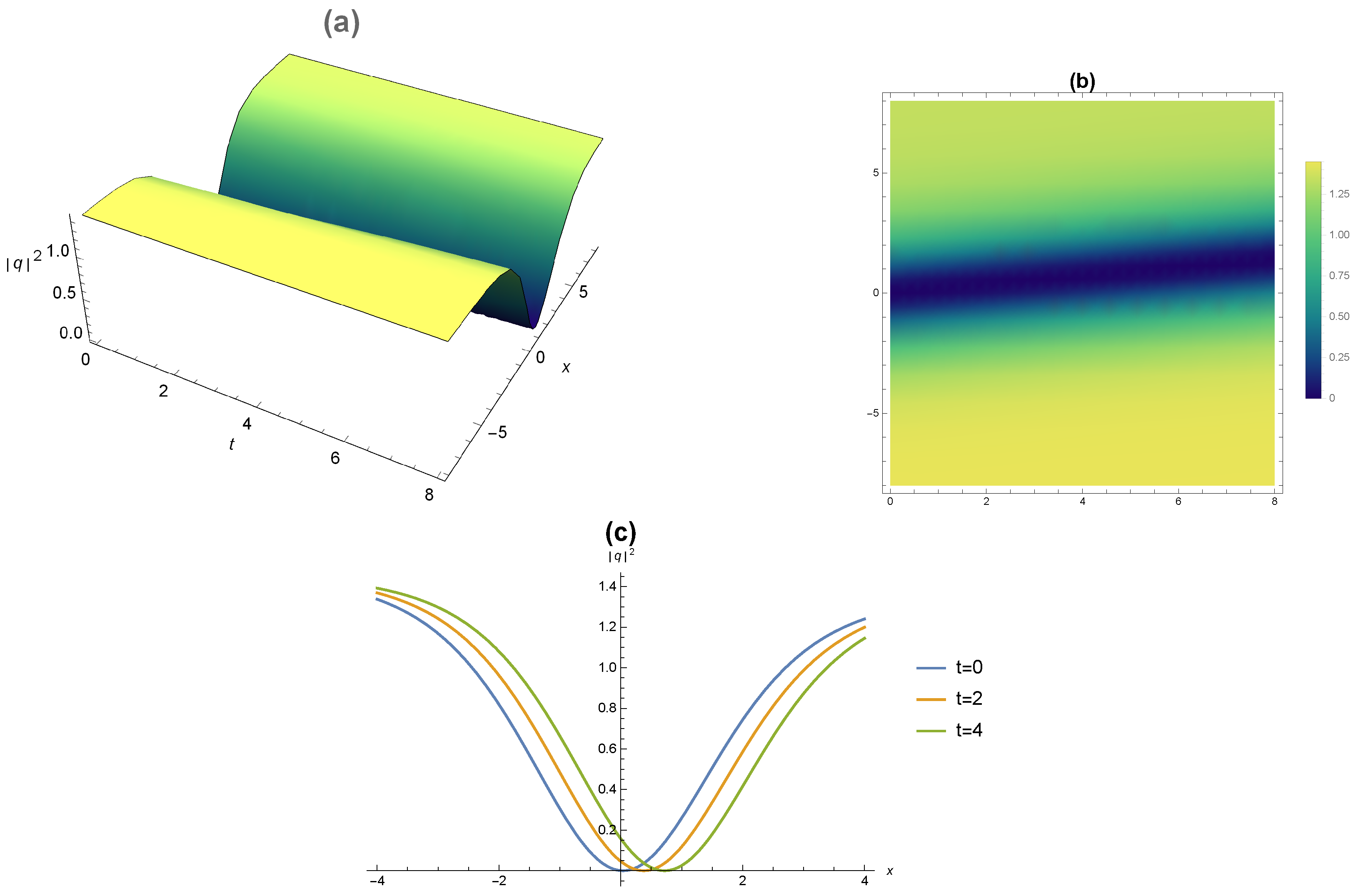
3. Soliton Solutions with CGL Model

In order to solve Equation (1), the following solution structure is selected.

$$\psi (x,t)=U\left(\xi \right)e^{i\varphi (x,t)},$$

(7)

with

$$\xi =k(x-vt).$$

(8)

$U\left(\xi \right)$ is the amplitude component of the soliton solution and v is the soliton speed, while $\varphi (x,t)$ is the phase component, defined as

$$\varphi (x,t)=-\kappa x+\omega t+\vartheta .$$

(9)

$$\left\{\begin{array}{c}\kappa :\mathrm{Frequency}\mathrm{of}\mathrm{the}\mathrm{soliton},\hfill \\ \omega :\mathrm{Wave}\mathrm{number},\hfill \\ \vartheta :\mathrm{Phase}\mathrm{constant}.\hfill \end{array}\right.$$

Substituting (10) into (1) and then decomposing into a real part and an imaginary part gives

$$\begin{array}{ccc}\hfill & & k^2(a+2\alpha )U^{4n+1}{U}^{″}+U^{4n+2}\left(-a{\kappa }^2+\gamma -\omega \right)+b_2{U}^{2n+2}+b_3{U}^{6n+2}+\hfill \\ & & b_4{U}^{8n+2}+b_1{U}^2+2k^2(\alpha -2\beta )U^{4n}{U}^{\prime 2}=0,\hfill \end{array}$$

(10)

and

$$-k(2a\kappa +v)U^{4n+1}{U}^{\prime }=0.$$

(11)

From the imaginary part, it is possible to obtain v as

$$v=-2a\kappa .$$

(12)

Using the transformation

$$U=V^{\frac{1}{2n}}.$$

(13)

So that (10) transforms to

$$G_2{V}^4+G_3{V}^3+G_4{V}^2+G_1{V}^{\prime 2}+G_{5}V+G_6+V{V}^{″}=0,$$

(14)

Balancing $V{V}^{″}$ with $V^4$ in Equation (1) yields $N=1$. Then solution (1) takes the form

$$V\left(\xi \right)={\alpha }_0+{\alpha }_1\Phi \left(\xi \right)+{\beta }_1\Phi {\left(\xi \right)}^{-1}.$$

(15)

Applying step-4 in Section 2 to obtain the following system of algebraic equations (Appendix A). Solving this system by Mathematica to obtain the following results:

• Case-1: Setting ${\delta }_0={\delta }_1={\delta }_3=0$ to get

$$\begin{array}{ccc}\hfill & & {\alpha }_0=-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2},{\delta }_2=\frac{3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4}{2\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2},{\delta }_4=-\frac{{\alpha }_1^2{G}_2}{G_1+2},\hfill \\ & & {\beta }_1=0,G_5=\frac{\left(2G_1^2+5G_1+2\right)G_3\left(\left(2G_1+3\right){}^2{G}_2{G}_4-\left(G_1^2+3G_1+2\right)G_3^2\right)}{2\left(G_1+1\right)\left(2G_1+3\right){}^3{G}_2^2},\hfill \\ & & G_6=\frac{G_1\left(G_1+2\right){}^2{G}_3^2\left(4\left(2G_1+3\right){}^2{G}_2{G}_4-5\left(G_1^2+3G_1+2\right)G_3^2\right)}{16\left(G_1+1\right)\left(2G_1+3\right){}^4{G}_2^3}.\hfill \end{array}$$

(16)

Plugging (16) into (15) reaches

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2}+\sqrt{\frac{\left(G_1+2\right)\left(3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4\right)}{4\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2^2}}\hfill \\ & & \times \sec \mathrm{h}\left[\sqrt{\frac{3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4}{2\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )},\hfill \end{array}$$

(17)

which represent a bright soliton solution with constraint

$$\left(G_1+1\right)G_2(\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4)>0,$$

and

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2}+\sqrt{\frac{\left(G_1+2\right)\left(3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4\right)}{4\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2^2}}\hfill \\ & & \times \sec \left[\sqrt{-\frac{3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4}{2\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )}.\hfill \end{array}$$

(18)

which represent a singular periodic wave solution with constraint

$$\left(G_1+1\right)G_2(\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4)<0.$$

• Case-2: Setting ${\delta }_1={\delta }_3=0$ and ${\delta }_0=\frac{{\delta }_2^2}{4{\delta }_4}$ to get
• Result-1

$$\begin{array}{ccc}\hfill & & {\alpha }_0=-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2},{\beta }_1=0,{\delta }_4=-\frac{{\alpha }_1^2{G}_2}{G_1+2},{\delta }_2=\frac{3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4}{2\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2},\hfill \\ & & G_5=\frac{\left(2G_1^2+5G_1+2\right)G_3\left({\left(2G_1+3\right)}^2{G}_2{G}_4-\left(G_1^2+3G_1+2\right)G_3^2\right)}{2\left(G_1+1\right)\left(2G_1+3\right){}^3{G}_2^2},\hfill \\ & & G_6=\frac{G_1\left(G_1+2\right)\left(\left(G_1^2+3G_1+2\right)G_3^2-\left(2G_1+3\right){}^2{G}_2{G}_4\right){}^2}{4\left(G_1+1\right){}^2\left(2G_1+3\right){}^4{G}_2^3}.\hfill \end{array}$$

(19)

Plugging (19) into (15) reaches

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2}+\sqrt{\frac{\left(G_1+2\right)\left(3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4\right)}{4\left(G_1+1\right){\left(2G_1+3\right)}^2{G}_2^2}}\hfill \\ & & \times tanh\left[\sqrt{-\frac{3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4}{4\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )},\hfill \end{array}$$

(20)

which represent a dark soliton solution with constraint

$$\left(G_1+1\right)G_2(\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4)<0,$$

and

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2}+\sqrt{-\frac{\left(G_1+2\right)\left(3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4\right)}{4\left(G_1+1\right){\left(2G_1+3\right)}^2{G}_2^2}}\hfill \\ & & \times tan\left[\sqrt{\frac{3\left(G_1^2+3G_1+2\right)G_3^2-2{\left(2G_1+3\right)}^2{G}_2{G}_4}{4\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )},\hfill \end{array}$$

(21)

which represent a singular periodic wave solution with constraint

$$\left(G_1+1\right)G_2(\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4)>0.$$

• Result-2

$$\begin{array}{ccc}\hfill & & {\alpha }_0=-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2},{\alpha }_1=0,{\delta }_2=\frac{3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4}{2\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2},\hfill \\ & & {\delta }_4=-\frac{\left(G_1+2\right)\left(3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4\right){}^2}{16{\beta }_1^2\left(G_1+1\right){}^2\left(2G_1+3\right){}^4{G}_2^3},\hfill \\ & & G_5=\frac{\left(2G_1^2+5G_1+2\right)G_3\left(\left(2G_1+3\right){}^2{G}_2{G}_4-\left(G_1^2+3G_1+2\right)G_3^2\right)}{2\left(G_1+1\right)\left(2G_1+3\right){}^3{G}_2^2},\hfill \\ & & G_6=\frac{G_1\left(G_1+2\right)\left(\left(G_1^2+3G_1+2\right)G_3^2-\left(2G_1+3\right){}^2{G}_2{G}_4\right){}^2}{4\left(G_1+1\right){}^2\left(2G_1+3\right){}^4{G}_2^3}.\hfill \end{array}$$

(22)

Plugging (22) into (15) reaches

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2}+\sqrt{\frac{\left(G_1+2\right)\left(3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4\right)}{4\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2^2}}\hfill \\ & & \times \cot \mathrm{h}\left[\sqrt{-\frac{3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4}{4\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )},\hfill \end{array}$$

(23)

which represent a singular soliton solution with constraint

$$\left(G_1+1\right)G_2(\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4)<0,$$

and

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2}+\sqrt{-\frac{\left(G_1+2\right)\left(3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4\right)}{4\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2^2}}\hfill \\ & & \times \cot \left[\sqrt{\frac{3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4}{4\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )}.\hfill \end{array}$$

(24)

which represent a singular periodic wave solution with constraint

$$\left(G_1+1\right)G_2(\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4)>0.$$

• Case-3: Setting ${\delta }_1={\delta }_3=0$ and ${\delta }_0=\frac{{\delta }_2^2{m}^2\left(1-m^2\right)}{{\delta }_4{\left(2m^2-1\right)}^2}$ to get
• Result-1

$$\begin{array}{ccc}\hfill & & {\alpha }_0=-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2},{\delta }_2=\frac{3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4}{2\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2},{\delta }_4=-\frac{{\alpha }_1^2{G}_2}{G_1+2},\hfill \\ & & G_5=\frac{\left(2G_1^2+5G_1+2\right)G_3\left(\left(2G_1+3\right){}^2{G}_2{G}_4-\left(G_1^2+3G_1+2\right)G_3^2\right)}{2\left(G_1+1\right)\left(2G_1+3\right){}^3{G}_2^2},{\beta }_1=0,\hfill \\ & & G_6=-\frac{{\varrho }_1}{16\left(G_1+1\right){}^2\left(2G_1+3\right){}^4{G}_2^3{\left(1-2m^2\right)}^2},\hfill \\ & & {\varrho }_1=G_1\left(G_1+2\right)(16G_2^2{G}_4^2\left(2G_1+3\right){}^4{m}^2\left(m^2-1\right)-4\left(G_1^2+3G_1+2\right)G_2{G}_3^2{G}_4\left(2G_1+3\right){}^2\hfill \\ & & \left(16m^4-16m^2+1\right)+\left(G_1^2+3G_1+2\right){}^2{G}_3^4\left(56m^4-56m^2+5\right)).\hfill \end{array}$$

(25)

Plugging (25) into (15) reaches

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2}+\sqrt{\frac{\left(G_1+2\right)\left(3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4\right)m^2}{2\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2^2\left(2m^2-1\right)}}\hfill \\ & & \mathrm{cn}\left[\sqrt{\frac{3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4}{2\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2\left(2m^2-1\right)}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )}.\hfill \end{array}$$

(26)

If we choose $m=1$, then we get a bright soliton solution

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2}+\sqrt{\frac{\left(G_1+2\right)\left(3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4\right)}{2\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2^2}}\hfill \\ & & \sec \mathrm{h}\left[\sqrt{\frac{3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4}{2\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )}.\hfill \end{array}$$

(27)

• Result-2

$$\begin{array}{ccc}\hfill & & {\alpha }_0=-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2},{\alpha }_1=0,{\delta }_4=\frac{\left(G_1+2\right)\left(3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4\right){}^2{m}^2\left(m^2-1\right)}{4{\beta }_1^2\left(G_1+1\right){}^2\left(2G_1+3\right){}^4{G}_2^3{\left(1-2m^2\right)}^2},\hfill \\ & & {\delta }_2=\frac{3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4}{2\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2},G_6=-\frac{{\varrho }_1}{16\left(G_1+1\right){}^2\left(2G_1+3\right){}^4{G}_2^3{\left(1-2m^2\right)}^2},\hfill \\ & & G_5=\frac{\left(2G_1^2+5G_1+2\right)G_3\left(\left(2G_1+3\right){}^2{G}_2{G}_4-\left(G_1^2+3G_1+2\right)G_3^2\right)}{2\left(G_1+1\right)\left(2G_1+3\right){}^3{G}_2^2}.\hfill \end{array}$$

(28)

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2}+\sqrt{\frac{\left(G_1+2\right)\left(2\left(2G_1+3\right){}^2{G}_2{G}_4-3\left(G_1^2+3G_1+2\right)G_3^2\right)\left(m^2-1\right)}{2\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2^2\left(2m^2-1\right)}}\hfill \\ & & \times \mathrm{nc}\left[\sqrt{\frac{3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4}{2\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2\left(2m^2-1\right)}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )}.\hfill \end{array}$$

(29)

If we choose $m=0$, then we get a singular periodic wave solution

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2}+\sqrt{\frac{\left(G_1+2\right)\left(2\left(2G_1+3\right){}^2{G}_2{G}_4-3\left(G_1^2+3G_1+2\right)G_3^2\right)}{2\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2^2}}\hfill \\ & & \times \sec \left[\sqrt{-\frac{3\left(G_1^2+3G_1+2\right)G_3^2-2\left(2G_1+3\right){}^2{G}_2{G}_4}{2\left(G_1+1\right)\left(2G_1+3\right){}^2{G}_2}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )}.\hfill \end{array}$$

(30)

• Case-4: Setting ${\delta }_1={\delta }_3=0$ and ${\delta }_0=\frac{{\delta }_2^2\left(1-m^2\right)}{{\delta }_4{\left(2-m^2\right)}^2}$ to get
• Result-1

$$\begin{array}{ccc}\hfill & & {\alpha }_0=-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2},{\beta }_1=0,{\delta }_2=\frac{\left(G_1+2\right){}^2\left(2G_1+1\right)G_3^3-4\left(2G_1+3\right){}^3{G}_2^2{G}_5}{2\left(2G_1+3\right){}^2\left(2G_1^2+5G_1+2\right)G_2{G}_3},\hfill \\ & & {\delta }_4=-\frac{{\alpha }_1^2{G}_2}{G_1+2},G_4=\frac{\left(G_1+1\right)\left(2G_2^2{G}_5\left(2G_1+3\right){}^3+\left(G_1+2\right){}^2\left(2G_1+1\right)G_3^3\right)}{\left(2G_1+3\right){}^2\left(2G_1^2+5G_1+2\right)G_2{G}_3},\hfill \\ & & G_6=\frac{{\varrho }_2}{16\left(2+G_1\right)\left(1+2G_1\right){}^2\left(2G_1+3\right){}^4{G}_2^3{G}_3^2{\left(m^2-2\right)}^2},\hfill \\ & & {\varrho }_2=G_1(8\left(G_1+2\right){}^2\left(2G_1+1\right)G_2^2{G}_3^3{G}_5\left(2G_1+3\right){}^3{m}^4-\hfill \\ & & \left(G_1+2\right){}^4\left(2G_1+1\right){}^2{G}_3^6{m}^4-64G_2^4{G}_5^2\left(2G_1+3\right){}^6\left(m^2-1\right)).\hfill \end{array}$$

(31)

Plugging (31) into (15) reaches

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{G_3\left(2+G_1\right)}{2\left(2G_1+3\right)G_2}+\sqrt{\frac{\left(2+G_1\right)\left(\left(2+G_1\right){}^2\left(2G_1+1\right)G_3^3-4\left(2G_1+3\right){}^3{G}_2^2{G}_5\right)m^2}{2\left(2G_1+3\right){}^2\left(2G_1^2+5G_1+2\right)G_2^2{G}_3\left(2-m^2\right)}}\hfill \\ & & \mathrm{dn}\left[\sqrt{\frac{\left(G_1+2\right){}^2\left(2G_1+1\right)G_3^3-4\left(2G_1+3\right){}^3{G}_2^2{G}_5}{2\left(2G_1+3\right){}^2\left(2G_1^2+5G_1+2\right)G_2{G}_3\left(2m^2-1\right)}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )}.\hfill \end{array}$$

(32)

If we choose $m=1$, then we get a bright soliton solution

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{G_3\left(2+G_1\right)}{2\left(2G_1+3\right)G_2}+\sqrt{\frac{\left(2+G_1\right)\left(\left(2+G_1\right){}^2\left(2G_1+1\right)G_3^3-4\left(2G_1+3\right){}^3{G}_2^2{G}_5\right)}{2\left(2G_1+3\right){}^2\left(2G_1^2+5G_1+2\right)G_2^2{G}_3}}\hfill \\ & & \sec \mathrm{h}\left[\sqrt{\frac{\left(G_1+2\right){}^2\left(2G_1+1\right)G_3^3-4\left(2G_1+3\right){}^3{G}_2^2{G}_5}{2\left(2G_1+3\right){}^2\left(2G_1^2+5G_1+2\right)G_2{G}_3}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )}.\hfill \end{array}$$

(33)

• Result-2

$$\begin{array}{ccc}\hfill & & {\alpha }_0=-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2},{\alpha }_1=0,{\delta }_2=\frac{\left(G_1+2\right){}^2\left(2G_1+1\right)G_3^3-4\left(2G_1+3\right){}^3{G}_2^2{G}_5}{2\left(2G_1+3\right){}^2\left(2G_1^2+5G_1+2\right)G_2{G}_3},\hfill \\ & & {\delta }_4=\frac{\left(\left(G_1+2\right){}^2{G}_3^3\left(1+2G_1\right)-4\left(2G_1+3\right){}^3{G}_2^2{G}_5\right){}^2\left(m^2-1\right)}{4{\beta }_1^2\left(2+G_1\right)\left(1+2G_1\right){}^2\left(2G_1+3\right){}^4{G}_2^3{G}_3^2{\left(m^2-2\right)}^2},\hfill \\ & & G_4=\frac{\left(G_1+1\right)\left(2G_2^2{G}_5\left(2G_1+3\right){}^3+\left(G_1+2\right){}^2\left(2G_1+1\right)G_3^3\right)}{\left(2G_1+3\right){}^2\left(2G_1^2+5G_1+2\right)G_2{G}_3},\hfill \\ & & G_6=\frac{{\varrho }_2}{16\left(2+G_1\right)\left(1+2G_1\right){}^2\left(2G_1+3\right){}^4{G}_2^3{G}_3^2{\left(m^2-2\right)}^2}\hfill \\ & & {\varrho }_2=G_1(8\left(G_1+2\right){}^2\left(2G_1+1\right)G_2^2{G}_3^3{G}_5\left(2G_1+3\right){}^3{m}^4-\hfill \\ & & \left(G_1+2\right){}^4\left(2G_1+1\right){}^2{G}_3^6{m}^4-64G_2^4{G}_5^2\left(2G_1+3\right){}^6\left(m^2-1\right)).\hfill \end{array}$$

(34)

Plugging (34) into (15) reaches

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2}+\sqrt{-\frac{\left(2G_1^2+5G_1+2\right)\left(\left(G_1+2\right){}^2\left(2G_1+1\right)G_3^3-4\left(2G_1+3\right){}^3{G}_2^2{G}_5\right)\left(m^2-1\right)}{2\left(G_1+2\right)\left(2G_1+1\right){}^2\left(2G_1+3\right){}^2{G}_2^2{G}_3{m}^2\left(2-m^2\right)}}\hfill \\ & & \times \mathrm{nd}\left[\sqrt{\frac{\left(G_1+2\right){}^2\left(2G_1+1\right)G_3^3-4\left(2G_1+3\right){}^3{G}_2^2{G}_5}{2\left(2G_1+3\right){}^2\left(2G_1^2+5G_1+2\right)G_2{G}_3\left(2m^2-1\right)}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )},\hfill \end{array}$$

(35)

which represents a Jacobi elliptic function solution.

• Case-5: Setting ${\delta }_1={\delta }_3=0$ and ${\delta }_0=\frac{{\delta }_2^2{m}^2}{{\delta }_4{\left(m^2+1\right)}^2}$ to get

$$\begin{array}{ccc}\hfill & & {\alpha }_0=-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2},{\beta }_1=0,{\delta }_2=\frac{\left(G_1+2\right){}^2\left(2G_1+1\right)G_3^3-4\left(2G_1+3\right){}^3{G}_2^2{G}_5}{2\left(2G_1+3\right){}^2\left(2G_1^2+5G_1+2\right)G_2{G}_3},\hfill \\ & & {\delta }_4=-\frac{{\alpha }_1^2{G}_2}{G_1+2},G_4=\frac{\left(G_1+1\right)\left(2G_2^2{G}_5\left(2G_1+3\right){}^3+\left(G_1+2\right){}^2\left(2G_1+1\right)G_3^3\right)}{\left(2G_1+3\right){}^2\left(2G_1^2+5G_1+2\right)G_2{G}_3},\hfill \\ & & G_6=\frac{{\varrho }_3}{16\left(G_1+2\right)\left(2G_1+1\right){}^2\left(2G_1+3\right){}^4{G}_2^3{G}_3^2{\left(m^2+1\right)}^2}\hfill \\ & & {\varrho }_3=G_1(64G_2^4{G}_5^2\left(2G_1+3\right){}^6{m}^2+8\left(G_1+2\right){}^2\left(2G_1+1\right)G_2^2{G}_3^3{G}_5\left(2G_1+3\right){}^3{\left(m^2-1\right)}^2-\hfill \\ & & \left(G_1+2\right){}^4\left(2G_1+1\right){}^2{G}_3^6{\left(m^2-1\right)}^2).\hfill \end{array}$$

(36)

Plugging (36) into (15) reaches

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{G_3\left(2+G_1\right)}{2\left(2G_1+3\right)G_2}+\sqrt{\frac{\left(2+G_1\right)\left(\left(2+G_1\right){}^2\left(2G_1+1\right)G_3^3-4\left(2G_1+3\right){}^3{G}_2^2{G}_5\right)m^2}{2\left(2G_1+3\right){}^2\left(2G_1^2+5G_1+2\right)G_2^2{G}_3\left(m^2+1\right)}}\hfill \\ & & \times \mathrm{sn}\left[\sqrt{-\frac{\left(G_1+2\right){}^2\left(2G_1+1\right)G_3^3-4\left(2G_1+3\right){}^3{G}_2^2{G}_5}{2\left(2G_1+3\right){}^2\left(2G_1^2+5G_1+2\right)G_2{G}_3\left(m^2+1\right)}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )}.\hfill \end{array}$$

(37)

If we choose $m=1$, then we get a dark soliton solution

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{G_3\left(2+G_1\right)}{2\left(2G_1+3\right)G_2}+\sqrt{\frac{\left(2+G_1\right)\left(\left(2+G_1\right){}^2\left(2G_1+1\right)G_3^3-4\left(2G_1+3\right){}^3{G}_2^2{G}_5\right)}{4\left(2G_1+3\right){}^2\left(2G_1^2+5G_1+2\right)G_2^2{G}_3}}\hfill \\ & & \times \tan \mathrm{h}\left[\sqrt{-\frac{\left(G_1+2\right){}^2\left(2G_1+1\right)G_3^3-4\left(2G_1+3\right){}^3{G}_2^2{G}_5}{4\left(2G_1+3\right){}^2\left(2G_1^2+5G_1+2\right)G_2{G}_3}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )}.\hfill \end{array}$$

(38)

• Case-6: Setting ${\delta }_1={\delta }_3={\delta }_4=0$ to get

$$\begin{array}{ccc}\hfill & & {\alpha }_1=0,{\beta }_1=\pm \sqrt{-\frac{{\delta }_0\left(G_1+2\right)}{G_2}},{\alpha }_0=-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2},{\delta }_2=\frac{3\left(G_1+2\right)G_3^2}{2\left(2G_1+3\right){}^2{G}_2}-\frac{G_4}{G_1+1},\hfill \\ & & G_5=\frac{\left(2G_1^2+5G_1+2\right)G_3\left(\left(2G_1+3\right){}^2{G}_2{G}_4-\left(G_1^2+3G_1+2\right)G_3^2\right)}{2\left(G_1+1\right)\left(2G_1+3\right){}^3{G}_2^2},\hfill \\ & & G_6=\frac{G_1\left(G_1+2\right){}^2{G}_3^2\left(4\left(2G_1+3\right){}^2{G}_2{G}_4-5\left(G_1^2+3G_1+2\right)G_3^2\right)}{16\left(G_1+1\right)\left(2G_1+3\right){}^4{G}_2^3}.\hfill \end{array}$$

(39)

Plugging (39) into (15) gives the singular soliton and singular periodic wave solution for $G_4\left(G_1+1\right)>0$ and $G_2\left(G_1+2\right)<0$

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2}\pm \sqrt{-\frac{\left(G_1+2\right)}{2G_2^2}\left(\frac{3\left(G_1+2\right)G_3^2}{\left(2G_1+3\right){}^2}+\frac{2G_2{G}_4}{G_1+1}\right)}\hfill \\ & & \times \csc \mathrm{h}\left[\sqrt{\frac{G_4}{G_1+1}-\frac{3\left(G_1+2\right)G_3^2}{2\left(2G_1+3\right){}^2{G}_2}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )},\hfill \end{array}$$

(40)

$$\begin{array}{ccc}\hfill & & \psi (x,t)=\{-\frac{\left(G_1+2\right)G_3}{2\left(2G_1+3\right)G_2}\pm \sqrt{\frac{\left(G_1+2\right)}{2G_2^2}\left(\frac{3\left(G_1+2\right)G_3^2}{\left(2G_1+3\right){}^2}+\frac{2G_2{G}_4}{G_1+1}\right)}\hfill \\ & & \times \csc \left[\sqrt{\frac{G_4}{G_1+1}-\frac{3\left(G_1+2\right)G_3^2}{2\left(2G_1+3\right){}^2{G}_2}}(x-vt)\right]{\}}^{\frac{1}{2n}}{e}^{i(-\kappa x+\omega t+\vartheta )}.\hfill \end{array}$$

(41)

The bright and dark solitons obtained for the governing model help to investigate the soliton dynamics in long-distance telecommunication systems applied to fiber optic communication technology and non-linear optics, as well as in other contexts wherever CGLE is considered.

4. Conclusions

This study recovered optical solitons with the complex Ginzburg–Landau equation with Kudryashov’s law of refractive index via an improved modified, extended tanh–function algorithm. The obtained solitons are bright and dark solitons, as well as singular solitons. As well as these solitons, other solutions in terms of Jacobi’s elliptic functions are obtained. These solutions recover solitons and periodic wave solutions when the modulus of ellipticity approaches unity or zero. Wave profile plots for soliton solutions (17) and (20) are given to provide physical illustrations; Figure 1 and Figure 2. Because of the importance of the CGL equation in the telecommunications industry and since it can be used to describe the propagation of pulses in a non-linear optical medium, the obtained solitons are significantly more valuable and effective in understanding various critical and complex physical phenomena. Therefore, the findings of the study will be released in stages. The influence of Kudryashov’s arbitrary refractive index is the main issue, in addition to obtaining numerous soliton types. The novelty of this investigation lies in the fact that this method is applied to a new equation, which was presented in [24]. The obtained solutions are new and original and have not previously been published in the literature. We hope that the approach and results will help in understanding the dynamics of physical behaviors and assist researchers in finding solutions to this and other non-linear problems.