Study on Vibration Friction Reducing Mechanism of Materials
Abstract
:1. Introduction
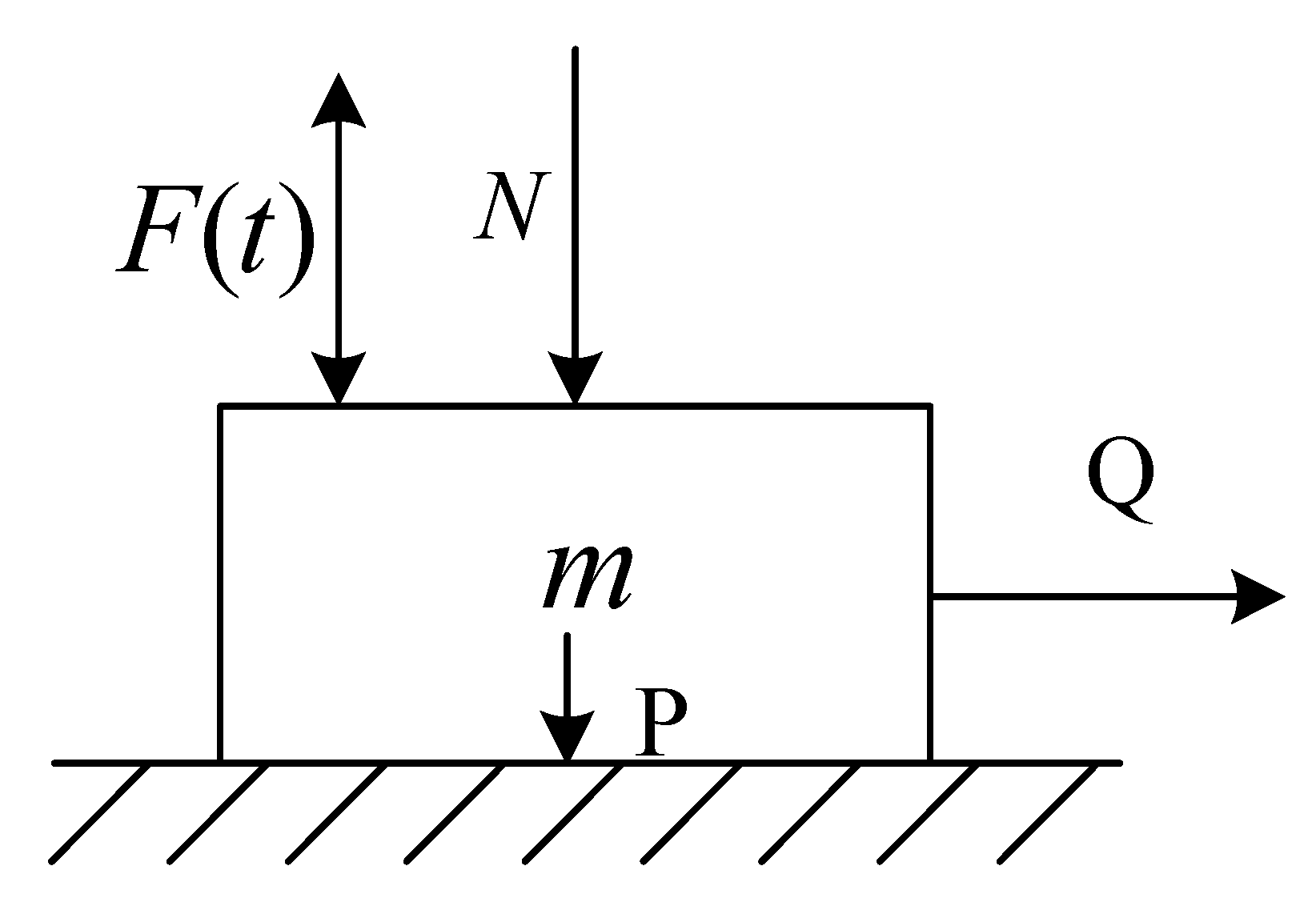
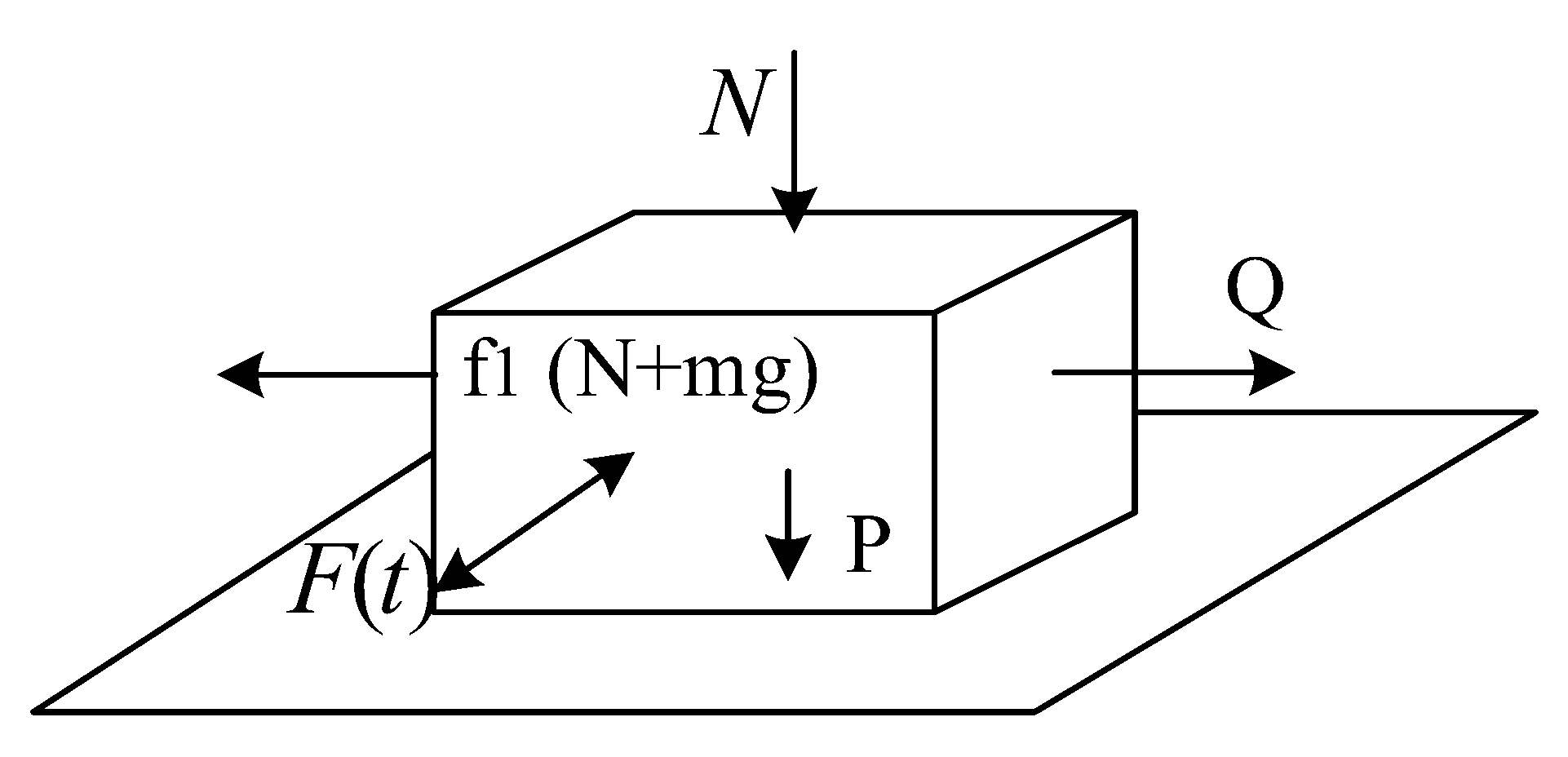
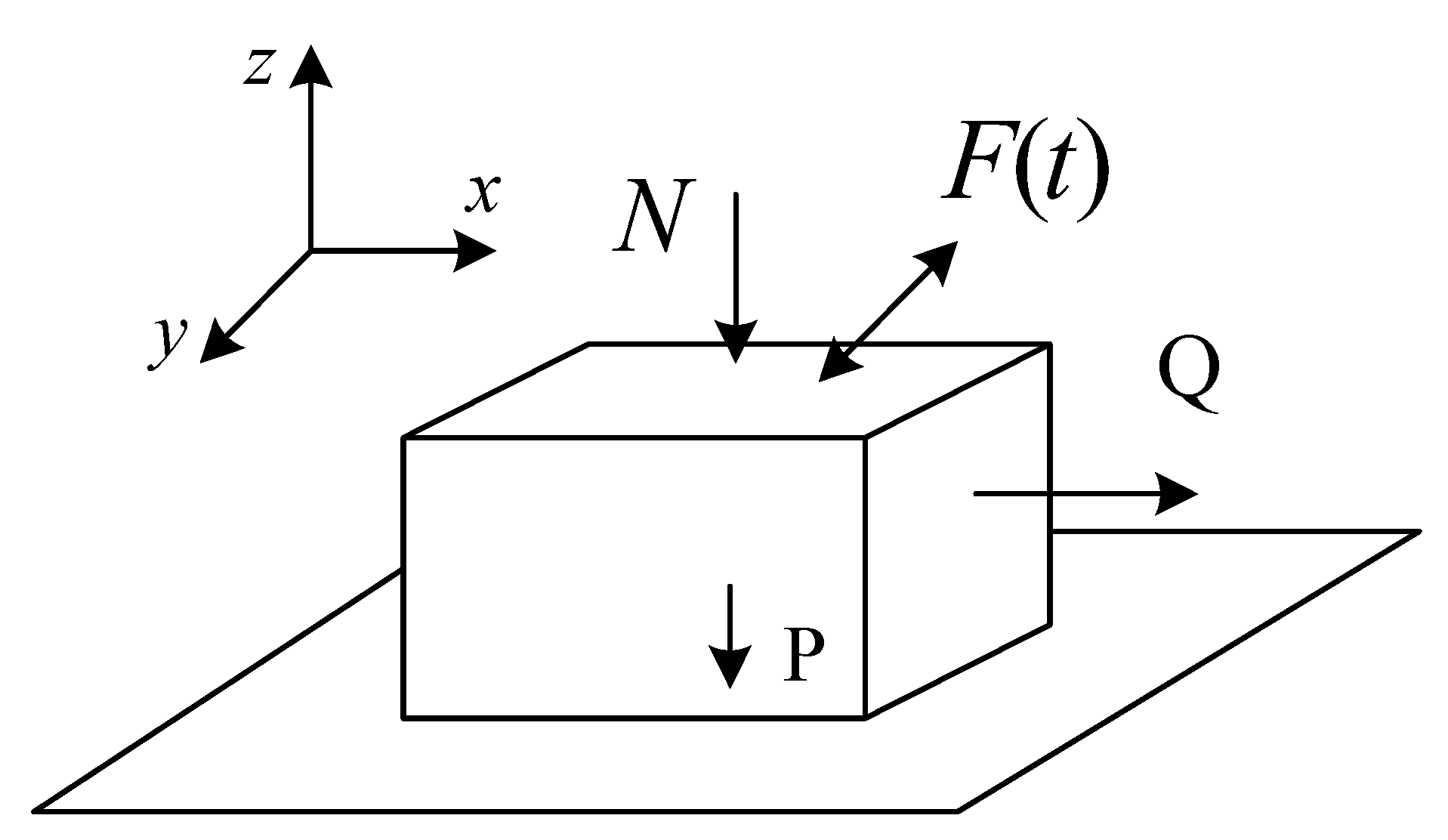
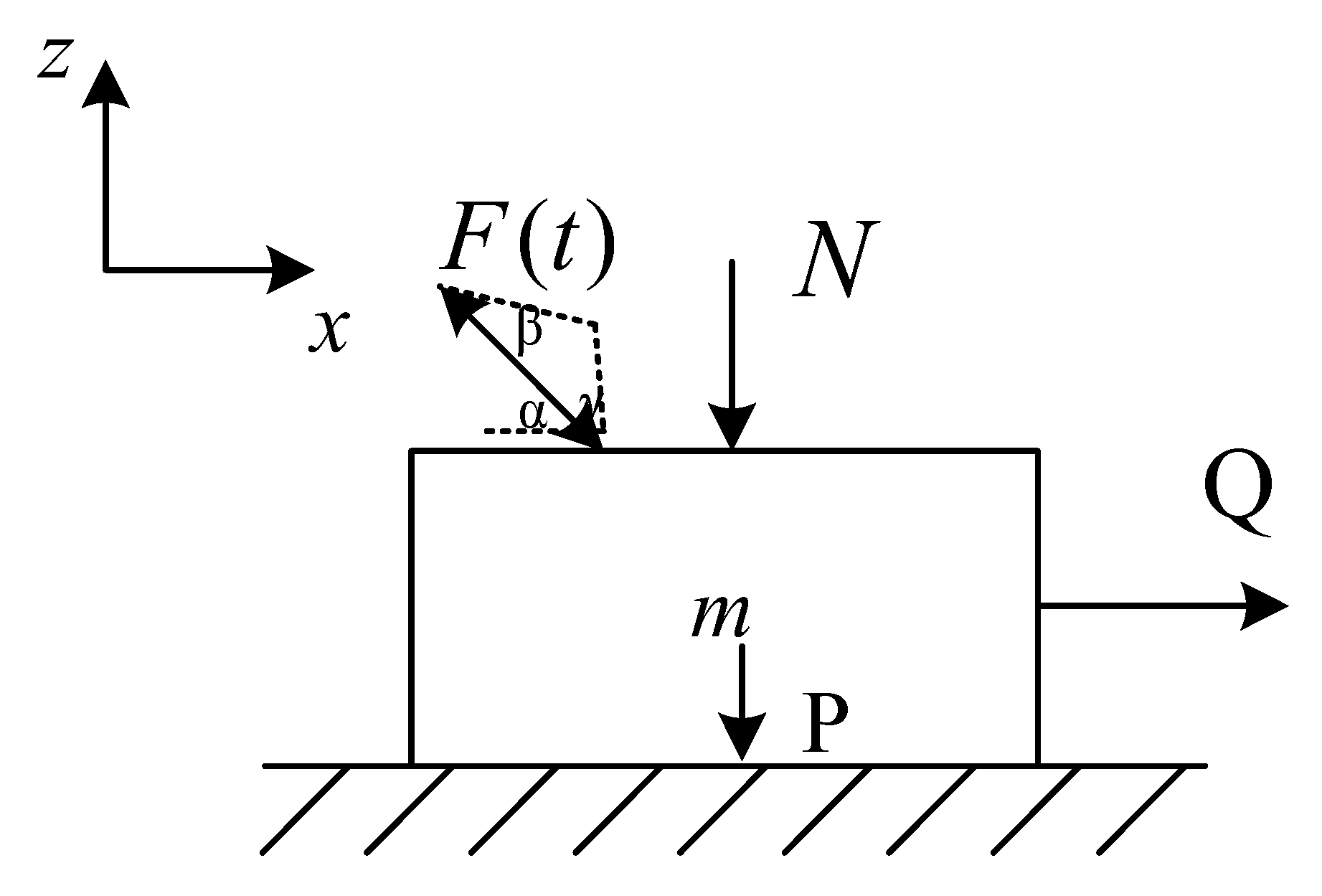
2. Model of Material
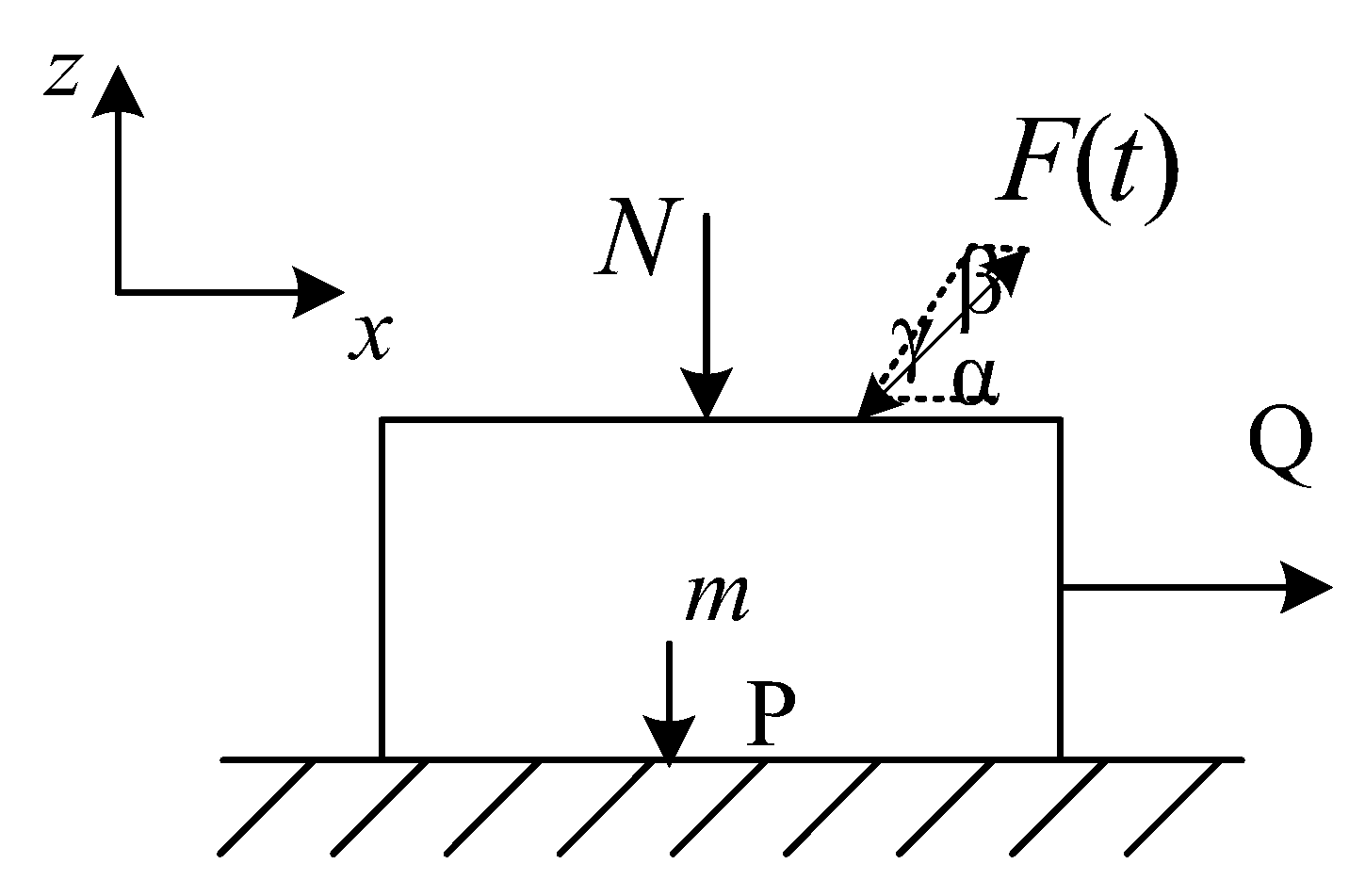
3. Effective Coefficient of Friction
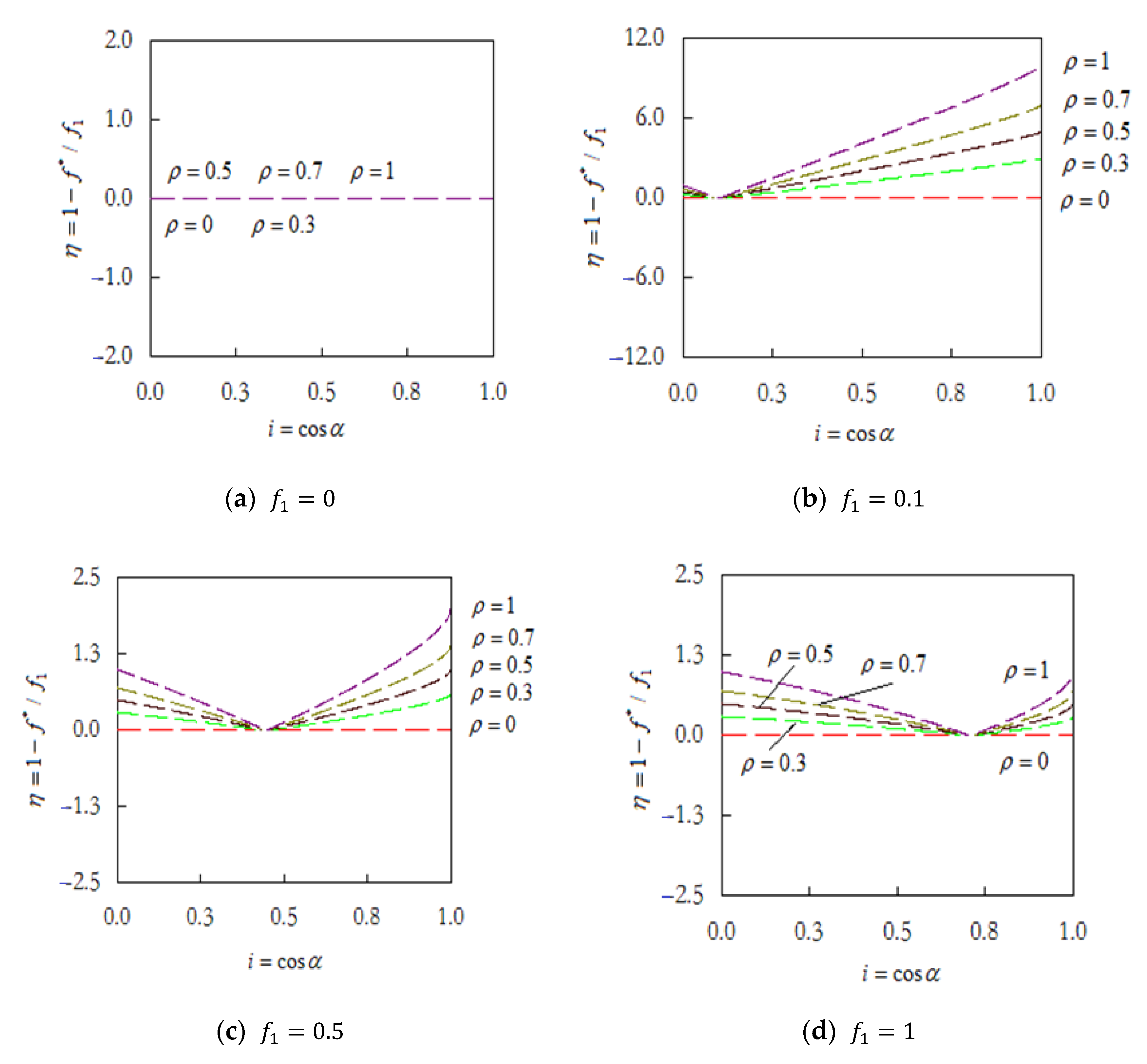
4. Negative Gradient of the Effective Friction Coefficient
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Pérez-Aracil, J.; Camacho-Gómez, C.; Pereira, E.; Vaziri, V.; Aphale, S.S.; Salcedo-Sanz, S. Eliminating Stick-Slip Vibrations in Drill-Strings witha Dual-Loop Control Strategy Optimised by the CRO-SL Algorithm. Mathmatics 2021, 9, 1526. [Google Scholar] [CrossRef]
- Sun, X.Q.; Wang, T.; Zhang, R.L.; Gu, F.S.; Ball, A.D. Numerical Modelling of Vibration Responses of Helical Gears under Progressive Tooth Wear for Condition Monitoring. Mathematics 2021, 9, 213. [Google Scholar] [CrossRef]
- Wu, J.T.; Yang, Y.; Yang, X.K.; Cheng, J.S. Fault feature analysis of cracked gear based on LOD and analytical-FE method. Mech. Syst. Signal Pr. 2018, 98, 951–967. [Google Scholar] [CrossRef]
- Sugiura, J.; Jones, S. Real-time stick-slip and vibration detection for 8 1/2”-hole-size rotary steerable tools in deeper wells and more aggressive drilling. In Proceedings of the AADE National Technical Conference, Houston, TX, USA, 10–12 April 2007. [Google Scholar]
- Robnett, E.; Hood, J.; Heisig, G.; Macpherson, J. Analysis of the stick-slip phenomenon using downhole drill string rotation data. In Proceedings of the SPE/IADC Drilling Conference, Amsterdam, The Netherlands, 9–11 March 1999. [Google Scholar]
- Baradaran-Nia, M.; Afizadeh, G.; Khanmohammadi, S.; Azar, B.F. Optimal sliding mode control of single degree-of-freedom hysteretic structural system. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 4455–4466. [Google Scholar] [CrossRef]
- Fu, X.; Ai, H.; Li, C. Repetitive Learning Sliding Mode Stabilization Control for a Flexible-Base, Flexible-Link and Flexible-Joint Space Robot Capturing a Satellite. Appl. Sci. 2021, 11, 8077. [Google Scholar] [CrossRef]
- Kumar, V.C.; Hutchings, I.M. Reduction of the sliding friction of metals by the application of longitudinal or transverse ultrasonic vibration. Tribol. Int. 2004, 37, 833–840. [Google Scholar] [CrossRef]
- Adachi, K.; Kato, K.; Sasatani, Y. The micro-mechanism of friction drive with ultrasonic wave. Wear 1996, 194, 137–142. [Google Scholar] [CrossRef]
- Tucker, W.; Wang, C. On the effective control of torsional vibrations in drilling systems. J. Sound Vib. 1999, 224, 101–122. [Google Scholar] [CrossRef]
- Carducci, G.; Giannoccaro, N.I.; Messina, A.; Rollo, G. Identification of viscous friction coefficients for a pneumatic system model using optimization methods. Math. Comput. Simul. 2006, 71, 385–394. [Google Scholar] [CrossRef]
- Tsai, C.C.; Tseng, C.H. The effect of friction reduction in the presence of in-plane vibrations. Arch. Appl. Mech. 2006, 75, 164–176. [Google Scholar] [CrossRef]
- Bao, H.; Huang, W.; Lu, F. Investigation of engagement characteristics of a multi-disc wet friction clutch. Tribol. Int. 2021, 159, 106940. [Google Scholar]
- Jia, S.H. The effective friction coefficient under the vibration. J. Tsinghua Univ. 1959, 5, 165–170. [Google Scholar]
- Jin, X.; Xu, H.; Wang, Y.; Huang, Z. Approximately analytical procedure to evaluate random stick-slip vibration of Duffing system including dry friction. J. Sound Vib. 2019, 443, 520–536. [Google Scholar] [CrossRef]
- Marino, L.; Cicirello, A. Experimental investigation of a single-degree-of-freedom system with Coulomb friction. Nonlinear Dyn. 2020, 99, 1781–1799. [Google Scholar] [CrossRef]
- Wang, D.W.; Mo, J.L.; Wang, Z.G.; Chen, G.X.; Ouyang, H.; Zhou, Z.R. Numerical study of friction-induced vibration and noise on groove-textured surface. Tribol. Int. 2013, 64, 1–7. [Google Scholar] [CrossRef]
- Wang, X.C.; Huang, B.; Wang, R.L.; Mo, J.L.; Ouyang, H. Friction-induced stick-slip vibration and its experimental validation. Mech. Syst. Signal Process. 2020, 142, 106705. [Google Scholar] [CrossRef]
- Sun, G.D.; Zhu, H.; Ding, C. Using Recurrence Plots for Stability Analysis of Ring-on-Disc Tribopairs. Ind. Lubr. Tribol. 2019, 71, 532–539. [Google Scholar] [CrossRef]
- Sun, G.D.; Zhu, H.; Ding, C.; Jiang, Y.; Wei, C.L. On the Boundedness of Running-In Attractors Based on Recurrence Plot and Recurrence Qualification Analysis. Friction 2019, 7, 432–443. [Google Scholar] [CrossRef]
- Liu, Y.; Pavlovskaia, E.; Hendry, D.; Wiercigroch, M. Vibro-impact responses of capsule system with various friction models. Int. J. Mech. Sci. 2013, 72, 39–54. [Google Scholar] [CrossRef]
- Marques, F.; Flores, P.; Pimenta Claro, J.C.; Lankarani, H.M. A survey and comparison of several friction force models for dynamic analysis of multibody mechanical systems. Nonlinear Dyn. 2016, 86, 1407–1443. [Google Scholar] [CrossRef]
- Mostaghel, N. A non-standard analysis approach to systems involving friction. J. Sound Vib. 2005, 284, 583–595. [Google Scholar] [CrossRef]
- Rusinek, R.; Wiercigroch, M.; Wahi, P. Modelling of frictional chatter in metal cutting. Int. J. Mech. Sci. 2014, 89, 167–176. [Google Scholar] [CrossRef]
- Saha, A.; Wiercigroch, M.; Jankowski, K.; Wahi, P.; Stefański, A. Investigation of two different friction models from the perspective of friction-induced vibrations. Tribol. Int. 2015, 90, 185–197. [Google Scholar] [CrossRef]
- Yan, Y.; Liu, G.; Wiercigroch, M.; Xu, J. Safety estimation for a new model of regenerative and frictional cutting dynamics. Int. J. Mech. Sci. 2021, 201, 106468. [Google Scholar] [CrossRef]
- Yoo, S.S.; Kim, D.E. Effects of vibration frequency and amplitude on friction reduction and wear characteristics of silicon. Tribol. Int. 2016, 16, 198–206. [Google Scholar] [CrossRef]
- Safaei, B. Frequency-dependent damped vibrations of multifunctional foam plates sandwiched and integrated by composite faces. Eur. Phys. J. Plus 2021, 136, 646. [Google Scholar] [CrossRef]
- Duc, D.H.; Thom, D.V.; Cong, P.H.; Minh, P.V.; Nguyen, N.X. Vibration and static buckling behavior of variable thickness flexoelectric nanoplates. Mech. Based Des. Struct. Mach. 2022, 8, 1–29. [Google Scholar] [CrossRef]
- Dung, N.T.; Van Ke, T.; Huyen, T.T.H.; Van Minh, P. Nonlinear static bending analysis of microplates resting on imperfect two-parameter elastic foundations using modified couple stress theory. Comptes Rendus Mec. 2022, 350, 121–141. [Google Scholar] [CrossRef]
- Tho, N.C.; Ta, N.T.; Thom, D.V. New Numerical Results from Simulations of Beams and Space Frame Systems with a Tuned Mass Damper. Materials 2019, 12, 1329. [Google Scholar] [CrossRef] [PubMed]
- Han, S.Y.; Narasimhan, M.N.L.; Kennedy, T.C. Finite crack propagation in a micropolar elastic solid. KSME J. 1989, 3, 103. [Google Scholar] [CrossRef]
- Kumar, R.; Singh, R.; Chadha, T.K. Eigen value approach to second dynamic problem of micropolar elastic solid. Indian J. Pure Appl. Math. 2003, 34, 743–754. [Google Scholar]
- Tuan, L.T.; Dung, N.T.; Van Thom, D.; Van Minh, P.; Zenkour, A.M. Propagation of non-stationary kinematic disturbances from a spherical cavity in the pseudo-elastic cosserat medium. Eur. Phys. J. Plus 2021, 136, 1199. [Google Scholar] [CrossRef]
- Tran, T.T.; Tran, V.K.; Le, P.B.; Phung, V.M.; Do, V.T.; Nguyen, H.N. Forced vibration analysis of laminated composite shells reinforced with graphene nanoplatelets using finite element method. Adv. Civ. Eng. 2020, 2020, 1471037. [Google Scholar] [CrossRef]
- Nam, V.H.; Nam, N.H.; Vinh, P.V.; Khoa, D.N.; Thom, D.V.; Minh, P.V. A new efficient modified first-order shear model for static bending and vibration behaviors of two-layer composite plate. Adv. Civ. Eng. 2019, 2019, 6814367. [Google Scholar] [CrossRef]
- van Thom, D.; Duc, D.H.; van Minh, P.; Tung, N.S. Finite element modelling for free vibration response of cracked stiffened fgm plates. Vietnam J. Sci. Technol. 2020, 58, 119. [Google Scholar] [CrossRef]
- Tho, N.C.; Thanh, N.T.; Tho, T.D.; van Minh, P.; Hoa, L.K. Modelling of the flexoelectric effect on rotating nanobeams with geometrical imperfection. J. Braz. Soc. Mech. Sci. Eng. 2021, 43, 510. [Google Scholar] [CrossRef]
- Dung, N.T.; van Minh, P.; Hung, H.M.; Tien, D.M. The third-order shear deformation theory for modelling the static bending and dynamic responses of piezoelectric bidirectional functionally graded plates. Adv. Mater. Sci. Eng. 2021, 2021, 5520240. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Teng, Y.; Wen, Q.; Xie, L.; Wen, B. Study on Vibration Friction Reducing Mechanism of Materials. Mathematics 2022, 10, 3529. https://doi.org/10.3390/math10193529
Teng Y, Wen Q, Xie L, Wen B. Study on Vibration Friction Reducing Mechanism of Materials. Mathematics. 2022; 10(19):3529. https://doi.org/10.3390/math10193529
Chicago/Turabian StyleTeng, Yunnan, Quan Wen, Liyang Xie, and Bangchun Wen. 2022. "Study on Vibration Friction Reducing Mechanism of Materials" Mathematics 10, no. 19: 3529. https://doi.org/10.3390/math10193529
APA StyleTeng, Y., Wen, Q., Xie, L., & Wen, B. (2022). Study on Vibration Friction Reducing Mechanism of Materials. Mathematics, 10(19), 3529. https://doi.org/10.3390/math10193529





