Dynamics of Heat Transfer Analysis of Convective-Radiative Fins with Variable Thermal Conductivity and Heat Generation: Differential Transformation Method
Abstract
:1. Introduction
2. Fundamental Operations of DTM
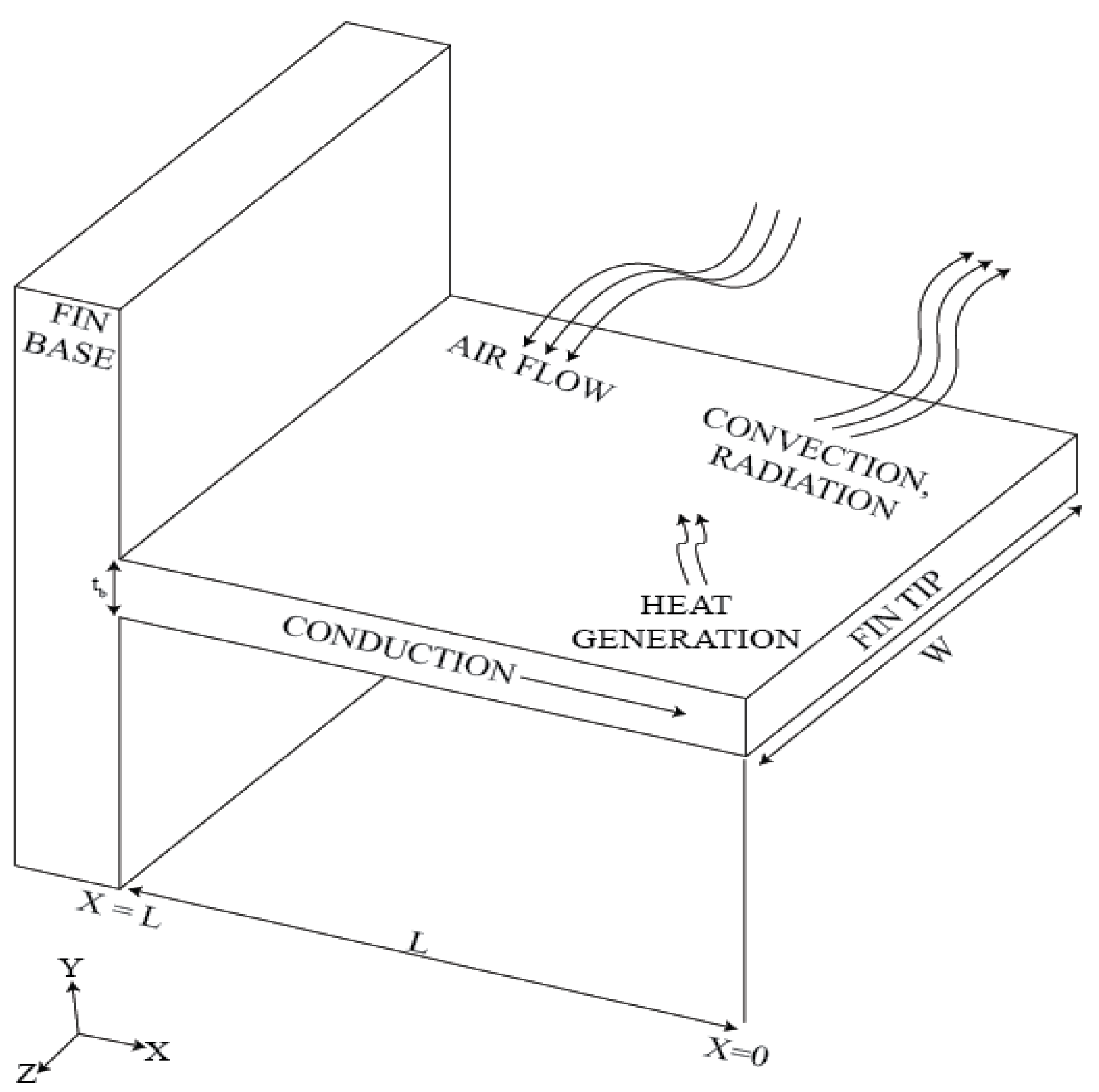
3. Mathematical Formulation
- The temperature is a function of and remains constant over time.
- The temperature variance due to fin thickness is neglected.
- The fin bed is kept at a steady temperature.
- Solid matrix and fluid are in a dynamic state of equilibrium.
- Fin is considered to be in a steady state.
- For quadrilateral fin
- For exponential fin
- For convex finDimensionless parameters are:
- For rectangular profile
- For exponential profile
- For convex profilewhere and convective environment boundary conditions are:
4. Solution Method with DTM
- For rectangular profile
- For exponential profile
- For convex profile
- For rectangular profileand so forth
- For exponential profile…and so forth
- For convex profile…and so forth
- For rectangular profile
Fin Efficiency
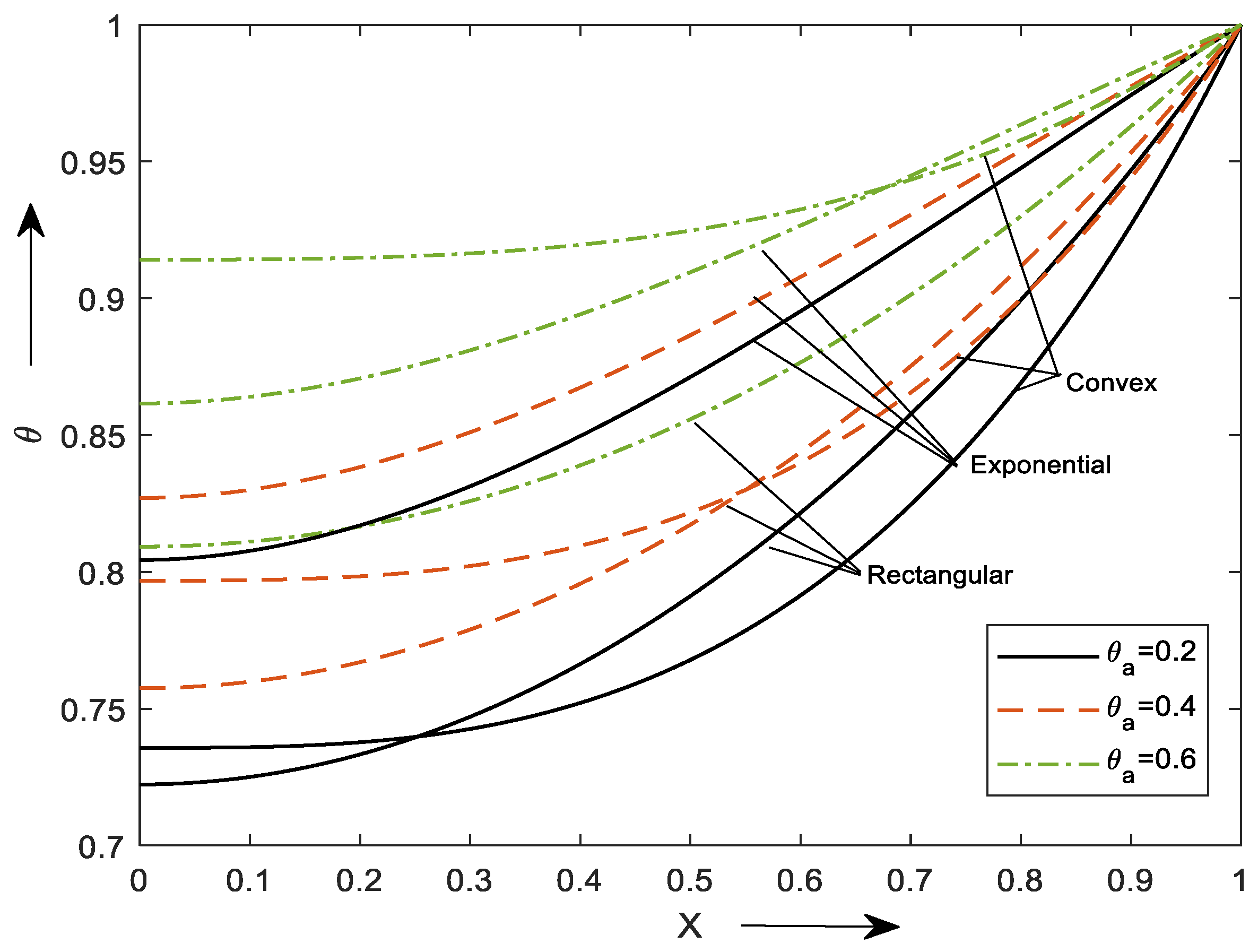
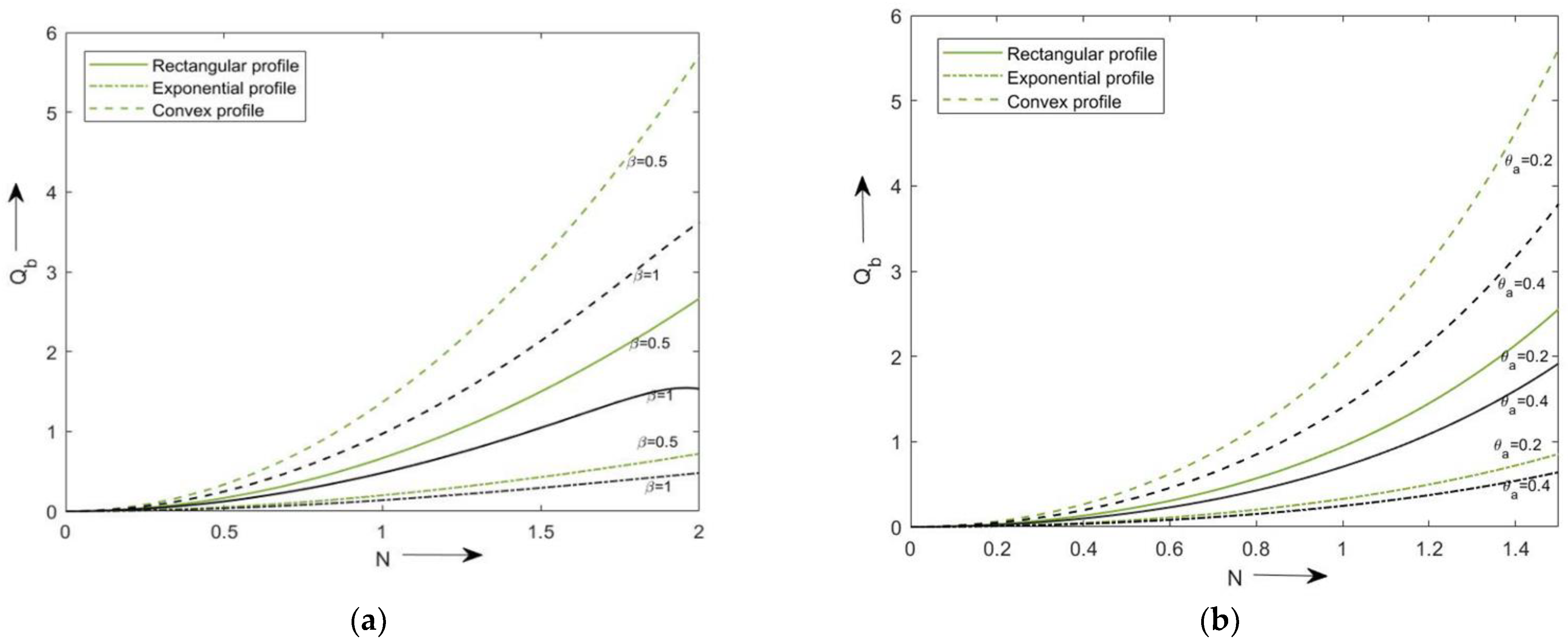
5. Results
6. Thermal Analysis
- Aluminum alloy (AA6061) is considered a fin material as it is a good thermal and electrical conductor with heat conduction of 300 W/m K.
- Heat conduction is considered 1D and longitudinal.
- h is considered to be 39.9 W/m2K above the fin surface.
- The fin base is kept at 550 K, and 283 is the ambient temperature.
7. Conclusions
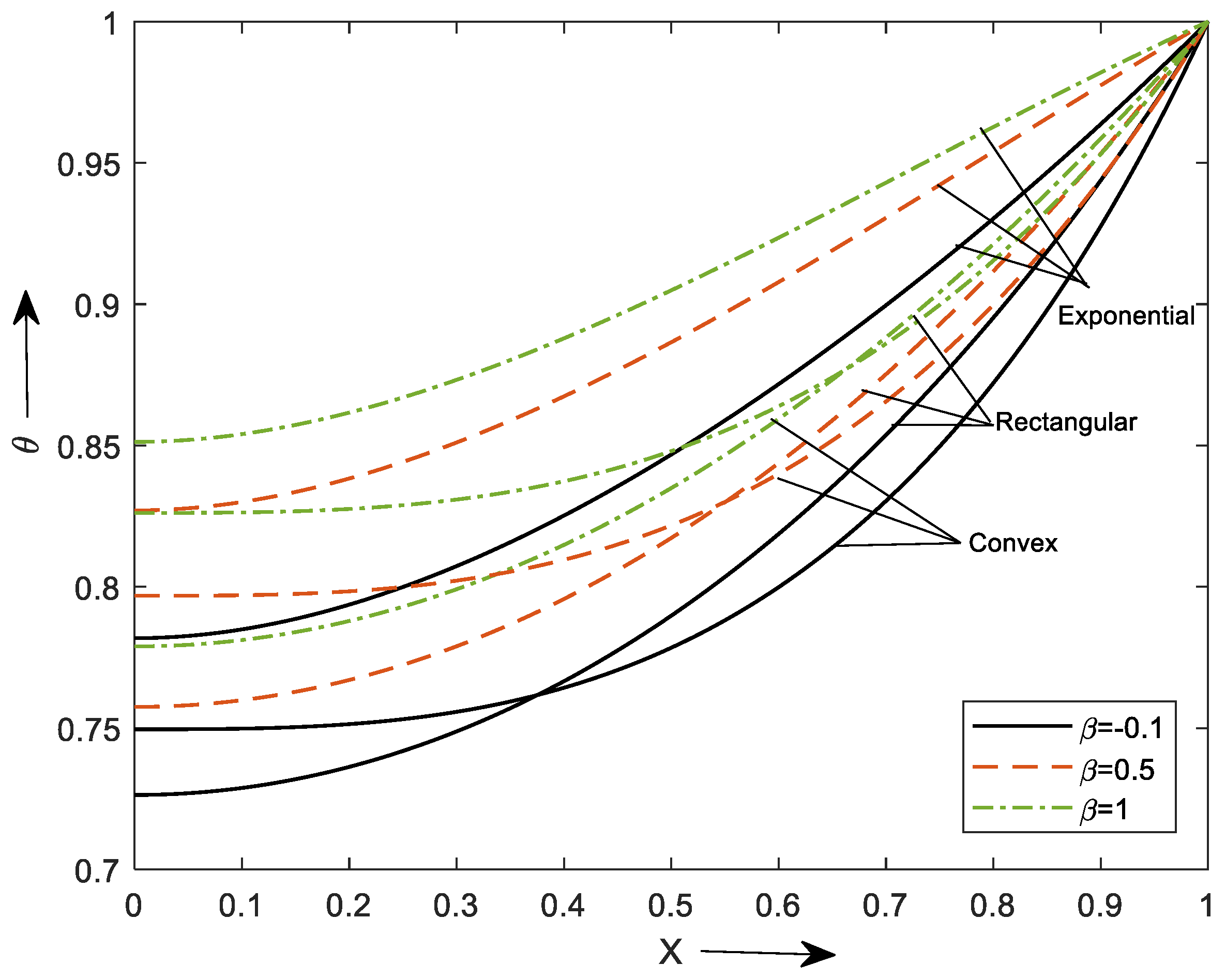
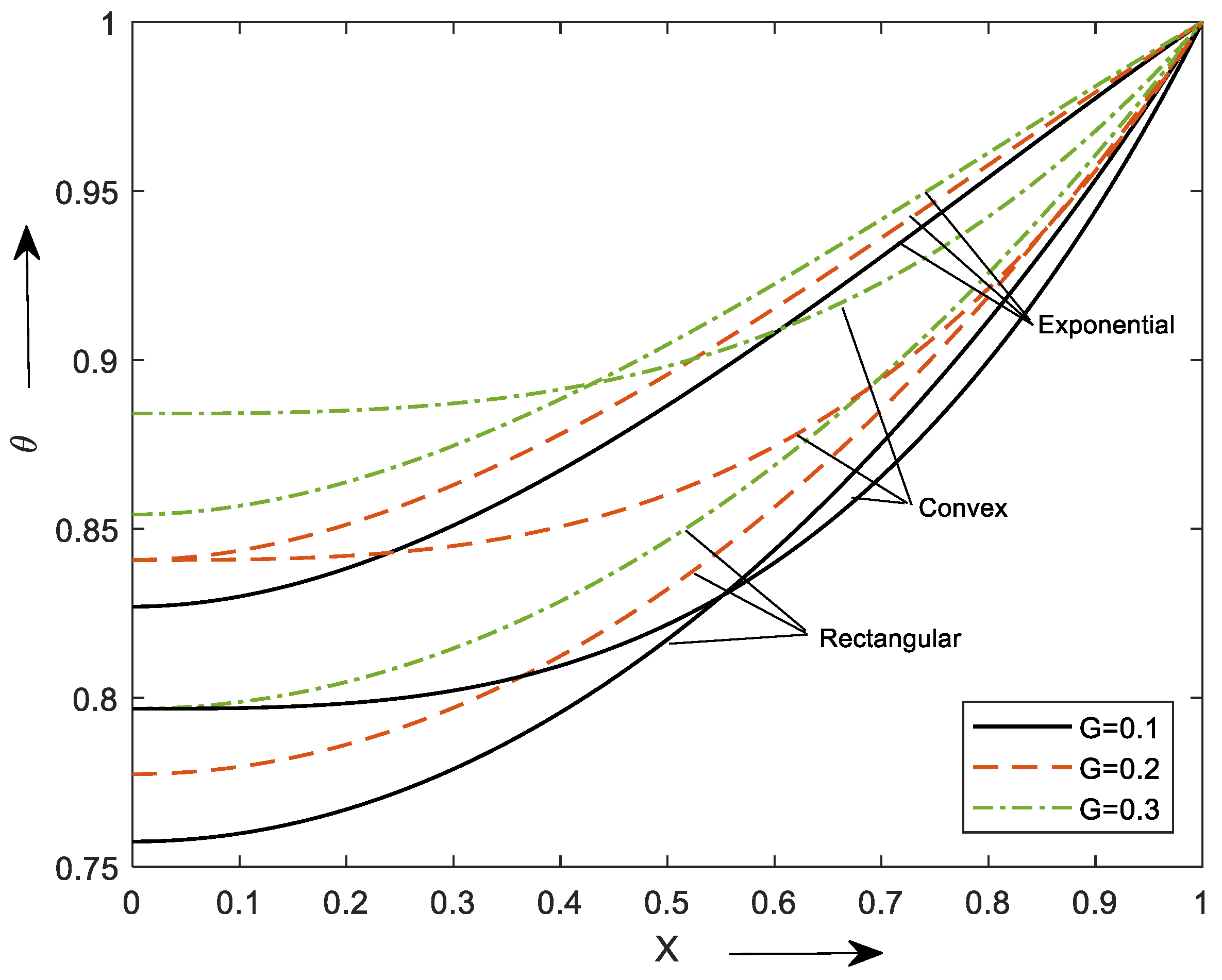
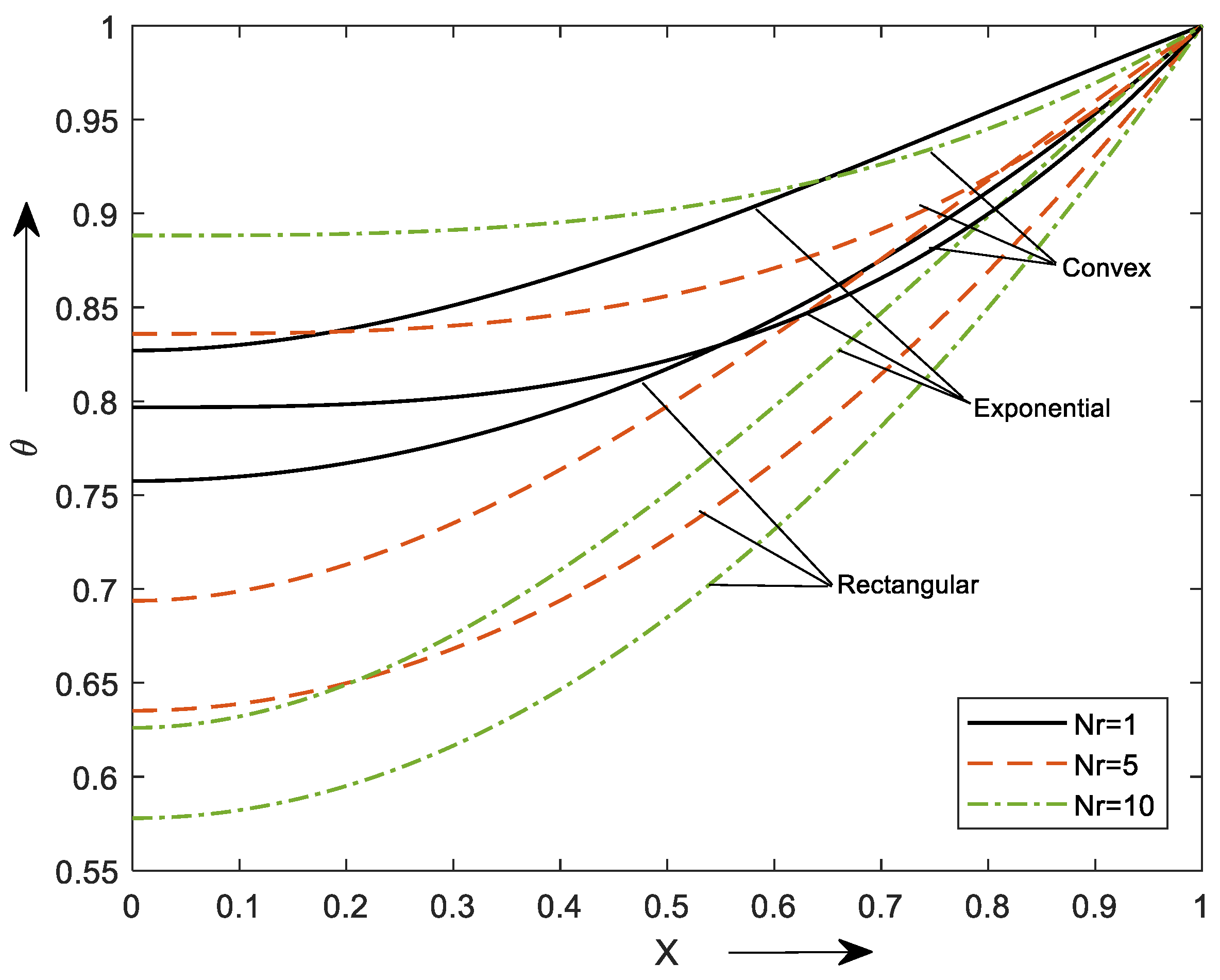
- Upon enhancing the convection–conduction parameter, the thermal dispersal in the fin lowers.
- A strengthened heat transfer fine is observed for the radiative-conduction constant.
- The thermal rate of the fin improves with an augmented change in a heat-generating parameter.
- This scrutiny convinces us that DTM algorithms are efficient and convenient methods for nonlinear differential systems.
- Thermal radiation and natural convection have a significant influence on the cooling of a fin.
- In the steady state, fins dissipate heat to the environment because heat production within a fin surges the temperature of the fins.
- The temperature scatters of a fin for different profiles are calculated using the ANSYS software, considering aluminum alloy (AA6061) as the fin body material. The fin base has a higher temperature and reduces drastically toward the fin tip.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| fin cross-section (m2) | |
| exponential parameter | |
| heat transfer coefficient (wm−1k−1) | |
| heat conduction (wm−1k−1) | |
| radiative parameter | |
| heat generation parameter | |
| fin length (m) | |
| convective parameter | |
| temperature (k) | |
| transformed function | |
| original analytic function | |
| fin base temperature | |
| thermal expansion coefficient (K−1) | |
| dimensional constant (K−1) | |
| efficiency of the fin | |
| transformed equation | |
| dimensionless temperature | |
| ambient temperature | |
| base of the fin |
References
- Kraus, A.D.; Aziz, A.; Welty, J.R. Extended Surface Heat Transfer; John Wiley: Hoboken, NJ, USA, 2002. [Google Scholar]
- Gireesha, B.J.; Sowmya, G. Heat transfer analysis of an inclined porous fin using Differential Transform Method. Int. J. Ambient Energy 2022, 43, 3189–3195. [Google Scholar] [CrossRef]
- Oleśkowicz-Popiel, C.; Blanch, R.O.; Wojtkowiak, J. Efficiency of the horizontal single pin fin subjected to free convection and radiation heat transfer. Heat Transf. Eng. 2007, 28, 299–309. [Google Scholar] [CrossRef]
- Minkler, W.S.; Rouleau, W.T. The Effects of Internal Heat Generation on Heat Transfer in Thin Fins. Nucl. Sci. Eng. 1960, 7, 400–406. [Google Scholar] [CrossRef]
- Venkitesh, V.; Mallick, A. Thermal analysis of a convective–conductive–radiative annular porous fin with variable thermal parameters and internal heat generation. J. Anal. Calorim. 2022, 147, 1519–1533. [Google Scholar] [CrossRef]
- Majhi, T.; Kundu, B. New Approach for Determining Fin Performances of an Annular Disc Fin with Internal Heat Generation. In Advances in Mechanical Engineering; Springer: Singapore, 2020; pp. 1033–1043. [Google Scholar]
- Das, R.; Kundu, B. Prediction of Heat-Generation and Electromagnetic Parameters from Temperature Response in Porous Fins. J. Thermophys. Heat Transf. 2021, 35, 761–769. [Google Scholar] [CrossRef]
- Sobamowo, M.G. Analysis of convective longitudinal fin with temperature-dependent thermal conductivity and internal heat generation. Alex. Eng. J. 2017, 56, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Turkyilmazoglu, M. Exact heat-transfer solutions to radial fins of general profile. J. Heat Trans. 2016, 30, 89–93. [Google Scholar] [CrossRef]
- Ndlovu, P.L.; Moitsheki, R.J. Steady state heat transfer analysis in a rectangular moving porous fin. Propuls. Power Res. 2020, 9, 188–196. [Google Scholar] [CrossRef]
- Singla, R.K.; Das, R. Application of decomposition method and inverse prediction of parameters in a moving fin. Energy Convers. Manag. 2014, 84, 268–281. [Google Scholar] [CrossRef]
- Shah, N.A.; Wakif, A.; El-Zahar, E.R.; Ahmad, S.; Yook, S.-J. Numerical simulation of a thermally enhanced EMHD flow of a heterogeneous micropolar mixture comprising (60%)-ethylene glycol (EG), (40%)-water (W), and copper oxide nanomaterials (CuO). Case Stud. Therm. Eng. 2022, 35, 102046. [Google Scholar] [CrossRef]
- Sajjan, K.; Shah, N.A.; Ahammad, N.A.; Raju, C.S.K.; Kumar, M.D.; Weera, W. Nonlinear Boussinesq and Rosseland approximations on 3D flow in an interruption of Ternary nanoparticles with various shapes of densities and conductivity properties. AIMS Math. 2022, 7, 18416–18449. [Google Scholar] [CrossRef]
- Aziz, A.; Khani, F. Convection-radiation from a continuously moving fin of variable thermal conductivity. J. Frankl. Inst. 2011, 348, 640–651. [Google Scholar] [CrossRef]
- Sowmya, G.; Sarris, I.E.; Vishalakshi, C.s.; Kumar, R.S.V.; Prasannakumara, B.C. Analysis of transient thermal distribution in a convective–radiative moving rod using two-dimensional differential transform method with MULTIVARIATE pade approximant. Symmetry 2021, 13, 1793. [Google Scholar] [CrossRef]
- Hatami, M.; Ganji, D.D. Thermal performance of circular convective-radiative porous fins with different section shapes and materials. Energy Convers. Manag. 2013, 76, 185–193. [Google Scholar] [CrossRef]
- Pasha, A.v.; Jalili, P.; Ganji, D.D. Analysis of unsteady heat transfer of specific longitudinal fins with Temperature-dependent thermal coefficients by DTM. Alex. Eng. J. 2018, 57, 3509–3521. [Google Scholar] [CrossRef]
- Torabi, M.; Aziz, A.; Zhang, K. A comparative study of longitudinal fins of rectangular, trapezoidal and concave parabolic profiles with multiple nonlinearities. Energy 2013, 51, 243–256. [Google Scholar] [CrossRef]
- Zhang, H.; Sun, K. Conduction from longitudinal fin of rectangular profile with exponential vary heat transfer coefficient. Adv. Mater. Res. 2013, 614–615, 311–314. [Google Scholar] [CrossRef]
- Buikis, A.; Pagodkina, I. Comparison of Analytical and Numerical Solutions for a Two-Dimensinal Longitudinal Fin of Rectangular Profile. Latv. J. Phys. Technol. Sci. 1996, 33, 177–187. [Google Scholar]
- Kezzar, M.; Tabet, I.; Eid, M.R. A new analytical solution of longitudinal fin with variable heat generation and thermal conductivity using DRA. Eur. Phys. J. Plus 2020, 135, 120. [Google Scholar] [CrossRef]
- Shi, Q.H.; Hamid, A.; Khan, M.I.; Naveen Kumar, R.; Punith Gowda, R.J.; Prasannakumara, B.C.; Shah, N.A.; Khan, S.U.; Chung, J.D. Numerical study of bio-convection flow of magneto-cross nanofluid containing gyrotactic microorganisms with activation energy. Sci Rep. 2021, 11, 16030. [Google Scholar] [CrossRef] [PubMed]
- Afsharpanah, F.; Ajarostaghi, S.S.M.; Arıcı, M. Parametric study of phase change time reduction in a shell-and-tube ice storage system with anchor-type fin design. Int. Commun. Heat Mass Transf. 2022, 137, 106281. [Google Scholar] [CrossRef]
- Afsharpanah, F.; Cheraghian, G.; Hamedani, F.A.; Shokri, E.; Soheil, S.; Ajarostaghi, M. Utilization of Carbon-Based Nanomaterials and Plate-Fin Networks in a Cold PCM Container with Application in Air Conditioning of Buildings. Nanomaterials 2022, 12, 1927. [Google Scholar] [CrossRef] [PubMed]
- Sabu, A.S.; Wakif, A.; Areekara, S.; Mathew, A.; Shah, N.A. Significance of nanoparticles’ shape and thermo-hydrodynamic slip constraints on MHD alumina-water nanoliquid flows over a rotating heated disk: The passive control approach. Int. Commun. Heat and Mass Transf. 2021, 129, 105711. [Google Scholar] [CrossRef]
- Salhi, J.E.; Ajarostaghi, S.S.M.; Zarrouk, T.; Pour, M.S.; Salhi, N.; Salhi, M. Turbulence and thermo-flow behavior of air in a rectangular channel with partially inclined baffles. Energy Sci. Eng. 2022, 10, 3540–3558. [Google Scholar] [CrossRef]
- Demartini, L.C.; Vielmo, H.A.; Möller, S.v. Numeric and Experimental Analysis of the Turbulent Flow through Channel With Baffle Plates. J. Braz. Soc. Mech. Sci. Eng. 2004, 28, 233–241. [Google Scholar] [CrossRef] [Green Version]
- Kadijani, O.N.; Moghadam, H.K.; Ajarostaghi, S.S.M.; Asadi, A.; Pour, M.S. Hydrothermal performance of humid air flow in a rectangular solar air heater equipped with V-shaped ribs. Energy Sci. Eng. 2022, 10, 2276–2289. [Google Scholar] [CrossRef]
- Zhou, J.K. Differential Transformation Method and Its Application for Electrical Circuits; Hauzhang University Press: Wuhan, China, 1986. [Google Scholar]
- Ayaz, F. Solutions of the system of differential equations by differential transform method. Appl. Math. Comput. 2004, 147, 547–567. [Google Scholar] [CrossRef]
- Chen, C.K.; Ho, S.H. Solving partial differential equations by two-dimensional differential transform method. Appl. Math. Comput. 1999, 106, 171–179. [Google Scholar]
- Ayaz, F. On the two-dimensional differential transform method. Appl. Math. Comput. 2003, 143, 361–374. [Google Scholar] [CrossRef]
- Kanth, A.S.V.R.; Aruna, K. Differential transform method for solving linear and non-linear systems of partial differential equations. Phys. Lett. Sect. A Gen. At. Solid State Phys. 2008, 372, 6896–6898. [Google Scholar] [CrossRef]
- Fallo, N.; Moitsheki, R.J.; Makinde, O.D. Analysis of heat transfer in a cylindrical spine fin with variable thermal properties. Defect Diffus. Forum 2018, 387, 10–22. [Google Scholar] [CrossRef]
- Chiba, R. A Series Solution for Heat Conduction Problem with Phase Change in a Finite Slab. Abstr. Appl. Anal. 2014, 684293. [Google Scholar] [CrossRef]
- Moradi, A.; Hayat, T.; Alsaedi, A. Convection-radiation thermal analysis of triangular porous fins with temperature-dependent thermal conductivity by DTM. Energy Convers. Manag. 2014, 77, 70–77. [Google Scholar] [CrossRef]
- Ndlovu, P.L. Analytical study of transient heat transfer in a triangular moving porous fin with temperature dependant thermal properties. Defect Diffus. Forum 2019, 393, 31–46. Available online: www.scientific.net/DDF.393.31 (accessed on 1 August 2022). [CrossRef]
- Abbasi, H.; Javed, A. Implementation of differential transform method (DTM) for large deformation analysis of cantilever beam. IOP Conf. Ser. Mater. Sci. Eng. 2020, 899, 012003. [Google Scholar] [CrossRef]
- Aksoy, G. Application of differential transformation method for an annular fin with variable thermal conductivity. Therm. Sci. 2021, 315. [Google Scholar] [CrossRef]
- Joneidi, A.A.; Ganji, D.D.; Babaelahi, M. Differential Transformation Method to determine fin efficiency of convective straight fins with temperature dependent thermal conductivity. Int. Commun. Heat Mass Transf. 2009, 36, 757–762. [Google Scholar] [CrossRef]
- Franco, A. An analytical method for the optimum thermal design of convective longitudinal fin arrays. Heat Mass Transf. 2009, 45, 1503–1517. [Google Scholar] [CrossRef] [Green Version]
- Languri, E.M.; Ganji, D.D.; Jamshidi, N. Variational Iteration and Homotopy perturbation methods for fin efficiency of convective straight fins with temperature dependent thermal conductivity. In Proceedings of the 5th WSEAS International Conference on Fluid Mechanics (Fluids 08), Acapulco, Mexico, 25–27 January 2008; Volume 25. [Google Scholar]
- Arslanturk, C. A decomposition method for fin efficiency of convective straight fins with temperature-dependent thermal conductivity. Int. Commun. Heat Mass Transf. 2005, 32, 831–841. [Google Scholar] [CrossRef]




| Initial Function | Converted Function |
|---|---|
| HPM (Languri et al. [42]) | ADM (Arslanturk [43]) | VIM (Languri et al. [42]) | DTM (Current Study) | |
|---|---|---|---|---|
| 0 | 0.886819 | 0.886819 | 0.886819 | 0.886818 |
| 0.2 | 0.891257 | 0.891257 | 0.891257 | 0.8912567 |
| 0.4 | 0.904614 | 0.904615 | 0.904614 | 0.940614 |
| 0.6 | 0.927026 | 0.927026 | 0.927026 | 0.927027 |
| 0.8 | 0.958715 | 0.958716 | 0.958715 | 0.958715 |
| 1 | 1.000000 | 1.000000 | 1.000000 | 1.000000 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ananth Subray, P.V.; Hanumagowda, B.N.; Varma, S.V.K.; Zidan, A.M.; Kbiri Alaoui, M.; Raju, C.S.K.; Shah, N.A.; Junsawang, P. Dynamics of Heat Transfer Analysis of Convective-Radiative Fins with Variable Thermal Conductivity and Heat Generation: Differential Transformation Method. Mathematics 2022, 10, 3814. https://doi.org/10.3390/math10203814
Ananth Subray PV, Hanumagowda BN, Varma SVK, Zidan AM, Kbiri Alaoui M, Raju CSK, Shah NA, Junsawang P. Dynamics of Heat Transfer Analysis of Convective-Radiative Fins with Variable Thermal Conductivity and Heat Generation: Differential Transformation Method. Mathematics. 2022; 10(20):3814. https://doi.org/10.3390/math10203814
Chicago/Turabian StyleAnanth Subray, P. V., B. N. Hanumagowda, S. V. K. Varma, A. M. Zidan, Mohammed Kbiri Alaoui, C. S. K. Raju, Nehad Ali Shah, and Prem Junsawang. 2022. "Dynamics of Heat Transfer Analysis of Convective-Radiative Fins with Variable Thermal Conductivity and Heat Generation: Differential Transformation Method" Mathematics 10, no. 20: 3814. https://doi.org/10.3390/math10203814
APA StyleAnanth Subray, P. V., Hanumagowda, B. N., Varma, S. V. K., Zidan, A. M., Kbiri Alaoui, M., Raju, C. S. K., Shah, N. A., & Junsawang, P. (2022). Dynamics of Heat Transfer Analysis of Convective-Radiative Fins with Variable Thermal Conductivity and Heat Generation: Differential Transformation Method. Mathematics, 10(20), 3814. https://doi.org/10.3390/math10203814







