A Practical Approach to Identify the Phases Sequence in Five Phase Machines with Combined Star–Pentagon Configuration
Abstract
:1. Introduction
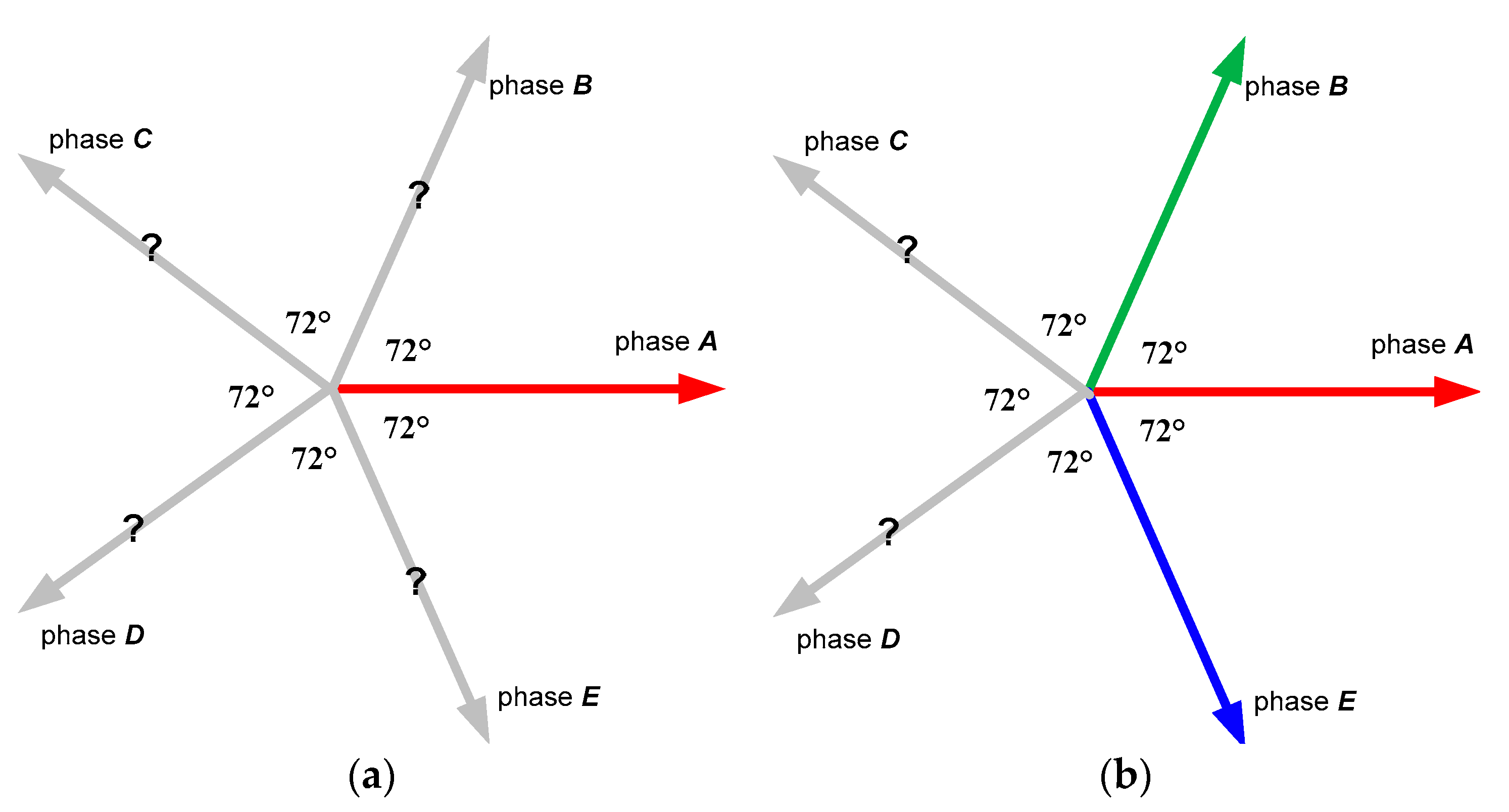
2. Proposed Method to Identify the Phase Sequence
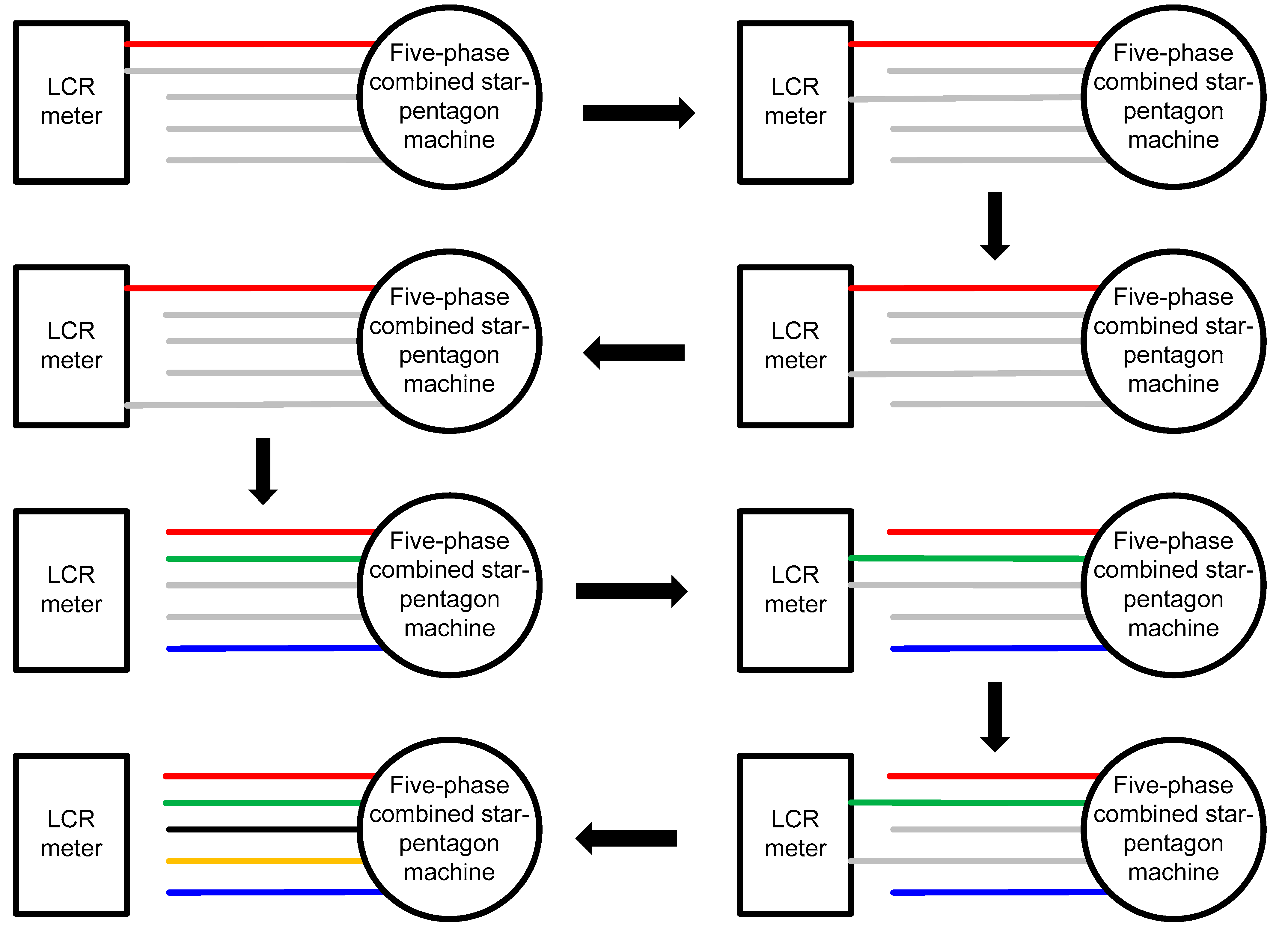
- The first terminal of the machine (consider it phase A) is connected to the positive terminal of the ohmmeter, and the negative terminal is connected to the other four terminals. The four readings of the ohmmeter will have two equal high values and two equal low values. The terminals that give the lower reading are the adjacent terminals (phases B and E) to the machine’s first terminal. Hence, the sequence of the three terminals of the five-phase machine (phases A, B and E) is known after performing the first step;
- In the second step, one of the adjacent terminals (select phase B) of the machine’s first terminal (phase A) is connected to the positive terminal of the ohmmeter, and the other terminal of the ohmmeter is connected to the other two terminals of the machine (nonadjacent terminals to the machine first terminal) (phases C and D). The terminal that gives the lower reading is the adjacent terminal (phase C) to the connected one (phase B) to the ohmmeter positive terminal. Hence, the sequence of phases is identified.
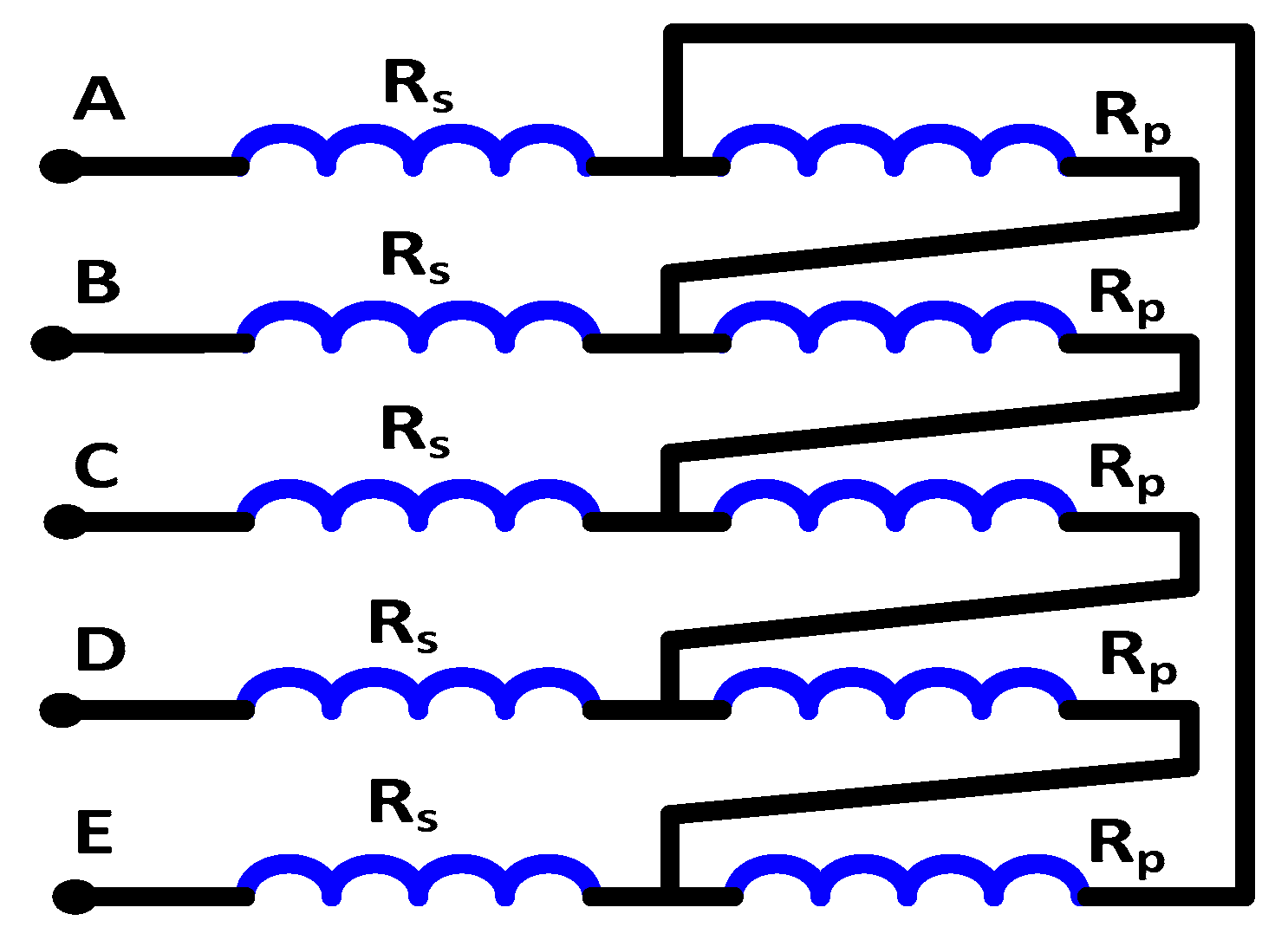
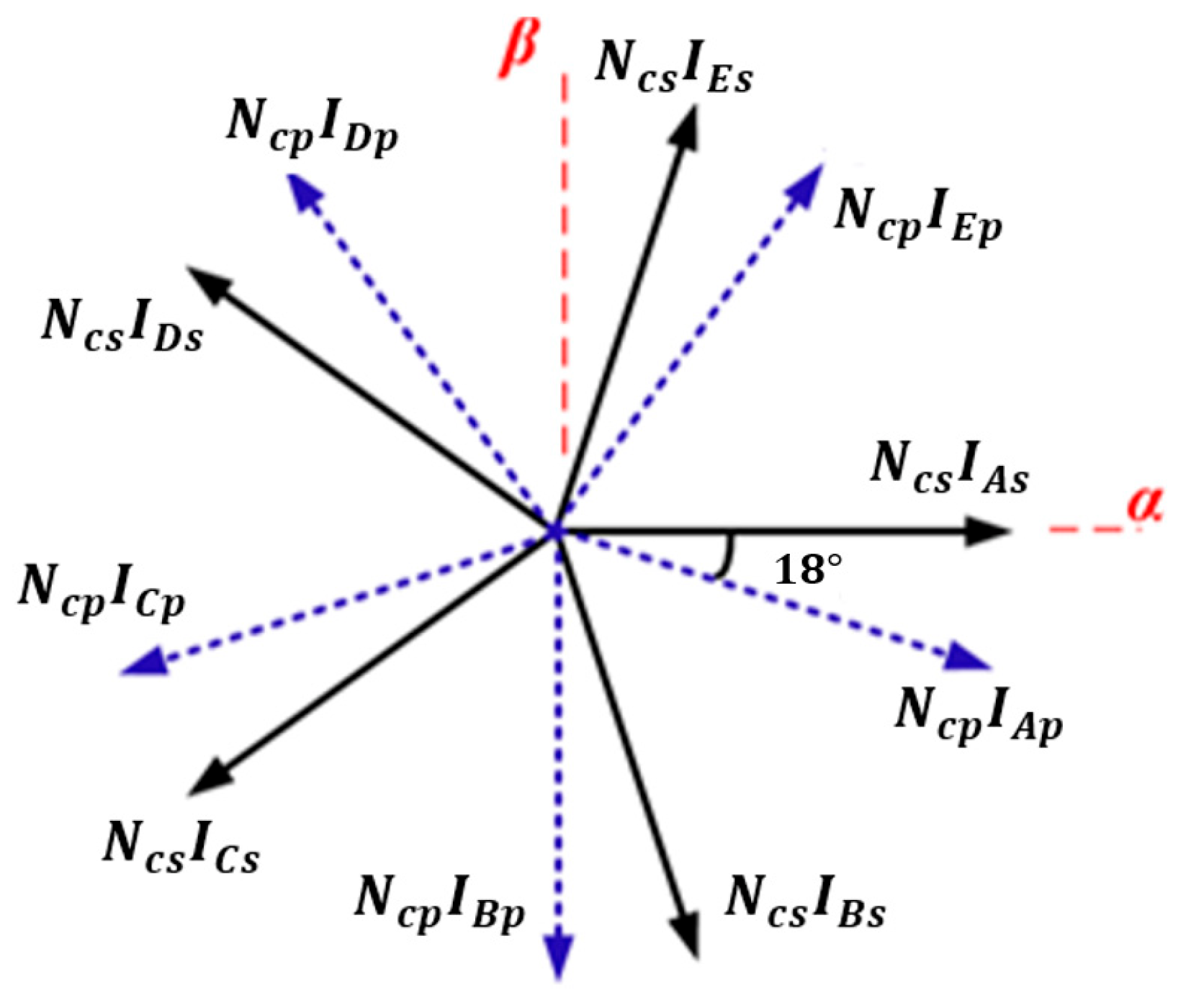
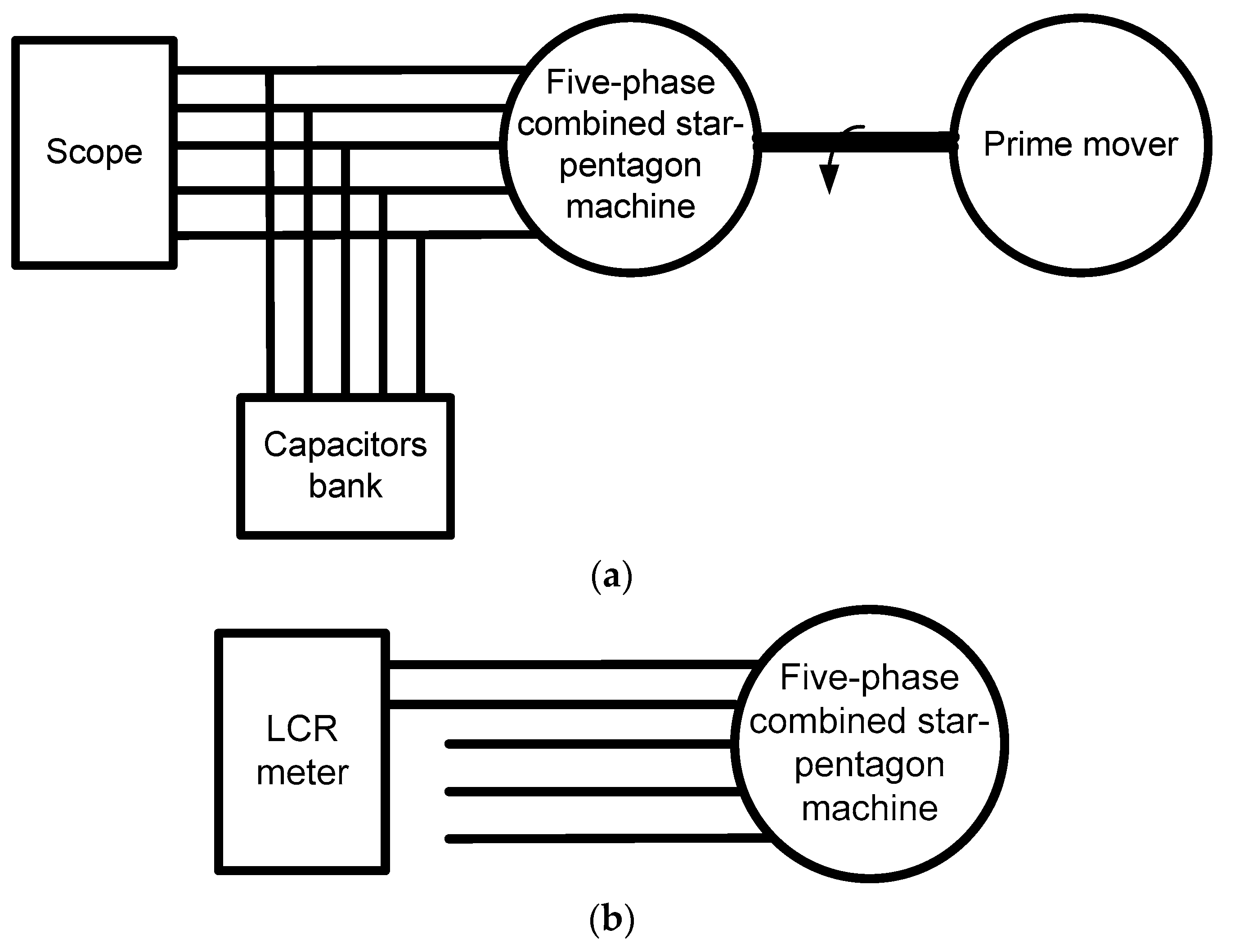
3. Case Study
- This terminal (red terminal or terminal that is considered as phase A) is connected to the positive terminal of the LCR meter, and the other terminal of the LCR meter is consecutively connected to the other four terminals of the machine (terminals k, g, y and b), as shown in Figure 10. The red terminal has two adjacent terminals and two nonadjacent terminals. Hence there are two higher values of resistance, and two lower values of resistance measured relative to the red terminal;
- However, it is not obvious which one of the yellow and the black terminals is phase C or D. In order to specify which one is phase C or D, the green terminal (phase B) is connected to the positive terminal of the LCR meter, and the other terminal of the LCR meter is connected once to the black terminal and once to the yellow terminal as shown in Figure 10. The terminal that gives the lower reading is the adjacent terminal to the green terminal (phase B);
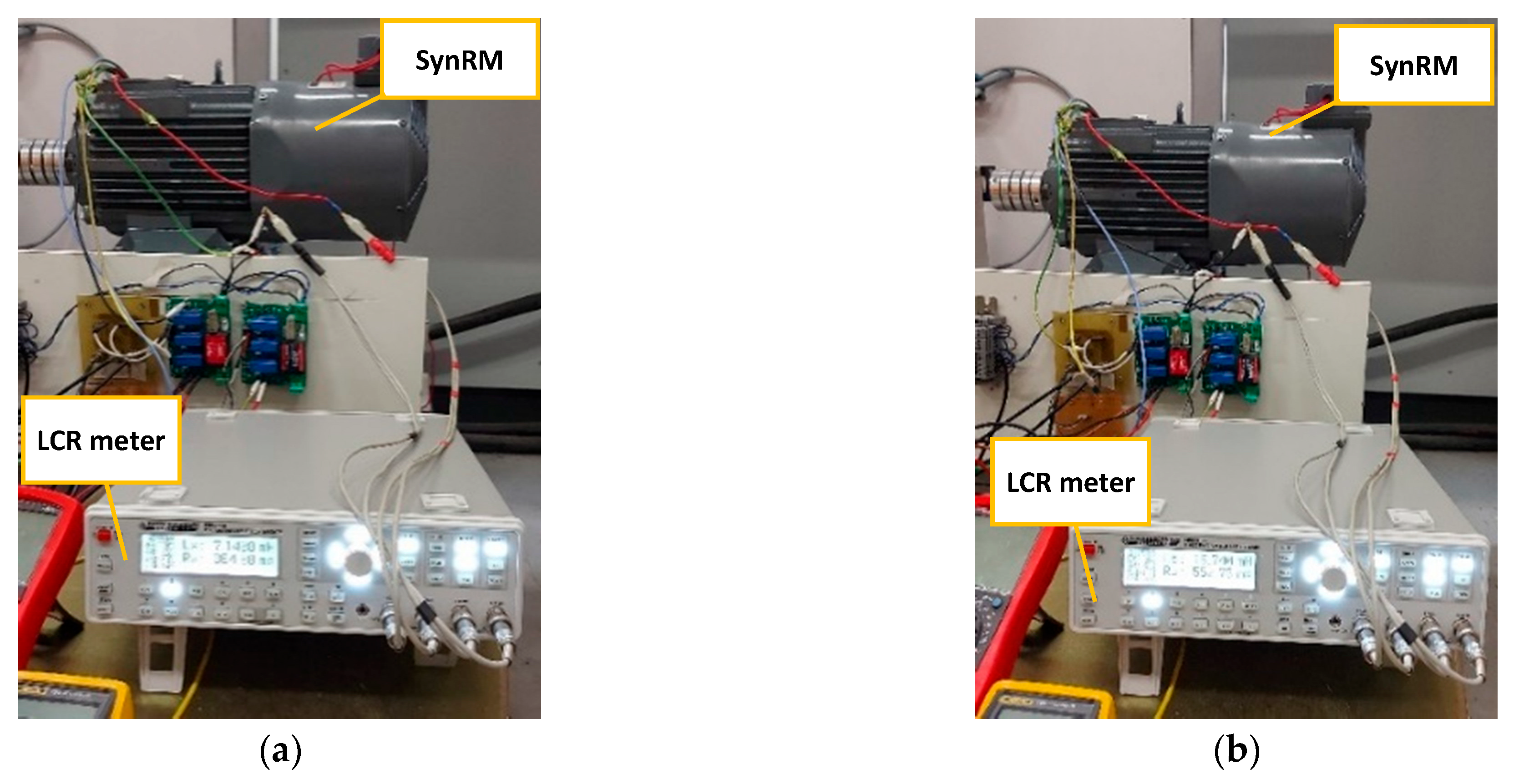
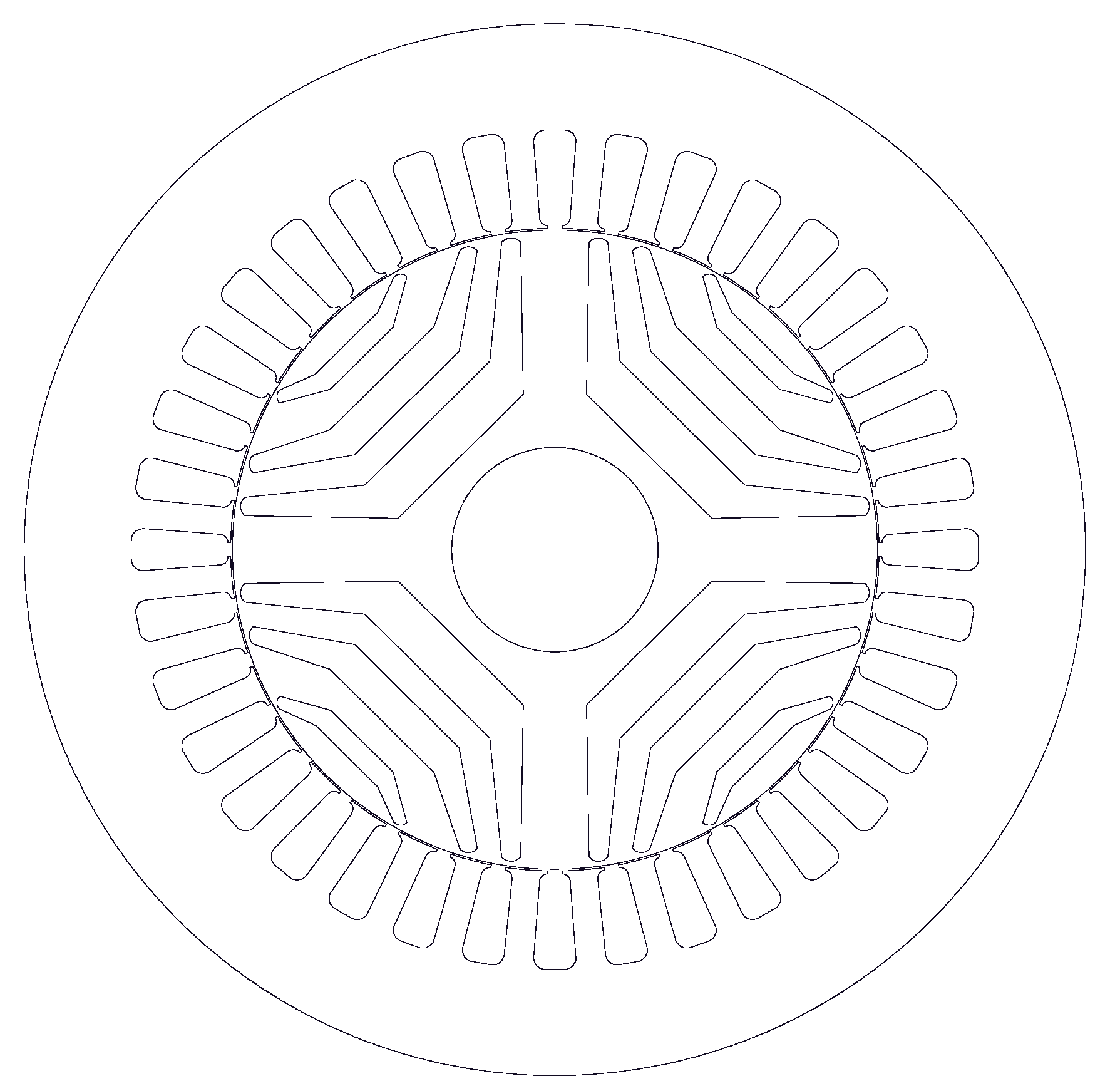

| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Stator bore diameter (D1O) | 110 mm | Air gap length (Lg) | 0.3 mm |
| Stator inner diameter (D) | 180 mm | Number of slots (S) | 36 |
| Rotor outer diameter (Dro) | 109.4 mm | Pole pairs (P) | 2 |
| Rotor inner diameter (Dri) | 35 mm | Rated frequency (F) | 100 Hz |
| Axial length (L) | 140 mm | Rated power (Pr) | 5.5 kW |
| Number of turns of star coil per phase | 24 | Number of turns of pentagon coil per phase | 29 |
| Stator/Rotor steel | M270-50A/M330-50A | Rated current (Is) | 12.3 A |
| Rotor flux barriers per pole (Nfb) | 3 | Number of phases (m) | 5 |
| ) | 0.25 | ) | 0.125 |
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Rrg | 0.365 Ω | Rry | 0.588 Ω |
| Rrb | 0.394 Ω | Rgk | 0.352 Ω |
| Rrk | 0.552 Ω | Rgy | 0.566 Ω |
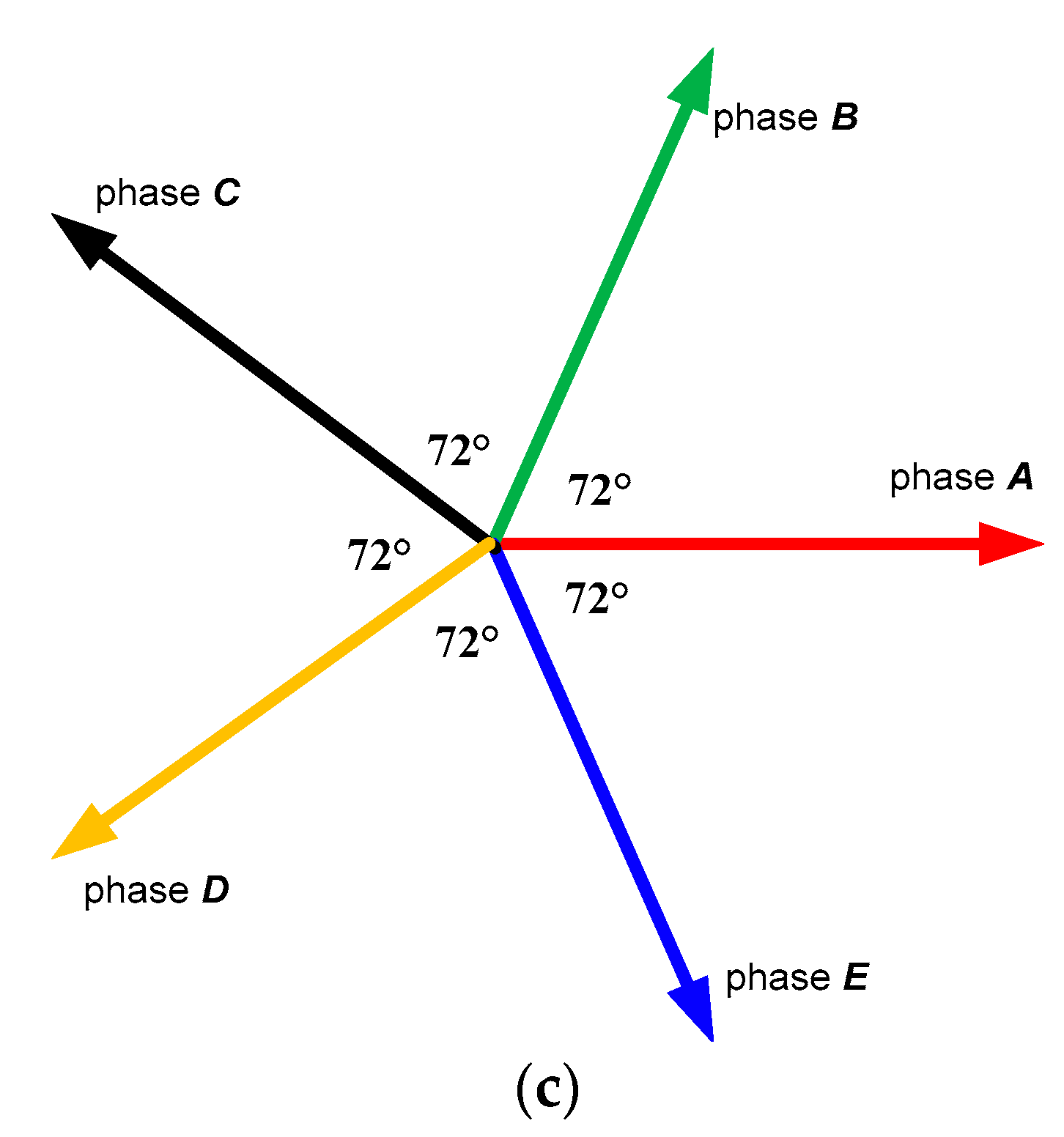
| Terminal | Phase | Terminal | Phase |
|---|---|---|---|
| Red terminal | A | Yellow terminal | D |
| Green terminal | B | Blue terminal | E |
| Black terminal | C |
4. Comparative Analysis of the Proposed and Conventional Methods
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chen, Q.; Gu, L.; Lin, Z.; Liu, G. Extension of Space-Vector-Signal-Injection-Based MTPA Control into SVPWM Fault-Tolerant Operation for Five-Phase IPMSM. IEEE Trans. Ind. Electron. 2020, 67, 7321–7333. [Google Scholar] [CrossRef]
- Arafat, A.K.M.; Haque, M.S.; Islam, M.Z.; Choi, S. Performance Comparison at Maximum Torque per Ampere Control between Rare Earth and Rare Earth Free Five-phase PMa-SynRM Under Open Phase Faults. In Proceedings of the 2018 IEEE Energy Conversion Congress and Exposition (ECCE), Portland, OR, USA, 23–27 September 2018; pp. 784–789. [Google Scholar] [CrossRef]
- Tawfiq, K.B.; Ibrahim, M.N.; Sergeant, P. An Enhanced Fault-Tolerant Control of a Five-Phase Synchronous Reluctance Motor Fed From a Three-to-Five-Phase Matrix Converter. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 10, 4182–4194. [Google Scholar] [CrossRef]
- Muteba, M. Influence of Mixed Stator Winding Configurations and Number of Rotor Flux-Barriers on Torque and Torque Ripple of Five-Phase Synchronous Reluctance Motors. In Proceedings of the 2019 IEEE Transportation Electrification Conference and Expo (ITEC), Novi, MI, USA, 19–21 June 2019; pp. 1–6. [Google Scholar] [CrossRef]
- Tawfiq, K.B.; Ibrahim, M.N.; El-Kholy, E.E.; Sergeant, P. Construction of Synchronous Reluctance Machines with Combined Star-Pentagon Configuration Using Standard Three-Phase Stator Frames. IEEE Trans. Ind. Electron. 2022, 69, 7582–7595. [Google Scholar] [CrossRef]
- Xu, L.; Fu, W. Evaluation of third harmonic component effects in five-phase synchronous reluctance motor drive using time-stepping finite-element method. IEEE Trans. Ind. Appl. 2002, 38, 638–644. [Google Scholar] [CrossRef]
- Tawfiq, K.B.; Ibrahim, M.N.; El-Kholy, E.E.; Sergeant, P. Comparative Analysis of Refurbishing Methods of Three-Phase Synchronous Reluctance Machines to Five-Phase With Minimum Cost. IEEE Trans. Ind. Appl. 2021, 57, 6007–6022. [Google Scholar] [CrossRef]
- Levi, E.; Bojoi, R.; Profumo, F.; Toliyat, H.A.; Williamson, S. Multiphase induction motor drives—A technology status review. IET Electr. Power Appl. 2007, 1, 489–516. [Google Scholar] [CrossRef] [Green Version]
- Tawfiq, K.B.; Ibrahim, M.N.; El-Kholy, E.E.; Sergeant, P. Refurbishing three-phase synchronous reluctance machines to multiphase machines. Electr. Eng. 2021, 103, 139–152. [Google Scholar] [CrossRef]
- Sala, G.; Mengoni, M.; Rizzoli, G.; Degano, M.; Zarri, L.; Tani, A. Impact of Star Connection Layouts on the Control of Multiphase Induction Motor Drives Under Open-Phase Fault. IEEE Trans. Power Electron. 2021, 36, 3717–3726. [Google Scholar] [CrossRef]
- Gary, E.; Magno, A.; Jorge, R. Design, Analysis and Validation of a Six-Phase Induction Machine from a Commercial Three-Phase for Academic Research. IEEE Lat. Am. Trans. 2020, 18, 1943–1952. [Google Scholar] [CrossRef]
- Farshadnia, M.; Cheema, M.A.M.; Pouramin, A.; Dutta, R.; Fletcher, J.E. Design of Optimal Winding Configurations for Symmetrical Multiphase Concentrated-Wound Surface-Mount PMSMs to Achieve Maximum Torque Density Under Current Harmonic Injection. IEEE Trans. Ind. Electron. 2018, 65, 1751–1761. [Google Scholar] [CrossRef]
- Abdel-Khalik, A.; Ahmed, S.; Hamad, M.; Massoud, A. Effect of Stator Winding Connection on Performance of Five-Phase Induction Machines. IEEE Trans. Ind. Electron. 2014, 61, 3–19. [Google Scholar] [CrossRef]
- Che, H.S.; Duran, M.J.; Levi, E.; Jones, M.; Hew, W.P.; Rahim, N.A. Postfault Operation of an Asymmetrical Six-Phase Induction Machine with Single and Two Isolated Neutral Points. IEEE Trans. Power Electron. 2014, 29, 5406–5416. [Google Scholar] [CrossRef] [Green Version]
- Mohammadpour, A.; Sadeghi, S.; Parsa, L. A Generalized Fault-Tolerant Control Strategy for Five-Phase PM Motor Drives Considering Star, Pentagon, and Pentacle Connections of Stator Windings. IEEE Trans. Ind. Electron. 2014, 61, 63–75. [Google Scholar] [CrossRef]
- Chen, H.; Liu, X.; El-Refaie, A.M.; Zhao, J.; Demerdash, N.A.O.; He, J. Comparative Study of Winding Configurations of a Five-Phase Flux-Switching PM Machine. IEEE Trans. Energy Convers. 2019, 34, 1792–1804. [Google Scholar] [CrossRef] [Green Version]
- Xu, H.; Huang, W.; Bu, F.; Liu, H.; Lin, X. Control of Five-Phase Dual Stator-Winding Induction Generator with an Open Phase. IEEE Trans. Ind. Electron. 2019, 66, 696–706. [Google Scholar] [CrossRef]
- Gu, Z.Y.; Wang, K.; Zhu, Z.Q.; Wu, Z.Z.; Liu, C.; Cao, R.W. Torque Improvement in Five-Phase Unequal Tooth SPM Machine by Injecting Third Harmonic Current. IEEE Trans. Veh. Technol. 2018, 67, 206–215. [Google Scholar] [CrossRef]
- Xu, H.; Bu, F.; Huang, W.; Hu, Y.; Liu, H. Control and Performance of Five-Phase Dual Stator-Winding Induction Generator DC Generating System. IEEE Trans. Ind. Electron. 2017, 64, 5276–5285. [Google Scholar] [CrossRef]
- Raziee, S.M.; Misir, O.; Ponick, B. Multiple Multiphase Combined Star–Polygon Winding Analysis. IEEE Trans. Ind. Electron. 2019, 66, 7468–7479. [Google Scholar] [CrossRef]
- Abdel-Khalik, A.S.; Elgenedy, M.A.; Ahmed, S.; Massoud, A.M. An Improved Fault-Tolerant Five-Phase Induction Machine Using a Combined Star/Pentagon Single Layer Stator Winding Connection. IEEE Trans. Ind. Electron. 2016, 63, 618–628. [Google Scholar] [CrossRef]
- Abdel-Khalik, A.S.; Ahmed, S.; Massoud, A.M. Dynamic Modelling of a Five-Phase Induction Machine with a Combined Star/Pentagon Stator Winding Connection. IEEE Trans. Energy Convers. 2016, 31, 1645–1656. [Google Scholar] [CrossRef]
- Abdel-Khalik, A.S.; Ahmed, S.; Massoud, A.M. Steady-State Mathematical Modelling of a Five-Phase Induction Machine with a Combined Star/Pentagon Stator Winding Connection. IEEE Trans. Ind. Electron. 2016, 63, 1331–1343. [Google Scholar] [CrossRef]
- Abdel-Khalik, A.S.; Ahmed, S.; Massoud, A.M. Low Space Harmonics Cancelation in Double-Layer Fractional Slot Winding Using Dual Multiphase Winding. IEEE Trans. Magn. 2015, 51, 8104710. [Google Scholar] [CrossRef]
- Tawfiq, K.B.; Ibrahim, M.N.; El-Kholy, E.E.; Sergeant, P. Performance Improvement of Existing Three Phase Synchronous Reluctance Machine: Stator Upgrading to 5-Phase with Combined Star-Pentagon Winding. IEEE Access 2020, 8, 143569–143583. [Google Scholar] [CrossRef]
- Teng, K.; Lu, Z.; Long, J.; Wang, Y.; Roskilly, A.P. Voltage Build-Up Analysis of Self-Excited Induction Generator with Multi-Timescale Reduced-Order Model. IEEE Access 2019, 7, 48003–48012. [Google Scholar] [CrossRef]
- Vanco, W.E.; Silva, F.B.; De Oliveira, C.M.R.; Monteiro, J.R.B.A.; De Oliveira, J.M.M. A Proposal of Expansion and Implementation in Isolated Generation Systems Using Self-Excited Induction Generator with Synchronous Generator. IEEE Access 2019, 7, 117188–117195. [Google Scholar] [CrossRef]
- Malik, N.H.; Mazi, A.A. Capacitance Requirements for Isolated Self-Excited Induction Generators. IEEE Power Eng. Rev. 1987, PER-7, 33–34. [Google Scholar]
- Chen, K.; Shi, J.; Wei, X.; Cai, S. Phase Identification with Single-Phase Meter and Concentrator Based on NMF Dimension Reduction and Label Propagation. In Proceedings of the 2021 IEEE 11th Annual International Conference on CYBER Technology in Automation, Control, and Intelligent Systems (CYBER), Jiaxian, China, 27–31 July 2021; pp. 1–6. [Google Scholar] [CrossRef]
- Mitra, R.; Kota, R.; Bandyopadhyay, S. Voltage Correlations in Smart Meter Data. In Proceedings of the 21th ACM SIGKDD International Conference on Knowledge Discovery and Data Mining, Sydney, Australia, 10–13 August 2015; pp. 1999–2008. [Google Scholar]
- Short, T.A. Advanced Metering for Phase Identification, Transformer Identification, and Secondary Modeling. IEEE Trans. Smart Grid 2013, 4, 651–658. [Google Scholar] [CrossRef]
- Li, G. Transformer topology identification method based on LoRa and GPU acceleration. Electr. Meas. Instrum. 2019, 21, 88–95. [Google Scholar]
- Hosseini, Z.S.; Khodaei, A.; Paaso, A. Machine Learning-Enabled Distribution Network Phase Identification. IEEE Trans. Power Syst. 2021, 36, 842–850. [Google Scholar] [CrossRef]
- Wang, W.; Yu, N.; Foggo, B.; Davis, J.; Li, J. Phase identification in electric power distribution systems by clustering of smart meter data. In Proceedings of the 2016 IEEE International Conference on Machine Learning and Applications (ICMLA), Anaheim, CA, USA, 18-20 December 2016; pp. 259–265. [Google Scholar]
- Ni, F.; Liu, J.Q.; Wei, F.; Zhu, C.D.; Xie, S.X. Phase identification in distribution systems by data mining methods. In Proceedings of the 2017 IEEE Conference on Energy Internet and Energy System Integration (EI2), Beijing, China, 26-28 November 2017; pp. 1–6. [Google Scholar]
- Lian, Z.; Yao, L.; Liu, S. Phase and meter box identification for single-phase users based on t-SNE dimension reduction and BIRCH clustering. Autom. Electr. Power Syst. 2020, 44, 176–184. [Google Scholar]
- Suzuki, K. A New Self-Calibration Method of an $LCR$ Meter for RF Resistance Calibration Using Capacitance Standards. IEEE Trans. Instrum. Meas. 2009, 58, 993–996. [Google Scholar] [CrossRef]
- Ibrahim, M.; Pillay, P. The Loss of Self-Excitation Capability in Stand-Alone Synchronous Reluctance Generators. IEEE Trans. Ind. Appl. 2018, 54, 6290–6298. [Google Scholar] [CrossRef]
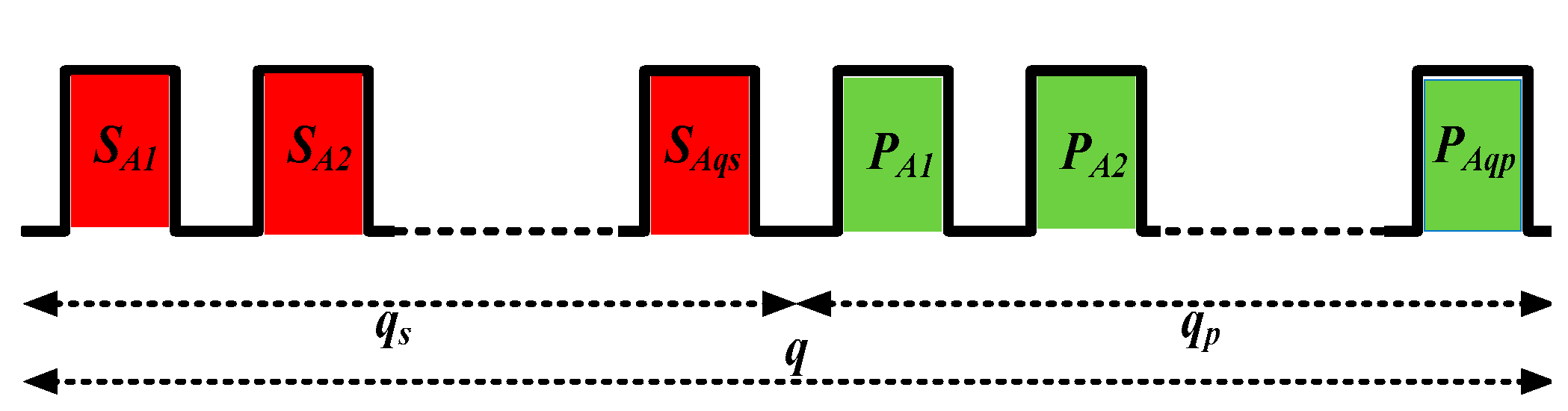


| Conventional Method | Proposed Method | |
|---|---|---|
| Prime mover | Yes | No |
| Scope | Yes | No |
| Capacitor bank | Yes | No |
| LCR meter | No | Yes |
| Cost | High | Low |
| Time | much | Few |
| Type | Dynamic test | Static test |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tawfiq, K.B.; Ibrahim, M.N.; EL-Refaie, A.M.; Sergeant, P. A Practical Approach to Identify the Phases Sequence in Five Phase Machines with Combined Star–Pentagon Configuration. Mathematics 2022, 10, 4086. https://doi.org/10.3390/math10214086
Tawfiq KB, Ibrahim MN, EL-Refaie AM, Sergeant P. A Practical Approach to Identify the Phases Sequence in Five Phase Machines with Combined Star–Pentagon Configuration. Mathematics. 2022; 10(21):4086. https://doi.org/10.3390/math10214086
Chicago/Turabian StyleTawfiq, Kotb B., Mohamed N. Ibrahim, Ayman M. EL-Refaie, and Peter Sergeant. 2022. "A Practical Approach to Identify the Phases Sequence in Five Phase Machines with Combined Star–Pentagon Configuration" Mathematics 10, no. 21: 4086. https://doi.org/10.3390/math10214086
APA StyleTawfiq, K. B., Ibrahim, M. N., EL-Refaie, A. M., & Sergeant, P. (2022). A Practical Approach to Identify the Phases Sequence in Five Phase Machines with Combined Star–Pentagon Configuration. Mathematics, 10(21), 4086. https://doi.org/10.3390/math10214086









