The Analytical Solutions of the Stochastic mKdV Equation via the Mapping Method
Abstract
:1. Introduction
2. Traveling Wave Equation for SmKdV
3. The Description of Mapping Method
4. Exact Solutions of mKdV
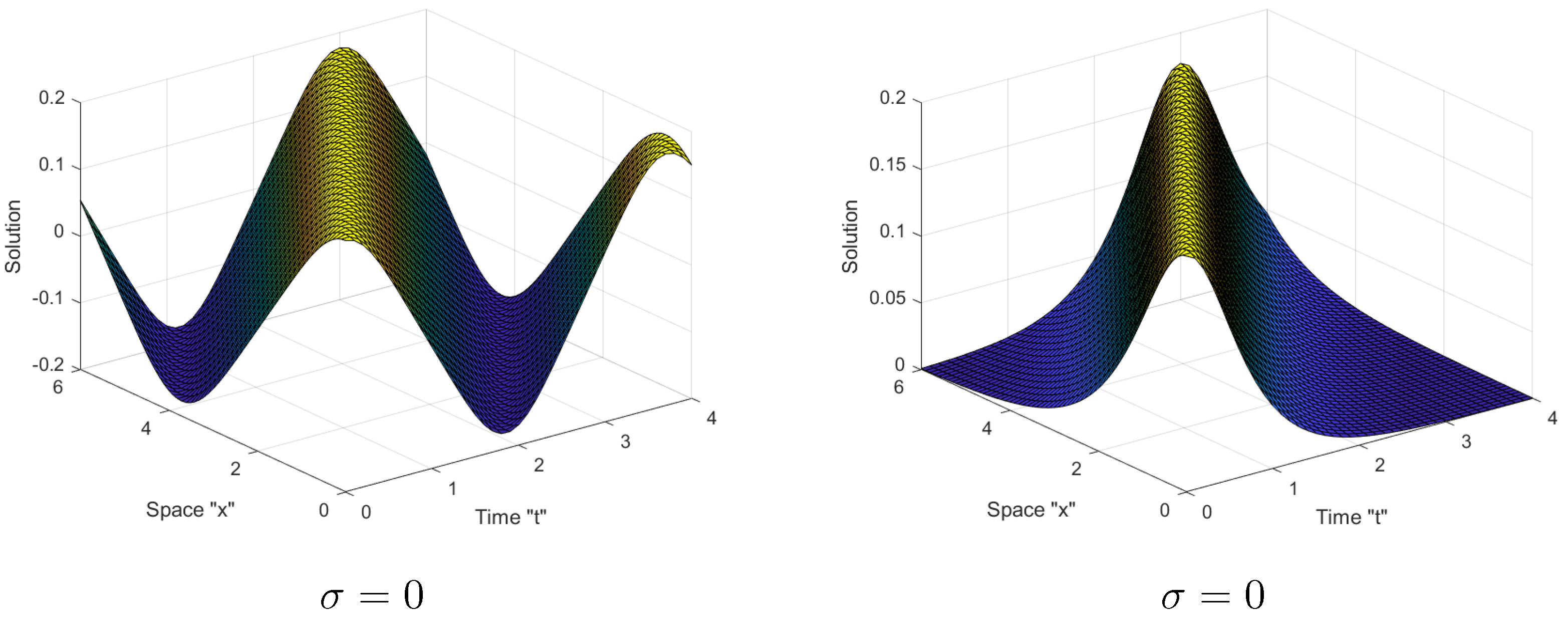
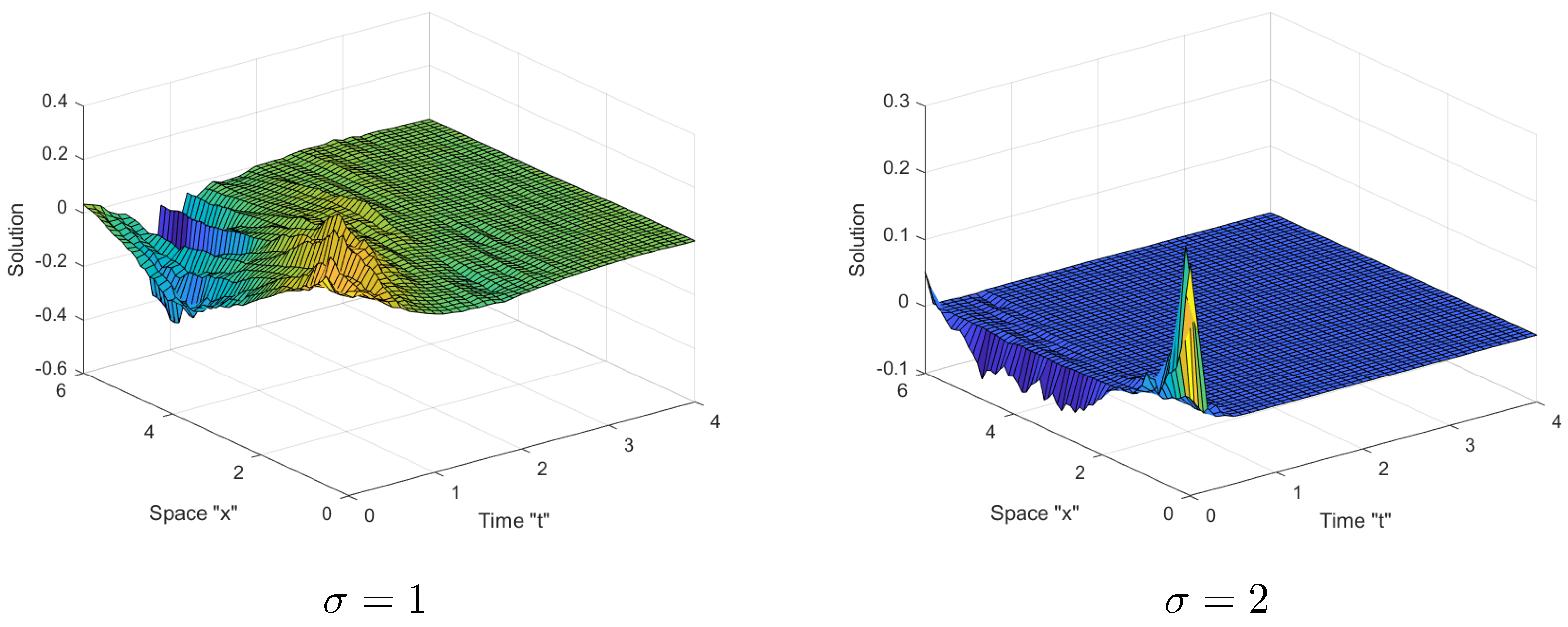
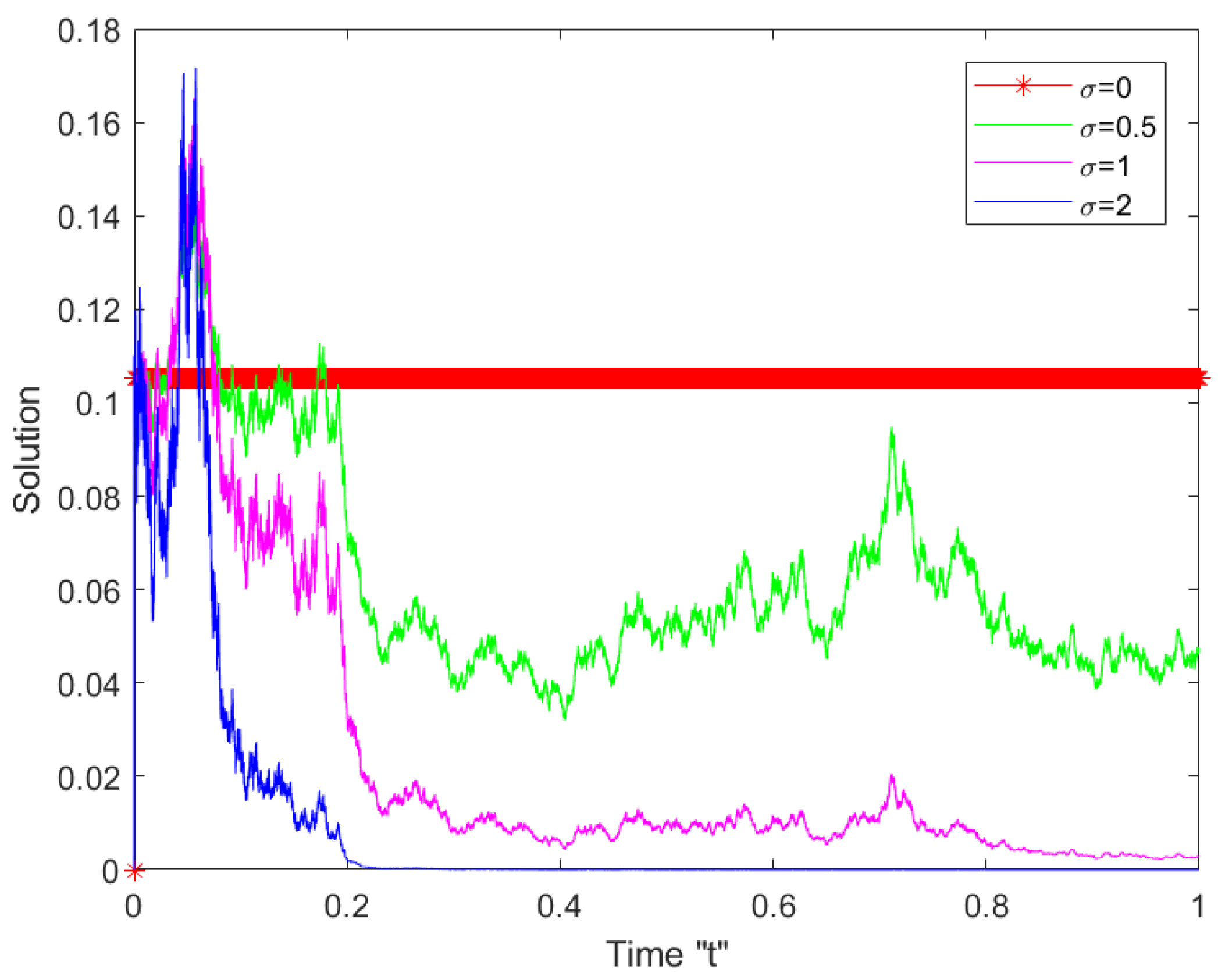
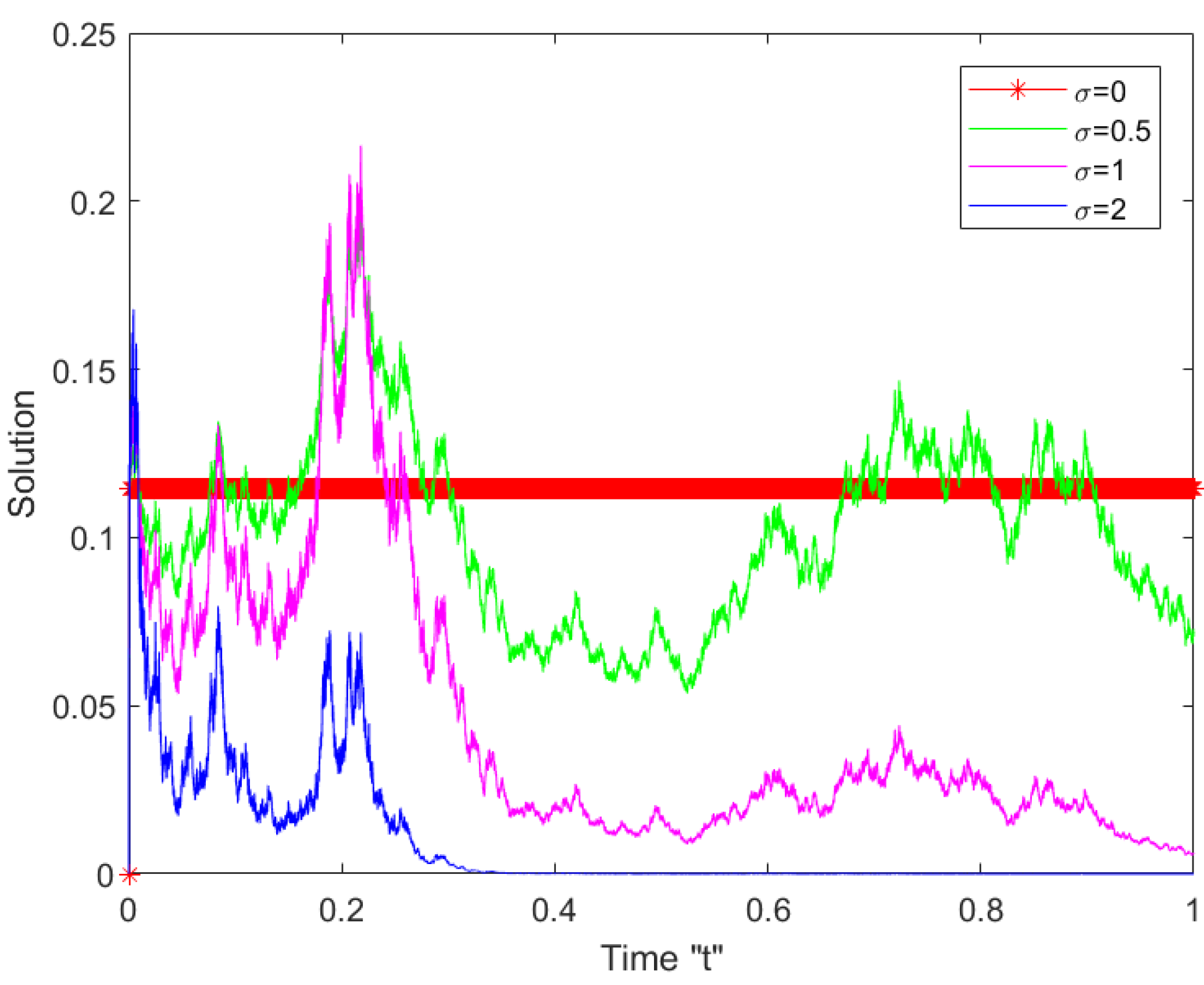
5. The Effect of Noise on SmKdV Solutions
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Johnson, R.S. Anon-linear equation incorporating damping and dispersion. J. Fluid Mech. 1970, 42, 49–60. [Google Scholar] [CrossRef]
- Younis, M.; Ali, S. Solitary wave and shock wave solutions to the transmission line modelfor nano-ionic currents along microtubules. Appl. Math. Comput. 2014, 246, 460–463. [Google Scholar]
- Younis, M.; Rizvi, S.T.R.; Ali, S. Analytical and soliton solutions: Nonlinear model of nanobioelectronics transmis sion lines. Appl. Math. Comput. 2015, 265, 994–1002. [Google Scholar] [CrossRef]
- Razborova, P.; Moraru, L.; Biswas, A. Perturbation of dis persive shallow water waves with Rosenau- KdV RLW equation and power law nonlinearity. Rom. J. Phys. 2014, 59, 7–8. [Google Scholar]
- Zhou, Q.; Ekici, M.; Sonmezoglu, A.; Manafian, J.; Khaleghizadeh, S.; Mirzazadeh, M. Exact solitary wave solutions to the generalized Fisher equation. Optik 2016, 127, 12085–12092. [Google Scholar] [CrossRef]
- Baskonus, H.M.; Bulut, H. New wave behaviors of the system of equations for the ion sound and Langmuir Waves. Waves Random Complex Media 2016, 26, 613–625. [Google Scholar] [CrossRef]
- Baskonus, H.M. New acoustic wave behaviors to the Davey-Stewartson equation with power-law nonlinearity arising in fluid dynamics. Nonlinear Dyn. 2016, 86, 177–183. [Google Scholar] [CrossRef]
- Manafian, J.; Lakestani, M. Optical solitons with Biswas-Milovic equation for Kerr law nonlinearity. Eur. Phys. J. Plus 2015, 130, 61. [Google Scholar] [CrossRef]
- Mohammed, W.W.; Blömker, D. Fast-diffusion limit for reaction-diffusion equations with multiplicative noise. J. Math. Anal. Appl. 2021, 496, 124808. [Google Scholar] [CrossRef]
- Mohammed, W.W.; Iqbal, N. Impact of the same degenerate additive noise on a coupled system of fractional space diffusion equations. Fractals 2022, 30, 2240033. [Google Scholar] [CrossRef]
- Tchier, F.; Yusuf, A.; Aliyu, A.I.; Inc, M. Soliton solutions and conservation laws for lossy nonlinear transmission line equation. Superlattices Microstruct. 2017, 107, 320–336. [Google Scholar] [CrossRef]
- Zhou, Q. Optical solitons in medium with parabolic law nonlinearity and higher order dispersion. Waves Random Complex Media 2016, 25, 52–59. [Google Scholar] [CrossRef]
- Salas, A.H. Solving nonlinear partial differential equations by the sn-ns method. Abstr. Appl. Anal. 2012, 2012, 340824. [Google Scholar] [CrossRef] [Green Version]
- Baskonus, H.M.; Bulut, H. Exponential prototype structures for (2+1)-dimensional Boiti-Leon-Pempinelli systems in mathematical physics. Waves Random Complex Media 2016, 26, 201–208. [Google Scholar] [CrossRef]
- Manafian, J. Optical soliton solutions for Schrodinger type nonlinear evolution equations by the tan(φ/2)-expansion method. Optik 2016, 127, 4222–4245. [Google Scholar] [CrossRef]
- Wazwaz, A.M. The tanh method: Exact solutions of the Sine–Gordon and Sinh–Gordon equations. Appl. Math. Comput. 2005, 167, 1196–1210. [Google Scholar] [CrossRef]
- Mohammed, W.W.; Alshammari, M.; Cesarano, C.; El-Morshedy, M. Brownian Motion Effects on the Stabilization of Stochastic Solutions to Fractional Diffusion Equations with Polynomials. Mathematics 2022, 10, 1458. [Google Scholar] [CrossRef]
- Al-Askar, E.M.; Mohammed, W.W.; Albalahi, A.M.; El-Morshedy, M. The Impact of the Wiener process on the analytical solutions of the stochastic (2+1)-dimensional breaking soliton equation by using tanh–coth method. Mathematics 2022, 10, 817. [Google Scholar] [CrossRef]
- Manafian, J.; Lakestani, M. Solitary wave and periodic wave solutions for Burgers, Fisher, Huxley and combined forms of these equations by the (G′/G)-expansion method. Pramana J. Phys. 2015, 130, 31–52. [Google Scholar] [CrossRef]
- Ablowitz, M.J.; Segur, H. Solitons and the Inverse Scattering Transform; SIAM: Philadelphia, PA, USA, 1981. [Google Scholar]
- Hirota, R. The Direct Method in Soliton Theory; Osaka City University: Osaka, Japan, 2004. [Google Scholar]
- Olver, P.J. Application of Lie Group to Differential Equation; Springer: New York, NY, USA, 1986. [Google Scholar]
- Wazwaz, A.M. A KdV6 hierarchy: Integrable members with distinct dispersion relations. Appl. Math. Lett. 2015, 45, 86–92. [Google Scholar] [CrossRef]
- Geng, X.; Xue, B. N-soliton and quasi-periodic solutions of the KdV6 equations. Appl. Math. Comp. 2012, 219, 3504–3510. [Google Scholar] [CrossRef]
- Azwaz, A.M.; Xu, G.Q. An extended modified KdV equation and its Painlevé integrability. Nonlinear Dyn. 2016, 86, 1455–1460. [Google Scholar] [CrossRef]
- Zhang, Y.; Dang, X.L.; Xu, H.X. Backlund transformations and soliton solutions for the KdV6 equation. Appl. Math. Comp. 2011, 217, 6230–6236. [Google Scholar] [CrossRef]
- Wen, X.Y.; Gao, Y.T.; Wang, L. Darboux transformation and explicit solutions for the integrable sixth-order KdV equation for nonlinear waves. Appl. Math. Comp. 2011, 218, 55–60. [Google Scholar] [CrossRef]
- Miura, R.M.; Gardner, C.S.; Kruskal, M.D. KdV equation and generalizations.II. Existence of conservation laws and constant of motion. J. Math. Phys. 1968, 9, 1204–1209. [Google Scholar] [CrossRef]
- Gardner, C.S.; Green, J.M.; Kruskal, M.D.; Miura, R.M. Method for solving the Korteweg–de Vries equation. Phys. Rev. Lett. 1967, 19, 1095–1097. [Google Scholar] [CrossRef]
- Raslan, K.R. The application of He’s Exp-function method for MKdV and Burgers’ equations with variable coefficients. Int. Nonlinear Sci. 2009, 7, 174–181. [Google Scholar]
- Yang, Y. Exact solutions of the mKdV equation. IOP Conf. Ser. Earth Environ. Sci. 2021, 769, 042040. [Google Scholar] [CrossRef]
- Taghizadeh, N. Comparison of solutions of mKdV equation by using the first integral method and (G′/G)-expansion method. Math. Aeterna 2012, 2, 309–320. [Google Scholar]
- Wazwaz, A.M. The tanh method for generalized forms of nonlinear heat conduction and Burgers–Fisher equations. Appl. Math. Comput. 2005, 169, 321–338. [Google Scholar] [CrossRef]
- Elmandouha, A.A.; Ibrahim, A.G. Bifurcation and travelling wave solutions for a (2+1)-dimensional KdV equation. J. Taibah Univ. Sci. 2020, 14, 139–147. [Google Scholar] [CrossRef] [Green Version]
- Wang, G.; Kara, A.H. A (2+1)-dimensional KdV equation and mKdV equation: Symmetries, group invariant solu tions and conservation laws. Phys. Lett. A 2019, 383, 728–731. [Google Scholar] [CrossRef]
- Helal, M.A. Soliton solution of some nonlinear partial differential equations and its applications in fluid mechanics. Chaos Solitons Fractals 2002, 13, 1917–1929. [Google Scholar] [CrossRef]
- Li, Z.P.; Liu, Y.C. Analysis of stability and density waves of traffic flow model in an ITS environment. Eur. Phys. J. B 2006, 53, 367–374. [Google Scholar] [CrossRef]
- Khater, A.H.; El-Kalaawy, O.H.; Callebaut, D.K. Bäcklund transformations and exact solutions for Alfven solitons in a relativistic electronpositron plasma. Phys. Scr. 1998, 58, 545–548. [Google Scholar] [CrossRef]
- Leblond, H.; Mihalache, D. Few-optical-cycle solitons: Modified Kortewegde Vries sine-Gordon equation versus other non-slowly-varying-envelopeapproximation models. Phys. Rev. A 2009, 79, 063835-1-7. [Google Scholar] [CrossRef] [Green Version]
- Kloeden, P.E.; Platen, E. Numerical Solution of Stochastic Differential Equations; Springer: New York, NY, USA, 1995. [Google Scholar]
- Peng, Y.Z. Exact solutions for some nonlinear partial differential equations. Phys. Lett. A 2013, 314, 401–408. [Google Scholar] [CrossRef]

| Case | p | q | r | |
|---|---|---|---|---|
| 1 | 1 | |||
| 2 | 1 | |||
| 3 | 1 | |||
| 4 | ||||
| 5 | ||||
| 6 | ||||
| 7 | ||||
| 8 | ||||
| 9 | ||||
| 10 | ||||
| 11 | ||||
| 12 | 1 | 0 | 0 | |
| 13 | 0 | 1 | 0 |
| Case | p | q | r | ||
|---|---|---|---|---|---|
| 1 | |||||
| 2 | |||||
| 3 | |||||
| 4 |
| Case | p | q | r | ||
|---|---|---|---|---|---|
| 1 | 1 | 0 | |||
| 2 | 2 | 0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mohammed, W.W.; Al-Askar, F.M.; Cesarano, C. The Analytical Solutions of the Stochastic mKdV Equation via the Mapping Method. Mathematics 2022, 10, 4212. https://doi.org/10.3390/math10224212
Mohammed WW, Al-Askar FM, Cesarano C. The Analytical Solutions of the Stochastic mKdV Equation via the Mapping Method. Mathematics. 2022; 10(22):4212. https://doi.org/10.3390/math10224212
Chicago/Turabian StyleMohammed, Wael W., Farah M. Al-Askar, and Clemente Cesarano. 2022. "The Analytical Solutions of the Stochastic mKdV Equation via the Mapping Method" Mathematics 10, no. 22: 4212. https://doi.org/10.3390/math10224212