Theory for the Beam Splitter in Quantum Optics: Quantum Entanglement of Photons and Their Statistics, HOM Effect
Abstract
1. Introduction
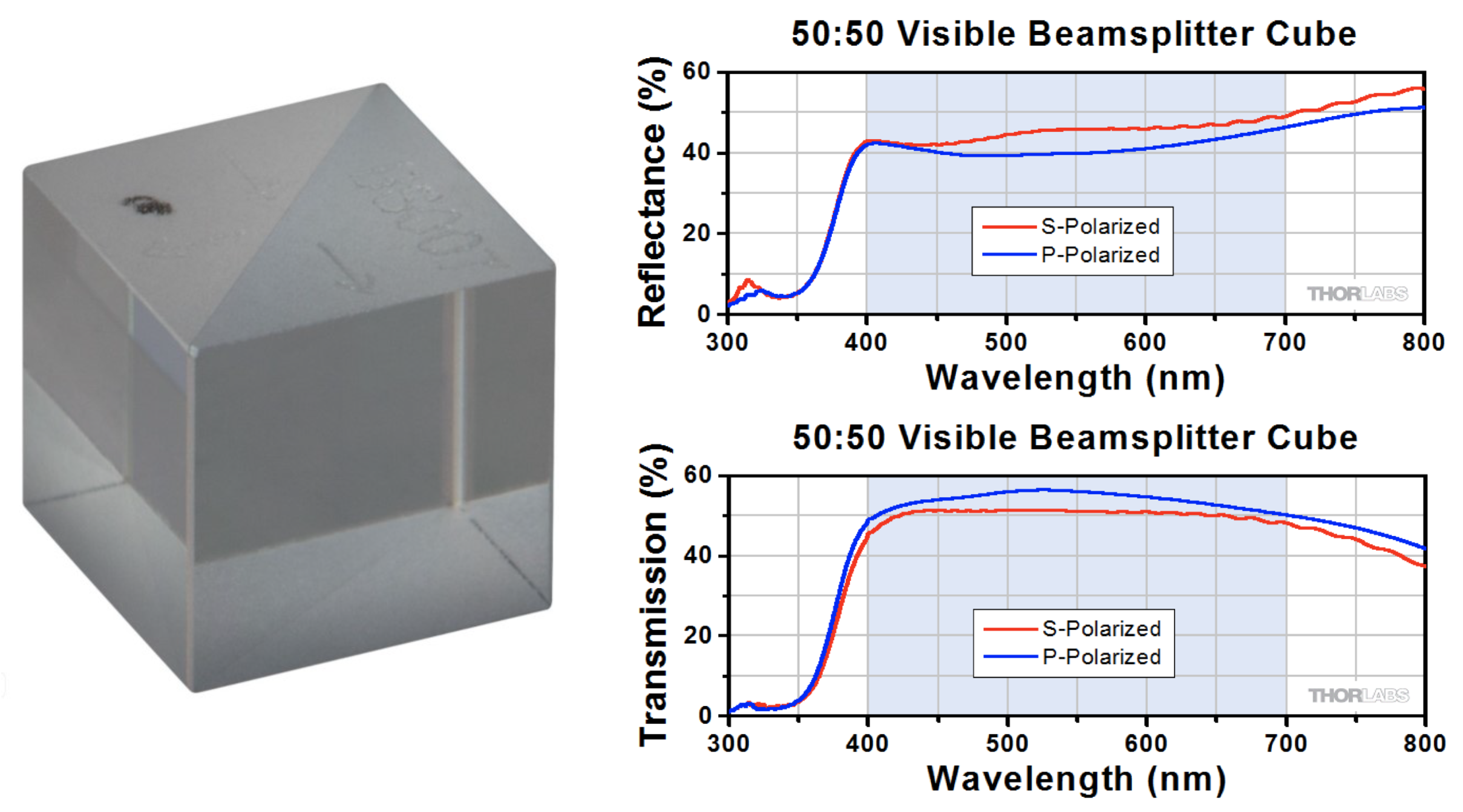
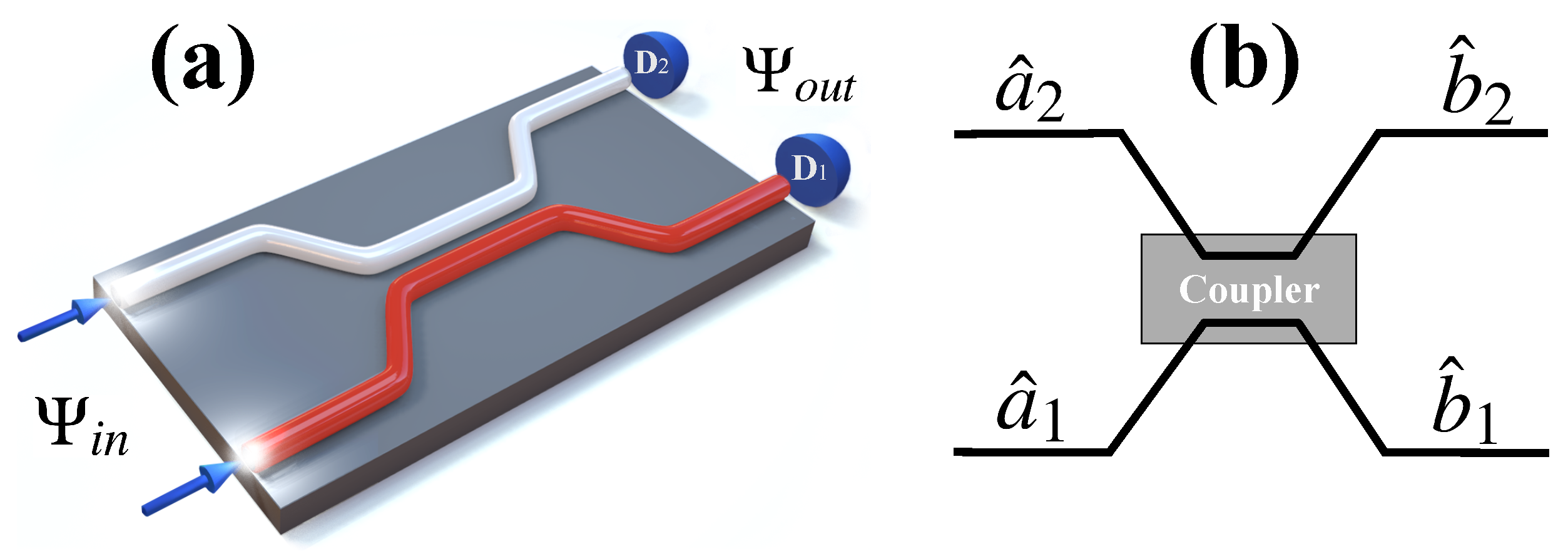
2. Beam Splitter in Quantum Optics
2.1. Basic Expressions for Beam Splitters of Any Type
2.2. “Conventional” Waveguide Beam Splitter
2.3. Frequency-Dependent Waveguide Beam Splitter
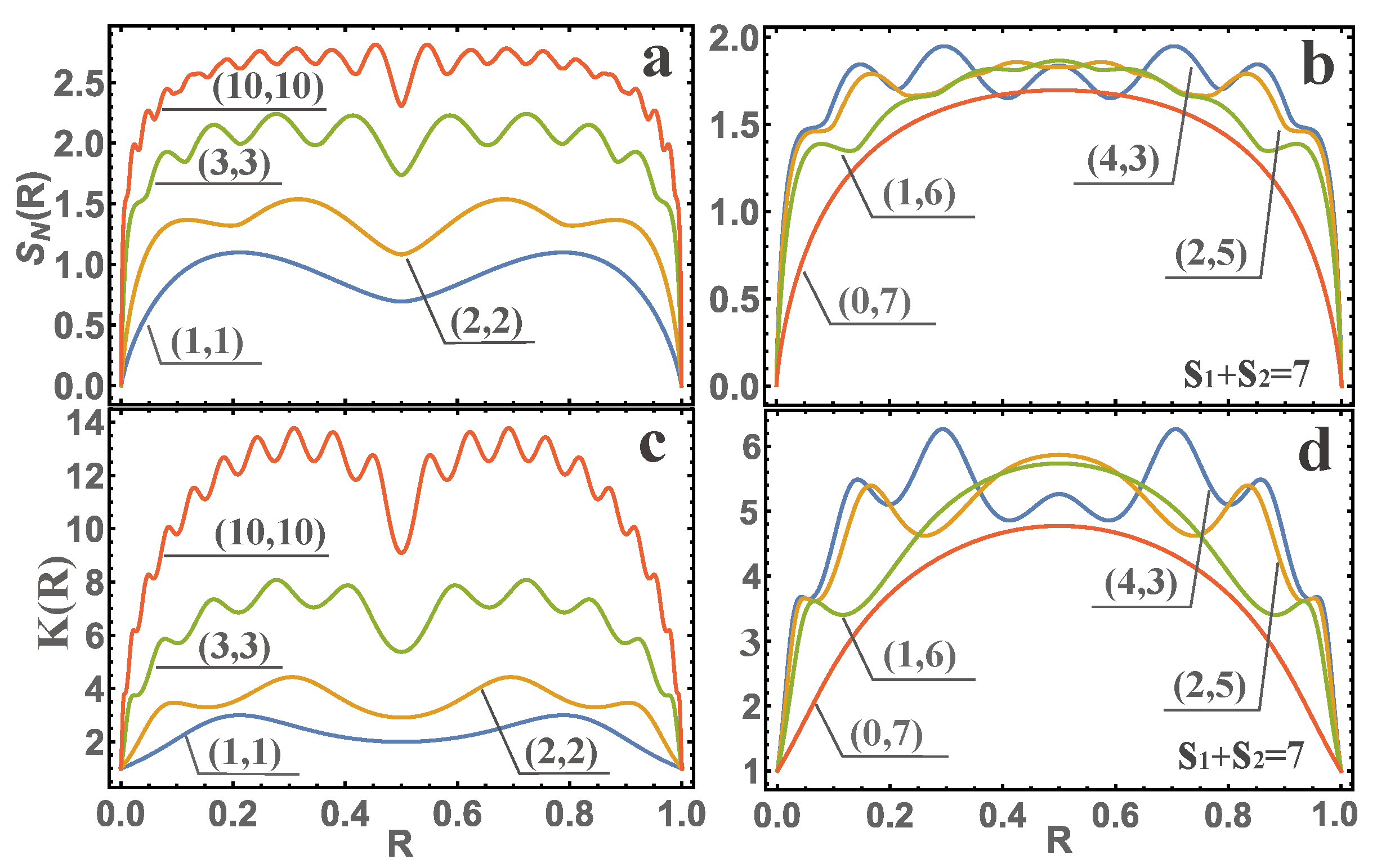
3. Quantum Entanglement of Photons on a Beam Splitter
3.1. Quantum Entanglement on a “Conventional” Beam Splitter
3.2. Quantum Entanglement on a Waveguide Beam Splitter
4. Photon Statistics on the Beam Splitter
4.1. Photon Statistics on a “Conventional” Beam Splitter
4.2. Photon Statistics on a Waveguide Beam Splitter
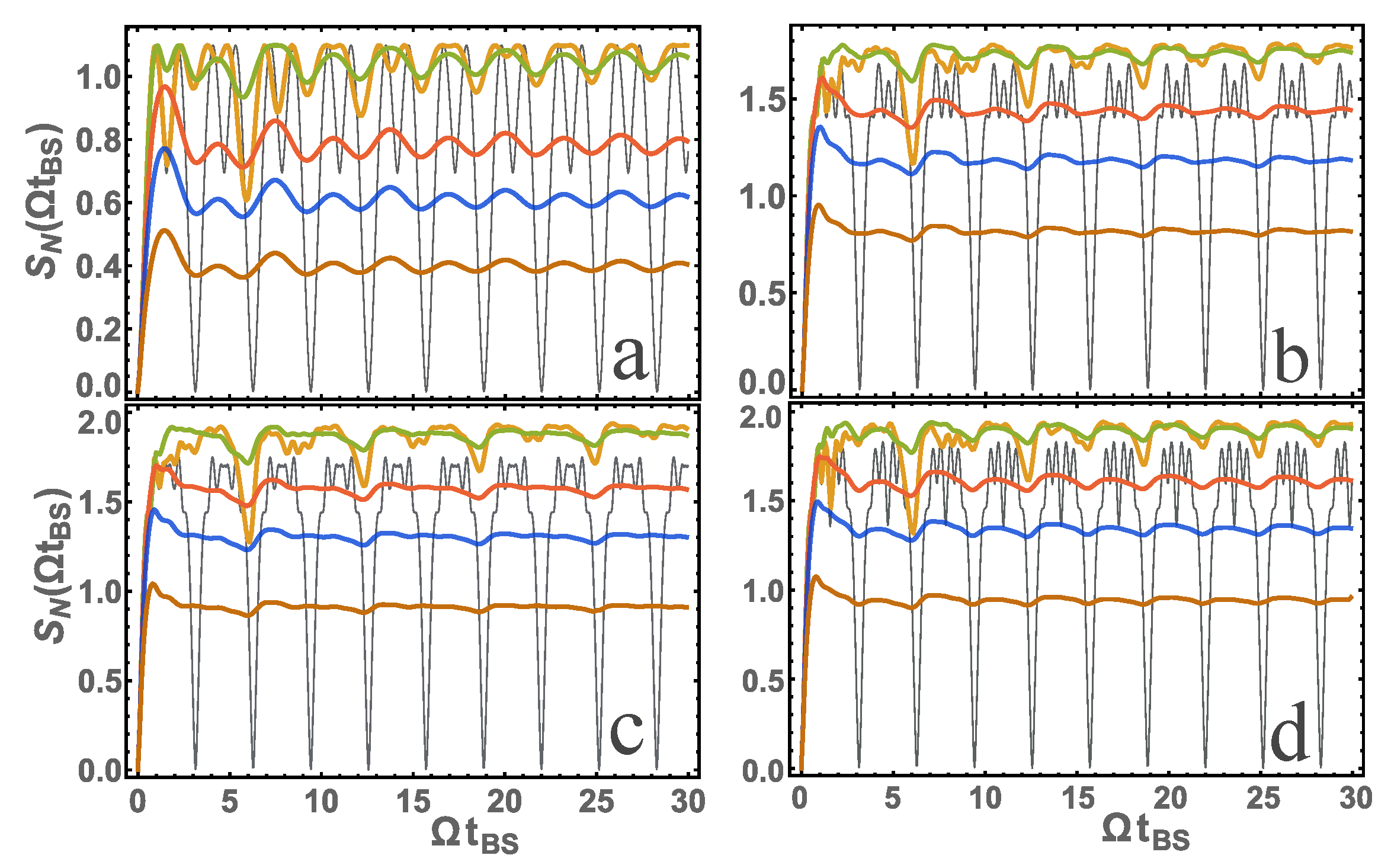
5. Hong–Ou–Mandel Effect
- The first and second photons fall on detectors 1 and 2, respectively;
- The first and second photons fall on detectors 2 and 1, respectively;
- The first and second photons fall on detector 1;
- The first and second photons fall on detector 2.
5.1. Hong–Ou–Mandel Effect on a “Conventional” Beam Splitter
5.2. Hong–Ou–Mandel Effect on a Waveguide Beam Splitter
6. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mandel, L.; Wolf, E. Optical Coherence and Quantum Optics; Cambridge University Press: Cambridge, UK, 1995; p. 1166. [Google Scholar]
- Scully, M.; Zubairy, M. Quantum Optics; Cambridge University Press: Cambridge, UK, 1997; p. 630. [Google Scholar]
- Biedenharn, L.; van Dam, H. The Quantum Theory of Light; Oxford: Oxford, UK, 2000; p. 448. [Google Scholar]
- Hong, C.K.; Ou, Z.Y.; Mandel, L. Measurement of subpicosecond time intervals between two photons by interference. Phys. Rev. Lett. 1987, 59, 2044–2046. [Google Scholar] [CrossRef] [PubMed]
- Agarwal, G.S. Quantum Optics; Cambridge University Press: Cambridge, UK, 2013; p. 491. [Google Scholar]
- Knill, E.; Laflamme, R.; Milburn, G.J. A Scheme for Efficient Quantum Computation With Linear Optics. Nature 2001, 409, 46–52. [Google Scholar] [CrossRef] [PubMed]
- Pan, J.W.; Chen, Z.; Lu, C.Y.; Weinfurter, H.; Zeilinger, A.; Zukowski, M. Multiphoton entanglement and interferometry. Rev. Mod. Phys. 2012, 84, 777. [Google Scholar] [CrossRef]
- Sangouard, N.; Simon, C.; de Riedmatten, H.; Gisin, N. Quantum repeaters based on atomic ensembles and linear optics. Rev. Mod. Phys. 2011, 83, 33. [Google Scholar] [CrossRef]
- Harris, N.C.; Steinbrecher, G.R.; Prabhu, M.; Lahini, Y.; Mower, J.; Bunandar, D.; Chen, C.; Wong, F.N.C.; Baehr-Jones, T. Quantum transport simulations in a programmable nanophotonic processor. Nat. Photonics 2017, 11, 447–452. [Google Scholar] [CrossRef]
- Tambasco, J.L.; Corrielli, G.; Chapman, R.J.; Crespi, A.; Zilberberg, O.; Osellame, R.; Peruzzo, A. Quantum interference of topological states of light. Sci. Adv. 2018, 4, eaat3187. [Google Scholar] [CrossRef]
- Pezze, L.; Smerzi, A.; Oberthaler, M.K.; Schmied, R.; Treutlein, P. Quantum metrology with nonclassical states of atomic ensembles. Rev. Mod. Phys. 2018, 90, 035005. [Google Scholar] [CrossRef]
- Weedbrook, C.; Pirandola, S.; Garcia-Patron, R.; Cerf, N.J.; Ralph, T.C.; Shapiro, J.H.; Lloyd, S. Gaussian quantum information. Rev. Mod. Phys. 2012, 84, 621. [Google Scholar] [CrossRef]
- Ou, Z.Y.J. Multi-Photon Quantum Interference; Springer: New York, NY, USA, 2007; p. 268. [Google Scholar]
- Bromberg, Y.; Lahini, Y.; Morandotti, R.; Silberberg, Y. Quantum and Classical Correlations in Waveguide Lattices. Phys. Rev. Lett. 2009, 102, 253904. [Google Scholar] [CrossRef]
- Politi, A.; Cryan, M.J.; Rarity, J.G.; Yu, S.; O’Brien, J.L. Silica-on-Silicon Waveguide Quantum Circuits. Sience 2008, 320, 646–649. [Google Scholar] [CrossRef]
- Tan, S.H.; Rohde, P.P. The resurgence of the linear optics quantum interferometer–recent advances and applications. Rev. Phys. 2019, 4, 100030. [Google Scholar] [CrossRef]
- Makarov, D.N. Theory of a frequency-dependent beam splitter in the form of coupled waveguides. Sci. Rep. 2021, 11, 5014. [Google Scholar] [CrossRef] [PubMed]
- Zeilinger, A. General properties of lossless beam splitters in interferometry. Am. J. Phys. 1981, 49, 882. [Google Scholar] [CrossRef]
- Campos, R.A.; Saleh, B.E.A.; Teich, M.C. Quantum-mechanical lossless beam splitter: SU(2) symmetry and photon statistics. Phys. Rev. A 1989, 40, 1371. [Google Scholar] [CrossRef] [PubMed]
- Kim, M.S.; Son, W.; Buzek, V.; Knight, P.L. Entanglement by a beam splitter: Nonclassicality as a prerequisite for entanglement. Phys. Rev. A 2002, 65, 032323. [Google Scholar] [CrossRef]
- Makarov, D. Quantum entanglement and reflection coefficient for coupled harmonic oscillators. Phys. Rev. E 2020, 102, 052213. [Google Scholar] [CrossRef] [PubMed]
- Makarov, D.; Gusarevich, E.; Goshev, A.; Makarova, K.; Kapustin, S.; Kharlamova, A.; Tsykareva, Y.V. Quantum entanglement and statistics of photons on a beam splitter in the form of coupled waveguides. Sci. Rep. 2021, 11, 10274. [Google Scholar] [CrossRef] [PubMed]
- Makarov, D.; Tsykareva, Y. Quantum Entanglement of Monochromatic and Non-Monochromatic Photons on a Waveguide Beam Splitter. Entropy 2022, 24, 49. [Google Scholar] [CrossRef]
- Makarov, D.N. Theory of HOM interference on coupled waveguides. Opt. Lett. 2020, 45, 6322–6325. [Google Scholar] [CrossRef]
- Makarov, D.N. Fluctuations in the detection of the HOM effect. Sci. Rep. 2020, 10, 20124. [Google Scholar] [CrossRef]
- Titulaer, U.; Glauber, R. Density Operators for Coherent Fields. Phys. Rev. 1966, 145, 1041. [Google Scholar] [CrossRef]
- Luis, A.; Sfinchez-Soto, L. A quantum description of the beam splitter. Quantum Semiclass. Opt. 1995, 7, 153–160. [Google Scholar] [CrossRef]
- Biedenharn, L.; van Dam, H. Quantum Theory of Angular Momentum; Academic Press: Cambridge, MA, USA, 1965; p. 332. [Google Scholar]
- Publicly Available Data from the Thorlabs Website. Available online: https://www.thorlabs.de (accessed on 1 September 2022).
- Huang, W.P. Coupled-mode theory for optical waveguides: An overview. J. Opt. Soc. Am. A 1994, 11, 963–983. [Google Scholar] [CrossRef]
- Makarov, D.N. Coupled harmonic oscillators and their quantum entanglement. Phys. Rev. E 2018, 97, 042203. [Google Scholar] [CrossRef]
- Tey, M.K.; Chen, Z.; Aljunid, S.A.; Chng, B.; Huber, F.; Maslennikov, G.; Kurtsiefer, C. Strong interaction between light and a single trapped atom without the need for a cavity. Nat. Phys. 2008, 4, 924–927. [Google Scholar] [CrossRef]
- Fearn, H.; Loudon, R. Quantum theory of the lossless beam splitter. Opt. Commun. 1987, 64, 485–490. [Google Scholar] [CrossRef]
- Horodecki, R.; Horodecki, P.; Horodecki, M.; Horodecki, K. Quantum entanglement. Rev. Mod. Phys. 2009, 81, 865. [Google Scholar] [CrossRef]
- Ekert, A. Quantum cryptography based on Bell’s theorem. Phys. Rev. Lett. 1991, 67, 661. [Google Scholar] [CrossRef]
- Bennett, C.H.; Wiesner, S.J. Communication via one- and two-particle operators on Einstein-Podolsky-Rosen states. Phys. Rev. Lett. 1992, 69, 2881. [Google Scholar] [CrossRef]
- Shor, P. Scheme for reducing decoherence in quantum computer memory. Phys. Rev. A 1995, 52, R2493. [Google Scholar] [CrossRef]
- Aspect, A.; Roger, P.G.G. Experimental Tests of Realistic Local Theories via Bell’s Theorem. Phys. Rev. Lett. 1981, 47, 460–463. [Google Scholar] [CrossRef]
- Samuel, L.; Braunstein, H.; Kimble, J. Teleportation of Continuous Quantum Variables. Phys. Rev. Lett. 1998, 80, 869–872. [Google Scholar]
- Chen, Y.F.; Hsieh, M.X.; Ke, H.T.; Yu, Y.T.; Liang, H.C.; Huang, K.F. Quantum entanglement by a beam splitter analogous to laser mode transformation by a cylindrical lens. Opt. Lett. 2021, 46, 5129–5132. [Google Scholar] [CrossRef] [PubMed]
- Hsieh, M.X.; Zheng, X.L.; Yu, Y.T.; Liang, H.C.; Huang, K.F.; Chen, Y.F. Characterizing the spatial entanglement from laser modes analogous to quantum wave functions. Opt. Lett. 2021, 46, 3713–3716. [Google Scholar] [CrossRef] [PubMed]
- Ekert, A.; Knight, P. Entangled quantum systems and the Schmidt decomposition. Am. J. Phys. 1995, 63, 415–423. [Google Scholar] [CrossRef]
- Grobe, R.; Rzazewski, K.; Eberly, J. Measure of electron-electron correlation in atomic physics. J. Phys. B 1994, 27, L503–L508. [Google Scholar] [CrossRef]
- Bennett, C.; Bernstein, H.; Popescu, S.; Schumacher, B. Concentrating partial entanglement by local operations. Phys. Rev. A 1996, 53, 2046–2052. [Google Scholar] [CrossRef]
- Casini, H.; Huerta, M. Entanglement entropy in free quantum field theory. J. Phys. A Math. Theor. 1996, 42, 504007. [Google Scholar] [CrossRef]
- Jiang, Z.; Lang, M.; Caves, C. Mixing nonclassical pure states in a linear-optical network almost always generates modal entanglement. Phys. Rev. A 2013, 88, 044301. [Google Scholar] [CrossRef]
- Berrada, K.; Baz, M.E.; Saif, F.; Hassouni, Y.; Mnia, S. Entanglement generation from deformed spin coherent states using a beam splitter. J. Phys. A Math. Theor. 2009, 42, 285306. [Google Scholar] [CrossRef]
- Xiang-bin, W. Theorem for the beam-splitter entangler. Phys. Rev. A 2002, 66, 024303. [Google Scholar] [CrossRef]
- Makarov, D.N. High Intensity Generation of Entangled Photons in a Two-Mode Electromagnetic Field. Ann. Der Phys. 2017, 549, 1600408. [Google Scholar] [CrossRef]
- Holland, M.; Burnett, K. Interferometric detection of optical phase shifts at the heisenberg limit. Phys. Rev. Lett. 1993, 71, 1355. [Google Scholar] [CrossRef] [PubMed]
- Polino, E.; Valeri, M.; Spagnolo, N.; Sciarrino, F. Photonic Quantum Metrology. AVS Quantum Sci. 2020, 2, 024703. [Google Scholar] [CrossRef]
- Phoenix, S.; Knight, P. Fluctuations and entropy in models of quantum optical resonance. Ann. Phys. 1988, 186, 381–407. [Google Scholar] [CrossRef]
- Gisin, N.; Ribordy, G.; Tittel, W.; Zbinden, H. Quantum cryptography. Rev. Mod. Phys. 2002, 74, 145–195. [Google Scholar] [CrossRef]
- Fearn, H.; Loudon, R. Theory of two-photon interference. J. Opt. Soc. Am. B 1989, 6, 917–927. [Google Scholar] [CrossRef]
- Steinberg, A.; Kwiat, P.; Chiao, R.Y. Dispersion cancellation and high-resolution time measurements in a fourth-order optical interferometer. Phys. Rev. A 1992, 45, 6659. [Google Scholar] [CrossRef]
- Legero, T.; Wilk, T.; Hennrich, M.; Rempe, G.; Peruzzo, A. Quantum Beat of Two Single Photons. Phys. Rev. Lett. 2004, 93, 070503. [Google Scholar] [CrossRef]
- Lyons, A.; Knee, G.; Bolduc, E.; Roger, T.; Leach, J.; Gauger, E.M.; Faccio, D. Attosecond-resolution Hong-Ou-Mandel interferometry. Phys. Rev. Lett. 2018, 4, 9416. [Google Scholar] [CrossRef]
- Wang, K. Quantum theory of two-photon wavepacket interference in a beamsplitter. J. Phys. B At. Mol. Opt. Phys. 2018, 39, R293. [Google Scholar] [CrossRef]
- Branczyk, A.M. Hong-ou-mandel interference. arXiv 2017, arXiv:1711.00080. [Google Scholar]
- Lim, Y.; Beige, A. Generalized Hong–Ou–Mandel experiments with bosons and fermions. New J. Phys. 2005, 7, 155. [Google Scholar] [CrossRef]
- Toyoda, K.; Hiji, R.; Noguchi, A.; Urabe, S. Quantum theory of two-photon wavepacket interference in a beamsplitter. Nature 2015, 527, 74–77. [Google Scholar] [CrossRef] [PubMed]
- Aspect, A. Hanbury Brown and Twiss, Hong Ou and Mandel effects and other landmarks in quantum optics: From photons to atoms. In Current Trends in Atomic Physics; Oxford University Press: Oxford, UK, 2019; pp. 428–449. [Google Scholar]
- Grice, W.; Walmsley, I. Spectral information and distinguishability in type-II down-conversion with a broadband pump. Phys. Rev. A 2000, 56, 1627. [Google Scholar] [CrossRef]
- Erdmann, R.; Branning, D.; Grice, W.; Walmsley, I.A. Restoring dispersion cancellation for entangled photons produced by ultrashort pulses. Phys. Rev. A 2000, 62, 053810. [Google Scholar] [CrossRef]
- Barbieri, M.; Roccia, E.; Mancino, L.; Sbroscia, M.; Gianani, I.; Sciarrino, F. What Hong-Ou-Mandel interference says on two-photon frequency entanglement. Sci. Rep. 2017, 7, 7247. [Google Scholar] [CrossRef] [PubMed]
- Shih, Y. Advances in Atomic, Molecular, and Optical Physics; Bederson, B., Walther, H., Eds.; Academic Press: Cambridge, MA, USA, 1999; Volume 41, pp. 2–42. [Google Scholar]



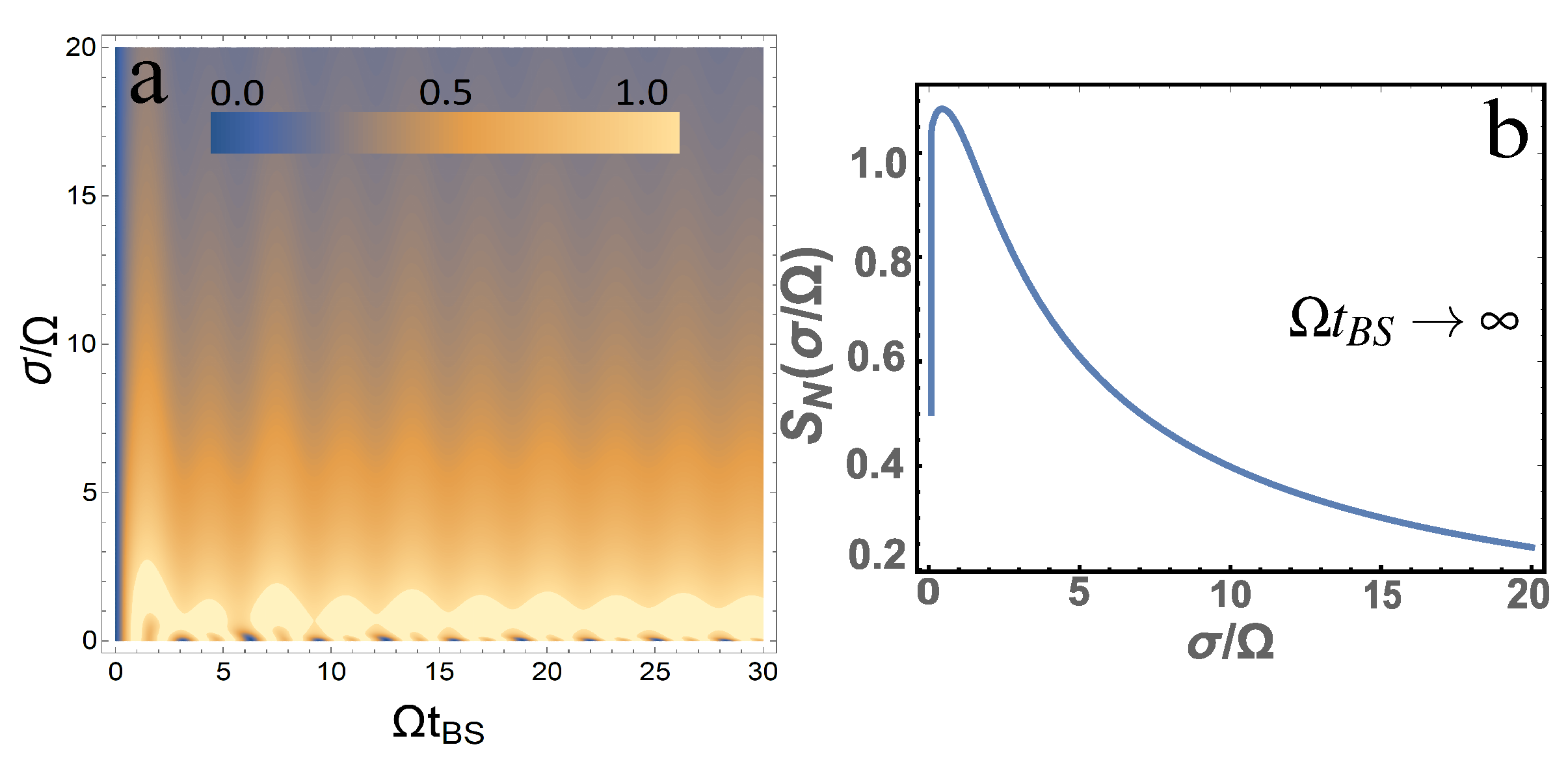




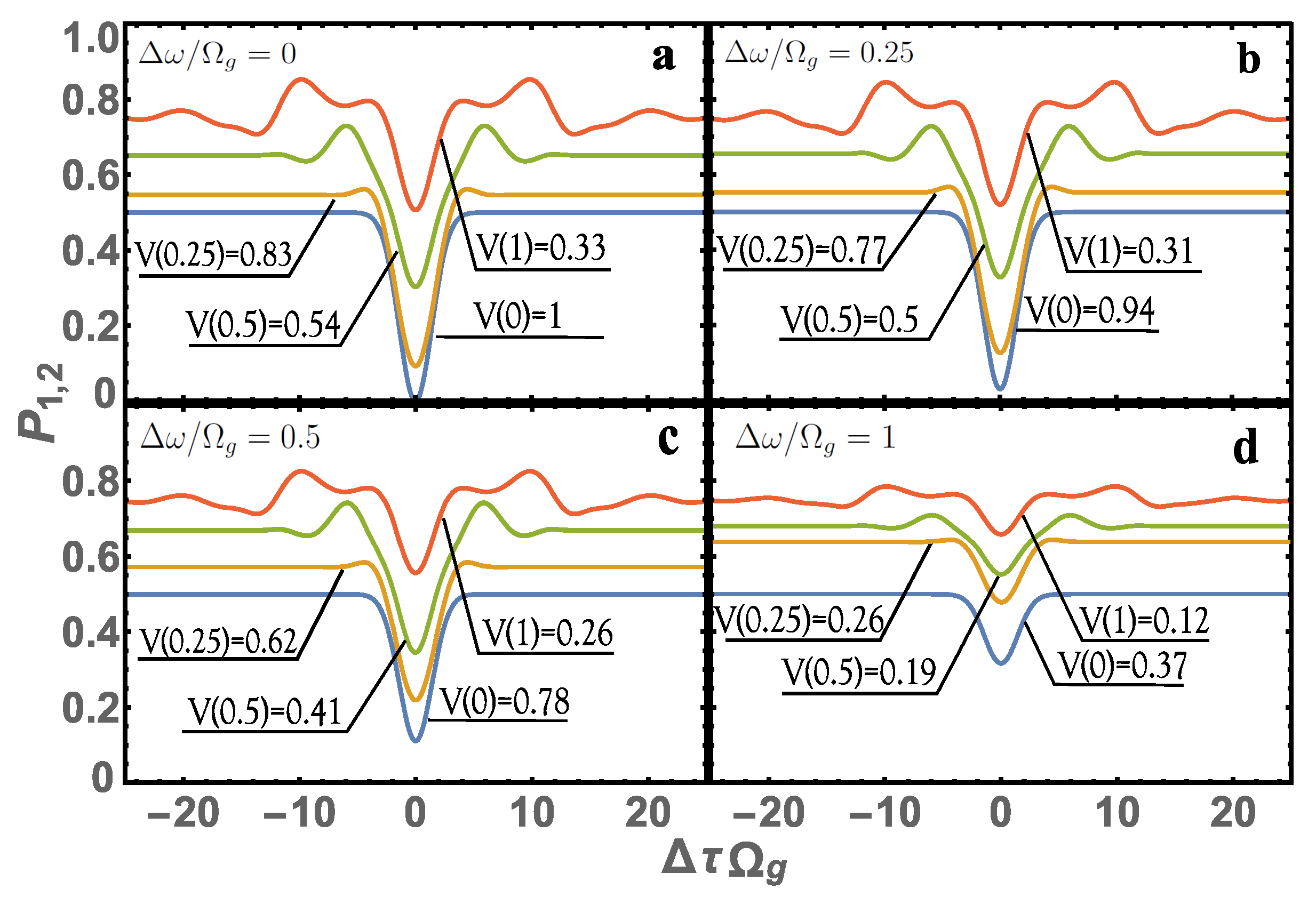
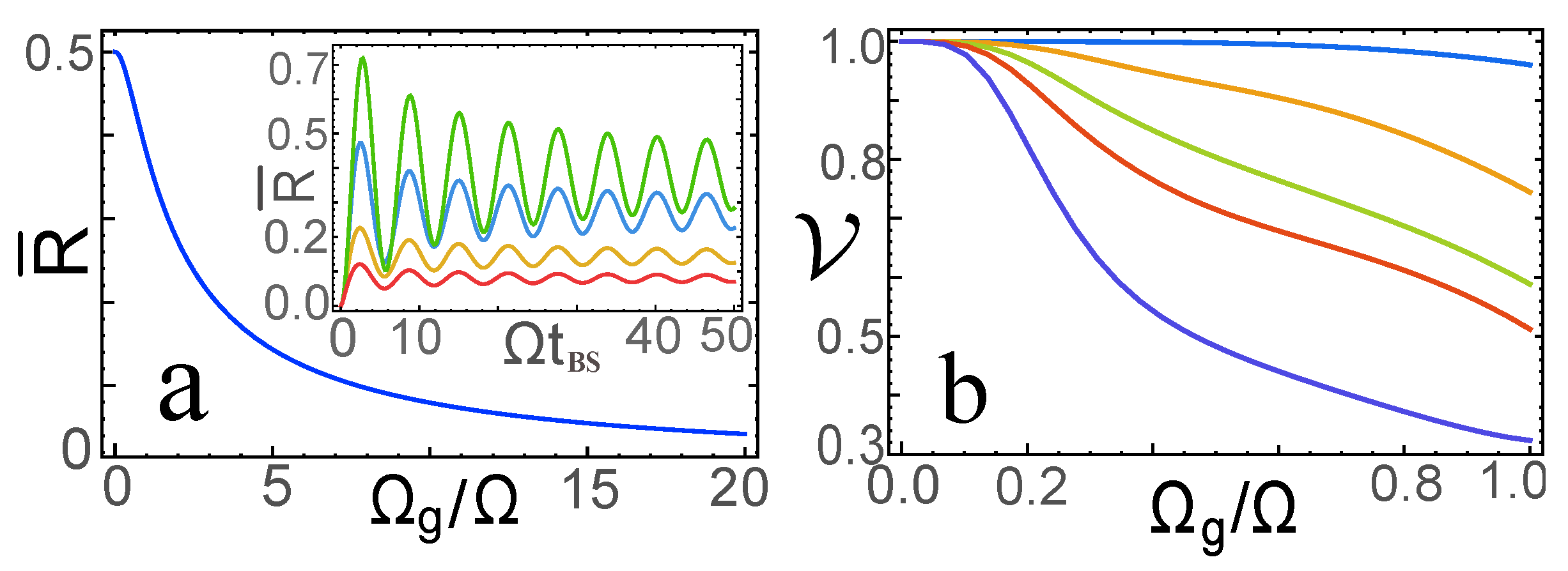
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Makarov, D. Theory for the Beam Splitter in Quantum Optics: Quantum Entanglement of Photons and Their Statistics, HOM Effect. Mathematics 2022, 10, 4794. https://doi.org/10.3390/math10244794
Makarov D. Theory for the Beam Splitter in Quantum Optics: Quantum Entanglement of Photons and Their Statistics, HOM Effect. Mathematics. 2022; 10(24):4794. https://doi.org/10.3390/math10244794
Chicago/Turabian StyleMakarov, Dmitry. 2022. "Theory for the Beam Splitter in Quantum Optics: Quantum Entanglement of Photons and Their Statistics, HOM Effect" Mathematics 10, no. 24: 4794. https://doi.org/10.3390/math10244794
APA StyleMakarov, D. (2022). Theory for the Beam Splitter in Quantum Optics: Quantum Entanglement of Photons and Their Statistics, HOM Effect. Mathematics, 10(24), 4794. https://doi.org/10.3390/math10244794





