Application of Game Method for Modelling and Temporal Intuitionistic Fuzzy Pairs to the Forest Fire Spread in the Presence of Strong Wind
Abstract
:1. Introduction
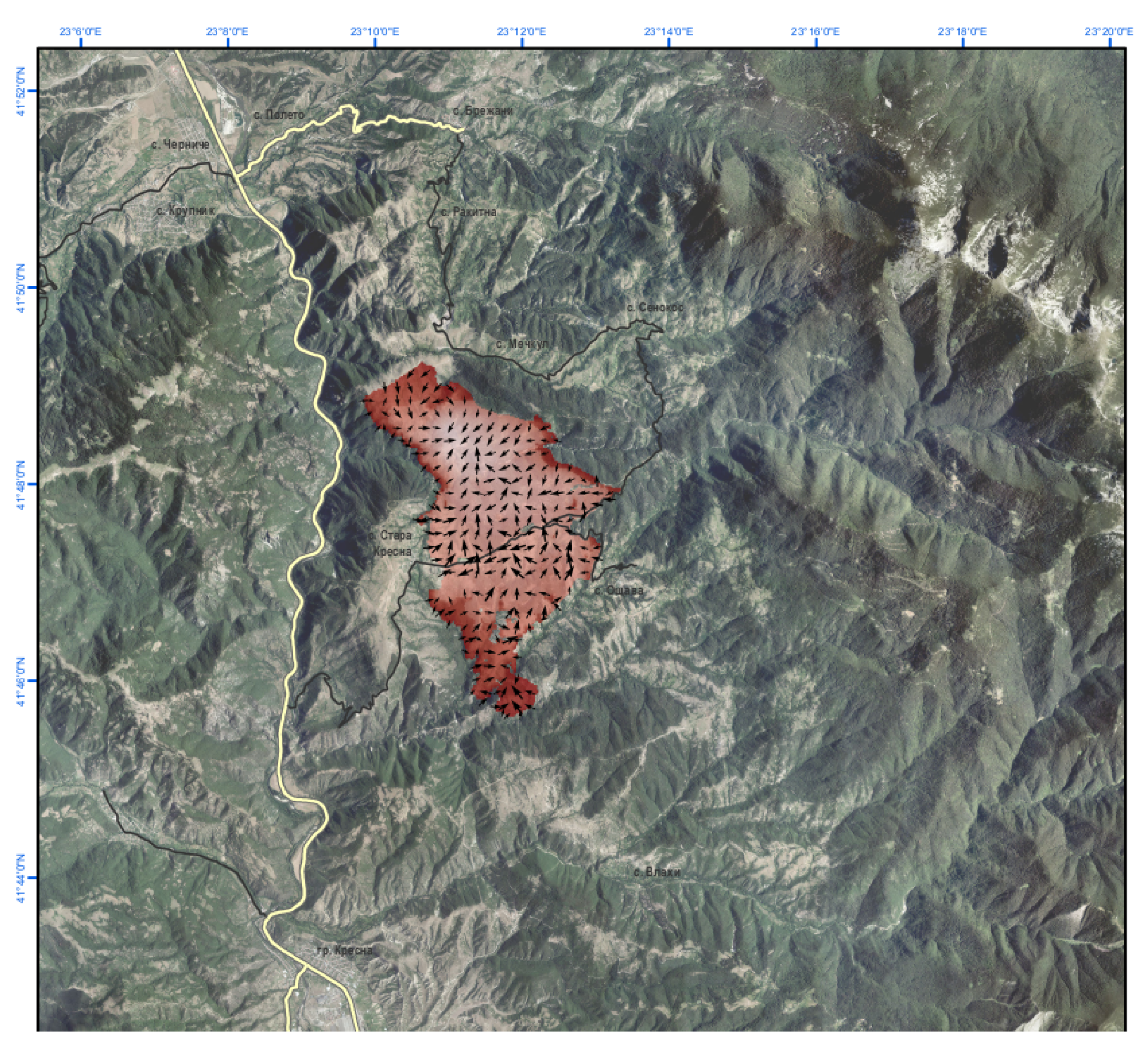
2. Wildfire in the Kresna Gorge, Bulgaria
3. Definition and Properties of Temporal Intuitionistic Fuzzy Pairs
4. Game Method for Modelling
4.1. GMM Basics
- [A.1.]
- ;
- [A.2.]
- ;
- [A.3.]
- ;
- [A.4.]
- for .
4.2. Application of GMM to Forest Fire Spread in the Presence of Wind
- (1)
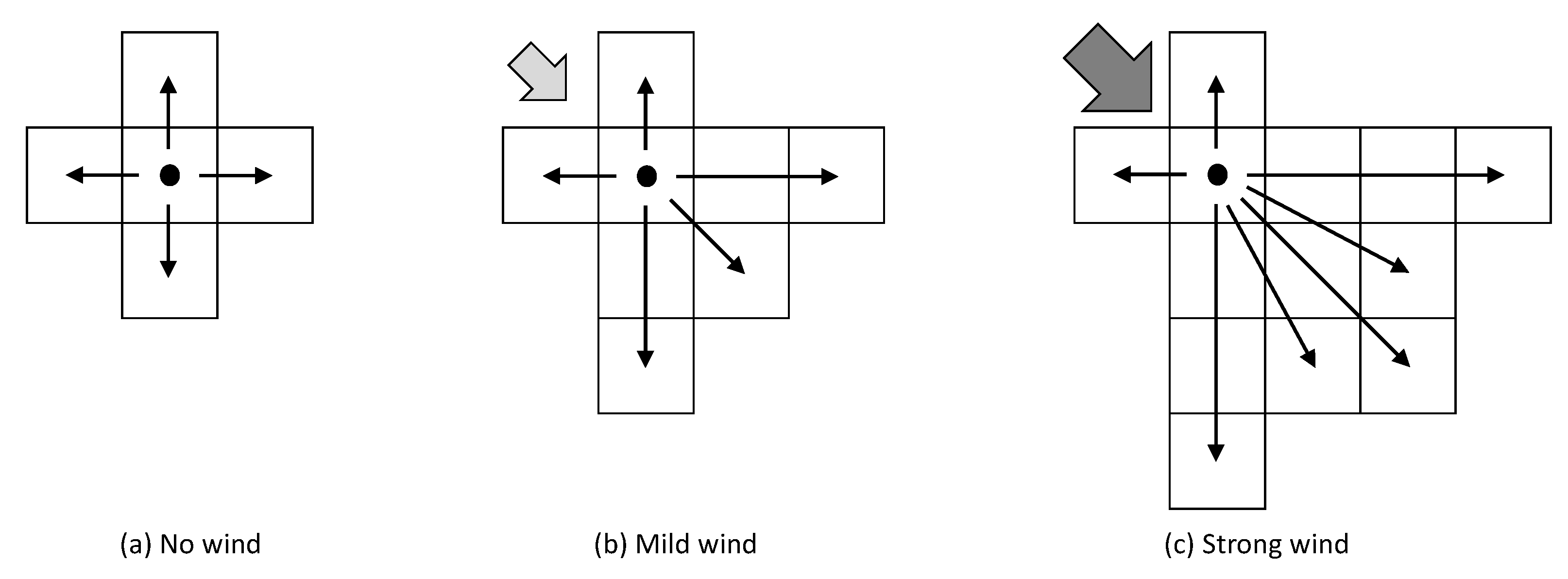
- The way of defining the new borders of the fire spread zone is visualized in Figure 5 for the three idealized cases of: (a) no fire, (b) mild wind, or (c) strong wind. (Nota bene: Here we visualize the three cases specifically for northwest wind).In particular, for every currently affected cell at this step, its own zone of subsequent fire spread is expanded:
- (a)
- with its four neighbouring cells, as shown in Figure 5a;
- (b)
- with its four neighbouring cells and the additional three cells in the direction of the mild wind as shown in Figure 5b, i.e., each currently burning cell affects seven other cells at the subsequent iteration;
- (c)
- with its four neighbouring cells and the additional eight cells in the direction of the strong wind as shown in Figure 5c, i.e., each currently burning cell affects 12 other cells at the subsequent iteration.
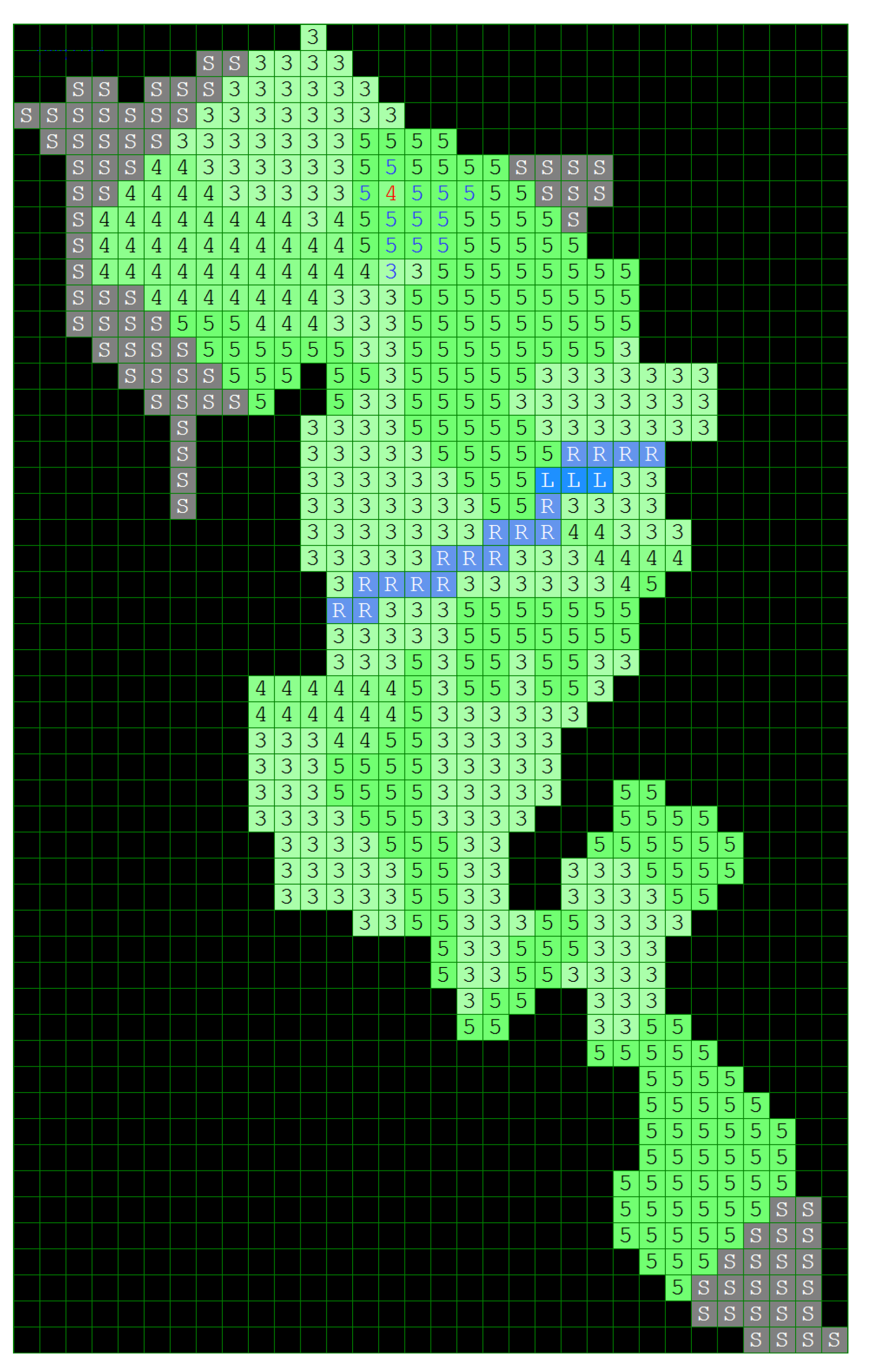
When these zones of subsequent fire spread are defined for all the currently affected (burning) cells at this step, the cumulative area, obtained as a union of these zones, defines the complete zone that at the next step will be affected (burning). - (2)
- Burning is represented by:
- −
- either leaving them as unchanged (according to rules [A.1.] to [A.3.] from Section 4.1) for unaffectable cells such as or S,
- −
- or decrementing them by 1 (according to rule [A.4.]) for the cells that are affectable, i.e., represent combustible forest mass labeled with a number in the interval with respect to the density of the “fuel”;
- (3)
- The algorithm terminates when all the cells in the grid reach the value of 0, meaning that all cells containing any flammable material have already burnt out, and/or the remaining cells are ones that may not be affected by the firespread.
5. Model and Simulation
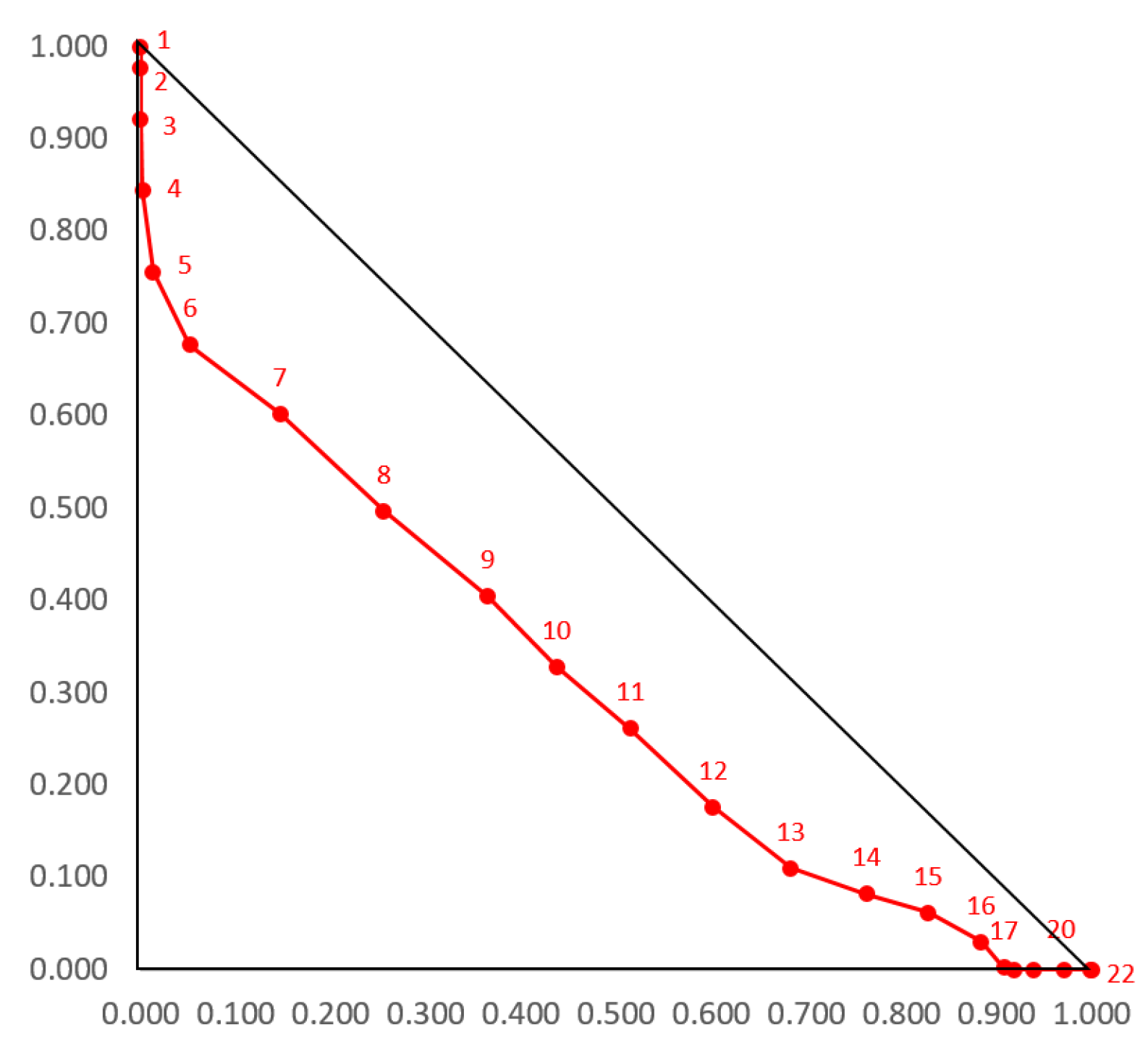
6. Results and Discussion
7. Conclusions and Directions for Future Work
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Beighley, M.; Hyde, A. Portugal Wildfire Management in a New Era Assessing Fire Risks, Resources and Reforms, Instituto Superior D Agronomia, Universidade de Lisboa. 2018. Available online: https://www.isa.ulisboa.pt/files/cef/pub/articles/2018-04/2018_Portugal_Wildfire_Management_in_a_New_Era_Engish.pdf (accessed on 14 March 2022).
- Živanović, S. Impact of drought in Serbia on fire vulnerability of forests. Int. J. Bioautom. 2017, 21, 217–226. [Google Scholar]
- Christensen, B.; Herries, D.; Hartley, R.J.L.; Parker, R. UAS and smartphone integration at wildfire management in Aotearoa New Zealand. N. Z. J. For. Sci. 2012, 51, 10. [Google Scholar] [CrossRef]
- Fernandez-Anez, N.; Krasovskiy, A.; Müller, M.; Vacik, H.; Baetens, J.; Hukić, E.; Solomun, M.K.; Atanassova, I.; Glushkova, M.; Bogunović, I.; et al. Current Wildland Fire Patterns and Challenges in Europe: A Synthesis of National Perspectives. Air Soil Water Res. 2021, 14, 1–19. [Google Scholar] [CrossRef]
- Dorrer, G.A.; Yarovoy, S.V. Description of wildfires spreading and extinguishing with the aid of agent-based models. IOP Conf. Ser. Mater. Sci. Eng. 2020, 822, 012010. [Google Scholar] [CrossRef]
- Rybski, D.; Butsic, V.; Kantelhardt, J.W. Self-organized multistability in the forest fire model. Phys. Rev. E 2021, 104, L012201. [Google Scholar] [CrossRef] [PubMed]
- Ghodrat, M.; Shakeriaski, F.; Nelson, D.; Simeoni, A. Existing Improvements in Simulation of Fire–Wind Interaction and Its Effects on Structures. Fire 2021, 4, 27. [Google Scholar] [CrossRef]
- Atanassov, K. Game Method for Modelling; “Prof. M. Drinov” Academic Publishing House: Sofia, Bulgaria, 2011. [Google Scholar]
- Atanassova, L.; Atanassov, K. On a game-method for modelling with intuitionistic fuzzy estimations: Part 1. Lect. Notes Comput. Sci. 2012, 7116, 182–189. [Google Scholar]
- Gardner, M. The Unexpected Hanging and Other Mathematical Diversions; Simon & Schuster: New York, NY, USA, 1969. [Google Scholar]
- Gardner, M. The fantastic combinations of John Conway’s new solitaire game ‘life’. Sci. Am. 1970, 223, 120–123. [Google Scholar] [CrossRef]
- Sotirova, E.; Dobrinkova, N.; Atanassov, K. On some applications of game method for modelling. Part 2: Development of forest fire. Proc. Jangjeon Math. Soc. 2012, 15, 335–342. [Google Scholar]
- Sotirova, E.; Atanassov, K.; Fidanova, S.; Velizarova, E.; Vassilev, P.; Shannon, A. Application of the game method for modelling the forest fire perimeter expansion. Part 2: A model fire intensity with effect of wind. In Proceedings of the IFAC Workshop on Dynamics and Control in Agriculture and Food Processing, Plovdiv, Bulgaria, 13–16 June 2012; pp. 165–169. [Google Scholar]
- Velizarova, E.; Sotirova, E.; Atanassov, K.; Vassilev, P.; Fidanova, S. On the game method for the forest fire spread modelling with considering the wind effect. In Proceedings of the 6th IEEE International Conference Intelligent Systems, Sofia, Bulgaria, 6–8 September 2012; pp. 216–220. [Google Scholar]
- Sotirova, E.; Atanassov, K.; Fidanova, S.; Velizarova, E.; Vassilev, P.; Shannon, A. Application of the game method for modelling the forest fire perimeter expansion. Part 3: A model of the forest fire speed propagation in different homogenous vegetation types. In Proceedings of the IFAC Workshop on Dynamics and Control in Agriculture and Food Processing, Plovdiv, Bulgaria, 13–16 June 2012; pp. 171–174. [Google Scholar]
- Green, M.E.; Deluca, T.F.; Kaiser, K.W.D. Modelling wildfire using evolutionary cellular automata. In Proceedings of the GECCO 2020—Proceedings of the 2020 Genetic and Evolutionary Computation Conference, Cancún, Mexico, 8–12 July 2020; pp. 1089–1097. [Google Scholar] [CrossRef]
- Sun, W.; Wei, W.; Chen, J.; Ren, K. Research on Amazon Forest Fire Based on Cellular Automata Simulation. In Proceedings of the 20th International Symposium on Distributed Computing and Applications for Business Engineering and Science, DCABES, Nanning, China, 10–12 December 2021; pp. 175–178. [Google Scholar] [CrossRef]
- Zhao, Y.; Geng, D. Simulation of Forest Fire Occurrence and Spread Based on Cellular Automata Model. In Proceedings of the ICAIIS 2021: 2021 2nd International Conference on Artificial Intelligence and Information Systems, Chongqing, China, 28–30 May 2021. Article Number 3471332. [Google Scholar] [CrossRef]
- Alexandridis, A.; Vakalis, D.; Siettos, C.I.; Bafas, G.V. A cellular automata model for forest fire spread prediction: The case of the wildfire that swept through Spetses Island in 1990. Appl. Math. Comput. 2008, 204, 191–201. [Google Scholar] [CrossRef]
- Li, X.; Zhang, M.; Zhang, S.; Liu, J.; Sun, S.; Hu, T.; Sun, L. Simulating Forest Fire Spread with Cellular Automation Driven by a LSTM Based Speed Model. Fire 2022, 5, 13. [Google Scholar] [CrossRef]
- Bureva, V.; Atanassova, L.; Atanassov, K. Game method for modelling with temporal intuitionistic fuzzy evaluations for locating the wildfire ignition point. Notes Intuit. Fuzz. Sets 2020, 26, 90–106. [Google Scholar] [CrossRef]
- Bureva, V.; Atanassova, L.; Atanassov, K.; Delkov, A. Application of the Game Method for Modelling for Locating the Wildfire Ignition Point. In Proceedings of the 4th International Conference on Numerical and Symbolic Computation Developments and Applications, ISEP—Instituto Superior de Engenharia do Porto, Porto, Portugal, 11–12 April 2019; pp. 397–413. [Google Scholar]
- Atanassov, K. On Intuitionistic Fuzzy Sets Theory; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Atanassov, K. Intuitionistic Fuzzy Logics; Springer: Cham, Switzerland, 2017. [Google Scholar]
- FlamMap, The Missoula Fire Sciences Lab. Available online: https://www.firelab.org/project/flammap (accessed on 14 March 2022).
- Technical Report PC-01-33-367, GeoPolymorphic. Available online: https://forestfires.info/techreport (accessed on 14 March 2022). (In Bulgarian)
- Mavrov, D.; Bureva, V. FireGrid—Software for 2D fire spread simulation using Game Method of Modelling. Int. J. Bioautom. 2022, 26, 5–18. [Google Scholar] [CrossRef]
- Wikipedia Contributors. Firebreak. Wikipedia, The Free Encyclopedia. 19 November 2021. 03:05 UTC. Available online: https://en.wikipedia.org/w/index.php?title=Firebreak&oldid=1055998934 (accessed on 14 March 2022).
- Khan, N.; Moinuddin, K. The role of heat flux in an idealised firebreak built in surface and crown fires. Atmosphere 2021, 12, 1395. [Google Scholar] [CrossRef]
- Moinuddin, K.; Khan, N.; Sutherland, D. Numerical study on effect of relative humidity (and fuel moisture) on modes of grassfire propagation. Fire Saf. J. 2021, 125, 103422. [Google Scholar] [CrossRef]












Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mavrov, D.; Atanassova, V.; Bureva, V.; Roeva, O.; Vassilev, P.; Tsvetkov, R.; Zoteva, D.; Sotirova, E.; Atanassov, K.; Alexandrov, A.; et al. Application of Game Method for Modelling and Temporal Intuitionistic Fuzzy Pairs to the Forest Fire Spread in the Presence of Strong Wind. Mathematics 2022, 10, 1280. https://doi.org/10.3390/math10081280
Mavrov D, Atanassova V, Bureva V, Roeva O, Vassilev P, Tsvetkov R, Zoteva D, Sotirova E, Atanassov K, Alexandrov A, et al. Application of Game Method for Modelling and Temporal Intuitionistic Fuzzy Pairs to the Forest Fire Spread in the Presence of Strong Wind. Mathematics. 2022; 10(8):1280. https://doi.org/10.3390/math10081280
Chicago/Turabian StyleMavrov, Deyan, Vassia Atanassova, Veselina Bureva, Olympia Roeva, Peter Vassilev, Radoslav Tsvetkov, Dafina Zoteva, Evdokia Sotirova, Krassimir Atanassov, Alexander Alexandrov, and et al. 2022. "Application of Game Method for Modelling and Temporal Intuitionistic Fuzzy Pairs to the Forest Fire Spread in the Presence of Strong Wind" Mathematics 10, no. 8: 1280. https://doi.org/10.3390/math10081280
APA StyleMavrov, D., Atanassova, V., Bureva, V., Roeva, O., Vassilev, P., Tsvetkov, R., Zoteva, D., Sotirova, E., Atanassov, K., Alexandrov, A., & Tsakov, H. (2022). Application of Game Method for Modelling and Temporal Intuitionistic Fuzzy Pairs to the Forest Fire Spread in the Presence of Strong Wind. Mathematics, 10(8), 1280. https://doi.org/10.3390/math10081280










