On Some Properties of the First Brocard Triangle in the Isotropic Plane

Abstract

In this paper we introduce the first Brocard triangle of an allowable triangle in the isotropic plane and derive the coordinates of its vertices in the case of a standard triangle. We prove that the first Brocard triangle is homologous to the given triangle and that these two triangles are parallelogic. We consider the relationships between the first Brocard triangle and the Steiner axis, the Steiner point, and the Kiepert parabola of the triangle. We also investigate some other interesting properties of this triangle and consider relationships between the Euclidean and the isotropic case.

1. Introduction and Motivation

The isotropic (or Galilean) plane is a projective-metric plane, where the absolute consists of one line—the absolute line ${\omega }_A$, and one point on that line—the absolute point ${\Omega }_A$.

When using homogeneous coordinates in the projective plane, $P=(x_0:x_1:x_2)$, $x_0^2+x_1^2+x_2^2\ne 0$, then we choose the absolute point ${\Omega }_A=(0:1:0)$ and the absolute line ${\omega }_A$ having the equation $x_2=0$. Points incident to the absolute line ${\omega }_A$ are called isotropic points and lines incident to the absolute point ${\Omega }_A$ are called isotropic lines. We will mention a few well known metric quantities in the isotropic plane for which we assume that $x=\frac{x_0}{x_2}$ and $y=\frac{x_1}{x_2}$.

Two lines are called parallel if they have the same isotropic point. Points which lie on the same isotropic line are said to be parallel.

For two non-parallel points $P_1=(x_1,y_1)$ and $P_2=(x_2,y_2)$ the isotropic distance is defined as $d(P_1,P_2):=x_2-x_1$. Notice that the isotropic distance is directed. For two parallel points $P_1=(x_1,y_1)$ and $P_2=(x_1,y_2)$, the isotropic span is defined as $s(P_1,P_2):=y_2-y_1$. The midpoint of the points $P_1=(x_1,y_1)$ and $P_2=(x_2,y_2)$ is defined as $M=\left(\frac{x_1+x_2}{2},\frac{y_1+y_2}{2}\right)$.

The angle formed by non-isotropic lines $l_1$ and $l_2$ given by $y=m_{1}x+b_1$ and $y=m_{2}x+b_2$ is defined by $\phi =\angle (l_1,l_2):=m_2-m_1$, and it is directed. The bisector of the lines $l_1$ and $l_2$ is given by the equation $y=\frac{m_1+m_2}{2}x+\frac{b_1+b_2}{2}$. A normal line to a line l at a point P is the isotropic line n passing through P.

All projective transformations that preserve the absolute figure are of the form

$$\begin{array}{cc}\hfill \overline{x}& =a+px,a,b,c,p,q\in \mathbf{R},\hfill \\ \hfill \overline{y}& =b+cx+qy,pq\ne 0,\hfill \end{array}$$

and form the 5-parametric group $G_5$ known as the group of similarities of the isotropic plane (see [1]).

Distances, spans and angles are kept invariant under the subgroup $G_3$ of $G_5$ which consists of transformations of the form

$$\begin{array}{cc}\hfill \overline{x}& =a+x\hfill \\ \hfill \overline{y}& =b+cx+y,a,b,c\in \mathbf{R}.\hfill \end{array}$$

$G_3$ is called the motion group of the isotropic plane.

Metric quantities and all the facts related to the geometry of the isotropic plane can be found in [1,2].

A triangle is called allowable if none of its sides are isotropic [1]. As it is explained in [3], according to [1], to any allowable triangle in the isotropic plane there is exactly one circumscribed circle. The equation of this circle is of the form $y=u{x}^2+vx+w$, $u\ne 0$. Choosing a suitable coordinate system and applying the group of similarities, we may assume that the equation of this circle is $y=x^2$, and that the vertices of the allowable triangle $ABC$ are $A=(a,a^2)$, $B=(b,b^2)$, $C=(c,c^2)$, where a, b, and c are mutually different numbers. For convenience, we will frequently use abbreviations $abc=p$ and $ab+bc+ca=q$. Choosing, without loss of generality, that $a+b+c=0$, the diameter of the circle circumscribed to the triangle $ABC$, passing through its centroid $G=\left(\frac{a+b+c}{3},\frac{a^2+b^2+c^2}{3}\right)=\left(0,-\frac{2}{3}q\right)$, lies on the y-axis, while the x-axis is tangent to this circle at the endpoint of that diameter.

For each allowable triangle $ABC$, one can, in the described way, achieve that its circumscribed circle has the equation $y=x^2$, and its vertices are of the form $A=(a,a^2)$, $B=(b,b^2)$, and $C=(c,c^2)$, with $a+b+c=0$. We shall say that such a triangle is in the standard position, or shorter, that the triangle $ABC$ is the standard triangle. To prove geometric facts for allowable triangles, it is sufficient to give a proof for a standard triangle. Its sides $BC$, $CA$, and $AB$ have equations $y=-ax-bc$, $y=-bx-ca$, and $y=-cx-ab$. Using the mentioned notations it can be proved that $q=bc-a^2$ and $(c-a)(a-b)=2q-3bc$.

The tangential triangle of a given triangle $ABC$ is the triangle $A_t{B}_t{C}_t$ determined by the three tangents to the circumscribed circle of the triangle $ABC$ at its vertices. It can be proved that the lines $A{A}_t$, $B{B}_t$, and $C{C}_t$ are symmetric, with respect to bisectors of the angles A, B, and C, to the medians $AG$, $BG$, and $CG$ of the triangle $ABC$. The lines $A{A}_t$, $B{B}_t$, and $C{C}_t$ meet at the point K which is called the symmedian center of the triangle $ABC$.

Let $ABC$ be a standard triangle and let $\mathcal{A}$, $\mathcal{B}$, and $\mathcal{C}$, respectively ${\mathcal{A}}^{\prime }$, ${\mathcal{B}}^{\prime }$, and ${\mathcal{C}}^{\prime }$, be lines through the points A, B, and C such that $\angle (AB,\mathcal{A})=\angle (BC,\mathcal{B})=\angle (CA,\mathcal{C})=:\phi$, $\angle ({\mathcal{A}}^{\prime },AC)=\angle ({\mathcal{B}}^{\prime },BA)=\angle ({\mathcal{C}}^{\prime },CB)=:\psi$. In [4] it is proved that the lines $\mathcal{A}$, $\mathcal{B}$, and $\mathcal{C}$ pass through a common point, say ${\Omega }_1$, if and only if $\phi =\omega$, and the lines ${\mathcal{A}}^{\prime }$, ${\mathcal{B}}^{\prime }$, and ${\mathcal{C}}^{\prime }$ pass through a common point, say ${\Omega }_2$, if and only if $\psi =\omega$, where $\omega$ is given by $\omega =-\frac{1}{3q}(b-c)(c-a)(a-b)$. The points ${\Omega }_1$ and ${\Omega }_2$ are called Crelle–Brocard points, and $\omega$ is called the Brocard angle.

The isotropic analogue of Brocard’s theorem was first obtained in [2].

The standard triangle $ABC$ has, by [4], the symmedian center K and Crelle–Brocard points ${\Omega }_1$ and ${\Omega }_2$ given by

$$K=\left(\frac{3p}{2q},-\frac{q}{3}\right),{\Omega }_1=\left(\frac{p-p_1}{q},\frac{27{p_1}^2-2q^3}{9q^2}\right),{\Omega }_2=\left(\frac{p-p_2}{q},\frac{27{p_2}^2-2q^3}{9q^2}\right)$$

(1)

where $p_1=\frac{1}{3}(b{c}^2+c{a}^2+a{b}^2)$, $p_2=\frac{1}{3}(b^{2}c+c^{2}a+a^{2}b)$. One can prove that $p_1+p_2+p=0$, $p_1^2+p_1{p}_2+p_2^2=-\frac{q^3}{9}$, $p^2+p{p}_1+p_1^2=-\frac{q^3}{9}$, and $p^2+p{p}_2+p_2^2=-\frac{q^3}{9}$.

These three points lie, according to [5], on the Brocard circle of the triangle $ABC$ (see Figure 1), given by

$$y=2x^2-\frac{3p}{q}x-\frac{q}{3}.$$

(2)

If $A_m$, $B_m$, and $C_m$ are midpoints of the sides $BC$, $CA$, and $AB$ of the allowable triangle $ABC$, and $A^{\prime }$, $B^{\prime }$ and $C^{\prime }$ are points on the perpendicular bisectors of these sides such that spans $s(A_m,A^{\prime })$, $s(B_m,B^{\prime })$, and $s(C_m,C^{\prime })$ are proportional to the lengths of sides $BC$, $CA$, and $AB$, then the points $BC\cap B^{\prime }{C}^{\prime }$, $CA\cap C^{\prime }{A}^{\prime }$, and $AB\cap A^{\prime }{B}^{\prime }$ lie on a line, call it $\mathcal{T}$. Triangles $A^{\prime }{B}^{\prime }{C}^{\prime }$ are the so-called Kiepert triangles of the triangle $ABC$, and the line $\mathcal{T}$ is the axis of homology of the triangle $ABC$ and the corresponding Kiepert triangle $A^{\prime }{B}^{\prime }{C}^{\prime }$. Axes of homology of an allowable triangle $ABC$ and its Kiepert triangles envelope a parabola which is called the Kiepert parabola [6].

The inscribed and the circumscribed Steiner’s ellipses of an allowable triangle have the same nonisotropic axis, which passes through the centroid G of that triangle and which in the case of a standard triangle has equation $y=-\frac{3p}{2q}x-\frac{2}{3}q$. This axis is called the Steiner’s axis of the considered triangle. In [7], the Steiner point of the allowable triangle $ABC$ is defined as the fourth (the first three being A, B, and C) common point S of the circumscribed circle and the circumscribed Steiner ellipse of that triangle. If $ABC$ is a standard triangle then $S=\left(-\frac{3p}{q},\frac{9p^2}{q^2}\right)$.

2. The First Brocard Triangle of a Triangle in the Isotropic Plane

In this section we will define the first Brocard triangle of a triangle in the isotropic plane.

Theorem 1.

Given a standard triangle $ABC$, the lines through its symmedian center K and parallel to its sides $BC$, $CA$, and $AB$ meet the Brocard circle, besides the point K, at points

$$\begin{array}{cc}\hfill A_1& =\left(-\frac{a}{2},\frac{1}{6q}(9ap+3a^{2}q-2q^2)\right),\hfill \\ \hfill B_1& =\left(-\frac{b}{2},\frac{1}{6q}(9bp+3b^{2}q-2q^2)\right),\hfill \\ \hfill C_1& =\left(-\frac{c}{2},\frac{1}{6q}(9cp+3c^{2}q-2q^2)\right),\hfill \end{array}$$

(3)

which lie on bisectors of the sides $BC$, $CA$, and $AB$, respectively (see Figure 1).

Proof. 

The point $A_1$ obviously lies on the bisector of $BC$, and with $x=-\frac{a}{2}$, from (2) and

$$y=-ax+\frac{3ap}{2q}-\frac{q}{3},$$

(4)

we get the ordinate of the point $A_1$

$$y=\frac{a^2}{2}+\frac{3ap}{2q}-\frac{q}{3}=\frac{1}{6q}(9ap+3a^{2}q-2q^2).$$

Therefore, $A_1$ is the intersection of that line and the Brocard circle (2). This line is parallel to $BC$ and passes through K, see (1). □

The points $A_1$, $B_1$, and $C_1$ from Theorem 1 determine the first Brocard triangle of the triangle $ABC$ (see Figure 1).

Theorem 2.

The sides of the first Brocard triangle $A_1{B}_1{C}_1$ of the standard triangle $ABC$ are given by

$$\begin{array}{ccc}\hfill B_1{C}_1& \dots & y=\left(a-\frac{3p}{q}\right)x-\frac{1}{6}(2q+3bc),\hfill \\ \hfill C_1{A}_1& \dots & y=\left(b-\frac{3p}{q}\right)x-\frac{1}{6}(2q+3ca),\hfill \\ \hfill A_1{B}_1& \dots & y=\left(c-\frac{3p}{q}\right)x-\frac{1}{6}(2q+3ab).\hfill \end{array}$$

(5)

Proof. 

The point $B_1$ satisfies the first equation in (5) because

$$\left(a-\frac{3p}{q}\right)\left(-\frac{b}{2}\right)-\frac{1}{6}(2q+3bc)=\frac{1}{6q}\left(9bp+3b^{2}q-2q^2\right),$$

and so does the point $C_1$. □

Theorem 3.

A triangle and its first Brocard triangle have the same centroid (see Figure 1).

Proof. 

According to [3], the triangle $ABC$ has the centroid $G=\left(0,-\frac{2}{3}q\right)$. The triangle $A_1{B}_1{C}_1$ with vertices (3), has the same centroid because $a+b+c=0$ and

$$\frac{1}{3}·\frac{1}{6q}\left(9(a+b+c)p+3(a^2+b^2+c^2)q-6q^2\right)=\frac{1}{18q}\left(3q(-2q)-6q^2\right)=-\frac{2}{3}q.$$

□

3. The First Brocard Triangle and Some Other Significant Elements

In this section we consider the relationships between the first Brocard triangle and some other objects related to a triangle in the isotropic plane.

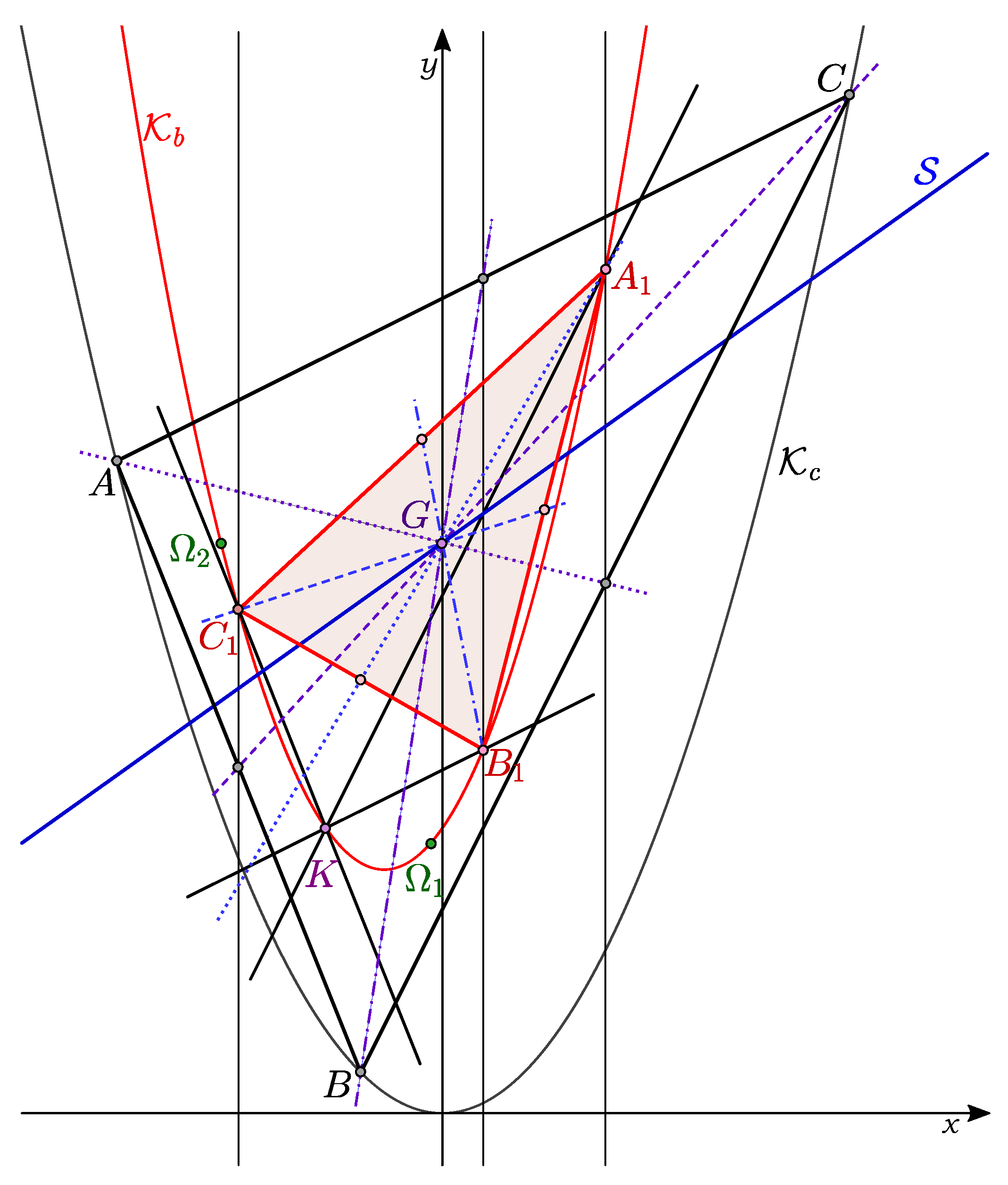
Theorem 4.

Let G be the centroid and $A_1{B}_1{C}_1$ the first Brocard triangle of an allowable triangle $ABC$. Then the pairs of lines $GA$, $G{A}_1$; $GB$, $G{B}_1$; and $GC$, $G{C}_1$ have the same bisector. This bisector is the Steiner axis of the triangle $ABC$ (Figure 1).

For the Euclidean case see [8,9].

Proof. 

The lines $GA$ and $G{A}_1$ have slopes

$$\begin{array}{cc}\hfill \frac{a^2+\frac{2}{3}q}{a}& =a+\frac{2q}{3a},\hfill \\ \hfill \frac{\frac{1}{6q}(9ap+3a^{2}q-2q^2)+\frac{2}{3}q}{-\frac{a}{2}}& =-\frac{1}{3aq}(9ap+3a^{2}q+2q^2)=-\frac{3p}{q}-a-\frac{2q}{3a}.\hfill \end{array}$$

The sum of slopes is equal to $-\frac{3p}{q}$, therefore the bisector of these lines has the slope $-\frac{3p}{2q}$. The Steiner axis of the triangle $ABC$ is given by $y=-\frac{3p}{2q}x-\frac{2}{3}q$ [7], which passes through the centroid $G=\left(0,-\frac{2}{3}q\right)$, and coincides with this bisector. □

Theorem 5.

The first Brocard triangle $A_1{B}_1{C}_1$ of an allowable triangle $ABC$ is homologous with this triangle, and the center of homology is $K^{\prime }$—the point reciprocal to the symmedian center of the triangle $ABC$ (see Figure 2).

For the Euclidean case see [9].

Proof. 

The line

$$9aqy=(2q^2+3a^{2}q-9ap)x+9a^{2}p+6a^{3}q-2a{q}^2$$

passes through the point $A=(a,a^2)$ and also through the point $A_1$, hence it is the line $A{A}_1$. In addition, this line passes through the point

$$K^{\prime }=\left(-\frac{3p}{q},\frac{27p^2-8q^3}{9q^2}\right),$$

(6)

which is, by [5], reciprocal to the symmedian center K and anticomplementary to the midpoint of Crelle–Brocard points ${\Omega }_1$ and ${\Omega }_2$. The same also holds for analogous lines $B{B}_1$ and $C{C}_1$. □

Theorem 6.

In case of the standard triangle $ABC$, the axis of homology of triangles $ABC$ and $A_1{B}_1{C}_1$ from Theorem 5, has the equation

$$y=-\frac{6p}{q}x-\frac{q}{6}.$$

(7)

Proof. 

It is enough to prove e.g., that the point $\left(\frac{q}{6a},-\frac{q}{6}-bc\right)$ lies on lines $BC$ and $B_1{C}_1$, and on the line defined by (7).

Indeed, we have

$$\begin{array}{cc}\hfill -a·\frac{q}{6a}-bc& =-\frac{q}{6}-bc,\hfill \\ \hfill \left(a-\frac{3p}{q}\right)·\frac{q}{6a}-\frac{1}{6}(2q+3bc)& =\frac{q}{6}-\frac{bc}{2}-\frac{q}{3}-\frac{bc}{2}=-\frac{q}{6}-bc,\hfill \\ \hfill -\frac{6p}{q}·\frac{q}{6a}-\frac{q}{6}& =-\frac{q}{6}-bc.\hfill \end{array}$$

□

In the discussion following Theorem 2 in [6] it is shown that the Kiepert triangle $A^{\prime }{B}^{\prime }{C}^{\prime }$ of a triangle $ABC$, with $t=-\omega$, coincides with the first Brocard triangle $A_1{B}_1{C}_1$ of the triangle $ABC$ and the axis of homology of triangles $ABC$ and $A_1{B}_1{C}_1$ touches the Kiepert parabola of the triangle $ABC$ (see Figure 2).

Theorem 7.

The triangle $ABC$ and its first Brocard triangle $A_1{B}_1{C}_1$ are three-homologous, and the centers of homologies are the point $K^{\prime }$ and Crelle–Brocard points ${\Omega }_1$ and ${\Omega }_2$ of that triangle. Triangles $ABC$ and $K^{\prime }{\Omega }_1{\Omega }_2$ have the same centroid G [9] (see Figure 2).

Proof. 

According to [4], the lines $A{\Omega }_1$ and $A{\Omega }_2$ have equations

$$\begin{array}{ccc}\hfill A{\Omega }_1& \dots & y=(\omega -c)x-a\omega -ab,\hfill \\ \hfill A{\Omega }_2& \dots & y=-(\omega +b)x+a\omega -ca.\hfill \end{array}$$

The point $C_1$ lies on the first line, and the point $B_1$ lies on the second one because

$$\begin{array}{cc}\hfill (\omega -c)·(-\frac{c}{2})-a\omega -ab& =\frac{1}{2}\left(c^2-2ab-\omega (2a+c)\right)\hfill \\ \hfill & =\frac{1}{2}\left(c^2-2(q+c^2)+\frac{1}{3q}(b-c)(c-a)(a-b)·(a-b)\right)\hfill \\ \hfill & =\frac{1}{6q}\left(-3q(2q+c^2)+(2q-3bc)(2q-3ca)\right)\hfill \\ \hfill & =\frac{1}{6q}\left(-2q^2-3c^{2}q-6q(bc+ca)+9ab{c}^2\right)\hfill \\ \hfill & =\frac{1}{6q}(9cp+3c^{2}q-2q^2),\hfill \\ \hfill -(\omega +b)·(-\frac{b}{2})+a\omega -ca& =\frac{1}{2}\left(b^2-2ca+\omega (2a+b)\right)\hfill \\ \hfill & =\frac{1}{2}\left(b^2-2(q+b^2)+\frac{1}{3q}(b-c)(c-a)(a-b)·(c-a)\right)\hfill \\ \hfill & =\frac{1}{6q}\left(-3q(2q+b^2)+(2q-3ab)(2q-3bc)\right)\hfill \\ \hfill & =\frac{1}{6q}(9bp+3b^{2}q-2q^2).\hfill \end{array}$$

Therefore, points ${\Omega }_1$ and ${\Omega }_2$ lie on lines $A{C}_1$ and $A{B}_1$, respectively, the point ${\Omega }_1$ lies on lines $B{A}_1$ and $C{B}_1$, and the point ${\Omega }_2$ lies on lines $B{C}_1$ and $C{A}_1$. Points ${\Omega }_1$, ${\Omega }_2$ and $K^{\prime }$ have the centroid $G=\left(0,-\frac{2}{3}q\right)$ because

$$\begin{array}{cc}\hfill \frac{1}{3}\left(\frac{p-p_1}{q}+\frac{p-p_2}{q}-\frac{3p}{q}\right)& =-\frac{p+p_1+p_2}{3q}=0\hfill \end{array}$$

and

$$\begin{array}{cc}\hfill \frac{1}{3}\left(\frac{27p_1^2-2q^3}{9q^2}+\frac{27p_2^2-2q^3}{9q^2}+\frac{27p^2-8q^3}{9q^2}\right)& =\frac{1}{9q^2}\left(9{(p_1+p_2)}^2+9p_1^2+9p_2^2-4q^3\right)\hfill \\ \hfill & =\frac{1}{9q^2}(-2q^3-4q^3)=-\frac{2}{3}q.\hfill \end{array}$$

□

The line $AS$ has the slope $a-\frac{3p}{q}$, where S is the Steiner point of the triangle $ABC$ [7]. $AS\Vert B_1{C}_1$ because the line $B_1{C}_1$ has the same slope as $AS$. Similarly, we get $BS\Vert C_1{A}_1$ and $CS\Vert A_1{B}_1$. Because of Theorem 1, we have $A_{1}K\Vert BC$, $B_{1}K\Vert CA$, and $C_{1}K\Vert AB$. Therefore, triangles $ABC$ and $A_1{B}_1{C}_1$ have the property that lines through the vertices of the first triangle parallel to the corresponding sides of the second triangle pass through a common point, and lines through the vertices of the second triangle parallel to the corresponding sides of the first triangle pass through another common point. These two triangles are called parallelogic, and the two mentioned points are the centers of parallelogy of these triangles. So we have the following theorem.

Theorem 8.

A triangle and its first Brocard triangle are parallelogic with the centers of parallelogy at the Steiner point and at the symmedian center of this triangle (see Figure 3).

Theorem 9.

Let $A_1{B}_1{C}_1$ be the first Brocard triangle of an allowable triangle $ABC$. Then the lines parallel to $B_1{C}_1$, $C_1{A}_1$, and $A_1{B}_1$ through C, A, and B, through B, C, and A, pass through a common point $S_1$, respectively $S_2$. In addition, the lines parallel to $BC$, $CA$, and $AB$ through $C_1$, $A_1$, and $B_1$, respectively $B_1$, $C_1$, and $A_1$, pass through a common point $K_1$, respectively $K_2$ (see Figure 3). In the case of the standard triangle $ABC$ these points are given by

$$\begin{array}{ccccccc}\hfill & \hfill & \hfill S_1& =\left(-\frac{3p_1}{q},\frac{9p{p}_1}{q^2}-q\right),\hfill & \hfill S_2& =\left(-\frac{3p_2}{q},\frac{9p{p}_2}{q^2}-q\right),\hfill & \end{array}$$

(8)

$$\begin{array}{cccccc}\hfill & \hfill & \hfill K_1& =\left(\frac{3p_1}{2q},-\frac{5}{6}q\right),\hfill & \hfill K_2& =\left(\frac{3p_2}{2q},-\frac{5}{6}q\right).\hfill \end{array}$$

(9)

Proof. 

According to (5), the slope of the line $B_1{C}_1$ is $a-\frac{3p}{q}$, and the line through C parallel to it is given by $y-c^2=\left(a-\frac{3p}{q}\right)(x-c),$ i.e., $qy=(aq-3p)x+(c^2-ca)q+3cp.$ The point $S_1$ lies on this line because

$$\begin{array}{cc}\hfill qy-(aq& -3p)x-(c^2-ca)q-3cp\hfill \\ \hfill & =\frac{9p{p}_1}{q}-q^2+3a{p}_1-\frac{9p{p}_1}{q}+(ca-c^2)q-3cp\hfill \\ \hfill & =(ca-c^2-q)q+a(b{c}^2+c{a}^2+a{b}^2)-3ab{c}^2\hfill \\ \hfill & =(ca-ab)q-2ab{c}^2+c{a}^3+a^2{b}^2\hfill \\ \hfill & =-a(a+2c)(a^2+ac+c^2)+2a{c}^2(a+c)+a^{3}c+a^2{(a+c)}^2=0.\hfill \end{array}$$

Substitutions $B\leftrightarrow C$ and $b\leftrightarrow c$ imply the substitution $p_1\to p_2$, and using this substitution, the previous proof shows that the line through B, parallel to $B_1{C}_1$, passes through $S_2$. Cyclic permutations $a\to b\to c\to a$ imply $C_1{A}_1\Vert A{S}_1\Vert C{S}_2$, and $A_1{B}_1\Vert B{S}_1\Vert A{S}_2$. The line

$$y=-ax+\frac{1}{6q}(9cp+3c^{2}q-3caq-2q^2)$$

is parallel to $BC$, and it passes through $C_1$. Let us show that this line also passes through $K_1$, i.e., that

$$-\frac{5}{6}q=-a·\frac{3p_1}{2q}+\frac{1}{6q}(9cp+3c^{2}q-3caq-2q^2),$$

i.e.,

$$3cp-3a{p}_1+c^{2}q-caq+q^2=0,$$

which, because $q+c^2=ab$ and $p=abc$, after dividing by a, becomes equivalent to

$$3b{c}^2-3p_1+(b-c)q=0.$$

However, we have

$$\begin{array}{cc}\hfill 3b{c}^2-3p_1+(b-c)q& =3b{c}^2-b{c}^2-c{a}^2-a{b}^2+(b-c)(bc+ca+ab)\hfill \\ \hfill & =b{c}^2+b^{2}c-a^{2}c-a{c}^2\hfill \\ \hfill & =bc(b+c)-ac(a+c)=-bca+acb=0.\hfill \end{array}$$

Other statements of the theorem are proved in a similar way. □

Using the obvious term three-parallelogy, statements of Theorems 8 and 9 can be briefly summarized as the following corollary.

Corollary 1.

The allowable triangle $ABC$ and its first Brocard triangle $A_1{B}_1{C}_1$ are three-parallelogic, and in the case of a standard triangle $ABC$, centers of parallelogy are the Steiner point S of the triangle $ABC$ and points $S_1$, $S_2$, and the symmedian center K of the triangle $ABC$ and points $K_1,K_2$ (see Figure 3).

In the Euclidean case, the statement about three-parallelogy, without proof, can be found in [10].

Theorem 10.

Points S, $S_1$, and $S_2$ from Theorem 9 lie on the circumscribed Steiner ellipse of the triangle $ABC$ (see Figure 3).

Proof. 

The statement for Steiner point S is proved in [7]. For the point $S_1=(x,y)$ we have

$$\begin{array}{cc}\hfill q^2{x}^2-& 9pxy-3q{y}^2-6pqx-4q^{2}y+9p^2\hfill \\ \hfill & =9p_1^2+243\frac{p^2{p}_1^2}{q^3}-27p{p}_1-3q{\left(\frac{9p{p}_1}{q^2}-q\right)}^2+18p{p}_1-36p{p}_1+4q^3+9p^2\hfill \\ \hfill & =9p^2+9p{p}_1+9p_1^2+q^3=0,\hfill \end{array}$$

and this point lies on the circumscribed Steiner ellipse of the triangle $ABC$, which, by [7], has the equation $q^2{x}^2-9pxy-3q{y}^2-6pqx-4q^{2}y+9p^2=0$. Analogous proof is for the point $S_2$. □

Theorem 11.

Points $K_1$ and $K_2$ from Theorems 9 are reciprocal to Crelle–Brocard points ${\Omega }_1$ and ${\Omega }_2$ of that triangle (see Figure 3).

In the Euclidean case this statement, without proof, can be found in [11].

Proof. 

For the point $K_1=(x,y)$ we get

$$\begin{array}{cc}\hfill q^2{x}^2-9pxy& - 3q{y}^2-6pqx-4q^{2}y+9p^2\hfill \\ \hfill & =\frac{9}{4}{p}_1^2+\frac{45}{4}p{p}_1-\frac{25}{12}{q}^3-9p{p}_1+\frac{10}{3}{q}^3+9p^2\hfill \\ \hfill & =9p^2+\frac{9}{4}p{p}_1+\frac{9}{4}{p}_1^2+\frac{15}{12}{q}^3\hfill \\ \hfill & =\frac{27}{4}{p}^2-\frac{1}{4}{q}^3+\frac{5}{4}{q}^3\hfill \\ \hfill & =\frac{1}{4}(27p^2+4q^3),\hfill \\ \hfill 3pq{x}^2+4q^{2}xy& - 9p{y}^2+(9p^2+4q^3)x-12pqy-4p{q}^2\hfill \\ \hfill & =\frac{27}{4}·\frac{p{p}_1^2}{q}-5p_1{q}^2-\frac{25}{4}p{q}^2+\frac{27}{2}·\frac{p^2{p}_1}{q}+6p_1{q}^2+10p{q}^2-4p{q}^2\hfill \\ \hfill & =\frac{27}{2}·\frac{p^2{p}_1}{q}+\frac{27}{4}·\frac{p{p}_1^2}{q}-\frac{1}{4}p{q}^2+p_1{q}^2\hfill \\ \hfill & =\frac{1}{4q}(54p^2{p}_1+27p{p}_1^2+3p{q}^3)-(p-p_1)q^2\hfill \\ \hfill & =\frac{3p}{4q}(18p{p}_1+9p_1^2-9p^2-9p{p}_1-9p_1^2)-(p-p_1)q^2\hfill \\ \hfill & =-\frac{3p}{4q}(9p^2-9p{p}_1)-(p-p_1)q^2\hfill \\ \hfill & =-\frac{1}{4q}(p-p_1)(27p^2+4q^3),\hfill \\ \hfill 9p^2{x}^2+12pqxy& + 4q^2{y}^2+8p{q}^{2}x-(9p^2-4q^3)y-12p^{2}q\hfill \\ \hfill & =\frac{81}{4}·\frac{p^2{p}_1^2}{q^2}-15p{p}_{1}q+\frac{25}{9}{q}^4+12p{p}_{1}q+\frac{15}{2}{p}^{2}q-\frac{10}{3}{q}^4-12p^{2}q\hfill \\ \hfill & =\frac{81}{4}·\frac{p^2{p}_1^2}{q^2}-\frac{9}{2}{p}^{2}q-3p{p}_{1}q-\frac{5}{9}{q}^4\hfill \\ \hfill & =\frac{1}{36q^2}(729p^2{p}_1^2-162p^2{q}^3-108p{p}_1{q}^3-20q^6)\hfill \\ \hfill & =\frac{1}{36q^2}\left(729p^2{p}_1^2-162p^2{q}^3-108p{p}_1{q}^3-8q^6+12q^3(9p^2+9p{p}_1+9p_1^2)\right)\hfill \\ \hfill & =\frac{1}{36q^2}(729p^2{p}_1^2-54p^2{q}^3+108p_1^2{q}^3-8q^6)\hfill \\ \hfill & =\frac{1}{36q^2}(27p^2+4q^3)(27p_1^2-2q^3),\hfill \end{array}$$

and, its reciprocal point has coordinates

$$\begin{array}{cc}\hfill \frac{\frac{1}{4q}(p-p_1)(27p^2+4q^3)}{\frac{1}{4}(27p^2+4q^3)}& =\frac{p-p_1}{q}\hfill \end{array}$$

and

$$\begin{array}{cc}\hfill \frac{\frac{1}{36q^2}(27p^2+4q^3)(27p_1^2-2q^3)}{\frac{1}{4}(27p^2+4q^3)}& =\frac{1}{9q^2}(27p_1^2-2q^3)\hfill \end{array}$$

which is the point ${\Omega }_1$. The proof for $K_2$ is analogous. □

Let us consider the indirect similarity given by

$$\begin{array}{cccccccc}\hfill & \hfill & \hfill x^{\prime }& =-\frac{1}{2}x,\hfill & \hfill y^{\prime }& =\frac{3p}{2q}x+\frac{1}{2}y-\frac{q}{3}\hfill & \hfill & \end{array}$$

(10)

and vice versa

$$\begin{array}{ccccccc}\hfill & \hfill & \hfill x& =-2x^{\prime },\hfill & \hfill y& =\frac{6p}{q}{x}^{\prime }+2y^{\prime }+\frac{2}{3}q.\hfill & \hfill \end{array}$$

(11)

The similarity (10) obviously maps the point $A=(a,a^2)$ to the point $A_1$, and points B and C to points $B_1$ and $C_1$. This similarity maps the Steiner point S, which is, according to [7], given by $S=(-\frac{3p}{q},\frac{9p^2}{q^2})$, to the point with abscissa $\frac{3p}{2q}$ and ordinate equal to $\frac{3p}{2q}·(-\frac{3p}{q})+\frac{1}{2}·\frac{9p^2}{q^2}-\frac{q}{3}=-\frac{q}{3}$, which is the point K. The point $S_1$ is mapped to the point with abscissa $\frac{3p_1}{2q}$ and ordinate $\frac{3p}{2q}(-\frac{3p_1}{q})+\frac{1}{2}(\frac{9p{p}_1}{q^2}-q)-\frac{q}{3}=-\frac{5}{6}q$, which is the point $K_1$. The centroid $G=(0,-\frac{2}{3}q)$ is under the similarity (10) mapped onto itself.

The circumscribed circle and the circumscribed Steiner ellipse of the triangle $ABC$ have, according to [3,7], equations

$$y=x^2$$

and

$$\begin{array}{c}\hfill q^2{x}^2-9pxy-3q{y}^2-6pqx-4q^{2}y+9p^2=0,\end{array}$$

which, after substitutions (11), become

$$\begin{array}{cc}\hfill \frac{6p}{q}{x}^{\prime }+2y^{\prime }+\frac{2}{3}q& =4{x^{\prime }}^2\hfill \end{array}$$

and

$$\begin{array}{cc}\hfill q^2·4{x^{\prime }}^2+18p{x}^{\prime }\left(\frac{6p}{q}{x}^{\prime }+2y^{\prime }+\frac{2}{3}q\right)-3q{\left(\frac{6p}{q}{x}^{\prime }+2y^{\prime }+\frac{2}{3}q\right)}^2+& \\ \hfill +12pq{x}^{\prime }-4q^2\left(\frac{6p}{q}{x}^{\prime }+2y^{\prime }+\frac{2}{3}q\right)+9p^2& =0.\hfill \end{array}$$

After rearrangements and replacements $x^{\prime }⇝x$, $y^{\prime }⇝y$ this can be written as

$$y=2x^2-\frac{3p}{q}x-\frac{q}{3}$$

and

$$4q^2{x}^2-36pxy-12q{y}^2-24pqx-16qy+9p^2-4q^3=0,$$

which are, according to [5,7], equations of the Brocard circle and the inscribed Steiner ellipse of the triangle $ABC$.

Let us find the equation of the fixed line, i.e., of the axis of similarity (10). The transformation $x\to -2x$, $y\to \frac{6p}{q}x+2y+\frac{2}{3}q$ maps the line

$$y=kx+l$$

(12)

to the line

$$\frac{6p}{q}x+2y+\frac{2}{3}q=-2kx+l,$$

i.e., to the line

$$y=-\left(k+\frac{3p}{q}\right)x+\frac{l}{2}-\frac{q}{3},$$

which coincides with the line (12) if and only if

$$-\left(k+\frac{3p}{q}\right)=k,\frac{l}{2}-\frac{q}{3}=l.$$

Therefore,

$$k=-\frac{3p}{2q},l=-\frac{2}{3}q,$$

and the required axis is the line

$$y=-\frac{3p}{2q}x-\frac{2}{3}q,$$

which is, according to [7], the Steiner axis of the triangle $ABC$. The last result is in accordance with Theorem 4.

Thus, we have proved:

Theorem 12.

An allowable triangle $ABC$ and its first Brocard triangle $A_1{B}_1{C}_1$ are indirectly similar. This similarity has the center at the common centroid of these two triangles, its coefficient equals $-\frac{1}{2}$, its axis is the Steiner axis of the triangle $ABC$, and it maps the points S, $S_1$, and $S_2$ from Theorem 9 to points K, $K_1$, and $K_2$ from the same theorem.

Corollary 2.

The symmedian center of the allowable triangle $ABC$ is the Steiner point of its Brocard triangle $A_1{B}_1{C}_1$.

For the Euclidean case see [9].

Corollary 3.

The circumscribed Steiner ellipse of the first Brocard triangle of a given allowable triangle is the inscribed Steiner ellipse of that triangle.

Corollary 4.

Segments $GS$ and $GK$, where G is the centroid of the allowable triangle $ABC$, S its Steiner point, and K its symmedian center, have the same perpendicular bisector as pairs of lines from Theorem 4.

For the Euclidean case see [12].

Theorem 13.

An allowable triangle is homologous with the complementary triangle of its first Brocard triangle.

Proof. 

Points $B_1$ and $C_1$ have the midpoint $A_{1m}=\left(\frac{a}{4},-\frac{1}{12q}(9ap+3bcq+7q^2)\right)$ because

$$9bp+3b^{2}q-2q^2+9cp+3c^{2}q-2q^2=-9ap+3(-q-bc)q-4q^2=-(9ap+3bcq+7q^2).$$

The line

$$9aqy=(9b^2{c}^2+6bcq-5q^2)x+3pq-9bcp-4a{q}^2$$

passes through $A=(a,a^2)$ and $A_1$ because

$$\begin{array}{cc}\hfill (9b^2{c}^2+6bcq-5q^2)a+3pq-9bcp-4a{q}^2& =9abcq-9a{q}^2=9a^{3}q\hfill \end{array}$$

and

$$\begin{array}{cc}\hfill (9b^2{c}^2+6bcq-5q^2)\frac{a}{4}+3pq-9bcp-4a{q}^2& =-\frac{27}{4}a{b}^2{c}^2+\frac{9}{2}abcq-\frac{21}{4}a{q}^2\hfill \\ \hfill & =-\frac{3a}{4}(9bc(q+a^2)-6bcq+7q^2)\hfill \\ \hfill & =-\frac{1}{12q}(9ap+3bcq+7q^2)·9aq,\hfill \end{array}$$

which is the line $A{A}_{1m}$. This line also passes through the point $\left(\frac{3p}{5q},\frac{1}{45q^2}(27p^2-20q^3)\right)$ because

$$\begin{array}{cc}\hfill (9b^2{c}^2+6bcq-5q^2)·\frac{3p}{5q}+3pq-9bcp-4a{q}^2& =\frac{27}{5}·\frac{b^2{c}^{2}p}{q}+\frac{18}{5}bcp-9bcp-4a{q}^2\hfill \\ \hfill & =\frac{1}{5q}(27b^2{c}^{2}p-27bcpq-20a{q}^3)\hfill \\ \hfill & =\frac{1}{5q}(27a^{2}bcp-20a{q}^3)\hfill \\ \hfill & =\frac{1}{45q^2}(27p^2-20q^3)·9aq.\hfill \end{array}$$

□

Theorem 14.

If $A_1{B}_1{C}_1$ is the first Brocard triangle of the allowable triangle $ABC$, then its Crelle–Brocard points ${\Omega }_1$ and ${\Omega }_2$ divide in equal proportions the pairs of segments $C{B}_1$, $B{C}_1$; $A{C}_1$, $C{A}_1$; $B{A}_1$, $A{B}_1$.

For the Euclidean case, without proof, see [10].

Proof. 

According to Theorem 7 and its proof, points ${\Omega }_1$ and ${\Omega }_2$ lie on lines $C{B}_1$, $A{C}_1$, $B{A}_1$, and $B{C}_1$, $C{A}_1$, $A{B}_1$. From (1) and (3) we get the ratios

$$\frac{d(B_1,{\Omega }_1)}{d(C,{\Omega }_1)}=\frac{\frac{p-p_1}{q}+\frac{b}{2}}{\frac{p-p_1}{q}-c}=\frac{2p-2p_1+bq}{2(p-p_1-cq)},\frac{d(C_1,{\Omega }_2)}{d(B,{\Omega }_2)}=\frac{\frac{p-p_2}{q}+\frac{c}{2}}{\frac{p-p_2}{q}-b}=\frac{2p-2p_2+cq}{2(p-p_2-bq)},$$

so we have to prove the equality

$$\begin{array}{cc}\hfill \left(2(p-p_1)+bq\right)(p-p_2-bq)& =\left(2(p-p_2)+cq\right)(p-p_1-cq),\hfill \end{array}$$

which is, after dividing by q, equivalent to

$$\begin{array}{cc}\hfill b(p-p_2-2p+2p_1-bq)& =c(p-p_1-2p+2p_2-cq),\hfill \end{array}$$

and since $p+p_1+p_2=0$, equivalent to

$$3b{p}_1-b^{2}q=3c{p}_2-c^{2}q.$$

This last equality holds true because

$$\begin{array}{cc}\hfill 3b{p}_1-3c{p}_2-(b^2-c^2)q& =b(b{c}^2+c{a}^2+a{b}^2)-c(b^{2}c+c^{2}a+a^{2}b)-(b+c)(b-c)q\hfill \\ \hfill & =a(b^3-c^3)+a(b-c)q\hfill \\ \hfill & =a(b-c)(b^2+bc+c^2+q)=0.\hfill \end{array}$$

□

Theorem 15.

If $A_1{B}_1{C}_1$ is the first Brocard triangle of the allowable triangle $ABC$ with the Brocard angle ω, then $BC{A}_1$, $CA{B}_1$, and $AB{C}_1$ are isosceles triangles, which have angles at sides $BC$, $CA$, and $AB$ equal to ω.

For the Euclidean case see [9].

Proof. 

According to the proof of Theorem 7, lines $A{\Omega }_1$ and $A{\Omega }_2$, containing points $C_1$ and $B_1$, have slopes $\omega -c$ and $-(\omega +b)$. By cyclic permutations it follows that lines $B{\Omega }_1$ and $C{\Omega }_2$, containing the point $A_1$, have slopes $\omega -a$ and $-(\omega +a)$. So we get

$$\begin{array}{cc}\hfill \angle (BC,B{A}_1)& =\omega -a-(-a)=\omega ,\hfill \\ \hfill \angle (C{A}_1,BC)& =-a+\omega +a=\omega .\hfill \end{array}$$

□

Theorem 16.

If P is the center of homology of the triangle $ABC$ and its first Brocard triangle, and if D, E, and F are points symmetrical to P with respect to the midpoints of sides $BC$, $CA$, and $AB$, then the triangles $ABC$ and $DEF$ are symmetrical with respect to the midpoint S of the Crelle–Brocard points ${\Omega }_1$ and ${\Omega }_2$.

For the Euclidean case see [13].

Proof. 

$P+D=B+C$ implies $D=B+C-P$. By Theorem 7, $P+{\Omega }_1+{\Omega }_2=3G$, where G is the centroid of $ABC$. We get $P+2S=3G$, i.e., $P+2S=A+B+C$ or $2S=A+D$. □

4. Conclusions

In this paper we introduce the first Brocard triangle of a triangle in the isotropic plane, and study its connections with some other objects related to the given triangle. Some of the most important statements that we prove are the following: the allowable triangle ABC and its first Brocard triangle are homologous, where the centers of homologies are the point reciprocal to the symmedian center of the triangle and the Crelle–Brocard points of that triangle; the allowable triangle ABC and its first Brocard triangle are three-parallelogic and indirectly similar, and the allowable triangle is homologous with the complementary triangle of its first Brocard triangle. The analytical approach used in this paper was introduced and developed in [3]. The obtained results are compared with similar results in the Euclidean plane.