Industrial and Management Applications of Type-2 Multi-Attribute Decision-Making Techniques Extended with Type-2 Fuzzy Sets from 2013 to 2022
Abstract
:1. Introduction
- (1)
- Which IT2FMADM techniques are being used frequently in (i) industrial engineering and (ii) computer science?
- (2)
- Which characteristics of IT2FNs are mostly employed?
- (3)
- Which method is mostly used for the aggregation of DMs’ assessment into unique opinion?
- (4)
- Which type of study is executed on these IT2FMADM techniques (distance between two IT2FNs, method of defuzzification, a method for the comparison of IT2FNs, etc.)? This paper provides a systematic survey that provides answers to the identified gap in the literature.
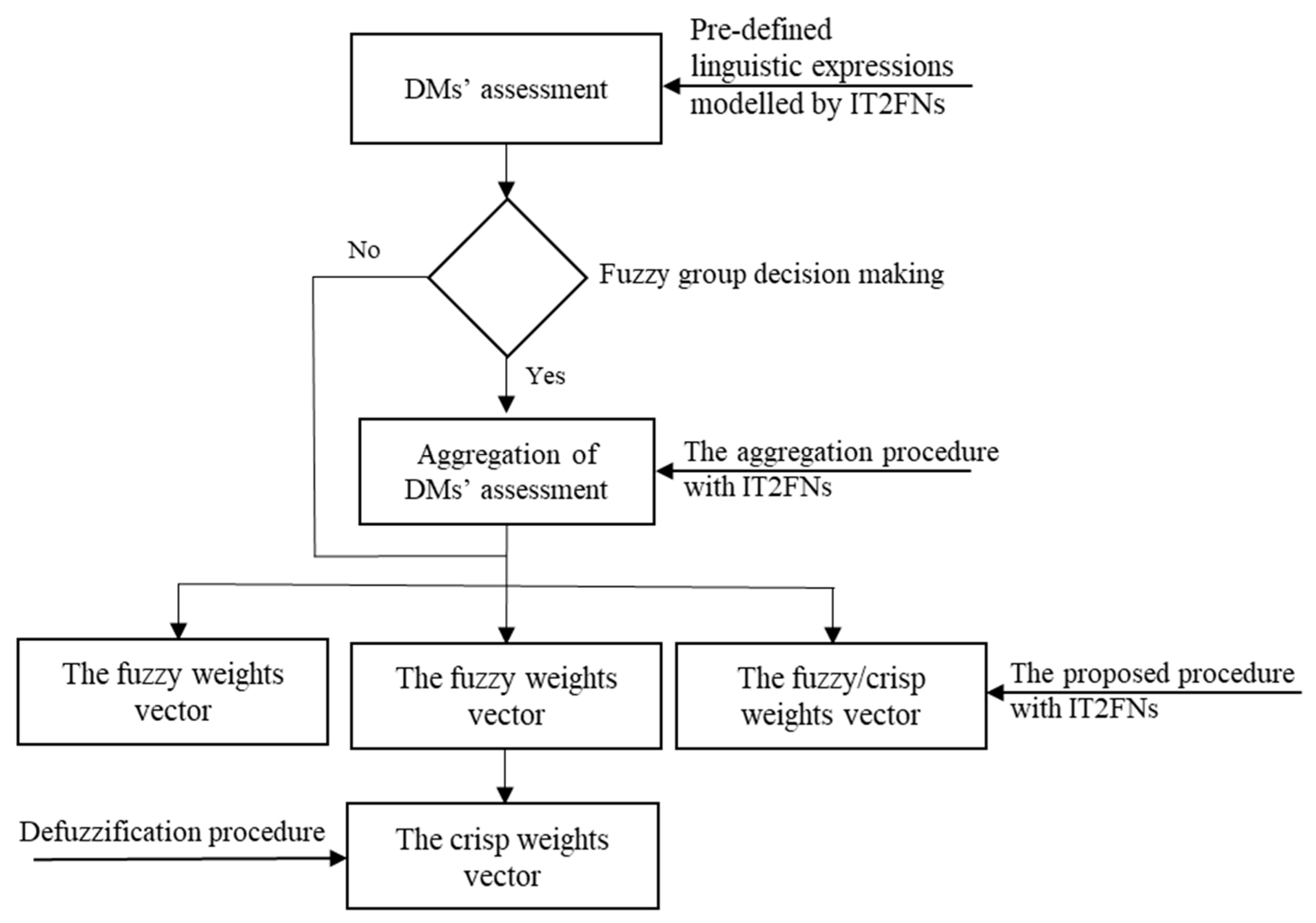
2. Materials and Methods
2.1. Basic Consideration of Type 2 Fuzzy Sets
2.2. Determining the Relative Importance of Attributes and Their Values
2.3. Determining of Attributes’ Weight
2.3.1. The Assessment in a Direct Way
2.3.2. Interval Type 2 Fuzzy Analytical Hierarchy Process-IT2FAHP
2.3.3. Interval Type 2 Fuzzy Best Worst Method-IT2FBWM
2.4. Analysis of Ranking Multi-Atrubutive Decision-Making Methods
- The utility-based IT2FMADM
2.4.1. Interval Type 2 Fuzzy Additive Ratio Assessment-IT2FARAS
2.4.2. Interval Type 2 Fuzzy Multi-Objective Optimization on the Basis of Ratio Analysis-IT2FMOORA (IT2FMULTIMOORA)
2.4.3. Interval Type 2 Fuzzy “An Acronym in Portuguese for Interactive and Multi-Criteria Decision-Making”-IT2FTODIM
2.4.4. Interval Type 2 Fuzzy Analytical Hierarchy Process-IT2FAHP
- The outranking IT2FMADM
2.4.5. Interval Type 2 Fuzzy Elimination et Choix Traduisant la Realité-IT2FELECTRE
- The compromise IT2FMADM
2.4.6. Interval Type 2 Fuzzy Complex Proportional Assessment-IT2FCOPRAS
2.4.7. Interval Type 2 Fuzzy Multi-Attributive Border Approximation Area Comparison Method-IT2FMABAC
2.4.8. Interval Type 2 Fuzzy Technique for Order Preference by Similarity to Ideal Solution-IT2FTOPSIS
2.4.9. Interval Type 2 Fuzzy VIekriterijumsko KOmpromisno Rangiranje-IT2FVIKOR
- The other IT2FMADM
2.4.10. Interval Type 2 Fuzzy Weighted Aggregated Sum Product Assessment-IT2FWASPAS
2.4.11. Interval Type 2 Fuzzy Decision-Making Trial and Evaluation Laboratory-IT2FDEMATEL
3. Results and Discussion of the Research








4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
| Authors | Type of IT2FNs | Granulation/The Domain | The Aggregation Operators | The Determination of Attribute Weights |
|---|---|---|---|---|
| Celik et al. [27] | IT2TrFN | 5/[0–10] | Fuzzy arithmetic mean | Fuzzy weights vector |
| Baležentis and Zeng [28] | IT2TrFN | 9/[0–1] | - | Crisp weights vector |
| Chen and Hong [30] | IT2TrFN | 7/[0–1] | Method for comparison of IT2FNS combined with arithmetic mean | Weight attributed to the largest variable/Crisp weights vector |
| Ghorabaee et al. [29] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean | Fuzzy weights vector |
| Abdullah and Zulkifli [33] | TrFN | 9/[1–9] | - | FAHP and fuzzy geometric mean/defuzzification are performed by using the centroid defuzzification method [89]/crisp weights vector |
| Ghorabaee [19] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean | Fuzzy weights vector |
| Kilic and Kaya [32] | IT2TrFN | 5/[1–9] | IT2FAHP and fuzzy geometric mean/defuzzification are performed by using the center of area method [77]/crisp weights vector | |
| Cebi and Otay [34] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean | Fuzzy weights vector |
| Qin et al. [31] | IT2TFN | 5/[0–10] | - | Fuzzy weights vector |
| Qin et al. [43] | IT2TrFN | 7/[0–10] | Type 2 fuzzy weighted aggregation method | KM algorithm [90] |
| Özkan et al. [37] | IT2TrFN | 5/[1–9] | IT2FAHP and fuzzy geometric mean/aggregation performed by using fuzzy arithmetic mean/fuzzy weights vector | |
| Liao [38] | IT2TrFN | 5/[0–1] | - | Fuzzy weights vector |
| Sang and Liu [39] | crisp | Crisp weights vector | ||
| Ghorabaee et al. [36] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean | Fuzzy weights vector |
| Qin et al. [35] | IT2TrFN | 7/[0–10] | - | Fuzzy weights vector |
| Ghorabaee et al. [41] | IT2TrFN | 7/[0–1] | Entropy method/fuzzy weights vector | |
| Celik et al. [40] | IT2TrFN | 7/[0–10] | Fuzzy arithmetic mean | - |
| Buyoukozkan [42] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean | Fuzzy weights vector |
| Gorener et al. [44] | IT2TrFN | 5/[1–9] | - | IT2FAHP and fuzzy geometric mean/fuzzy weights vector |
| Deveci et al. [45] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean | Fuzzy weights vector |
| Mousakhani [46] | IT2TrFN | 7/[0–1] | Fuzzy geometric mean | Fuzzy weights vector |
| Soner et al. [47] | IT2TrFN | 9/[1–10] | IT2FAHP and fuzzy geometric mean/fuzzy weights vector | |
| Zhong and Yao [48] | IT2TrFN | 7/[0–1] | - | The information entropy/crisp weights vector |
| Deveci et al. [49] | IT2TrFN | 5/[0–10] | Fuzzy arithmetic mean | Fuzzy weights vector |
| Celik and Akyuz [20] | IT2TrFN | 9/[1–10] | - | IT2FAHP and fuzzy geometric mean/defuzzification procedure [76]/crisp weights vector |
| Debnath and Biswas [50] | IT2TrFN | 5/[1–9] | - | IT2FAHP and fuzzy geometric mean/the proposed defuzzification procedure/fuzzy weights vector |
| Meng et al. [51] | IT2TrFN | 7/[0–1] | The linear normalization procedure | |
| Xu et al. [54] | crisp | / | / | AHP/crisp vector weights |
| Dinçer et al. [53] | IT2TrFN | 7/[0–1] | - | IT2DEMATEL combined with IT2FANP and defuzzification procedure [76]/crisp weights vector |
| Wu et al. [55] | IT2TrFN | 9/[1–9] | - | IT2FBWM and fuzzy geometric mean and defuzzification by using the centroid area method [91] |
| Aleksic et al. [56] | IT2TrFN | 3/[1–5] | Fuzzy averaging mean | Ranking of IT2FNs [74]/crisp weights vector |
| Yucesan et al. [57] | BWM/crisp weights vector | |||
| Đurić et al. [52] | IT2TrFN | 3/[1–5] | IT2FAHP and fuzzy geometric mean/fuzzy weights vector | |
| Dorfeshan and Mousavi [58] | IT2TrFNs | 7/[0–1] | IT2FWASPAS/crisp weights vector | |
| Bera et al. [59] | IT2TrFN | 7/[0–1] | - | Fuzzy weights vector |
| Mohamadghasemi et al. [26] | (GIT2FN) | 7/[3–15] | - | Crisp weights vector |
| Ayyildiz et al. [60] | IT2TrFN | 9/[1–10] | - | IT2FAHP and fuzzy geometric mean/defuzzification procedure/linear normalization procedure/crisp weights vector |
| Kiraci and Akan [21] | IT2TrFN | 5/[1–9] | IT2FAHP and fuzzy geometric mean/defuzzification are performed by using the center of area method [77]/arithmetic mean/crisp weights vector | |
| Pourmand et al. [62] | IT2TrFN | 7/[0–1] | - | IT2FTOPSIS combined with the ranking of IT2FNs [74] and linear normalization procedure/crisp weights vector |
| Özdemir and Üsküdar [63] | IT2TrFN | 5/[1–9] | - | IT2FAHP and fuzzy geometric mean/fuzzy weights vector |
| Deveci et al. [64] | IT2TFN | 7/[0–1] | Fuzzy arithmetic mean | Fuzzy weights vector |
| Mirnezami et al. [65] | - | - | ||
| Komatina et al. [67] | IT2TFN | 9/[0–1] | - | IT2FDelphi technique |
| Karagöz et al. [68] | IT2TFN | 7/[0–1] | Fuzzy arithmetic mean | Fuzzy weights vector |
| Kaya and Aycin [92] | IT2TrFN | 5/[1–9] | IT2FAHP and fuzzy geometric mean/fuzzy weights vector | |
| Celik et al. [69] | IT2TrFN | 9/[1–10] | IT2FBWM based on [55]/fuzzy weights vector | |
| Zhang et al. [70] | IT2TrFN | 5/[0–10] | Fuzzy arithmetic mean | Fuzzy weights vector |
| Sharaf [66] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean | Fuzzy weights vector |
| Komatina et al. [71] | IT2TFN | 5/[1–9] | IT2FAHP and fuzzy geometric mean/fuzzy weights vector | |
| Aleksić et al. [72] | IT2TFN | 6/[1–9] | Geometric mean | IT2FBWM [55] |
| Ecer [73] | IT2TFN | 5/[1–9] | - | IT2FAHP and fuzzy geometric mean/fuzzy weights vector |
Appendix B
| Authors | Type of IT2FNs | Granulation/The Domain | The Aggregation Operators |
|---|---|---|---|
| Celik et al. (2013) [27] | IT2TrFN | 5/[0–10] | Fuzzy arithmetic mean |
| Baležentis and Zeng (2013) [28] | IT2TrFN | 9/[0–1] | The weighted geometric average operator |
| Chen and Hong (2014) [30] | IT2TrFN | 7/[0–10] | Fuzzy arithmetic mean |
| Ghorabaee et al. (2014) [29] | IT2TrFN | 7/[0–10] | Fuzzy arithmetic mean |
| Abdullah and Zulkifli (2015) [33] | IT2TrFN | 5/[0–1] | - |
| Cebi and Otay (2015) [34] | IT2TrFN | 7/[0–10] | Fuzzy arithmetic mean |
| Qin et al. (2015) [31] | IT2TrFN | 7/[0–1] | - |
| Ghorabaee et al. (2016) [19] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean |
| Kilic and Kaya (2015) [32] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean |
| Özkan et al. (2015) [37] | IT2TrFN | 5/[1–9] | Fuzzy arithmetic mean |
| Liao (2015) [38] | IT2TrFN | 5/[0–1] | - |
| Sang and Liu (2015) [39] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean |
| Qin et al. (2015) [35] | IT2TrFN | 5/[0–10] | |
| Ghorabaee et al. (2015) [36] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean |
| Ghorabaee et al. (2016) [41] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean |
| Buyoukozkan (2016) [42] | IT2TrFN | 7/[0–1] | fuzzy arithmetic mean |
| Celik et al. (2016) [40] | IT2TrFN | 7/[1–10] | Fuzzy arithmetic mean |
| Qin et al. (2017) [43] | TrFN | 7/[0–1] | - |
| Soner et al. (2017) [47] | IT2TrFN | 7/[0–10] | Fuzzy arithmetic mean |
| Deveci et al. (2017) [45] | IT2TrFN | 7/[0–10] | Fuzzy arithmetic mean |
| Gorener et al. (2017) [44] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean |
| Zhong and Yao (2017) [48] | IT2TrFN | 7/[0–1] | - |
| Mousakhani (2017) [46] | IT2TrFN | 7/[0–10] | Fuzzy geometric mean |
| Debnath and Biswas (2018) [50] | IT2TrFN | 5/[1–9] | - |
| Celik and Akyuz (2018) [20] | IT2TrFN | 7/[0–1] | - |
| Deveci et al. (2018) [49] | IT2TrFN | 9/[0–10] | Fuzzy arithmetic mean |
| Meng et al. (2019) [51] | IT2TrFN | 7/[0–1] | - |
| Dinçer et al. (2019) [53] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean |
| Xu et al. (2019) [54] | IT2TrFN | 5/[0–1] | - |
| Yucesan et al. (2019) [57] | IT2TrFN | -/[0–1] | Fuzzy arithmetic mean |
| Aleksic et al. (2019) [56] | IT2TrFN | 7/[0–1] and 5/[0–1] | - |
| Đurić et al. (2019) [52] | IT2TrFN | 5/[0–1] and 7/[0–1] | - |
| Wu et al. (2019) [55] | IT2TrFN | 7/[0–10] | The interval type 2 fuzzy weighted average operator |
| Dorfeshan and Mousavi (2020) [58] | IT2TrFN | 7/[0–1] | - |
| Bera et al. (2020) [59] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean |
| Mohamadghasemi et al. (2020) [26] | GIT2FN | 7/[3–15] | - |
| Ayyildiz et al. (2020) [60] | IT2TrFN | 9/[1–10] | Fuzzy arithmetic mean |
| Kiraci and Akan (2020) [21] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean |
| Pourmand et al. (2020) [62] | IT2TrFN | 7/[0–1] | - |
| Özdemir and Üsküdar (2020) [63] | IT2TrFN | 7/[0–1] | Fuzzy arithmetic mean |
| Deveci et al. (2020) [64] | IT2TFN | 7/[0–10] | - |
| Mirnezami et al. (2021) [65] | IT2TrFN | 7/[0–1] | - |
| Sharaf (2021) [66] | IT2TrFN | 7/[0–1] | - |
| Zhang et al. (2022) [70] | IT2TrFN | 5/[0–10] | |
| Komatina et al. (2021) [67] | IT2TFN | 7/[1–9] | - |
| Karagöz et al. (2021) [68] | IT2TFN | 7/[1–10] | Fuzzy arithmetic mean |
| Kaya and Aycin (2021) [92] | 7/[0–10] | - | |
| Celik et al. (2021) [69] | IT2TrFN | 9/[1–10] | - |
| Komatina et al. (2022) [71] | IT2TFN | 7/[1–9] | - |
| Aleksić et al. (2022) [72] | IT2TFN | 5/[1–10] | |
| Ecer, F. (2022) [73] | IT2TFN | 5/[1–9] | - |
References
- Mardani, A.; Jusoh, A.; Zavadskas, E.K. Fuzzy Multiple Criteria Decision-Making Techniques and Applications—Two Decades Review from 1994 to 2014. Expert Syst. Appl. 2015, 42, 4126–4148. [Google Scholar] [CrossRef]
- Behzadian, M.; Khanmohammadi Otaghsara, S.; Yazdani, M.; Ignatius, J. A State-of the-Art Survey of TOPSIS Applications. Expert Syst. Appl. 2012, 39, 13051–13069. [Google Scholar] [CrossRef]
- Danesh, D.; Ryan, M.J.; Abbasi, A. Multi-Criteria Decision-Making Methods for Project Portfolio Management: A Literature Review. Int. J. Manag. Decis. Mak. 2018, 17, 75–94. [Google Scholar] [CrossRef]
- Sousa, M.; Almeida, M.F.; Calili, R. Multiple Criteria Decision Making for the Achievement of the UN Sustainable Development Goals: A Systematic Literature Review and a Research Agenda. Sustainability 2021, 13, 4129. [Google Scholar] [CrossRef]
- Zayat, W.; Kilic, H.S.; Yalcin, A.S.; Zaim, S.; Delen, D. Application of MADM Methods in Industry 4.0: A Literature Review. Comput. Ind. Eng. 2023, 177, 109075. [Google Scholar] [CrossRef]
- Zavadskas, E.K.; Turskis, Z.; Antucheviciene, J.; Zakarevicius, A. Optimization of Weighted Aggregated Sum Product Assessment. Elektron. Elektrotechnika 2012, 122, 3–6. [Google Scholar] [CrossRef]
- Hwang, C.-L.; Yoon, K. Methods for Multiple Attribute Decision Making. In Multiple Attribute Decision Making: Methods and Applications A State-of-the-Art Survey; Hwang, C.-L., Yoon, K., Eds.; Lecture Notes in Economics and Mathematical Systems; Springer: Berlin/Heidelberg, Germany, 1981; pp. 58–191. ISBN 978-3-642-48318-9. [Google Scholar]
- Opricovic, S. Multicriteria Optimization of Civil Engineering Systems. PhD. Thesis, Faculty of Civil Engineering, University of Belgrade, Belgrade, Serbia, 1998. [Google Scholar]
- Zavadskas, E.K.; Kaklauskas, A.; Šarka, V. The New Method of Multicriteria Complex Proportional Assessment of Projects. Technol. Econ. Dev. Econ. 1994, 1, 131–139. [Google Scholar]
- Brauers, W.K.M.; Zavadskas, E.K. The MOORA Method and Its Application to Privatization in a Transition Economy. Control Cybern. 2006, 35, 445–469. [Google Scholar]
- Zavadskas, E.K.; Turskis, Z. A New Additive Ratio Assessment (ARAS) Method in Multicriteria Decision-making. Ukio Technol. Ekon. Vystym. 2010, 16, 159–172. [Google Scholar] [CrossRef]
- Roy, B. Classement et choix en présence de points de vue multiples. Rev. Franįaise D’informatique Rech. Opérationnelle 1968, 2, 57–75. [Google Scholar] [CrossRef]
- Saaty, T.L. Decisions with the Analytic Network Process (ANP). In Proceedings of the International Symposium on the Analytic Hierarchy Process, Vancouver, BC, Canada, 12–15 July 1996. [Google Scholar]
- Saaty, T.L. The Modern Science of Multicriteria Decision Making and Its Practical Applications: The AHP/ANP Approach. Oper. Res. 2013, 61, 1101–1118. [Google Scholar] [CrossRef]
- Gomes, L.; Lima, M. TODIM: Basics and Application to Multicriteria Ranking of Projects with Environmental Impacts. Found. Comput. Decis. Sci. 1992, 16, 113–127. [Google Scholar]
- Rezaei, J. Best-Worst Multi-Criteria Decision-Making Method. Omega 2015, 53, 49–57. [Google Scholar] [CrossRef]
- Pamučar, D.; Ćirović, G. The Selection of Transport and Handling Resources in Logistics Centers Using Multi-Attributive Border Approximation Area Comparison (MABAC). Expert Syst. Appl. 2015, 42, 3016–3028. [Google Scholar] [CrossRef]
- Fontela, E.; Gabus, A. DEMATEL, Innovative Methods; Battelle Geneva Research Institute: Geneva, Switzerland, 1974. [Google Scholar]
- Ghorabaee, M.K. Developing an MCDM Method for Robot Selection with Interval Type-2 Fuzzy Sets. Robot. Comput. Integr. Manuf. 2016, 37, 221. [Google Scholar] [CrossRef]
- Celik, E.; Akyuz, E. An Interval Type-2 Fuzzy AHP and TOPSIS Methods for Decision-Making Problems in Maritime Transportation Engineering: The Case of Ship Loader. Ocean. Eng. 2018, 155, 371–381. [Google Scholar] [CrossRef]
- Kiracı, K.; Akan, E. Aircraft Selection by Applying AHP and TOPSIS in Interval Type-2 Fuzzy Sets. J. Air Transp. Manag. 2020, 89, 101924. [Google Scholar] [CrossRef]
- Celik, E.; Gul, M.; Aydin, N.; Gumus, A.T.; Guneri, A.F. A Comprehensive Review of Multi Criteria Decision Making Approaches Based on Interval Type-2 Fuzzy Sets. Knowl. Based Syst. 2015, 85, 329–341. [Google Scholar] [CrossRef]
- Zadeh, L.A. The Concept of a Linguistic Variable and Its Application to Approximate Reasoning-III. Inf. Sci. 1975, 9, 43–80. [Google Scholar] [CrossRef]
- Zimmermann, H.-J. Fuzzy Set Theory—And Its Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2011; ISBN 978-94-010-0646-0. [Google Scholar]
- Derelï, T.; Baykasoglu, A.; Altun, K.; Durmuşoğlu, A.; Türkşen, İ. Industrial Applications of Type-2 Fuzzy Sets and Systems: A Concise Review. Comput. Ind. 2011, 62, 125–137. [Google Scholar] [CrossRef]
- Mohamadghasemi, A.; Hadi-Vencheh, A.; Lotfi, F.H.; Khalilzadeh, M. An Integrated Group FWA-ELECTRE III Approach Based on Interval Type-2 Fuzzy Sets for Solving the MCDM Problems Using Limit Distance Mean. Complex Intell. Syst. 2020, 6, 355–389. [Google Scholar] [CrossRef]
- Celik, E.; Bilisik, O.N.; Erdogan, M.; Gumus, A.T.; Baracli, H. An Integrated Novel Interval Type-2 Fuzzy MCDM Method to Improve Customer Satisfaction in Public Transportation for Istanbul. Transp. Res. Part E 2013, 58, 28–51. [Google Scholar] [CrossRef]
- Baležentis, T.; Zeng, S. Group Multi-Criteria Decision Making Based upon Interval-Valued Fuzzy Numbers: An Extension of the MULTIMOORA Method. Expert Syst. Appl. 2013, 40, 543–550. [Google Scholar] [CrossRef]
- Ghorabaee, M.K.; Amiri, M.; Sadaghiani, J.S.; Goodarzi, G.H. Multiple Criteria Group Decision-Making for Supplier Selection Based on COPRAS Method with Interval Type-2 Fuzzy Sets. Int. J. Adv. Manuf. Technol. 2014, 75, 1115. [Google Scholar] [CrossRef]
- Chen, S.-M.; Hong, J.-A. Fuzzy Multiple Attributes Group Decision-Making Based on Ranking Interval Type-2 Fuzzy Sets and the TOPSIS Method. IEEE Trans. Syst. Man Cybern. Syst. 2014, 44, 1665–1673. [Google Scholar] [CrossRef]
- Qin, Y.; Zhang, Z.; Liu, X.; Li, M.; Kou, L. Dynamic Risk Assessment of Metro Station with Interval Type-2 Fuzzy Set and TOPSIS Method. J. Intell. Fuzzy Syst. 2015, 29, 93–106. [Google Scholar] [CrossRef]
- Kiliç, M.; Kaya, İ. Investment Project Evaluation by a Decision Making Methodology Based on Type-2 Fuzzy Sets. Appl. Soft Comput. 2015, 27, 399–410. [Google Scholar] [CrossRef]
- Abdullah, L.; Zulkifli, N. Integration of Fuzzy AHP and Interval Type-2 Fuzzy DEMATEL: An Application to Human Resource Management. Expert Syst. Appl. 2015, 42, 4397–4409. [Google Scholar] [CrossRef]
- Cebi, F.; Otay, İ. Multi-Criteria and Multi-Stage Facility Location Selection under Interval Type-2 Fuzzy Environment: A Case Study for a Cement Factory. Int. J. Comput. Intell. Syst. 2015, 8, 330–344. [Google Scholar] [CrossRef]
- Qin, J.; Liu, X.; Pedrycz, W. An Extended VIKOR Method Based on Prospect Theory for Multiple Attribute Decision Making under Interval Type-2 Fuzzy Environment. Knowl.-Based Syst. 2015, 86, 116–130. [Google Scholar] [CrossRef]
- Ghorabaee, M.K.; Amiri, M.; Sadaghiani, J.S.; Zavadskas, E.K. Multi-Criteria Project Selection Using an Extended VIKOR Method with Interval Type-2 Fuzzy Sets. Int. J. Inf. Tech. Dec. Mak. 2015, 14, 993–1016. [Google Scholar] [CrossRef]
- Özkan, B.; Kaya, İ.; Cebeci, U.; Başlıgil, H. A Hybrid Multicriteria Decision Making Methodology Based on Type-2 Fuzzy Sets for Selection among Energy Storage Alternatives. Int. J. Comput. Intell. Syst. 2015, 8, 914–927. [Google Scholar] [CrossRef]
- Liao, T.W. Two Interval Type 2 Fuzzy TOPSIS Material Selection Methods. Mater. Des. 2015, 88, 1088–1099. [Google Scholar] [CrossRef]
- Sang, X.; Liu, X. An Interval Type-2 Fuzzy Sets-Based TODIM Method and Its Application to Green Supplier Selection. J. Oper. Res. Soc. 2016, 67, 722–734. [Google Scholar] [CrossRef]
- Celik, E.; Gumus, A.; Erdogan, M. A New Extension of the ELECTRE Method Based upon Interval Type-2 Fuzzy Sets for Green Logistic Service Providers Evaluation. J. Test. Eval. 2016, 44, 20140046. [Google Scholar] [CrossRef]
- Ghorabaee, K.M.; Zavadskas, E.K.; Amiri, M.; Esmaeili, A. Multi-Criteria Evaluation of Green Suppliers Using an Extended WASPAS Method with Interval Type-2 Fuzzy Sets. J. Clean. Prod. 2016, 137, 213–229. [Google Scholar] [CrossRef]
- Büyüközkan, G.; Parlak, I.B.; Tolga, A.C. Evaluation of Knowledge Management Tools by Using An Interval Type-2 Fuzzy TOPSIS Method. Int. J. Comput. Intell. Syst. 2016, 9, 812–826. [Google Scholar] [CrossRef]
- Qin, J.; Liu, X.; Pedrycz, W. An Extended TODIM Multi-Criteria Group Decision Making Method for Green Supplier Selection in Interval Type-2 Fuzzy Environment. Eur. J. Oper. Res. 2017, 258, 626–638. [Google Scholar] [CrossRef]
- Görener, A.; Ayvaz, B.; Kuşakcı, A.O.; Altınok, E. A Hybrid Type-2 Fuzzy Based Supplier Performance Evaluation Methodology: The Turkish Airlines Technic Case. Appl. Soft Comput. 2017, 56, 436–445. [Google Scholar] [CrossRef]
- Deveci, M.; Demirel, N.Ç.; Ahmetoğlu, E. Airline New Route Selection Based on Interval Type-2 Fuzzy MCDM: A Case Study of New Route between Turkey- North American Region Destinations. J. Air Transp. Manag. 2017, 59, 83–99. [Google Scholar] [CrossRef]
- Mousakhani, S.; Nazari-Shirkouhi, S.; Bozorgi-Amiri, A. A Novel Interval Type-2 Fuzzy Evaluation Model Based Group Decision Analysis for Green Supplier Selection Problems: A Case Study of Battery Industry. J. Clean. Prod. 2017, 168, 205–218. [Google Scholar] [CrossRef]
- Soner, O.; Celik, E.; Akyuz, E. Application of AHP and VIKOR Methods under Interval Type 2 Fuzzy Environment in Maritime Transportation. Ocean. Eng. 2017, 129, 107–116. [Google Scholar] [CrossRef]
- Zhong, L.; Yao, L. An ELECTRE I-Based Multi-Criteria Group Decision Making Method with Interval Type-2 Fuzzy Numbers and Its Application to Supplier Selection. Appl. Soft Comput. 2017, 57, 556–576. [Google Scholar] [CrossRef]
- Deveci, M.; Canıtez, F.; Gökaşar, I. WASPAS and TOPSIS Based Interval Type-2 Fuzzy MCDM Method for a Selection of a Car Sharing Station. Sustain. Cities Soc. 2018, 41, 777–791. [Google Scholar] [CrossRef]
- Debnath, J.; Biswas, A. Analytic Hierarchy Process Based on Interval Type-2 Intuitionistic Fuzzy Sets with Their Application to Multicriteria Decision Making. Intell. Decis. Technol. 2018, 12, 359–370. [Google Scholar] [CrossRef]
- Meng, Q.; Liu, X.; Song, Y.; Wang, W. An Extended Generalized TODIM Method for Risk Assessment of Supply Chain in Social Commerce under Interval Type-2 Fuzzy Environment. J. Intell. Fuzzy Syst. 2019, 37, 8551–8565. [Google Scholar] [CrossRef]
- Ðurić, G.; Mitrović, Č.; Komatina, N.; Tadić, D.; Vorotović, G. The Hybrid MCDM Model with the Interval Type-2 Fuzzy Sets for the Software Failure Analysis. J. Intell. Fuzzy Syst. 2019, 37, 7747–7759. [Google Scholar] [CrossRef]
- Dinçer, H.; Yüksel, S.; Martínez, L. Interval Type 2-Based Hybrid Fuzzy Evaluation of Financial Services in E7 Economies with DEMATEL-ANP and MOORA Methods. Appl. Soft Comput. 2019, 79, 186–202. [Google Scholar] [CrossRef]
- Xu, Z.; Qin, J.; Liu, J.; Martínez, L. Sustainable Supplier Selection Based on AHPSort II in Interval Type-2 Fuzzy Environment. Inf. Sci. 2019, 483, 273–293. [Google Scholar] [CrossRef]
- Wu, Q.; Zhou, L.; Chen, Y.; Chen, H. An Integrated Approach to Green Supplier Selection Based on the Interval Type-2 Fuzzy Best-Worst and Extended VIKOR Methods. Inf. Sci. 2019, 502, 394–417. [Google Scholar] [CrossRef]
- Aleksic, A.; Runic Ristic, M.; Komatina, N.; Tadic, D. Advanced Risk Assessment in Reverse Supply Chain Processes: A Case Study in Republic of Serbia. Adv. Prod. Eng. Manag. 2019, 14, 421–434. [Google Scholar] [CrossRef]
- Yucesan, M.; Mete, S.; Serin, F.; Celik, E.; Gul, M. An Integrated Best-Worst and Interval Type-2 Fuzzy TOPSIS Methodology for Green Supplier Selection. Mathematics 2019, 7, 182. [Google Scholar] [CrossRef]
- Dorfeshan, Y.; Mousavi, S.M. A Novel Interval Type-2 Fuzzy Decision Model Based on Two New Versions of Relative Preference Relation-Based MABAC and WASPAS Methods (with an Application in Aircraft Maintenance Planning). Neural Comput. Appl. 2020, 32, 3367–3385. [Google Scholar] [CrossRef]
- Bera, A.K.; Jana, D.K.; Banerjee, D.; Nandy, T. Supplier Selection Using Extended IT2 Fuzzy TOPSIS and IT2 Fuzzy MOORA Considering Subjective and Objective Factors. Soft Comput. 2020, 24, 8899–8915. [Google Scholar] [CrossRef]
- Ayyildiz, E.; Taskin Gumus, A.; Erkan, M. Individual Credit Ranking by an Integrated Interval Type-2 Trapezoidal Fuzzy Electre Methodology. Soft Comput. 2020, 24, 16149–16163. [Google Scholar] [CrossRef]
- Yang, Y.-Y.; Liu, X.-W.; Liu, F. Trapezoidal Interval Type-2 Fuzzy TOPSIS Using Alpha-Cuts. Int. J. Fuzzy Syst. 2020, 22, 293–309. [Google Scholar] [CrossRef]
- Pourmand, E.; Mahjouri, N.; Hosseini, M.; Nik-Hemmat, F. A Multi-Criteria Group Decision Making Methodology Using Interval Type-2 Fuzzy Sets: Application to Water Resources Management. Water Res. Manag. 2020, 34, 4067–4092. [Google Scholar] [CrossRef]
- Özdemir, Y.S.; Üsküdar, A. Strategy Selection by Using Interval Type-2 Fuzzy Mcdm and an Application. J. Eng. Res. 2020, 8, 172–189. [Google Scholar] [CrossRef]
- Deveci, M.; Cali, U.; Kucuksari, S.; Erdogan, N. Interval Type-2 Fuzzy Sets Based Multi-Criteria Decision-Making Model for Offshore Wind Farm Development in Ireland. Energy 2020, 198, 117317. [Google Scholar] [CrossRef]
- Mirnezami, S.A.; Mousavi, S.M.; Mohagheghi, V. An Innovative Interval Type-2 Fuzzy Approach for Multi-Scenario Multi-Project Cash Flow Evaluation Considering TODIM and Critical Chain with an Application to Energy Sector. Neural Comput. Appl. 2021, 33, 2263–2284. [Google Scholar] [CrossRef]
- Sharaf, I.M. An Interval Type-2 Fuzzy TOPSIS for MAGDM Applied to Solar Power Systems. Pak. J. Stat. Oper. Res. 2021, 17, 559–575. [Google Scholar] [CrossRef]
- Komatina, N.; Djapan, M.; Ristić, I.; Aleksić, A. Fulfilling External Stakeholders’ Demands—Enhancement Workplace Safety Using Fuzzy MCDM. Sustainability 2021, 13, 2892. [Google Scholar] [CrossRef]
- Karagöz, S.; Deveci, M.; Simic, V.; Aydin, N. Interval Type-2 Fuzzy ARAS Method for Recycling Facility Location Problems. Appl. Soft Comput. 2021, 102, 107107. [Google Scholar] [CrossRef]
- Celik, E.; Yucesan, M.; Gul, M. Green Supplier Selection for Textile Industry: A Case Study Using BWM-TODIM Integration under Interval Type-2 Fuzzy Sets. Environ. Sci. Pollut. Res. 2021, 28, 64793–64817. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhao, X.; Qin, Y.; Si, H.; Zhou, L. Interval Type-2 Fuzzy TOPSIS Approach with Utility Theory for Subway Station Operational Risk Evaluation. J. Ambient. Intell. Hum. Comput. 2022, 13, 4849–4863. [Google Scholar] [CrossRef]
- Komatina, N.; Tadić, D.; Aleksić, A.; Jovanović, A.D. The Assessment and Selection of Suppliers Using AHP and MABAC with Type-2 Fuzzy Numbers in Automotive Industry. Proc. Inst. Mech. Eng. Part O 2022, 1748006X221095359. [Google Scholar] [CrossRef]
- Aleksić, A.; Milanović, D.D.; Komatina, N.; Tadić, D. Evaluation and Ranking of Failures in Manufacturing Process by Combining Best-Worst Method and VIKOR under Type-2 Fuzzy Environment. Expert Syst. 2022, 40, e13148. [Google Scholar] [CrossRef]
- Ecer, F. Multi-Criteria Decision Making for Green Supplier Selection Using Interval Type-2 Fuzzy AHP: A Case Study of a Home Appliance Manufacturer. Oper. Res. Int. J. 2022, 22, 199–233. [Google Scholar] [CrossRef]
- Chen, S.-M.; Lee, L.-W. Fuzzy Multiple Attributes Group Decision-Making Based on the Interval Type-2 TOPSIS Method. Expert Syst. Appl. 2010, 37, 2790–2798. [Google Scholar] [CrossRef]
- Mendel, J.M. Type-1 Fuzzy Sets and Fuzzy Logic. In Uncertain Rule-Based Fuzzy Systems: Introduction and New Directions, 2nd ed.; Mendel, J.M., Ed.; Springer International Publishing: Cham, Switzerland, 2017; pp. 25–99. ISBN 978-3-319-51370-6. [Google Scholar]
- Kahraman, C.; Öztayşi, B.; Uçal Sarı, İ.; Turanoğlu, E. Fuzzy Analytic Hierarchy Process with Interval Type-2 Fuzzy Sets. Knowl. Based Syst. 2014, 59, 48–57. [Google Scholar] [CrossRef]
- Bellman, R.E.; Zadeh, L.A. Decision-Making in a Fuzzy Environment. Manag. Sci. 1970, 17, B141–B164. [Google Scholar] [CrossRef]
- Chang, D.-Y. Applications of the Extent Analysis Method on Fuzzy AHP. Eur. J. Oper. Res. 1996, 95, 649–655. [Google Scholar] [CrossRef]
- Badri Ahmadi, H.; Kusi-Sarpong, S.; Rezaei, J. Assessing the Social Sustainability of Supply Chains Using Best Worst Method. Resour. Conserv. Recycl. 2017, 126, 99–106. [Google Scholar] [CrossRef]
- Pomerol, J.-C.; Barba-Romero, S. Multicriterion Decision in Management; International Series in Operations Research & Management Science; Springer: Boston, MA, USA, 2000; Volume 25, ISBN 978-1-4613-7008-6. [Google Scholar]
- Figueroa-García, J.C.; Chalco-Cano, Y.; Román-Flores, H. Distance Measures for Interval Type-2 Fuzzy Numbers. Discret. Appl. Math. 2015, 197, 93–102. [Google Scholar] [CrossRef]
- Zhou, S.; John, R.I.; Chiclana, F.; Garibaldi, J.M. On Aggregating Uncertain Information by Type-2 OWA Operators for Soft Decision Making. Int. J. Intell. Syst. 2010, 25, 540–558. [Google Scholar] [CrossRef]
- Kuo, M.-S.; Liang, G.-S. A Soft Computing Method of Performance Evaluation with MCDM Based on Interval-Valued Fuzzy Numbers. Appl. Soft Comput. 2012, 12, 476–485. [Google Scholar] [CrossRef]
- Chen, T.-Y. A Signed-Distance-Based Approach to Importance Assessment and Multi-Criteria Group Decision Analysis Based on Interval Type-2 Fuzzy Set. Knowl. Inf. Syst. 2013, 35, 193–231. [Google Scholar] [CrossRef]
- Liu, P.; Jin, F. A Multi-Attribute Group Decision-Making Method Based on Weighted Geometric Aggregation Operators of Interval-Valued Trapezoidal Fuzzy Numbers. Appl. Math. Model. 2012, 36, 2498–2509. [Google Scholar] [CrossRef]
- Lee, L.-W.; Chen, S.-M. Fuzzy Multiple Attributes Group Decision-Making Based on the Extension of TOPSIS Method and Interval Type-2 Fuzzy Sets. In Proceedings of the 2008 International Conference on Machine Learning and Cybernetics, Kunming, China, 12–15 July 2008; pp. 3260–3265. [Google Scholar] [CrossRef]
- Wang, Y.-M.; Elhag, T.M.S. Fuzzy TOPSIS Method Based on Alpha Level Sets with an Application to Bridge Risk Assessment. Expert Syst. Appl. 2006, 31, 309–319. [Google Scholar] [CrossRef]
- Qin, J.; Liu, X. Multi-Attribute Group Decision Making Using Combined Ranking Value under Interval Type-2 Fuzzy Environment. Inf. Sci. 2015, 297, 293–315. [Google Scholar] [CrossRef]
- Wang, Y.-M. Centroid Defuzzification and the Maximizing Set and Minimizing Set Ranking Based on Alpha Level Sets. Comput. Ind. Eng. 2009, 57, 228–236. [Google Scholar] [CrossRef]
- Karnik, N.N.; Mendel, J.M. Centroid of a Type-2 Fuzzy Set. Inf. Sci. 2001, 132, 195–220. [Google Scholar] [CrossRef]
- Mendel, J.M.; Wu, H. New Results about the Centroid of an Interval Type-2 Fuzzy Set, Including the Centroid of a Fuzzy Granule. Inf. Sci. 2007, 177, 360–377. [Google Scholar] [CrossRef]
- Kayapinar Kaya, S.; Aycin, E. An Integrated Interval Type 2 Fuzzy AHP and COPRAS-G Methodologies for Supplier Selection in the Era of Industry 4.0. Neural Comput. Appl. 2021, 33, 10515–10535. [Google Scholar] [CrossRef]
| Classification is Given by [5] | Classification is Given by [1] | Classification is Given by [3,4] | |
|---|---|---|---|
| Weighted Aggregated Sum Product Assessment-WASPAS [6] | Utility-based | Other MADMs | |
| Technique for Order Preference by Similarity to Ideal Solution-TOPSIS [7] | Distance-based | Normalizing models-additive types | Compromise |
| VIekriterijumsko KOmpromisno Rangiranje-VIKOR [8] | Distance-based | Normalizing models-additive types | Compromise |
| Complex Proportional Assessment-COPRAS [9] | Utility-based | Compromise | |
| Multi-Objective Optimization on the basis of Ratio Analysis-MOORA [10] | Other | ||
| Additive Ratio ASsessment-ARAS [11] | Other | Utility-based | |
| Elimination et Choix Traduisant la Realité-ELECTRE [12] | Outranking | Normalizing models-additive types | Outranking |
| Analytical Network Process-ANP [13] | Pairwise comparison | Weighting models | Utility-based |
| Analytic Hierarchy Process-AHP [14] | Pairwise comparison | Weighting models | Utility-based |
| (An acronym in Portuguese for interactive and multi-criteria decision-making)-TODIM [15] | Outranking | Utility-based | |
| Best Worst Method-BWM [16] | Pairwise comparison | Compromise | |
| Multi-attributive border approximation area comparison method-MABAC [17] | Other | Compromise | |
| Decision Making Trial and Evaluation Laboratory-DEMATEL [18] | Interaction based | Evaluating or choosing models | Other |
| Authors | Year | Research Focus | Rank of Alternatives |
|---|---|---|---|
| Celik et al. [27] | 2013 | The satisfaction of customers with public transportation | IT2FTOPSIS |
| Baležentis and Zeng [28] | 2013 | Selection of manager for research and development | IT2FMULTIMOORA |
| Ghorabaee et al. [29] | 2014 | Supplier selection | IT2FCOPRAS |
| Chen and Hong [30] | 2014 | The selection of a system analysis engineer | IT2FTOPSIS |
| Qin et al. [31] | 2015 | Metro station dynamic risk assessment | IT2FTOPSIS |
| Kilic and Kaya [32] | 2015 | Evaluation and selection of investment projects | IT2FTOPSIS |
| Abdullah and Zulkifli [33] | 2015 | Human resource management problem | IT2FDEMATEL |
| Cebi and Otay [34] | 2015 | Cement factory selection | IT2FTOPSIS |
| Qin et al. [35] | 2015 | Evaluation of the high-tech risk investment project | IT2FVIKOR |
| Ghorabaee et al. [36] | 2015 | Selecting a suitable hydroelectric power station project | IT2FVIKOR |
| Özkan et al. [37] | 2015 | Determining the best electrical energy storage technology | IT2FTOPSIS |
| Liao [38] | 2015 | Evaluation of materials | IT2FTOPSIS |
| Sang and Liu [39] | 2015 | Green supplier selection in the automotive industry | IT2FTODIM |
| Ghorabaee [19] | 2016 | Selecting the suitable robot for its production process | IT2FVIKOR |
| Celik et al. [40] | 2016 | Green Logistic Service Providers Evaluation | IT2FELECTRE |
| Ghorabaee et al. [41] | 2016 | Green supplier selection | IT2FWASPAS |
| Buyoukozkan et al. [42] | 2016 | Evaluation of Knowledge Management Tools | IT2FTOPSIS |
| Qin et al. [43] | 2017 | Green supplier selection | IT2FTODIM |
| Gorener et al. [44] | 2017 | Supplier selection in a high-stake aviation company | IT2FTOPSIS |
| Deveci et al. [45] | 2017 | Airline new route selection | IT2FTOPSIS |
| Mousakhani et al. [46] | 2017 | Green supplier evaluation | IT2FTOPSIS |
| Soner et al. [47] | 2017 | Selecting the right hatch cover design in maritime transportation industry | IT2FVIKOR |
| Zhong and Yao [48] | 2017 | Supplier selection | IT2FELECTRE |
| Deveci et al. [49] | 2018 | Selection for car-sharing station | IT2FWASPAS |
| Celik and Akyuz [20] | 2018 | Selecting the appropriate ship loader type | IT2FTOPSIS |
| Debnath and Biswas [50] | 2018 | The supplier selection problem | IT2FAHP |
| Meng et al. [51] | 2019 | Risk assessment of supply chain in social commerce | IT2FTODIM |
| Đurić et al. [52] | 2019 | The software failure analysis | IT2FCOPRAS |
| Dinçer et al. [53] | 2019 | Evaluate the financial service performance in E7 economies | IT2FMOORA |
| Xu et al. [54] | 2019 | Green supplier selection | IT2FAHP Sort II |
| Wu et al. [55] | 2019 | Green supplier selection | IT2FVIKOR |
| Aleksic et al. [56] | 2019 | Ranking failures in a recycling center | IT2FTOPSIS |
| Yucesan et al. [57] | 2019 | Green supplier selection | IT2FTOPSIS |
| Dorfeshan and Mousavi [58] | 2020 | Aircraft maintenance planning | IT2FMABAC |
| Bera et al. [59] | 2020 | Supplier selection | IT2FTOPSIS |
| Mohamadghasemi et al. [26] | 2020 | Selection of conveyors | IT2FELECTRE |
| Ayyildiz et al. [60] | 2020 | Credit application | IT2FELECTRE |
| Yang et al. [61] | 2020 | Choosing the best investment option | IT2FTOPSIS |
| Kiraci and Akan [21] | 2020 | Aircraft selection | IT2FTOPSIS |
| Pourmand et al. [62] | 2020 | Water Resources Management | IT2FTOPSIS |
| Özdemir and Üsküdar [63] | 2020 | Strategy selection | IT2FTOPSIS |
| Deveci et al. [64] | 2020 | Offshore wind farm development | IT2FTOPSIS |
| Mirnezami et al. [65] | 2021 | Project cash flow evaluation | IT2FTODIM |
| Sharaf [66] | 2021 | Solar power systems | IT2FTOPSIS |
| Komatina et al. [67] | 2021 | Evaluation of different risk factors | IT2FTOPSIS |
| Karagöz et al. [68] | 2021 | Facility location | IT2FARAS |
| Celik et al. [69] | 2021 | Green supplier selection | IT2FTODIM |
| Zhang et al. [70] | 2022 | The subway station’s risks | IT2FTOPSIS |
| Komatina et al. [71] | 2022 | Supplier selection | IT2FMABAC |
| Aleksić et al. [72] | 2022 | Evaluation and ranking of failures in the automotive industry | IT2FVIKOR |
| Ecer [73] | 2022 | Green supplier selection in-home appliance manufacturer | IT2FAHP |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aleksić, A.; Tadić, D. Industrial and Management Applications of Type-2 Multi-Attribute Decision-Making Techniques Extended with Type-2 Fuzzy Sets from 2013 to 2022. Mathematics 2023, 11, 2249. https://doi.org/10.3390/math11102249
Aleksić A, Tadić D. Industrial and Management Applications of Type-2 Multi-Attribute Decision-Making Techniques Extended with Type-2 Fuzzy Sets from 2013 to 2022. Mathematics. 2023; 11(10):2249. https://doi.org/10.3390/math11102249
Chicago/Turabian StyleAleksić, Aleksandar, and Danijela Tadić. 2023. "Industrial and Management Applications of Type-2 Multi-Attribute Decision-Making Techniques Extended with Type-2 Fuzzy Sets from 2013 to 2022" Mathematics 11, no. 10: 2249. https://doi.org/10.3390/math11102249
APA StyleAleksić, A., & Tadić, D. (2023). Industrial and Management Applications of Type-2 Multi-Attribute Decision-Making Techniques Extended with Type-2 Fuzzy Sets from 2013 to 2022. Mathematics, 11(10), 2249. https://doi.org/10.3390/math11102249







