Super Spanning Connectivity of the Folded Divide-and-SwapCube
Abstract
1. Introduction
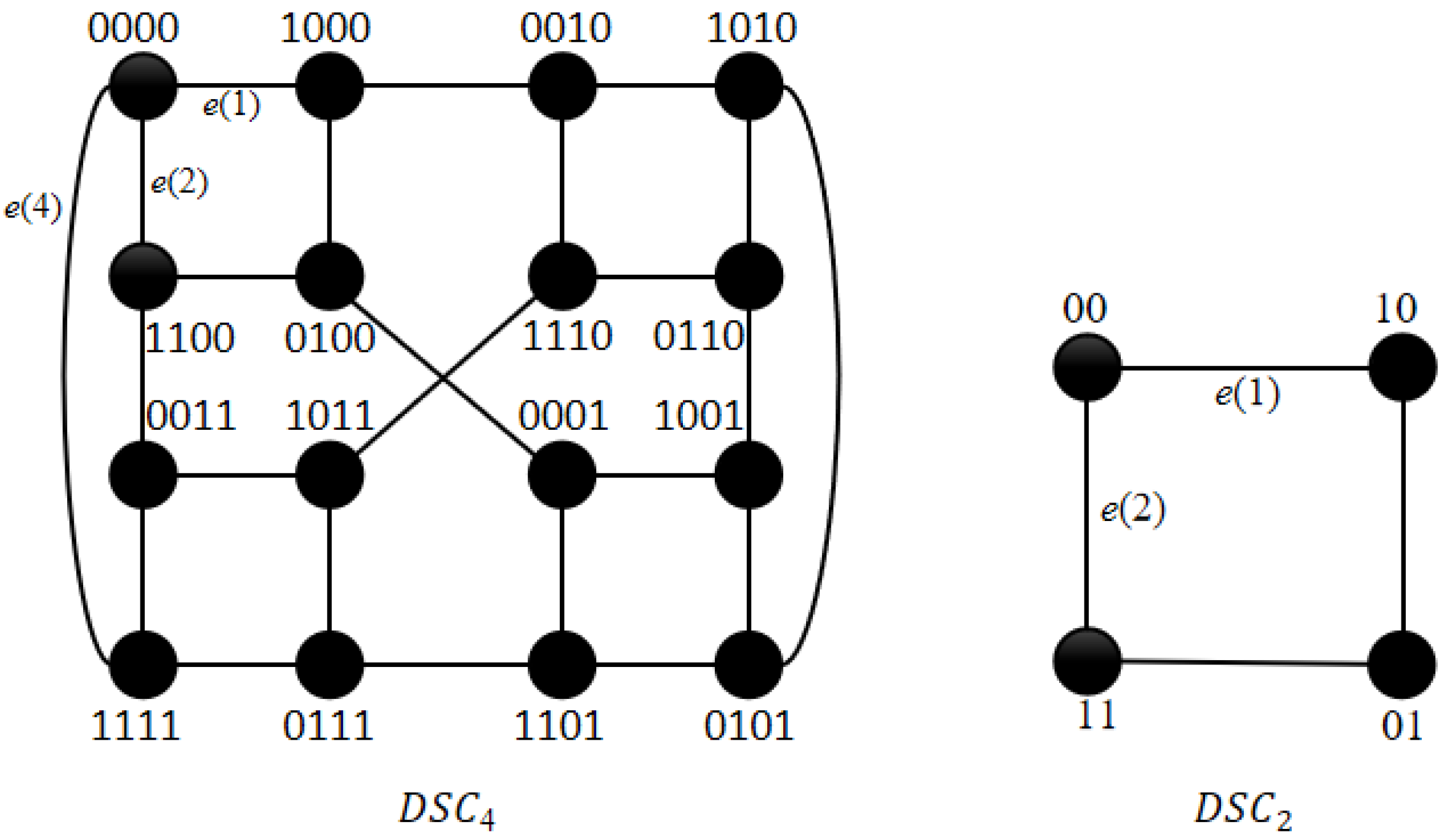
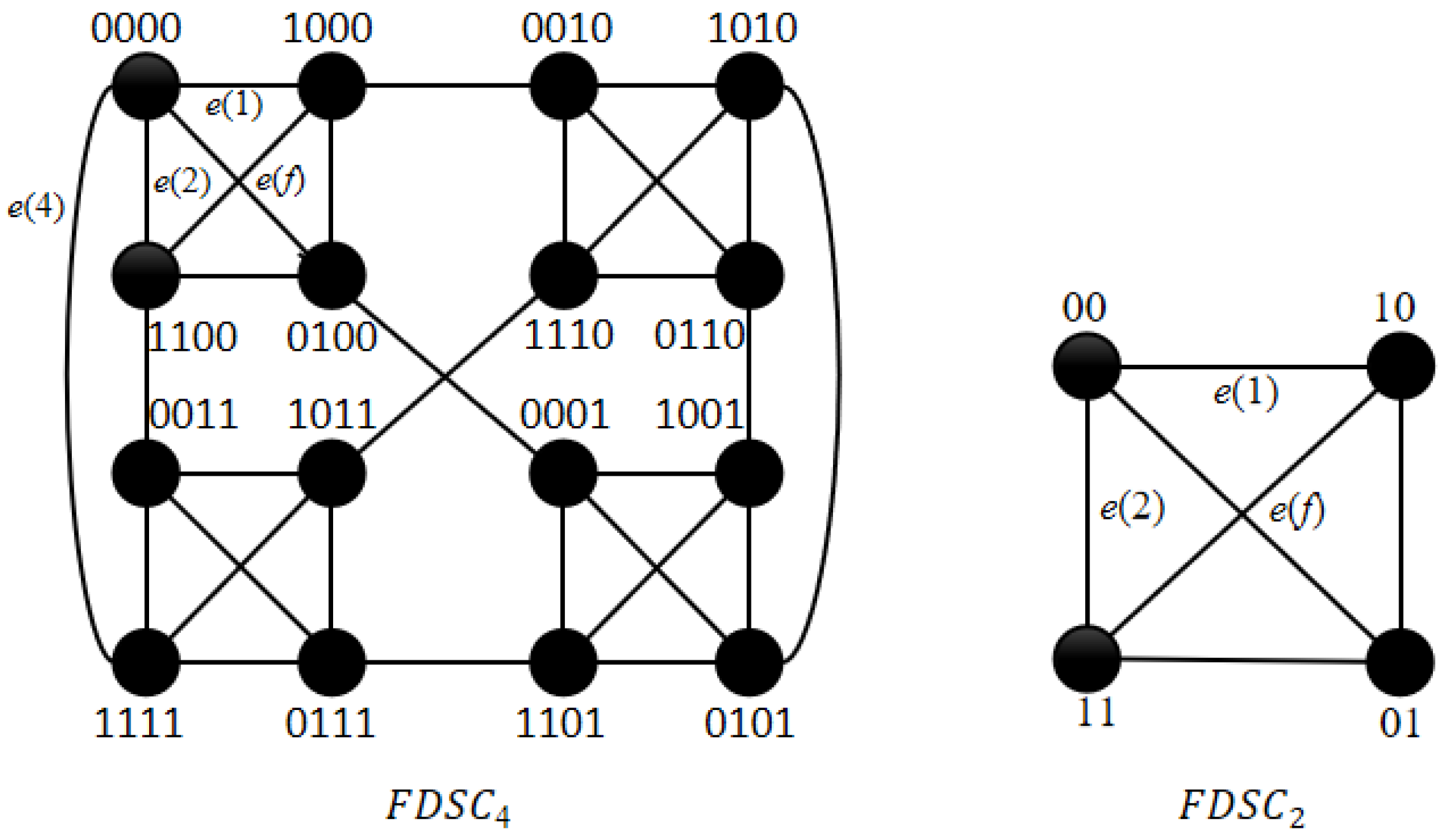
2. Preliminaries
- (1)
- , where is the complement of . Here, is called an -edge.
- (2)
- Here, is called an -edge.
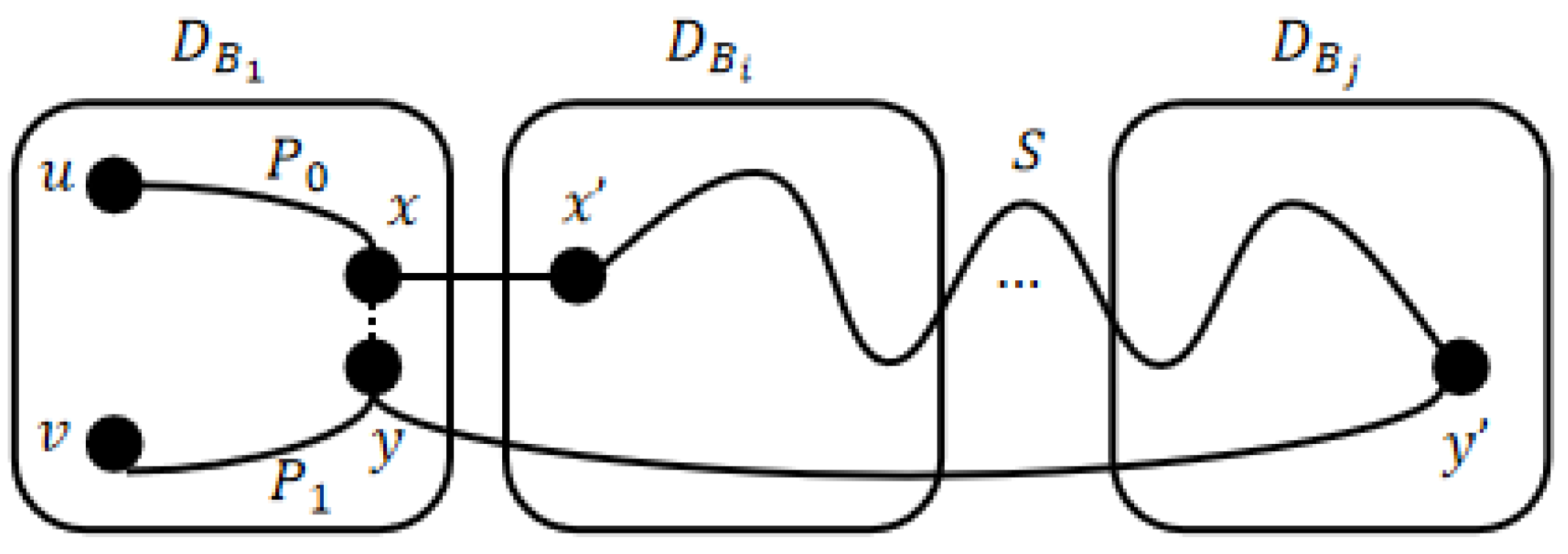
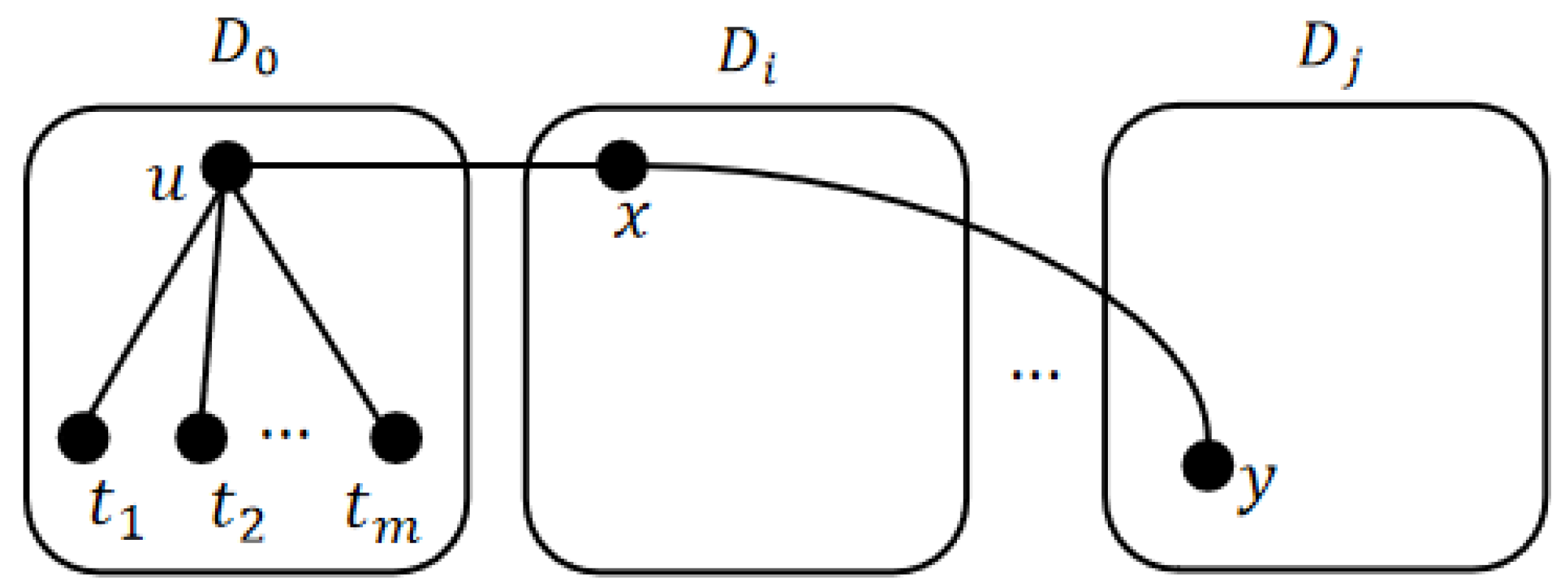
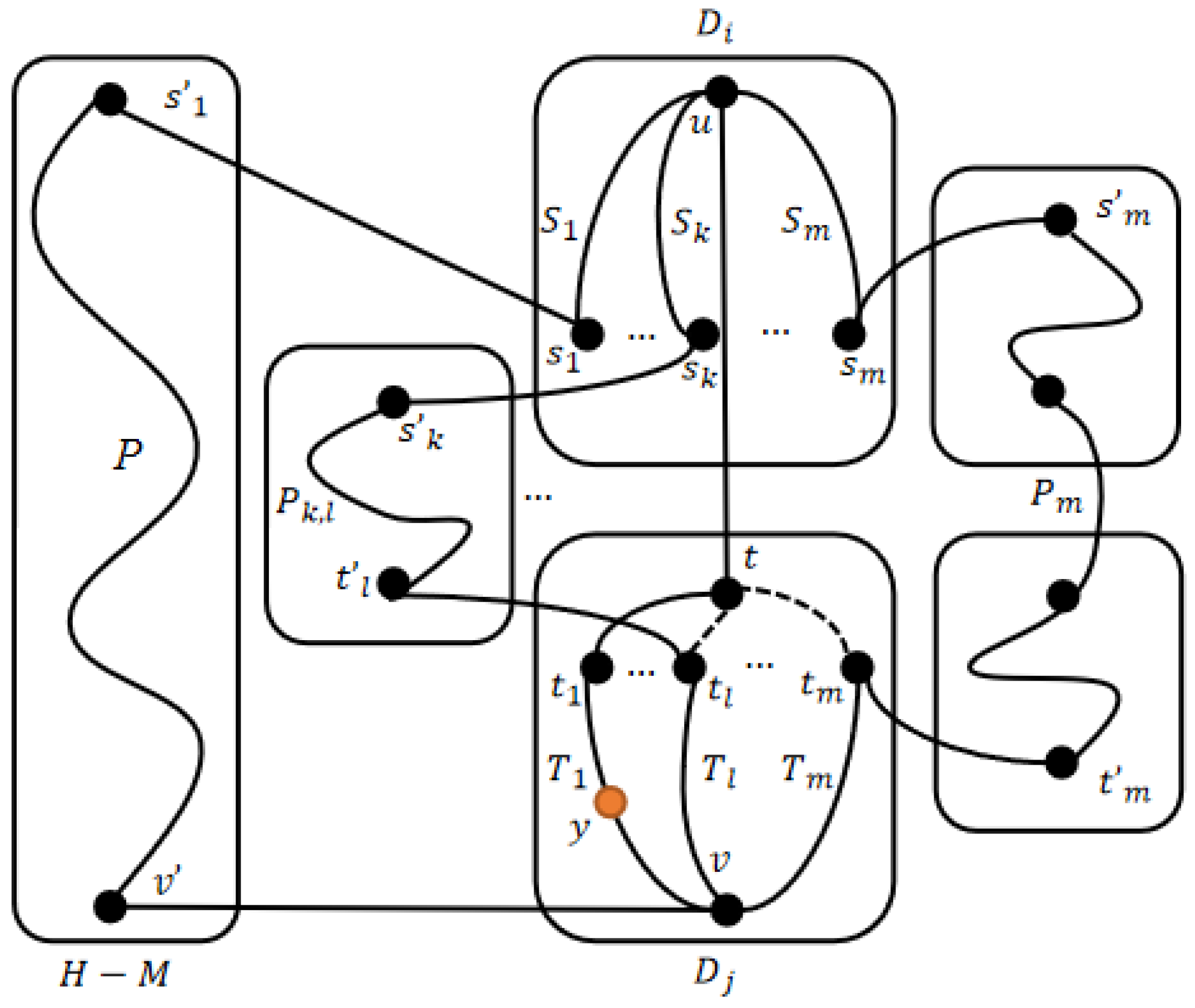
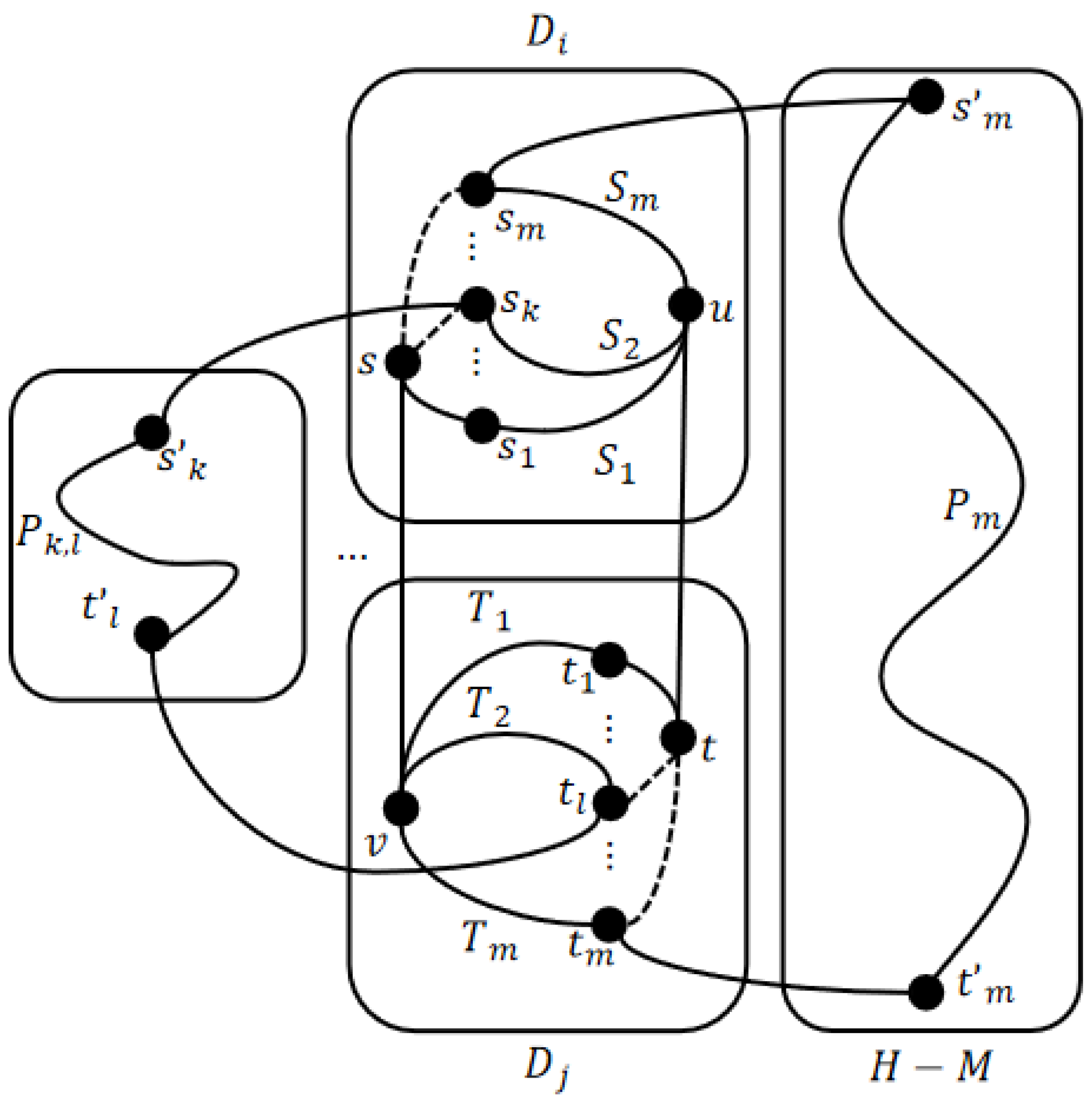
3. The Super Spanning Connectivity of
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhou, S.; Xiao, W.; Parhami, B. Construction of vertex-disjoint paths in alternating group networks. J. Supercomput. 2010, 54, 206–228. [Google Scholar] [CrossRef]
- Cheng, D.; Hao, R.; Feng, Y. Two node-disjoint paths in balanced hypercubes. Appl. Math. Comput. 2014, 242, 127–142. [Google Scholar] [CrossRef]
- Wang, X.; Fan, J.; Lin, C.-K.; Jia, X. Vertex-disjoint paths in dcell networks. J. Parallel Distrib. Comput. 2016, 96, 38–44. [Google Scholar] [CrossRef]
- Lai, P.-L.; Hsu, H.-C. On the two-equal-disjoint path cover problem of crossed cubes. In Proceedings of the 9th Joint Conference on Information Sciences, JCIS, Kaohsiung, Taiwan, 8–11 October 2006; pp. 603–606. [Google Scholar]
- Gomes, T.; Craveirinha, J.; Jorge, L. An effective algorithm for obtaining the minimal cost pair of disjoint paths with dual arc costs. Comput. Oper. Res. 2009, 36, 1670–1682. [Google Scholar] [CrossRef]
- Liu, C.; Yarvis, M.; Conner, W.S.; Guo, X. Guaranteed on-demand discovery of node-disjoint paths in Ad Hoc networks. Comput. Commun. 2007, 30, 2917–2930. [Google Scholar] [CrossRef]
- Day, K.; Al-Ayyoub, A.E. Fault diameter of k-ary n-cube networks. IEEE Trans. Parallel Distrib. Syst. 1997, 8, 903–907. [Google Scholar] [CrossRef]
- Shih, Y.-K.; Kao, S.-S. One-to-one disjoint path covers on k-ary n-cubes. Theoret. Comput. Sci. 2011, 412, 4513–4530. [Google Scholar] [CrossRef]
- Lai, P.-L.; Hsub, H.-C. The two-equal-disjoint path cover problem of Matching Composition Network. Inform. Process. Lett. 2008, 107, 18–23. [Google Scholar] [CrossRef]
- Ntafos, S.C.; Hakimi, S.L. On path cover problems in digraphs and applications to program testing. IEEE Trans. Softw. Eng. 1979, 5, 520–529. [Google Scholar] [CrossRef]
- Lin, C.K.; Tan, J.J.; Hsu, D.F.; Hsu, L.H. On the spanning connectively and spanning laceability of hypercube-like networks. Theor. Comput. Sci. 2007, 381, 218–229. [Google Scholar] [CrossRef]
- You, L.; Fan, J.; Han, Y.; Jia, X. One-to-one disjoint path covers on alternating group graphs. Theoret. Comput. Sci. 2015, 562, 146–164. [Google Scholar] [CrossRef]
- Li, J.; Liu, D.; Yang, Y.; Yuan, J. One-to-one disjoint path covers on multi-dimensional tori. Int. J. Comput. Math. 2015, 92, 1114–1123. [Google Scholar] [CrossRef]
- Wang, X.; Fan, J.; Jia, X.; Lin, C.-K. An efficient algorithm to construct disjoint path covers of DCell networks. Theoret. Comput. Sci. 2016, 609, 197–210. [Google Scholar] [CrossRef]
- You, L.; Fan, J.; Han, Y. Super spanning connectivity on WK-recursive networks. Theor. Comput. Sci. 2018, 713, 42–55. [Google Scholar] [CrossRef]
- Wang, J.-J.; Hsu, L.-H. On the spanning connectivity of the generalized Petersen graphs P(n, 3). Discret. Math. 2018, 341, 672–690. [Google Scholar] [CrossRef]
- Li, J.; Li, X.; Cheng, E. Super spanning connectivity of split-star networks. Inf. Process. Lett. 2021, 166, 106037. [Google Scholar] [CrossRef]
- Kim, J.S.; Kim, D.Y.; Qiu, K.; Lee, H.O. The divide-and-swap cube: A new hypercube variant with small network cost. J. Supercomput. 2019, 75, 3621–3639. [Google Scholar] [CrossRef]
- Ning, W. Connectivity and super connectivity of the divide-and-swap cube. Theor. Comput. Sci. 2020, 842, 1–5. [Google Scholar] [CrossRef]
- Zhou, Q.; Zhou, S.; Liu, J.; Liu, X. Structure and substructure connectivity of divide-and-swap cube. Theor. Comput. Sci. 2021, 880, 20–36. [Google Scholar] [CrossRef]
- Zhou, Q.; Zhou, S.; Liu, X.; Yu, Z. Reliability of divide-and-swap cube based on r-component connectivity and diagnosability. J. Interconnect. Netw. 2022, 22, 2142021. [Google Scholar] [CrossRef]
- Zhao, S.; Chang, J. Reliability assessment of the divide-and-swap cube in terms of generalized connectivity. Theor. Comput. Sci. 2023, 943, 1–15. [Google Scholar] [CrossRef]
- Zhao, S.-L.; Chang, J.-M. Connectivity, super connectivity and generalized 3-connectivity of folded divide-and-swap cubes. Inf. Process. Lett. 2023, 182, 106377. [Google Scholar] [CrossRef]
- Chang, Y.-H.; Pai, K.-J.; Hsu, C.-C.; Yang, J.-S.; Chang, J.-M. Constructing dual-CISTs of folded divide-and-swap cubes. Theor. Comput. Sci. 2021, 856, 75–87. [Google Scholar] [CrossRef]
- Li, X.; Fan, J.; Lin, C.-K.; Jia, X. Diagnosability Evaluation of the Data Center Network DCell. Comput. J. 2018, 61, 129–143. [Google Scholar] [CrossRef]
- Gu, M.; Hao, R.; Jiang, L. Fault-tolerance and diagnosability of hierarchical star networks. Int. J. Comput. Math. Comput. Syst. Theory 2018, 3, 106–121. [Google Scholar] [CrossRef]
- Zhang, H.; Zhou, S.; Liu, X.; Yu, Z. Extra (component) connectivity and diagnosability of bubble sort networks. Theor. Comput. Sci. 2023, 940, 180–189. [Google Scholar] [CrossRef]




| Symbol | Definition |
|---|---|
| the n-dimensional divide-and-swap cube where and d is any positive integer | |
| the n-dimensional folded divide-and-swap cube | |
| disjoint path cover | |
| the ith module of with | |
| the spanning connectivity of a graph G |
| 3-DPC | 4-DPC | |
|---|---|---|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
You, L.; Jiang, J.; Han, Y. Super Spanning Connectivity of the Folded Divide-and-SwapCube. Mathematics 2023, 11, 2581. https://doi.org/10.3390/math11112581
You L, Jiang J, Han Y. Super Spanning Connectivity of the Folded Divide-and-SwapCube. Mathematics. 2023; 11(11):2581. https://doi.org/10.3390/math11112581
Chicago/Turabian StyleYou, Lantao, Jianfeng Jiang, and Yuejuan Han. 2023. "Super Spanning Connectivity of the Folded Divide-and-SwapCube" Mathematics 11, no. 11: 2581. https://doi.org/10.3390/math11112581
APA StyleYou, L., Jiang, J., & Han, Y. (2023). Super Spanning Connectivity of the Folded Divide-and-SwapCube. Mathematics, 11(11), 2581. https://doi.org/10.3390/math11112581







