In the following definition, we adapt this concept to the non-autonomous context.
3.1. The Case Maximal
We will first study the case wherein is a maximal sequence. From Lemma 1, this implies that for all i such that , so for any i, and . This means that if is maximal, then in the entire orbit set , it makes no difference to apply or T.
For an iteration pattern
, let
Let be a p-periodic sequence, and be the non-autonomous set such that for all ().
Theorem 2 (Existence of Milnor attractors). Let be a p-periodic sequence and be such that:
- 1.
.
- 2.
is maximal.
- 3.
.
Then, is a Milnor attractor.
Proof. Since X is periodic, then is projection-closed.
From Condition 1
, so from Condition 2 and Theorem 1, for all
and
is
-invariant. From its definition, no other non-autonomous set
such that
could be
invariant so
is projection-minimal.
For any
, let
,
exists from Condition 3. Then, from Condition 2, for all
so
and, since
, then
has positive measure. □
Remark 3. In [20] is introduced a notion of finite-time attractivity, mainly focused in the speed of convergence. It warrants that some kind of convergence happens in finite time. In the present context, the convergence always happens in finite time, for each m. Theorem 3 (Existence of local attractors). Let be such that:
- 1.
There is p minimal such that .
- 2.
is maximal.
- 3.
There is j such that for all .
Then, the non-autonomous set such that if and for all is an local attractor if and only if . Moreover, this local attractor is p-cyclic.
Proof. Since , is projection-closed. Since U is maximal, for all , so is invariant and this implies that it is also projection-minimal.
Now, we will see that is locally attractive if and only if .
U maximal and p-periodic implies that is a pre-image of u under T, so .
We will make the proof only considering , since it follows analogously for . We will also suppose without loss of generality that and consequently for all n.
Suppose first that . If then for all .
Since
then
, so
and
is decreasing and onto in a neighborhood of
u, so in this neighborhood there is
such that
. Analogously, in this neighborhood there is
such that
. Consider now the neighborhood of
,
,
and
. On the one hand, since
U is maximal, then
and, because
,
On the other hand, , so and . We conclude that is attracting. The cyclicity of is immediate from the definition.
If , then since , is increasing in a neighborhood of u, so for any n, if is close enough to u, then and . □
It follows from the definitions that, for pattern iterations of flat top tent maps, any local attractor or Milnor attractor must attract and consequently u. Since if U is maximal then the iterates do not depend on the starting time k, we can conclude the following.
Theorem 4. If is maximal then, for any , there can not exist two attractors and , local or Milnor, with different fiber projections .
3.2. The Case Non-Maximal
Let us now study what happens when is not maximal. In this context, we must consider defined in the following way:
Let
be such that
, then
Theorem 5 (Existence of Milnor attractors). Let be a p-periodic sequence and be such that is not maximal and
- 1.
For all , .
- 2.
.
- 3.
.
Then is an Milnor attractor.
Proof. Condition 1 allows us to use the same arguments as in Theorem 2 to conclude that is invariant and projection-minimal.
For any
, let
,
exists due to condition 3. Now, let
be the left branch of the tent map,
and
For we have that and for all j. Moreover, for , we have that, for all j, , so for all , and, since then , so and . □
Remark 4. Conditions 1 and 2 in the previous Theorem imply that for all i.
Remark 5. Note that Milnor attractors, on one hand, are not robust in relation with the parameter u, in the sense that the attractor does not persist under small perturbations of u, but on the other hand they present a certain robustness in relation with the iteration pattern s. From Theorem 2, if is maximal then the attractor persists for all patterns s such that and, from Theorem 5, if is not maximal then the attractor persists for all patterns s such that .
Consider now
, then
is not maximal because
, so
implies that
and there is
such that
and
. Let
be such that
; then, for all
,
and we have that, if
s is such that
for all
n, then the non-autonomous set
such that
and
for all
is a
local attractor. Therefore, the introduction of the condition
for all
n created a
2-cyclic local attractor, see
Figure 1.
More generally, if
U is such that
for some
k, let
then we have the following Theorem.
Theorem 6 (Existence of local attractors). Let be such that:
- 1.
is not maximal, i.e., there exists k such that .
- 2.
There is such that, for all and , and .
Then, the non-autonomous set such that for all and, for all , and , , is a k-cyclic local attractor.
Proof. We will consider
. If
, then, from Lemma 1
. On the other hand,
for all
implies that
and so
. Moreover, for all
and
so
is
invariant and
k-cyclic and so it is also projection-minimal. On the other hand, since, for all
n,
is onto in each of its branches of monotonicity, there are
such that
is monotonous and
. For each
and each
we consider the neighborhoods of
,
. To simplify the notation, we will consider
.
If there is no
such that
and
, then
On the other hand, if there is
such that
and
then, from Condition (2) and the monotonicity of
, we have that
or
for some
.
Without loss of generality, we will only consider the first situation.
Suppose that
i is unique, then
If
i is not unique, i.e., if there is
such that, for all
,
and
, then, as before, we can take
such that
so
If we continue this procedure, then
Just as for
we can consider
such that
and
Therefore, we conclude that
and, recursively, that
□
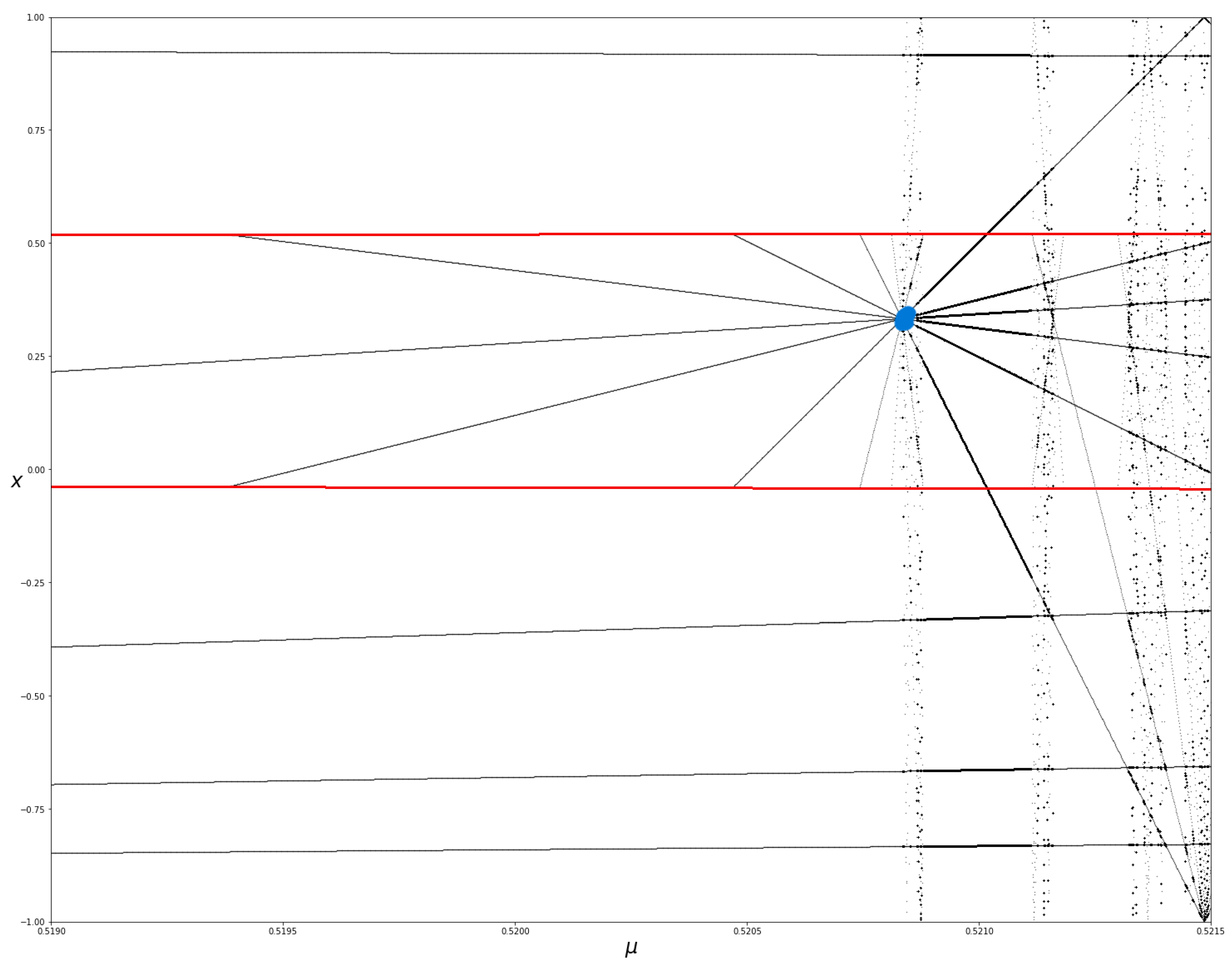
Example 1. Let us consider the iteration pattern , and . We have that and , so, from Lemma 1, for any parameter , and, since , and for all , from Theorem 6 the system has a 2-cyclic local attractor. This can be seen in Figure 2, where the attractor is depicted in red. Looking now at the point depicted in blue in Figure 2, it has coordinates and Numerically, is a fixed point of T.
Having in mind Theorem 5, for , , so . On the other hand, for all , and , therefore . Therefore, considering , is a Milnor attractor. We conclude that, for and one local attractor and one Milnor attractor coexist. Note that this is just possible because is not maximal.
We can merge Theorems 5 and 6 to obtain the following.
Theorem 7 (Coexistence of local attractors and Milnor attractors). Let be a p-periodic sequence and be such that:
- 1.
For all , .
- 2.
such that .
- 3.
For there is l such that .
- 4.
.
- 5.
There is an order such that, for all , and for all .
Then, the non-autonomous set such that for all and , for is an local attractor and is a Milnor attractor.
We will see in the following example that, in the non-maximal case two local attractors can also coexist.
Example 2. Consider again, and, now, . We have that It is easy to verify that and are the only such that , so, regarding Theorem 6, . Since s is 6-periodic, and , then and for all , so, from Theorem 6, the non-autonomous set such that for all and all is a 12-cyclic local attractor. Since , this 12-cyclic local attractor coexists with the 2-cyclic one studied in Example 1. Looking at Figure 2, we see that this situation persists in a neighborhood around . There is another remarkable difference between the maximal and non-maximal cases: From Theorem 3, in the maximal case all
local attractors are cyclic. To illustrate the non-maximal case consider now
and
. Evidently,
U is not maximal because
and
. Now, adapting the arguments of the proof of Theorem 6, for any iteration pattern
, where
, the non-autonomous set
such that
and
for all
is an
local attractor but, in spite of
unlike in the maximal case, it may be non-cyclic. This is the case when the sequence
is neither periodic nor eventually periodic.
In this case, we may still have coexistence of a
local attractor with an
Milnor attractor. Consider for example a pattern
such that, for all
i,
and
.
On one hand, we have a subsequence such that, for all j, and and this generates the local attractor. On the other hand, we have an infinite subsequence such that, for all j, and and this generates the Milnor attractor.
Remark 6. A more general formulation for sufficient conditions for the existence of an local attractor is the existence of such that for all and one sequence such that, for all , and there exists such that and for all .