Exact Solution of Non-Homogeneous Fractional Differential System Containing 2n Periodic Terms under Physical Conditions

Abstract

This paper solves a generalized class of first-order fractional ordinary differential equations (1st-order FODEs) by means of Riemann–Liouville fractional derivative (RLFD). The principal incentive of this paper is to generalize some existing results in the literature. An effective approach is applied to solve non-homogeneous fractional differential systems containing $2n$ periodic terms. The exact solutions are determined explicitly in a straightforward manner. The solutions are expressed in terms of entire functions with fractional order arguments. Features of the current solutions are discussed and analyzed. In addition, the existing solutions in the literature are recovered as special cases of our results.

1. Introduction

The fractional calculus (FC) [1,2,3] considers the derivatives of arbitrary (non-integer) order instead of integer-order derivatives in the classical calculus (CC). Really, the FC is a natural extension of the CC. In recent decades, many physical and biological problems have been modeled in view of the FC utilizing several definitions, such as the Caputo fractional derivative (CFD), the Riemann–Liouville fractional derivative (RLFD), and others [4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25,26]. The objective of this paper is to extend the application of the RLFD to the following class of 1st-order FODEs

$${}_{-\infty }^{RL}{D}_t^{\alpha }y\left(t\right)+{\omega }^{2}y\left(t\right)=\sum _{j=1}^n(a_{j}cos\left({\mathsf{\Omega }}_{j}t\right)+b_{j}sin\left({\mathsf{\Omega }}_{j}t\right)),y\left(0\right)=A,0<\alpha \le 1,$$

(1)

where $\alpha$ is the order of the RLFD ($\alpha$ is a real non-integer number). The RLFD of order $\alpha \in {\mathbb{R}}_0^{+}$ of function $f:[c,d]\to \mathbb{R}(-\infty <c<d<\infty )$ is [1,2,3]

$${}_c^{RL}{D}_t^{\alpha }f\left(t\right)=\frac{1}{\mathsf{\Gamma }\left(n-\alpha \right)}\frac{d^n}{d{t}^n}\left({\int }_c^t\frac{f\left(\tau \right)}{{\left(t-\tau \right)}^{\alpha -n+1}}d\tau \right),n=\left[\alpha \right]+1,t>c,$$

(2)

where $\left[\alpha \right]$ is the integer part of $\alpha$. As $c\to -\infty$ and for $0<\alpha \le 1$, we have:

$${}_{-\infty }^{RL}{D}_t^{\alpha }f\left(t\right)=\frac{1}{\mathsf{\Gamma }\left(1-\alpha \right)}\frac{d}{dt}\left({\int }_{-\infty }^t\frac{f\left(\tau \right)}{{\left(t-\tau \right)}^{\alpha }}d\tau \right).$$

(3)

For the class (1), the quantities A, $\omega$, $a_j$, $b_j$, and ${\mathsf{\Omega }}_j$ are constants $\forall j=1,2,3,\cdots ,n$. The present class is of practical applications in engineering for harmonic oscillators in view of the FC. Moreover, the class (1) splits to several interesting classes at special cases of ${\mathsf{\Omega }}_j$, $a_j$, and $b_j$ as follows. For ${\mathsf{\Omega }}_j=i{\sigma }_j$ and $b_j=-i{d}_j$, where $i=\sqrt{-1}$, we have the following class in terms of hyperbolic functions:

$${}_{-\infty }^{RL}{D}_t^{\alpha }y\left(t\right)+{\omega }^{2}y\left(t\right)=\sum _{j=1}^n(a_{j}cosh\left({\sigma }_{j}t\right)+d_{j}sinh\left({\sigma }_{j}t\right)),y\left(0\right)=A,0<\alpha \le 1.$$

(4)

For ${\mathsf{\Omega }}_j=i{\mu }_j$ and $b_j=i{a}_j$, the right hand side of class (1) reduces to exponential functions and hence the class

$${}_{-\infty }^{RL}{D}_t^{\alpha }y\left(t\right)+{\omega }^{2}y\left(t\right)=\sum _{j=1}^n{a}_j{e}^{-{\mu }_{j}t},y\left(0\right)=A,0<\alpha \le 1,$$

(5)

is resulting. The most important notice here is that the initial condition (IC) is considered in a physical sense, i.e., $y\left(0\right)=A$. Usually, the researchers in the field of FC use the IC in the fractional form $D_t^{\alpha -1}y\left(0\right)=A$ when dealing with the RLFD. This is because the Laplace transform (LT) of the RLFD is expressed in terms of $D_t^{\alpha -1}y\left(0\right)$, where the LT of the RLFD (2) (as $c\to 0,\alpha \in (0,1]$) is:

$$\mathcal{L}\left[{}_0^{RL}{D}_t^{\alpha }y\left(t\right)\right]=s^{\alpha }Y\left(s\right)-D_t^{\alpha -1}y\left(0\right).$$

(6)

However, the IC in such fractional form $D_t^{\alpha -1}y\left(0\right)$ has no physical meaning till the moment. So, we consider in this paper $y\left(0\right)=A$ instead of $D_t^{\alpha -1}y\left(0\right)=A$. In this regard, the authors in Ref. [26] recently utilized the same concept to solve a special case of the present model.

One can find in Refs. [21,22,23,24,25,26,27] that the approximate/exact solution of a physical model mainly depends on both the formulated ICs and the chosen method of solution. The following properties are essential [27,28]:

$$\begin{array}{ccc}& & {}_{-\infty }^{RL}{D}_t^{\alpha }{e}^{i\omega t}={\left(i\omega \right)}^{\alpha }{e}^{i\omega t},\hfill \end{array}$$

(7)

$$\begin{array}{ccc}& & {}_{-\infty }^{RL}{D}_t^{\alpha }cos\left(\omega t\right)={\omega }^{\alpha }cos\left(\omega t+\frac{\alpha \pi }{2}\right),\hfill \end{array}$$

(8)

$$\begin{array}{ccc}& & {}_{-\infty }^{RL}{D}_t^{\alpha }sin\left(\omega t\right)={\omega }^{\alpha }sin\left(\omega t+\frac{\alpha \pi }{2}\right).\hfill \end{array}$$

(9)

The structure of this paper is as follows. In Section 2, the complementary and the particular solutions for the present class are provided theoretically. Section 3 is assigned to the analytic solution of the present class. Special cases of the FODEs (1) are discussed in Section 4. Section 5 analyzes three cases of ODEs at the special case $\alpha \to 1$. In Section 6, a theoretical analysis for the values of $\alpha$ that lead to real solutions is discussed. In Section 7, the main conclusions are summarized.

2. Analysis

In Ref. [28], the authors derived the complementary solution $y_c\left(t\right)$ of Equation (1) in the form

$$y_c\left(t\right)=c{e}^{i\delta t},\delta =-i{\left(-{\omega }^2\right)}^{1/\alpha },$$

(10)

where c is unknown constant. The $y_c\left(t\right)$ satisfies the homogeneous equation:

$${}_{-\infty }^{RL}{D}_t^{\alpha }y\left(t\right)+{\omega }^{2}y\left(t\right)=0.$$

(11)

The constant c can be determined later by applying the given IC. The following theorem determines the particular solution $y_p\left(t\right)$ of the non-homogeneous Equation (1).

Theorem 1. 

The particular solution $y_p\left(t\right)$ of Equation (1) can be obtained in the form

$$y_p\left(t\right)=\sum _{j=1}^n({\rho }_{1j}cos\left({\mathsf{\Omega }}_{j}t\right)+{\rho }_{2j}sin\left({\mathsf{\Omega }}_{j}t\right)),$$

(12)

where

$${\rho }_{1j}=\frac{a_j{\omega }^2+{\mathsf{\Omega }}^{\alpha }\left(a_{j}cos\left(\frac{\pi \alpha }{2}\right)-b_{j}sin\left(\frac{\pi \alpha }{2}\right)\right)}{{\omega }^4+{\mathsf{\Omega }}_j^{2\alpha }+2{\omega }^2{\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)},{\rho }_{2j}=\frac{b_j{\omega }^2+{\mathsf{\Omega }}_j^{\alpha }\left(b_{j}cos\left(\frac{\pi \alpha }{2}\right)+a_{j}sin\left(\frac{\pi \alpha }{2}\right)\right)}{{\omega }^4+{\mathsf{\Omega }}_j^{2\alpha }+2{\omega }^2{\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}.$$

(13)

Proof. 

In view of the assumption (12), we have

$$\begin{array}{ccc}\hfill {}_{-\infty }^{RL}{D}_t^{\alpha }{y}_p& =& \sum _{j=1}^n\left({\rho }_{1j}{}_{-\infty }^{RL}{D}_t^{\alpha }cos\left({\mathsf{\Omega }}_{j}t\right)+{\rho }_{2j}{}_{-\infty }^{RL}{D}_t^{\alpha }sin\left({\mathsf{\Omega }}_{j}t\right)\right),\hfill \\ & =& \sum _{j=1}^n{\mathsf{\Omega }}_j^{\alpha }cos\left({\mathsf{\Omega }}_{j}t\right)\left({\rho }_{1j}cos\left(\frac{\pi \alpha }{2}\right)+{\rho }_{2j}sin\left(\frac{\pi \alpha }{2}\right)\right)+\hfill \\ & & \sum _{j=1}^n{\mathsf{\Omega }}_j^{\alpha }sin\left({\mathsf{\Omega }}_{j}t\right)\left({\rho }_{2j}cos\left(\frac{\pi \alpha }{2}\right)-{\rho }_{1j}sin\left(\frac{\pi \alpha }{2}\right)\right),\hfill \end{array}$$

(14)

and hence

$$\begin{array}{ccc}\hfill {}_{-\infty }^{RL}{D}_t^{\alpha }{y}_p+{\omega }^2{y}_p& =& \sum _{j=1}^n\left[\left({\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)+{\omega }^2\right){\rho }_{1j}+{\mathsf{\Omega }}_j^{\alpha }sin\left(\frac{\pi \alpha }{2}\right){\rho }_{2j}\right]cos\left({\mathsf{\Omega }}_{j}t\right)+\hfill \\ & & \sum _{j=1}^n\left[\left({\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)+{\omega }^2\right){\rho }_{2j}-{\mathsf{\Omega }}_j^{\alpha }sin\left(\frac{\pi \alpha }{2}\right){\rho }_{1j}\right]sin\left({\mathsf{\Omega }}_{j}t\right).\hfill \end{array}$$

(15)

Substituting (15) into (1) and comparing both sides, we obtain the algebraic system:

$$\begin{array}{cc}& \left({\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)+{\omega }^2\right){\rho }_{1j}+{\mathsf{\Omega }}_j^{\alpha }sin\left(\frac{\pi \alpha }{2}\right){\rho }_{2i}=a_j,\hfill \\ & \left({\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)+{\omega }^2\right){\rho }_{2j}-{\mathsf{\Omega }}_j^{\alpha }sin\left(\frac{\pi \alpha }{2}\right){\rho }_{1j}=b_j.\hfill \end{array}$$

(16)

Solving this system for the coefficients ${\rho }_{1j}$ and ${\rho }_{2j}$, yields

$${\rho }_{1j}=\frac{a_j{\omega }^2+{\mathsf{\Omega }}_j^{\alpha }\left(a_{j}cos\left(\frac{\pi \alpha }{2}\right)-b_{j}sin\left(\frac{\pi \alpha }{2}\right)\right)}{{\omega }^4+{\mathsf{\Omega }}_j^{2\alpha }+2{\omega }^2{\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)},{\rho }_{2j}=\frac{b_j{\omega }^2+{\mathsf{\Omega }}_j^{\alpha }\left(b_{j}cos\left(\frac{\pi \alpha }{2}\right)+a_{j}sin\left(\frac{\pi \alpha }{2}\right)\right)}{{\omega }^4+{\mathsf{\Omega }}_j^{2\alpha }+2{\omega }^2{\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}.$$

(17)

Inserting (17) into $y_p$ in (12) and simplifying, we obtain

$$y_p\left(t\right)=\sum _{j=1}^n\frac{{\omega }^2\left[a_{j}cos\left({\mathsf{\Omega }}_{j}t\right)+b_{j}sin\left({\mathsf{\Omega }}_{j}t\right)\right]+{\mathsf{\Omega }}_j^{\alpha }\left[a_{j}cos\left({\mathsf{\Omega }}_{j}t-\frac{\pi \alpha }{2}\right)+b_{j}sin\left({\mathsf{\Omega }}_{j}t-\frac{\pi \alpha }{2}\right)\right]}{{\omega }^4+{\mathsf{\Omega }}_j^{2\alpha }+2{\omega }^2{\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}.$$

(18)

□

Lemma 1. 

The particular solution of the (FODE):

$${}_{-\infty }^{RL}{D}_t^{\alpha }y\left(t\right)+{\omega }^{2}y\left(t\right)=\sum _{j=1}^n{b}_{j}sin\left({\mathsf{\Omega }}_{j}t\right),0<\alpha \le 1,$$

(19)

is

$$y_p\left(t\right)=\sum _{j=1}^n{b}_j\left(\frac{{\omega }^{2}sin\left({\mathsf{\Omega }}_{j}t\right)+{\mathsf{\Omega }}_j^{\alpha }sin\left({\mathsf{\Omega }}_{j}t-\frac{\pi \alpha }{2}\right)}{{\omega }^4+{\mathsf{\Omega }}_j^{2\alpha }+2{\omega }^2{\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}\right),$$

(20)

which agrees with Ref. [26].

Proof. 

The proofs follow immediately by setting $a_j=0$$\forall j=1,2,3,\cdots ,n$ into Equation (18). Furthermore, the particular solution at $b_j=1$$\forall j=1,2,3,\cdots ,n$ becomes:

$$y_p\left(t\right)=\sum _{j=1}^n\frac{{\omega }^{2}sin\left({\mathsf{\Omega }}_{j}t\right)+{\mathsf{\Omega }}_j^{\alpha }sin\left({\mathsf{\Omega }}_{j}t-\frac{\pi \alpha }{2}\right)}{{\omega }^4+{\mathsf{\Omega }}_j^{2\alpha }+2{\omega }^2{\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}.$$

(21)

□

Lemma 2. 

The FODE

$${}_{-\infty }^{RL}{D}_t^{\alpha }y\left(t\right)+{\omega }^{2}y\left(t\right)=\sum _{j=1}^n{a}_{j}cos\left({\mathsf{\Omega }}_{j}t\right),0<\alpha \le 1,$$

(22)

has the particular solution:

$$y_p\left(t\right)=\sum _{j=1}^n{a}_j\left(\frac{{\omega }^{2}cos\left({\mathsf{\Omega }}_{j}t\right)+{\mathsf{\Omega }}_j^{\alpha }cos\left({\mathsf{\Omega }}_{j}t-\frac{\pi \alpha }{2}\right)}{{\omega }^4+{\mathsf{\Omega }}_j^{2\alpha }+2{\omega }^2{\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}\right).$$

(23)

Proof. 

The proofs follow immediately by setting $b_j=0$$\forall j=1,2,3,\cdots ,n$ into Equation (18). In addition, the particular solution at $a_1=1$ and $a_j=0$$\forall j=2,3,\cdots ,n$ becomes

$$y_p\left(t\right)=\frac{{\omega }^{2}cos\left(\mathsf{\Omega }t\right)+{\mathsf{\Omega }}^{\alpha }cos\left(\mathsf{\Omega }t-\frac{\pi \alpha }{2}\right)}{{\omega }^4+{\mathsf{\Omega }}^{2\alpha }+2{\omega }^2{\mathsf{\Omega }}^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)},$$

(24)

where ${\mathsf{\Omega }}_1=\mathsf{\Omega }$ is assumed for simplicity. This particular solution agrees with the corresponding one in Ref. [27], Equation (7) using an operator approach for a single term in the right hand side of Equation (22), i.e.,

$${}_{-\infty }^{RL}{D}_t^{\alpha }y\left(t\right)+{\omega }^{2}y\left(t\right)=cos\left(\mathsf{\Omega }t\right).$$

(25)

□

3. The Analytic Solution

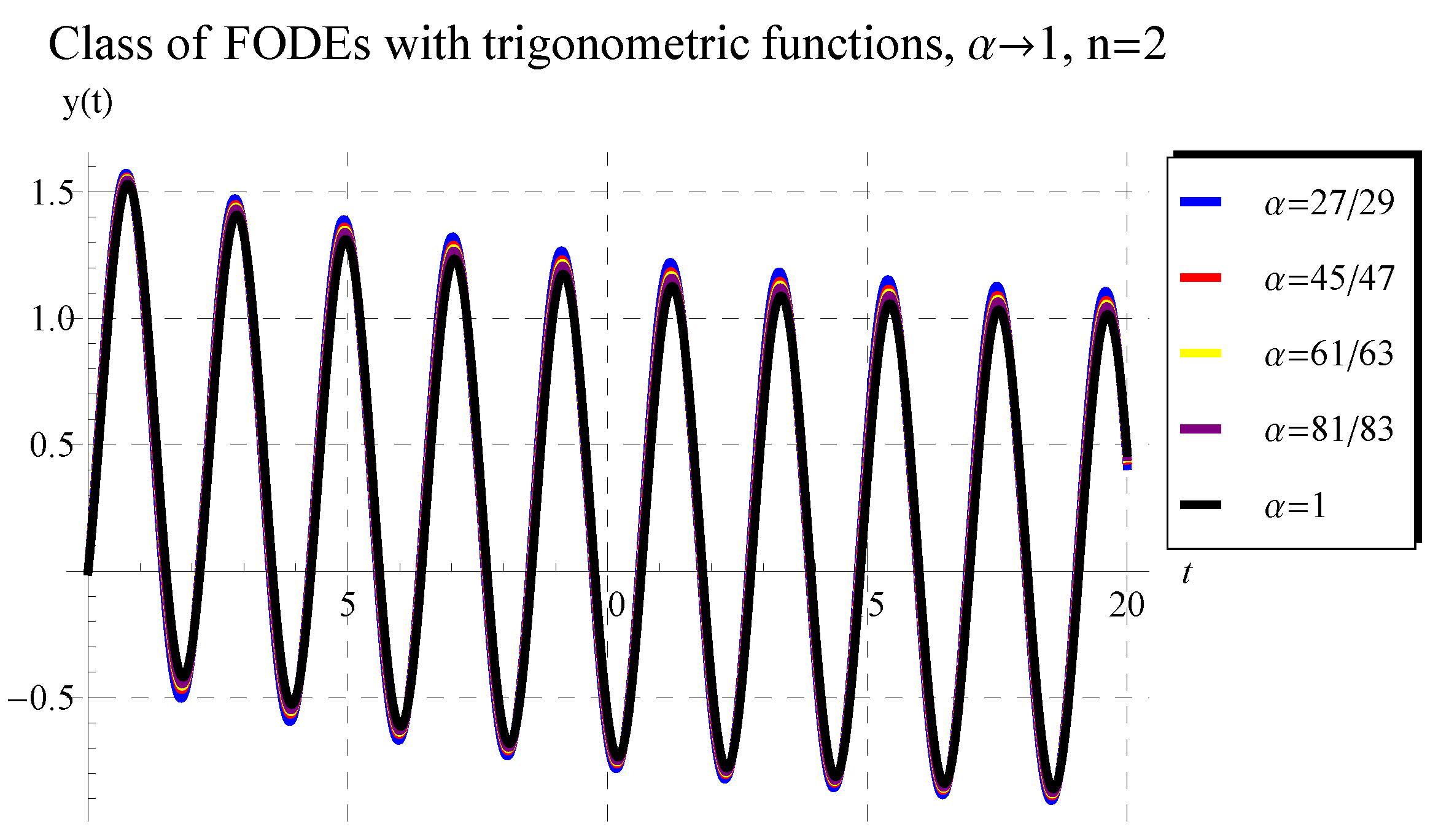
3.1. For Fractional Derivative ($0<\alpha <1$)

According to Section 2, the general solution of Equation (1) is:

$$y\left(t\right)=c{e}^{i\delta t}+\sum _{j=1}^n\left({\rho }_{1j}cos\left(\mathsf{\Omega }t\right)+{\rho }_{2j}sin\left(\mathsf{\Omega }t\right)\right).$$

(26)

At $t=0$, we find that:

$$y\left(0\right)=c+\sum _{j=1}^n{\rho }_{1j}.$$

(27)

Applying the initial condition $y\left(0\right)=A$, then:

$$c=A-\sum _{j=1}^n{\rho }_{1j}.$$

(28)

Therefore:

$$y\left(t\right)=\left(A-\sum _{j=1}^n{\rho }_{1j}\right)e^{{\left(-{\omega }^2\right)}^{\frac{1}{\alpha }}t}+\sum _{j=1}^n\left({\rho }_{1j}cos\left(\mathsf{\Omega }t\right)+{\rho }_{2j}sin\left(\mathsf{\Omega }t\right)\right).$$

(29)

Inserting ${\rho }_{1j}$ and ${\rho }_{2j}$, we obtain the general solution in the form

$$\begin{array}{ccc}\hfill y\left(t\right)& =& \left(A-\sum _{j=1}^n\frac{a_j{\omega }^2+{\mathsf{\Omega }}_j^{\alpha }\left(a_{j}cos\left(\frac{\pi \alpha }{2}\right)-b_{j}sin\left(\frac{\pi \alpha }{2}\right)\right)}{{\omega }^4+{\mathsf{\Omega }}_j^{2\alpha }+2{\omega }^2{\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}\right)e^{{\left(-{\omega }^2\right)}^{\frac{1}{\alpha }}t}+\hfill \\ & & \sum _{j=1}^n\left(\frac{a_j{\omega }^2+{\mathsf{\Omega }}_j^{\alpha }\left(a_{j}cos\left(\frac{\pi \alpha }{2}\right)-b_{j}sin\left(\frac{\pi \alpha }{2}\right)\right)}{{\omega }^4+{\mathsf{\Omega }}_j^{2\alpha }+2{\omega }^2{\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}\right)cos\left({\mathsf{\Omega }}_{j}t\right)+\hfill \\ & & \sum _{j=1}^n\left(\frac{b_j{\omega }^2+{\mathsf{\Omega }}_j^{\alpha }\left(b_{j}cos\left(\frac{\pi \alpha }{2}\right)+a_{j}sin\left(\frac{\pi \alpha }{2}\right)\right)}{{\omega }^4+{\mathsf{\Omega }}_j^{2\alpha }+2{\omega }^2{\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}\right)sin\left({\mathsf{\Omega }}_{j}t\right),\hfill \end{array}$$

(30)

which can be put in the compact form:

$$\begin{array}{ccc}\hfill y\left(t\right)& =& \left(A-\sum _{j=1}^n\frac{a_j{\omega }^2+{\mathsf{\Omega }}_j^{\alpha }\left(a_{j}cos\left(\frac{\pi \alpha }{2}\right)-b_{j}sin\left(\frac{\pi \alpha }{2}\right)\right)}{{\omega }^4+{\mathsf{\Omega }}_j^{2\alpha }+2{\omega }^2{\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}\right)e^{{\left(-{\omega }^2\right)}^{\frac{1}{\alpha }}t}+\hfill \\ & & \sum _{j=1}^n\frac{{\omega }^2\left[a_{j}cos\left({\mathsf{\Omega }}_{j}t\right)+b_{j}sin\left({\mathsf{\Omega }}_{j}t\right)\right]+{\mathsf{\Omega }}_j^{\alpha }\left[a_{j}cos\left({\mathsf{\Omega }}_{j}t-\frac{\pi \alpha }{2}\right)+b_{j}sin\left({\mathsf{\Omega }}_{j}t-\frac{\pi \alpha }{2}\right)\right]}{{\omega }^4+{\mathsf{\Omega }}_j^{2\alpha }+2{\omega }^2{\mathsf{\Omega }}_j^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}.\hfill \end{array}$$

(31)

It is clear from Equation (31) that the IC $y\left(0\right)=A$ is satisfied. Although the solution (31) is analytic in the whole domain $t\in \mathbb{R},\forall \alpha \in (0,1]$, it is real at specific/certain values of $\alpha$ as will be shown in a subsequent section.

3.2. For Ordinary Derivative ($\alpha \to 1$)

As $\alpha \to 1$, the solution in Equation (29) becomes

$$y\left(t\right)=\left(A-\sum _{j=1}^n{\left[{\rho }_{1j}\right]}_{\alpha \to 1}\right)e^{-{\omega }^{2}t}+\sum _{j=1}^n\left({\left[{\rho }_{1j}\right]}_{\alpha \to 1}cos\left({\mathsf{\Omega }}_{j}t\right)+{\left[{\rho }_{2j}\right]}_{\alpha \to 1}sin\left({\mathsf{\Omega }}_{j}t\right)\right).$$

(32)

where:

$${\left[{\rho }_1\right]}_{\alpha \to 1}=\sum _{j=1}^n\frac{a_j{\omega }^2-b_j{\mathsf{\Omega }}_j}{{\omega }^4+{\mathsf{\Omega }}_j^2},{\left[{\rho }_2\right]}_{\alpha \to 1}=\sum _{j=1}^n\frac{b_j{\omega }^2+a_j{\mathsf{\Omega }}_j}{{\omega }^4+{\mathsf{\Omega }}_j^2}.$$

(33)

Substituting (33) into (32) we get:

$$y\left(t\right)=\left(A-\sum _{j=1}^n\frac{a_j{\omega }^2-b_j{\mathsf{\Omega }}_j}{{\omega }^4+{\mathsf{\Omega }}_j^2}\right)e^{-{\omega }^{2}t}+\sum _{j=1}^n\left[\left(\frac{a_j{\omega }^2-b_j{\mathsf{\Omega }}_j}{{\omega }^4+{\mathsf{\Omega }}_j^2}\right)cos\left({\mathsf{\Omega }}_{j}t\right)+\left(\frac{b_j{\omega }^2+a_j{\mathsf{\Omega }}_j}{{\omega }^4+{\mathsf{\Omega }}_j^2}\right)sin\left({\mathsf{\Omega }}_{j}t\right)\right].$$

(34)

The solution (34) satisfies the class of ODEs:

$$y^{\prime }\left(t\right)+{\omega }^{2}y\left(t\right)=\sum _{j=1}^n(a_{j}cos\left({\mathsf{\Omega }}_{j}t\right)+b_{j}sin\left({\mathsf{\Omega }}_{j}t\right)),y\left(0\right)=A.$$

(35)

The analytic solutions of some special classes of the FODEs (1) and the ODEs (35) are obtained in the next sections.

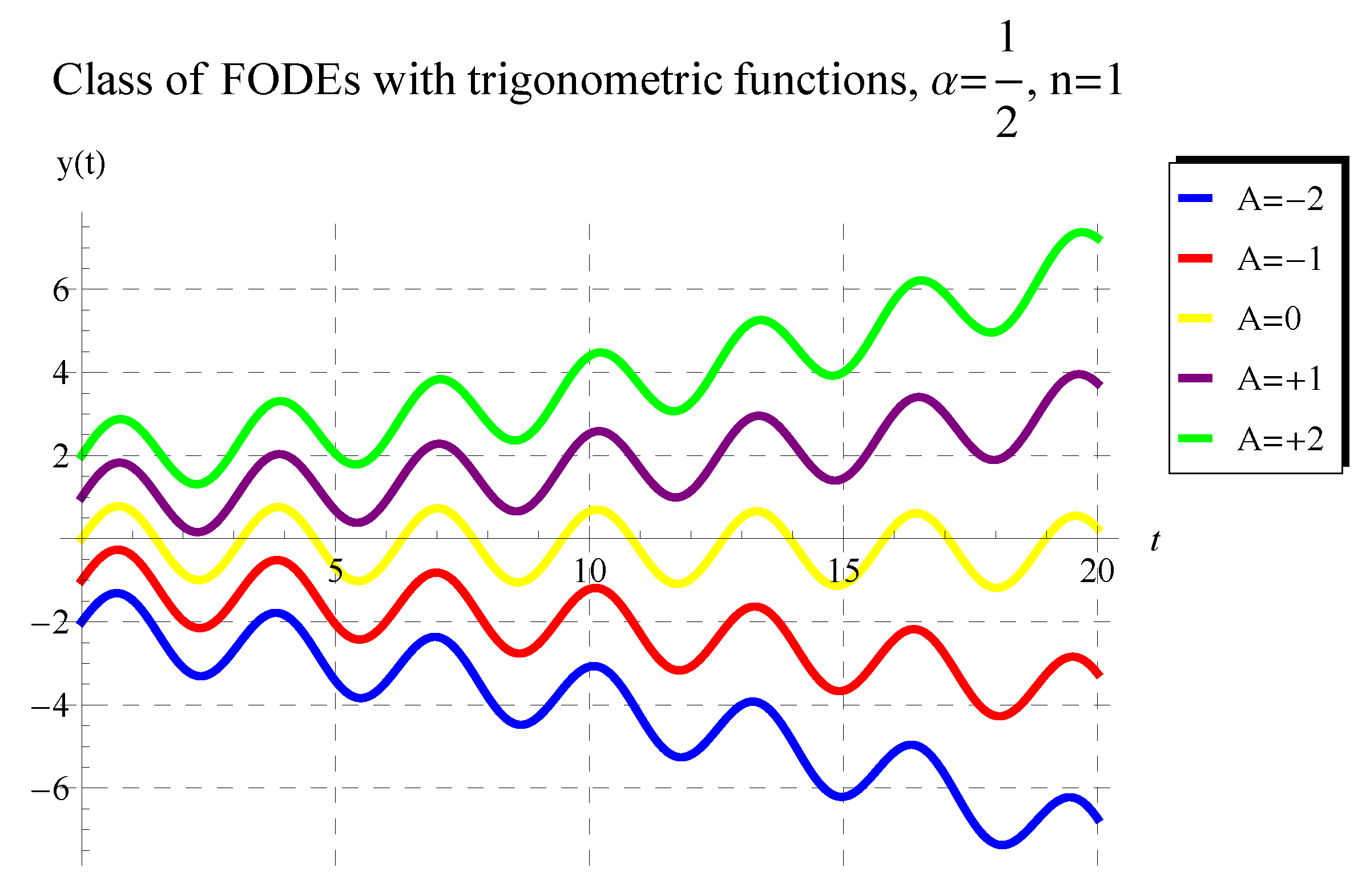
4. Analytic Solution of Special Classes of FODEs

For ${\mathsf{\Omega }}_j=i{\mu }_j$ and $b_j=i{a}_j$, the right hand side of Equation (1) becomes

$$\begin{array}{ccc}\hfill \sum _{j=1}^n(a_{j}cos\left({\mathsf{\Omega }}_{j}t\right)+b_{j}sin\left({\mathsf{\Omega }}_{j}t\right))& =& \sum _{j=1}^n(a_{j}cos\left(i{\mu }_{j}t\right)+i{a}_{j}sin\left(i{\mu }_{j}t\right)),\hfill \\ & =& \sum _{j=1}^n{a}_j(cosh\left({\mu }_{j}t\right)-sinh\left({\mu }_{j}t\right)),\hfill \\ & =& \sum _{j=1}^n{a}_j{e}^{-{\mu }_{j}t},\hfill \end{array}$$

(36)

and, hence, we find the following class of FODEs in terms of exponential functions

$${}_{-\infty }^{RL}{D}_t^{\alpha }y\left(t\right)+{\omega }^{2}y\left(t\right)=\sum _{j=1}^n{a}_j{e}^{-{\mu }_{j}t},y\left(0\right)=A,0<\alpha \le 1,$$

(37)

and in this case we can write the solution (31) as:

$$\begin{array}{ccc}\hfill y\left(t\right)& =& \left(A-\sum _{j=1}^n\frac{a_j{\omega }^2+i^{\alpha }{\mu }_j^{\alpha }{a}_j\left(cos\left(\frac{\pi \alpha }{2}\right)-isin\left(\frac{\pi \alpha }{2}\right)\right)}{{\omega }^4+{\left(i{\mu }_j\right)}^{2\alpha }+2{\omega }^2{\left(i{\mu }_j\right)}^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}\right)e^{{\left(-{\omega }^2\right)}^{\frac{1}{\alpha }}t}+\hfill \\ & & \sum _{j=1}^n\frac{{\omega }^2{a}_j\left[cos\left(i{\mu }_{j}t\right)+isin\left(i{\mu }_{j}t\right)\right]+{\left(i{\mu }_j\right)}^{\alpha }{a}_j\left[cos\left(i{\mu }_{j}t-\frac{\pi \alpha }{2}\right)+isin\left(i{\mu }_{j}t-\frac{\pi \alpha }{2}\right)\right]}{{\omega }^4+{\left(i{\mu }_j\right)}^{2\alpha }+2{\omega }^2{\left(i{\mu }_j\right)}^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}.\hfill \end{array}$$

(38)

Implementing the identities $i^{\alpha }={\left(e^{i\pi /2}\right)}^{\alpha }=e^{i\pi \alpha /2}$ and $cos\left(\frac{\pi \alpha }{2}\right)-isin\left(\frac{\pi \alpha }{2}\right)=e^{-i\pi \alpha /2}$, we find that

$$\begin{array}{ccc}& & i^{\alpha }\left(cos\left(\frac{\pi \alpha }{2}\right)-isin\left(\frac{\pi \alpha }{2}\right)\right)=1,\hfill \end{array}$$

(39)

$$\begin{array}{ccc}& & cos\left(i{\mu }_{j}t\right)+isin\left(i{\mu }_{j}t\right)=cosh\left({\mu }_{j}t\right)-sinh\left({\mu }_{j}t\right)=e^{-{\mu }_{j}t},\hfill \end{array}$$

(40)

$$\begin{array}{ccc}& & cos\left(i{\mu }_{j}t-\frac{\pi \alpha }{2}\right)+isin\left(i{\mu }_{j}t-\frac{\pi \alpha }{2}\right)=e^{i\left(i{\mu }_{j}t-\frac{\pi \alpha }{2}\right)}=e^{-{\mu }_{j}t}.e^{-i\frac{\pi \alpha }{2}}=i^{-\alpha }{e}^{-{\mu }_{j}t},\hfill \end{array}$$

(41)

$$\begin{array}{ccc}& & i^{\alpha }\left[cos\left(i{\mu }_{j}t-\frac{\pi \alpha }{2}\right)+isin\left(i{\mu }_{j}t-\frac{\pi \alpha }{2}\right)\right]=e^{-{\mu }_{j}t}.\hfill \end{array}$$

(42)

Accordingly, the solution (38) becomes:

$$y\left(t\right)=\left(A-\sum _{j=1}^n\frac{a_j({\omega }^2+{\mu }_j^{\alpha })}{{\omega }^4+{\left(i{\mu }_j\right)}^{2\alpha }+2{\omega }^2{\left(i{\mu }_j\right)}^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}\right)e^{{\left(-{\omega }^2\right)}^{\frac{1}{\alpha }}t}+\sum _{j=1}^n\frac{a_j({\omega }^2+{\mu }_j^{\alpha })e^{-{\mu }_{j}t}}{{\omega }^4+{\left(i{\mu }_j\right)}^{2\alpha }+2{\omega }^2{\left(i{\mu }_j\right)}^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}.$$

(43)

Also, it is noticed that for ${\mathsf{\Omega }}_j=i{\mu }_j$ and $b_j=-i{a}_j$, the right hand side of Equation (1) becomes

$$\begin{array}{ccc}\hfill \sum _{j=1}^n(a_{j}cos\left({\mathsf{\Omega }}_{j}t\right)+b_{j}sin\left({\mathsf{\Omega }}_{j}t\right))& =& \sum _{j=1}^n(a_{j}cos\left(i{\mu }_{j}t\right)-i{a}_{j}sin\left(i{\mu }_{j}t\right)),\hfill \\ & =& \sum _{j=1}^n{a}_j(cosh\left({\mu }_{j}t\right)+sinh\left({\mu }_{j}t\right)),\hfill \\ & =& \sum _{j=1}^n{a}_j{e}^{{\mu }_{j}t},\hfill \end{array}$$

(44)

which leads to the class of FODEs:

$${}_{-\infty }^{RL}{D}_t^{\alpha }y\left(t\right)+{\omega }^{2}y\left(t\right)=\sum _{j=1}^n{a}_j{e}^{{\mu }_{j}t},y\left(0\right)=A,0<\alpha \le 1,$$

(45)

The solution of (45) can be obtained similarly as above, which yields:

$$\begin{array}{ccc}\hfill y\left(t\right)& =& \left(A-\sum _{j=1}^n\frac{a_j({\omega }^2+{(-{\mu }_j)}^{\alpha })}{{\omega }^4+{(-i{\mu }_j)}^{2\alpha }+2{\omega }^2{(-i{\mu }_j)}^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}\right)e^{{\left(-{\omega }^2\right)}^{\frac{1}{\alpha }}t}+\hfill \\ & & \sum _{j=1}^n\frac{a_j({\omega }^2+{(-{\mu }_j)}^{\alpha })e^{{\mu }_{j}t}}{{\omega }^4+{(-i{\mu }_j)}^{2\alpha }+2{\omega }^2{(-i{\mu }_j)}^{\alpha }cos\left(\frac{\pi \alpha }{2}\right)}.\hfill \end{array}$$

(46)

Note that this last solution can be directly obtained from (43) through replacing ${\mu }_j$ by $-{\mu }_j$.

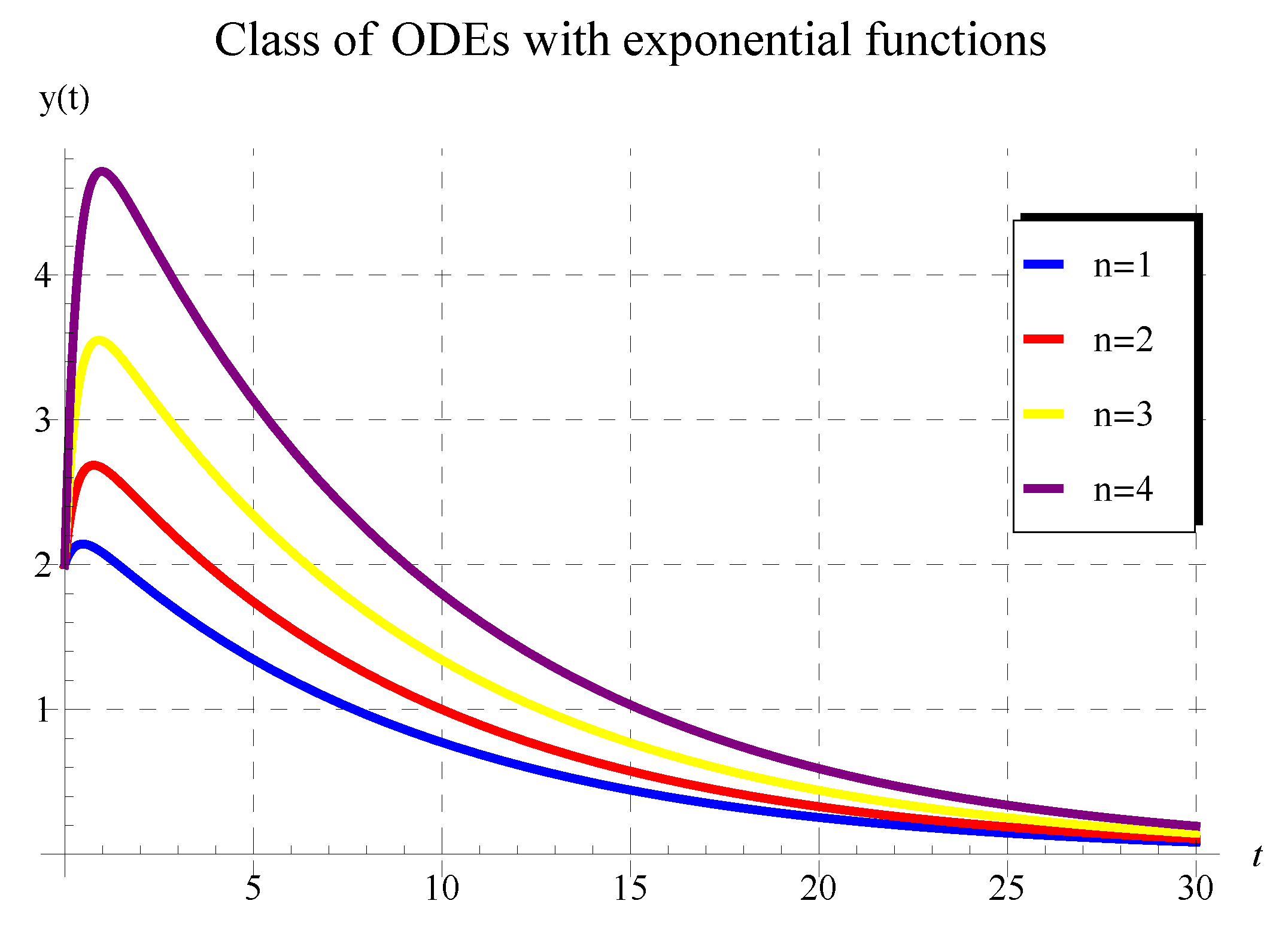
5. Analytic Solutions of Other Classes of ODEs as Special Cases

In this section, we show that the analytic solutions of other classes, as $\alpha \to 1$, can be obtained as special cases. Consider the following class of ODEs in terms of hyperbolic functions:

$$y^{\prime }\left(t\right)+{\omega }^{2}y\left(t\right)=\sum _{j=1}^n(a_{j}cosh\left({\sigma }_{j}t\right)+d_{j}sinh\left({\sigma }_{j}t\right)),y\left(0\right)=A.$$

(47)

It is obvious that this class is a special case of our class in Equation (1) when ${\mathsf{\Omega }}_j=i{\sigma }_j$ and $b_j=-i{d}_j$, where $i=\sqrt{-1}$. In order to obtain the analytic solution of class (47) we just make substitutions of ${\mathsf{\Omega }}_j=i{\sigma }_j$ and $b_j=-i{d}_j$ into the generalized solution (31). In this case, we have:

$$y\left(t\right)=\left(A-\sum _{j=1}^n\frac{a_j{\omega }^2-d_j{\sigma }_j}{{\omega }^4-{\sigma }_j^2}\right)e^{-{\omega }^{2}t}+\sum _{j=1}^n\left[\left(\frac{a_j{\omega }^2-d_j{\sigma }_j}{{\omega }^4-{\sigma }_j^2}\right)cos\left(i{\sigma }_{j}t\right)+\left(\frac{-i{d}_j{\omega }^2+i{a}_j{\sigma }_j}{{\omega }^4-{\sigma }_j^2}\right)sin\left(i{\sigma }_{j}t\right)\right].$$

(48)

Making use of the identities $cos\left(i{\sigma }_{j}t\right)=cosh\left({\sigma }_{j}t\right)$ and $sin\left(i{\sigma }_{j}t\right)=isinh\left({\sigma }_{j}t\right)$, then:

$$y\left(t\right)=\left(A-\sum _{j=1}^n\frac{a_j{\omega }^2-d_j{\sigma }_j}{{\omega }^4-{\sigma }_j^2}\right)e^{-{\omega }^{2}t}+\sum _{j=1}^n\left[\left(\frac{a_j{\omega }^2-d_j{\sigma }_j}{{\omega }^4-{\sigma }_j^2}\right)cosh\left({\sigma }_{j}t\right)+\left(\frac{d_j{\omega }^2-a_j{\sigma }_j}{{\omega }^4-{\sigma }_j^2}\right)sinh\left({\sigma }_{j}t\right)\right].$$

(49)

This solution is real provided that $a_j,d_j,{\sigma }_j\in \mathbb{R}$ and ${\sigma }_j\ne {\omega }^2$$\forall j=1,2,3,\cdots ,n$.

Similarly, for ${\mathsf{\Omega }}_j=i{\mu }_j$ and $b_j=i{a}_j$ we find the following class of ODEs in terms of exponential functions:

$$y^{\prime }\left(t\right)+{\omega }^{2}y\left(t\right)=\sum _{j=1}^n{a}_j{e}^{-{\mu }_{j}t},y\left(0\right)=A.$$

(50)

Accordingly, the solution of this class is

$$y\left(t\right)=\left(A-\sum _{j=1}^n\frac{a_j({\omega }^2+{\mu }_j)}{{\omega }^4-{\mu }_j^2}\right)e^{-{\omega }^{2}t}+\sum _{j=1}^n\left[\left(\frac{a_j({\omega }^2+{\mu }_j)}{{\omega }^4-{\mu }_j^2}\right)cos\left(i{\mu }_{j}t\right)+\left(\frac{i{a}_j({\omega }^2+{\mu }_j)}{{\omega }^4-{\mu }_j^2}\right)sin\left(i{\mu }_{j}t\right)\right],$$

(51)

which can be simplified to

$$y\left(t\right)=\left(A-\sum _{j=1}^n\frac{a_j}{{\omega }^2-{\mu }_j}\right)e^{-{\omega }^{2}t}+\sum _{j=1}^n\left[\left(\frac{a_j}{{\omega }^2-{\mu }_j}\right)cosh\left({\mu }_{j}t\right)-\left(\frac{a_j}{{\omega }^2-{\mu }_j}\right)sinh\left({\mu }_{j}t\right)\right],$$

(52)

i.e.,

$$y\left(t\right)=\left(A-\sum _{j=1}^n\frac{a_j}{{\omega }^2-{\mu }_j}\right)e^{-{\omega }^{2}t}+\sum _{j=1}^n\left(\frac{a_j}{{\omega }^2-{\mu }_j}\right)e^{-{\mu }_{j}t},{\mu }_j\ne {\omega }^2\forall j=1,2,3,\cdots ,n.$$

(53)

Also, for the class

$$y^{\prime }\left(t\right)+{\omega }^{2}y\left(t\right)=\sum _{j=1}^n{a}_j{e}^{{\mu }_{j}t},y\left(0\right)=A,$$

(54)

we have the solution:

$$y\left(t\right)=\left(A-\sum _{j=1}^n\frac{a_j}{{\omega }^2+{\mu }_j}\right)e^{-{\omega }^{2}t}+\sum _{j=1}^n\left(\frac{a_j}{{\omega }^2+{\mu }_j}\right)e^{{\mu }_{j}t},{\mu }_j+{\omega }^2\ne 0\forall j=1,2,3,\cdots ,n.$$

(55)

The solutions (53) and (55) can also be determined directly by letting $\alpha \to 1$ in (43) and (46), respectively.

6. Behavior of Solution

The solution given by Equation (31) is real when the quantity ${\left(-{\omega }^2\right)}^{\frac{1}{\alpha }}\in \mathbb{R}$, i.e., if ${(-1)}^{\frac{1}{\alpha }}=ϵ\left(\mathrm{say}\right)\in \mathbb{R}$. In Ref. [28], the authors introduced the next theorem which determines the values of $\alpha$ so that $ϵ\in \mathbb{R}$.

Theorem 2 

([28]). Values of α for real ϵ. For $n,k\in {\mathbb{N}}^{+}$, $y\left(t\right)\in \mathbb{R}$ when $\alpha =\frac{2n-1}{2(k+n-1)}$ ($ϵ=1$) and $\alpha =\frac{2n-1}{2(k+n)-1}$ ($ϵ=-1$).

Based on Theorem 2, the solution (31) is displayed in Figure 1 for $\alpha =\frac{1}{2}$ at different values of n. In addition, Figure 2 and Figure 3 show the impact of the initial condition A on the behavior of the solution at $n=1$ and $n=3$, respectively. Furthermore, the effect of the fractional order $\alpha$ on the oscillations is indicated in Figure 4 when $n=4$. Moreover, as $\alpha \to 1$ the present fractional solution is identical to the ordinary one as can be observed from Figure 5. The periodicity/oscillatory of the solution appear in Figure 1, Figure 2, Figure 3, Figure 4 and Figure 5. Finally, the solutions (49) and (53) for the classes of ODEs (47) and (50) are depicted in Figure 6 and Figure 7, respectively, at different values of $n=1,2,3,4$. In these figures, oscillations of the solutions (49) and (53) are not observed, i.e., unlike the fractional case.

7. Conclusions

A generalized class of first-order fractional ordinary differential equations was solved in this paper by means of Riemann–Liouville fractional derivative (RLFD). The non-homogeneous fractional differential systems containing $2n$ periodic terms were solved utilizing an efficient approach in terms of entire functions with fractional order arguments. The obtained results generalized previous results in the literature. The main features of the obtained solutions are interpreted and analyzed. The present analysis can be further extended in the near future to include similar models of higher orders.