Optimal Fair-Workload Scheduling: A Case Study at Glorytek
Abstract
:1. Introduction
2. Literature Review
3. Problem Description and Model Construction
3.1. Problem Description
- Staff scheduling is time-consuming.
- The scheduler must manually check to ensure a 12 h interval between a shift and the following shift, which often fails to be fully carried out. Hence, when consecutive shifts occur, workers must be temporarily transferred to another shift.
- If an employee joins or leaves during the month, the scheduler must manually adjust the shifts again, which leads to a lack of timeliness in scheduling.
- Shifts are not averaged fairly: some workers have a greater number of shifts than others in a given month.
3.2. Model Constructions
- Manpower of assembly line engineers: The total number of personnel for the assembly line is 17, including 2 section managers and 15 engineers. All personnel are equipped with the necessary professional knowledge to adjust the parameters of the assembly machine and troubleshoot anomalies and have passed the pre-service training provided by the unit. Therefore, all engineers are fit to perform their duties.
- All personnel can meet the shift demands of all assembly machines in the production line. There is no problem with matching machines and engineering staff.
- In terms of scheduling, the engineering staff are on call during weekday night shifts (16:00 p.m.–12:00 a.m.) and weekday graveyard shifts (12:00 a.m.–8:00 a.m.) because the normal working hours for all engineers are 8:00 a.m.–5:00 p.m. Therefore, after working hours, there are only two shifts on weekdays. However, on weekends, three shifts are required: holiday day shifts (8:00 a.m.–16:00 p.m.), holiday night shifts (16:00 p.m.–12:00 a.m.), and holiday graveyard shifts (12:00 a.m.–8:00 a.m.). They must stay on call at all times. When notified by the production line, the staff must arrive at the site within one hour to troubleshoot the problems. To protect employees’ physical and mental health and balance their labor, no employees are allowed to work two consecutive shifts in a day, and a 12 h interval should separate two shifts.
- Taking into account employees’ living conditions of rest and labor balance, the scheduling condition that employees shall not be on call for two consecutive weeks during holidays shall be met, given sufficient manpower for shifting.
- This study model does not consider personnel salary costs. In the future, if each unit considers labor costs, this can be included in the input cost factor of the model. The shift allocation and adjustment for scheduling can be carried out according to the model’s output.
- At the same time, the scheduling model established in this study is based on the assumption that all staff members are available and present. Special leave, sick leave, and so on are unanticipated conditions and are thus not included in the scope of this study.
3.3. Mathematical Model
- Parameter settings
| P | Number of employees | (P = 17) |
| D | Number of days in a month | (D = 30) |
| Cj | The set of all shift numbers for each day | |
| Z | Maximum number of attendances per month | |
| k | Shift index k (Morning shift: 1/night shift: 2/graveyard shift: 3) | k = 1, 2, 3 |
| xijk | i-th worker on date j and k shift on call or not | xijk = 1 or 0 |
| i | i-th worker on call | i = 1, …, 17 |
| j | Day j of each month | j = 1, …, 30 |
- Objective Function
- Constraints:
3.4. Model Description
3.5. Solution
4. Case Validation
4.1. Real Case Example
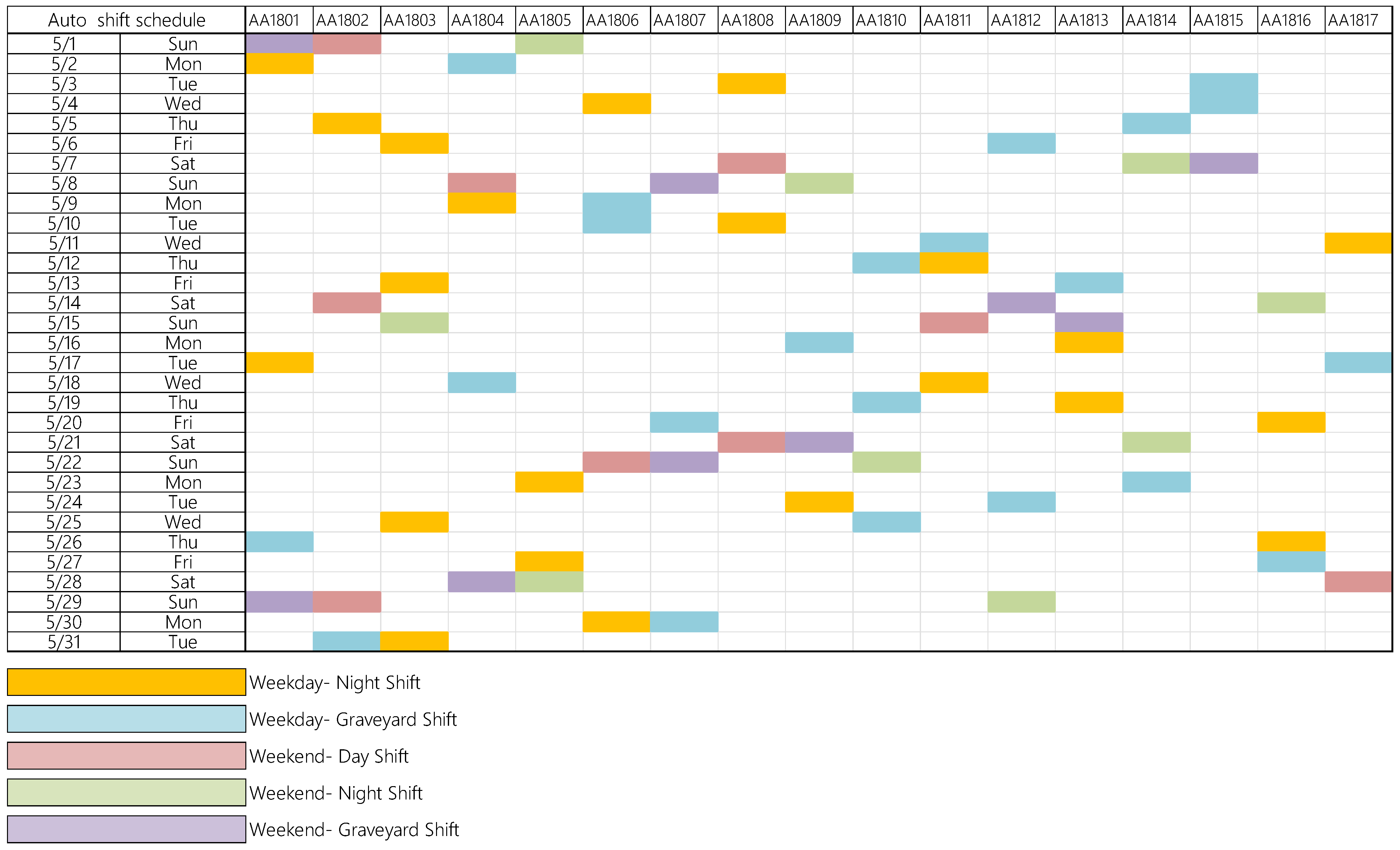
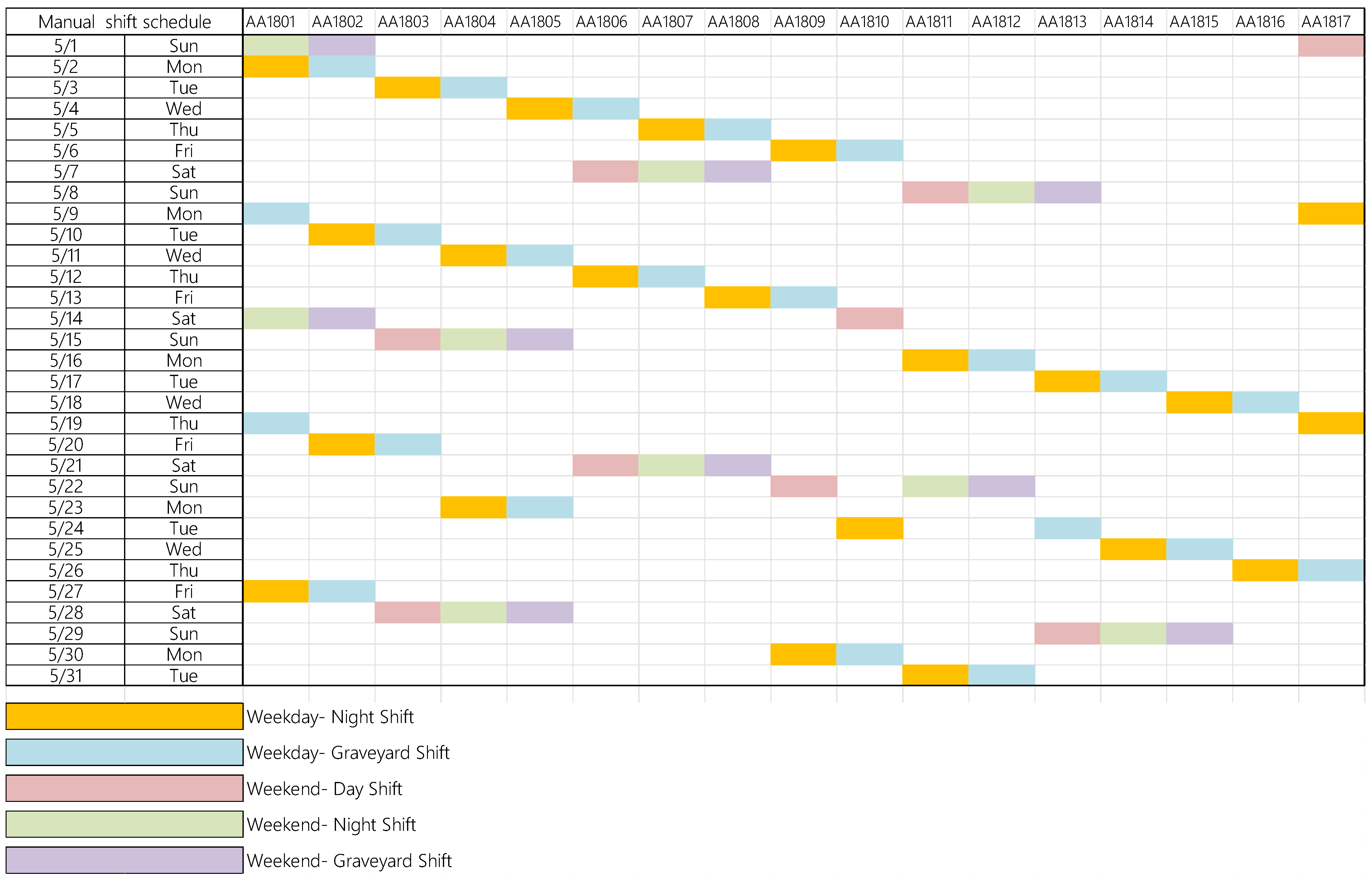
4.1.1. Model Outcome
4.1.2. Comparison of Results and Benefits of Automatic Scheduling and Manual Assignment
- Duty Allocation
- Scheduling Efficiency
- Achievement of Shift Restrictions
- Fairness
- Rotation Flexibility
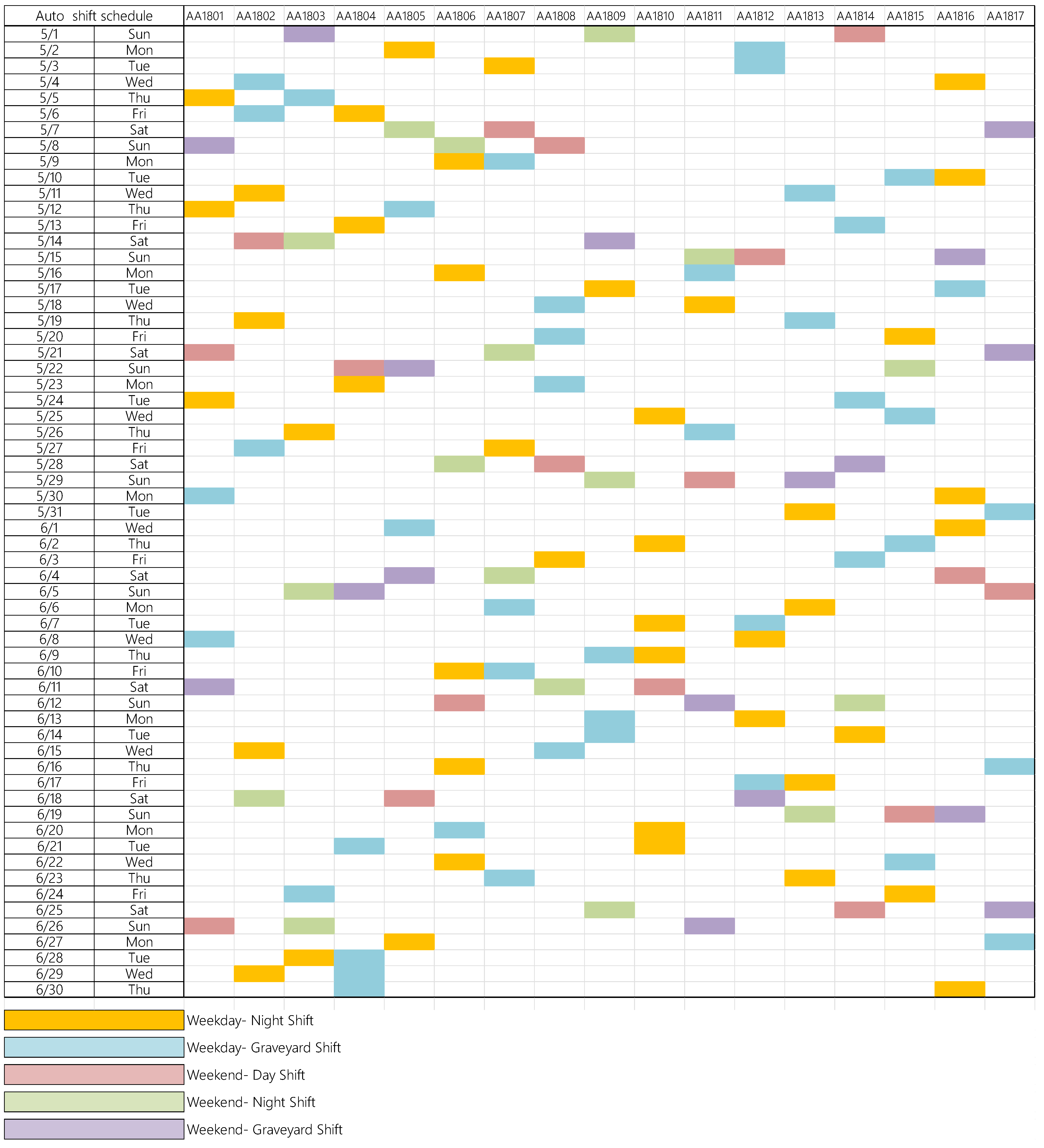
4.2. Large-Size Problem Scenarios
4.2.1. Schedule for Two Consecutive Months
4.2.2. Schedule for Increasing the Number of Engineers
4.2.3. Model Outcome
5. Conclusions and Recommendations
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Edie, L.C. Traffic delays at toll booths. J. Oper. Res. Soc. Am. 1954, 2, 107–138. [Google Scholar] [CrossRef]
- Dantzig, G.B. A comment on Edie’s “Traffic delays at toll booths”. J. Oper. Res. Soc. Am. 1954, 2, 339–341. [Google Scholar]
- Baker, K.R. Workforce allocation in cyclical scheduling problems: A survey. J. Oper. Res. Soc. 1976, 27, 155–167. [Google Scholar] [CrossRef]
- Ernst, A.T.; Jiang, H.; Krishnamoorthy, M.; Sier, D. Staff scheduling and rostering: A review of applications, methods and models. Eur. J. Oper. Res. 2004, 153, 3–27. [Google Scholar] [CrossRef]
- Alfares, H.K. Survey, categorization, and comparison of recent tour scheduling literature. Ann. Oper. Res. 2004, 127, 145–175. [Google Scholar] [CrossRef]
- Stolletz, R.; Brunner, J.O. Fair optimization of fortnightly physician schedules with flexible shifts. Eur. J. Oper. Res. 2012, 219, 622–629. [Google Scholar] [CrossRef]
- Uhde, A.; Schlicker, N.; Wallach, D.P.; Hassenzahl, M. Fairness and decision-making in collaborative shift scheduling systems. In Proceedings of the 2020 CHI Conference on Human Factors in Computing Systems (CHI’20), Honolulu, HI, USA, 25–30 April 2020; Association for Computing Machinery: New York, NY, USA, 2020; pp. 1–13. [Google Scholar]
- Howard, F.M.; Gao, C.A.; Sankey, C. Implementation of an automated scheduling tool improves schedule quality and resident satisfaction. PLoS ONE 2020, 15, e0236952. [Google Scholar] [CrossRef] [PubMed]
- Topaloglu, S. A multi-objective programming model for scheduling emergency medicine residents. Comput. Ind. Eng. 2006, 51, 375–388. [Google Scholar] [CrossRef]
- Lee, J.; Jeong, I.S. Compliance with recommendations on work schedule for shift nurses in South Korea. Saf. Health Work 2021, 12, 255–260. [Google Scholar] [CrossRef] [PubMed]
- Becker, T.; Steenweg, P.M.; Werners, B. Cyclic shift scheduling with on-call duties for emergency medical services. Health Care Manag. Sci. 2019, 22, 676–690. [Google Scholar] [CrossRef] [PubMed]
- Härmä, M. Circadian Adaptation to Shift Work. A Review. In Shiftwork in the 21st Century; Peter Lang: Berlin, Germany, 2000; pp. 125–130. [Google Scholar]
- Jansen, D.; Nachreiner, F. Differential psychological effects of different shift systems. A comparison of the effects of shift work under different systems in the chemical industry. Shift Int. News 2001, 18, 10. [Google Scholar]
- Kundi, M.; Wöckinger, G. Psychological Aspects of Flexible Shift Work Arrangements in Hospital Nurses. In Shift Work in the 21st Century; Peter Lang: Frankfurt, Germany, 2000; pp. 393–398. [Google Scholar]
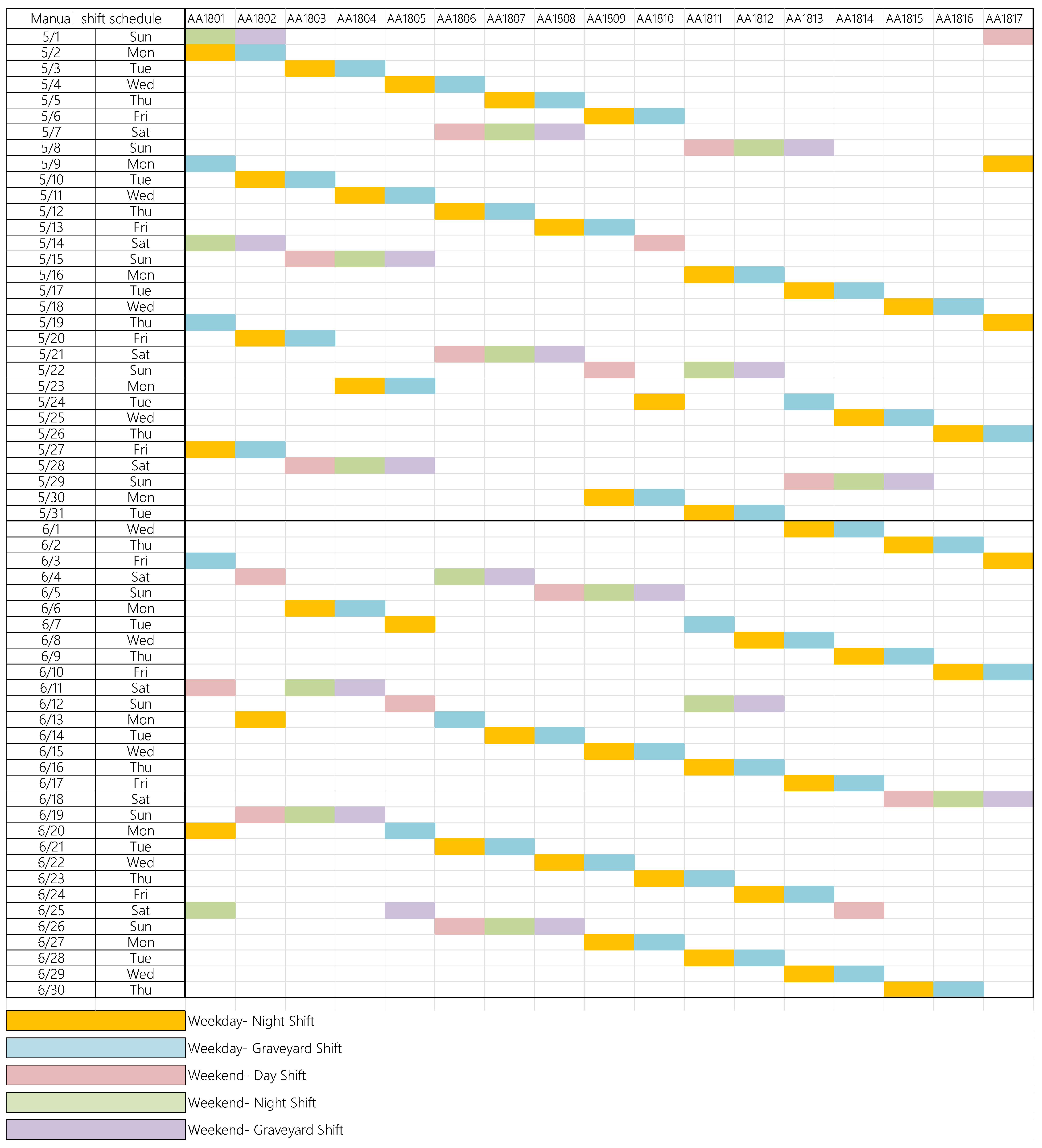
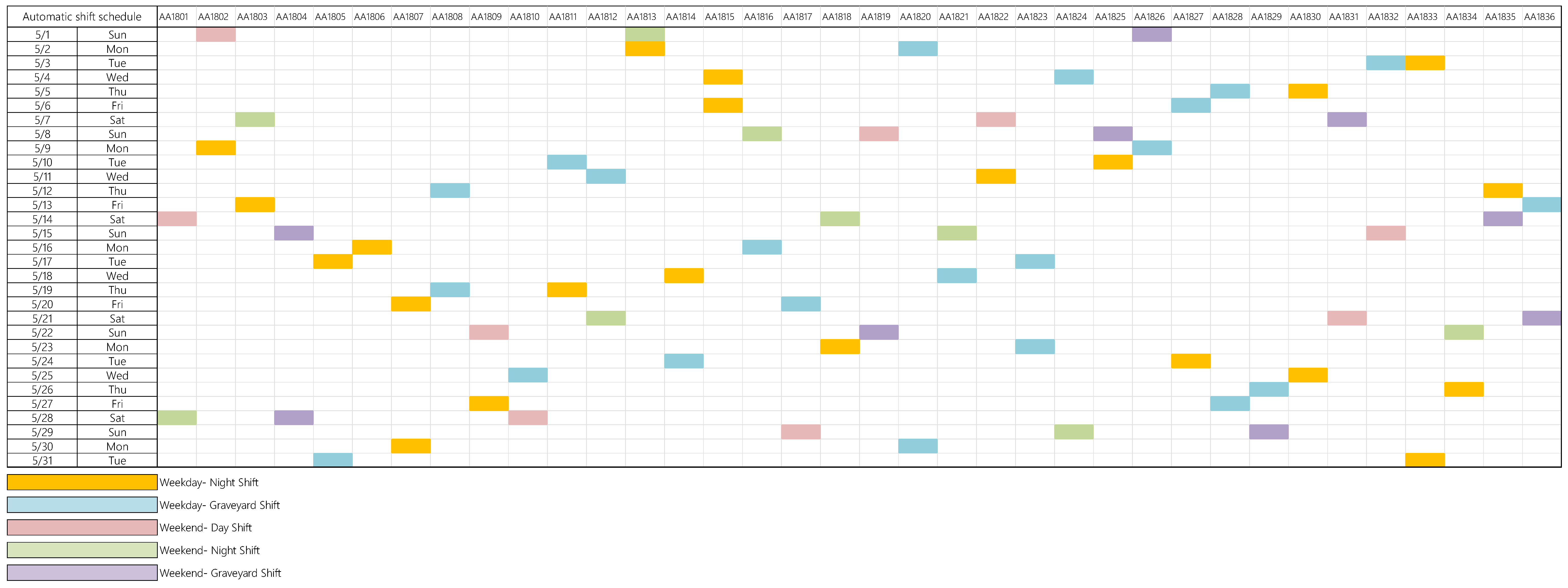
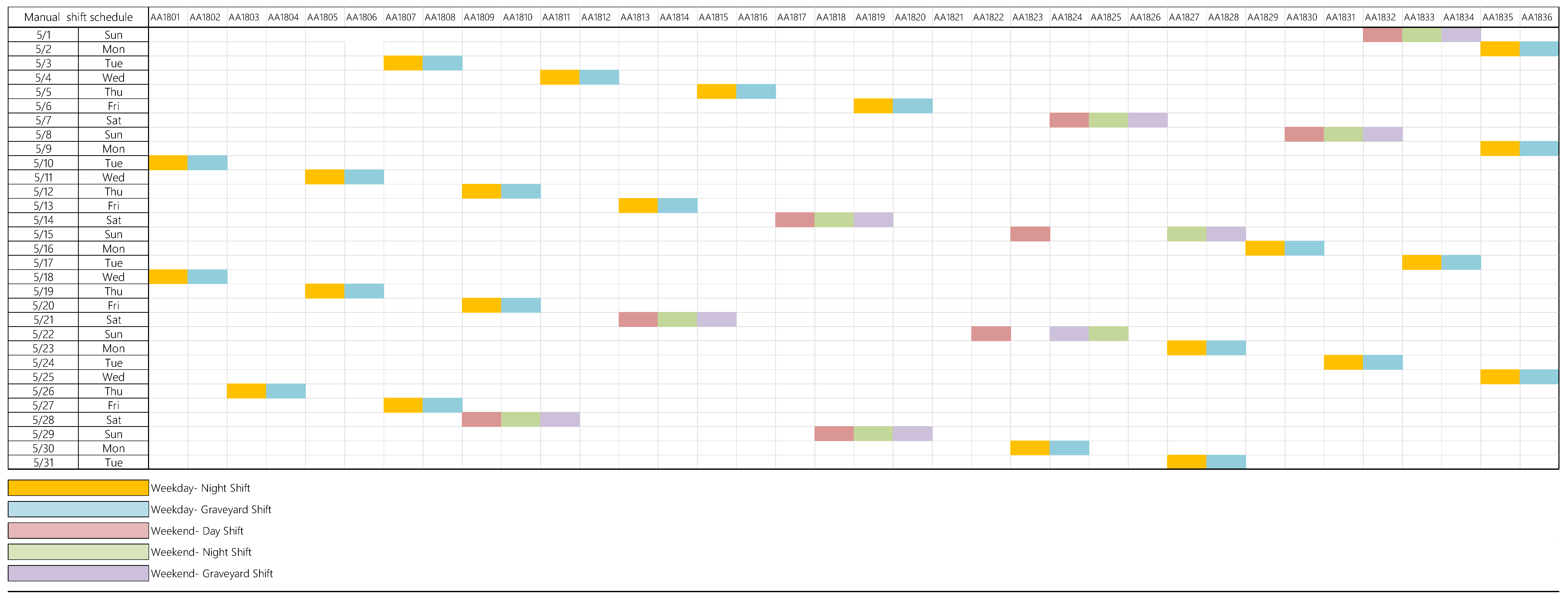
| Auto shift schedule | AA1801 | AA1802 | AA1803 | AA1804 | AA1805 | AA1806 | AA1807 | AA1808 | AA1809 | AA1810 | AA1811 | AA1812 | AA1813 | AA1814 | AA1815 | AA1816 | AA1817 |
| Weekday—Night Shift | 2 | 1 | 4 | 1 | 2 | 2 | 0 | 2 | 1 | 0 | 2 | 0 | 2 | 0 | 0 | 2 | 1 |
| Weekday—Graveyard Shift | 1 | 1 | 0 | 2 | 0 | 2 | 2 | 0 | 1 | 3 | 1 | 2 | 1 | 2 | 2 | 1 | 1 |
| Weekend—Day Shift | 0 | 3 | 0 | 1 | 0 | 1 | 0 | 2 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 1 |
| Weekend—Night Shift | 0 | 0 | 1 | 0 | 2 | 0 | 0 | 0 | 1 | 1 | 0 | 1 | 0 | 2 | 0 | 1 | 0 |
| Weekend—Graveyard Shift | 2 | 0 | 0 | 1 | 0 | 0 | 2 | 0 | 1 | 0 | 0 | 1 | 1 | 0 | 1 | 0 | 0 |
| On Call on Weekdays | 3 | 2 | 4 | 3 | 2 | 4 | 2 | 2 | 2 | 3 | 3 | 2 | 3 | 2 | 2 | 3 | 2 |
| On Call on Weekends | 2 | 3 | 1 | 2 | 2 | 1 | 2 | 2 | 2 | 1 | 1 | 2 | 1 | 2 | 1 | 1 | 1 |
| Total On Call Days | 5 | 5 | 5 | 5 | 4 | 5 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 3 | 4 | 3 |
| Manual shift schedule | AA1801 | AA1802 | AA1803 | AA1804 | AA1805 | AA1806 | AA1807 | AA1808 | AA1809 | AA1810 | AA1811 | AA1812 | AA1813 | AA1814 | AA1815 | AA1816 | AA1817 |
| Weekday—Night Shift | 2 | 2 | 1 | 2 | 1 | 1 | 1 | 1 | 2 | 1 | 2 | 0 | 1 | 1 | 1 | 1 | 2 |
| Weekday—Graveyard Shift | 2 | 2 | 2 | 1 | 2 | 1 | 1 | 1 | 1 | 2 | 0 | 2 | 1 | 1 | 1 | 1 | 1 |
| Weekend—Day Shift | 0 | 0 | 2 | 0 | 0 | 2 | 0 | 0 | 1 | 1 | 1 | 0 | 1 | 0 | 0 | 0 | 1 |
| Weekend—Night Shift | 2 | 0 | 0 | 2 | 0 | 0 | 2 | 0 | 0 | 0 | 1 | 1 | 0 | 1 | 0 | 0 | 0 |
| Weekend—Graveyard Shift | 0 | 2 | 0 | 0 | 2 | 0 | 0 | 2 | 0 | 0 | 0 | 1 | 1 | 0 | 1 | 0 | 0 |
| On Call on Weekdays | 4 | 4 | 3 | 3 | 3 | 2 | 2 | 2 | 3 | 3 | 2 | 2 | 2 | 2 | 2 | 2 | 3 |
| On Call on Weekends | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 1 | 1 | 2 | 2 | 2 | 1 | 1 | 0 | 1 |
| Total On Call Days | 6 | 6 | 5 | 5 | 5 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 3 | 3 | 2 | 4 |
| Auto shift schedule | AA1801 | AA1802 | AA1803 | AA1804 | AA1805 | AA1806 | AA1807 | AA1808 | AA1809 | AA1810 | AA1811 | AA1812 | AA1813 | AA1814 | AA1815 | AA1816 | AA1817 |
| Weekday—Night Shift | 3 | 4 | 2 | 3 | 2 | 5 | 2 | 1 | 1 | 6 | 1 | 2 | 4 | 1 | 2 | 5 | 0 |
| Weekday—Graveyard Shift | 2 | 3 | 2 | 4 | 2 | 1 | 4 | 4 | 3 | 0 | 2 | 4 | 2 | 3 | 4 | 1 | 3 |
| Weekend—Day Shift | 2 | 1 | 0 | 1 | 1 | 1 | 1 | 2 | 0 | 1 | 1 | 1 | 0 | 2 | 1 | 1 | 1 |
| Weekend—Night Shift | 0 | 1 | 3 | 0 | 1 | 2 | 2 | 1 | 3 | 0 | 1 | 0 | 1 | 1 | 1 | 0 | 0 |
| Weekend—Graveyard Shift | 2 | 0 | 1 | 1 | 2 | 0 | 0 | 0 | 1 | 0 | 2 | 1 | 1 | 1 | 0 | 2 | 3 |
| On Call on Weekdays | 5 | 7 | 4 | 7 | 4 | 6 | 6 | 5 | 4 | 6 | 3 | 6 | 6 | 4 | 6 | 6 | 3 |
| On Call on Weekends | 4 | 2 | 4 | 2 | 4 | 3 | 3 | 3 | 4 | 1 | 4 | 2 | 2 | 4 | 2 | 3 | 4 |
| Total On Call Days | 9 | 9 | 8 | 9 | 8 | 9 | 9 | 8 | 8 | 7 | 7 | 8 | 8 | 8 | 8 | 9 | 7 |
| Manual shift schedule | AA1801 | AA1802 | AA1803 | AA1804 | AA1805 | AA1806 | AA1807 | AA1808 | AA1809 | AA1810 | AA1811 | AA1812 | AA1813 | AA1814 | AA1815 | AA1816 | AA1817 |
| Weekday—Night Shift | 3 | 3 | 2 | 2 | 2 | 2 | 2 | 2 | 4 | 2 | 4 | 2 | 4 | 2 | 3 | 2 | 3 |
| Weekday—Graveyard Shift | 3 | 2 | 2 | 2 | 3 | 2 | 2 | 2 | 2 | 4 | 2 | 4 | 3 | 4 | 2 | 3 | 2 |
| Weekend—Day Shift | 1 | 2 | 2 | 0 | 1 | 3 | 0 | 1 | 1 | 1 | 1 | 0 | 1 | 1 | 1 | 0 | 1 |
| Weekend—Night Shift | 3 | 0 | 2 | 2 | 0 | 1 | 3 | 0 | 1 | 0 | 2 | 1 | 0 | 1 | 0 | 1 | 0 |
| Weekend—Graveyard Shift | 0 | 2 | 0 | 2 | 3 | 0 | 1 | 3 | 0 | 1 | 0 | 2 | 1 | 0 | 1 | 0 | 1 |
| On Call on Weekdays | 6 | 5 | 4 | 4 | 5 | 4 | 4 | 4 | 6 | 6 | 6 | 6 | 7 | 6 | 5 | 5 | 5 |
| On Call on Weekends | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 2 | 2 | 3 | 3 | 2 | 2 | 2 | 1 | 2 |
| Total On Call Days | 10 | 9 | 8 | 8 | 9 | 8 | 8 | 8 | 8 | 8 | 9 | 9 | 9 | 8 | 7 | 6 | 7 |
| Auto shift schedule | AA1801 | AA1802 | AA1803 | AA1804 | AA1805 | AA1806 | AA1807 | AA1808 | AA1809 | AA1810 | AA1811 | AA1812 | AA1813 | AA1814 | AA1815 | AA1816 | AA1817 | AA1818 | AA1819 | AA1820 | AA1821 | AA1822 | AA1823 | AA1824 | AA1825 | AA1826 | AA1827 | AA1828 | AA1829 | AA1830 | AA1831 | AA1832 | AA1833 | AA1834 | AA1835 | AA1836 |
| Weekday—Night Shift | 0 | 1 | 1 | 0 | 1 | 1 | 2 | 0 | 1 | 0 | 1 | 0 | 1 | 1 | 2 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 2 | 0 | 0 | 2 | 1 | 1 | 0 |
| Weekday—Graveyard Shift | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 2 | 0 | 1 | 1 | 1 | 0 | 1 | 0 | 1 | 1 | 0 | 0 | 2 | 1 | 0 | 2 | 1 | 0 | 1 | 1 | 2 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 1 |
| Weekend—Day Shift | 1 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 1 | 0 | 0 | 0 | 0 |
| Weekend—Night Shift | 1 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 |
| Weekend—Graveyard Shift | 0 | 0 | 0 | 2 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 1 | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 0 | 1 | 1 |
| On Call on Weekdays | 0 | 1 | 1 | 0 | 2 | 1 | 2 | 2 | 1 | 1 | 2 | 1 | 1 | 2 | 2 | 1 | 1 | 1 | 0 | 2 | 1 | 1 | 2 | 1 | 1 | 1 | 2 | 2 | 1 | 2 | 0 | 1 | 2 | 1 | 1 | 1 |
| On Call on Weekends | 2 | 1 | 1 | 2 | 0 | 0 | 0 | 0 | 1 | 1 | 0 | 1 | 1 | 0 | 0 | 1 | 1 | 1 | 2 | 0 | 1 | 1 | 0 | 1 | 1 | 1 | 0 | 0 | 1 | 0 | 2 | 1 | 0 | 1 | 1 | 1 |
| Total On Call Days | 2 | 2 | 2 | 2 | 2 | 1 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 |
| Manual shift schedule | AA1801 | AA1802 | AA1803 | AA1804 | AA1805 | AA1806 | AA1807 | AA1808 | AA1809 | AA1810 | AA1811 | AA1812 | AA1813 | AA1814 | AA1815 | AA1816 | AA1817 | AA1818 | AA1819 | AA1820 | AA1821 | AA1822 | AA1823 | AA1824 | AA1825 | AA1826 | AA1827 | AA1828 | AA1829 | AA1830 | AA1831 | AA1832 | AA1833 | AA1834 | AA1835 | AA1836 |
| Weekday—Night Shift | 2 | 0 | 1 | 0 | 2 | 0 | 2 | 0 | 2 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 2 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 3 | 0 |
| Weekday—Graveyard Shift | 0 | 2 | 0 | 1 | 0 | 2 | 0 | 2 | 0 | 2 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 2 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 3 |
| Weekend—Day Shift | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 1 | 0 | 0 | 0 | 1 | 1 | 1 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 0 | 0 |
| Weekend—Night Shift | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 1 | 0 | 0 | 0 | 0 | 0 | 2 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 1 | 0 | 0 | 0 |
| Weekend—Graveyard Shift | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 1 | 0 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | 0 | 1 | 0 | 1 | 0 | 0 |
| On Call on Weekdays | 2 | 2 | 1 | 1 | 2 | 2 | 2 | 2 | 2 | 2 | 1 | 1 | 1 | 1 | 1 | 1 | 0 | 0 | 1 | 1 | 0 | 0 | 1 | 1 | 0 | 0 | 2 | 2 | 1 | 1 | 1 | 1 | 1 | 1 | 3 | 3 |
| On Call on Weekends | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 1 | 1 | 0 | 1 | 1 | 1 | 0 | 1 | 2 | 2 | 1 | 0 | 1 | 1 | 2 | 2 | 1 | 1 | 1 | 0 | 1 | 1 | 2 | 1 | 1 | 0 | 0 |
| Total On Call Days | 2 | 2 | 1 | 1 | 2 | 2 | 2 | 2 | 3 | 3 | 2 | 1 | 2 | 2 | 2 | 1 | 1 | 2 | 3 | 2 | 0 | 1 | 2 | 3 | 2 | 1 | 3 | 3 | 1 | 2 | 2 | 3 | 2 | 2 | 3 | 3 |
| Results Comparison | Duty Allocation (Z Auto < Z Manual) | Scheduling Efficiency | Achievement of Shift Restrictions | Fairness (Minimum Number of Shifts Worked) | Rotation Flexibility | ||
|---|---|---|---|---|---|---|---|
| Case | |||||||
| Real Case Example | ✓ Z Auto: 5 < Z Manual: 6 | ✓ | ✓ | ✓ | Auto: 3 days Manual: 2 days | ✓ | |
| Scenarios 1—Schedule for consecutive two months | ✓ Z Auto: 9 < Z Manual: 10 | ✓ | ✓ | ✓ | Auto: 7 days Manual: 6 days | ✓ | |
| Scenarios 2—Schedule for consecutive two months | ✓ Z Auto: 2 < Z Manual: 3 | ✓ | ✓ | ✓ | Auto: 1 days (one staff) Manual: 1 days (eight staff) | ✓ | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, T.-C.; Lin, B.M.T. Optimal Fair-Workload Scheduling: A Case Study at Glorytek. Mathematics 2023, 11, 4051. https://doi.org/10.3390/math11194051
Lin T-C, Lin BMT. Optimal Fair-Workload Scheduling: A Case Study at Glorytek. Mathematics. 2023; 11(19):4051. https://doi.org/10.3390/math11194051
Chicago/Turabian StyleLin, Tzu-Chin, and Bertrand M. T. Lin. 2023. "Optimal Fair-Workload Scheduling: A Case Study at Glorytek" Mathematics 11, no. 19: 4051. https://doi.org/10.3390/math11194051
APA StyleLin, T.-C., & Lin, B. M. T. (2023). Optimal Fair-Workload Scheduling: A Case Study at Glorytek. Mathematics, 11(19), 4051. https://doi.org/10.3390/math11194051





