Modeling and Analyzing Homogeneous Tumor Growth under Virotherapy
Abstract
1. Introduction
2. Model Formulation
3. Equilibrium Analysis
3.1. Trivial Equilibria
3.2. Immunity-Free Equilibria
3.3. Single-Immunity Equilibria
3.4. Dual-Immunity Equilibria
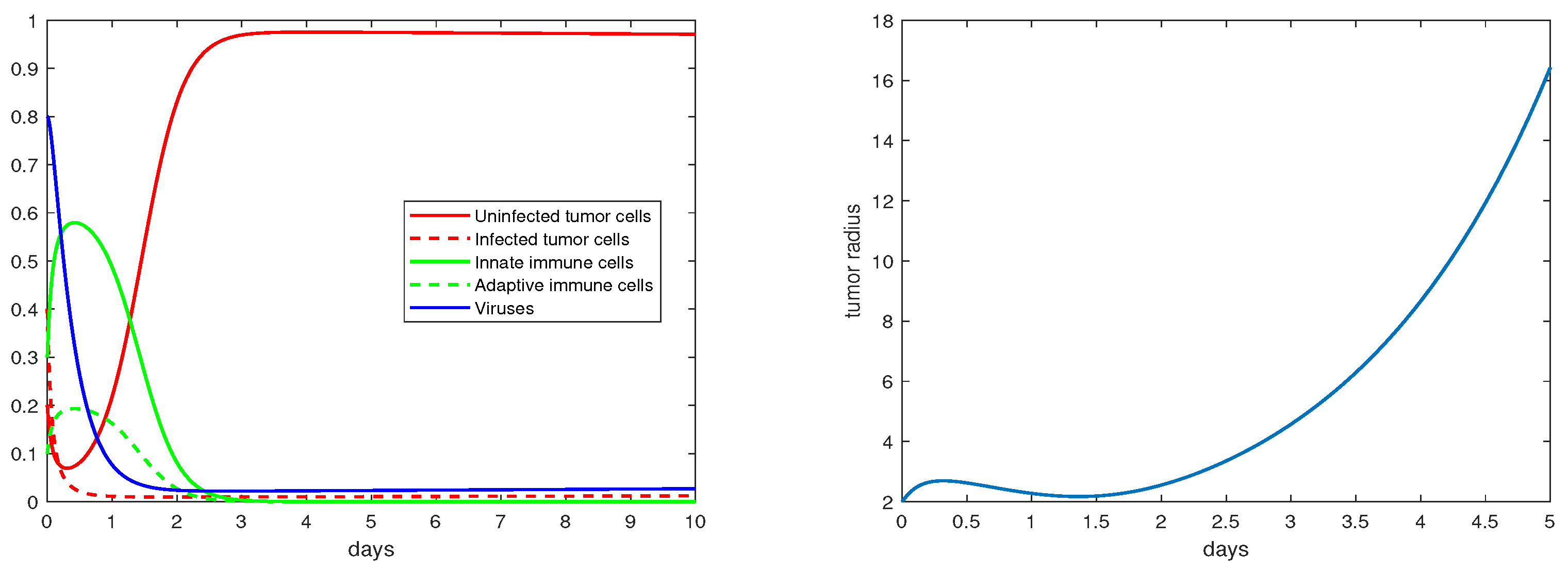
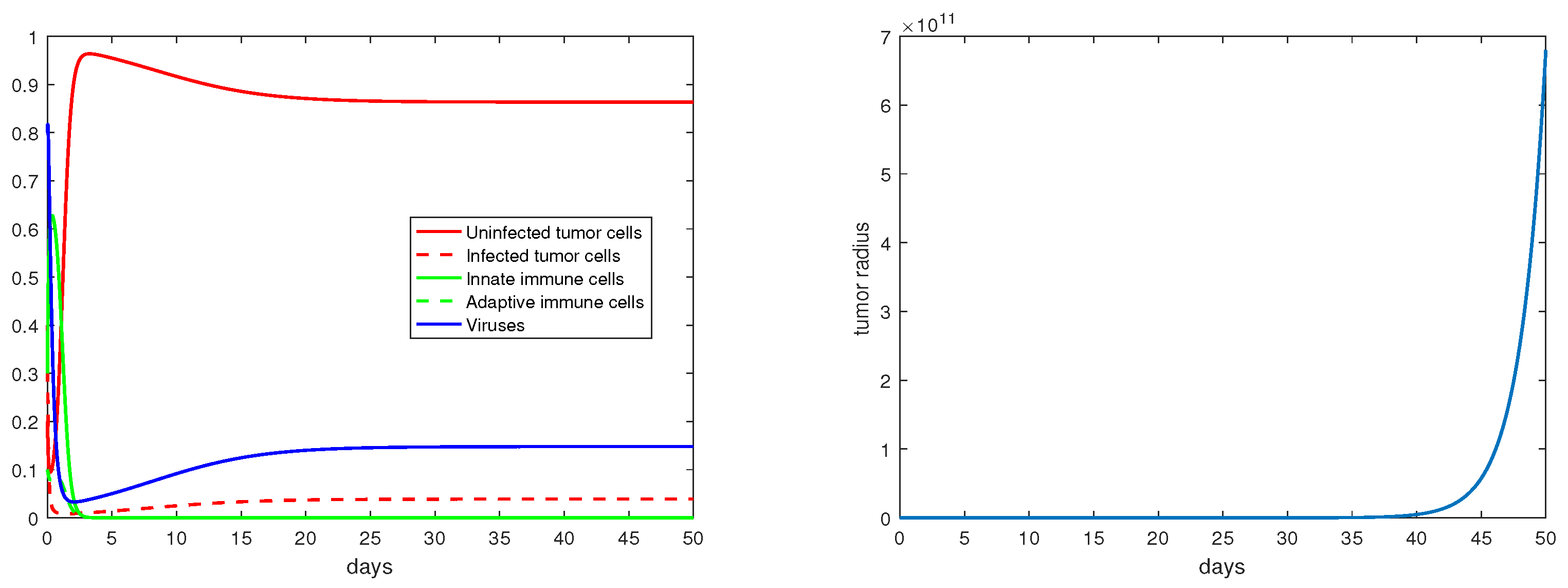
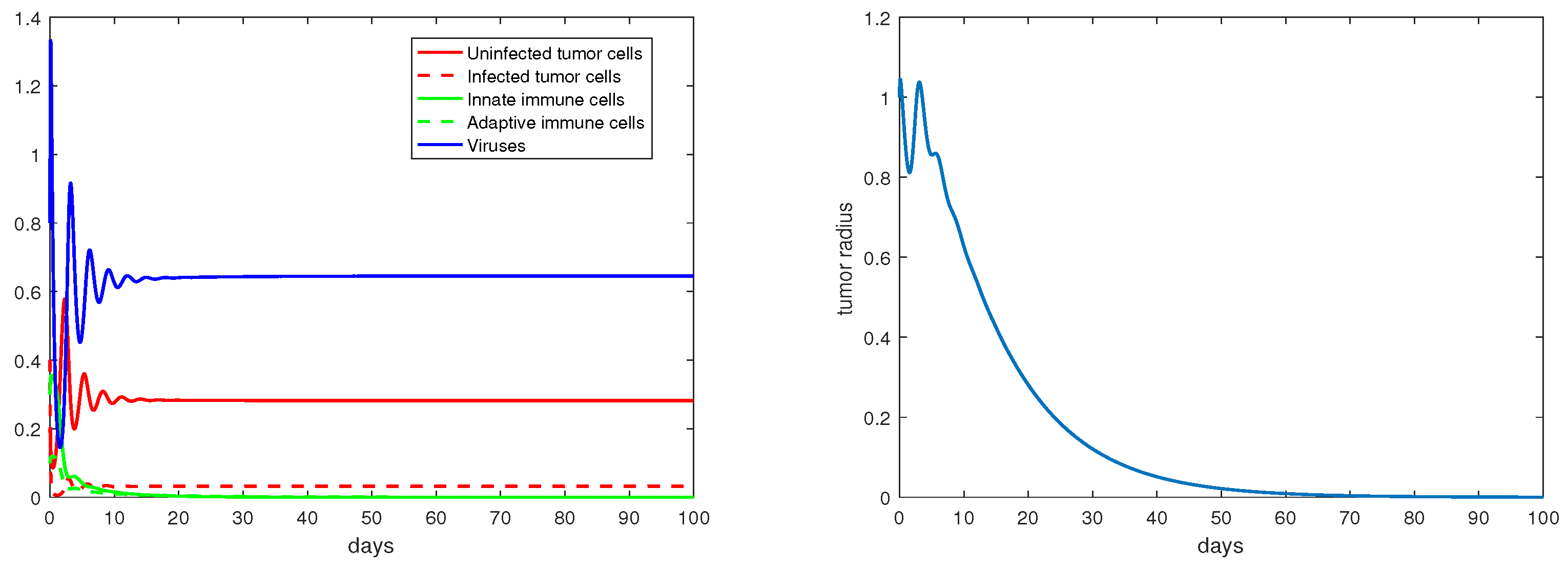
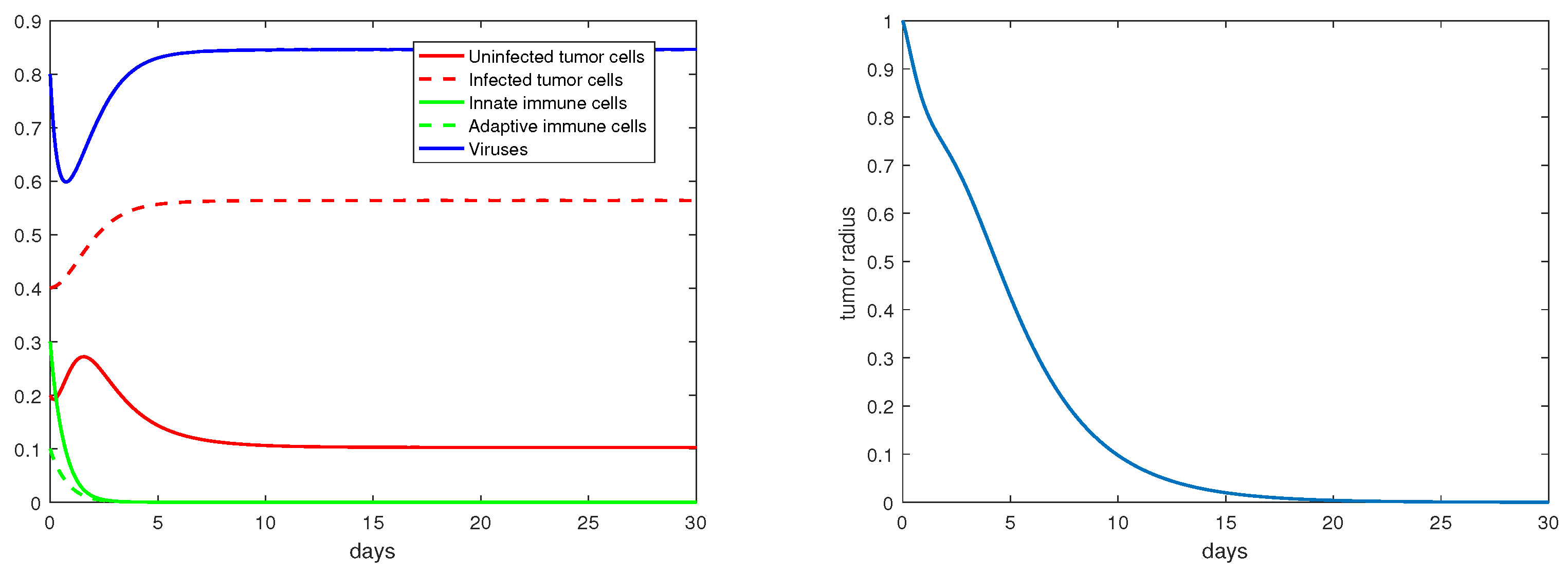
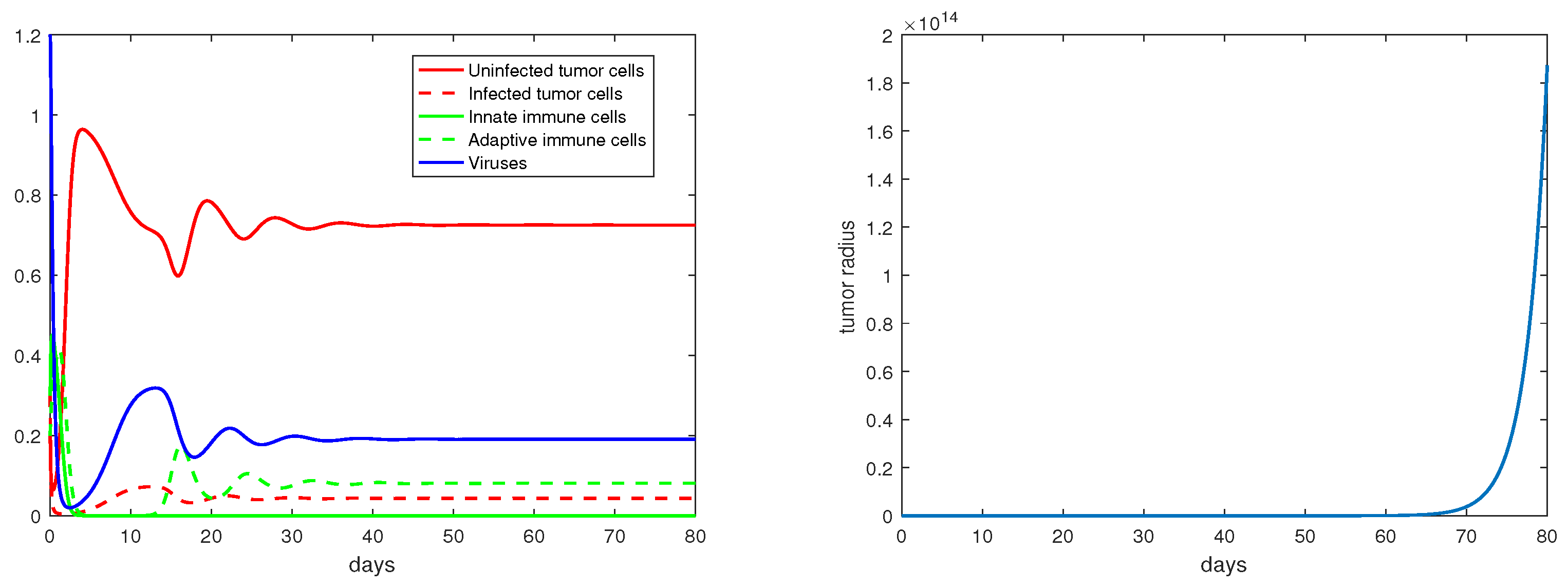
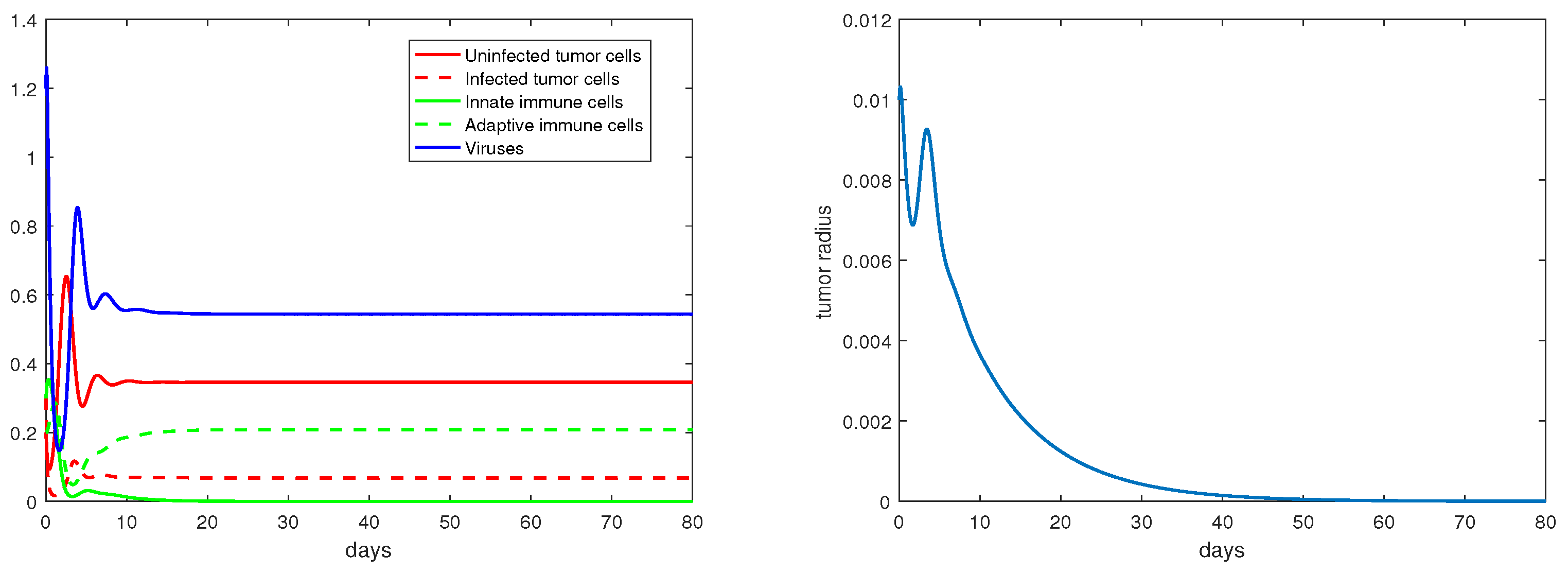
4. Numerical Results
5. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Homogeneous State with a Fixed Boundary
References
- Shah, A.C.; Benos, D.; Gillespie, G.Y.; Markert, J.M. Oncolytic viruses: Clinical applications as vectors for the treatment of malignant gliomas. J. Neurooncol. 2003, 65, 203–226. [Google Scholar] [CrossRef] [PubMed]
- Kasuya, H.; Takeda, S.; Nomoto, S.; Nakao, A. The potential of oncolytic virus therapy for pancreatic cancer. Cancer Gene Ther. 2005, 12, 725–736. [Google Scholar] [CrossRef] [PubMed]
- Kirn, D.H.; McCormick, F. Replicating viruses as selective cancer therapeutics. Mol. Med. Today 1996, 2, 519–527. [Google Scholar] [CrossRef] [PubMed]
- Kirn, D.; Hermiston, T.; McCormick, F. ONYX-015: Clinical data are encouraging. Nat. Med. 1998, 4, 1341–1342. [Google Scholar] [CrossRef]
- Parato, K.A.; Senger, D.; Forsyth, P.A.; Bell, J.C. Recent progress in the battle between oncolytic viruses and tumours. Nat. Rev. Cancer 2005, 5, 965–976. [Google Scholar] [CrossRef] [PubMed]
- Chiocca, E.A.; Rabkin, S.D. Oncolytic viruses and their application to cancer immunotherapy. Cancer Immunol. Res. 2014, 2, 295–300. [Google Scholar] [CrossRef]
- Lawler, S.E.; Chiocca, E.A. Oncolytic virus-mediated immunotherapy: A combinatorial approach for cancer treatment. J. Clin. Oncol. 2015, 33, 2812–2814. [Google Scholar] [CrossRef]
- Fulci, G.; Breymann, L.; Gianni, D.; Kurozomi, K.; Rhee, S.S.; Yu, J.; Kaur, B.; Louis, D.N.; Weissleder, E.; Caligiuri, M.A.; et al. Cyclophosphamide enhances glioma virotherapy by inhibiting innate immune responses. Proc. Natl. Acad. Sci. USA 2006, 103, 12873–12878. [Google Scholar] [CrossRef]
- Wein, L.M.; Wu, J.T.; Kirn, D.H. Validation and analysis of a mathematical model of a replication-competent oncolytic virus for cancer treatment: Implications for virus design and delivery. Cancer Res. 2003, 63, 1317–1324. [Google Scholar]
- Melcher, A.; Parato, K.; Rooney, C.M.; Bell, J.C. Thunder and lightning: Immunotherapy and oncolytic viruses collide. Mol. Ther. 2011, 19, 1008–1016. [Google Scholar] [CrossRef]
- Wodarz, D. Viruses as antitumor weapons: Defining conditions for tumor remission. Cancer Res. 2001, 61, 3501–3507. [Google Scholar]
- Komarova, N.L.; Wodarz, D. ODE models for oncolytic virus dynamics. J. Theor. Biol. 2010, 263, 530–543. [Google Scholar] [CrossRef] [PubMed]
- Karev, G.P.; Novozhilov, A.S.; Koonin, E.V. Mathematical modeling of tumor therapy with oncolytic viruses: Effects of parametric heterogeneity on cell dynamics. Biol. Direct 2006, 1, 30. [Google Scholar] [CrossRef] [PubMed]
- Novozhilov, A.S.; Berezovskaya, F.S.; Koonin, E.V.; Karev, G.P. Mathematical modeling of tumor therapy with oncolytic viruses: Regimes with complete tumor elimination within the framework of deterministic models. Biol. Direct 2006, 1, 6. [Google Scholar] [CrossRef]
- Tian, J.P. The replicability of oncolytic virus: Defining conditions on tumor virotherapy. Math. Biosci. Eng. 2011, 8, 841–860. [Google Scholar] [PubMed]
- Wang, Z.; Guo, Z.; Peng, H. A mathematical model verifying potent oncolytic efficacy of M1 virus. Math. Biosci. 2016, 276, 19–27. [Google Scholar] [CrossRef]
- Wu, J.T.; Byrne, H.M.; Kirn, D.H.; Wein, L.M. Modeling and analysis of a virus that replicates selectively in tumor cells. Bull. Math. Biol. 2001, 63, 731–768. [Google Scholar] [CrossRef]
- Wu, J.T.; Kirn, D.H.; Wein, L.M. Analysis of a three-way race between tumor growth, a replication-competent virus and an immune response. Bull. Math. Biol. 2004, 66, 605–625. [Google Scholar] [CrossRef]
- Friedman, A.; Tian, J.P.; Fulci, G.; Chiocca, E.A.; Wang, J. Glioma virotherapy: Effects of innate immune suppression and increased viral replication capacity. Cancer Res. 2005, 66, 2314–2319. [Google Scholar] [CrossRef]
- Timalsina, A.; Tian, J.P.; Wang, J. Mathematical and computational modeling of tumor virotherapy with mediated immunity. Bull. Math. Biol. 2017, 79, 1736–1758. [Google Scholar] [CrossRef]
- Murray, J.D. Mathematical Biology, 3rd ed.; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Asamoah, J.K.K.; Nyabadza, F.; Jin, Z.; Bonyah, E.; Khan, M.A.; Li, M.Y.; Hayat, T. Backward bifurcation and sensitivity analysis for bacterial meningitis transmission dynamics with a nonlinear recovery rate. Chaos Solitons Fractals 2020, 140, 110237. [Google Scholar] [CrossRef]
- Liu, Y.T.; Sun, Z.J. Turning cold tumors into hot tumors by improving T-cell infiltration. Theranostics 2021, 11, 5365–5386. [Google Scholar] [CrossRef] [PubMed]
- Hinow, P.; Gerlee, P.; McCawley, L.J.; Quaranta, V.; Ciobanu, M.; Wang, S.; Graham, J.M.; Ayati, B.P.; Claridge, J.; Swanson, K.R.; et al. A spatial model of tumor-host interaction: Application of chemotherapy. Math. Biosci. Eng. 2009, 6, 521–546. [Google Scholar] [PubMed]
- Preziosi, L. Cancer Modelling and Simulation; Chapman Hall/CRC Press: Boca Raton, FL, USA, 2003. [Google Scholar]





| Symbol | Description | Unit |
|---|---|---|
| Proliferation rate of tumor cells | h | |
| Infection rate of viruses | mm h virus | |
| Killing rate of innate immune response | mm h cell | |
| Killing rate of adaptive immune response | mm h cell | |
| Stimulation rate of innate immunity | mm h cell | |
| Stimulation rate of adaptive immunity | mm h cell | |
| Clearance rate of innate immune cells | h | |
| Clearance rate of adaptive immune cells | h | |
| D | Diffusion coefficient of viruses | mm h |
| b | Burst size of viruses | virus cell |
| Lysis rate of infected tumor cell | h | |
| Take-up rate of viruses by innate immunity | mm h cell | |
| Clearance rate of viruses | h | |
| Removal rate of dead tumor cells | h |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, C.; Wang, J. Modeling and Analyzing Homogeneous Tumor Growth under Virotherapy. Mathematics 2023, 11, 360. https://doi.org/10.3390/math11020360
Yang C, Wang J. Modeling and Analyzing Homogeneous Tumor Growth under Virotherapy. Mathematics. 2023; 11(2):360. https://doi.org/10.3390/math11020360
Chicago/Turabian StyleYang, Chayu, and Jin Wang. 2023. "Modeling and Analyzing Homogeneous Tumor Growth under Virotherapy" Mathematics 11, no. 2: 360. https://doi.org/10.3390/math11020360
APA StyleYang, C., & Wang, J. (2023). Modeling and Analyzing Homogeneous Tumor Growth under Virotherapy. Mathematics, 11(2), 360. https://doi.org/10.3390/math11020360






