Identification of Inhomogeneities: The Selected Coordinate Descent Method Applied in the Drilling Area
Abstract
:1. Introduction
2. Modeling of the Elastic Medium Structure
3. The SCD’s Ability to Recognize the Large-Size Inhomogeneity
3.1. Special Tactic of SCD in Comparison with CD and the Conjugate Gradient Methods
3.2. The SCD Iterative Technique
3.3. How to Distinguish Well and Poorly Resolved Elements in the Solution Vector?
4. The SCD Application to Seismic Data in the Region of Geothermal Power Stations
4.1. Why a Site for the Production Well Should Be Thoroughly Selected?
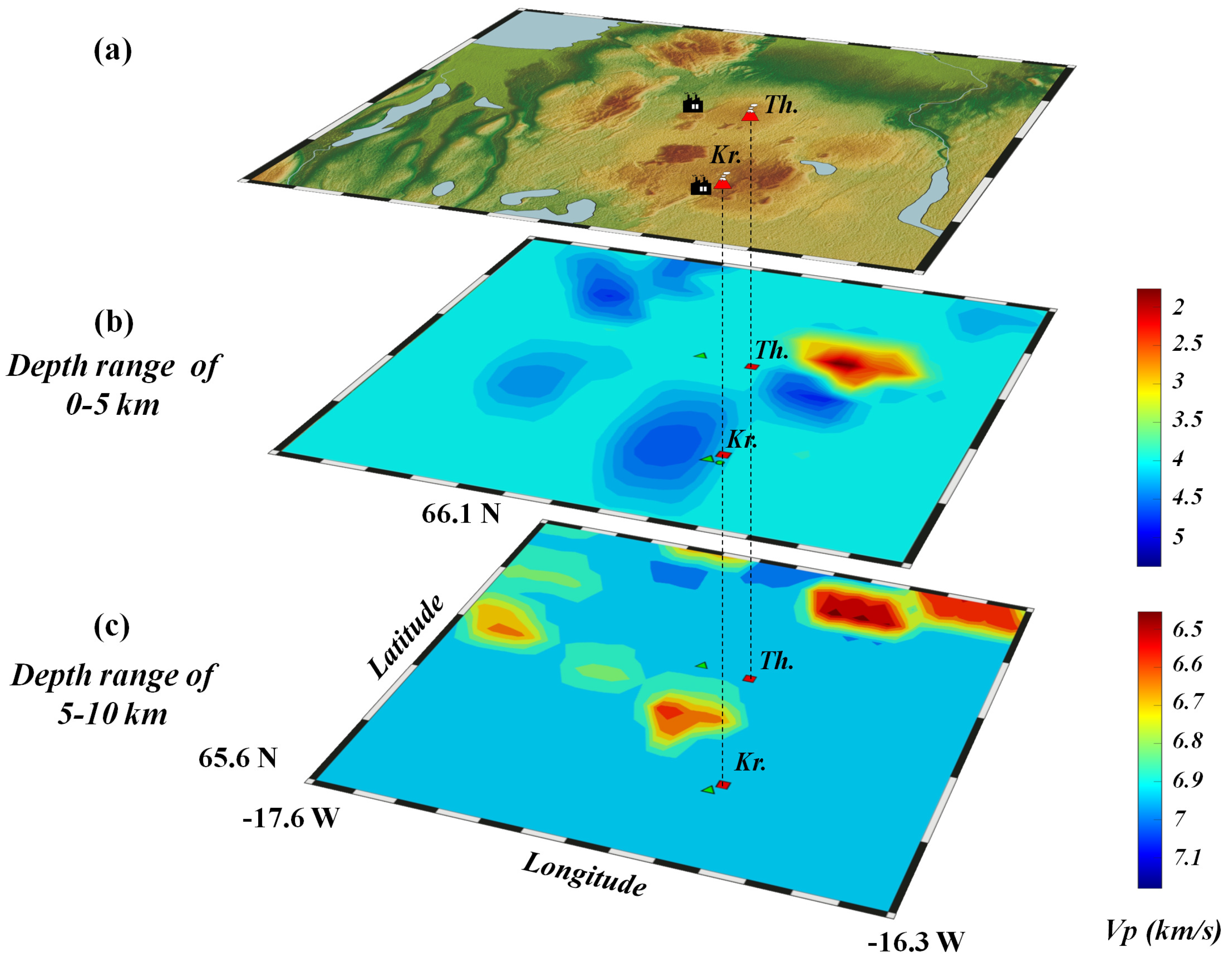
4.2. Measurements and Numerical Example of the System
4.3. Identification of Well-Resolved Seismic Anomalies
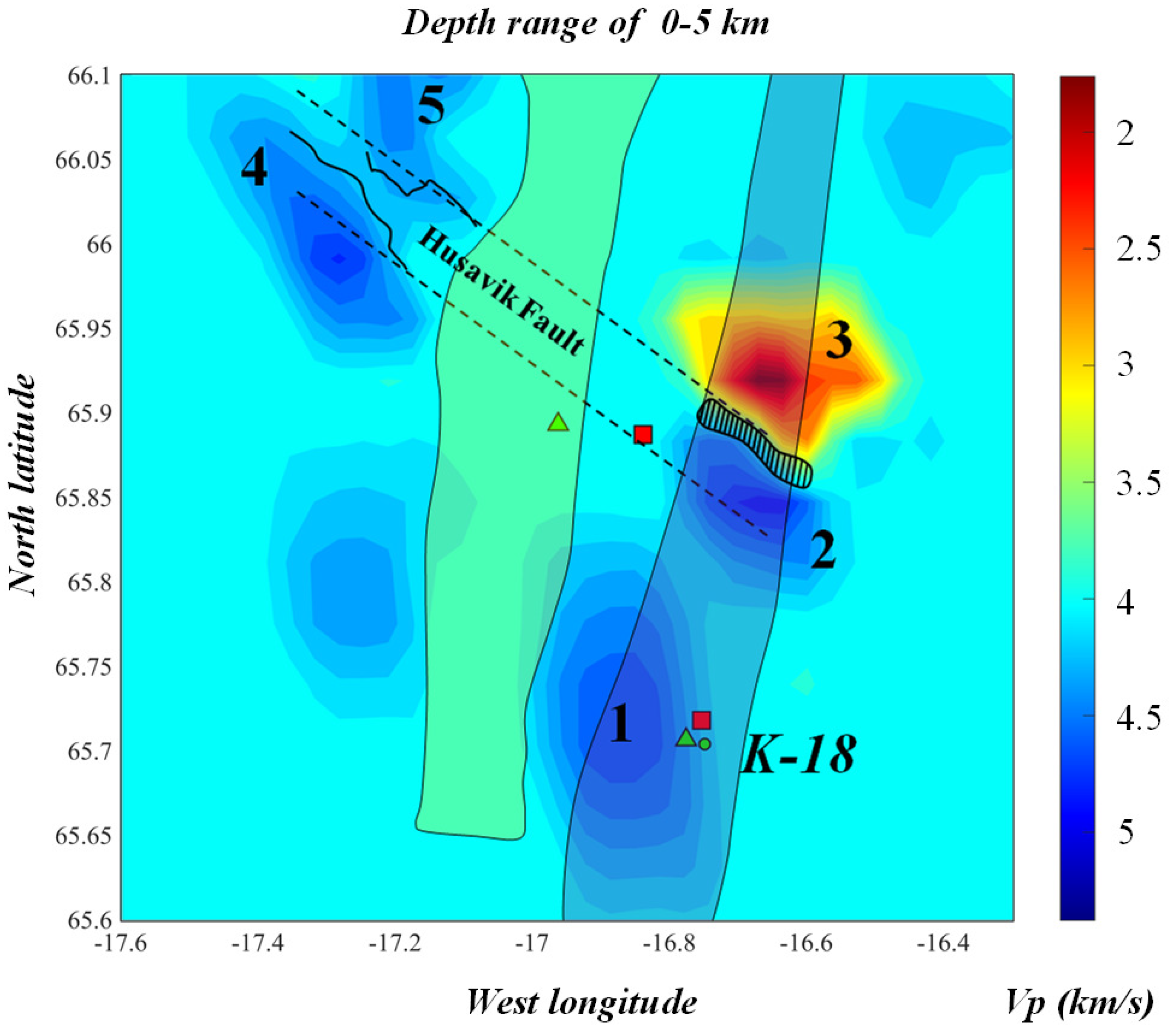
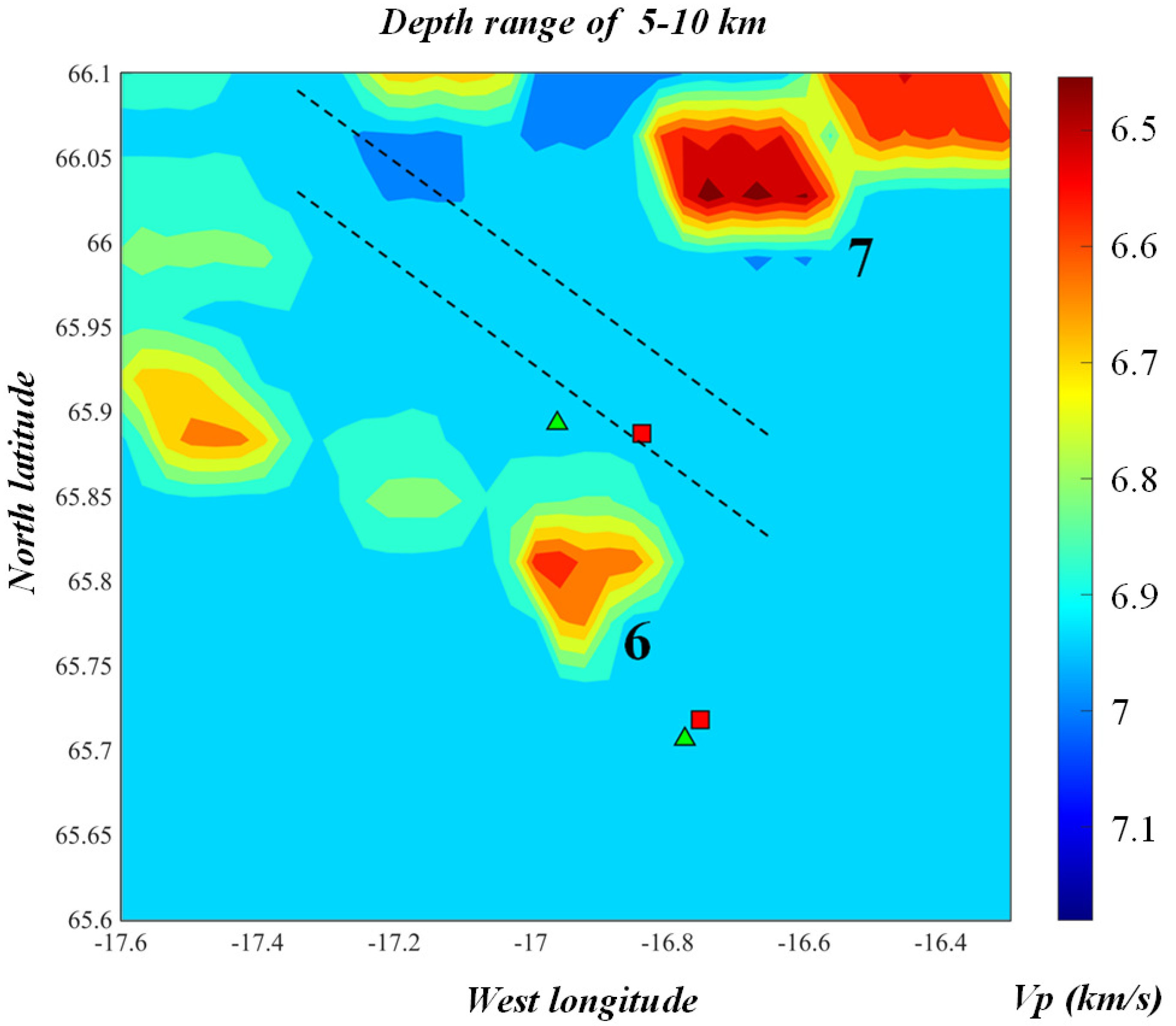
5. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ratliff, N.; Toussaint, M.; Schaal, S. Understanding the geometry of workspace obstacles in Motion Optimization. In Proceedings of the 2015 IEEE International Conference on Robotics and Automation (ICRA), Seattle, WA, USA, 26–30 May 2015; pp. 4202–4209. [Google Scholar] [CrossRef]
- Chen, Y.; Luo, X.; Han, B.; Jia, Y.; Liang, G.; Wang, X. A General Approach Based on Newton’s Method and Cyclic Coordinate Descent Method for Solving the Inverse Kinematics. Appl. Sci. 2019, 9, 5461. [Google Scholar] [CrossRef]
- Walley, S.M.; Field, J.E. Elastic Wave Propagation in Materials. In Reference Module in Materials Science and Materials Engineering; Elsevier: Cambridge, UK, 2016. [Google Scholar] [CrossRef]
- Hernandez, P.M.; Socas, A.; Benitez, A.N.; Ortega, F.; Diaz, N.; Marrero, M.D.; Monzon, M.D. Boundary Element Method Applied to Electroforming Process. Mater. Sci. Forum 2014, 797, 125–132. [Google Scholar] [CrossRef]
- Xie, C.G. Applications of tomography in oil-gas industry—Part 1. In Industrial Tomography, Woodhead Publishing Series in Electronic and Optical Materials; Wang, M., Ed.; Woodhead Publishing: Cambridge, UK, 2015; pp. 591–632. [Google Scholar] [CrossRef]
- Pariseau, W.G. Finite Element Analysis of Barrier Pillar Mining at Crandall Canyon. In Mine Safety and Health Administration; University of Utah: Salt Lake City, UT, USA, 2008. [Google Scholar]
- Mishra, D.; Yadav, R.S.; Agrawal, K.K. Kinematic modelling and emulation of robot for traversing over the pipeline in the refinery. Microsyst. Technol. 2020, 26, 1011–1020. [Google Scholar] [CrossRef]
- Hachtel, G.D.; Brayton, R.K.; Gustavson, F.G. The Sparse Tableau Approach to Network Analysis and Design. IEEE Trans. Circuit Theory 1971, 18, 101–113. [Google Scholar] [CrossRef]
- de Araujo, L.R.; Penido, D.R.R.; Pereira, J.L.R.; Carneiro, S., Jr. A Sparse Linear Systems Implementation for Electric Power Systems Solution Algorithms. J. Control Autom. Electr. Syst. 2013, 24, 504–512. [Google Scholar] [CrossRef]
- Werff, H.; Noomen, M.; Meijde, M.; Kooistra, J.; Meer, F. Use of hyperspectral remote sensing to detect hazardous gas leakage from pipelines. In New Developments and Challenges in Remote Sensing; Millpress: Rotterdam, The Netherlands, 2007; pp. 707–715. [Google Scholar]
- Horiuchi, S.; Negishi, H.; Abe, K.; Kamimura, A.; Fujinawa, Y. An Automatic Processing System for Broadcasting Earthquake Alarms. Bull. Seismol. Soc. Am. 2005, 95, 708–718. [Google Scholar] [CrossRef]
- Engquist, B.; Rimborg, O. Multi-phase computations in Geometrical Optics. J. Comput. Appl. Math. 1996, 74, 175–192. [Google Scholar] [CrossRef]
- Dehghanghadikolaei, A.; Ibrahim, H.; Amerinatanzi, A.; Elahinia, M. 9—Biodegradable magnesium alloys. In Woodhead Publishing Series in Biomaterials, Metals for Biomedical Devices, 2nd ed.; Niinomi, M., Ed.; Woodhead Publishing: Cambridge, UK, 2019; pp. 265–289. [Google Scholar] [CrossRef]
- Ghosh, A.; Mallik, A.K. Theory of Mechanisms and Machines, 3rd ed.; Affiliated East West Press: New Delhi, India, 2015. [Google Scholar]
- Wang, L.; Chen, C.A. Combined optimization method for solving the inverse kinematics problem of mechanical manipulators. IEEE Trans. Robot. Autom. 1991, 7, 489–498. [Google Scholar] [CrossRef]
- Toussaint, M. Robot trajectory optimization using approximate inference. In Proceedings of the 26th International Conference on Machine Learning (ICML), Montreal, QC, Canada, 14–18 June 2009; pp. 1049–1056. [Google Scholar]
- Luenberger, D.G.; Ye, Y. Conjugate Direction Methods. In Linear and Nonlinear Programming; International Series in Operations Research & Management Science; Springer: New York, NY, USA, 2008; Volume 116, pp. 263–284. [Google Scholar] [CrossRef]
- Wright, S.J. Coordinate descent algorithms. Math. Program. 2015, 151, 3–34. [Google Scholar] [CrossRef]
- Rodgers, C.D. Inverse Methods for Atmospheric Soundings: Theory and Practice; Series on Atmospheric, Oceanic and Planetary Physics; World Scientific: Singapore, 2000; Volume 2. [Google Scholar] [CrossRef]
- Aliabadi, M.H. Boundary Element Methods in Linear Elastic Fracture Mechanics. In Reference Module in Materials Science and Materials Engineering; Elsevier: Amsterdam, The Netherlands, 2016. [Google Scholar] [CrossRef]
- LaForce, T. PE281 Boundary Element Method Course Notes; Stanford University: Stanford, CA, USA, 2006. [Google Scholar]
- Valente, F.P.; Pina, H.L.G. Iterative solvers for BEM algebraic systems of equations. Eng. Anal. Bound. Elem. 1998, 22, 117–124. [Google Scholar] [CrossRef]
- Lanczos, C. An iteration method for the solution of the eigenvalue problem of linear differential and integral operators. J. Abbr. 1950, 45, 255–282. [Google Scholar] [CrossRef]
- Smaglichenko, T.A.; Smaglichenko, A.V. Resolution Estimates for Selected Coordinate Descent: Identification of Seismic Structure in the Area of Geothermal Plants. In Advances in Production Management Systems. Artificial Intelligence for Sustainable and Resilient Production Systems. IFIP Advances in Information and Communication Technology; Dolgui, A., Bernard, A., Lemoine, D., von Cieminski, G., Romero, D., Eds.; Springer: Cham, Switzerland, 2021; Volume 630, pp. 580–588. [Google Scholar] [CrossRef]
- Dahm, T.; Cesca, S.; Hainzl, S.; Braun, T.; Kruger, F. Discrimination between induced, triggered, and natural earthquakes close to hydrocarbon reservoirs: A probabilistic approach based on the modeling of depletion-induced stress changes and seismological source parameters. J. Geophys. Res. Solid Earth 2015, 120, 2491–2509. [Google Scholar] [CrossRef]
- Wilson, M.P.; Davies, R.J.; Foulger, G.R.; Julian, B.R.; Styles, P.; Gluyas, J.G.; Almond, S. Anthropogenic earthquakes in the UK: A national baseline prior to shale exploitation. Mar. Pet. Geol. 2015, 68, 1–17. [Google Scholar] [CrossRef]
- Foulger, G.R.; Wilson, M.P.; Gluyas, J.G.; Julian, B.R.; Davies, R.J. Global review of human-induced earthquakes. Earth-Sci. Rev. 2018, 178, 438–514. [Google Scholar] [CrossRef]
- Paige, C.; Saunders, M. LSQR: An algorithm for sparse linear equations and sparse least squares. ACM Trans. Math. Soft. 1982, 8, 43–71. [Google Scholar] [CrossRef]
- Fong, D.C.L.; Saunders, M. LSMR: An iterative algorithm for sparse least-squares problems. SIAM J. Sci. Comput. 2011, 33, 2950–2971. [Google Scholar] [CrossRef]
- Widiyantoro, S.; van der Hilst, R. Mantle structure beneath Indonesia inferred from high-resolution tomographic imaging. Geophys. J. Int. 1997, 130, 167–182. [Google Scholar] [CrossRef]
- Tryggvason, A.; Rognvaldsson, S.T.; Flovenz, O.G. Three-dimensional imaging of the P- and S-wave velocity structure and earthquake locations beneath Southwest Iceland. Geophys. J. Int. 2002, 151, 848–866. [Google Scholar] [CrossRef]
- Bianco, M.J.; Gerstoft, P.; Olsen, K.B.; Lin, F. High-resolution seismic tomography of Long Beach, CA using machine learning. Sci. Rep. 2019, 9, 14987. [Google Scholar] [CrossRef]
- Yao, Z.S.; Roberts, R.G.; Tryggvason, A. Calculating resolution and covariance matrices for seismic tomography with the LSQR method. Geophys. J. Int. 1999, 138, 886–894. [Google Scholar] [CrossRef]
- Leveque, J.J.; Rivera, L.; Wittlinger, G. On the use of the checker-board test to assess the resolution of tomographic inversions. Geophys. J. Int. 1993, 115, 313–318. [Google Scholar] [CrossRef]
- Smaglichenko, T.A.; Nikolaev, A.V.; Horiuchi, S.; Hasegawa, A. The method for consecutive subtraction of selected anomalies: The estimated crustal velocity structure in the 1996 Onikobe (M = 5.9) earthquake area (1996), northeastern Japan. Geophys. J. Int. 2003, 153, 627–644. [Google Scholar] [CrossRef]
- Smaglichenko, T.A.; Jacoby, W.; Smaglichenko, A.V. Alternative 3D Tomography Methods and Their Applications to Identify Seismic Structure Around the Hydrothermal Gas Field. In Proceedings of the 2020 Eleventh International Conference “Management of Large-Scale System Development” (MLSD), Moscow, Russia, 28–30 September 2020; Institute of Electrical and Electronics Engineers (IEEE): Piscataway Township, NJ, USA, 2020; p. 9247697. [Google Scholar] [CrossRef]
- Warga, J. Minimizing certain convex functions. J. Soc. Ind. Appl. Math. 1963, 11, 588–593. [Google Scholar] [CrossRef]
- Twomey, S. On the numerical solution of Fredholm integral equation of the first kind by the inversion of the linear system produced by quadrature. J. Assoc. Comput. Mach. 1963, 10, 97–101. [Google Scholar] [CrossRef]
- Tikhonov, A.N. On the solution of incorrectly stated problems and a method of regularization. Dokl. Acad. Nauk SSSR 1963, 151, 501–504. [Google Scholar]
- Mateer, C.L. On the information content of umkehr observations. J. Atmos. Sci. 1965, 22, 370–381. [Google Scholar] [CrossRef]
- Smaglichenko, T.; Smaglichenko, A.; Sayankina, M.; Chigarev, B. Seismic Anomalies in the Geothermal District Revealed by the Relaxation Algorithm of Selected Coordinate Descent. In Proceedings of the 2021 14th International Conference “Management of Large-Scale System Development” (MLSD), Moscow, Russia, 27–29 September 2021; Institute of Electrical and Electronics Engineers (IEEE): Piscataway Township, NJ, USA, 2021; p. 21507066. [Google Scholar] [CrossRef]
- Strauss, Z. Unearthing Potential: The Value of Geothermal Energy to US Decarbonization; Atlantic Council: Washington, DC, USA, 2022. [Google Scholar]
- Saner, D.; Juraske, R.; Kübert, M.; Blum, P.; Hellweg, S.; Bayer, P. Is it only CO2 that matters? A life cycle perspective on shallow geothermal systems. Renew. Sustain. Energy Rev. 2010, 14, 1798–1813. [Google Scholar] [CrossRef]
- Gkousis, S.; Welkenhuysen, K.; Compernolle, T. Deep geothermal energy extraction, a review on environmental hotspots with focus on geo-technical site conditions. Renew. Sustain. Energy Rev. 2022, 162, 112430. [Google Scholar] [CrossRef]
- Fridleifsson, G.O.; Elders, W.A. Progress Report on the Iceland Deep Drilling Project (IDDP). Sci. Drill. 2007, 4, 26–29. [Google Scholar] [CrossRef]
- Zang, A.; Oye, V.; Jousset, P.; Deichmann, N.; Gritto, R.; McGarr, A.; Majer, E.; Bruhn, D. Analysis of induced seismicity in geothermal reservoirs—An overview. Geothermics 2014, 52, 6–21. [Google Scholar] [CrossRef]
- Bjornsson, S.; Gudmundsdottir, I.D.; Ketilsson, J. (Eds.) Geothermal Development and Research in Iceland; Orkustofnun: Reykjavik, Iceland, 2010. [Google Scholar]
- Dobson, P.F.; Asanuma, H.; Huenges, E.; Poletto, F.; Reinsch, T.; Sanjuan, B. Supercritical Geothermal Systems—A Review of Past Studies and Ongoing Research Activities. In Proceedings of the 42nd Workshop on Geothermal Reservoir Engineering, Stanford, CA, USA, 13–15 February 2017; pp. 670–682. [Google Scholar]
- Elders, W.; Fridleifsson, G.O. The Science Program of the Iceland Deep Drilling Project (IDDP): A study of supercritical geothermal resources. In Proceedings of the World Geothermal Congress 2010, Bali, Indonesia, 25–29 April 2010. [Google Scholar]
- Poletto, F.; Corubolo, P.; Farina, B.; Schleifer, A.; Pollard, J.; Peronio, M.; Bohm, G. Drill-bit SWD and seismic interferometry for imaging around geothermal wells. In Proceedings of the SEG Technical Program Expanded Abstracts, San Antonio Annual Meeting, San Antonio, TX, USA, 18–23 September 2011; Volume 2011, pp. 4319–4324. [Google Scholar]
- McClelland, L. (Ed.) Global Volcanism Program. Report on Krafla (Iceland). In Scientific Event Alert Network Bulletin; Smithsonian Institution: Washington, DC, USA, 1989; Volume 14. [Google Scholar] [CrossRef]
- Hjartardottir, A.R.; Einarsson, P.; Bramham, E.; Wright, T.J. The Krafla fissure swarm, Iceland, and its formation by rifting events. Bull. Volcanol. 2012, 74, 2139–2153. [Google Scholar] [CrossRef]
- Millett, J.M.; Planke, S.; Kastner, F.; Blischke, A.; Hersir, G.P.; Halldorsdottir, S.; Flovenz, O.G.; Arnadottir, S.; Helgadottir, H.M.; Vakulenko, S.; et al. Sub-surface geology and velocity structure of the Krafla high temperature geothermal field, Iceland: Integrated ditch cuttings, wireline and zero offset vertical seismic profile analysis. J. Volcanol. Geotherm. Res. 2020, 391, 106342. [Google Scholar] [CrossRef]
- Kastner, F.; Giese, R.; Planke, S.; Millett, J.M.; Flovenz, O.G. Seismic imaging in the Krafla high-temperature geothermal field, NE Iceland, using zero- and far-offset vertical seismic profiling (VSP) data. J. Volcanol. Geotherm. Res. 2020, 391, 106315. [Google Scholar] [CrossRef]
- Einarsson, P. S-wave shadows in the Krafla Caldera in NE-Iceland, evidence for a magma chamber in the crust. Bull. Volcanol. 1978, 41, 187–195. [Google Scholar] [CrossRef]
- Gudmundsson, A.; Mortensen, A.K. Well locations-consideration of purpose, objectives and achievement with emphasis on recent drilling in the Krafla Geothermal area. In Proceedings of the World Geothermal Congress, Melbourne, Australia, 19–25 April 2015. [Google Scholar]
- Murai, Y.; Katsumata, K.; Takanami, T.; Watanabe, T.; Yamashina, T.; Cho, I.; Tanaka, M.; Azuma, R. Seismic velocity structure in southern Hokkaido deduced from ocean-bottom seismographic and land observations. In Proceedings of the Japan Geoscience Union Meeting, Makuhari Messe, Chiba, Japan, 26–30 May 2019. [Google Scholar]
- Rognvaldsson, S.; Gudmundsson, A.; Slunga, R. Seismotectonic Analysis of the Tjornes Fracture Zone, an Active Transform Fault in North Iceland. J. Geophys. Res. 1998, 103, 30117–30129. [Google Scholar] [CrossRef]
- Lebedev, E.B.; Ryzhenko, B.N.; Burkhardt, H.; Zharikov, A.V.; Roshchina, I.A.; Kononkova, N.N.; Zebrin, S.R. Influence of fluid composition on the elastic properties of sandstone and quartzite at high temperature and pressure in application to the problem of crustal waveguides. Izv. Phys. Solid Earth 2014, 50, 367–377. [Google Scholar] [CrossRef]
- Fridleifsson, G.O.; Armannsson, H.; Arnason, K.; Bjarnason, I.T.; Gislason, G. Part I: Geosciences and Site Selection. In Iceland Deep Drilling Project, Feasibility Report Number: OS-2003/007; Fridleifsson, G.O., Ed.; Orkustofnun: Reykjavik, Iceland, 2003. [Google Scholar]
- Hutchings, L.; Bonner, B.; Saltiel, S.; Jarpe, S.; Nelson, M. Rock Physics Interpretation of Tomographic Solutions for Geothermal Reservoir Properties. In Applied Geophysics with Case Studies on Environmental, Exploration and Engineering Geophysics; IntechOpen: Rijeka, Croatia, 2019. [Google Scholar] [CrossRef]

| k | D(k) | |
|---|---|---|
| 1 | 0.0697 | 0.5304 |
| 2 | −0.0002 | 0.000001 |
| k | |||
|---|---|---|---|
| 1 | 0.0998 | 0.998 | 0.0009 |
| 2 | −0.096 | 0.6799 | −0.0029 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Smaglichenko, T.; Smaglichenko, A. Identification of Inhomogeneities: The Selected Coordinate Descent Method Applied in the Drilling Area. Mathematics 2023, 11, 4297. https://doi.org/10.3390/math11204297
Smaglichenko T, Smaglichenko A. Identification of Inhomogeneities: The Selected Coordinate Descent Method Applied in the Drilling Area. Mathematics. 2023; 11(20):4297. https://doi.org/10.3390/math11204297
Chicago/Turabian StyleSmaglichenko, Tatyana, and Alexander Smaglichenko. 2023. "Identification of Inhomogeneities: The Selected Coordinate Descent Method Applied in the Drilling Area" Mathematics 11, no. 20: 4297. https://doi.org/10.3390/math11204297
APA StyleSmaglichenko, T., & Smaglichenko, A. (2023). Identification of Inhomogeneities: The Selected Coordinate Descent Method Applied in the Drilling Area. Mathematics, 11(20), 4297. https://doi.org/10.3390/math11204297





