Mathematical Models for Forecasting Unstable Economic Processes in the Eurozone
Abstract
:1. Introduction
2. Literature Review
3. Mathematical Model
- The main factor that increases the inflation rate is the growth in the money supply;
- The key role is played by the equilibrium state of the money market;
- The nominal interest rate is determined through the real interest rate and the expected inflation rate (Fisher identity).
- Model of the dynamics of economic growth (recession):
- Model of forecasting inflation rates:where α, ρ, and κ are constant coefficients; is the monetary base growth rate; M is the monetary base; shows the GDP growth rates; is the equilibrium potential level of the GDP growth rates in the medium term; Y is the GDP volume; d is the state budget deficit (in shares of GDP); r is the real interest rate; and π is inflation.
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Akaev, A.; Zvyagintsev, A.I.; Sarygulov, A.; Devezas, T.; Tick, A.; Ichkitidze, A. Growth Recovery and COVID-19 Pandemic Model: Comparative Analysis for Selected Emerging Economies. Mathematics 2022, 10, 3654. [Google Scholar] [CrossRef]
- Gajdosikova, D.; Lăzăroiu, G.; Valaskova, K. How Particular Firm-Specific Features Influence Corporate Debt Level: A Case Study of Slovak Enterprises. Axioms 2023, 12, 183. [Google Scholar] [CrossRef]
- Kliestik, T.; Valaskova, K.; Lazaroiu, G.; Kovacova, M.; Vrbka, J. Remaining Financially Healthy and Competitive: The Role of Financial Predictors. J. Compet. 2020, 12, 74–92. [Google Scholar] [CrossRef]
- Oranburg, S.C. A History of Financial Technology and Regulation: From American Incorporation to Cryptocurrency and Crowdfunding, 1st ed.; Cambridge University Press: Cambridge, UK, 2022. [Google Scholar] [CrossRef]
- Barbu, C.M.; Florea, D.L.; Dabija, D.C.; Barbu, M.C.R. Customer Experience in Fintech. J. Theor. Appl. Electron. Commer. Res. 2021, 16, 1415–1433. [Google Scholar] [CrossRef]
- Evgenidis, A.; Papadamou, S.; Siriopoulos, C. The yield spread’s ability to forecast economic activity: What have we learned after 30 years of studies? J. Bus. Res. 2020, 106, 221–232. [Google Scholar] [CrossRef]
- Molnár, A.; Csiszárik-Kocsir, Á. Forecasting Economic Growth with the Hungarian Composite Stock Market Index—A Granger Causality Test. Acta Polytech. Hung. 2022, 19, 205–227. [Google Scholar] [CrossRef]
- Molnár, A.; Csiszárik-Kocsir, Á. Forecasting Economic Growth with V4 Countries’ Composite Stock Market Indexes—A Granger Causality Test. Acta Polytech. Hung. 2023, 20, 135–154. [Google Scholar] [CrossRef]
- Asteriou, D.; Lalountas, D.A.; Siriopoulos, C. A Small Macro-Econometric Model for Greece: Implications about the Sustainability of the Greek External Debt. SSRN J. 2011, 1–33. [Google Scholar] [CrossRef]
- Friedman, M. Money and Economic Development; Lexington Books: Toronto, ON, Canada, 1973. [Google Scholar]
- Fischer, S. The role of macroeconomic factors in growth. J. Monet. Econ. 1993, 32, 485–512. [Google Scholar] [CrossRef]
- Barro, R.J. Inflation and Growth. Fed. Reserve Bank St. Louis Rev. 1996, 78, 153–169. [Google Scholar] [CrossRef]
- Andres, J.; Hernando, I. Does Inflation Harm Economic Growth? Evidence for the OECD. In The Costs and Effects of Price Stability; Feldstein, M., Ed.; Working Paper 6062; The University of Chicago Press: Chicago, IL, USA, 1999; pp. 315–341. [Google Scholar] [CrossRef]
- Bruno, M.; Easterly, W. Inflation crises and long-run growth. J. Monet. Econ. 1998, 41, 3–26. [Google Scholar] [CrossRef]
- Mallik, G.; Chowdhury, A. Inflation and Economic Growth: Evidence from Four South Asian Countries. Asia-Pac. Dev. J. 2001, 8, 123–135. [Google Scholar]
- Ito, T.; Sato, K. Exchange Rate Changes and Inflation in Post-Crisis Asian Economies: Vector Autoregression Analysis of the Exchange Rate Pass-Through. J. Money Credit. Bank. 2008, 40, 1407–1438. [Google Scholar] [CrossRef]
- Chiu, P. From Inflation to Deflation: A Novel Experience for Hong Kong: To speed up its economic recovery, the Special Administrative Region is betting on a more intense economic integration with mainland China. China Perspect. 2003, 6, 1–16. [Google Scholar] [CrossRef]
- Kremer, S.; Bick, A.; Nautz, D. Inflation and growth: New evidence from a dynamic panel threshold analysis. Empir. Econ. 2013, 44, 861–878. [Google Scholar] [CrossRef]
- Ekinci, R.; Tüzün, O.; Ceylan, F. The relationship between inflation and economic growth: Experiences of some inflation targeting countries. Financ. Stud. 2020, 24, 6–20. [Google Scholar]
- Chen, W.; Mrkaic, M.; Nabar, M. The Global Economic Recovery 10 Years after the 2008 Financial Crisis; IMF Working Papers; Working Paper No. 2019/083; IMF: Washington, DC, USA, 2019. [Google Scholar]
- Lane, P.R. Low Inflation: Macroeconomic Risks and the Monetary Policy Stance. Presented at the Financial Markets Workshop of the Economic Council (Finanzmarktklausur des Wirtschaftsrats der CDU), Berlin, Germany, 11 February 2020; Available online: https://www.ecb.europa.eu/press/key/date/2020/html/ecb.sp200211_2~eae18c54ff.en.html (accessed on 12 July 2023).
- Arlt, J.; Arltova, M. Forecasting of the Annual Inflation Rate in the Unstable Economic Conditions. WSEAS Trans. Bus. Econ. 2016, 12, 410–415. [Google Scholar] [CrossRef]
- Minsky, H.P. The Financial Instability Hypothesis; Working Papers No. 74; Levy Economics Institute: Annandale-On-Hudson, NY, USA, 1992. [Google Scholar]
- Akaev, A.A.; Sadovnichiy, V.A. Forecasting a Cyclical Downturn (Recession) in the US Economy Using a Mathematical Model of Hyman Minsky’s Theory of Financial Instability. Dokl. Math. 2020, 102, 422–426. [Google Scholar] [CrossRef]
- ECLAC. United States Economic Outlook: 2022 Year-in-Review and Early 2023 Developments; LC/WAS/TS.2023/2; Economic Commission for Latin America and the Caribbean: Santiago, Chile, 2022. [Google Scholar]
- Asada, T. Mathematical Modeling of Financial Instability and Macroeconomic Stabilization Policies. In Nonlinear Economic Dynamics and Financial Modelling; Dieci, R., He, X.Z., Hommes, C., Eds.; Discussion Paper No. 219; Springer: Cham, Switzerland, 2014; pp. 41–63. [Google Scholar]
- Tsvetkov, T.; Georgieva, S. Inflation, Inflation Instability and Nominal Uncertainty in Bulgarian Economy. Econ. Stud. 2022, 8, 41–64. [Google Scholar]
- Akaev, A.A.; Ziyadullaev, N.S.; Sarygulov, A.I.; Sokolov, V.N. Medium-term forecast of the dynamics of the development of the Russian economy. Stud. Russ. Econ. Dev. 2016, 27, 510–517. [Google Scholar] [CrossRef]
- Akaev, A.A.; Sarygulov, A.I.; Sokolov, V.N. Mathematical models of economic dynamics in the context of high inflation and unstable development. Dokl. Math. 2015, 92, 757–760. [Google Scholar] [CrossRef]
- Akaev, A.A.; Ziyadullaev, N.S.; Sarygulov, A.I.; Sokolov, V.N. A predictive model of economic dynamics during stagflation taking into account the volatility of the national currency. Stud. Russ. Econ. Dev. 2017, 28, 266–270. [Google Scholar] [CrossRef]
- Ascari, G.; Bonomolo, P.; Hoeberichts, M.; Trezzi, R. The Euro Area Great Inflation Surge. SUERF–The European Money and Finance Forum Policy Brief No. 548. 2023. Available online: https://www.dnb.nl/media/ijjmy42c/dnb-analysis-the-euro-area-great-inflation-surge.pdf (accessed on 13 September 2023).
- Benigno, P.; Canofari, P.; Bartolomeo, G.D.; Messori, M. Inflation Dynamics and Monetary Policy in the Euro Area; Monetary Dialogue Papers; European Parliament: Strasbourg, France, 2023; pp. 1–33. [Google Scholar]
- Jobst, A.; Krizan, P.; Utermöhl, K. Eurozone Inflation: How Bad Can It Get in 2022? 2022. Available online: https://www.allianz-trade.com/content/dam/onemarketing/aztrade/allianz-trade_com/en_gl/erd/publications/the-watch/2022_05_03EZ_inflation_AZT.pdf (accessed on 20 August 2023).
- ECB. Economic, financial and monetary developments. Econ. Bull. 2023, 4, 1–144. Available online: https://www.ecb.europa.eu/pub/economic-bulletin/html/eb202304.en.html (accessed on 20 June 2023).
- Baba, C.; Duval, R.; Lan, T.; Topalova, P. The Inflation Surge in Europe; IMF Working Paper; Working Paper No. 2023/030; IMF: Washington, DC, USA, 2023; pp. 1–22. [Google Scholar]
- Sadovnichiy, V.A.; Akaev, A.A.; Zvyagintsev, A.I.; Sarygulov, A.I. Refined Mathematical Model of Economic Dynamics under High Inflation and Unstable Development. Dokl. Math. 2023, 107, 105–108. [Google Scholar] [CrossRef]
- Romer, R. Advanced Macroeconomics, 4th ed.; The McGraw-Hill Series in Economics; McGraw-Hill/Irwin: New York, NY, USA, 2012. [Google Scholar]
- Cagan, P. The Monetary Dynamics of Hyperinflation. In Studies in the Quantity Theory of Money; Friedman, M., Ed.; The University of Chicago Press: Chicago, IL, USA, 1956; pp. 22–117. [Google Scholar]
- Bruno, M.; Fischer, S. Seigniorage, Operating Rules and The High Inflation Trap. Q. J. Econ. 1990, 105, 353–374. [Google Scholar] [CrossRef]
- ECB. Economic Bulletin. September 2023. Available online: https://www.ecb.europa.eu/pub/economic-bulletin/html/all_releases.en.html (accessed on 28 July 2023).
- European Commission. Statistical Annex, European Economic Forecast, Spring 2023, European Commission, Statistical Annex. 2023. Available online: https://economy-finance.ec.europa.eu/system/files/2023-05/SF_2023_Statistical%20Annex.pdf (accessed on 25 June 2023).
- Schnabel, I. The Risks of Stubborn Inflation, Speech by Isabel Schnabel, Member of the Executive Board of the ECB, at the Euro50 Group Conference on “New Challenges for the Economic and Monetary Union in the Post-Crisis Environment”, Luxemburg, 19 June 2023. Available online: https://www.ecb.europa.eu/press/key/date/2023/html/ecb.sp230619_1~2c0bdf2422.en.html (accessed on 24 June 2023).
- Noyan, O. Insolvencies on the Rise in Germany Amid Economic Downturn. Available online: https://www.euractiv.com/section/economy-jobs/news/insolvencies-on-the-rise-in-germany-amid-economic-downturn/ (accessed on 13 July 2023).
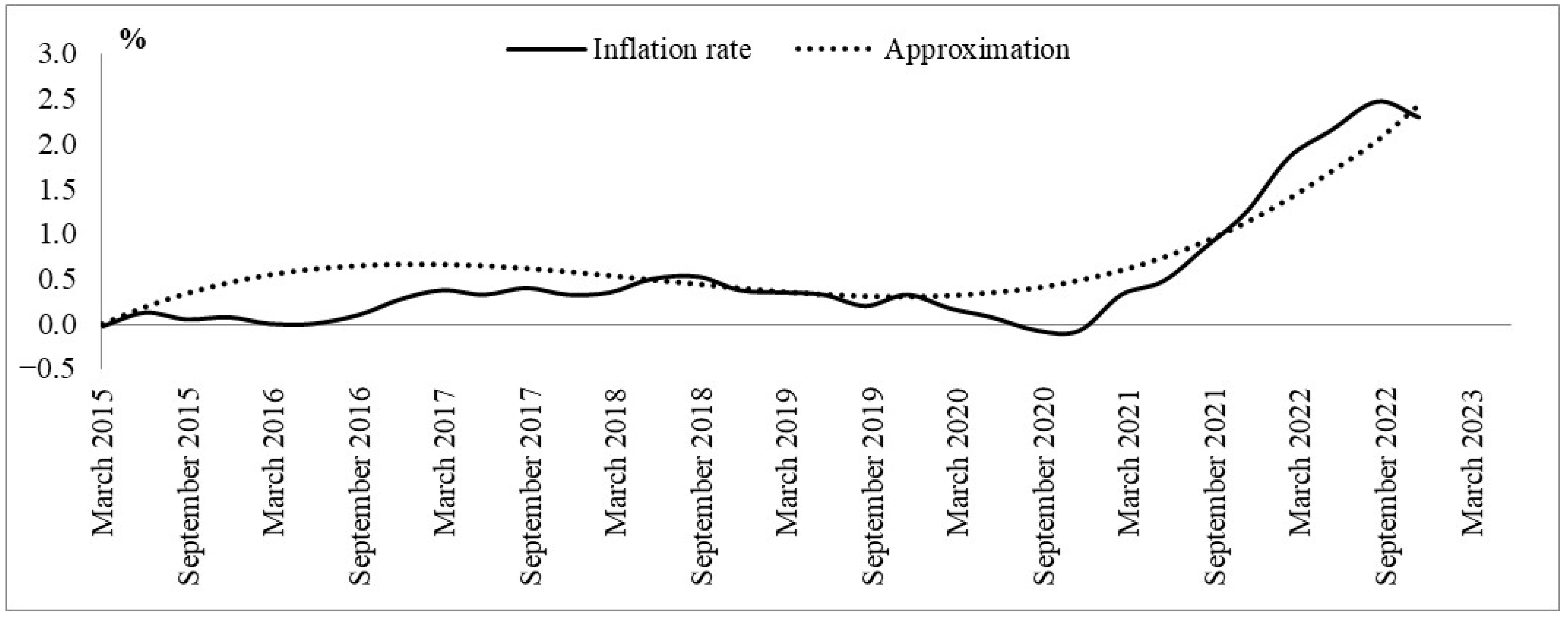


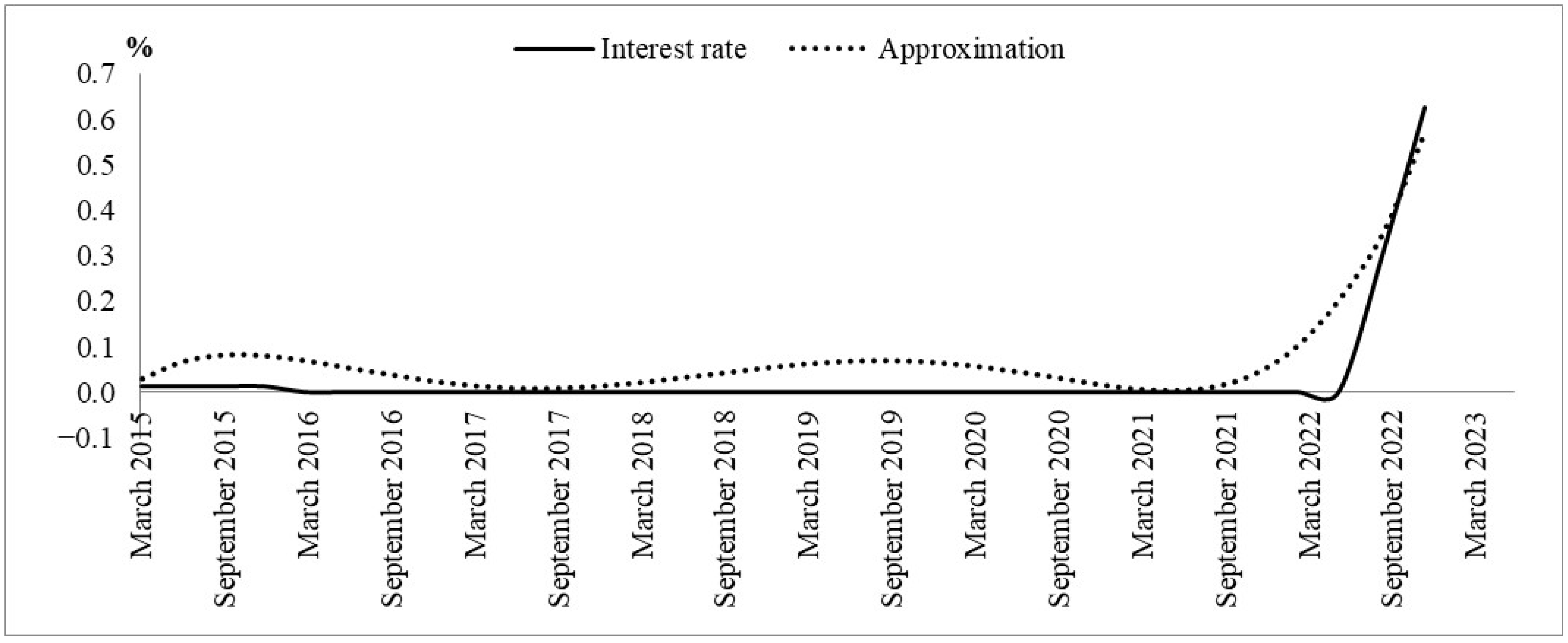
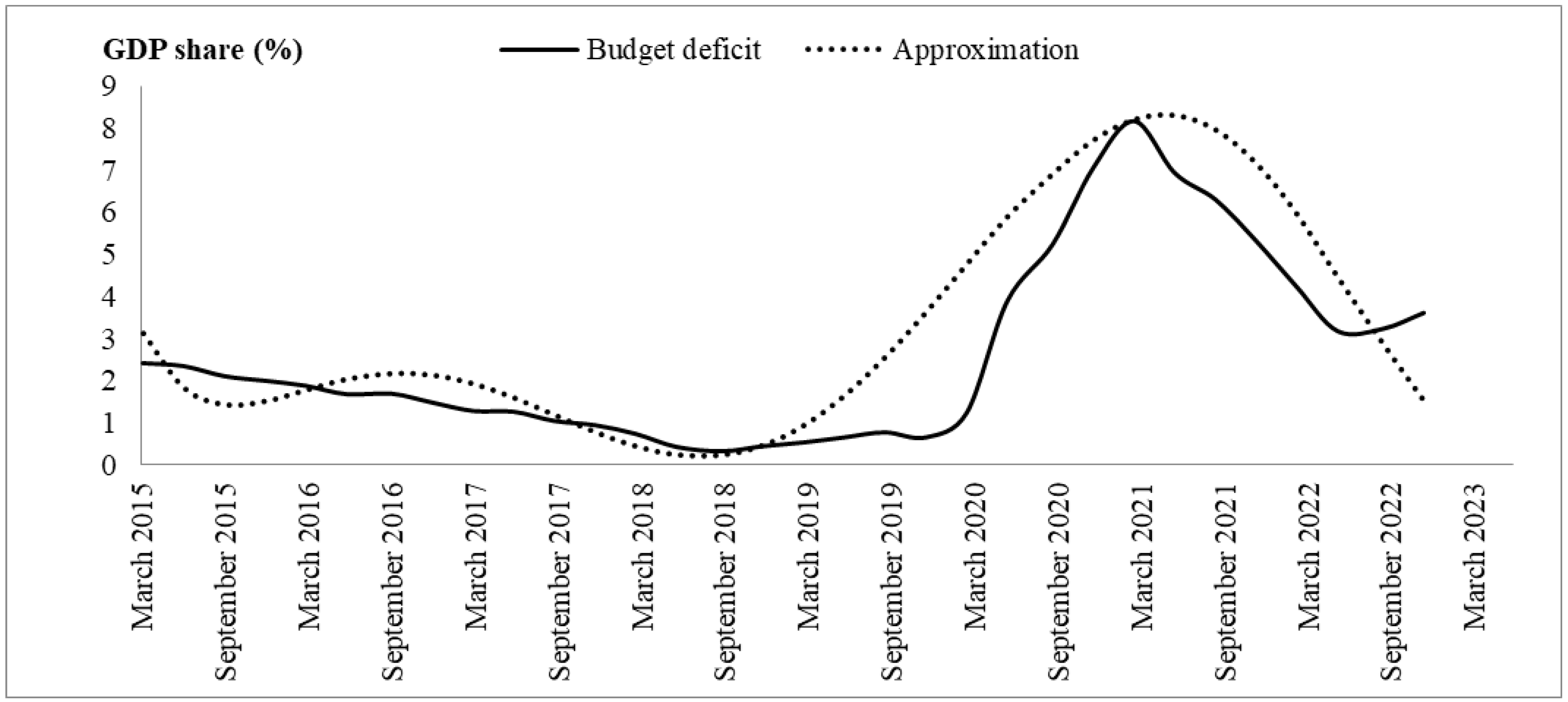

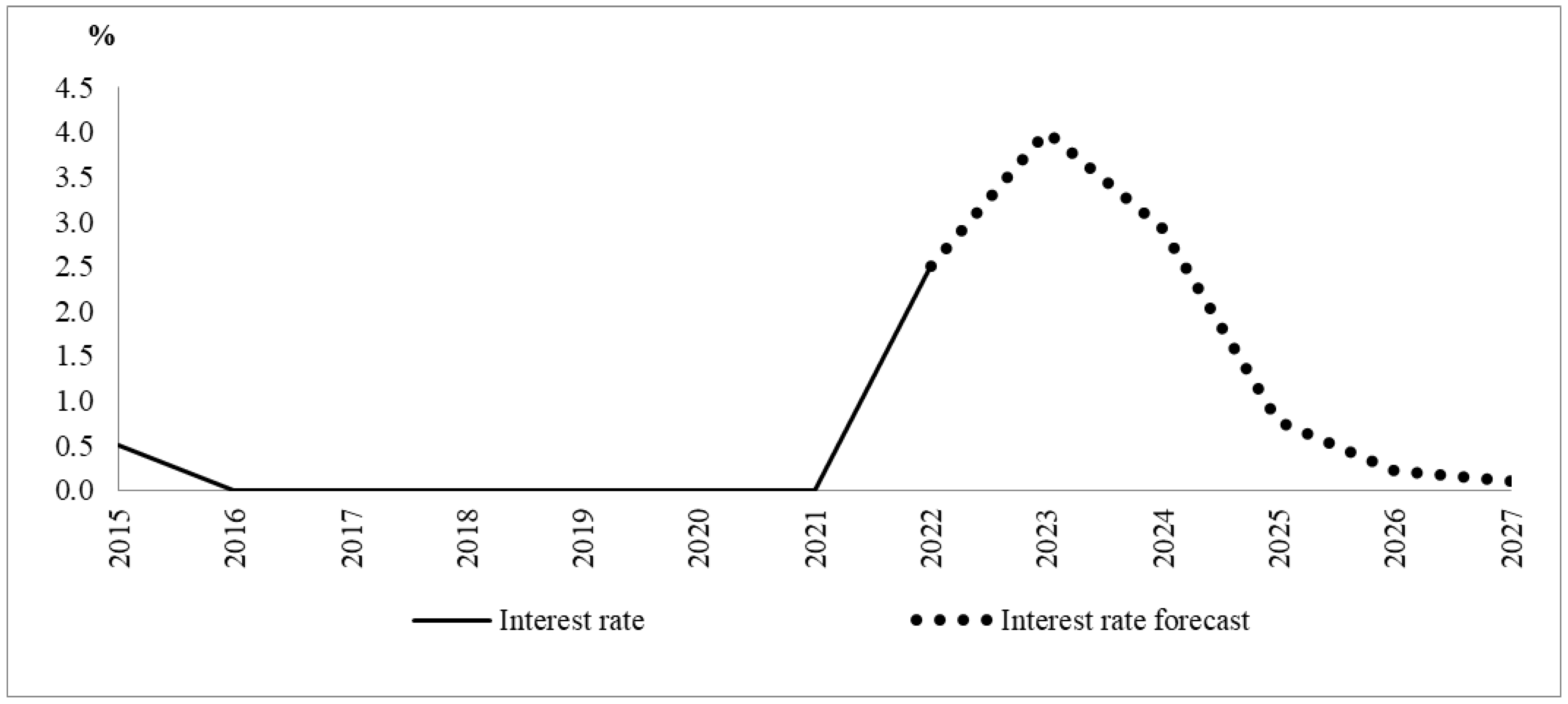
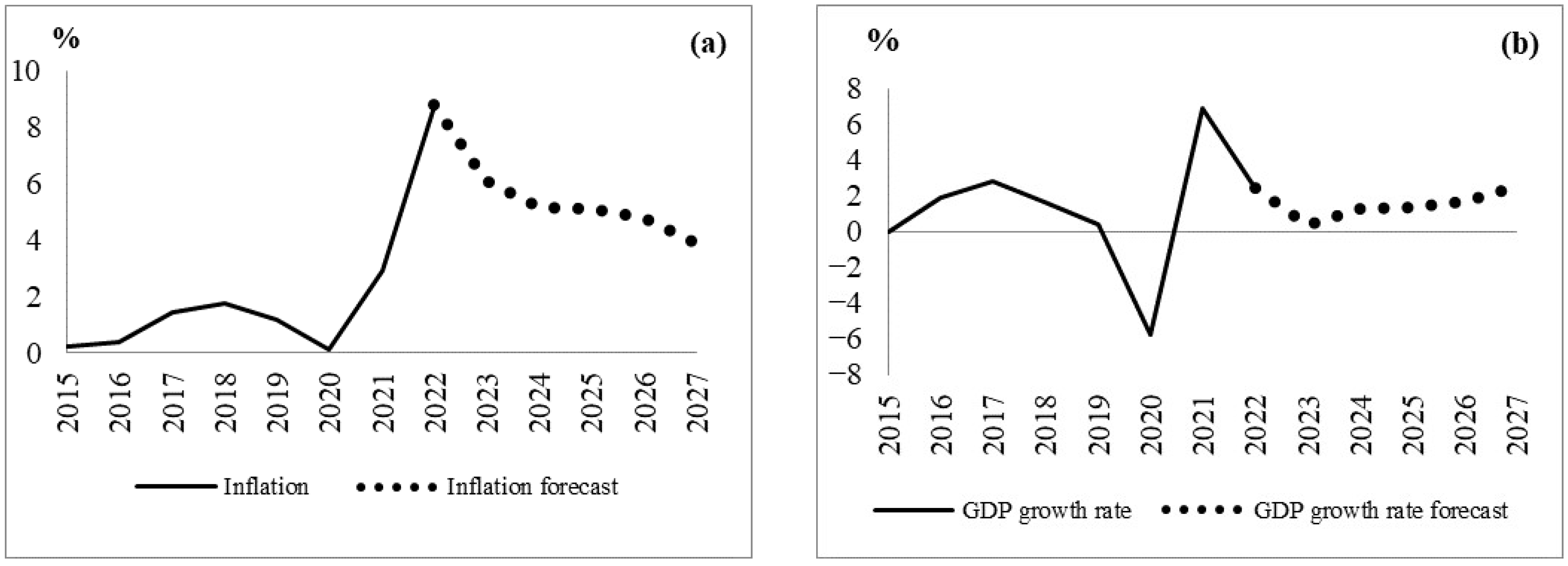
| Eurozone Forecasts | 2023 | 2024 |
|---|---|---|
| Average annual inflation rate (%) | 5.8 | 2.775 |
| GDP dynamics (%) | +0.95 | +1.425 |
| Main Indicators | 2023 | 2024 | 2025 | 2026 | 2027 |
|---|---|---|---|---|---|
| Money supply growth (%) | 0.06 | 0.05 | 0.05 | 0.06 | 0.06 |
| Interest rate (%) | 4.00 | 2.93 | 0.78 | 0.21 | 0.10 |
| Budget deficit (% of GDP) | 3.00 | 3.00 | 3.00 | 3.00 | 3.00 |
| Inflation (%) | 6.11 | 5.13 | 5.12 | 4.77 | 3.88 |
| GDP growth (%) | 0.34 | 1.32 | 1.34 | 1.69 | 2.58 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Akaev, A.; Zvyagintsev, A.; Devezas, T.; Sarygulov, A.; Tick, A. Mathematical Models for Forecasting Unstable Economic Processes in the Eurozone. Mathematics 2023, 11, 4544. https://doi.org/10.3390/math11214544
Akaev A, Zvyagintsev A, Devezas T, Sarygulov A, Tick A. Mathematical Models for Forecasting Unstable Economic Processes in the Eurozone. Mathematics. 2023; 11(21):4544. https://doi.org/10.3390/math11214544
Chicago/Turabian StyleAkaev, Askar, Alexander Zvyagintsev, Tessaleno Devezas, Askar Sarygulov, and Andrea Tick. 2023. "Mathematical Models for Forecasting Unstable Economic Processes in the Eurozone" Mathematics 11, no. 21: 4544. https://doi.org/10.3390/math11214544
APA StyleAkaev, A., Zvyagintsev, A., Devezas, T., Sarygulov, A., & Tick, A. (2023). Mathematical Models for Forecasting Unstable Economic Processes in the Eurozone. Mathematics, 11(21), 4544. https://doi.org/10.3390/math11214544








