Fixed/Preassigned-Time Synchronization of Fully Quaternion-Valued Cohen–Grossberg Neural Networks with Generalized Time Delay
Abstract
:1. Introduction
- (1)
- (2)
- Compared with previous research on the synchronization issue of QVNNs [27,28], the synchronization problem of CGQVNNs with discontinuous activation functions is investigated for the first time. An effective analytical method is introduced to investigate the FIT synchronization and PET synchronization of CGQVNNs with quaternion-valued amplification function without separation, and high-precision ST is estimated.
- (3)
- Different from the methods used in [28], the FIT synchronization and PET synchronization of CGQVNNs are discussed through a direct analytical approach. Consequently, several effective quaternion-valued controllers are directly designed for the original CGQVNNs rather than for the four real-valued subsystems obtained by separation, so as to obtain more economical control gains and derive more concise synchronization conditions.
2. Model Description and Preliminaries
- (i)
- If then for where
- (ii)
- If and then for wherewhere , , the incomplete Beta function ratio was given in [43].
- (1)
- .
- (2)
- .
- (3)
- .
- (4)
- .
- (1)
- ,
- (2)
- .
3. Fixed-Time Synchronization
- (1)
- (2)
- (1)
- (2)
- (1)
- (2)
- (1)
- (2)
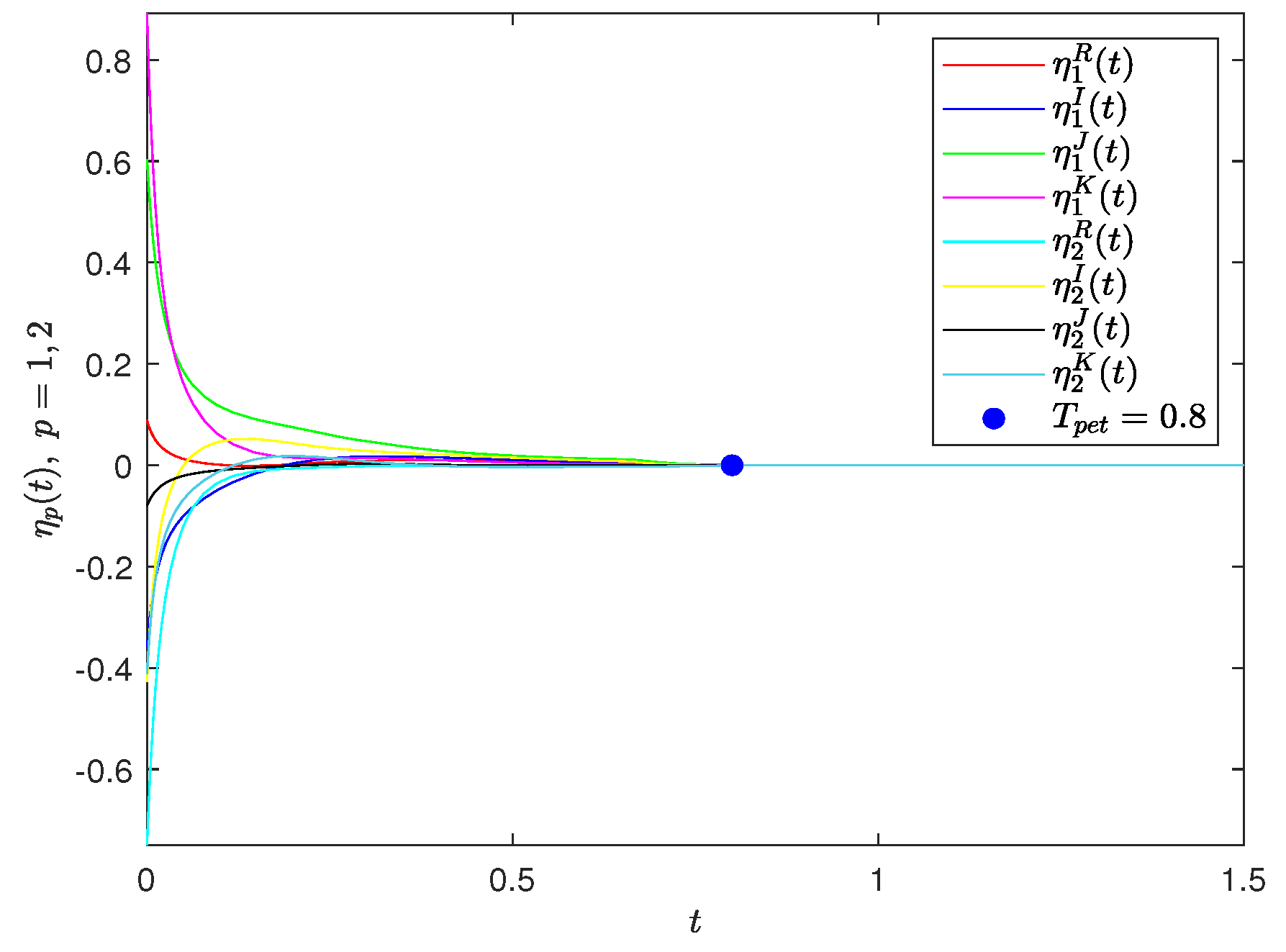
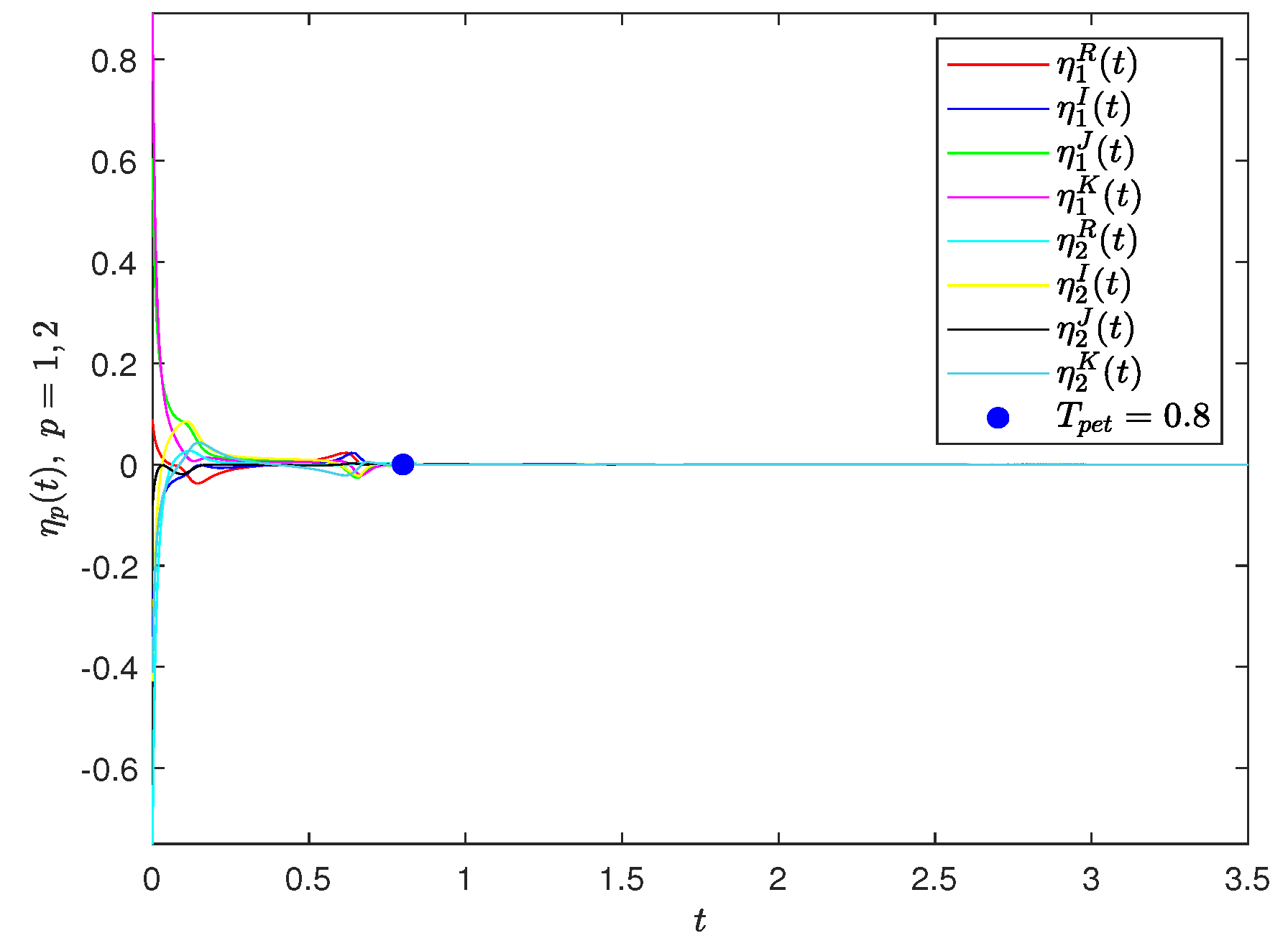
4. Preassigned-Time Synchronization
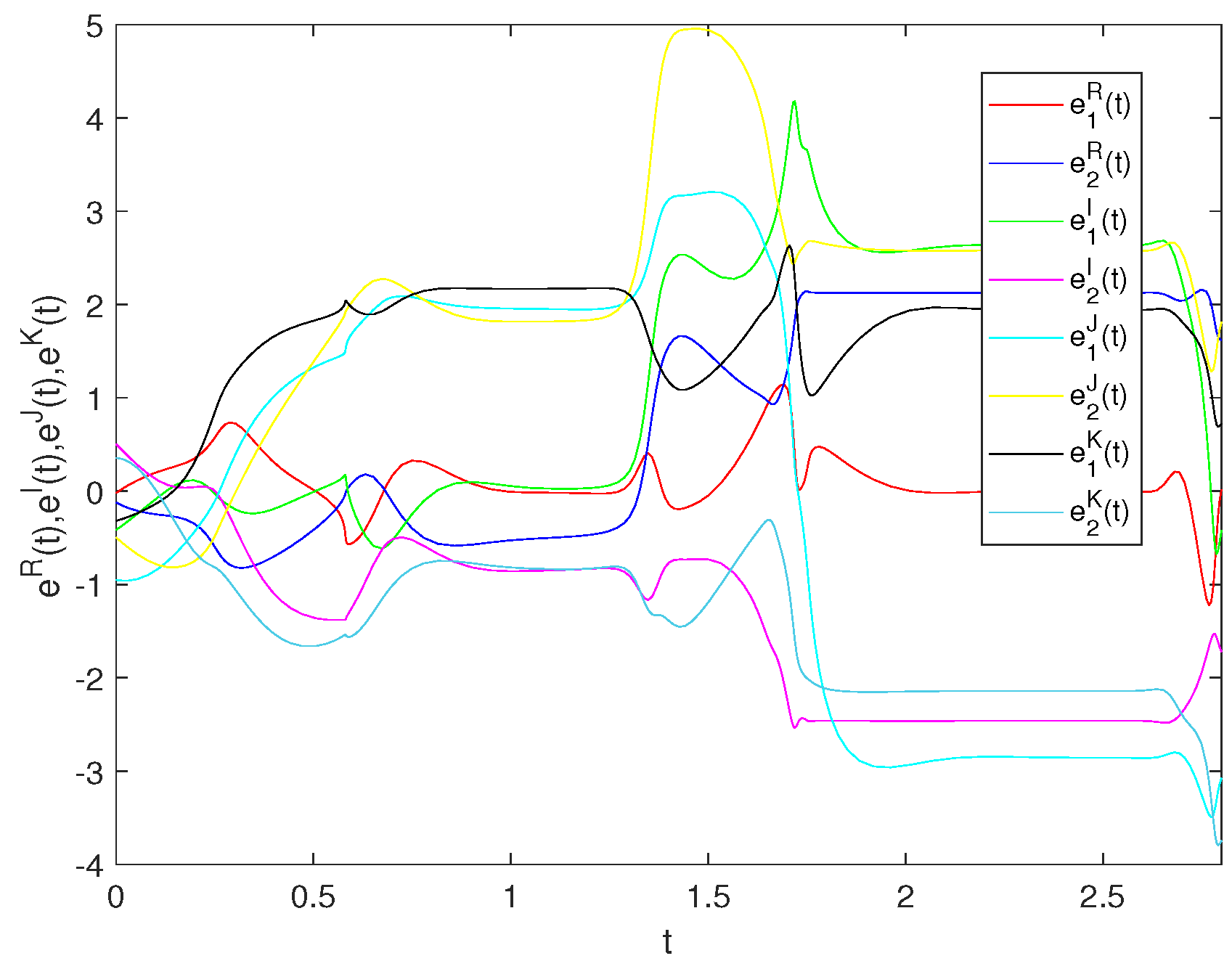
5. Numerical Simulations
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Miron, S.; Flamant, J.; Bihan, N.L.; Chainais, P.; Brie, D. Quaternions in Signal and Image Processing: A comprehensive and objective overview. IEEE Signal Process. Mag. 2023, 40, 26–40. [Google Scholar] [CrossRef]
- Guizzo, E.; Weyde, T.; Comminiello, D. Learning speech emotion representations in the quaternion domain. IEEE/Acm Trans. Audio Speech Lang. Process. 2023, 31, 1200–1212. [Google Scholar] [CrossRef]
- Bayro-Corrochano, E.; Lechuga-Gutierrez, L.; Garza-Burgos, M. Geometric techniques for robotics and HMI: Interpolation and haptics in conformal geometric algebra and control using quaternion spike neural networks. Robot. Auton. Syst. 2018, 104, 72–84. [Google Scholar] [CrossRef]
- Zhang, H.; Wang, Z.; Chen, D.; Zhu, S.; Xu, D. Quaternion Extreme Learning Machine Based on Real Augmented Representation. IEEE Signal Process. Lett. 2023, 30, 175–179. [Google Scholar] [CrossRef]
- Zhou, Y.; Jin, L.; Ma, G.; Xu, X. Quaternion capsule neural network with region attention for facial expression recognition in color images. IEEE Trans. Emerg. Top. Comput. Intell. 2022, 6, 893–911. [Google Scholar] [CrossRef]
- Liu, J.; Liao, X.; Dong, J. A quaternion-valued neural network approach to nonsmooth nonconvex nonstrained optimization in quaternion domain. IEEE Trans. Emerg. Top. Comput. Intell. 2023, 1–16. [Google Scholar] [CrossRef]
- Aouiti, C.; Touati, F. Global dissipativity of quaternion-valued fuzzy cellular fractional-order neural networks with time delays. Neural Process. Lett. 2023, 55, 481–503. [Google Scholar] [CrossRef]
- Li, R.; Cao, J. Dissipativity and synchronization control of quaternion-valued fuzzy memristive neural networks: Lexicographical order method. Fuzzy Sets Syst. 2021, 443, 70–89. [Google Scholar] [CrossRef]
- Song, Q.; Yang, L.; Liu, Y.; Alsaadi, F. Stability of quaternion-valued neutral-type neural networks with leakage delay and proportional delays. Neurocomputing 2022, 521, 191–198. [Google Scholar] [CrossRef]
- Rezaie, A.; Mobayen, S.; Ghaemi, M.; Fekih, A.; Zhilenkov, A. Design of a Fixed-Time Stabilizer for Uncertain Chaotic Systems Subject to External Disturbances. Mathematics 2023, 15, 3273. [Google Scholar] [CrossRef]
- Zhang, Z.; Yang, Z. Asymptotic stability for quaternion-valued fuzzy BAM neural networks via integral inequality approach. Chaos Solitons Fractals 2023, 169, 113227. [Google Scholar] [CrossRef]
- Wang, J.; Zhu, S.; Liu, X.; Wen, S. Mittag-Leffler stability of fractional-order quaternion-valued memristive neural networks with generalized piecewise constant argument. Neural Net. 2023, 162, 175–185. [Google Scholar] [CrossRef] [PubMed]
- Singh, S.; Das, S.; Chouhan, S.; Cao, J. Anti-synchronization of inertial neural networks with quaternion-valued and unbounded delays: Non-reduction and non-separation approach. Knowl.-Based Syst. 2023, 278, 110903. [Google Scholar] [CrossRef]
- Zhao, M.; Li, H.; Zhang, L.; Hu, C.; Jiang, H. Quasi-synchronization of discrete-time fractional-order quaternion-valued memristive neural networks with time delays and uncertain parameters. Appl. Math. Comput. 2023, 453, 128095. [Google Scholar] [CrossRef]
- Xiao, J.; Cao, J.; Cheng, J.; Zhong, S.; Wen, S. Novel methods to finite-time Mittag-Leffler synchronization problem of fractional-order quaternion-valued neural networks. Inf. Sci. 2020, 526, 221–244. [Google Scholar] [CrossRef]
- Ping, J.; Zhu, S.; Shi, M.; Wu, S.; Shen, M.; Liu, X.; Wen, S. Event-triggered finite-time synchronization control for quaternion-valued memristive neural networks by an non-decomposition method. IEEE Trans. Netw. Sci. Eng. 2023, 10, 3609–3619. [Google Scholar] [CrossRef]
- Polyakov, A. Nonlinear feedback design for fixed-time stabilization of linear control systems. IEEE Trans. Autom. Control. 2012, 57, 2106–2110. [Google Scholar] [CrossRef]
- Sadik, H.; Abdurahman, A.; Tohti, R. Fixed-time synchronization of reaction-diffusion fuzzy neural networks with stochastic perturbations. Mathematics 2023, 6, 1493. [Google Scholar] [CrossRef]
- Yang, W.; Xiao, L.; Huang, J.; Yang, J. Fixed-time synchronization of neural networks based on quantized intermittent control for image protection. Mathematics 2021, 23, 3086. [Google Scholar] [CrossRef]
- Pang, L.; Hu, C.; Yu, J.; Jiang, H. Fixed-time synchronization for fuzzy-based impulsive complex networks. Mathematics 2022, 9, 1533. [Google Scholar] [CrossRef]
- Pu, H.; Li, F. Fixed/predefined-time synchronization of complex-valued discontinuous delayed neural networks via non-chattering and saturation control. Physical A 2022, 610, 128425. [Google Scholar] [CrossRef]
- Wu, E.; Wang, Y.; Li, Y.; Li, K.; Luo, F. Fixed-Time Synchronization of Complex-Valued Coupled Networks with Hybrid Perturbations via Quantized Control. Mathematics 2023, 18, 3845. [Google Scholar] [CrossRef]
- Cheng, Y.; Hu, T.; Xu, W.; Zhang, X.; Zhong, S. Fixed-time synchronization of fractional-order complex-valued neural networks with time-varying delay via sliding mode control. Neurocomputing 2022, 505, 339–352. [Google Scholar] [CrossRef]
- Deng, H.; Bao, H. Fixed-time synchronization of quaternion-valued neural networks. Physical A 2019, 527, 121351. [Google Scholar] [CrossRef]
- Wei, R.; Cao, J. Fixed-time synchronization of quaternion-valued memristive neural networks with time delays. Neural Net. 2019, 113, 1–10. [Google Scholar] [CrossRef] [PubMed]
- Wei, W.; Yu, J.; Wang, L.; Hu, C.; Jiang, H. Fixed/Preassigned-time synchronization of quaternion-valued neural networks via pure power-law control. Neural Net. 2021, 146, 341–349. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Yang, L.; Kou, K.; Liu, Y. Fixed-time synchronization for quaternion-valued memristor-based neural networks with mixed delays. Neural Net. 2023, 165, 274–289. [Google Scholar] [CrossRef]
- Peng, T.; Wu, Y.; Tu, Z.; Alofi, A.; Lu, J. Fixed-time and prescribed-time synchronization of quaternion-valued neural networks: A control strategy involving Lyapunov functions. Neural Net. 2023, 160, 108–121. [Google Scholar] [CrossRef]
- Li, X.; Wu, H.; Cao, J. Prescribed-time synchronization in networks of piecewise smooth systems via a nonlinear dynamic event-triggered control strategy. Math. Comput. Simul. 2022, 203, 647–668. [Google Scholar] [CrossRef]
- Liu, X.; Ho, D.; Xie, C. Prespecified-time cluster synchronization of complex networks via a smooth control approach. IEEE Trans. Cybern. 2020, 50, 1771–1775. [Google Scholar] [CrossRef]
- Shao, S.; Liu, X.; Cao, J. Prespecified-time synchronization of switched coupled neural networks via smooth controllers. Neural Net. 2020, 133, 32–39. [Google Scholar] [CrossRef]
- Yang, J.; Chen, G.; Zhu, S.; Wen, S.; Hu, J. Fixed/prescribed-time synchronization of BAM memristive neural networks with time-varying delays via convex analysis. Neural Net. 2023, 163, 53–63. [Google Scholar] [CrossRef] [PubMed]
- Tan, F.; Zhou, L.; Xia, J. Adaptive quantitative exponential synchronization in multiplex Cohen–Grossberg neural networks under deception attacks. J. Frankl. Inst. 2022, 359, 10558–10577. [Google Scholar] [CrossRef]
- Jamal, M.; Kumar, R.; Mukhopadhyay, S.; Kwon, O. Fixed-time stability of Cohen–Grossberg BAM neural networks with impulsive perturbations. Neurocomputing 2023, 550, 126501. [Google Scholar] [CrossRef]
- Kong, F.; Zhu, Q.; Karimi, H. Fixed-time periodic stabilization of discontinuous reaction-diffusion Cohen–Grossberg neural networks. Neural Net 2023, 166, 354–365. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Chen, X.; Ye, R.; Stamova, I.; Cao, J. Adaptive quasi-synchronization analysis for Caputo delayed Cohen–Grossberg neural networks. Math. Comput. Simul. 2023, 212, 49–65. [Google Scholar] [CrossRef]
- Clarke, F. Optimization and Nonsmooth Analysis; Wiley: New York, NY, USA, 1983. [Google Scholar]
- Filippov, A. Differential Equations with Discontinuous Right-Hand Sides; Kluwer: Dordrecht, The Netherlands, 1988. [Google Scholar]
- Huang, L.; Guo, Z.; Wang, J. Theory and Applications of Differential Equations with Discontinuous Right-Hand Sides; Science Press: Beijing, China, 2011. [Google Scholar]
- Aubin, J.; Frankowska, H. Set-Valued Analysis; Birkhäuser: Boston, MA, USA, 1990. [Google Scholar]
- Wei, W.; Hu, C.; Yu, J.; Jiang, H. Fixed/preassigned-time synchronization of quaternion-valued neural networks involving delays and discontinuous activations: A direct approach. Acta Math. Sci. 2022, 43, 1439–1461. [Google Scholar] [CrossRef]
- Liu, H.; Cheng, J.; Cao, J.; Katib, I. Preassigned-time synchronization for complex-valued memristive neural networks with reaction-diffusion terms and Markov parameters. Neural Net. 2023, 169, 520–531. [Google Scholar] [CrossRef]
- Hu, C.; He, H.; Jiang, H. Fixed/Preassigned-time synchronization of complex networks via improving fixed-time stability. IEEE Trans. Cybern. 2021, 51, 2882–2892. [Google Scholar] [CrossRef]
- Li, Z.; Liu, X. Finite time anti-synchronization of quaternion-valued neural networks with asynchronous time-varying delays. Neural Process. Lett. 2020, 52, 2253–2274. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jia, S.; Hu, C.; Jiang, H. Fixed/Preassigned-Time Synchronization of Fully Quaternion-Valued Cohen–Grossberg Neural Networks with Generalized Time Delay. Mathematics 2023, 11, 4825. https://doi.org/10.3390/math11234825
Jia S, Hu C, Jiang H. Fixed/Preassigned-Time Synchronization of Fully Quaternion-Valued Cohen–Grossberg Neural Networks with Generalized Time Delay. Mathematics. 2023; 11(23):4825. https://doi.org/10.3390/math11234825
Chicago/Turabian StyleJia, Shichao, Cheng Hu, and Haijun Jiang. 2023. "Fixed/Preassigned-Time Synchronization of Fully Quaternion-Valued Cohen–Grossberg Neural Networks with Generalized Time Delay" Mathematics 11, no. 23: 4825. https://doi.org/10.3390/math11234825
APA StyleJia, S., Hu, C., & Jiang, H. (2023). Fixed/Preassigned-Time Synchronization of Fully Quaternion-Valued Cohen–Grossberg Neural Networks with Generalized Time Delay. Mathematics, 11(23), 4825. https://doi.org/10.3390/math11234825