Numerical Study on the Orthogonality of the Fields Radiated by an Aperture
Abstract
:1. Introduction
2. Modal Expansion of the Far-Field Produced by a Radiating Aperture
3. Radiated Field Cross-Products Matrix
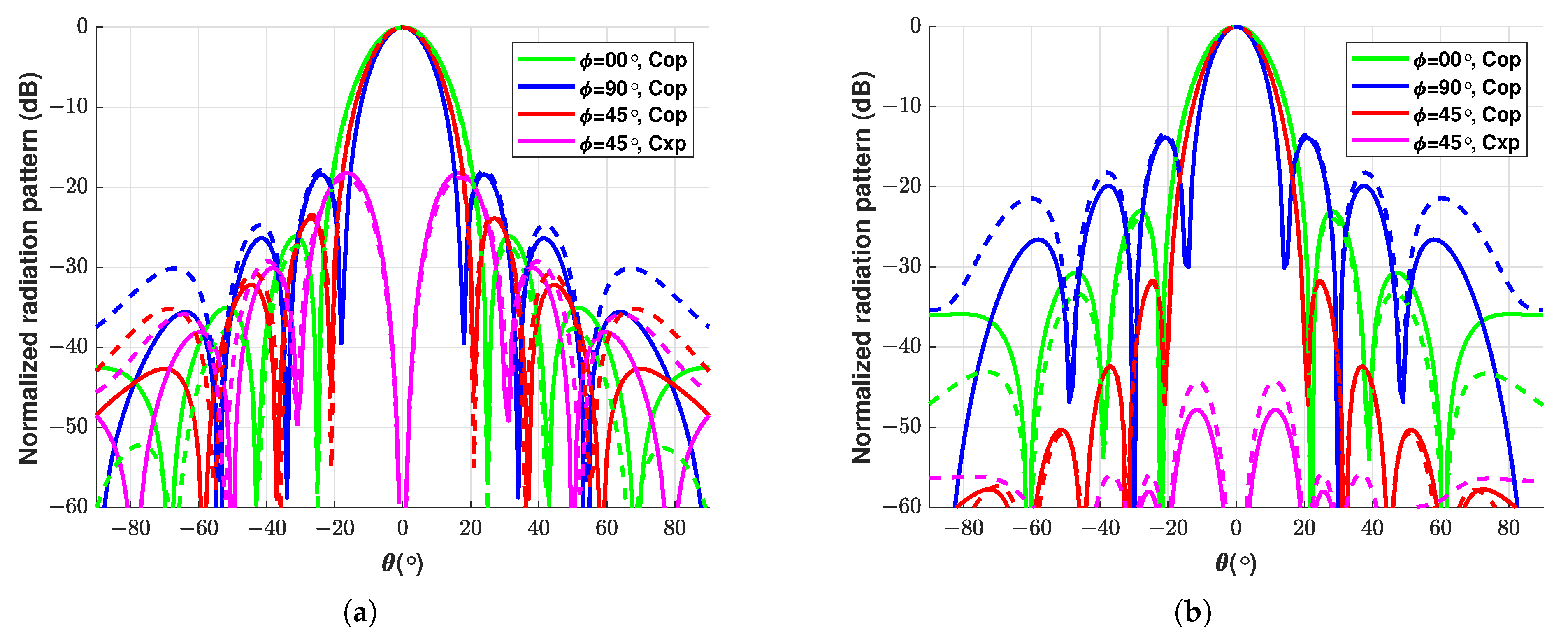
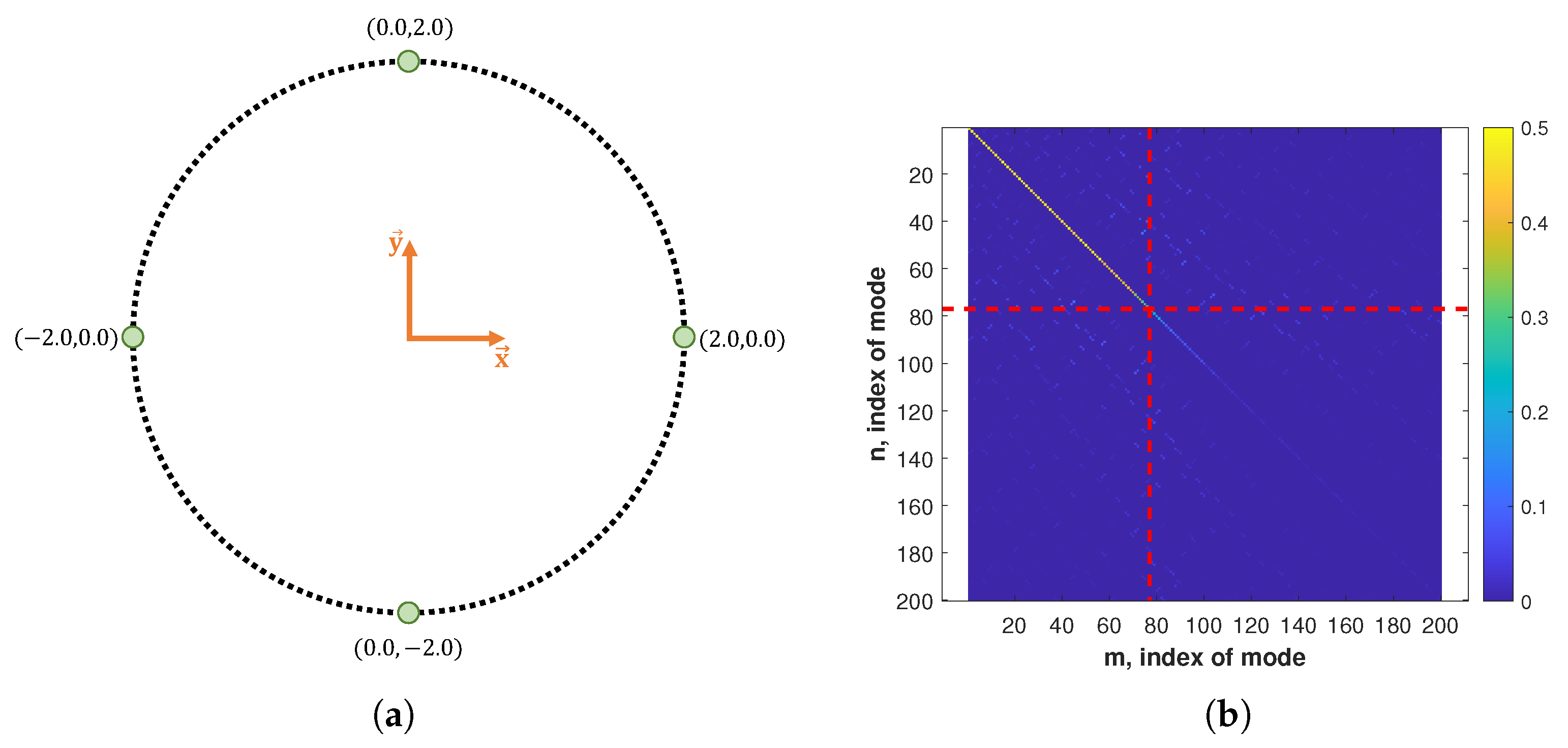
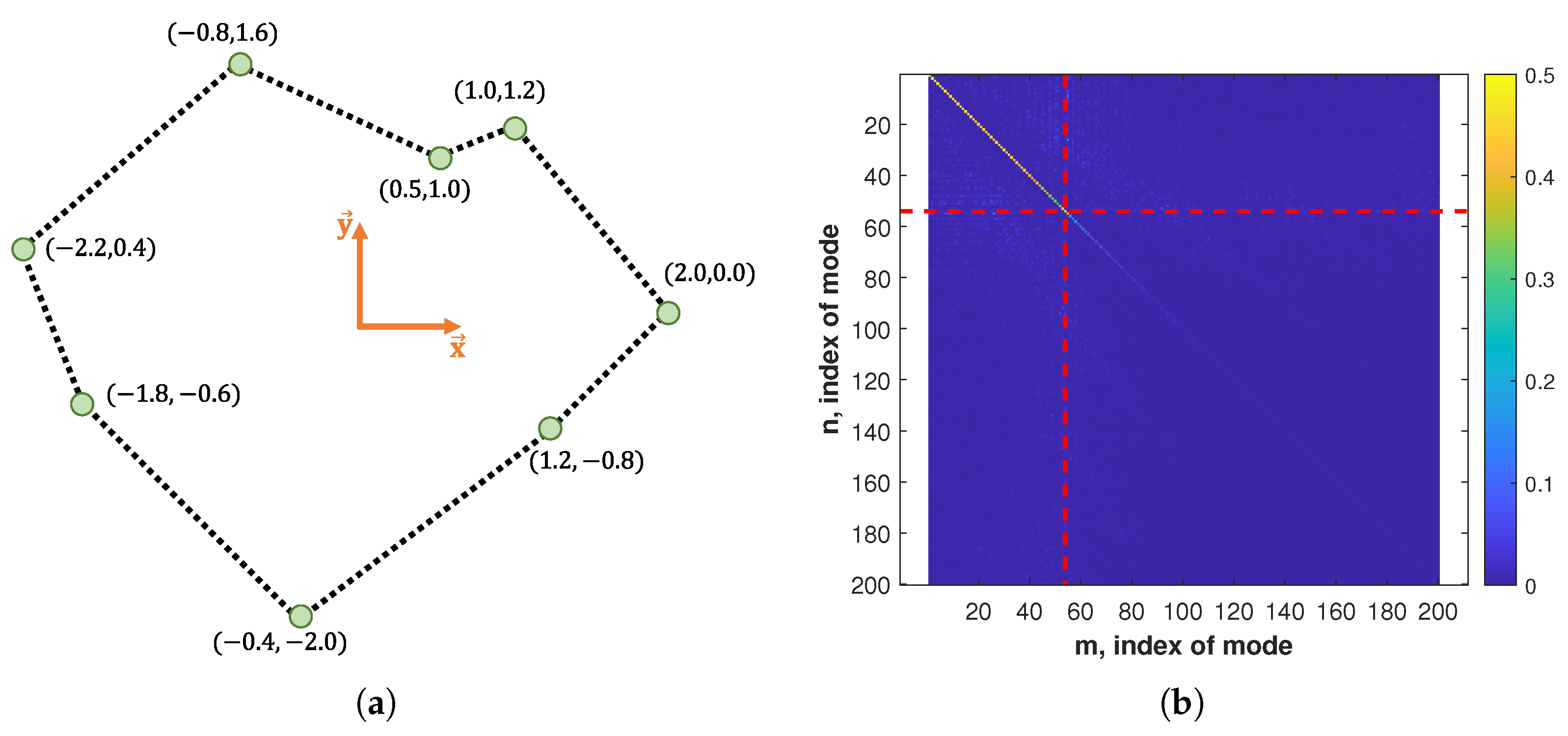
4. Numerical Study of the Orthogonality for the Radiated Fields of Each Mode
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Balanis, C. Antenna Theory: Analysis and Design; Wiley: Hoboken, NJ, USA, 2015. [Google Scholar]
- Shafai, L.; Sharma, S.; Rao, S. Handbook of Reflector Antennas and Feed Systems Volume II: Feed Systems; Antennas and Propagation, Artech House: Norwood, MA, USA, 2013. [Google Scholar]
- Bucci, O.M.; Isernia, T.; Morabito, A.F. Optimal Synthesis of Directivity Constrained Pencil Beams by Means of Circularly Symmetric Aperture Fields. IEEE Antennas Wirel. Propag. Lett. 2009, 8, 1386–1389. [Google Scholar] [CrossRef]
- Ghosh, S.; Lee-Yow, C. A multimode monopulse feed with equalized gain in sum and difference patterns. In Proceedings of the Antennas and Propagation Society Symposium 1991 Digest, London, ON, Canada, 24–28 June 1991; Volume 3, pp. 1832–1835. [Google Scholar] [CrossRef]
- Subbarao, B.; Fusco, V.F. Single aperture monopulse horn antenna. IEEE Microw. Wireless Compon. Lett. 2005, 15, 80–82. [Google Scholar] [CrossRef]
- Stoumpos, C.; Fraysse, J.P.; Goussetis, G.; Sauleau, R.; Legay, H. Quad-Furcated Profiled Horn: The Next Generation Highly Efficient GEO Antenna in Additive Manufacturing. IEEE Open J. Antennas Propag. 2022, 3, 69–82. [Google Scholar] [CrossRef]
- Morabito, A.F.; Di Donato, L.; Laganà, A.R.; Isernia, T.; Bucci, O.M. Recent advances in the optimal synthesis of multibeam satellite antennas. In Proceedings of the 2013 7th European Conference on Antennas and Propagation (EuCAP), Gothenburg, Sweden, 8–12 April 2013; pp. 3911–3912. [Google Scholar]
- Shen, R.; Ye, X.; Miao, J. Design of a Multimode Feed Horn Applied in a Tracking Antenna. IEEE Trans. Antennas Propag. 2017, 65, 2779–2788. [Google Scholar] [CrossRef]
- Bhattacharyya, A.K.; Goyette, G. A novel horn radiator with high aperture efficiency and low cross-polarization and applications in arrays and multibeam reflector antennas. IEEE Trans. Antennas Propag. 2004, 52, 2850–2859. [Google Scholar] [CrossRef]
- Savenko, P.O.; Tkach, M.D. The mathematical features of the synthesis of antennas with a flat aperture according to the prescribed amplitude directivity pattern. In Proceedings of the 2011 VIII International Conference on Antenna Theory and Techniques, Kyiv, Ukraine, 20–23 September 2011; pp. 247–249. [Google Scholar] [CrossRef]
- Andriychuk, M.I.; Kravchenko, V.F.; Savenko, P.O.; Tkach, M.D. Synthesis of plane radiating systems according to the prescribed power radiation pattern. In Proceedings of the 2013 IX Internatioal Conference on Antenna Theory and Techniques, Odessa, Ukraine, 16–20 September 2013; pp. 126–132. [Google Scholar] [CrossRef]
- Polo-López, L.; Córcoles, J.; Ruiz-Cruz, J.A.; Montejo-Garai, J.R.; Rebollar, J.M. On the Theoretical Maximum Directivity of a Radiating Aperture From Modal Field Expansions. IEEE Trans. Antennas Propag. 2019, 67, 2781–2786. [Google Scholar] [CrossRef]
- Polo-López, L.; Córcoles, J.; Ruiz-Cruz, J.A. Modal Field Synthesis of Monopulse Difference Patterns for Radiating Aperture. IEEE Trans. Antennas Propag. 2020, 68, 8203–8208. [Google Scholar] [CrossRef]
- Polo-López, L.; Córcoles, J.; Ruiz-Cruz, J.A. Contribution of the Evanescent Modes to the Power Radiated by an Aperture. In Proceedings of the 2021 IEEE MTT-S International Microwave Symposium (IMS), Atlanta, GA, USA, 6–11 June 2021; pp. 474–477. [Google Scholar] [CrossRef]
- Collin, R. Field Theory of Guided Waves; IEEE/OUP Series on Electromagnetic Wave Theory; IEEE Press: Piscataway, NJ, USA, 1991. [Google Scholar]
- Stutzman, W.L.; Thiele, G. Antenna Theory and Design; Wiley: Hoboken, NJ, USA, 1998. [Google Scholar]
- Olver, A.; Clarricoats, P.; Kishk, A.; Shafai, L. Microwave Horns and Feeds; Electromagnetic Waves Series; IET: London, UK; New York, NY, USA, 1994. [Google Scholar]
- Abramowitz, M.; Stegun, I. Handbook of Mathematical Functions; Dover Publications Inc.: New York, NY, USA, 1956. [Google Scholar]
- CST Microwave Studio 2020. Available online: https://www.cst.com/ (accessed on 21 February 2023).
- IEEE Std 145-2013 (Revision of IEEE Std 145-1993); IEEE Standard for Definitions of Terms for Antennas. IEEE: Piscataway, NJ, USA, 2014; pp. 1–50. [CrossRef]
- Lebedev, V.; Laikov, D. A quadrature formula for the sphere of the 131st algebraic order of accuracy. Dokl. Math. 1999, 59, 477–481. [Google Scholar]



| Circular | Squared | Polygonal | ||||||
|---|---|---|---|---|---|---|---|---|
| 1 | 0.146 | 0.484 | 1 | 0.125 | 0.480 | 1 | 0.154 | 0.482 |
| 2 | 0.146 | 0.484 | 2 | 0.125 | 0.480 | 2 | 0.186 | 0.485 |
| 3 | 0.191 | 0.459 | 3 | 0.177 | 0.461 | 3 | 0.241 | 0.467 |
| 4 | 0.243 | 0.471 | 4 | 0.177 | 0.459 | 4 | 0.265 | 0.475 |
| 5 | 0.243 | 0.471 | 5 | 0.250 | 0.481 | 5 | 0.314 | 0.479 |
| ⋮ | ⋮ | ⋮ | ||||||
| 74 | 0.972 | 0.479 | 95 | 0.976 | 0.489 | 51 | 0.972 | 0.294 |
| 75 | 0.972 | 0.479 | 96 | 0.976 | 0.489 | 52 | 0.983 | 0.376 |
| 76 | 0.981 | 0.499 | 97 | 0.999 | 0.033 | 53 | 0.990 | 0.410 |
| 77 | 0.981 | 0.499 | 98 | 0.999 | 0.033 | 54 | 0.999 | 0.911 |
| 78 | 1.009 | 0.118 | 99 | 1.007 | 0.091 | 55 | 1.001 | 0.410 |
| 79 | 1.009 | 0.117 | 100 | 1.007 | 0.092 | 56 | 1.020 | 0.176 |
| 80 | 1.020 | 0.071 | 101 | 1.007 | 0.092 | 57 | 1.022 | 0.123 |
| 81 | 1.020 | 0.071 | 102 | 1.007 | 0.091 | 58 | 1.032 | 0.160 |
| ⋮ | ⋮ | ⋮ | ||||||
| 196 | 1.560 | 0.001 | 196 | 1.397 | 0.033 | 196 | 1.922 | 0.006 |
| 197 | 1.581 | 0.005 | 197 | 1.397 | 0.003 | 197 | 1.927 | 0.007 |
| 198 | 1.581 | 0.005 | 198 | 1.397 | 0.003 | 198 | 1.927 | 0.010 |
| 199 | 1.586 | 0.025 | 199 | 1.397 | 0.003 | 199 | 1.929 | 0.004 |
| 200 | 1.586 | 0.025 | 200 | 1.397 | 0.003 | 200 | 1.942 | 0.010 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Polo-López, L.; Córcoles, J.; Ruiz-Cruz, J.A.; Montejo-Garai, J.R.; Rebollar, J.M. Numerical Study on the Orthogonality of the Fields Radiated by an Aperture. Mathematics 2023, 11, 1198. https://doi.org/10.3390/math11051198
Polo-López L, Córcoles J, Ruiz-Cruz JA, Montejo-Garai JR, Rebollar JM. Numerical Study on the Orthogonality of the Fields Radiated by an Aperture. Mathematics. 2023; 11(5):1198. https://doi.org/10.3390/math11051198
Chicago/Turabian StylePolo-López, Lucas, Juan Córcoles, Jorge A. Ruiz-Cruz, José R. Montejo-Garai, and Jesús M. Rebollar. 2023. "Numerical Study on the Orthogonality of the Fields Radiated by an Aperture" Mathematics 11, no. 5: 1198. https://doi.org/10.3390/math11051198
APA StylePolo-López, L., Córcoles, J., Ruiz-Cruz, J. A., Montejo-Garai, J. R., & Rebollar, J. M. (2023). Numerical Study on the Orthogonality of the Fields Radiated by an Aperture. Mathematics, 11(5), 1198. https://doi.org/10.3390/math11051198






